Effects of Temperature and Axial Strain on Four-Wave Mixing Parametric Frequencies in Microstructured Optical Fibers Pumped in the Normal Dispersion Regime
Abstract
:1. Introduction
2. Experimental Setup and Characteristics of the MOFs
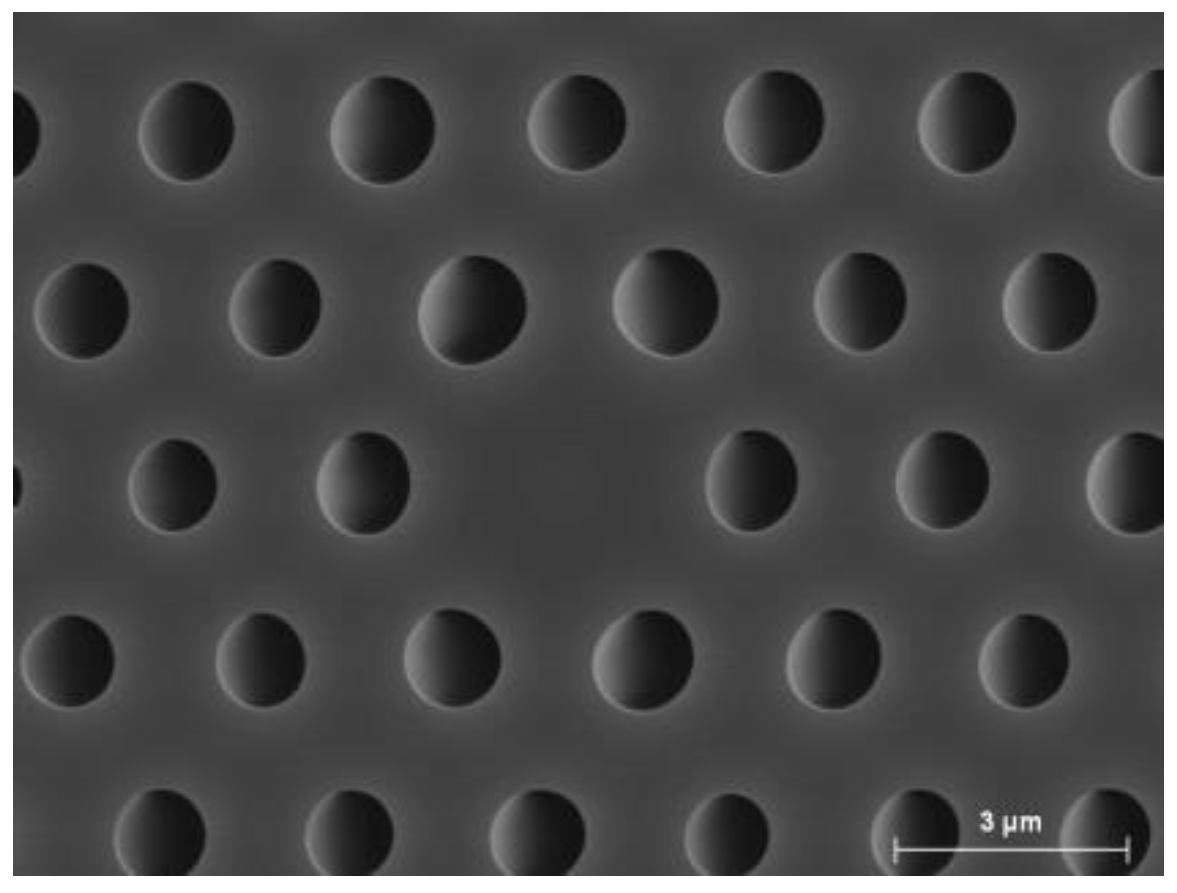
| Λ (μm) | d/Λ (μm) | Dn (μm) | λZ (nm) | |
|---|---|---|---|---|
| Fiber I | 3.0 | 0.56 | 1.3 | 1100 |
| Fiber II | 2.5 | 0.52 | 1.2 | 1080 |
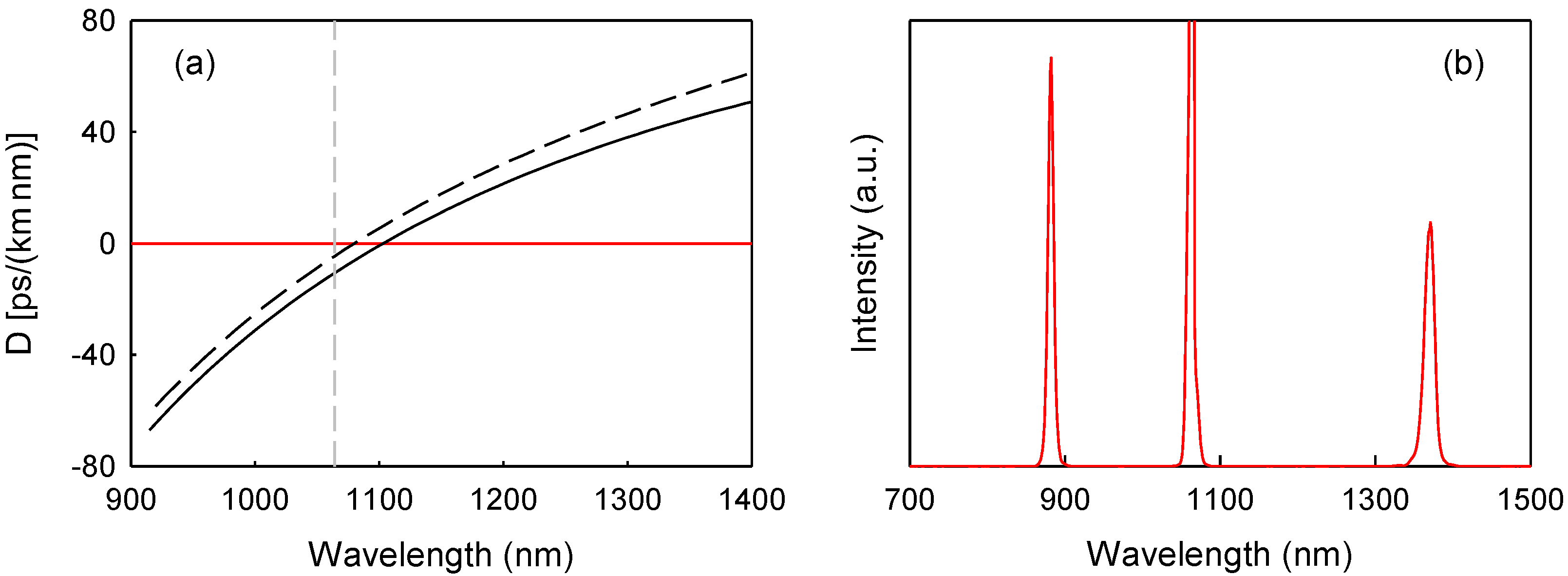
3. Experimental Results
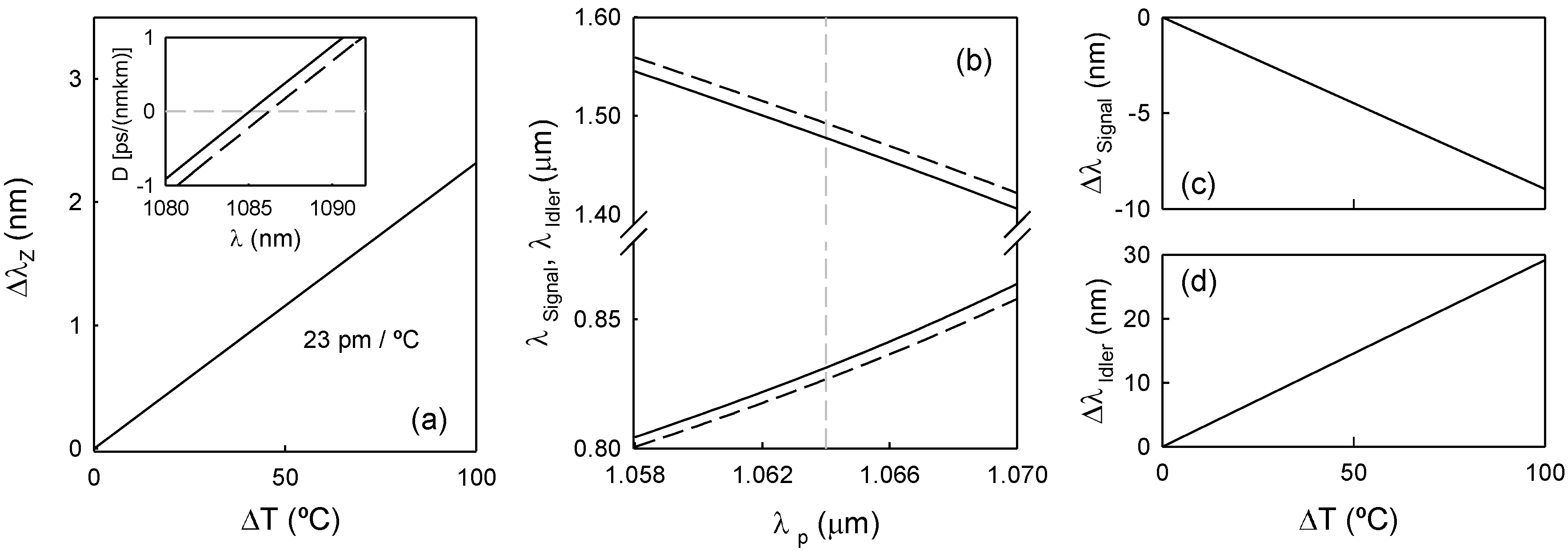
3.1. Parametric Wavelengths vs. Temperature


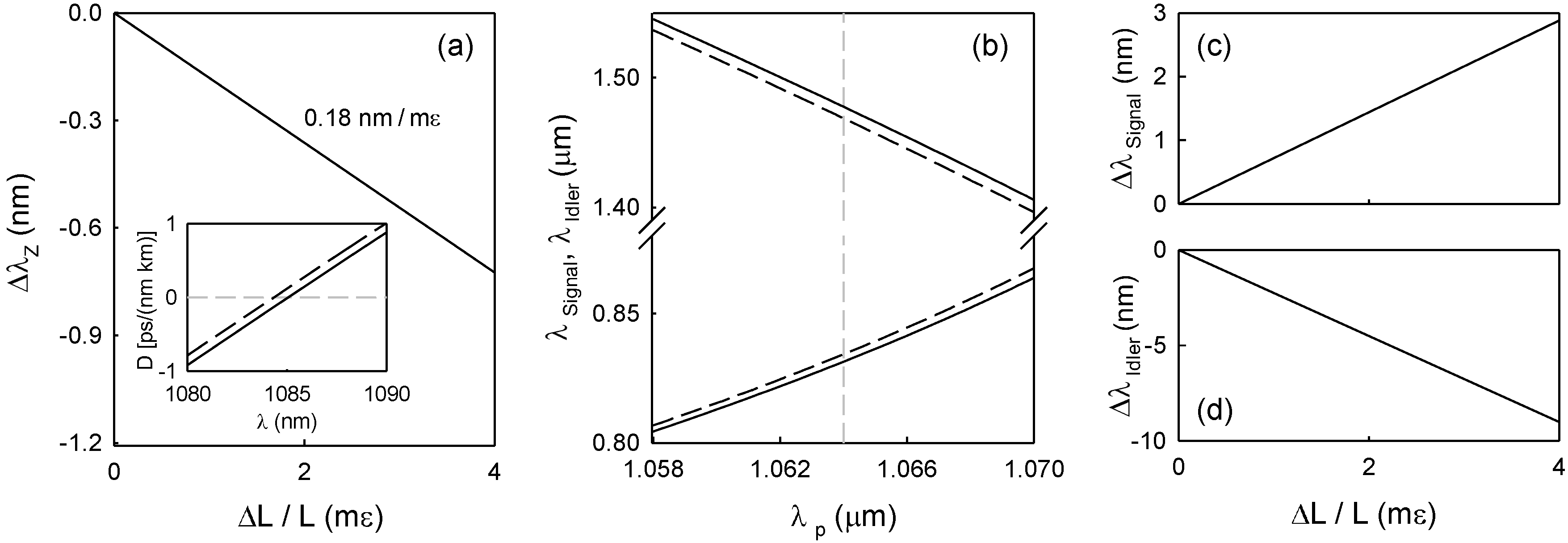
3.2. Parametric Wavelengths vs. Axial Strain
4. Discussion
4.1. The Effect of Temperature
4.2. The Effect of Axial Strain
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Agrawal, G.P. Nonlinear Fiber Optics, 3rd ed.; Academic Press: Boston, MA, USA, 2001. [Google Scholar]
- Serkland, D.K.; Kumar, P. Tunable fiber-optic parametric oscillator. Opt. Lett. 1999, 24, 92–94. [Google Scholar] [CrossRef] [PubMed]
- Sharping, J.E.; Fiorentino, M.; Kumar, P.; Windeler, R.S. Optical parametric oscillator based on four wave mixing in microstructure fiber. Opt. Lett. 2002, 27, 1675–1677. [Google Scholar] [CrossRef] [PubMed]
- Rarity, J.; Fulconis, J.; Duligall, J.; Wadsworth, W.J.; Russell, P.J., St. Photonic crystal fiber source of correlated photon pairs. Opt. Exp. 2005, 13, 534–545. [Google Scholar] [CrossRef]
- Bjarklev, A.; Broeng, J.; Bjarklev, A.S. Photonic Crystal Fibres; Kluwer Academic Publishers: Boston, USA, 2003. [Google Scholar]
- Harvey, J.D.; Leonhardt, R.; Coen, S.; Wong, G.K.L.; Knight, J.C.; Wadsworth, W.J.; Russell, P.J., St. Scalar Modulation instability in the normal dispersion regime by use of a photonic crystal fiber. Opt. Lett. 2003, 28, 2225–2227. [Google Scholar] [CrossRef]
- Wadsworth, W.; Joly, N.; Knight, J.; Birks, T.A.; Biancalana, F.; Russell, P.J., St. Supercontinuum and four-wave mixing with Q-switched pulses in endlessly single-mode photonic crystal fibres. Opt. Exp. 2004, 12, 299–309. [Google Scholar] [CrossRef]
- Hlubina, P.; Szpulak, M.; Ciprian, D.; Martynkien, T.; Urbanczyk, W. Measurement of the group dispersion of the fundamental mode of holey fiber by white-light spectral interferometry. Opt. Exp. 2007, 15, 11073–11081. [Google Scholar] [CrossRef]
- Gu, B.; Wu, Y.; Frosz, M.H.; Zhang, P.; He, S.; Bang, O. Nonlinear fiber-optic strain sensor based on four-wave mixing in microstructured optical fiber. Opt. Lett. 2012, 37, 794–796. [Google Scholar] [CrossRef] [PubMed]
- Saitoh, K.; Koshiba, M. Empirical relations for simple design of photonic crystal fibers. Opt. Exp. 2005, 13, 267–274. [Google Scholar] [CrossRef]
- Ghosh, G. Temperature dispersion of refractive indexes in some silicate fiber glasses. IEEE Pho. Technol. Lett. 1994, 6, 431–433. [Google Scholar] [CrossRef]
- Yariv, A.; Yeh, P. Optical Waves in Crystals; John Wiley & Sons Inc.: New York, NY, USA, 1984. [Google Scholar]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abreu-Afonso, J.; Díez, A.; Cruz, J.L.; Andrés, M.V. Effects of Temperature and Axial Strain on Four-Wave Mixing Parametric Frequencies in Microstructured Optical Fibers Pumped in the Normal Dispersion Regime. Photonics 2014, 1, 404-411. https://doi.org/10.3390/photonics1040404
Abreu-Afonso J, Díez A, Cruz JL, Andrés MV. Effects of Temperature and Axial Strain on Four-Wave Mixing Parametric Frequencies in Microstructured Optical Fibers Pumped in the Normal Dispersion Regime. Photonics. 2014; 1(4):404-411. https://doi.org/10.3390/photonics1040404
Chicago/Turabian StyleAbreu-Afonso, Javier, Antonio Díez, Jose Luis Cruz, and Miguel V. Andrés. 2014. "Effects of Temperature and Axial Strain on Four-Wave Mixing Parametric Frequencies in Microstructured Optical Fibers Pumped in the Normal Dispersion Regime" Photonics 1, no. 4: 404-411. https://doi.org/10.3390/photonics1040404
APA StyleAbreu-Afonso, J., Díez, A., Cruz, J. L., & Andrés, M. V. (2014). Effects of Temperature and Axial Strain on Four-Wave Mixing Parametric Frequencies in Microstructured Optical Fibers Pumped in the Normal Dispersion Regime. Photonics, 1(4), 404-411. https://doi.org/10.3390/photonics1040404





