Effect of Freeze-Drying on Quality and Grinding Process of Food Produce: A Review
Abstract
:1. Introduction
2. Freeze-Drying of Food Produce
2.1. Basis of Freeze-Drying
2.2. Glass Transition during Freeze-Drying
2.3. Selected Changes Associated with Freeze-Drying
2.4. Drying Kinetic
3. Relationship between Drying and Grinding
3.1. Energy Consumption
3.2. Grinding Yield and Morphological Characteristics
4. Limitations in Grinding as a Result of the Drying Process
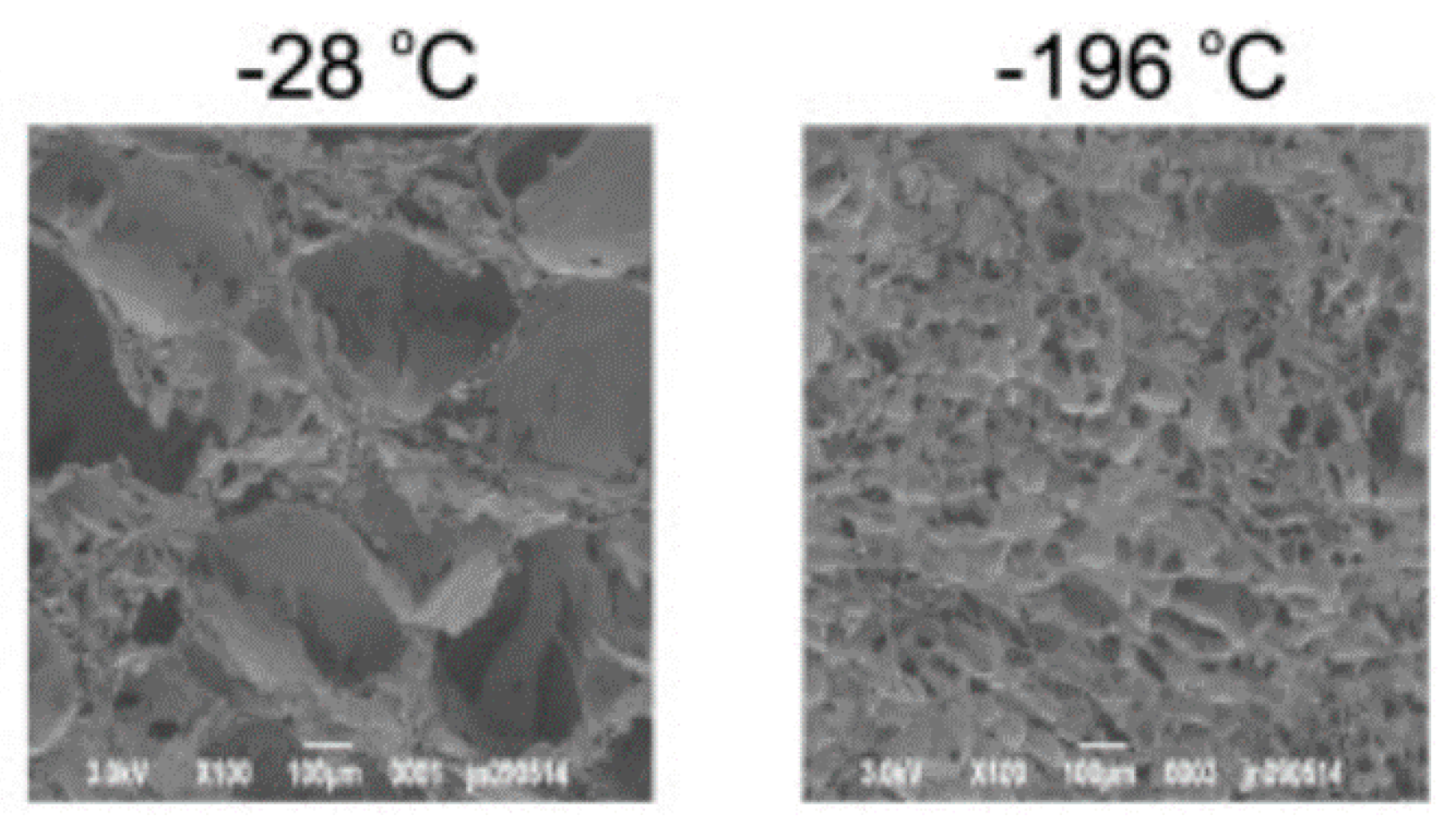
4.1. Impact of Freezing Rate On Crystallization and Microstructure
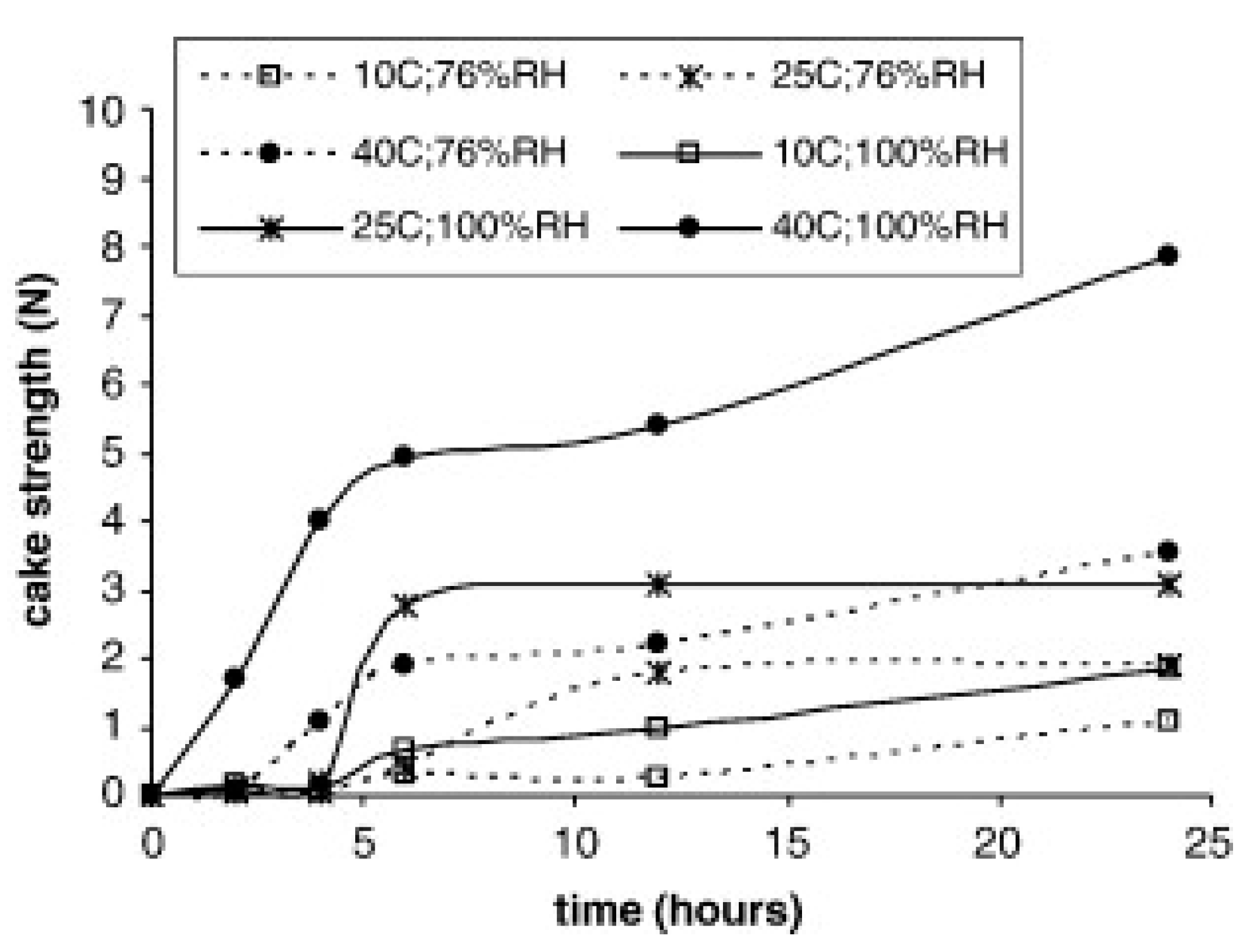
4.2. Caking during Grinding
5. Effect of Grinding Technology on Food Powder Component
6. Innovations in Freeze-Drying Technology towards Achieving Grinding Efficiency
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Afolabi, I.S. Moisture migration and bulk nutrients interaction in a drying food systems: A review. Food Nutr. Sci. 2014, 58, 692–714. [Google Scholar] [CrossRef] [Green Version]
- Dalmau, M.E.; Eim, V.; Rosselló, C.; Cárcel, J.A.; Simal, S. Effects of convective drying and freeze-drying on the release of bioactive compounds from beetroot during in vitro gastric digestion. Food Funct. 2019, 10, 3209–3223. [Google Scholar] [CrossRef] [PubMed]
- Deng, Y.; Luo, Y.; Wang, Y.; Yue, J.; Liu, Z.; Zhong, Y.; Zhao, Y.; Yang, H. Drying-induced protein and microstructure damages of squid fillets affected moisture distribution and rehydration ability during rehydration. J. Food Eng. 2014, 123, 23–31. [Google Scholar] [CrossRef]
- Moreira, R.; Figueiredo, A.; Sereno, A. Shrinkage of apple disks during drying by warm air convection and freeze drying. Dry. Technol. 2000, 18, 279–294. [Google Scholar] [CrossRef]
- Gupta, S.V.; Bhagyashree, N.P. Convective drying of osmo-dehydrated sapota slice. Int. J. Agric. Food Sci. Technol. 2014, 5, 219–226. [Google Scholar]
- Lee, Y.J.; Lee, M.J.; Yoon, W.B. Effect of seed moisture content on the grinding kinetics, yield and quality of soybean oil. J. Food Eng. 2013, 119, 758–764. [Google Scholar] [CrossRef]
- Baudelaire, E.D. Grinding for food powder production. In Handbook of Food Powders; Woodhead Publishing: Sawston, Cambridge, UK, 2013; pp. 132–149. [Google Scholar]
- Wu, X.; Wang, R. Factors impacting ethanol production from grain sorghum in the dry-grind process. Cereal Chem. 2007, 84, 130–136. [Google Scholar] [CrossRef]
- Karam, M.C.; Petit, J.; Zimmer, D.; Djantou, E.B.; Scher, J. Effects of drying and grinding in production of fruit and vegetable powders. Rev. J. Food Eng. 2016, 188, 32–49. [Google Scholar] [CrossRef]
- Rácz, A.; Csőke, B. Application of the product related stress model for product dispersity control in dry stirred media milling. Int. J. Miner. Process. 2016, 157, 28–35. [Google Scholar] [CrossRef]
- Kamdem, A.T.K.; Hardy, J. Influence of various conditions on meat grinding characteristics. J. Food Eng. 1995, 25, 179–196. [Google Scholar] [CrossRef]
- Vadivambal, R.; Jayas, D.S. Changes in quality of microwave-treated agricultural products—A review. Biosyst. Eng. 2007, 98, 1–16. [Google Scholar] [CrossRef]
- Liao, T.W.; Chen, L.J. A neural network approach for grinding processes: Modelling and optimization. Int. J Mach. Tools Manuf. 1994, 34, 919–937. [Google Scholar] [CrossRef]
- Pan, S.Y.; Wang, K.X.; Liu, Q. Study on physical and chemical properties of different sizes rice powder. J. Food Sci. 2004, 5. [Google Scholar]
- Zhang, M.; Tang, J.; Mujumdar, A.S.; Wang, S. Trends in microwave-related drying of fruits and vegetables. Trends Food Sci. Technol. 2006, 17, 524–534. [Google Scholar] [CrossRef]
- Camire, M.E.; Dougherty, M.P.; Briggs, J.L. Functionality of fruit powders in extruded corn breakfast cereals. Food Chem. 2007, 101, 765–770. [Google Scholar] [CrossRef]
- Wang, H.C.; Zhang, M.; Adhikari, B. Drying of shiitake mushroom by combining freeze-drying and mid-infrared radiation. Food Bioprod. Process. 2015, 94, 507–517. [Google Scholar] [CrossRef]
- Shofian, N.M.; Hamid, A.A.; Osman, A.; Saari, N.; Anwar, F.; Pak Dek, M.S.; Hairuddin, M.R. Effect of freeze-drying on the antioxidant compounds and antioxidant activity of selected tropical fruits. Int. J. Mol. Sci. 2011, 12, 4678–4692. [Google Scholar] [CrossRef] [Green Version]
- Voda, A.; Homan, N.; Witek, M.; Duijster, A.; van Dalen, G.; van der Sman, R.; Nijsse, J.; van Vliet, L.; Van As, H.; van Duynhoven, J. The impact of freeze-drying on microstructure and rehydration properties of carrot. Food Res. Int. 2012, 49, 687–693. [Google Scholar] [CrossRef]
- Fan, K.; Zhang, M.; Mujumdar, A.S. Recent developments in high efficient freeze-drying of fruits and vegetables assisted by microwave: A review. Crit. Rev. Food Sci. Nutr. 2019, 59, 1357–1366. [Google Scholar] [CrossRef]
- Bhatta, S.; Stevanovic Janezic, T.; Ratti, C. Freeze-Drying of Plant-Based Foods. Foods 2020, 9, 87. [Google Scholar] [CrossRef] [Green Version]
- Ciurzyńska, A.; Lenart, A. Freeze-drying-application in food processing and biotechnology-a review. Polish J. Food Nutri. Sci. 2011, 61, 165–171. [Google Scholar] [CrossRef] [Green Version]
- Harper, J.C.; Tappel, A.L. Freeze-drying of food products. Adv. Food Res. 1957, 7, 171. [Google Scholar]
- Franks, F. Freeze-drying of bioproducts: Putting principles into practice. Eur. J. Pharm. Biopharm. 1998, 45, 221–229. [Google Scholar] [CrossRef]
- Livesey, R.G.; Rowe, T.W. A discussion of the effect of chamber pressure on heat and mass transfer in freeze-drying. PDA J. Pharm. Sci. Technol. 1987, 41, 169–171. [Google Scholar]
- Zhang, Z.; Liu, X.Y. Control of ice nucleation: Freezing and antifreeze strategies. Chem. Soc.Rev. 2018, 47, 7116–7139. [Google Scholar] [CrossRef]
- Arakawa, T.; Prestrelski, S.J.; Kenney, W.C.; Carpenter, J.F. Factors affecting short-term and long-term stabilities of proteins. Adv. Drug Deliv. Rev. 2001, 46, 307–326. [Google Scholar] [CrossRef]
- Franks, F. Freeze-drying: From empiricism to predictability. The significance of glass transitions. Dev. Biol. Stand. 1992, 74, 9–18. [Google Scholar]
- Franks, F. Freeze-Drying: A Combination of Physics, Chemistry, Engineering and Economics (Papers presented at the 38th Annual Meeting). Jpn. J. Freez. Dry. 1992, 38, 5–16. [Google Scholar]
- Nowak, D.; Piechucka, P.; Witrowa-Rajchert, D.; Wiktor, A. Impact of material structure on the course of freezing and freeze-drying and on the properties of dried substance, as exemplified by celery. J. Food Eng. 2016, 180, 22–28. [Google Scholar] [CrossRef]
- Craig, D.Q.; Royall, P.G.; Kett, V.L.; Hopton, M.L. The relevance of the amorphous state to pharmaceutical dosage forms: Glassy drugs and freeze dried systems. Int. J. Pharm. 1999, 179, 179–207. [Google Scholar] [CrossRef] [Green Version]
- Hatley, R.H. The effective use of differential scanning calorimetry in the optimisation of freeze-drying processes and formulations. Dev. Biol. Stand. 1992, 74, 105–109. [Google Scholar] [PubMed]
- Ratti, C. Freeze drying for food powder production. In Hand Book of Food Powders; Woodhead Publishing: Sawston, UK, 2013; pp. 57–84. [Google Scholar]
- Goula, A.M.; Karapantsios, T.D.; Achilias, D.S.; Adamopoulos, K.G. Water sorption isotherms and glass transition temperature of spray dried tomato pulp. J. Food Eng. 2008, 85, 73–83. [Google Scholar] [CrossRef]
- Roos, Y.H. Glass transition temperature and its relevance in food processing. Annu. Rev. Food Sci. Technol. 2010, 1, 469–496. [Google Scholar] [CrossRef] [PubMed]
- Khalloufi, S.; El-Maslouhi, Y.; Ratti, C. Mathematical model for prediction of glass transition temperature of fruit powders. J. Food Sci. 2000, 65, 842–848. [Google Scholar] [CrossRef]
- Meda, L.; Ratti, C. Rehydration of freeze-dried strawberries at varying temperatures. J. Food Process Eng. 2005, 28, 233–246. [Google Scholar] [CrossRef]
- Tsourouflis, S.; Flink, J.M.; Karel, M. Loss of structure in freeze-dried carbohydrates solutions: Effect of temperature, moisture content and composition. J. Sci. Food Agric. 1976, 27, 509–519. [Google Scholar] [CrossRef]
- Rahman, M.S. Food Properties Handbook; CRC Press: Boca Raton, FL, USA, 2009. [Google Scholar]
- Passot, S.; Fonseca, F.; Alarcon-Lorca, M.; Rolland, D.; Marin, M. Physical characterisation of formulations for the development of two stable freeze-dried proteins during both dried and liquid storage. Eur. J. Pharm. Biopharm. 2005, 60, 335–348. [Google Scholar] [CrossRef]
- Roos, Y.; Laine, J.J. Freeze-drying and its application to some Finnish agricultural products. Agric. Food Sci. 1985, 57, 125–131. [Google Scholar] [CrossRef]
- Adams, G. The principles of freeze-drying. In Cryopreservation and Freeze-Drying Protocols; Humana Press: Totowa, NJ, USA, 2007; pp. 15–38. [Google Scholar]
- Bhatnagar, B.S.; Bogner, R.H.; Pikal, M.J. Protein stability during freezing: Separation of stresses and mechanisms of protein stabilization. Pharm. Dev. Technol. 2007, 12, 505–523. [Google Scholar] [CrossRef]
- Karmas, R.; Pilar Buera, M.; Karel, M. Effect of glass transition on rates of nonenzymic browning in food systems. J. Agric. Food Chem. 1992, 40, 873–879. [Google Scholar] [CrossRef]
- Bhandari, B.R.; Howes, T. Implication of glass transition for the drying and stability of dried foods. J. Food Eng. 1999, 40, 71–79. [Google Scholar] [CrossRef]
- Schenz, T.W. Glass transitions and product stability—An overview. Food Hydrocolloids 1995, 9, 307–315. [Google Scholar] [CrossRef]
- Chuy, L.; Labuza, T.P. Caking and stickiness of dairy-based food powders as related to glass transition. J. Food Sci. 1994, 59, 43–46. [Google Scholar] [CrossRef]
- Palzer, S. The effect of glass transition on the desired and undesired agglomeration of amorphous food powders. Chem. Eng. Sci. 2005, 60, 3959–3968. [Google Scholar] [CrossRef]
- Schmelzer, J.W.; Tropin, T.V. Glass transition, crystallization of glass-forming melts, and entropy. Entropy 2018, 20, 103. [Google Scholar] [CrossRef] [Green Version]
- Roos, Y.H.; Drusch, S. Phase Transitions in Foods; Academic Press: Cambridge, MA, USA, 2015; p. 5. [Google Scholar]
- Pansare, S.K.; Patel, S.M. Practical considerations for determination of glass transition temperature of a maximally freeze concentrated solution. AAPS PharmSciTech 2016, 17, 805–819. [Google Scholar] [CrossRef]
- Moraga, G.; Talens, P.; Moraga, M.J.; Martínez-Navarrete, N. Implication of water activity and glass transition on the mechanical and optical properties of freeze-dried apple and banana slices. J. Food Eng. 2011, 106, 212–219. [Google Scholar] [CrossRef]
- Anglea, S.A.; Karathanos, V.; Karel, M. Low-temperature transitions in fresh and osmotically dehydrated plant materials. Biotechnol. Progress 1993, 9, 204–209. [Google Scholar] [CrossRef]
- Krokida, M.K.; Karathanos, V.T.; Maroulis, Z.B. Effect of freeze-drying conditions on shrinkage and porosity of dehydrated agricultural products. J. Food Eng. 1998, 35, 369–380. [Google Scholar] [CrossRef]
- Caballero-Cerón, C.; Serment-Moreno, V.; Velazquez, G.; Torres, J.A.; Welti-Chanes, J. Hygroscopic properties and glass transition of dehydrated mango, apple and banana. J. Food Sci. Technol. 2018, 55, 540–549. [Google Scholar] [CrossRef]
- Maidannyk, V.A.; Lim, A.S.; Auty, M.A.; Roos, Y.H. Effects of lipids on the water sorption, glass transition and structural strength of carbohydrate-protein systems. Food Res. Int. 2019, 116, 1212–1222. [Google Scholar] [CrossRef] [Green Version]
- Chirife, J.; Buera, M.P. A critical review of some non-equilibrium situations and glass transitions on water activity values of foods in the microbiological growth range. J. Food Eng. 1995, 25, 531–552. [Google Scholar] [CrossRef]
- Khalloufi, S.; Ratti, C. Quality deterioration of freeze-dried foods as explained by their glass transition temperature and internal structure. J. Food Sci. 2003, 68, 892–903. [Google Scholar] [CrossRef]
- López-Malo, A.; Palou, E. Freeze-drying of fruits and vegetables: Process variables, quality, and stability. In Food Drying, Science and Technology: Microbiology, Chemistry, Application; DEStech Publications: Lancaster, PA, USA, 2008; pp. 417–431. [Google Scholar]
- Izli, N.; Polat, A. Freeze and convective drying of quince (Cydonia oblonga Miller.): Effects on drying kinetics and quality attributes. Heat Mass Transf. 2019, 55, 1317–1326. [Google Scholar] [CrossRef]
- Pei, F.; Yang, W.; Shi, Y.; Sun, Y.; Mariga, A.M.; Zhao, L.; Fang, Y.; Ma, N.; An, X.; Hu, Q. Comparison of freeze-drying with three different combinations of drying methods and their influence on colour, texture, microstructure and nutrient retention of button mushroom (Agaricus bisporus) slices. Food Bioprocess Technol. 2014, 7, 702–710. [Google Scholar] [CrossRef]
- Litvin, S.; Mannheim, C.H.; Miltz, J. Dehydration of carrots by a combination of freeze drying, microwave heating and air or vacuum drying. J. Food Eng. 1998, 36, 103–111. [Google Scholar] [CrossRef]
- Apinan, S.; Yujiro, I.; Hidefumi, Y.; Takeshi, F.; Myllärinen, P.; Forssell, P.; Poutanen, K. Visual observation of hydrolyzed potato starch granules by α-amylase with confocal laser scanning microscopy. Starch-Stärke 2007, 59, 543–548. [Google Scholar] [CrossRef]
- European Journal of Pharmaceutics and Biopharmaceutics: Für Pharmazeutische Verfahrenstechnik A, International Association for Pharmaceutical Technology; Wissenschaftliche Verlagsgesellschaft: Stuttgart, Germany, 1991.
- Mirhosseini, H.; Amid, B.T. Effect of different drying techniques on flowability characteristics and chemical properties of natural carbohydrate-protein Gum from durian fruit seed. Chem. Central J. 2013, 7, 1. [Google Scholar] [CrossRef] [Green Version]
- Arumuganathan, T.; Manikantan, M.R.; Indurani, C.; Rai, R.D.; Kamal, S. Texture and quality parameters of oyster mushroom as influenced by drying methods. Int. Agrophys. 2010, 24, 339–342. [Google Scholar]
- Crapiste, G.H.; Whitaker, S.; Rotstein, E. Drying of cellular material—I. A mass transfer theory. Chem. Eng. Sci. 1988, 43, 2919–2928. [Google Scholar] [CrossRef]
- Ratti, C.; Crapiste, G.H. A generalized drying curve for shrinking food materials. In Drying ’92; Elsevier Publisher BV: Amsterdam, The Netherlands, 1992; Volume 1, pp. 864–873. [Google Scholar]
- Ratti, C. Hot air and freeze-drying of high-value foods: A review. J. Food Eng. 2001, 49, 311–319. [Google Scholar] [CrossRef]
- George, J.P.; Datta, A.K. Development and validation of heat and mass transfer models for freeze-drying of vegetable slices. J. Food Eng. 2002, 52, 89–93. [Google Scholar] [CrossRef]
- Krokida, M.; Maroulis, Z. Quality changes during drying of food materials. Dry. Technol. Agric. Food Sci. 2000, 4, 61–68. [Google Scholar]
- Kirleis, A.W.; Stroshine, R.L. Effects of hardness and drying air temperature on breakage susceptibility and dry-milling characteristics of yellow dent corn. Cereal Chem. 1990, 67, 523–528. [Google Scholar]
- Thompson, R.A. Stress Cracks and Breakage in Artificially Dried Corn. No. 631; US Department of Agriculture, Agricultural Marketing Service, Transportation and Facilities Research Division: Washington, DC, USA, 1963.
- Gunasekaran, S.; Paulsen, M.R. Breakage resistance of corn as a function of drying rates. Trans. ASAE 1985, 28, 2071–2076. [Google Scholar] [CrossRef]
- Babić, J.; Cantalejo, M.J.; Arroqui, C. The effects of freeze-drying process parameters on Broiler chicken breast meat. LWT Food Sci. Technol. 2009, 42, 1325–1334. [Google Scholar] [CrossRef]
- Chen, Z.; Schols, H.A.; Voragen, A.G. Starch granule size strongly determines starch noodle processing and noodle quality. J. Food Sci. 2003, 68, 1584–1589. [Google Scholar] [CrossRef]
- Ghorbani, Z.; Masoumi, A.A.; Hemmat, A. Specific energy consumption for reducing the size of alfalfa chops using a hammer mill. Biosyst. Eng. 2010, 105, 34–40. [Google Scholar] [CrossRef]
- Dziki, D. The crushing of wheat kernels and its consequence on the grinding process. Powder Technol. 2008, 185, 181–186. [Google Scholar] [CrossRef]
- Lee, Y.J.; Yoo, J.S.; Yoon, W.B. Grinding characteristics of black soybeans (Glycine max) at varied moisture contents: Particle size, energy consumption, and grinding kinetics. Int. J. Food Eng. 2014, 10, 347–356. [Google Scholar] [CrossRef]
- Moon, J.H.; Yoon, W.B. Effect of moisture content and particle size on grinding kinetics and flowability of balloon flower (Platycodon grandiflorum). Food Sci. Biotechnol. 2018, 27, 641–650. [Google Scholar] [CrossRef] [PubMed]
- Rozalli, N.M.; Chin, N.L.; Yusof, Y.A. Grinding characteristics of Asian originated peanuts (Arachishypogaea L.) and specific energy consumption during ultra-high speed grinding for natural peanut butter production. J. Food Eng. 2015, 152, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Hammami, C.; René, F. Determination of freeze-drying process variables for strawberries. J. Food Eng. 1997, 32, 133–154. [Google Scholar] [CrossRef]
- Zhang, H.; Cooper, A.I. Aligned porous structures by directional freezing. Adv. Mater. 2007, 19, 1529–1533. [Google Scholar] [CrossRef]
- Vafai, K.; Tien, C.L. Boundary and inertia effects on convective mass transfer in porous media. Int. J. Heat Mass Transf. 1982, 25, 1183–1190. [Google Scholar] [CrossRef]
- Deng, Y.; Zhao, Y. Effect of pulsed vacuum and ultrasound osmopretreatments on glass transition temperature, texture, microstructure and calcium penetration of dried apples (Fuji). LWT Food Sci. Technol. 2008, 41, 1575–1585. [Google Scholar] [CrossRef]
- Park, H.W.; Kim, S.T.; Choung, M.G.; Han, W.Y.; Yoon, W.B. Analysis of grinding kinetics and flow behavior of adzuki bean (P haseolus Angularis) flour for hopper design. J. Food Process Eng. 2016, 39, 366–376. [Google Scholar] [CrossRef]
- Kwade, A. Wet comminution in stirred media mills—Research and its practical application. Powder Technol. 1999, 105, 14–20. [Google Scholar] [CrossRef]
- Mucsi, G.; Rácz, A. Grinding kinetics of red grape seed residue in stirred media mill. Adv. Powder Technol. 2017, 28, 2564–2571. [Google Scholar] [CrossRef]
- Bunge, F. Mechanischer ZellaufschluB in Ri. Ihrwerkskugelmi. ihlen. Ph.D. Thesis, TU Braunschweig, Braunschweig, Germany, 1992. [Google Scholar]
- Caparino, O.A.; Tang, J.; Nindo, C.I.; Sablani, S.S.; Powers, J.R.; Fellman, J.K. Effect of drying methods on the physical properties and microstructures of mango (Philippine ‘Carabao’var.) powder. J. Food Eng. 2012, 111, 135–148. [Google Scholar] [CrossRef]
- Larder, C.E.; Abergel, M.; Kubow, S.; Donnelly, D.J. Freeze-drying affects the starch digestibility of cooked potato tubers. Food Res. Int. 2018, 103, 208–214. [Google Scholar] [CrossRef] [PubMed]
- Hsien-Chih, H.W.; Sarko, A. The double-helical molecular structure of crystalline A-amylose. Carbohydr. Res. 1978, 61, 27–40. [Google Scholar] [CrossRef]
- Zhang, B.; Chen, L.; Zhao, Y.; Li, X. Structure and enzymatic resistivity of debranched high temperature–pressure treated high-amylose corn starch. J. Cereal Sci. 2013, 57, 348–355. [Google Scholar] [CrossRef]
- Chi, C.; Li, X.; Zhang, Y.; Miao, S.; Chen, L.; Li, L.; Liang, Y. Understanding the effect of freeze-drying on microstructures of starch hydrogels. Food Hydrocoll. 2020, 101, 105509. [Google Scholar] [CrossRef]
- Busin, L.; Montazavi, F.; Bimbenet, J.J. Étude expérimentale du séchage par atomisation de solutions sucrées. Ind. Alimentaires Agric. 1995, 112, 485–489. [Google Scholar]
- Hartel, R.W.; Shastry, A.V. Sugar crystallization in food products. Crit. Rev. Food Sci. Nutr. 1991, 30, 49–112. [Google Scholar] [CrossRef]
- Maity, T.; Raju, P.S.; Bawa, A.S. Effect of freezing on textural kinetics in snacks during frying. Food Bioprocess Technol. 2012, 5, 155–165. [Google Scholar] [CrossRef]
- Mazur, P. Freezing of living cells: Mechanisms and implications. Am. J. Physiol. Cell Physiol. 1984, 247, C125–C142. [Google Scholar] [CrossRef]
- Silva-Espinoza, M.A.; Ayed, C.; Foster, T.; Camacho, M.D.; Martínez-Navarrete, N. The Impact of Freeze-Drying Conditions on the Physico-Chemical Properties and Bioactive Compounds of a Freeze-Dried Orange Puree. Foods 2020, 9, 32. [Google Scholar] [CrossRef] [Green Version]
- Fitzpatrick, J.J.; Hodnett, M.; Twomey, M.; Cerqueira, P.S.; O’flynn, J.; Roos, Y.H. Glass transition and the flowability and caking of powders containing amorphous lactose. Powder Technol. 2007, 178, 119–128. [Google Scholar] [CrossRef]
- Djantou, E.B.; Mbofung, C.M.; Scher, J.; Desobry, S. A modelling approach to determine the effect of pre-treatment on the grinding ability of dried mangoes for powder production (Mangifera indica var Kent). J. Food Eng. 2007, 80, 668–677. [Google Scholar] [CrossRef]
- Fitzpatrick, J.J. Food Powder Flowability. In Encapsulated and Powdered Foods; CRC Press: Boca Raton, FL, USA, 2005; pp. 259–272. [Google Scholar]
- Ho, T.M.; Truong, T.; Bhandari, B. Spray-drying and non-equilibrium states/glass transition. In Non-Equilibrium States and Glass Transitions in Foods; Woodhead Publishing: Sawston, UK, 2017; pp. 111–136. [Google Scholar]
- Fayed, M.; Otten, L. Handbook of Powder Science & Technology; Springer Science & Business Media: Berlin, Germany, 2013. [Google Scholar]
- Truong, V.; Bhandari, B.R.; Howes, T. Optimization of co-current spray drying process of sugar-rich foods. Part I—Moisture and glass transition temperature profile during drying. J. Food Eng. 2005, 71, 55–65. [Google Scholar] [CrossRef]
- Li, T.; Yang, Y.; Chen, L.; Yang, W. Effects of Grinding Methods on Physicochemical Properties of Crude Polysaccharides from Phellinus baumii. EDP Sciences. InE3S Web Conf. 2019, 78, 2004. [Google Scholar] [CrossRef]
- Dewettinck, K.; Van Bockstaele, F.; Kühne, B.; Van de Walle, D.; Courtens, T.M.; Gellynck, X. Nutritional value of bread: Influence of processing, food interaction and consumer perception. J. Cereal Sci. 2008, 48, 243–257. [Google Scholar] [CrossRef]
- Sopade, P.A. Cereal processing and glycaemic response. Int. J. Food Sci. Technol. 2017, 52, 22–37. [Google Scholar] [CrossRef]
- Violeta, I.; Iuliana, A.; Iuliana, B. The influence of processing on the bioactive compounds of multigrain flours based on wheat, rye and triticale. Ann. Univ. Dunarea Jos Galati. Fascicle VI Food Technol. 2019, 43, 21–32. [Google Scholar]
- Gil, A. Polyunsaturated fatty acids and inflammatory diseases. Biomed. Pharmacother. 2002, 56, 388–396. [Google Scholar] [CrossRef]
- Oarada, M.; Miyazawa, T. The effect of rancid oils on lymphoid tissues. J. Jpn. Oil Chem. Soc. 1990, 39, 373–379. [Google Scholar] [CrossRef] [Green Version]
- Barbosa-Cánovas, G.V.; Ortega-Rivas, E.; Juliano, P.; Yan, H. Food Powders: Physical Properties, Processing, and Functionality; Kluwer Academic/Plenum Publishers: New York, NY, USA, 2005. [Google Scholar]
- Valentina, V.; Pratiwi, R.A.; Hsiao, P.Y.; Tseng, H.T.; Hsieh, J.F.; Chen, C.C. Sensorial Characterization of Foods Before and After Freeze-drying. Sens. Character. Foods Before After Freeze Dry. 2016, 1, 1–5. [Google Scholar]
- Sansiribhan, S.; Devahastin, S.; Soponronnarit, S. Generalized microstructural change and structure-quality indicators of a food product undergoing different drying methods and conditions. J. Food Eng. 2012, 109, 148–154. [Google Scholar] [CrossRef]
- Rahman, M.S. A theoretical model to predict the formation of pores in foods during drying. Int. J. Food Prop. 2003, 6, 61–72. [Google Scholar] [CrossRef]
- Harguindeguy, M.; Fissore, D. On the effects of freeze-drying processes on the nutritional properties of foodstuff: A review. Dry. Technol. 2019, 6, 1–23. [Google Scholar] [CrossRef]
- Fan, J.; Mitchell, J.R.; Blanshard, J.M. The effect of sugars on the extrusion of maize grits: I. The role of the glass transition in determining product density and shape. Int. J. Food Sci. Technol. 1996, 31, 55–65. [Google Scholar] [CrossRef]
- Ozmen, L.; Langrish, T.A. Comparison of glass transition temperature and sticky point temperature for skim milk powder. Dry. Technol. 2002, 20, 1177–1192. [Google Scholar] [CrossRef]
- Ratti, C. Shrinkage during drying of foodstuffs. J. Food Eng. 1994, 23, 91–105. [Google Scholar] [CrossRef]
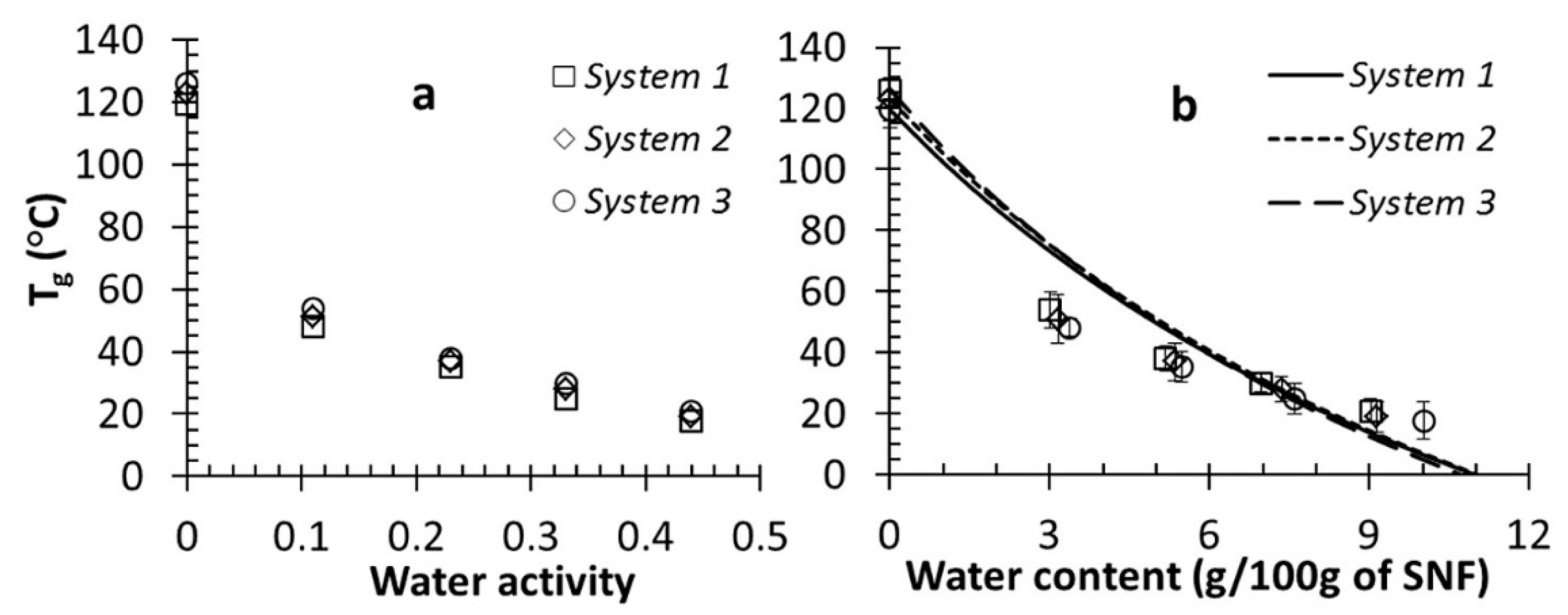
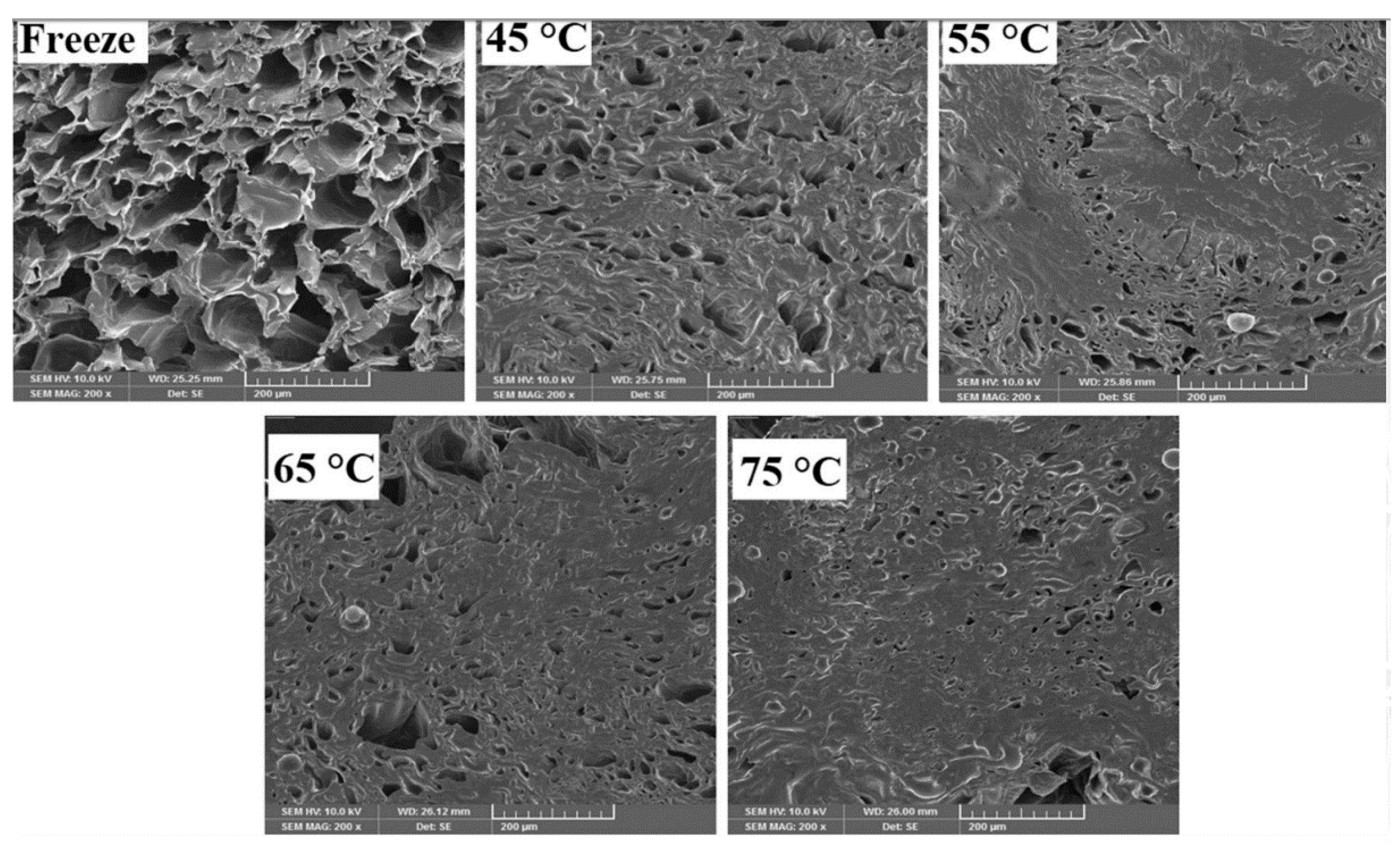
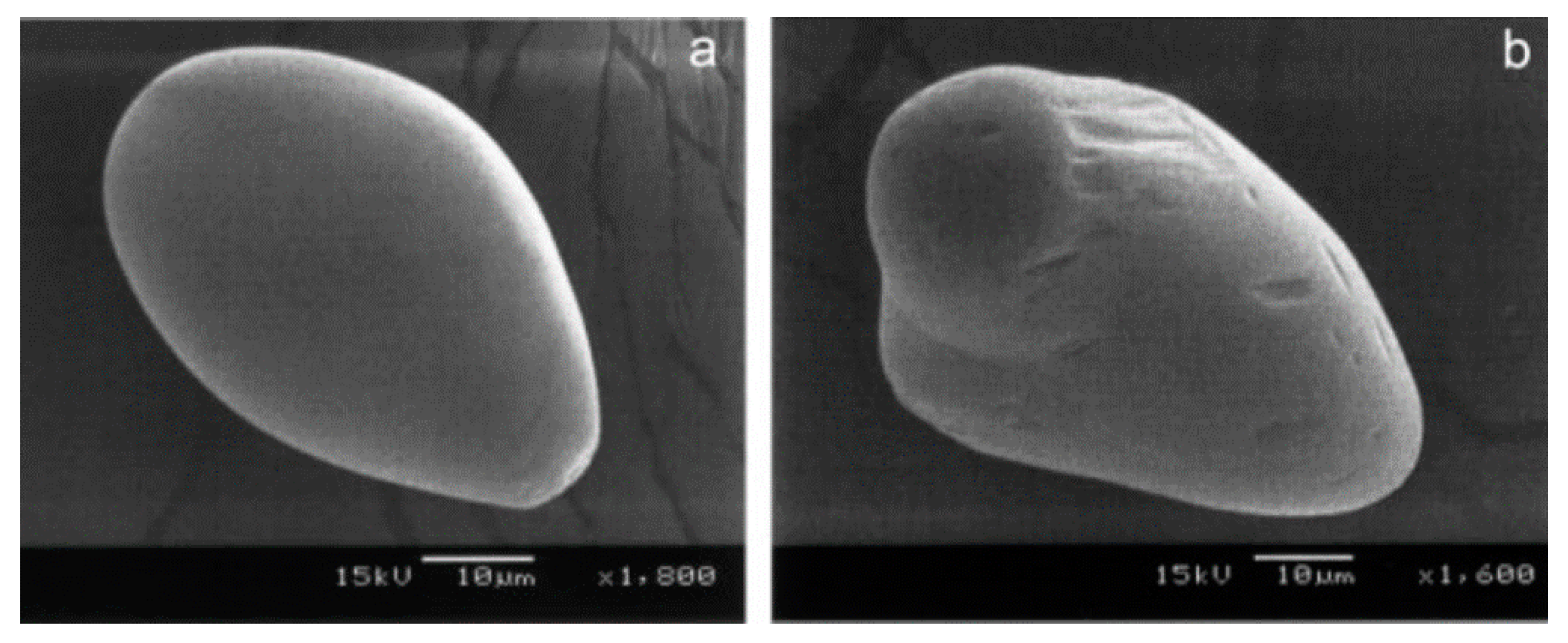
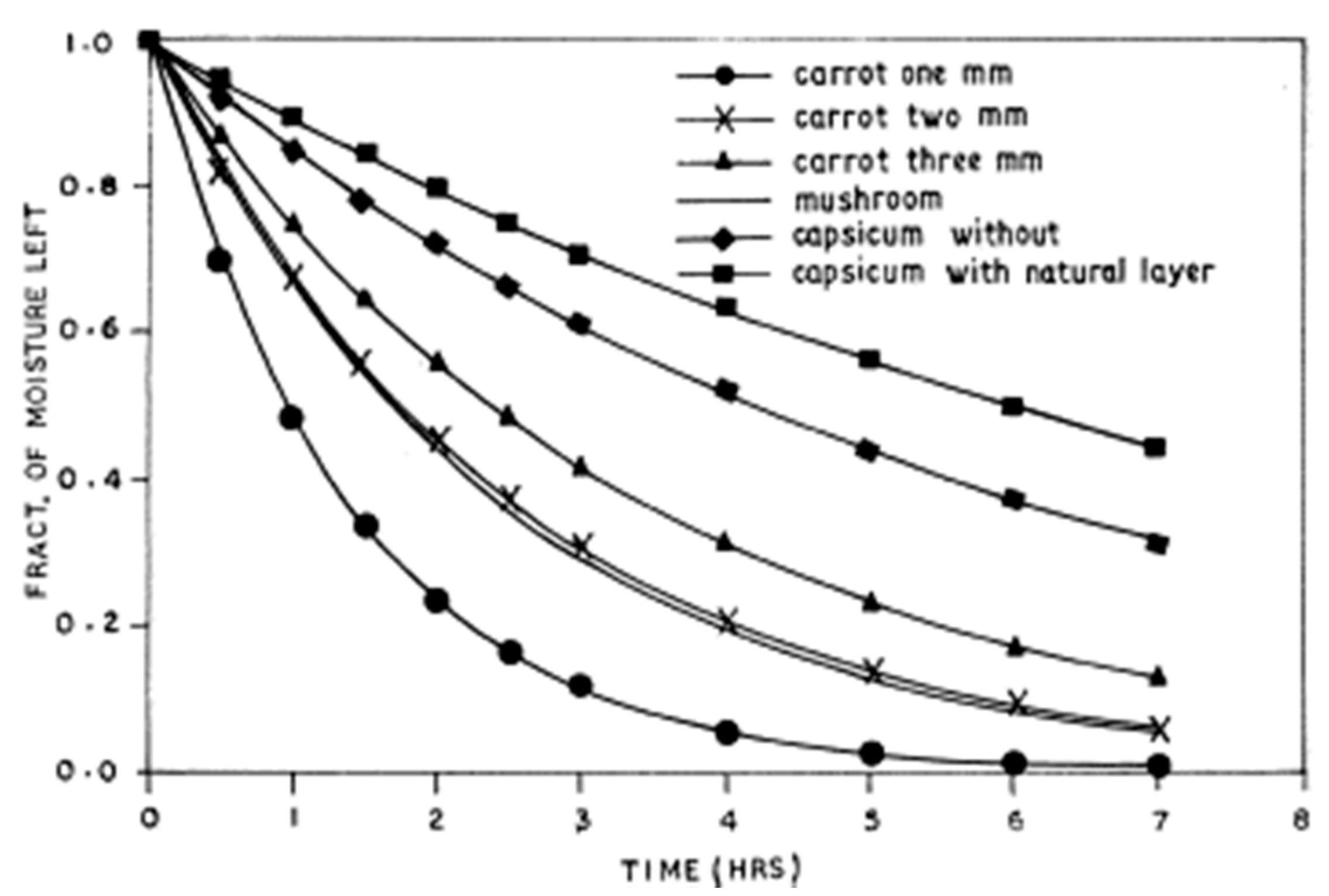
| Food | Collapse Temperature (°C) | Reference |
|---|---|---|
| Coffee extract (25%) | [33] | |
| Apple juice (22%) | [33] | |
| Grape juice (23%) | [33] | |
| Sweetcorn | [33] | |
| Potato | [33] | |
| Tomato | [34] | |
| Glucose | [35] | |
| Blackberry powder | 41 | [36] |
| Strawberry | 45 | [37] |
| Orange juice (14.2% w/v) | 51.6 | [38] |
| Lactose | [39] | |
| Glucose | [39] | |
| Gelatin | [39] | |
| Dextran | [40] | |
| Pineapple Juice (10%) | [40] | |
| Billberry | [41] | |
| Carrot | [41] | |
| Radish | [41] |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Oyinloye, T.M.; Yoon, W.B. Effect of Freeze-Drying on Quality and Grinding Process of Food Produce: A Review. Processes 2020, 8, 354. https://doi.org/10.3390/pr8030354
Oyinloye TM, Yoon WB. Effect of Freeze-Drying on Quality and Grinding Process of Food Produce: A Review. Processes. 2020; 8(3):354. https://doi.org/10.3390/pr8030354
Chicago/Turabian StyleOyinloye, Timilehin Martins, and Won Byong Yoon. 2020. "Effect of Freeze-Drying on Quality and Grinding Process of Food Produce: A Review" Processes 8, no. 3: 354. https://doi.org/10.3390/pr8030354
APA StyleOyinloye, T. M., & Yoon, W. B. (2020). Effect of Freeze-Drying on Quality and Grinding Process of Food Produce: A Review. Processes, 8(3), 354. https://doi.org/10.3390/pr8030354






