A Weak Convergence Self-Adaptive Method for Solving Pseudomonotone Equilibrium Problems in a Real Hilbert Space
Abstract
1. Introduction
2. Preliminaries
- (1)
- strongly monotone on C if
- (2)
- monotone on C if
- (3)
- strongly pseudomonotone on C if
- (4)
- pseudomonotone on C if
- (5)
- L-Lipschitz continuous on C if there exists a constant such that
- (1)
- strongly monotone if
- (2)
- monotone if
- (3)
- strongly pseudomonotone if
- (4)
- pseudomonotone if
- (i)
- with
- (ii)
- (i)
- For each exists;
- (ii)
- All sequentially weak cluster point of lies in C.
3. Main Results
- (A1)
- and also f is pseudomonotone on C;
- (A2)
- f satisfies the Lipschitz-type condition on through positive constants and ;
- (A3)
- for every and satisfying ;
- (A4)
- needs to be convex and subdifferentiable on C for each fixed
| Algorithm 1 Inertial Explicit Extragradient Algorithm for Pseudomonotone EP |
|
- (F1)
- F satisfy the following condition on C for some positive constant
- (F2)
- The solution set and F is pseudomonotone operator on C;
- (F3)
- for every and satisfying
- (i)
- Choose and nondecreasing sequence Set
- (ii)
- Let and are known for Computewhere and
- (iii)
- Moreover, assume thatand revise the stepsize as follows:
4. Numerical Experiments
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Blum, E. From optimization and variational inequalities to equilibrium problems. Math. Student 1994, 63, 123–145. [Google Scholar]
- Muu, L.D.; Oettli, W. Convergence of an adaptive penalty scheme for finding constrained equilibria. Nonlinear Anal. Theory Methods Appl. 1992, 18, 1159–1166. [Google Scholar] [CrossRef]
- Facchinei, F.; Pang, J.S. Finite-Dimensional Variational Inequalities and Complementarity Problems; Springer Science & Business Media: Berlin, Germany, 2007. [Google Scholar]
- Fan, K. A Minimax Inequality and Applications, Inequalities III; Shisha, O., Ed.; Academic Press: New York, NY, USA, 1972. [Google Scholar]
- Hieu, D.V. Projected subgradient algorithms on systems of equilibrium problems. Optim. Lett. 2017, 12, 551–566. [Google Scholar] [CrossRef]
- Scheimberg, S.; Santos, P. A relaxed projection method for finite-dimensional equilibrium problems. Optimization 2011, 60, 1193–1208. [Google Scholar] [CrossRef]
- Muu, L.D.; Quoc, T.D. Regularization Algorithms for Solving Monotone Ky Fan Inequalities with Application to a Nash-Cournot Equilibrium Model. J. Optim. Theory Appl. 2009, 142, 185–204. [Google Scholar] [CrossRef]
- ur Rehman, H.; Kumam, P.; Argyros, I.K.; Deebani, W.; Kumam, W. Inertial Extra-Gradient Method for Solving a Family of Strongly Pseudomonotone Equilibrium Problems in Real Hilbert Spaces with Application in Variational Inequality Problem. Symmetry 2020, 12, 503. [Google Scholar] [CrossRef]
- Moudafi, A. Proximal point algorithm extended to equilibrium problems. J. Nat. Geometry 1999, 15, 91–100. [Google Scholar]
- Flåm, S.D.; Antipin, A.S. Equilibrium programming using proximal-like algorithms. Math. Progr. 1996, 78, 29–41. [Google Scholar] [CrossRef]
- Quoc, T.D.; Anh, P.N.; Muu, L.D. Dual extragradient algorithms extended to equilibrium problems. J. Glob. Optim. 2011, 52, 139–159. [Google Scholar] [CrossRef]
- Lyashko, S.I.; Semenov, V.V. A New Two-Step Proximal Algorithm of Solving the Problem of Equilibrium Programming. In Optimization and Its Applications in Control and Data Sciences; Springer International Publishing: Berlin, Germany, 2016; pp. 315–325. [Google Scholar] [CrossRef]
- ur Rehman, H.; Kumam, P.; Cho, Y.J.; Yordsorn, P. Weak convergence of explicit extragradient algorithms for solving equilibirum problems. J. Inequalities Appl. 2019, 2019. [Google Scholar] [CrossRef]
- Anh, P.N.; Hai, T.N.; Tuan, P.M. On ergodic algorithms for equilibrium problems. J. Glob. Optim. 2015, 64, 179–195. [Google Scholar] [CrossRef]
- ur Rehman, H.; Kumam, P.; Argyros, I.K.; Shutaywi, M.; Shah, Z. Optimization Based Methods for Solving the Equilibrium Problems with Applications in Variational Inequality Problems and Solution of Nash Equilibrium Models. Mathematics 2020, 8, 822. [Google Scholar] [CrossRef]
- Hieu, D.V.; Quy, P.K.; Vy, L.V. Explicit iterative algorithms for solving equilibrium problems. Calcolo 2019, 56. [Google Scholar] [CrossRef]
- Hieu, D.V. New extragradient method for a class of equilibrium problems in Hilbert spaces. Appl. Anal. 2017, 97, 811–824. [Google Scholar] [CrossRef]
- ur Rehman, H.; Kumam, P.; Je Cho, Y.; Suleiman, Y.I.; Kumam, W. Modified Popov’s explicit iterative algorithms for solving pseudomonotone equilibrium problems. Optim. Methods Softw. 2020, 1–32. [Google Scholar] [CrossRef]
- ur Rehman, H.; Kumam, P.; Abubakar, A.B.; Cho, Y.J. The extragradient algorithm with inertial effects extended to equilibrium problems. Comput. Appl. Math. 2020, 39. [Google Scholar] [CrossRef]
- ur Rehman, H.; Kumam, P.; Kumam, W.; Shutaywi, M.; Jirakitpuwapat, W. The Inertial Sub-Gradient Extra-Gradient Method for a Class of Pseudo-Monotone Equilibrium Problems. Symmetry 2020, 12, 463. [Google Scholar] [CrossRef]
- Hieu, D.V. Convergence analysis of a new algorithm for strongly pseudomontone equilibrium problems. Numer. Algorithms 2017, 77, 983–1001. [Google Scholar] [CrossRef]
- Hieu, D.V.; Strodiot, J.J. Strong convergence theorems for equilibrium problems and fixed point problems in Banach spaces. J. Fixed Point Theory Appl. 2018, 20. [Google Scholar] [CrossRef]
- ur Rehman, H.; Kumam, P.; Argyros, I.K.; Alreshidi, N.A.; Kumam, W.; Jirakitpuwapat, W. A Self-Adaptive Extra-Gradient Methods for a Family of Pseudomonotone Equilibrium Programming with Application in Different Classes of Variational Inequality Problems. Symmetry 2020, 12, 523. [Google Scholar] [CrossRef]
- Hieu, D.V.; Gibali, A. Strong convergence of inertial algorithms for solving equilibrium problems. Optim. Lett. 2019. [Google Scholar] [CrossRef]
- Abubakar, J.; Kumam, P.; ur Rehman, H.; Ibrahim, A.H. Inertial Iterative Schemes with Variable Step Sizes for Variational Inequality Problem Involving Pseudomonotone Operator. Mathematics 2020, 8, 609. [Google Scholar] [CrossRef]
- Abubakar, J.; Sombut, K.; ur Rehman, H.; Ibrahim, A.H. An Accelerated Subgradient Extragradient Algorithm for Strongly Pseudomonotone Variational Inequality Problems. Thai J. Math. 2019, 18, 166–187. [Google Scholar]
- ur Rehman, H.; Kumam, P.; Shutaywi, M.; Alreshidi, N.A.; Kumam, W. Inertial Optimization Based Two-Step Methods for Solving Equilibrium Problems with Applications in Variational Inequality Problems and Growth Control Equilibrium Models. Energies 2020, 13, 3292. [Google Scholar] [CrossRef]
- Iusem, A.N.; Sosa, W. On the proximal point method for equilibrium problems in Hilbert spaces. Optimization 2010, 59, 1259–1274. [Google Scholar] [CrossRef]
- Martinet, B. Brève communication. Régularisation d’inéquations variationnelles par approximations successives. Revue Française D’inf. Rech. Opérationnelle. Série Rouge 1970, 4, 154–158. [Google Scholar] [CrossRef]
- Rockafellar, R.T. Monotone operators and the proximal point algorithm. SIAM J. Control Optim. 1976, 14, 877–898. [Google Scholar] [CrossRef]
- Konnov, I. Application of the Proximal Point Method to Nonmonotone Equilibrium Problems. J. Optim. Theory Appl. 2003, 119, 317–333. [Google Scholar] [CrossRef]
- Cohen, G. Auxiliary problem principle and decomposition of optimization problems. J. Optim. Theory Appl. 1980, 32, 277–305. [Google Scholar] [CrossRef]
- Cohen, G. Auxiliary problem principle extended to variational inequalities. J. Optim. Theory Appl. 1988, 59, 325–333. [Google Scholar] [CrossRef]
- Mastroeni, G. On Auxiliary Principle for Equilibrium Problems. In Nonconvex Optimization and Its Applications; Springer: Berlin, Germany, 2003; pp. 289–298. [Google Scholar] [CrossRef]
- Korpelevich, G. The extragradient method for finding saddle points and other problems. Matecon 1976, 12, 747–756. [Google Scholar]
- Quoc Tran, D.; Le Dung, M.N.V.H. Extragradient algorithms extended to equilibrium problems. Optimization 2008, 57, 749–776. [Google Scholar] [CrossRef]
- Censor, Y.; Gibali, A.; Reich, S. The Subgradient Extragradient Method for Solving Variational Inequalities in Hilbert Space. J. Optim. Theory Appl. 2010, 148, 318–335. [Google Scholar] [CrossRef] [PubMed]
- Goebel, K.; Reich, S. Uniform convexity, Hyperbolic Geometry, and Nonexpansive Mappings. 1984. Available online: https://www.researchgate.net/publication/248772020_Uniform_Convexity_Hyperbolic_Geometry_and_Nonexpansive_Mappings (accessed on 10 June 2020).
- Minty, G.J. Monotone (nonlinear) operators in Hilbert space. Duke Math. J. 1962, 29, 341–346. [Google Scholar] [CrossRef]
- Karamardian, S.; Schaible, S. Seven kinds of monotone maps. J. Optim. Theory Appl. 1990, 66, 37–46. [Google Scholar] [CrossRef]
- Bianchi, M.; Schaible, S. Generalized monotone bifunctions and equilibrium problems. J. Optim. Theory Appl. 1996, 90, 31–43. [Google Scholar] [CrossRef]
- Tiel, J.V. Convex Analysis: An Introductory Text, 1st ed.; Wiley: New York, NY, USA, 1984. [Google Scholar]
- Heinz, H.; Bauschke, P.L.C.A. Convex Analysis and Monotone Operator Theory in Hilbert Spaces, 2nd ed.; CMS Books in Mathematics; Springer: Berlin, Germany, 2017. [Google Scholar]
- Attouch, F.A.H. An Inertial Proximal Method for Maximal Monotone Operators via Discretization of a Nonlinear Oscillator with Damping. Set-Valued Var. Anal. 2001, 9, 3–11. [Google Scholar] [CrossRef]
- Opial, Z. Weak convergence of the sequence of successive approximations for nonexpansive mappings. Bull. Amer. Math. Soc. 1967, 73, 591–598. [Google Scholar] [CrossRef]
- Hieu, D.V. Parallel extragradient-proximal methods for split equilibrium problems. Math. Modell. Anal. 2016, 21, 478–501. [Google Scholar] [CrossRef]
- ur Rehman, H.; Pakkaranang, N.; Hussain, A.; Wairojjana, N. A modified extra-gradient method for a family of strongly pseudomonotone equilibrium problems in real Hilbert spaces. J. Math. Comput. Sci. 2020, 22, 38–48. [Google Scholar] [CrossRef]
- Hu, X.; Wang, J. Solving Pseudomonotone Variational Inequalities and Pseudoconvex Optimization Problems Using the Projection Neural Network. IEEE Trans. Neural Netw. 2006, 17, 1487–1499. [Google Scholar] [CrossRef] [PubMed]
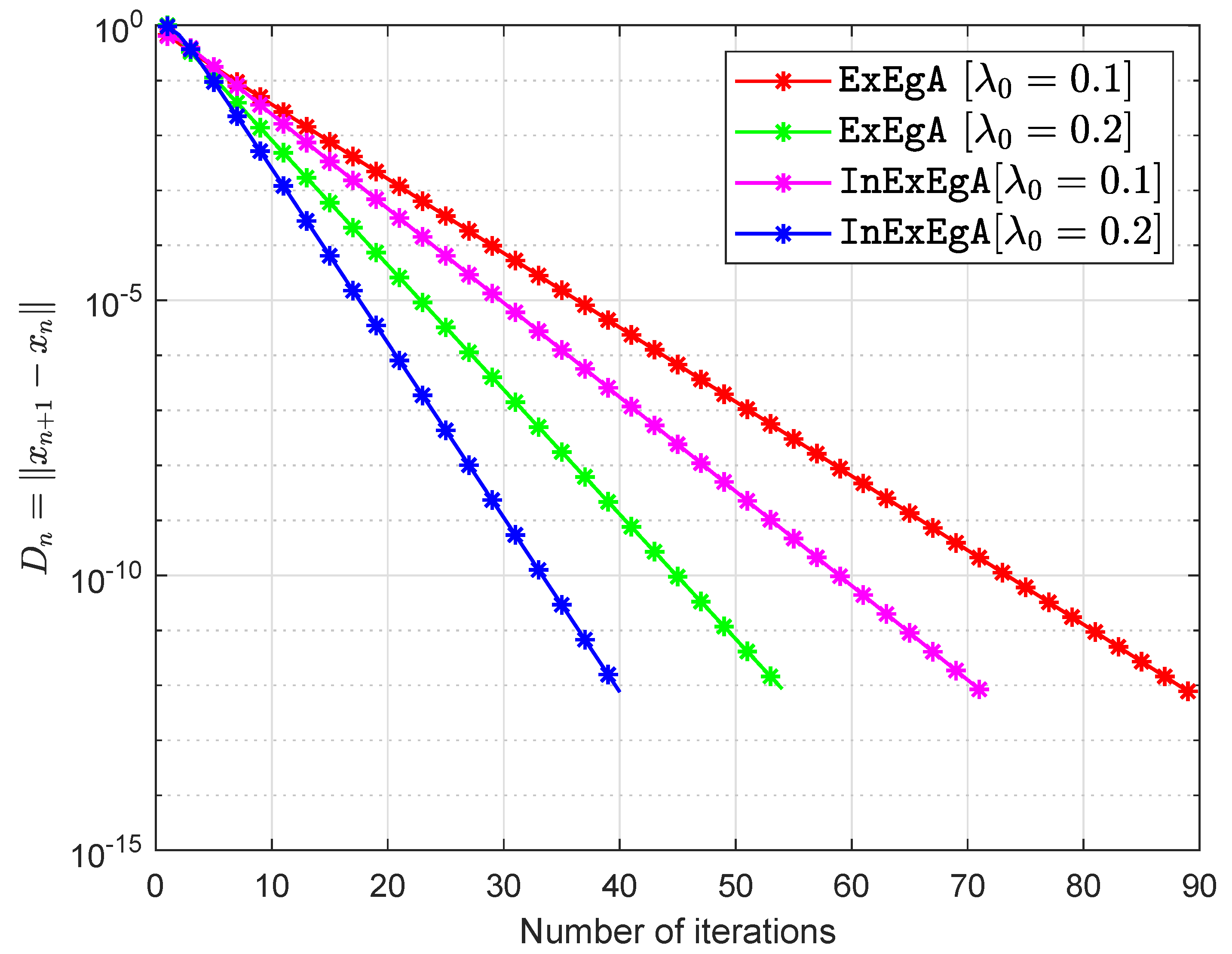
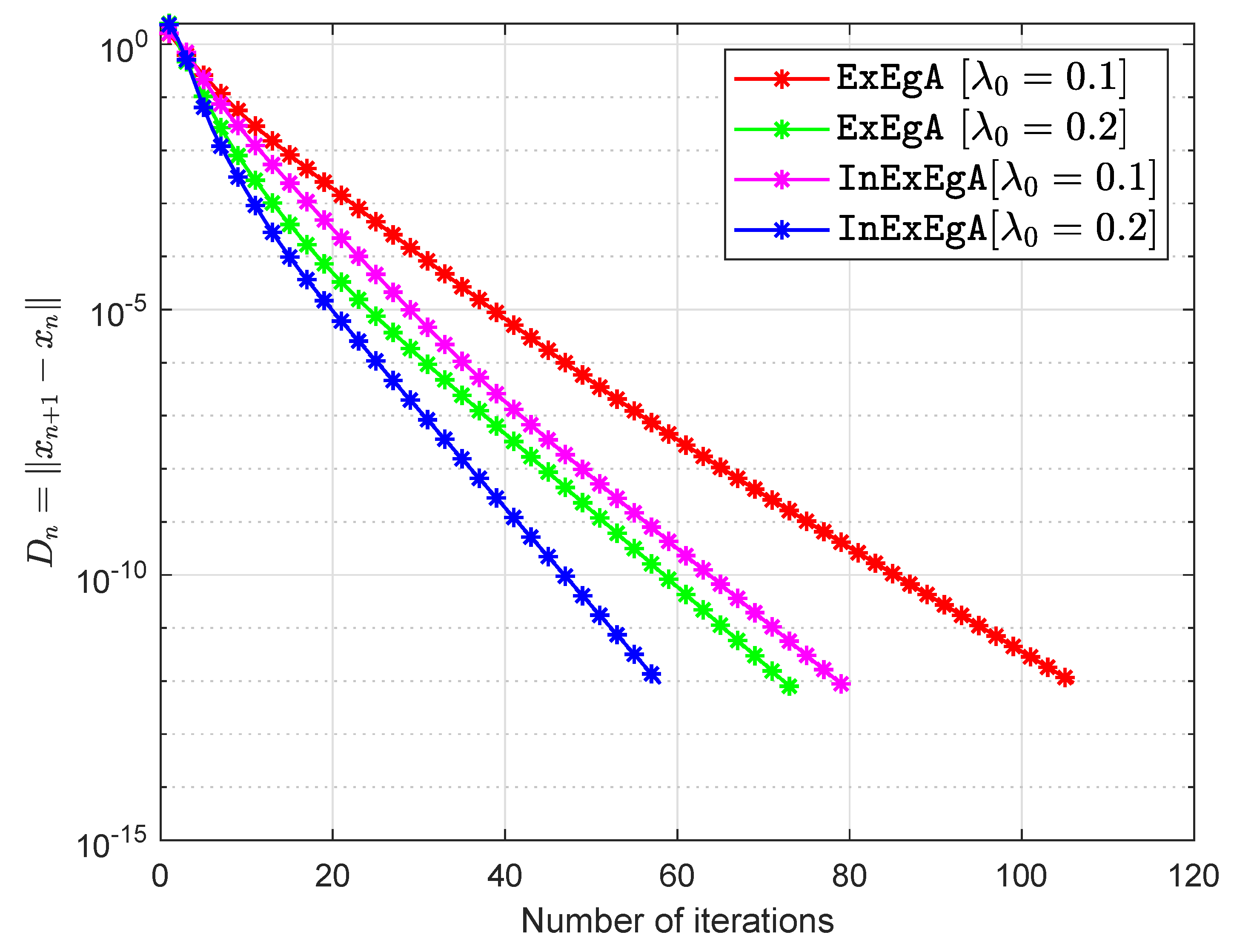
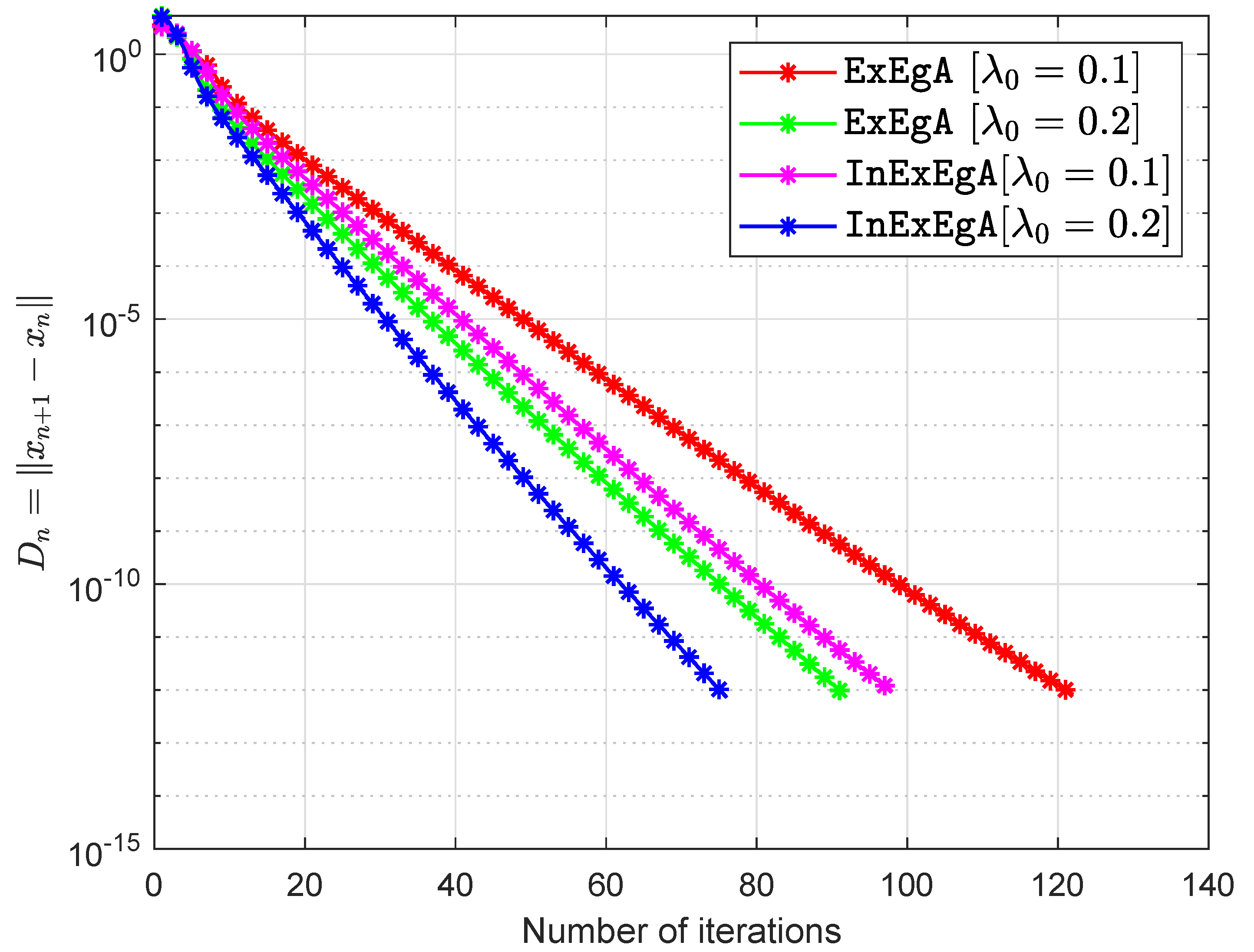
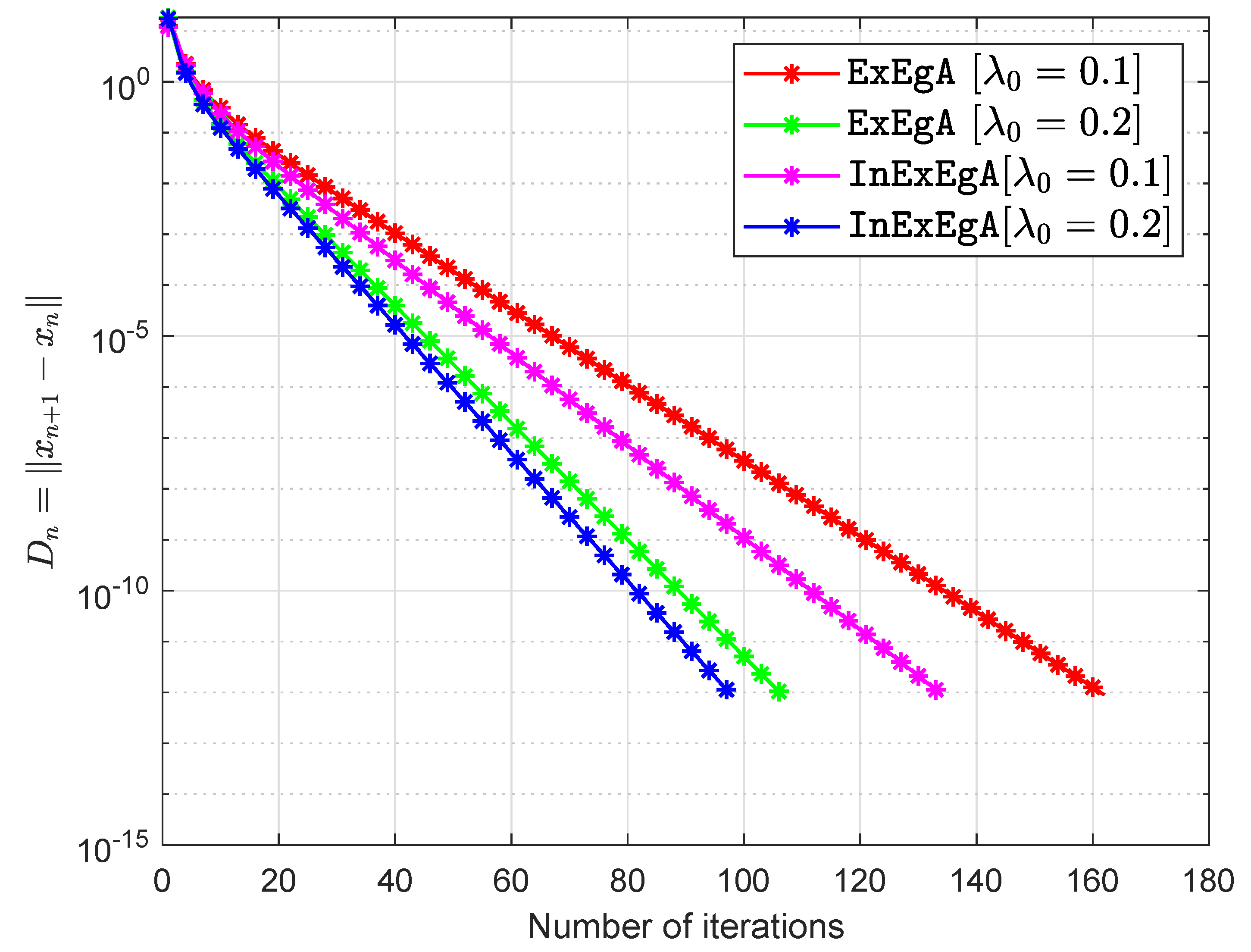

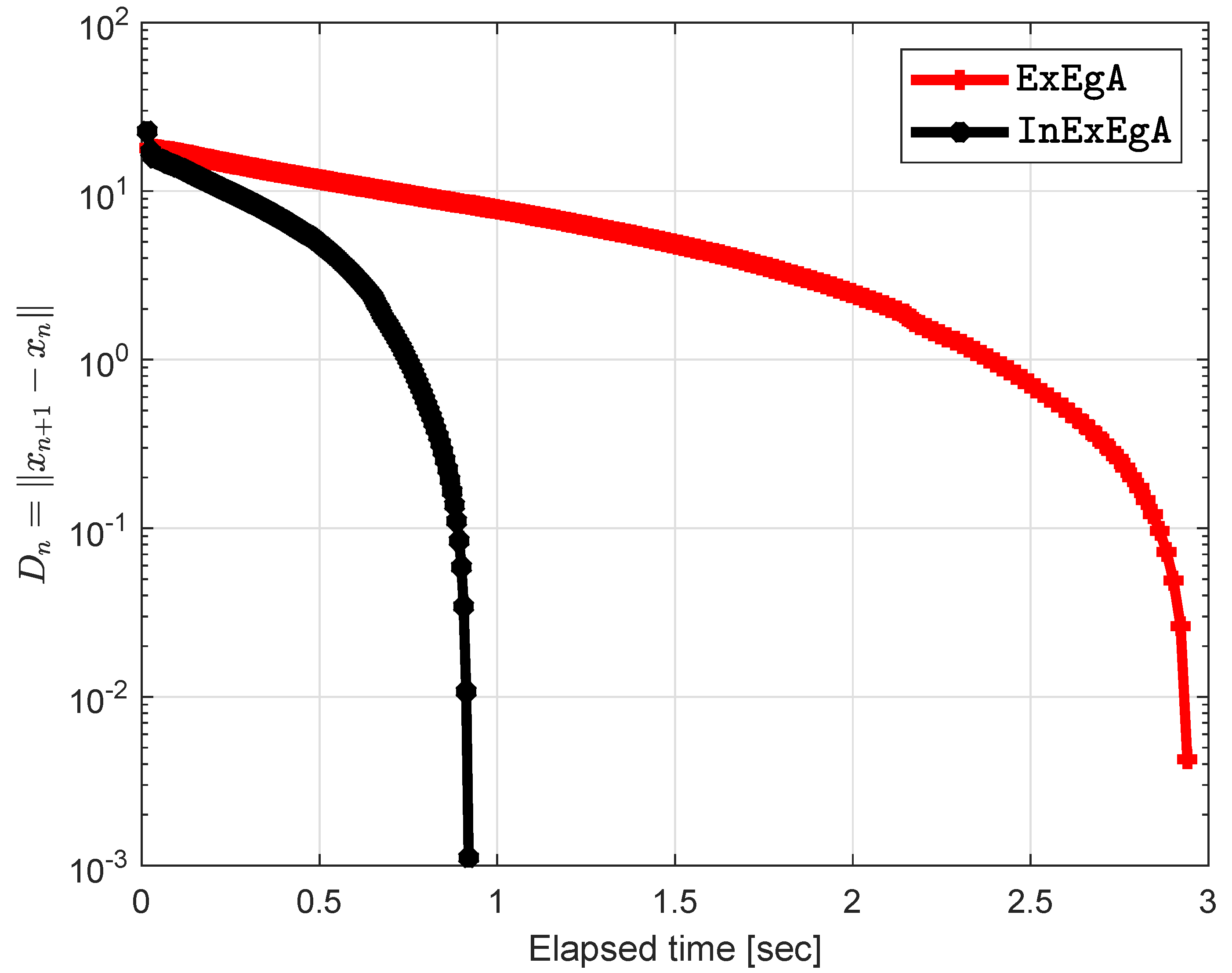


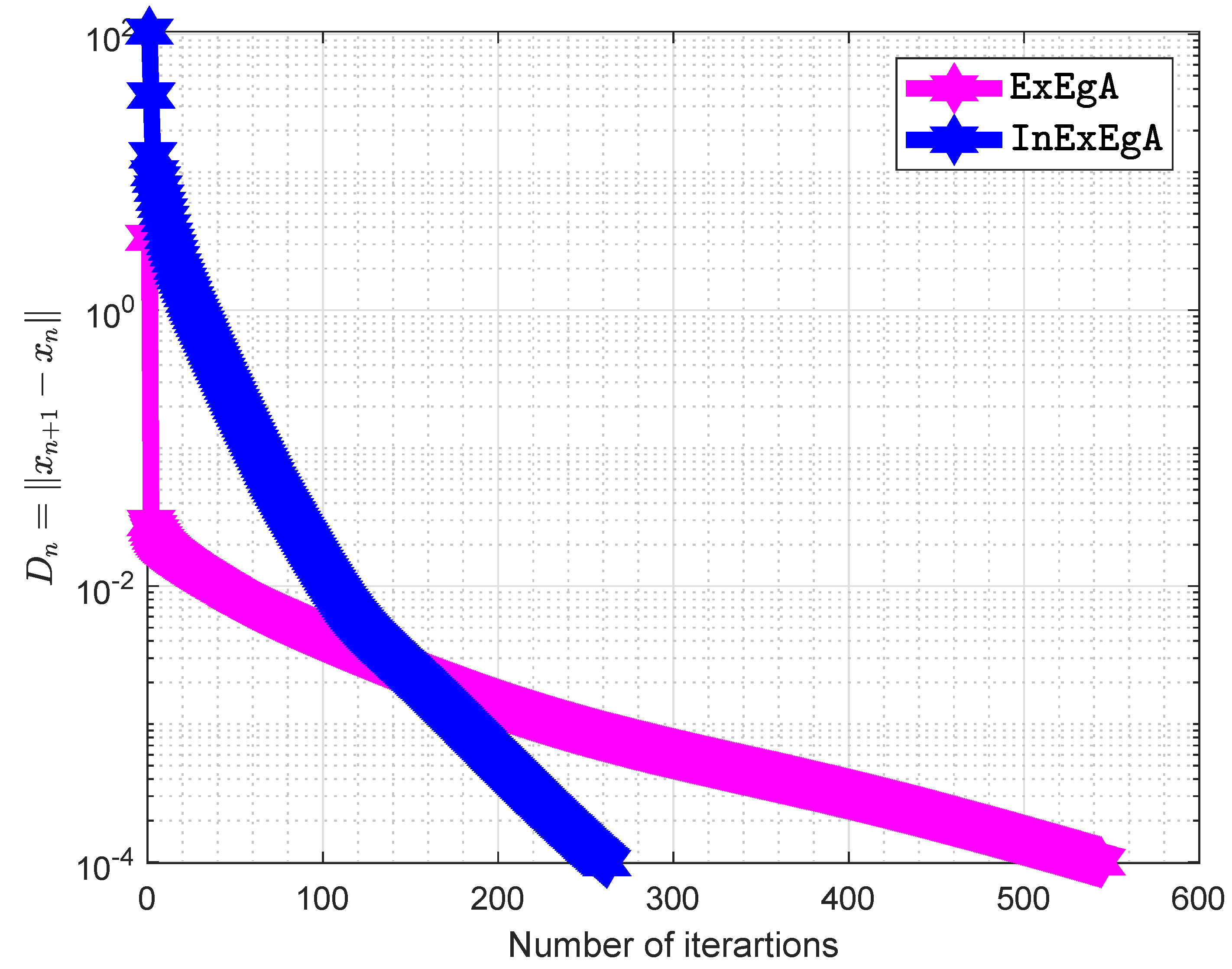
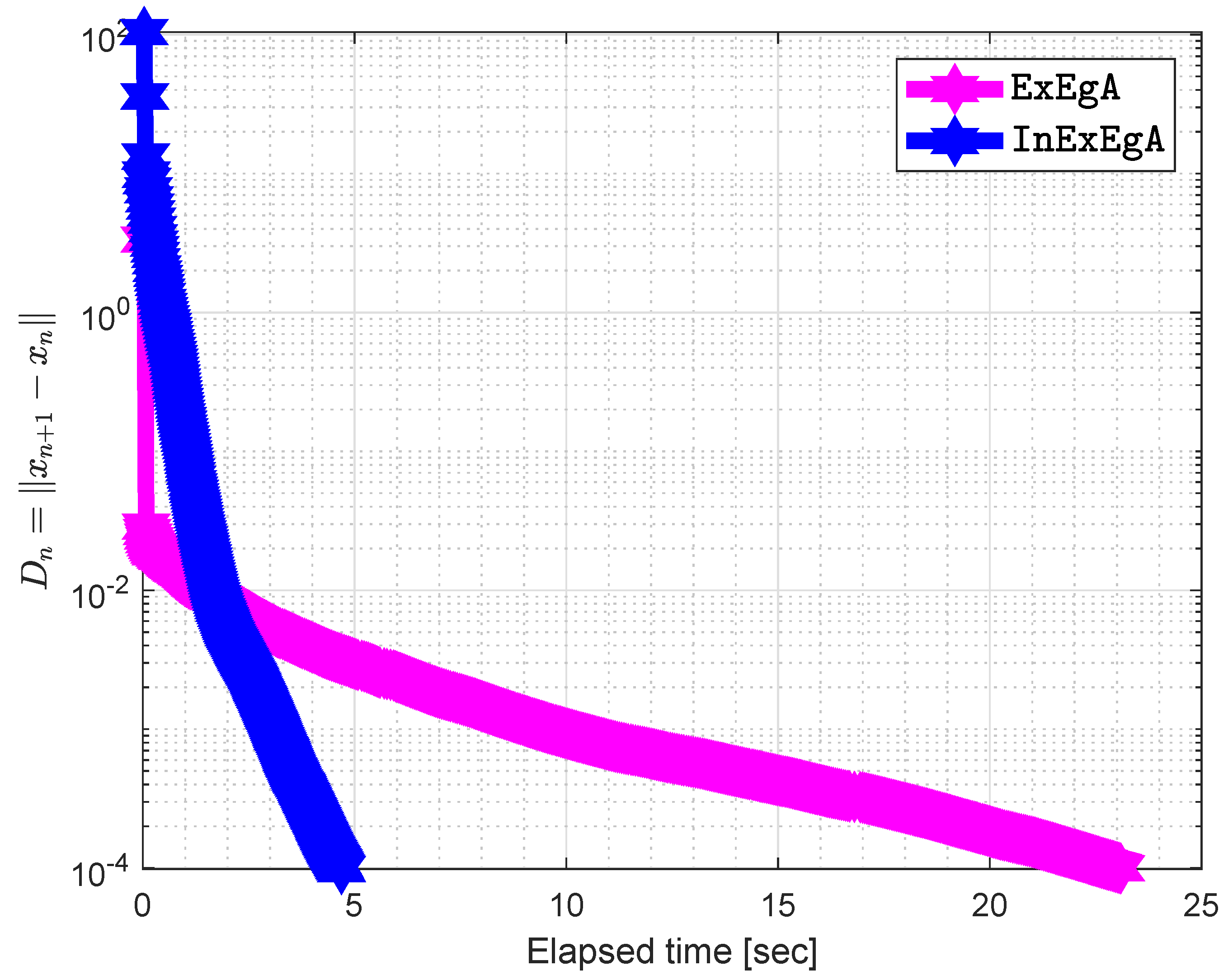




| ExEgA | InExEgA | |||||
|---|---|---|---|---|---|---|
| n | TOL | Iter. | CPU(s) | Iter. | CPU(s) | |
| 0.1 | 89 | 0.8725 | 71 | 0.5018 | ||
| 5 | 0.2 | 54 | 0.4895 | 40 | 0.2080 | |
| 0.1 | 106 | 1.1755 | 79 | 0.6562 | ||
| 10 | 0.2 | 73 | 0.8509 | 58 | 0.4067 | |
| 0.1 | 122 | 1.5985 | 98 | 0.9870 | ||
| 20 | 0.2 | 91 | 1.0032 | 76 | 0.7689 | |
| 0.1 | 162 | 2.4567 | 134 | 1.4567 | ||
| 50 | 0.2 | 107 | 1.4356 | 98 | 1.0067 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yordsorn, P.; Kumam, P.; Rehman, H.u.; Hassan Ibrahim, A. A Weak Convergence Self-Adaptive Method for Solving Pseudomonotone Equilibrium Problems in a Real Hilbert Space. Mathematics 2020, 8, 1165. https://doi.org/10.3390/math8071165
Yordsorn P, Kumam P, Rehman Hu, Hassan Ibrahim A. A Weak Convergence Self-Adaptive Method for Solving Pseudomonotone Equilibrium Problems in a Real Hilbert Space. Mathematics. 2020; 8(7):1165. https://doi.org/10.3390/math8071165
Chicago/Turabian StyleYordsorn, Pasakorn, Poom Kumam, Habib ur Rehman, and Abdulkarim Hassan Ibrahim. 2020. "A Weak Convergence Self-Adaptive Method for Solving Pseudomonotone Equilibrium Problems in a Real Hilbert Space" Mathematics 8, no. 7: 1165. https://doi.org/10.3390/math8071165
APA StyleYordsorn, P., Kumam, P., Rehman, H. u., & Hassan Ibrahim, A. (2020). A Weak Convergence Self-Adaptive Method for Solving Pseudomonotone Equilibrium Problems in a Real Hilbert Space. Mathematics, 8(7), 1165. https://doi.org/10.3390/math8071165







