Contactless Determination of a Permanent Magnet’s Stable Position within Ferrofluid
Abstract
:1. Introduction
2. Materials and Methods
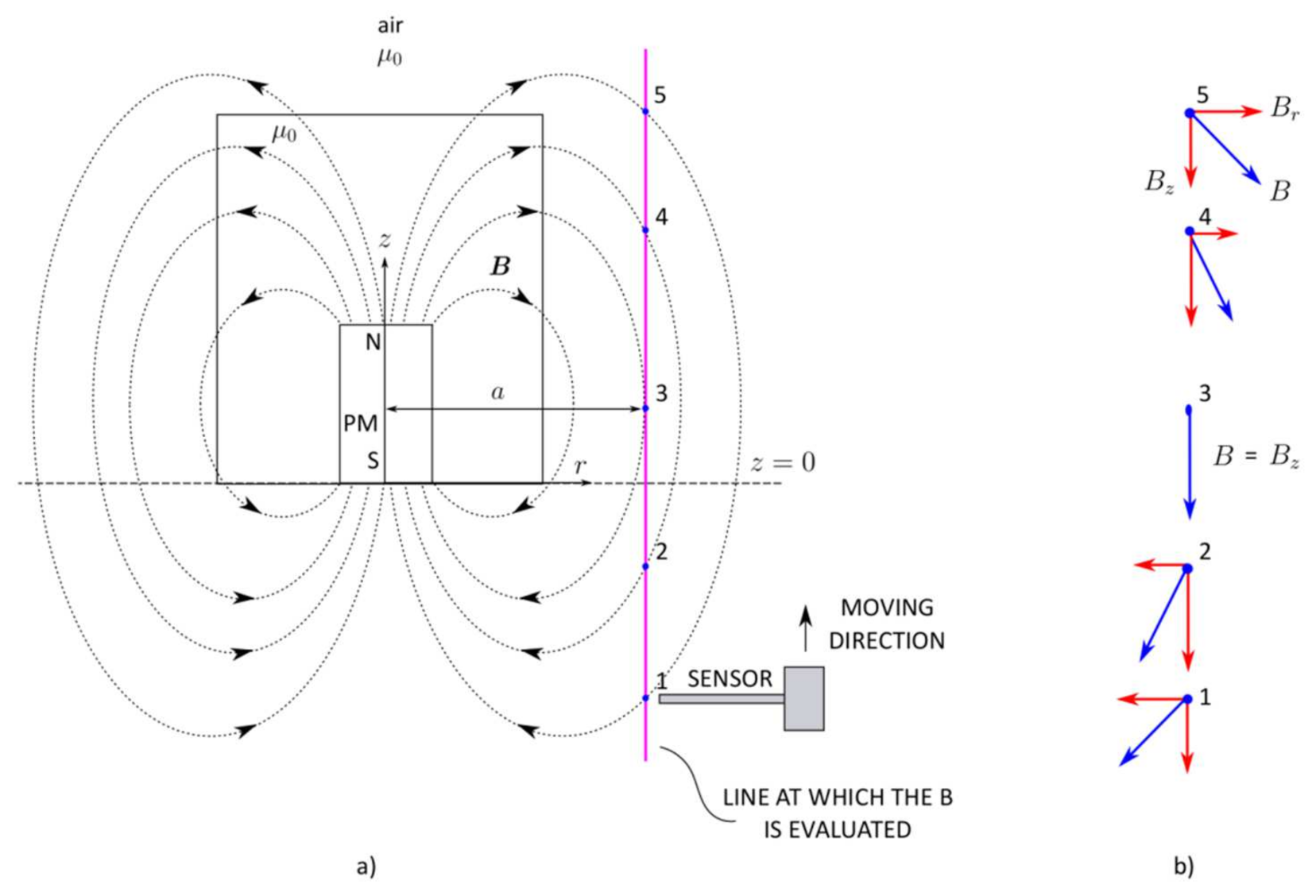
2.1. Theoretical Background
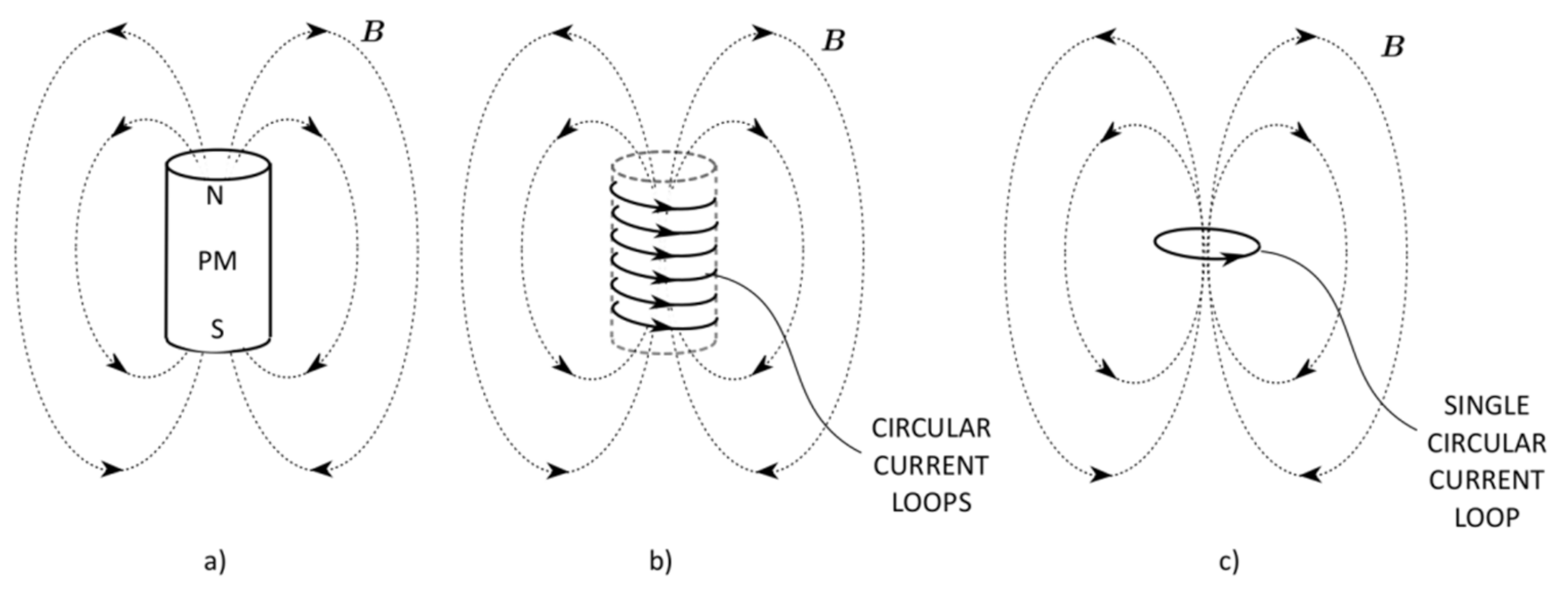
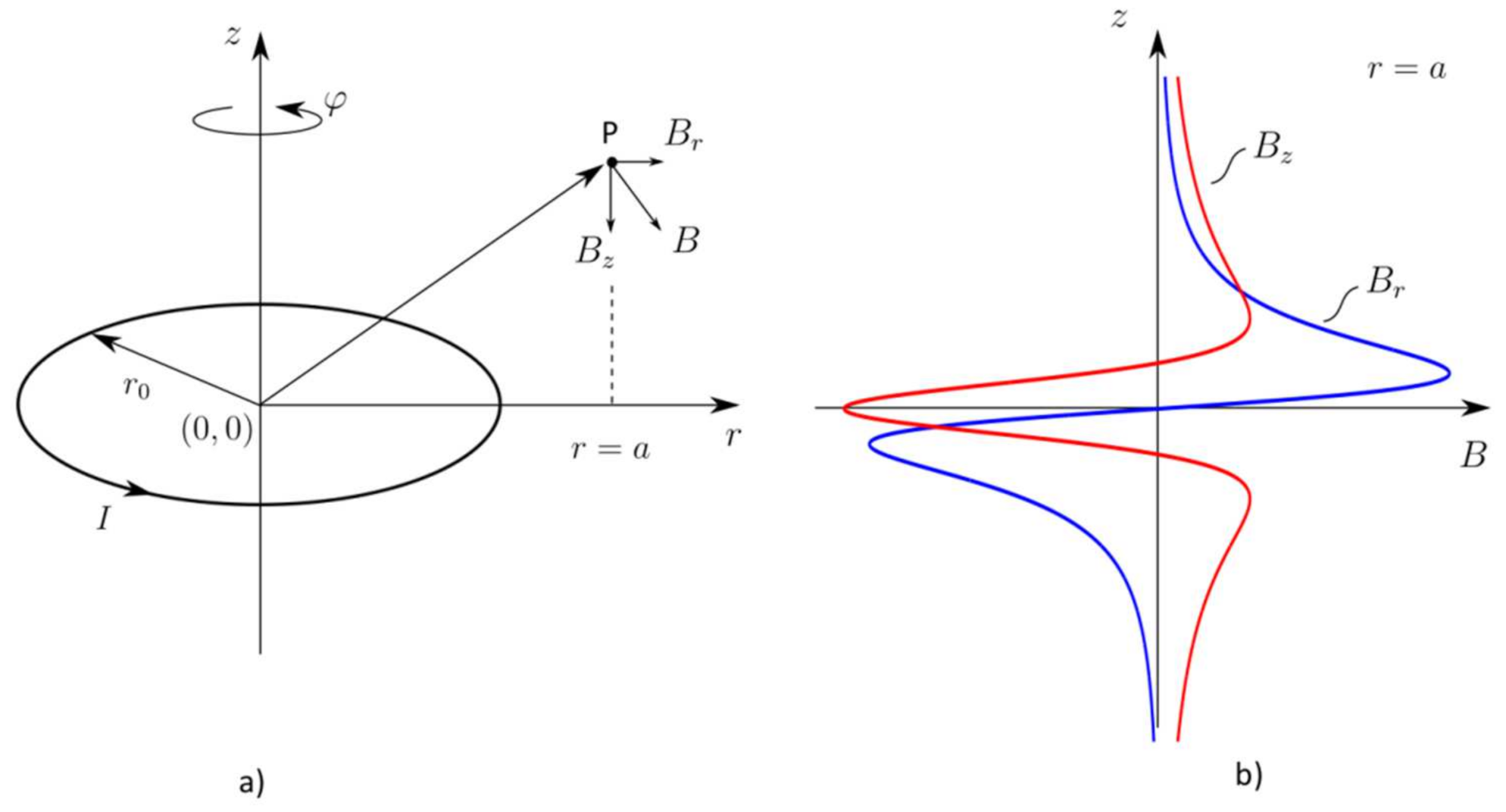
2.1.1. Permanent Magnet in Nonmagnetic Media
2.1.2. Permanent Magnet within Ferrofluid
2.2. FEM Model and Simulation Setup
3. Results and Discussion
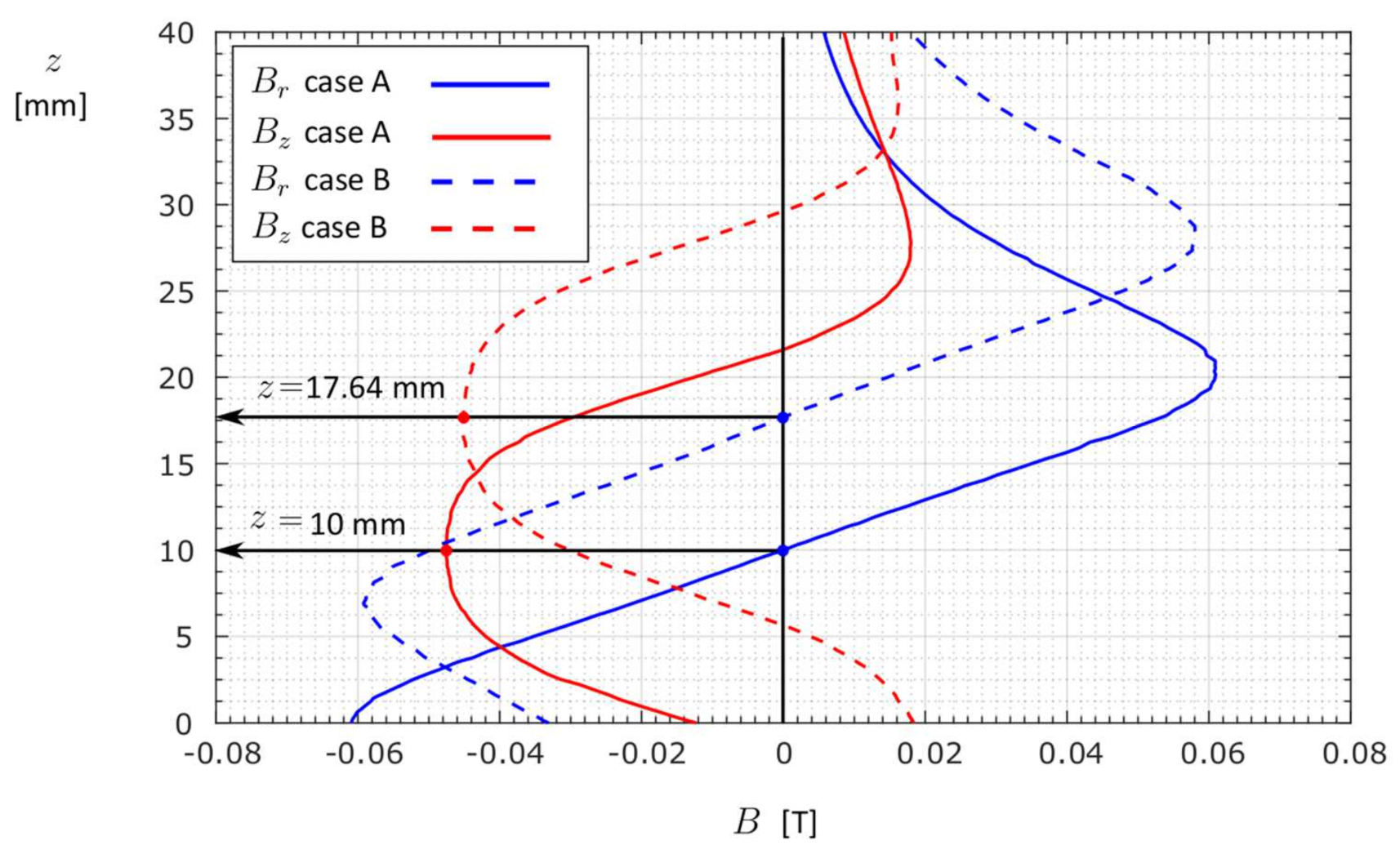
3.1. Position Detection of the PM within an Empty Container—Case A
3.2. Position Detection of the PM within a Ferrofluid-Filled Container—Case B
3.3. Parametric Analysis
3.3.1. Variation in the Ferrofluid’s Relative Permeability—
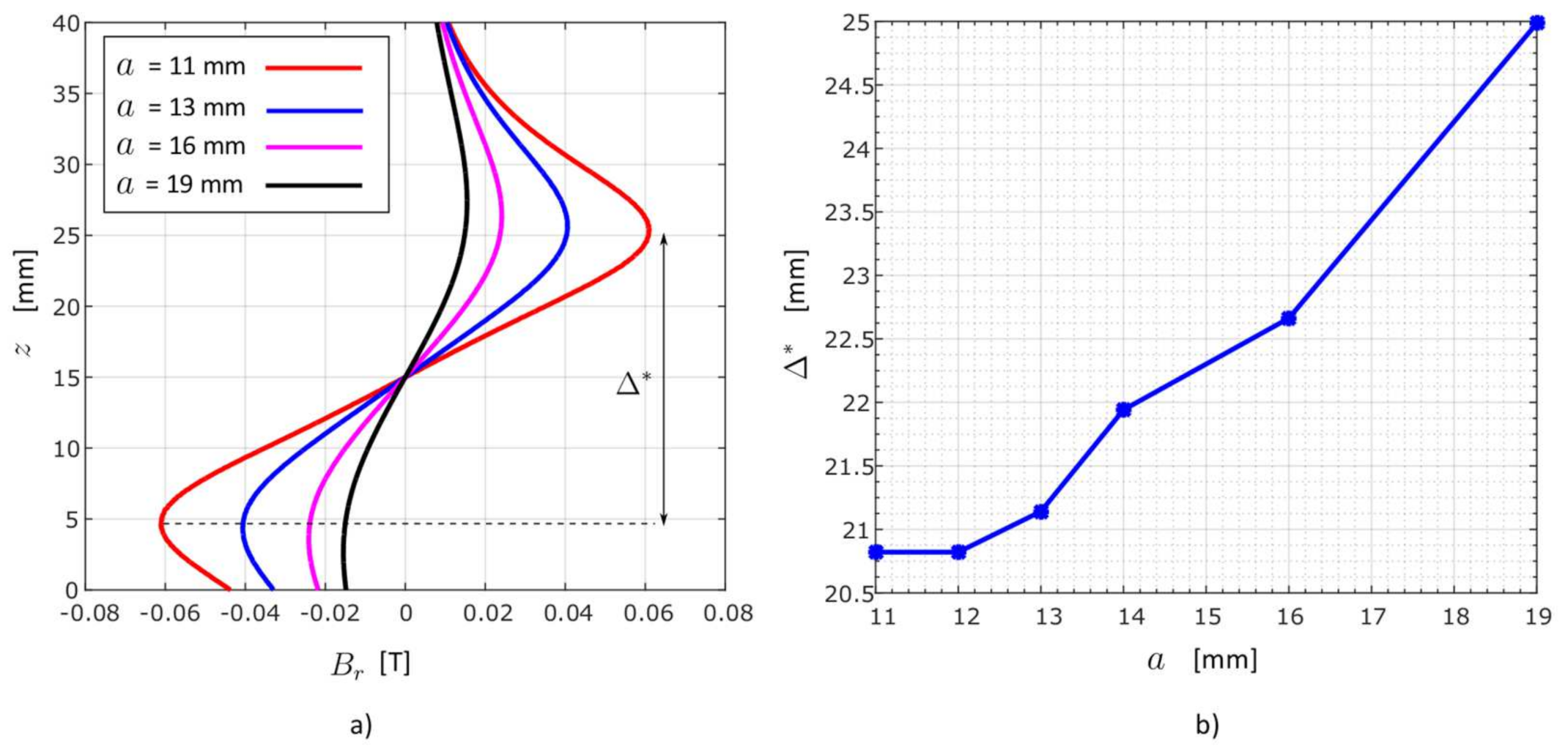
3.3.2. Variation in the Sensor Distance from the PM Center—
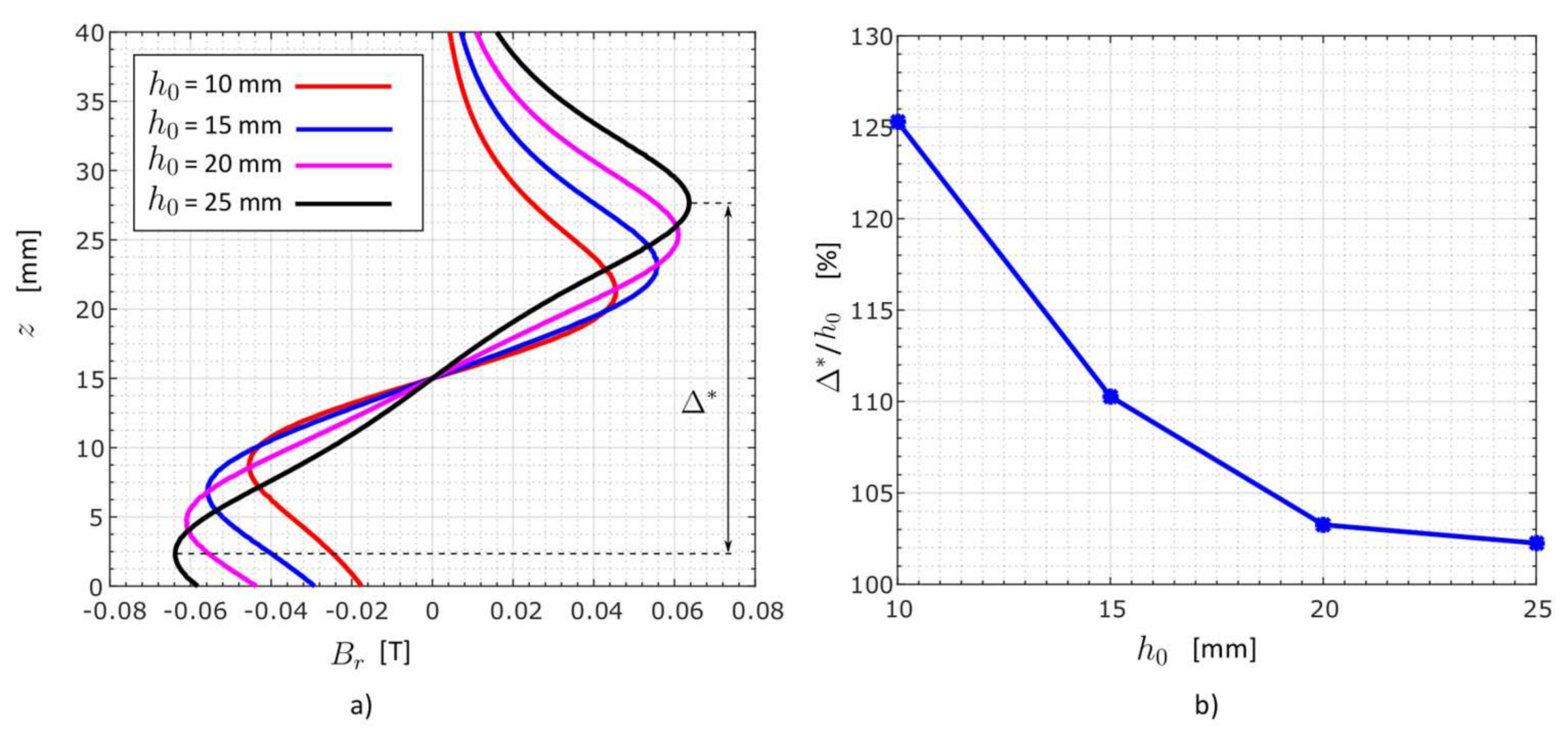
3.3.3. Variation of the PM’s Height—
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Moskowitz, R.; Stahl, P.; Reed, W.R. Inertia Damper Using Ferrofluid. US Patent 4123675, 31 October 1978. [Google Scholar]
- Miller, D.L. Magnetic Viscous Damper. US Patent 4200003, 29 April 1980. [Google Scholar]
- Kogure, T. Damper Device for a Motor. US Patent 5191811, 9 March 1993. [Google Scholar]
- Elsaady, W.; Oyadiji, S.O.; Nasser, A. A one-way coupled numerical magnetic field and CFD simulation of viscoplastic compressible fluids in MR dampers. Int. J. Mech. Sci. 2020, 167, 105265. [Google Scholar] [CrossRef]
- Yoon, D.-S.; Par, Y.-J.; Choi, S.-B. An eddy current effect on the response time of a magnetorheological damper: Analysis and experimental validation. Mech. Syst. Sig. Process. 2019, 127, 136–158. [Google Scholar] [CrossRef]
- Piso, M.I. Applications of magnetic fluids for inertial sensors. J. Magn. Magn. Mater. 1999, 201, 380–384. [Google Scholar] [CrossRef]
- Qian, L.; Li, D.; Yu, J. Study of the Second-Order Levitation Force in the Magnetic Fluid Accelerometer. IEEE Sens. J. 2015, 15, 6805–6810. [Google Scholar] [CrossRef]
- Yu, J.; He, X.; Li, D.; Li, W. Effective and Practical Methods to Calculate the Second-Order Buoyancy in Magnetic Fluid Acceleration Sensor. IEEE Sens. J. 2018, 18, 2278–2284. [Google Scholar] [CrossRef]
- Bashtovoi, V.G.; Kabachikov, D.N.; Kolobov, A.Y.; Samoylov, V.B.; Vikoulenkov, A.V. Research of the dynamics of a magnetic fluid dynamic absorber. J. Magn. Magn. Mater. 2002, 252, 312–314. [Google Scholar] [CrossRef]
- Wang, Z.; Bossis, G.; Volkova, O.; Bashtovoi, K.M. Active Control of Rod Using Magnetic Fluids. J. Intell. Mater. Syst. Struct. 2003, 14, 93–97. [Google Scholar] [CrossRef]
- Yang, W. Magnetic levitation force exerted on the cylindrical magnet in a ferrofluid damper. J. Vib. Control 2015, 23, 2345–2354. [Google Scholar] [CrossRef]
- Yao, J.; Chang, J.; Li, D.; Yang, X. The dynamics analysis of a ferrofluid shock absorber. J. Magn. Magn. Mater. 2016, 402, 28–33. [Google Scholar] [CrossRef]
- Yao, J.; Li, D.; Chen, X.; Huang, C.; Xu, D. Damping performance of a novel ferrofluid dynamic vibration absorber. J. Fluid Struct. 2019, 90, 190–204. [Google Scholar] [CrossRef]
- Li, Y.; Li, D. The dynamics analysis of a magnetic fluid shock absorber with different inner surface materials. J. Magn. Magn. Mater 2022, 542, 168473. [Google Scholar] [CrossRef]
- Volkova, T.I.; Böhm, V.; Naletova, V.A.; Kaufhold, T.; Becker, F.; Zeidis, I.; Zimmermann, K. A ferrofluid based artificial tactile sensor with magnetic field control. J. Magn. Magn. Mater. 2017, 431, 277–280. [Google Scholar] [CrossRef]
- Alberto, N.; Domingues, M.F.; Marques, C.; André, P.; Antunes, P. Optical Fiber Magnetic Field Sensors Based on Magnetic Fluid: A Review. Sensors 2018, 18, 4325. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ruan, Z.; Pei, L.; Ning, T.; Wang, J.; Wang, J.; Li, J.; Xie, Y.; Zhao, Q.; Zheng, J. Simple structure of tapered FBG filled with magnetic fluid to realize magnetic field sensor. Opt. Fiber Technol. 2021, 67, 102698. [Google Scholar] [CrossRef]
- Rosensweig, R.E. Fluidmagnetic Buoyancy. AIAA J. 1966, 4, 1751–1758. [Google Scholar] [CrossRef]
- Rosensweig, R.E. Buoyancy and Stable Levitation of a Magnetic Body immersed in a Magnetizable Fluid. Nature 1966, 210, 613–614. [Google Scholar] [CrossRef]
- Yang, W.; Li, D.; He, X.; Li, Q. Calculation of magnetic levitation force exerted on the cylindrical magnets immersed in ferrofluid. Int. J. Appl. Electromagn. Mech. 2012, 40, 37–49. [Google Scholar] [CrossRef]
- Yang, W.; Wang, P.; Hao, R.; Ma, B. Experimental verification of radial magnetic levitation force on the cylindrical magnets in ferrofluid dampers. J. Magn. Magn. Mater. 2017, 426, 334–339. [Google Scholar] [CrossRef]
- He, X.; Li, D.; Yang, W.; Zhang, H. Experimental Study on the Second- Order Buoyancy of Magnetic Fluid. Key Eng. Mater. 2012, 512–515, 1464–1469. [Google Scholar] [CrossRef]
- Yu, J.; Chen, J.; Li, D. Experimental error analysis of measuring the magnetic self-levitation force experienced by a permanent magnet suspended in magnetic fluid with a nonmagnetic rod. J. Magn. Magn. Mater. 2019, 469, 323–328. [Google Scholar] [CrossRef]
- Yu, J.; Chen, D.; Cai, Z.; Li, D.; Cao, Q.; Qian, L. Research on the magnetic fluid levitation force received by a permanent magnet suspended in magnetic fluid: Consideration a surface instability. J. Magn. Magn. Mater. 2019, 492, 165678. [Google Scholar] [CrossRef]
- Wei, Y.; Zhou, H.; Li, D.; Yao, Y.; Chen, Y. Numerical simulation and experimental study on the ferrofluid second-order buoyancy with a free surface. J. Magn. Magn. Mater. 2022, 553, 169013. [Google Scholar] [CrossRef]
- Trbušić, M.; Jesenik, M.; Trlep, M.; Hamler, A. Energy Based Calculation of the Second-Order Levitation in Magnetic Fluid. Mathematics 2021, 9, 2507. [Google Scholar] [CrossRef]
- Curti, M.; Van Beek, T.A.; Jansen, J.W.; Gysen, B.L.J.; Lomonova, E.A. General Formulation of the Magnetostatic Field and Temperature Distribution in Electrical Machines Using Spectral Element Analysis. IEEE Trans. Magn. 2018, 54, 54–8100809. [Google Scholar] [CrossRef]
- Mahariq, I.; Erciyas, A. A spectral element method for the solution of magnetostatic fields. Turk. J. Electr. Eng. Comput. Sci. 2017, 25, 31. Available online: https://journals.tubitak.gov.tr/elektrik/vol25/iss4/31 (accessed on 5 July 2022). [CrossRef] [Green Version]
- Koch, S.; De Gersem, H.; Weiland, T. Magnetostatic Formulation With Hybrid Finite-Element, Spectral-Element Discretizations. IEEE Trans. Magn. 2009, 45, 1136–1139. [Google Scholar] [CrossRef]
- Mahariq, I.; Abdeljawad, T.; Karar, A.S.; Alboon, S.A.; Kurt, H.; Maslov, A.V. Photonic Nanojets and Whispering Gallery Modes in Smooth and Corrugated Micro-Cylinders under Point-Source Illumination. Photonics 2020, 7, 50. [Google Scholar] [CrossRef]
- Kokelj, P. Electromagnetic Structures, 3rd ed.; Založba FE in FRI: Ljubljana, Slovenia, 2003; pp. 147–149. [Google Scholar]
- Rosensweig, R.E. Ferrohydrodynamics, 1st ed.; Dover Publications, Inc.: New York, NY, USA, 2014; pp. 150–151. [Google Scholar]
- Meeker, D. Finite Element Method Magnetics—User’s Manual. 2007. Available online: www.femm.com (accessed on 31 May 2022).

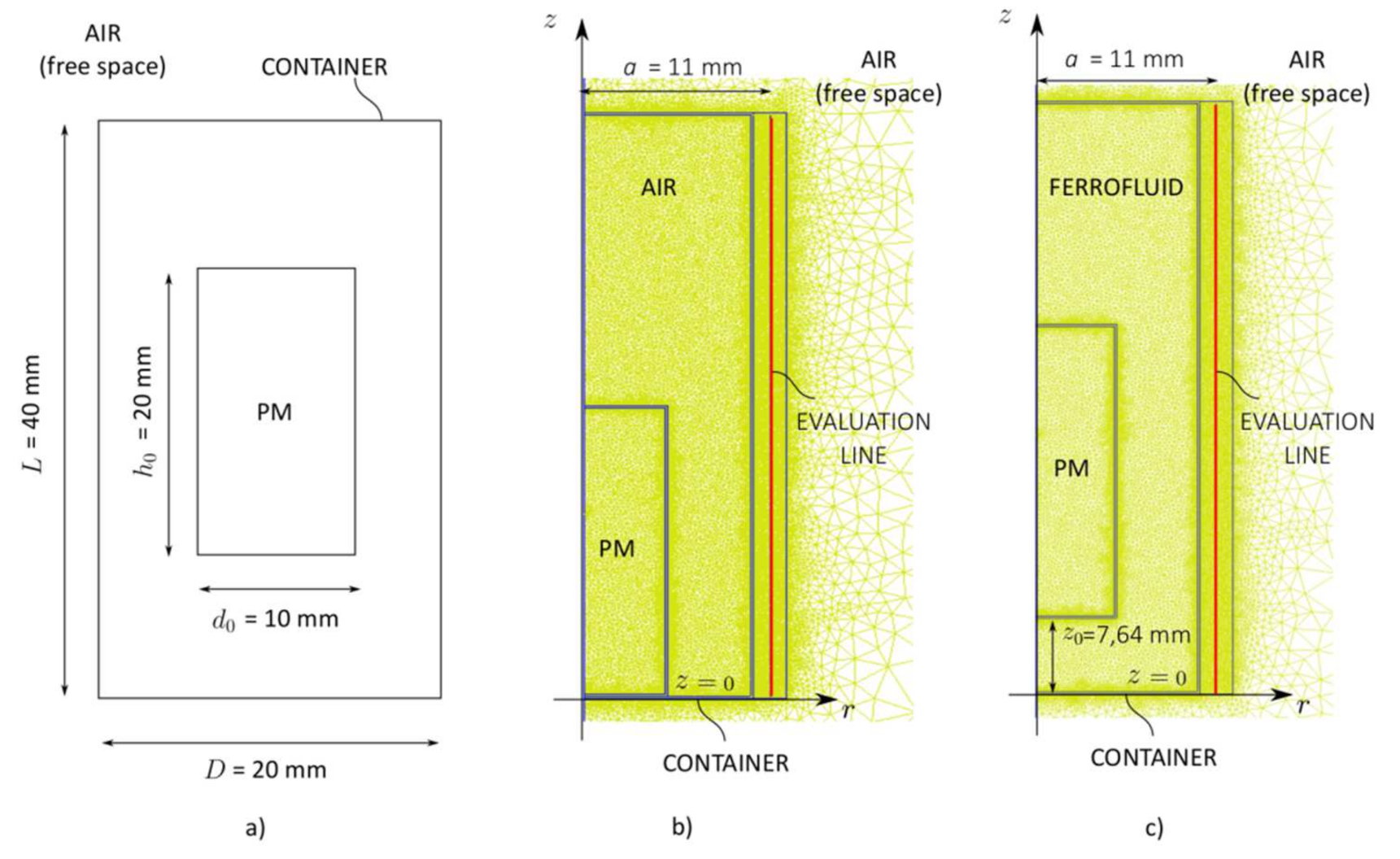




| Permanent Magnet (PM) | Ferrofluid | Free Space (AIR) | |
|---|---|---|---|
| material | NdFeB (N38) | - | Air |
| (kgm−3) | 7450 | 1377.6 | 1.2 |
| 1 | 1.31 | 1 | |
| (T) | 1.24 | - | - |
| (kAm−1) | 986.7 | - | - |
| 0 | 0.31 | 0 | |
| Max. mesh size in material (mm) | 0.25 | 0.25 | 10 |
| Max. mesh size at boundary (mm) | 0.05 | 0.05 | - |
| Max. mesh size near the eval. line (mm) | - | - | 0.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trbušić, M.; Hamler, A.; Goričan, V.; Jesenik, M. Contactless Determination of a Permanent Magnet’s Stable Position within Ferrofluid. Mathematics 2022, 10, 2499. https://doi.org/10.3390/math10142499
Trbušić M, Hamler A, Goričan V, Jesenik M. Contactless Determination of a Permanent Magnet’s Stable Position within Ferrofluid. Mathematics. 2022; 10(14):2499. https://doi.org/10.3390/math10142499
Chicago/Turabian StyleTrbušić, Mislav, Anton Hamler, Viktor Goričan, and Marko Jesenik. 2022. "Contactless Determination of a Permanent Magnet’s Stable Position within Ferrofluid" Mathematics 10, no. 14: 2499. https://doi.org/10.3390/math10142499
APA StyleTrbušić, M., Hamler, A., Goričan, V., & Jesenik, M. (2022). Contactless Determination of a Permanent Magnet’s Stable Position within Ferrofluid. Mathematics, 10(14), 2499. https://doi.org/10.3390/math10142499







