Students’ Understanding of Microscopic Models of Electrical and Thermal Conductivity: Findings within the Development of a Multiple-Choice Concept Inventory
Abstract
1. Introduction
2. Materials and Methods
2.1. METCS Concept Inventory Design
2.2. Participants
2.3. Procedures
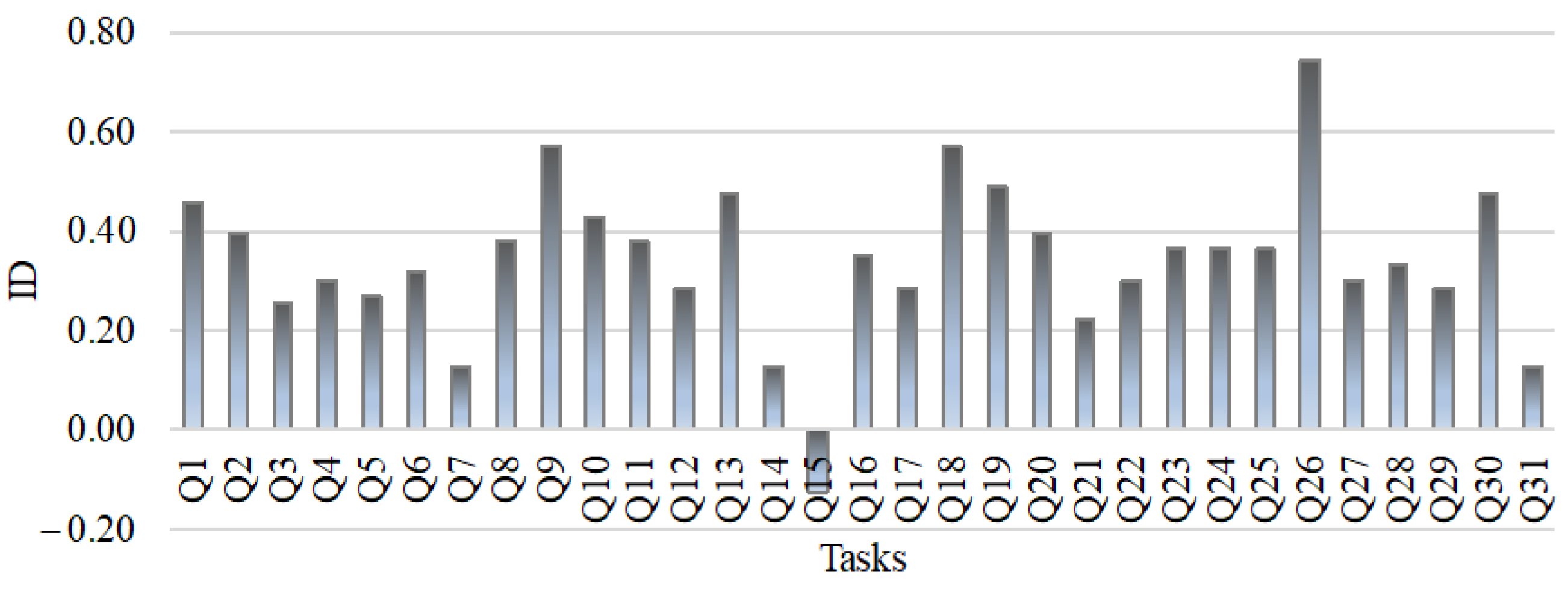
2.4. Item Discrimination Index and Item Difficulty
2.5. Validity and Reliability Evidence
- Check whether they can agree that the individual tasks can be associated with the given concepts and categories, as has been displayed in Table 1.
- Check whether the tasks have been clearly and comprehensibly formulated.
- Check the correct answer and examine whether the alternative answers are correctly defined.
3. Results and Discussion
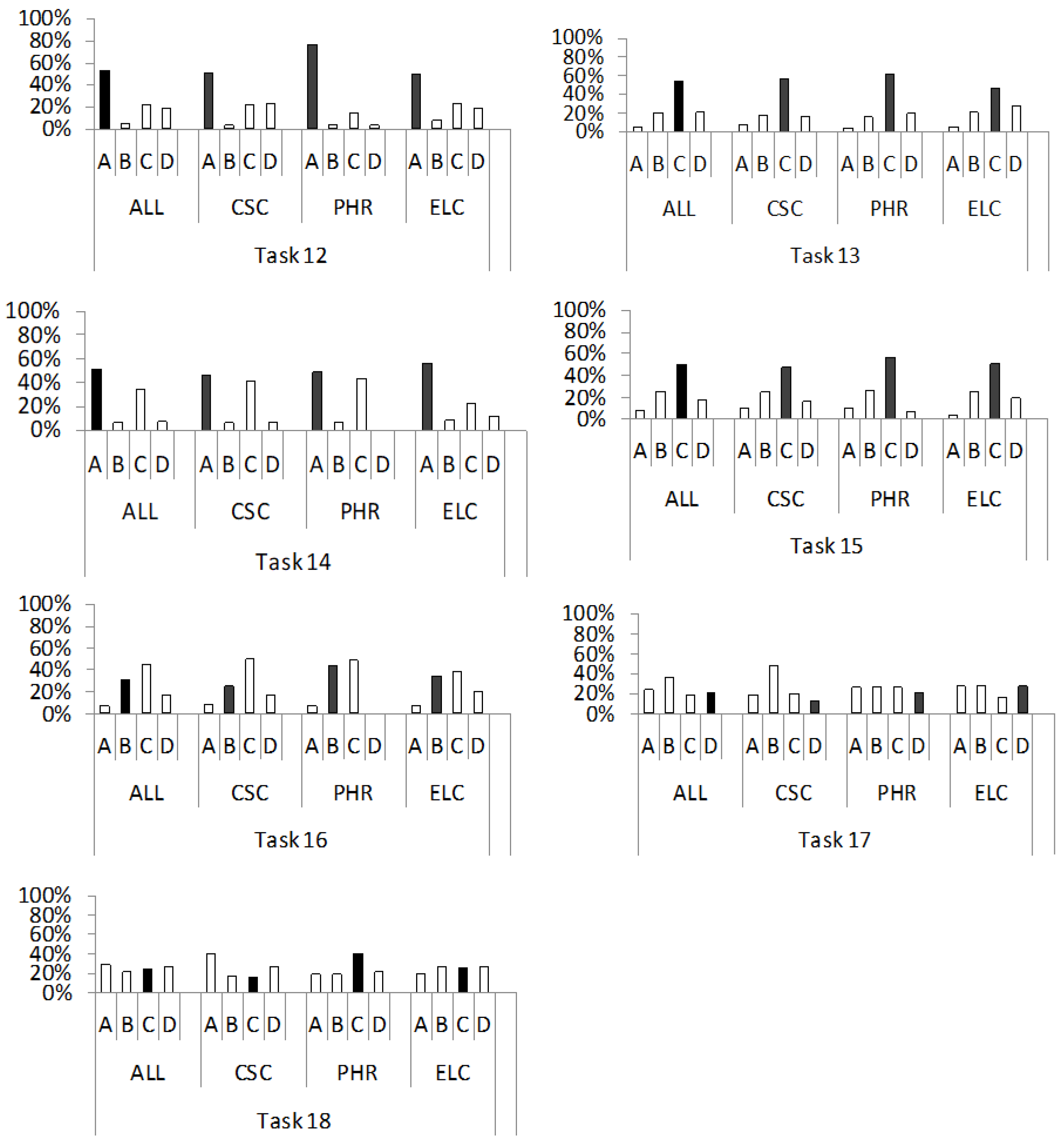
3.1. Microscopic Structure of Solids
3.2. Motion of Particles in Solids at a Certain Temperature
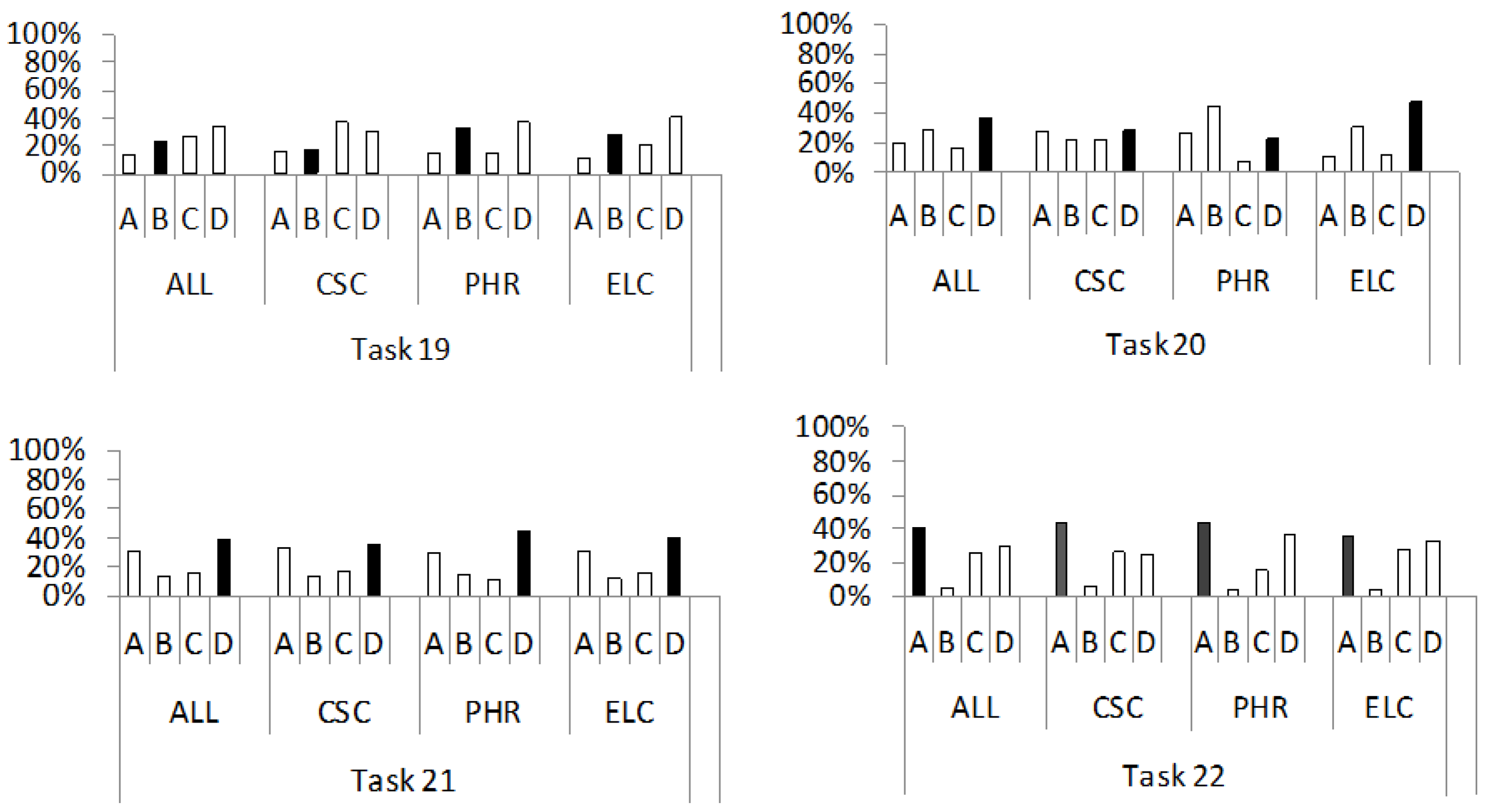
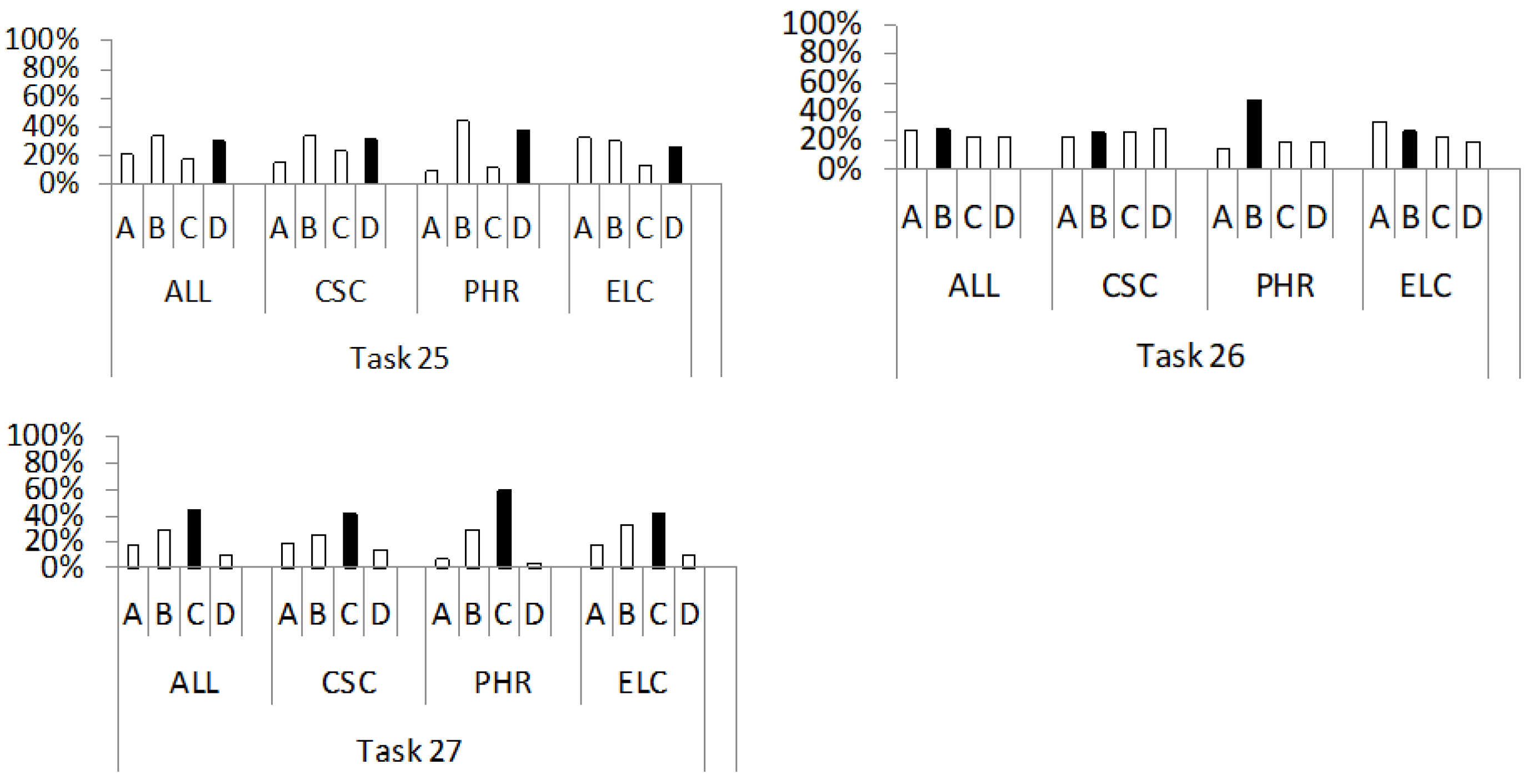
3.3. Motion of Charge Carriers in Solids in the External Electric Field
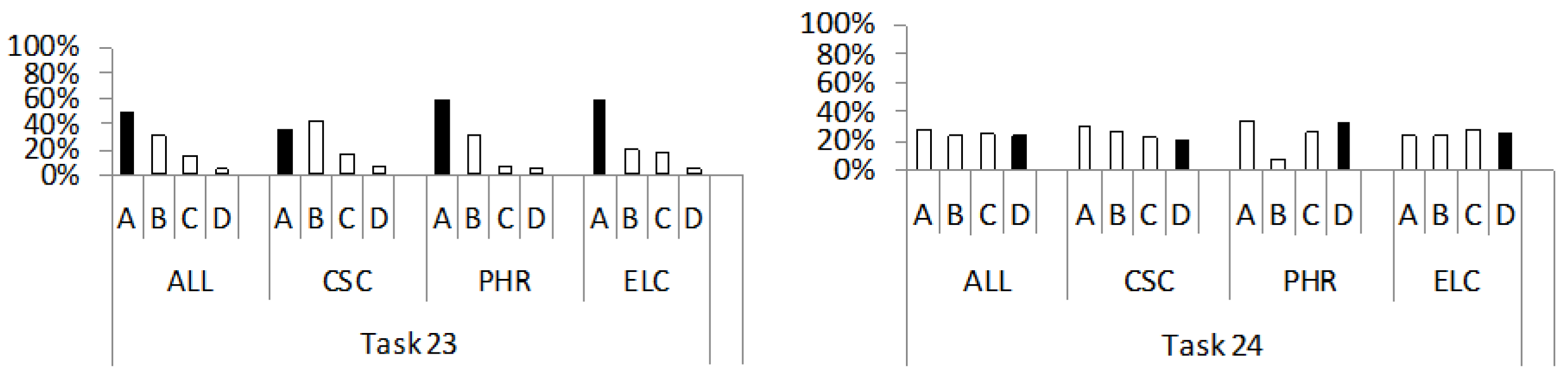
3.4. Electrical Conductivity/Resistance of Solids as a Function of Temperature
3.5. Thermal Conductivity of Solids
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. The Close-Ended Version of the METCS Concept Inventory
- The structure of insulators, conductors and semiconductors as solids can be
- (A)
- Crystalline or amorphous.
- (B)
- Crystalline only.
- (C)
- Crystalline for conductors and semiconductors, and amorphous for insulators.
- (D)
- Crystalline for insulators and amorphous for conductors and semiconductors.
- The lattice of the solid insulator consists of
- (A)
- Atoms connected by covalent bonds.
- (B)
- Atoms connected by covalent or metallic bonds.
- (C)
- Atoms connected by covalent or ionic bonds.
- (D)
- Ions connected by Van der Waals forces.
- Charge carriers in the insulator
- (A)
- Are electrons that do not have a pair in a covalent bond.
- (B)
- Are free electrons.
- (C)
- Are ions.
- (D)
- Do not exist.
- The solid conductor–metal lattice consists of
- (A)
- Positive ions connected by free electrons.
- (B)
- Atoms connected by covalent bonds.
- (C)
- Molecules attracted by Van der Waals forces.
- (D)
- Positive and negative ions connected by a metal bond.
- Electrical current carriers in metals are
- (A)
- Free electrons that form a metallic bond.
- (B)
- Free excitons.
- (C)
- Free electrons generated by energetic excitation.
- (D)
- Free electrons generated under the influence of an electric field.
- A solid semiconductor lattice consists of
- (A)
- Electrons and holes.
- (B)
- Atoms connected by covalent bonds.
- (C)
- Molecules connected by Van der Waals forces.
- (D)
- Atoms connected by a metallic bond.
- Why do free electrons in a pure semiconductor, as opposed to those in a metal, form pairs with holes?
- (A)
- In pure semiconductors, the mobility of free electrons is lower than in metals.
- (B)
- Free electrons also form pairs with holes in metals, but they are rarely mentioned.
- (C)
- The covalent bond between the atoms of a semiconductor is weaker than that of a conductor, which is why holes form in it.
- (D)
- In metals, there are already free electrons, and in pure semiconductors, they are only created by the excitation of the electron–hole pair.
- Semiconductors of type N or P are produced by doping pure semiconductors.What is doping?
- (A)
- The replacement of atoms of the crystal lattice by atoms with a similar electronic structure.
- (B)
- The addition of holes or protons to a pure semiconductor.
- (C)
- The addition of conductive substances to a pure semiconductor.
- (D)
- The addition of electrons to fill holes as a kind of defect in the crystal lattice.
- Donor impurities that dope the semiconductor are
- (A)
- Negative electrons.
- (B)
- Positive ions with a lack of electrons.
- (C)
- Negative ions with an excess of electrons.
- (D)
- Neutral atoms with more valence electrons compared to the semiconductor atoms.
- Acceptor impurities with which the semiconductor is doped are
- (A)
- Positive ions with a lack of electrons.
- (B)
- Neutral atoms with fewer valence electrons compared to the semiconductor atoms.
- (C)
- Positive holes.
- (D)
- Negative ions with an excess of electrons.
- What are holes?
- (A)
- Holes are positively charged real particles with which the semiconductor is doped.
- (B)
- Holes are empty spaces on some atoms of the crystal lattice that are created by the release of electrons.
- (C)
- Holes are empty states in the electron band that are created by electron excitation or doping.
- (D)
- Holes are empty spaces created by electrons on acceptor impurities.
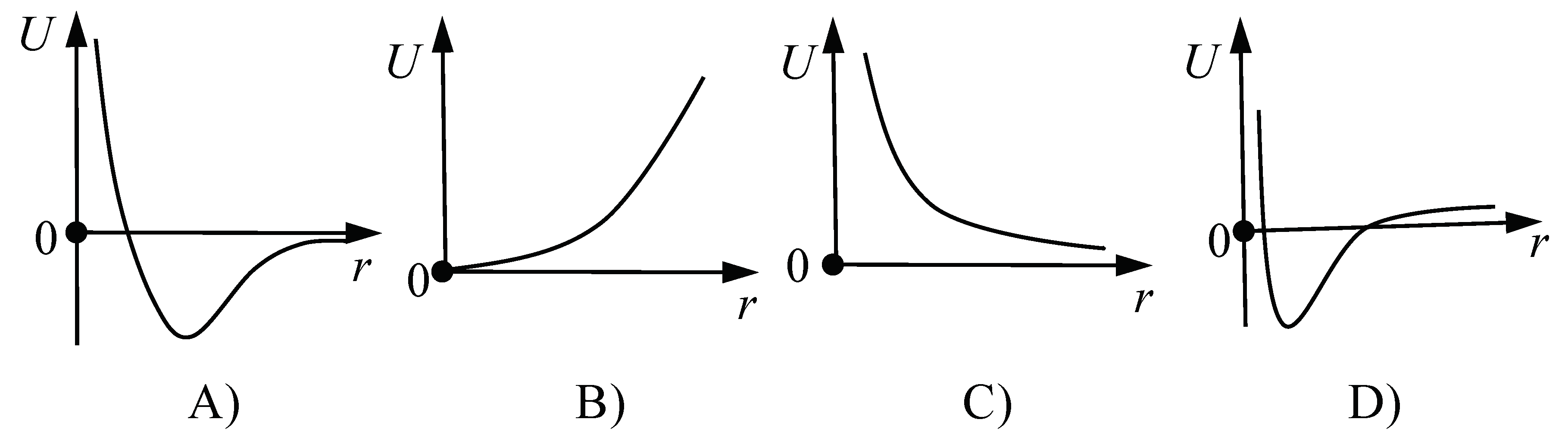
- What is the graphical representation of the dependence of the mutual potential energy of two atoms of a solid, on their distance?
- Do free electrons collide with each other and/or with the ions of the crystal lattice?
- (A)
- Free electrons move freely without colliding with each other.
- (B)
- Free electrons mainly collide with each other like gas molecules.
- (C)
- Free electrons collide with ions of the crystal lattice that are not in the equilibrium position.
- (D)
- Free electrons collide with ions in the crystal lattice that are in the equilibrium position.
- At low temperatures, the collision of free electrons with lattice ions
- (A)
- Significantly reduced due to the smaller amplitude of ion oscillation.
- (B)
- Almost unchanged, as the ions are still in their positions in the lattice.
- (C)
- Reduced due to the lower kinetic energy of the free electrons.
- (D)
- Unchanged as the free electrons mainly collide with each other.
- How do free electrons move in a metal?
- (A)
- They are at rest because no force acts on them.
- (B)
- They move along atomic orbitals, but at a greater distance from the atomic nucleus.
- (C)
- They move at high speeds of the order of 106 m/s through the entire crystal.
- (D)
- They move randomly at low speeds of the order of 1 m/s.
- How do electrons move in the conduction band of a semiconductor?
- (A)
- They are at rest because no force acts on them.
- (B)
- They move freely like the conduction electrons in a metal.
- (C)
- They move with restrictions, as the electronic states are more occupied than in a metal.
- (D)
- They do not move freely, but in a straight line from atom to atom.
- How do the holes in a semiconductor move?
- (A)
- They do not move freely, but in a straight line from atom to atom.
- (B)
- They are at rest because no external electric field acts on them.
- (C)
- They move from orbital to orbital without moving towards other atoms.
- (D)
- They move at high speed like free electrons.
- The mobility of the free electrons of a semiconductor in relation to the holes is
- (A)
- Greater because the holes do not move without the influence of an external electric field.
- (B)
- The same, because they are the same charge carriers in the semiconductor.
- (C)
- Higher, because the energy of the electrons in the conduction band is higher than that of the electrons in the valence band.
- (D)
- Larger in N-type semiconductors and smaller in P-type semiconductors.
- The free electrons in a metal move under the influence of an external electric field
- (A)
- Like a Brownian motion.
- (B)
- In all directions, whereby the direction opposite to the direction of the electric field predominates.
- (C)
- Straight in the direction of the electric field.
- (D)
- In a straight line in the direction opposite to the direction of the electric field.
- The light bulb lights up almost instantly after the circuit is closed because the free electrons are set in motion
- (A)
- Together by an electrical signal travelling at the Fermi speed.
- (B)
- With a drift velocity that directs their motion and is comparable to the speed of light.
- (C)
- By the domino effect, in which the electrons collide and produce a common directional motion.
- (D)
- Almost simultaneously under the effect of an electric field generated at the speed of light.
- The following applies to free charge carriers in a semiconductor under the influence of an external electric field
- (A)
- Electrons move against the direction of the electric field and holes are at rest.
- (B)
- Electrons and holes move in a straight line in the same direction.
- (C)
- The electrons move directionally and the holes move randomly.
- (D)
- Charge carriers move in all directions, with the predominant direction being determined by the electric field.
- Adding an acceptor increases the electrical conductivity of the semiconductor by
- (A)
- Increasing the number of holes.
- (B)
- Increasing the speed of movement of the holes.
- (C)
- Increasing the mobility of the electrons in the conduction band.
- (D)
- Decreasing the energy gap between the valence band and the conduction band.
- As the temperature rises, the electrical resistance of metals
- (A)
- Increases due to the stronger vibration of the ions, which hinders the movement of free electrons.
- (B)
- Decreases because the free electrons move faster.
- (C)
- Increases because the expansion of the metal means that the free electrons collide less and it is more difficult to transfer current.
- (D)
- Does not change because the number of free electrons does not change.
- With increasing temperature, the electrical resistance of a pure semiconductor
- (A)
- Decreases due to the faster movement of the charge carriers.
- (B)
- Increases due to a stronger vibration of the ion, which hinders the motion of the charge carriers.
- (C)
- Changes inversely in relation to metals, due to a higher hole concentration.
- (D)
- Decreases as the number of free charge carriers increases.
- Heat transfer in metal occurs mainly
- (A)
- By the transfer of the vibrational energy of the ions of the solid lattice.
- (B)
- By the transfer of kinetic energy between free electrons.
- (C)
- By the transfer of vibrational energy from free electrons to ions.
- (D)
- By the transfer of kinetic energy from free electrons to ions.
- Insulators are weaker heat conductors than metals because the heat in them is mainly conducted by
- (A)
- The vibrations of the lattice atoms, which are weaker due to the stronger interatomic bonds.
- (B)
- The vibrations of the lattice atoms, but not by free electrons.
- (C)
- The vibrations of electrons that are not free but are bound to lattice ions.
- (D)
- Free charge carriers, the number of which is small.
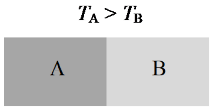
We bring two solid bodies with different temperatures into contact in a thermally insulated system (see image), whereupon heat is transferred from body A to body B: - At the contact surface, by collision of the molecules of body A and body B
- (A)
- Mainly intermolecular potential energy is transferred.
- (B)
- Internal energy is transferred.
- (C)
- Only the kinetic energy of the molecules is transferred.
- (D)
- Additional heat is generated.
References
- Jenkins, T. A Brief History of... Semiconductors. Phys. Educ. 2005, 40, 430–439. [Google Scholar] [CrossRef]
- Žuvela, E.V. FER Osniva Hrvatski Centar Kompetencija za Poluvodiče. Available online: https://hr.bloombergadria.com/ekonomija/hrvatska/51708/fer-osniva-hrvatski-centar-kompetencija-za-poluvodice/news (accessed on 5 February 2024).
- Erceg, N.; Jelovica, L.; Hrepić, Z.; Mešić, V.; Karuza, M.; Aviani, I. University Students’ Conceptual Understanding of Microscopic Models of Electrical and Thermal Conduction in Solids. Eur. J. Phys. 2021, 42, 045702. [Google Scholar] [CrossRef]
- Acar, B.; Tarhan, L. Effects of Cooperative Learning on Students’ Understanding of Metallic Bonding. Res. Sci. Educ. 2008, 38, 401–420. [Google Scholar] [CrossRef]
- García-Carmona, A.; Criado, A.M. Introduction to Semiconductor Physics in Secondary Education: Evaluation of a Teaching Sequence. Int. J. Sci. Educ. 2009, 31, 2205–2245. [Google Scholar] [CrossRef][Green Version]
- Fayyaz, F.; Ashraf Iqbal, M.; Hashmi, Y. Problems of Learning Fundamentals of Semiconductor Electronics. Int. J. Pedagog. Learn. 2005, 1, 3–17. [Google Scholar] [CrossRef]
- Wettergren, J. Understanding Concepts Needed for Semiconductor Physics. Eur. J. Eng. Educ. 2002, 27, 105–111. [Google Scholar] [CrossRef]
- De Posada, J.M. Conceptions of High School Students Concerning the Internal Structure of Metals and Their Electric Conduction: Structure and Evolution. Sci. Ed. 1997, 81, 445–467. [Google Scholar] [CrossRef]
- Coll, R.K.; Taylor, N. Alternative Conceptions of Chemical Bonding Held by Upper Secondary and Tertiary Students. Res. Sci. Technol. Educ. 2001, 19, 171–191. [Google Scholar] [CrossRef]
- Taber, K.S. Building the Structural Concepts of Chemistry: Some Considerations from Educational Research. Chem. Educ. Res. Pract. 2001, 2, 123–158. [Google Scholar] [CrossRef]
- Taber, K.S. Mediating Mental Models of Metals: Acknowledging the Priority of the Learner’s Prior Learning. Sci. Ed. 2003, 87, 732–758. [Google Scholar] [CrossRef]
- Mi, S.; Ye, J.; Yan, L.; Bi, H. Development and Validation of a Conceptual Survey Instrument to Evaluate Senior High School Students’ Understanding of Electrostatics. Phys. Rev. Phys. Educ. Res. 2023, 19, 010114. [Google Scholar] [CrossRef]
- Santana, L.M.; Hickman, C.; Bilak, J.; Singh, C. Investigating and Improving Student Understanding of Conductors and Insulators. Educ. Sci. 2023, 13, 242. [Google Scholar] [CrossRef]
- Ali, M.T.; Woldu, A.R.; Yohannes, A.G. High School Students’ Learning Difficulties in Electrochemistry: A Mini Review. Afr. J. Chem. Educ. 2022, 12, 202–237. [Google Scholar]
- Nelson, K.G.; McKenna, A.F.; Brem, S.K.; Hilpert, J.; Husman, J.; Pettinato, E. Students’ Misconceptions about Semiconductors and Use of Knowledge in Simulations. J. Eng. Educ. 2017, 106, 218–244. [Google Scholar] [CrossRef]
- Liu, Z.; Pan, S.; Zhang, X.; Bao, L. Assessment of Knowledge Integration in Student Learning of Simple Electric Circuits. Phys. Rev. Phys. Educ. Res. 2022, 18, 020102. [Google Scholar] [CrossRef]
- Fenditasari, K.; Jumadi; Istiyono, E.; Hendra. Identification of Misconceptions on Heat and Temperature among Physics Education Students Using Four-Tier Diagnostic Test. J. Phys. Conf. Ser. 2020, 1470, 012055. [Google Scholar] [CrossRef]
- Kotsis, K.T.; Stylos, G.; Houssou, P.; Kamaratos, M. Students’ Perceptions of the Heat and Temperature Concepts: A Comparative Study between Primary, Secondary, and University Levels. Eur. J. Educ. Pedagog. 2023, 4, 136–144. [Google Scholar] [CrossRef]
- Wittmann, M.C.; Steinberg, R.N.; Redish, E.F. Investigating Student Understanding of Quantum Physics: Spontaneous Models of Conductivity. Am. J. Phys. 2002, 70, 218–226. [Google Scholar] [CrossRef]
- Pathare, S.R.; Pradhan, H.C. Students’ Misconceptions about Heat Transfer Mechanisms and Elementary Kinetic Theory. Phys. Educ. 2010, 45, 629–634. [Google Scholar] [CrossRef]
- Lee, Y.; Law, N. Explorations In Promoting Conceptual Change in Electrical Concepts via Ontological Category Shift. Int. J. Sci. Educ. 2001, 23, 111–149. [Google Scholar] [CrossRef]
- Lee, C.K. A Conceptual Change Model for Teaching Heat Energy, Heat Transfer and Insulation. Sci. Educ. Int. 2014, 25, 417–437. [Google Scholar]
- Prince, M.; Vigeant, M.; Nottis, K. Repairing Student Misconceptions in Heat Transfer Using Inquiry-Based Activities. Chem. Eng. Educ. 2016, 50, 52–61. [Google Scholar]
- Knight, R.D.; Burciaga, J.R. Five Easy Lessons: Strategies for Successful Physics Teaching. Am. J. Phys. 2004, 72, 414. [Google Scholar] [CrossRef]
- Dayal, P.; Chand, Z. Effective Teaching and Learning Strategies in a Chemistry Classroom. N. Z. J. Educ. Stud. 2022, 57, 425–443. [Google Scholar] [CrossRef]
- Devetak, I.; Vogrinc, J.; Glažar, S.A. States of Matter Explanations in Slovenian Textbooks for Students Aged 6 to 14. Int. J. Environ. Sci. Educ. 2010, 5, 217–235. [Google Scholar]
- Clifford, P. The Pressure-Flow Hypothesis of Phloem Transport: Misconceptions in the A-Level Textbooks. J. Biol. Educ. 2002, 36, 110–112. [Google Scholar] [CrossRef]
- Obafemi, D.; Aderonmu, T. Identification and Sources of Misconceptions Held by Secondary School Physics Students in Heat Energy in Rivers State. Eur. J. Educ. Stud. 2022, 9. [Google Scholar] [CrossRef]
- Si, M.; Lestari, D. Mustafa Identification of the Causes of Misconception on the Concept of Dynamic Electricity. J. Phys. Conf. Ser. 2019, 1280, 052060. [Google Scholar] [CrossRef]
- Stylos, G.; Sargioti, A.; Mavridis, D.; Kotsis, K.T. Validation of the Thermal Concept Evaluation Test for Greek University Students’ Misconceptions of Thermal Concepts. Int. J. Sci. Educ. 2021, 43, 247–273. [Google Scholar] [CrossRef]
- diSessa, A.A. Toward an Epistemology of Physics. Cogn. Instr. 1993, 10, 105–225. [Google Scholar] [CrossRef]
- Madsen, A.; McKagan, S.; Sayre, E. Best Practices for Administering Concept Inventories. Phys. Teach. 2017, 55, 530–536. [Google Scholar] [CrossRef]
- Lindell, R.S.; Peak, E.; Foster, T.M. Are They All Created Equal? A Comparison of Different Concept Inventory Development Methodologies. AIP Conf. Proc. 2007, 883, 14–17. [Google Scholar] [CrossRef]
- Adams, W.K.; Wieman, C.E. Development and Validation of Instruments to Measure Learning of Expert-Like Thinking. Int. J. Sci. Educ. 2011, 33, 1289–1312. [Google Scholar] [CrossRef]
- Cohen, L.; Manion, L.; Morrison, K. Research Methods in Education; Routledge: London, UK, 2017; ISBN 978-1-315-45652-2. [Google Scholar]
- PhysPort Assessments-Electricity. Available online: https://www.physport.org/assessments/?Q=&Subjects=85&fCI=& (accessed on 4 September 2023).
- PhysPort Assessments-Thermal. Available online: https://www.physport.org/assessments/?Q=&Subjects=89&fCI=& (accessed on 4 September 2023).
- Yeo, S.; Zadnik, M. Introductory Thermal Concept Evaluation: Assessing Students’ Understanding. Phys. Teach. 2001, 39, 496–504. [Google Scholar] [CrossRef]
- Odluka o Donošenju Kurikuluma Za Nastavni Predmet Fizike Za Osnovne Škole i Gimnazije u Republici Hrvatskoj. Available online: https://narodne-novine.nn.hr/clanci/sluzbeni/2019_01_10_210.html (accessed on 4 September 2023).
- Odluka o Donošenju Nacionalnog Kurikuluma za Strukovno Obrazovanje. Available online: https://narodne-novine.nn.hr/clanci/sluzbeni/2018_07_62_1295.html (accessed on 4 September 2023).
- INP Fizika, II. 2023. Available online: http://www.riteh.uniri.hr/nast/program/spoint/izvedbeni84169.pdf (accessed on 10 September 2023).
- INP Uvod u Fiziku. 2023. Available online: http://www.riteh.uniri.hr/nast/program/spoint/izvedbeni226055.pdf (accessed on 10 September 2023).
- INP Fizika za Farmaceute. 2023. Available online: https://medri.uniri.hr/wp-content/uploads/2022/10/Fizika-za-farmaceute_INP_2022_23.pdf (accessed on 10 September 2023).
- Young, H.D.; Freedman, R.A.; Young, H.D. Sears and Zemansky’s University Physics, 14th ed.; Pearson Education: Harlow, UK, 2016; ISBN 978-0-13-396929-0. [Google Scholar]
- Kelley, T.L. The Selection of Upper and Lower Groups for the Validation of Test Items. J. Educ. Psychol. 1939, 30, 17–24. [Google Scholar] [CrossRef]
- Bardar, E.; Prather, E.; Brecher, K.; Slater, T. First Results from the Light and Spectroscopy Concept Inventory. Astron. Educ. Rev. 2005, 6, 1209. [Google Scholar] [CrossRef][Green Version]
- Barić Tominac, M.; Habuš, A.; Liber, S.; Vladušić, R. Kemija 1, Udžbenik Kemije za Prvi Razred Gimnazije; Profil Klett: Zagreb, Croatia, 2019. [Google Scholar]
- Lukić, S.; Marić Zerdun, I.; Trenčevska, N.; Varga, M.; Rupčić Petelinc, S. Kemija 7, Udžbenik Kemije u Sedmom Razredu Osnovne Škole; Školska Knjiga: Zagreb, Croatia, 2020. [Google Scholar]
- Dropuljić, M.; Ivković, S.; Paris, T.; Petričević, I.; Takač, D.; Tuhtan, S.; Zakanji, I. Fizika 8, Udžbenik za Istraživačku Nastavu Fizike u Osmom Razredu Osnovne Škole; Profil Klett: Zagreb, Croatia, 2020. [Google Scholar]
- Paar, V.; Martinko, S.; Ćulibrk, T. Fizika Oko Nas 8, Udžbenik Fizike u Osmom Razredu Osnovne Škole; Školska Knjiga: Zagreb, Croatia, 2021. [Google Scholar]
- Furčić, N. Elektronika I, Udžbenik za 2. Razred Srednjih Strukovnih Škola; Element: Zagreb, Croatia, 2021. [Google Scholar]
- Furčić, N.; Varga, Z. Elektrotehnika i Elektronika za Brodostrojarstvo, Udžbenik Za 3. Razred Srednjih Strukovnih Škola, (Za Zanimanje Tehničar za Brodostrojarstvo); Element: Zagreb, Croatia, 2021. [Google Scholar]
- RiTeh. Studijski Program Prijediplomskog Sveučilišnog Studija Elektrotehnike: Tehnologija Materijala i Elektronika I; RiTeh: Rijeka, Croatia, 2021. [Google Scholar]
- Ünal, S.; Coştu, B.; Ayas, A. Secondary School Students’ Misconceptions of Covalent Bonding. J. Turk. Sci. Educ. 2010, 7, 3–29. [Google Scholar]
- Reif, F. Applying Cognitive Science to Education: Thinking and Learning in Scientific and Other Complex Domains; A Bradford Book: Cambridge, MA, USA, 2010; ISBN 978-0-262-51514-6. [Google Scholar]
- Kasap, S.O. Principles of Electronic Materials and Devices, 3rd ed.; McGraw-Hill: Boston, MA, USA, 2006; ISBN 978-0-07-295791-4. [Google Scholar]
- Kittel, C. Introduction to Solid State Physics, 8th ed.; Wiley: Hoboken, NJ, USA, 2005; ISBN 978-0-471-41526-8. [Google Scholar]
- Furčić, N.; Varga, Z. Osnove Elektrotehnike 1, Udžbenik za Prvi Razred Srednjih Strukovnih Škola; Element: Zagreb, Croatia, 2021. [Google Scholar]
- Paar, V.; Hrlec, A.; Vadlja Rešetar, K.; Sambolek, M. Fizika Oko Nas 2, Udžbenik Fizike u Drugom Razredu Srednjih Škola s Četverogodišnjim Programom Fizike; Školska Knjiga: Zagreb, Croatia, 2021. [Google Scholar]
- Luetić, M.; Petrović Peroković, V.; Preočanin, T.; Rupčić Petelinc, S.; Turčinović, D. Kemija 1, Udžbenik Kemije u Prvom Razredu Gimnazije; Školska Knjiga: Zagreb, Croatia, 2022. [Google Scholar]
- Erceg, N.; Aviani, I.; Mešić, V.; Glunčić, M.; Žauhar, G. Development of the Kinetic Molecular Theory of Gases Concept Inventory: Preliminary Results on University Students’ Misconceptions. Phys. Rev. Phys. Educ. Res. 2016, 12, 020139. [Google Scholar] [CrossRef]
- Erceg, N.; Aviani, I.; Grlaš, K.; Karuza, M.; Mešić, V. Development of the Kinetic Molecular Theory of Liquids Concept Inventory: Preliminary Results on University Students’ Misconceptions. Eur. J. Phys. 2019, 40, 025704. [Google Scholar] [CrossRef]
- Hofmann, P. Solid State Physics: An Introduction; John Wiley & Sons: Hoboken, NJ, USA, 2022; ISBN 978-3-527-41410-9. [Google Scholar]
- Epifanov, G.I. Solid State Physics; MIR Publishers: Moscow, Russia, 1979. [Google Scholar]
- Redish, E.F. Teaching Physics with the Physics Suite CD; John Wiley & Sons Inc.: New York, NY, USA, 2003; ISBN 978-0-471-39378-8. [Google Scholar]
- Meltzer, D.E. Investigation of Students’ Reasoning Regarding Heat, Work, and the First Law of Thermodynamics in an Introductory Calculus-Based General Physics Course. Am. J. Phys. 2004, 72, 1432–1446. [Google Scholar] [CrossRef]

| Conceptual Groups | Concepts | Tasks | ES | SS | U |
|---|---|---|---|---|---|
| 1. Microscopic structure of solids |
| 1,2 | X | X | X |
| 1,4 | X | X | X | |
| 1,6 | X | X | X | |
| 3,5,7,8 | X | X | ||
| 9 | X | X | ||
| 10 | X | X | ||
| 11 | X | X | ||
| 12 | X | X | ||
| 2. Interparticle potential energy of solids |
| 13 | X | X | |
| 14 | X | X | ||
| 3. Motion of particles in solids at a certain temperature |
| 15 | X | X | X |
| 16 | X | X | ||
| 17 | X | X | ||
| 18 | X | X | ||
| 19 | X | X | ||
| 20 | X | X | ||
| 21 | X | |||
| 4. Motion of charge carriers in solids in the external electric field |
| 22,23 | X | X | X |
| 24 | X | X | ||
| 25 | X | X | ||
| 5. Electrical conductivity/resistance of solids as a function of temperature |
| 26 | X | X | |
| 27 | X | |||
| 6. Thermal conductivity of solids |
| 28 | X | X | X |
| 29 | X | X | X | |
| 30 | X | X | ||
| 31 | X | X |
| Name of the Group and Number of Participants | Name of the University Study Programme | Name of the Course, the Number of Hours (L, AE, PE) and Place in Curriculum | Number of the Class Hours Devoted to ETCS Concepts |
|---|---|---|---|
| PHR (NPHR = 27) | University integrated undergraduate and graduate study of Pharmacy | Physics for Pharmacists (30L + 30AE + 15PE) 1st year, 1st semester | 6L |
| CSC (NCSC = 106) | University undergraduate study of Computer Science | Introduction to Physics (30L + 30AE) 1st year, 1st semester | 6L |
| ELC (NELC = 100) | University undergraduate study of Electrical Engineering | Physics II (30L + 30AE) 1st year, 2nd semester | 6L |
| Item | Q1 | Q2 | Q3 | Q4 | Q5 | Q6 | Q7 | Q8 | Q9 | Q10 | Q11 | Q12 | Q13 | Q14 |
| mean | 0.64 | 0.42 | 0.66 | 0.36 | 0.32 | 0.33 | 0.61 | 0.39 | 0.34 | 0.34 | 0.31 | 0.54 | 0.53 | 0.51 |
| Item | Q15 | Q16 | Q17 | Q18 | Q19 | Q20 | Q21 | Q22 | Q23 | Q24 | Q25 | Q26 | Q27 | |
| mean | 0.50 | 0.31 | 0.21 | 0.24 | 0.24 | 0.36 | 0.39 | 0.41 | 0.48 | 0.24 | 0.30 | 0.28 | 0.44 |
| Statistic | df | Sig. | |
|---|---|---|---|
| total score | 0.138 | 233 | 0.000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jelovica, L.; Erceg, N.; Mešić, V.; Aviani, I. Students’ Understanding of Microscopic Models of Electrical and Thermal Conductivity: Findings within the Development of a Multiple-Choice Concept Inventory. Educ. Sci. 2024, 14, 275. https://doi.org/10.3390/educsci14030275
Jelovica L, Erceg N, Mešić V, Aviani I. Students’ Understanding of Microscopic Models of Electrical and Thermal Conductivity: Findings within the Development of a Multiple-Choice Concept Inventory. Education Sciences. 2024; 14(3):275. https://doi.org/10.3390/educsci14030275
Chicago/Turabian StyleJelovica, Lejla, Nataša Erceg, Vanes Mešić, and Ivica Aviani. 2024. "Students’ Understanding of Microscopic Models of Electrical and Thermal Conductivity: Findings within the Development of a Multiple-Choice Concept Inventory" Education Sciences 14, no. 3: 275. https://doi.org/10.3390/educsci14030275
APA StyleJelovica, L., Erceg, N., Mešić, V., & Aviani, I. (2024). Students’ Understanding of Microscopic Models of Electrical and Thermal Conductivity: Findings within the Development of a Multiple-Choice Concept Inventory. Education Sciences, 14(3), 275. https://doi.org/10.3390/educsci14030275