Iron X-ray Transmission at Temperature Near 150 eV Using the National Ignition Facility: First Measurements and Paths to Uncertainty Reduction
Abstract
:1. Introduction
1.1. Structure of the Paper
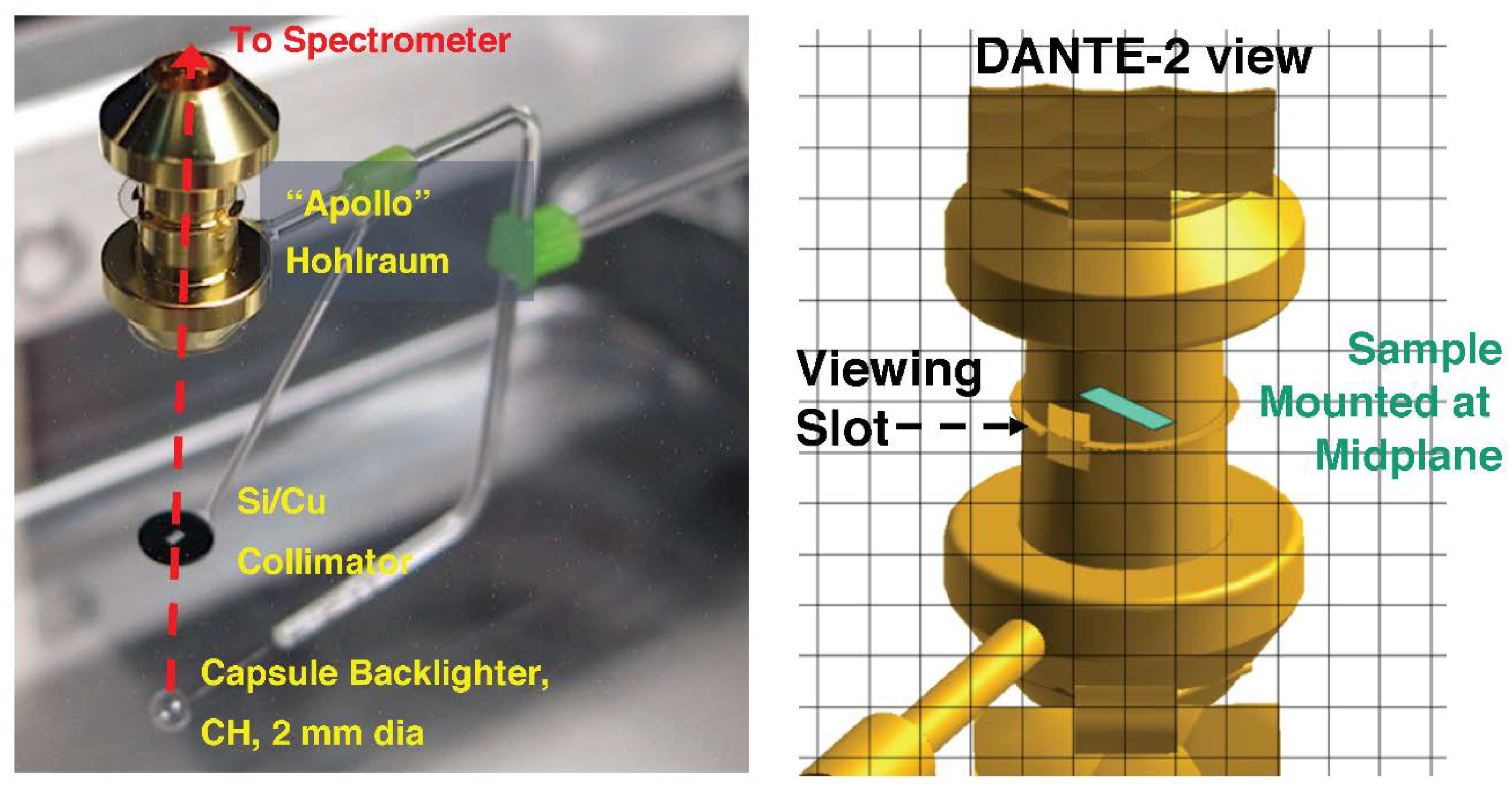
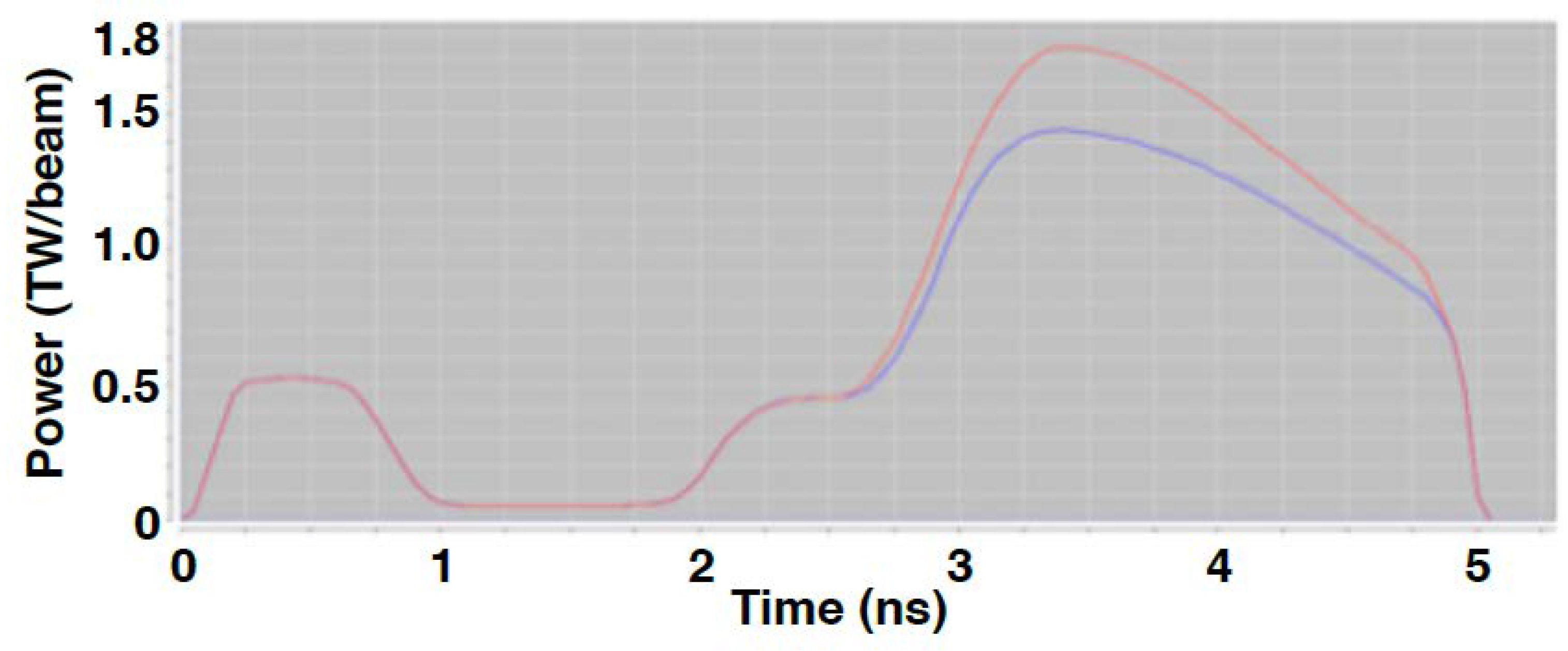
1.2. Introduction to the NIF Opacity Experiment
1.3. Introduction to the Measurements on NIF Shot N171214-001
2. Results
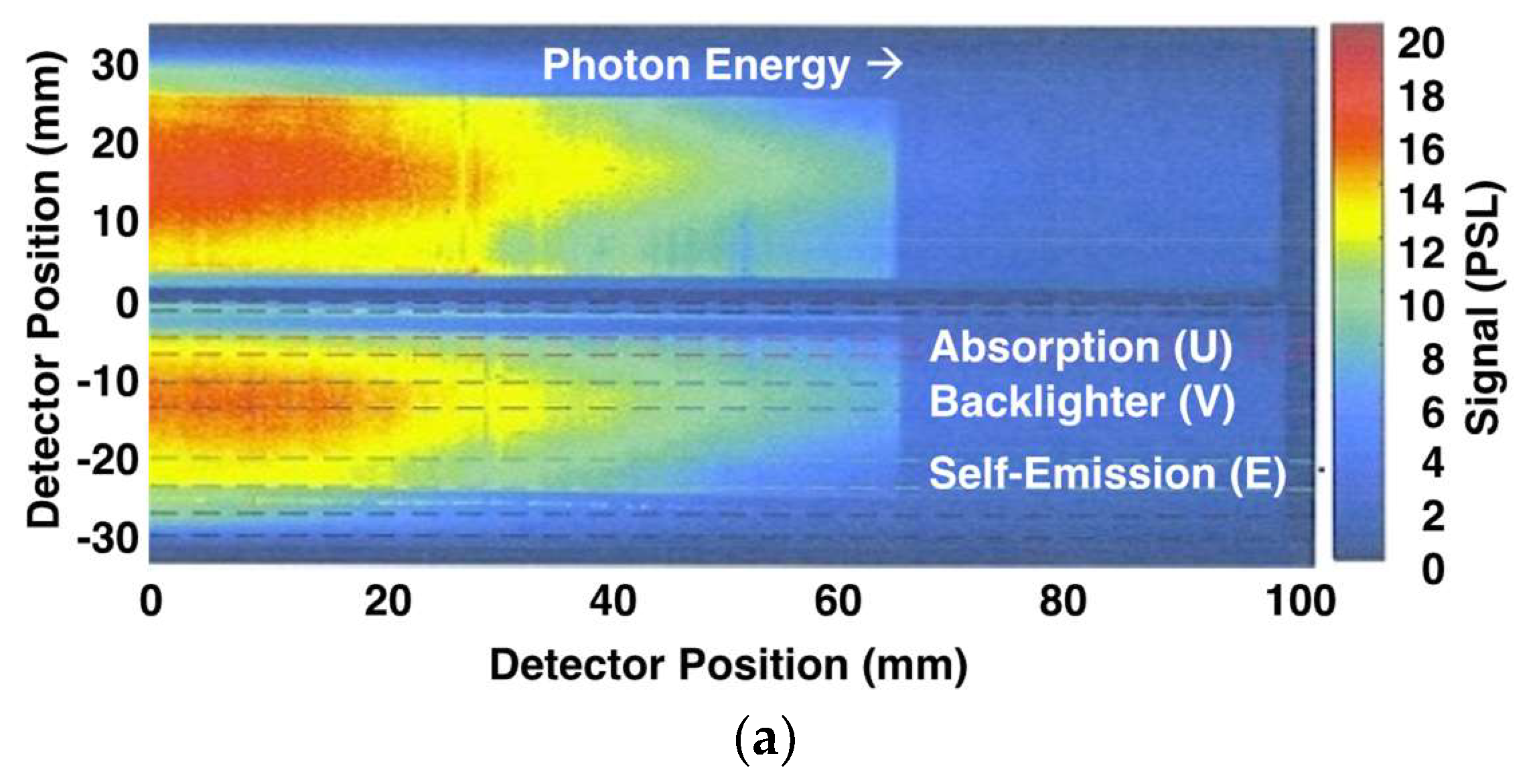
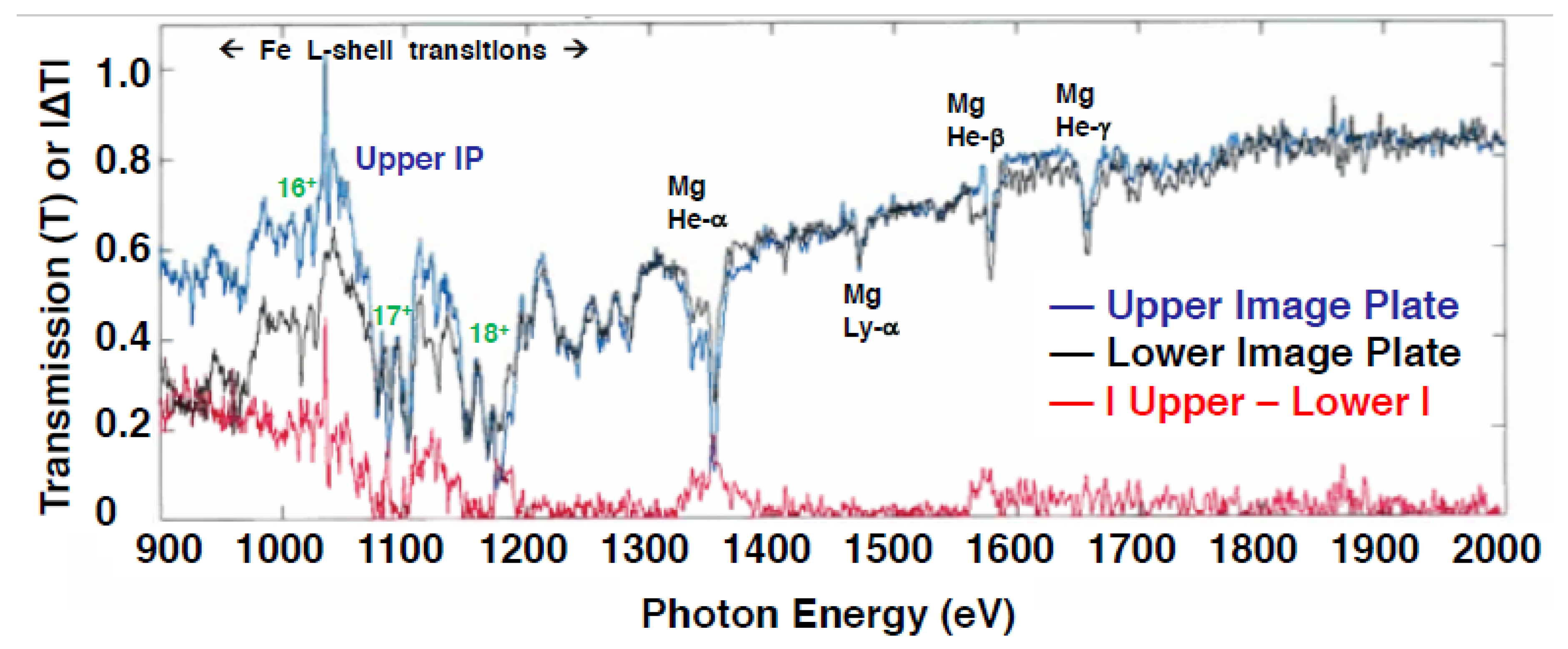
2.1. X-ray Transmission Spectra
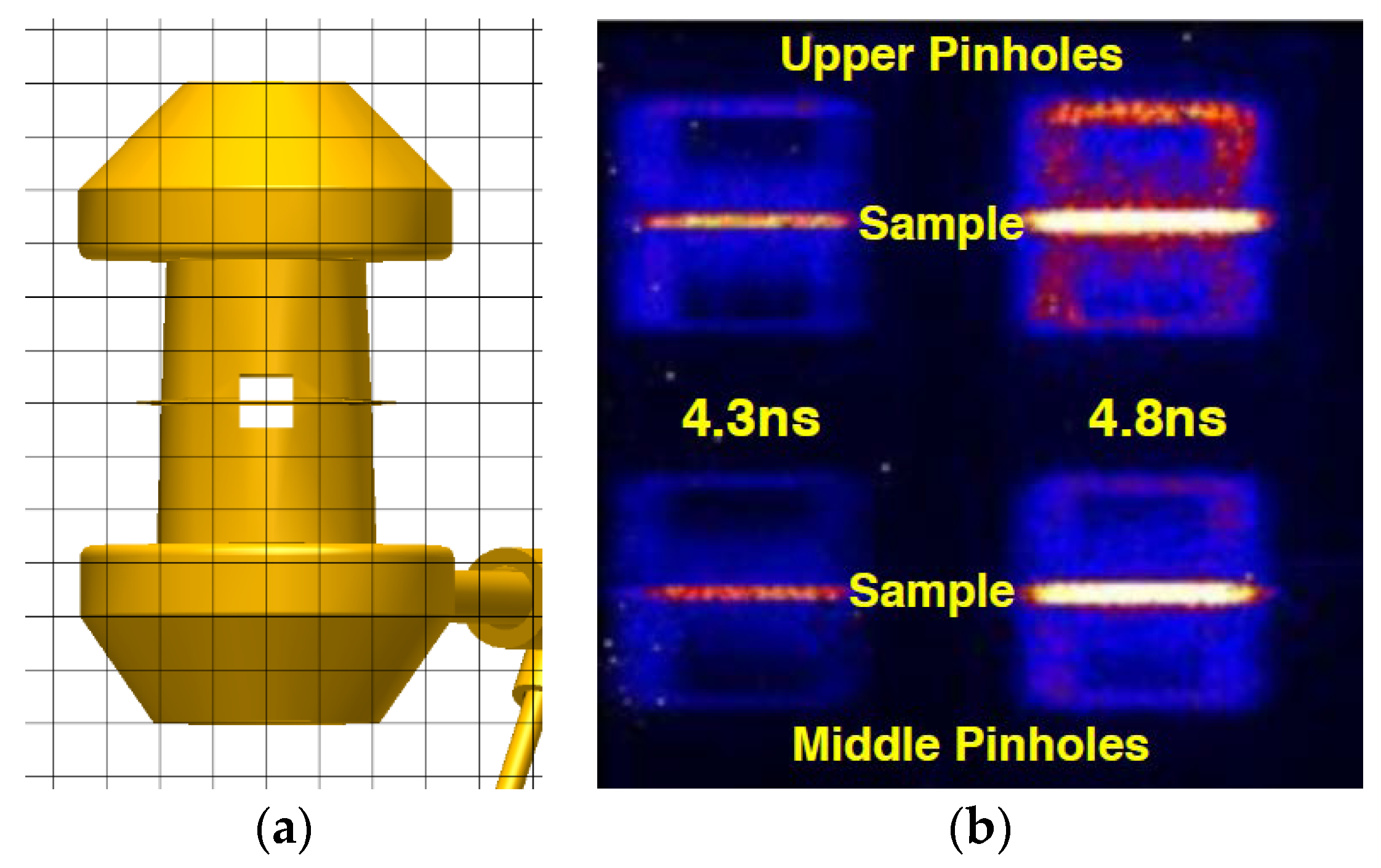
2.2. Plasma Conditions
3. Discussion
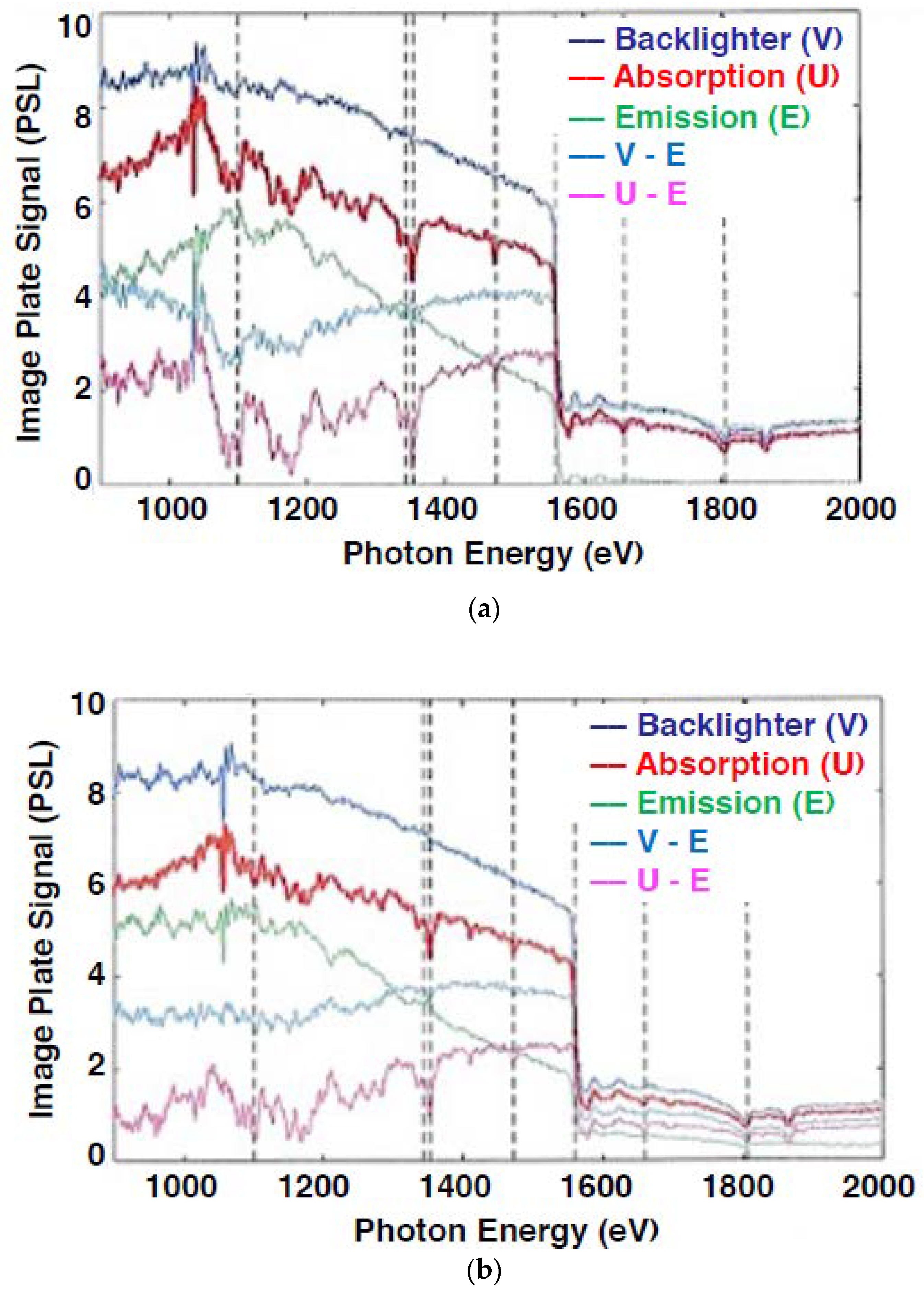
4. Methods: Processing of the Transmission Spectra
4.1. Lineouts
4.2. Background Subtraction
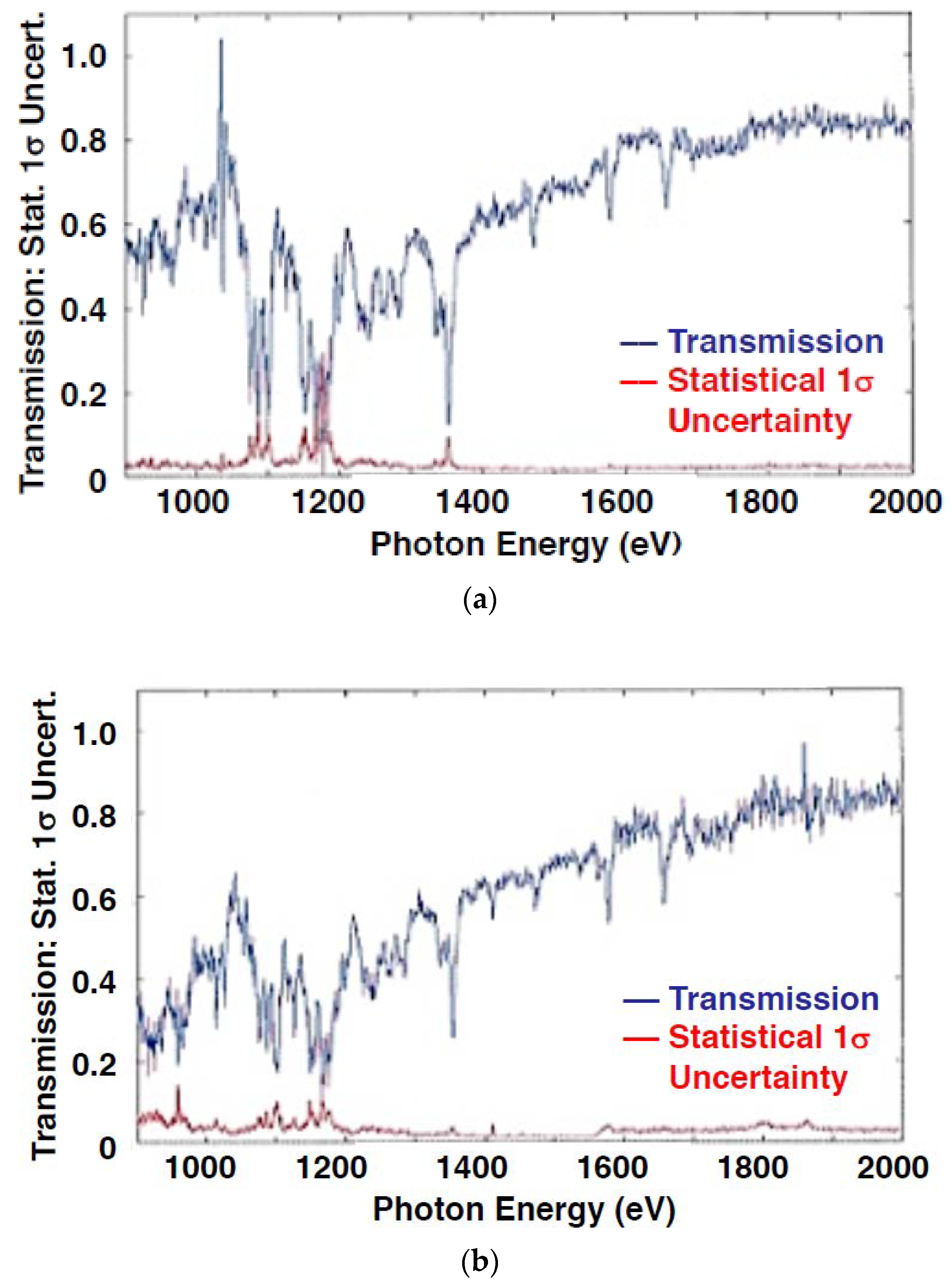
4.3. Transmission and Statistical Uncertainty
5. Sources of Statistical Uncertainty
6. Sources of Systematic Uncertainty
6.1. Diffuse Scattering and Fluorescence
6.2. Diffraction from Alternative Crystal Planes
6.3. Higher-Order Bragg Diffraction from the Primary (001) Plane
6.4. Potential Additional Sources of Systematic Error
7. Summary and Future Directions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bailey, J.; Rochau, G.; Iglesias, C.; Abdallah, J.; MacFarlane, J.; Golovkin, I.; Wang, P.; Mancini, R.; Lake, P.; Moore, T.; et al. Iron-plasma transmission measurements at temperatures above 150 eV. Phys. Rev. Lett. 2007, 99, 265002. [Google Scholar] [CrossRef] [PubMed]
- Bailey, J.; Nagayama, T.; Loisel, G.; Rochau, G.; Blancard, C.; Colgan, J.; Cosse, P.; Faussurier, G.; Fontes, C.; Gilleron, F.; et al. A higher-than-predicted measurement of iron opacity at solar interior temperatures. Nature 2015, 517, 56–59. [Google Scholar] [CrossRef] [PubMed]
- Heeter, R.; Bailey, J.; Craxton, R.; DeVolder, B.; Dodd, E.; Garcia, E.; Huffman, E.; Iglesias, C.; King, J.; Kline, J.; et al. Conceptual design of initial opacity experiments on the national ignition facility. J. Plasma Phys. 2017, 83, 595830103. [Google Scholar] [CrossRef]
- Perry, T.; Heeter, R.; Opachich, Y.; Ross, P.; Kline, J.; Flippo, K.; Sherrill, M.; Dodd, E.; DeVolder, B.; Cardenas, T.; et al. Replicating the Z iron opacity experiments on the NIF. High Energy Density Phys. 2017, 23, 223–227. [Google Scholar] [CrossRef]
- Moses, E.; Wuest, C. The national ignition facility: Laser performance and first experiments. Fusion Sci. Technol. 2005, 47, 314–322. [Google Scholar] [CrossRef]
- Haynam, C.; Wegner, P.; Auerbach, J.; Bowers, M.; Dixit, S.; Erbert, G.; Heestand, G.; Henesian, M.; Hermann, M.; Jancaitis, K.; et al. National ignition facility laser performance status. Appl. Opt. 2007, 46, 3276–3303. [Google Scholar] [CrossRef] [PubMed]
- Perry, T.; Budil, K.; Cauble, R.; Ward, R.; Bach, D.; Iglesias, C.; Wilson, B.; Nash, J.; Smith, C.; Foster, J.; et al. Quantitative measurement of mid-z opacities. J. Quant. Spectrosc. Radiat. Transf. 1995, 54, 317–324. [Google Scholar] [CrossRef]
- Bailey, J.; Rochau, G.; Mancini, R.; Iglesias, C.; MacFarlane, J.; Golovkin, I.; Blancard, C.; Cosse, P.; Faussurier, G. Experimental investigation of opacity models for stellar interior, inertial fusion, and high energy density plasmas. Phys. Plasmas 2009, 16, 058101. [Google Scholar] [CrossRef]
- Dodd, E.S.; DeVolder, B.G.; Martin, M.E.; Krasheninnikova, N.S.; Tregillis, I.L.; Perry, T.S.; Heeter, R.F.; Opachich, Y.P.; Moore, A.S.; Kline, J.L.; et al. Hohlraum modeling for opacity experiments on the National Ignition Facility. Phys. Plasmas 2018, 25, 063301. [Google Scholar] [CrossRef] [Green Version]
- Cardenas, T.; Schmidt, D.; Dodd, E.; Perry, T.; Capelli, D.; Quintana, J.; Oertel, J.; Peterson, D.; Giraldez, E.; Heeter, R. Design and fabrication of opacity targets for the national ignition facility. Fusion Sci. Technol. 2017, 73, 458–466. [Google Scholar] [CrossRef]
- Opachich, Y.; Heeter, R.; Barrios, M.; Garcia, E.; Craxton, R.; King, J.; Liedahl, A.; McKenty, P.; Schneider, M.; May, M.; et al. Capsule implosions for continuum X-ray backlighting of opacity samples at the national ignition facility. Phys. Plasmas 2017, 24, 063301. [Google Scholar] [CrossRef] [PubMed]
- Ross, P.; Heeter, R.; Ahmed, M.; Dodd, E.; Huffman, E.; Liedahl, D.; King, J.; Opachich, Y.; Schneider, M.; Perry, T. Design of the opacity spectrometer for opacity measurements at the national ignition facility. Rev. Sci. Instrum. 2016, 87, 11D623. [Google Scholar] [CrossRef] [PubMed]
- King, J.A.; Opachich, Y.P.; Huffman, E.J.; Knight, R.; Heeter, R.F.; Ahmed, M.F.; Liedahl, D.A.; Schneider, M.B.; Thompson, N.B.; Johns, H.M.; et al. Implementation of a 1–2 keV Point-Projection X-ray Spectrometer on the National Ignition Facility. Rev. Sci. Instrum. 2018, 89, 10F101. [Google Scholar] [CrossRef]
- Perry, T.; Heeter, R.; Opachich, Y.; King, J.; Dodd, E.; DeVolder, B.; Sherrill, M.; Wilson, B.; Iglesias, C.; Kline, J.; et al. Update on the Iron Opacity Experiments on the NIF. Workshop Astrophys. Opacities 2018, 515, 115–122. [Google Scholar]
- Nagayama, T.; Bailey, J.; Loisel, G.; Hansen, S.; Rochau, G.; Mancini, R.; MacFarlane, J.; Golovkin, I. Control and diagnosis of temperature, density, and uniformity in X-ray heated iron/magnesium samples for opacity measurements. Phys. Plasmas 2014, 21, 056502. [Google Scholar] [CrossRef]
- Meadowcroft, A.L.; Bentley, C.D.; Stott, E.N. Evaluation of the sensitivity and fading characteristics of an image plate system for X-ray diagnostics. Rev. Sci. Instrum. 2008, 79, 113102. [Google Scholar] [CrossRef] [PubMed]
- Haugh, M.; Lee, J.; Romano, E.; Schneider, M.; Bell, P.; Grim, G. Calibrating image plate sensitivity in the 700 to 5000 eV spectral energy range. Proc. SPIE 2013, 8850, 885007. [Google Scholar]
- Lanier, N.; Hamilton, C.; Taccetti, J. A monochromatic x-ray imaging system for characterizing low-density foams. Rev. Sci. Instrum. 2012, 83, 10E521. [Google Scholar] [CrossRef] [PubMed]
- Beeman, B.; Moore, A.; Wargo, A.; Bell, P.; Widmann, W.; Clancy, T.; Barbosa, F.; Prat, B.; Allouche, V.; Koch, J.; et al. Recording system upgrade for the dante X-ray diagnostic on the national ignition facility. Proc. SPIE 2017, 10390, 1039005. [Google Scholar]
- Kline, J.; Widmann, K.; Warrick, A.; Olson, R.; Thomas, C.; Moore, A.; Suter, L.; Landen, O.; Callahan, D.; Azevedo, S.; et al. The first measurements of soft X-ray flux from ignition scale hohlraums at the national ignition facility using dante (invited). Rev. Sci. Instrum. 2010, 81, 10E321. [Google Scholar] [CrossRef] [PubMed]
- Dewald, E.L.; Campbell, K.M.; Turner, R.E.; Holder, J.P.; Landen, O.L.; Glenzer, S.H.; Kauffman, R.L.; Suter, L.J.; Landon, M.; Rhodes, M.; et al. Dante soft X-ray power diagnostic for national ignition facility. Rev. Sci. Instrum. 2004, 75, 3759–3761. [Google Scholar] [CrossRef]
- Kornblum, H.N.; Kauffman, R.L.; Smith, J.A. Measurement of 0.1–3-kev X-rays from laser plasmas. Rev. Sci. Instrum. 1986, 57, 2179–2181. [Google Scholar] [CrossRef]
- Nagayama, T.; Bailey, J.; Loisel, G.; Rochau, G.; MacFarlane, J.; Golovkin, I. Numerical investigations of potential systematic uncertainties in iron opacity measurements at solar interior temperatures. Phys. Rev. E 2017, 95, 063206. [Google Scholar] [CrossRef] [PubMed]
- Williams, G.J.; Maddox, B.R.; Chen, H.; Kojima, S.; Millecchia, M. Calibration and equivalency analysis of image plate scanners. Rev. Sci. Instrum. 2014, 85, 11E604. [Google Scholar] [CrossRef] [PubMed]
- Maddox, B.; Park, H.; Remington, B.; Izumi, N.; Chen, S.; Chen, C.; Kimminau, G.; Ali, Z.; Haugh, M.; Ma, Q. High-energy X-ray backlighter spectrum measurements using calibrated image plates. Rev. Sci. Instrum. 2011, 82, 023111. [Google Scholar] [CrossRef] [PubMed]
- Burkhalter, P.G.; Brown, D.B.; Gersten, M. A high-resolution X-ray diagnostic technique using simultaneous diffraction from several planes of acid phthalate crystals. J. Appl. Phys. 1981, 52, 4379–4386. [Google Scholar] [CrossRef]
- Anderson, S.; Heeter, R.; Booth, R.; Emig, J.; Fulkerson, S.; McCarville, T.; Norman, D.; Young, B. Broadband high-resolution elliptical crystal X-ray spectrometer for high energy density physics experiments. Rev. Sci. Instrum. 2006, 77, 063115. [Google Scholar] [CrossRef]
- Heeter, R.; Anderson, S.; Booth, R.; Brown, G.; Emig, J.; Fulkerson, S.; McCarville, T.; Norman, D.; Schneider, M.; Young, B. Ozspec-2: An improved broadband high-resolution elliptical crystal X-ray spectrometer for high-energy density physics experiments (invited). Rev. Sci. Instrum. 2008, 79, 10E303. [Google Scholar] [CrossRef] [PubMed]
- Loisel, G.P.; Lake, P.W.; Nielsen-Weber, L.B.; Wu, M.; Dunham, G.S.; Bailey, J.E.; Rochau, G.A. A compact multi-crystal-plane broad-band (0.5–17 keV) spectrometer. Rev. Sci. Instrum. 2018, 89, 10F117. [Google Scholar] [CrossRef]

| Source | Measured From | Uncertainty (Pixel-to-Pixel) | Uncertainty (Transmission) |
|---|---|---|---|
| Photon statistics | Photometric calculation | 1% | 0.1–0.3% |
| IP uniformity | Pixel-to-pixel variation at constant hυ | ~3–10% | ~1–4% |
| Digitization noise | Scan to scan variation of spectra | <3% | <1% |
| Source | Quantified by | Est. Uncertainty (Transmission) |
|---|---|---|
| Uncertainty in subtraction of diffuse scattering/fluorescence background | Varying subtraction method within plausible bounds | 0.02 (E > 1.4 keV) to 0.2 (E < 1.2 keV) |
| Alternate crystal planes | Observation of step-ups in data, particularly off-crystal perimeter data Texture from ends of crystal with known defects | 0.1 for E < 1.3 keV |
| Higher-order diffraction for (001) | Approximate calculation using data on RbAP reflectivity, filter transmission and backlighter spectral shape | 0.02 for T = 0.8, 0.08 for T = 0.1 |
| Other error sources | Not yet quantified | Unknown, but expect <0.05 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Heeter, R.; Perry, T.; Johns, H.; Opachich, K.; Ahmed, M.; Emig, J.; Holder, J.; Iglesias, C.; Liedahl, D.; London, R.; et al. Iron X-ray Transmission at Temperature Near 150 eV Using the National Ignition Facility: First Measurements and Paths to Uncertainty Reduction. Atoms 2018, 6, 57. https://doi.org/10.3390/atoms6040057
Heeter R, Perry T, Johns H, Opachich K, Ahmed M, Emig J, Holder J, Iglesias C, Liedahl D, London R, et al. Iron X-ray Transmission at Temperature Near 150 eV Using the National Ignition Facility: First Measurements and Paths to Uncertainty Reduction. Atoms. 2018; 6(4):57. https://doi.org/10.3390/atoms6040057
Chicago/Turabian StyleHeeter, Robert, Ted Perry, Heather Johns, Kathy Opachich, Maryum Ahmed, Jim Emig, Joe Holder, Carlos Iglesias, Duane Liedahl, Richard London, and et al. 2018. "Iron X-ray Transmission at Temperature Near 150 eV Using the National Ignition Facility: First Measurements and Paths to Uncertainty Reduction" Atoms 6, no. 4: 57. https://doi.org/10.3390/atoms6040057
APA StyleHeeter, R., Perry, T., Johns, H., Opachich, K., Ahmed, M., Emig, J., Holder, J., Iglesias, C., Liedahl, D., London, R., Martin, M., Thompson, N., Wilson, B., Archuleta, T., Cardenas, T., Dodd, E., Douglas, M., Flippo, K., Fontes, C., ... Rochau, G. (2018). Iron X-ray Transmission at Temperature Near 150 eV Using the National Ignition Facility: First Measurements and Paths to Uncertainty Reduction. Atoms, 6(4), 57. https://doi.org/10.3390/atoms6040057






