Graphene Nanoplatelet Distribution Governs Thermal Conductivity and Stability of Paraffin-Based PCMs
Abstract
1. Introduction
- (a)
- (b)
- Can store large amounts of energy and thus have a high latent heat (>200 kJ/kg).
- (c)
- Exhibit a stable and well-defined phase transition at the operation temperature, i.e., a congruent phase change with little supercooling.
- (d)
- Have a long lifetime (10 years or >3650 cycles). This means that the material should be thermally and chemically stable during repeated cycling and non-corrosive.
- (e)
- For commercialization, the synthesis/preparation process needs to be industrially scalable, and materials need to be available on a large scale at low cost.
2. Materials and Methods
2.1. Materials
2.2. Preparation of GNP–Paraffin Composites
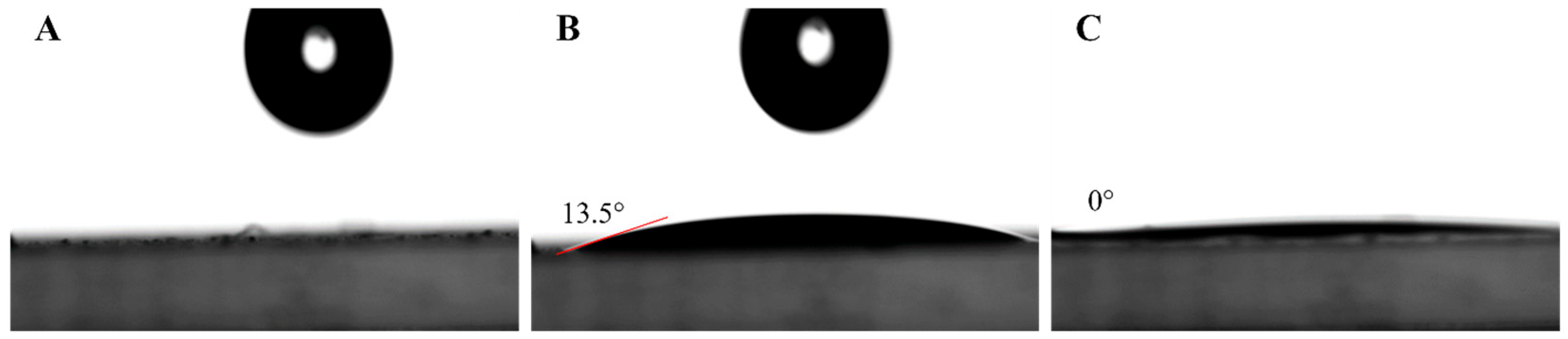
2.3. Paraffin Contact Angle Measurement/Graphene Thin Film Formation
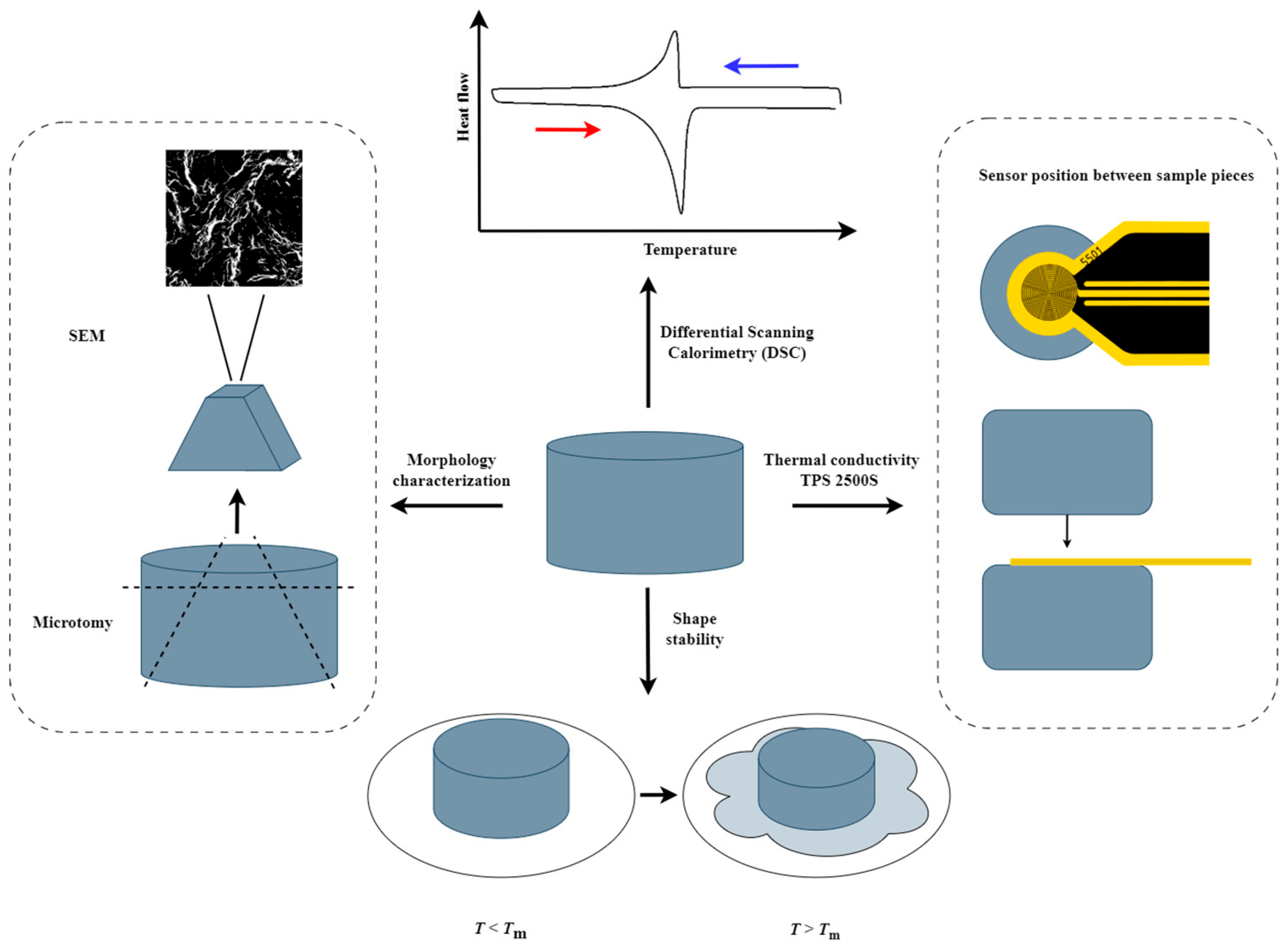
2.4. Morphology Characterization
2.5. Thermal Conductivity Measurements
2.6. Differential Scanning Calorimetry (DSC) Measurements
2.7. Shape Stability
3. Results
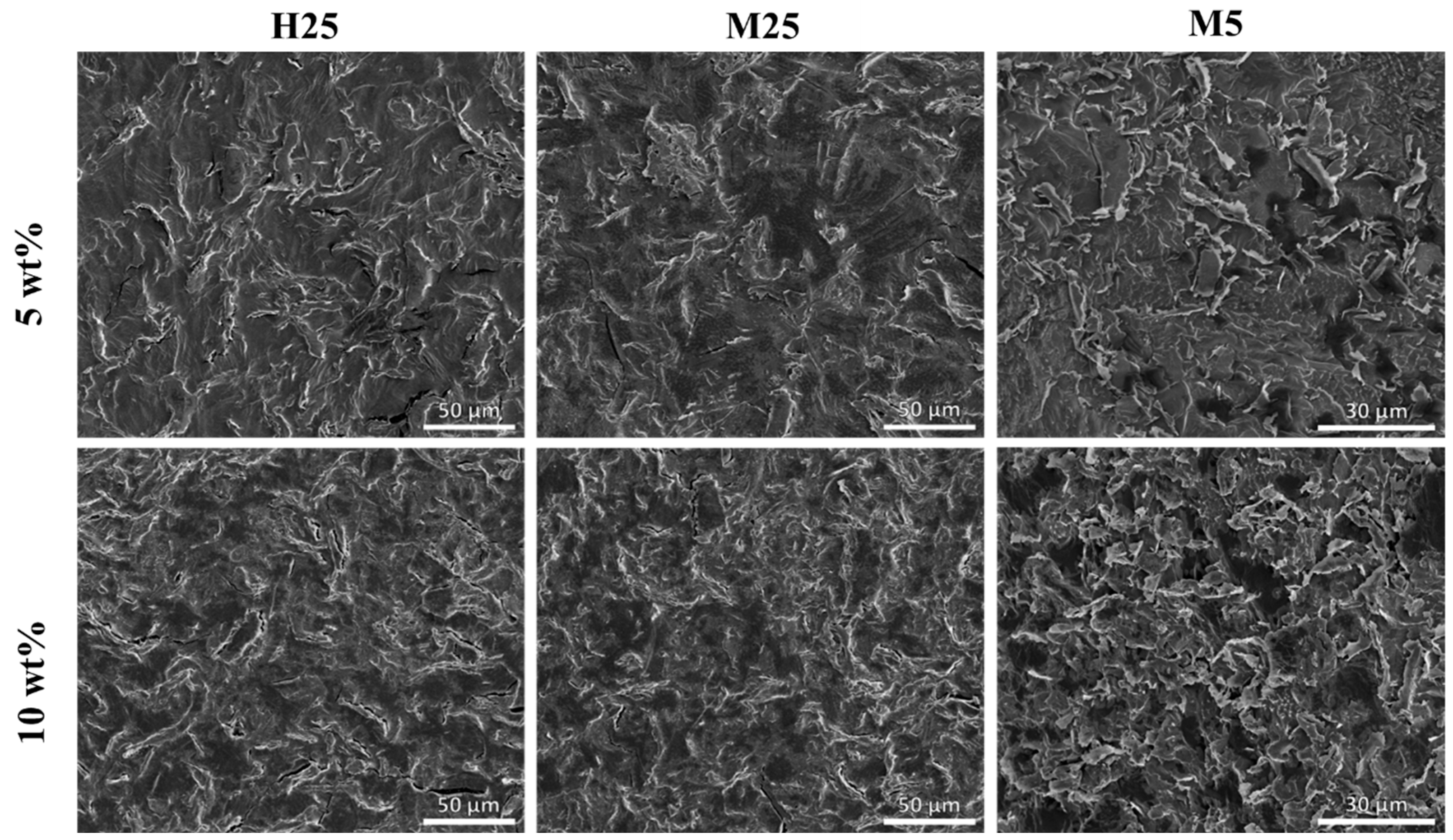
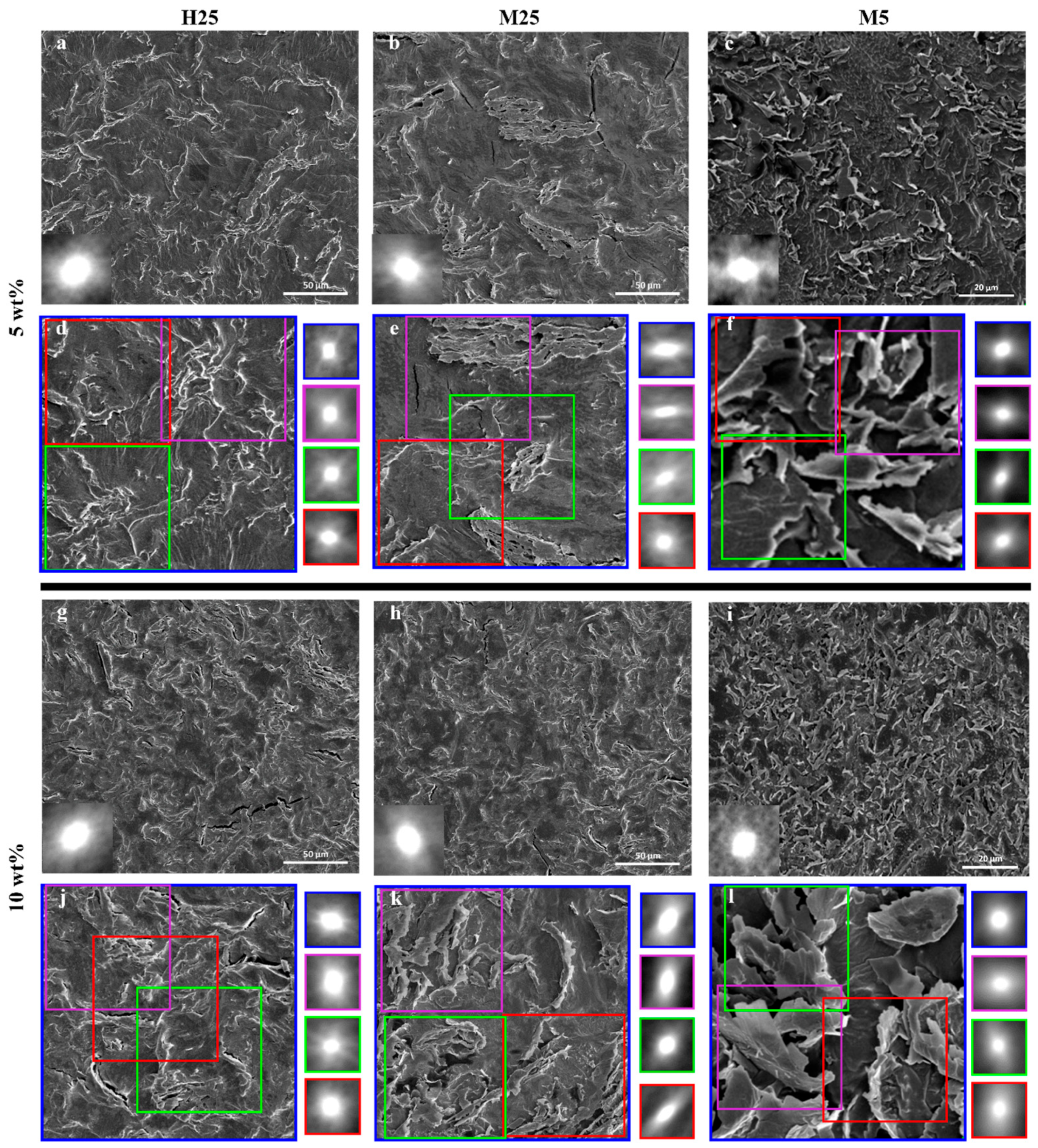
3.1. Effects of Mixing Time on GNP Distribution
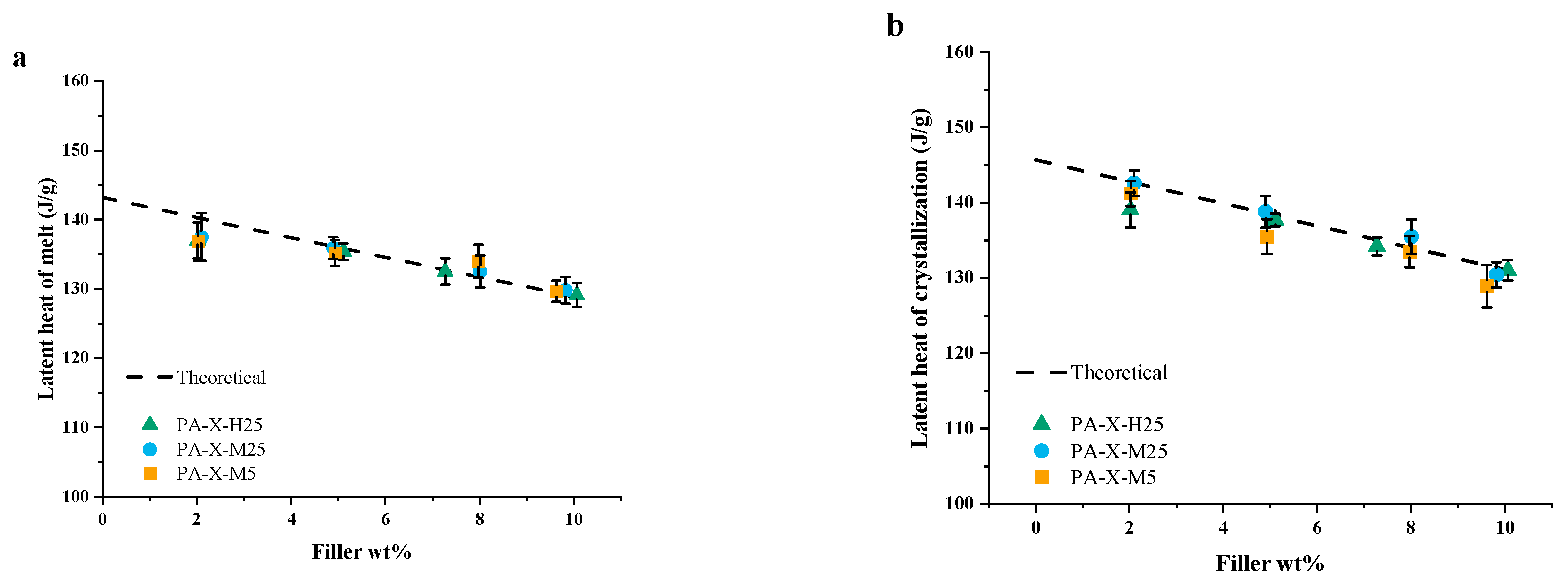
3.2. Melting and Crystallization Temperatures and Latent Heat
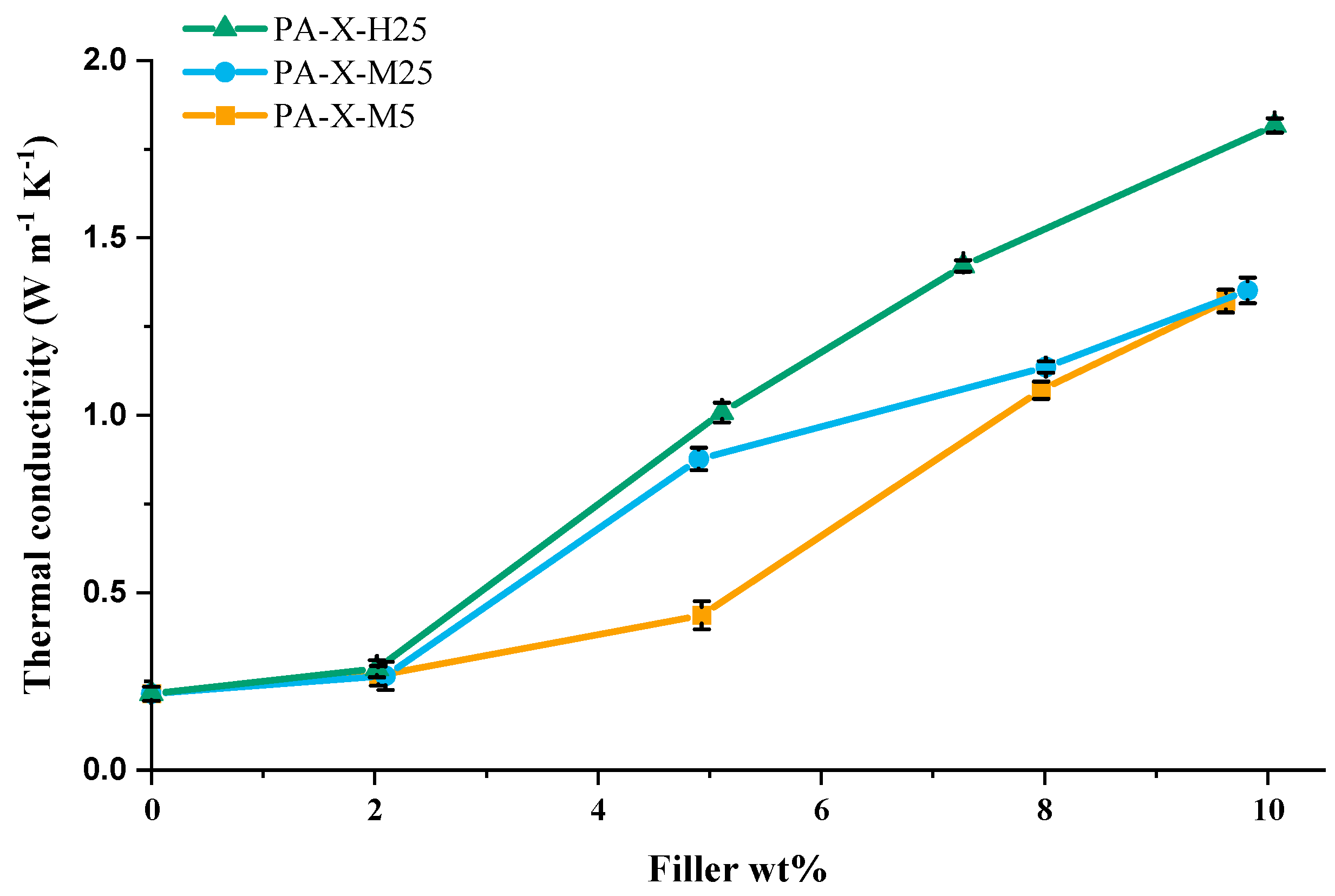
3.3. Effect of Filler Aspect Ratio and Amount on Thermal Conductivity
3.4. Paraffin-GNP Interactions
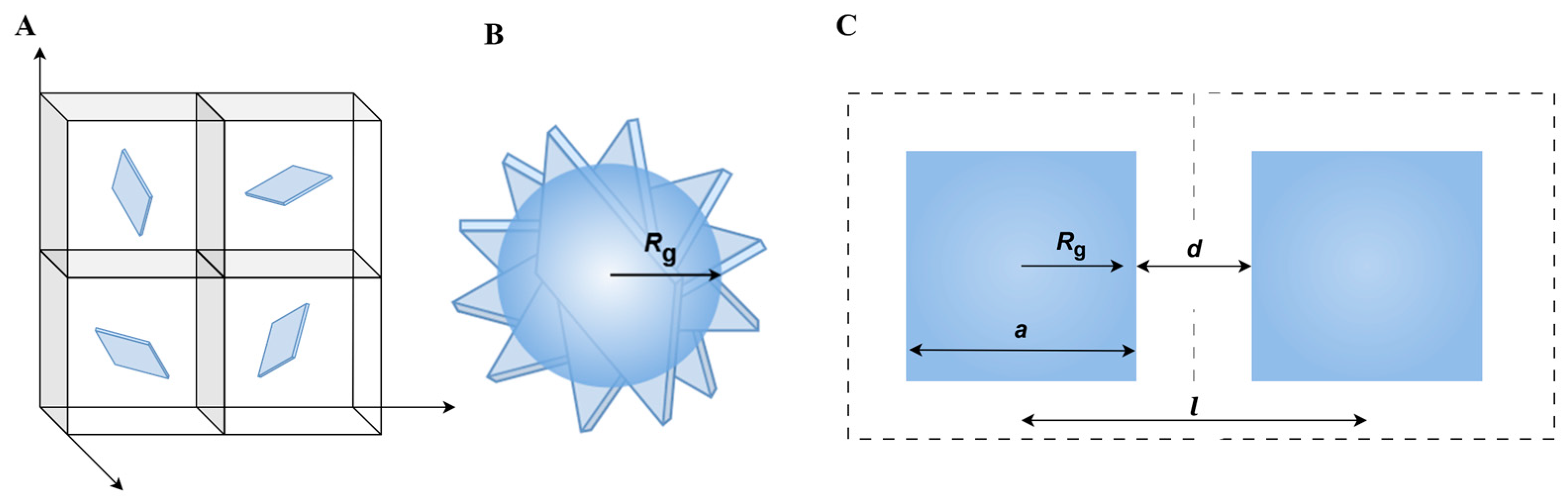
3.5. Percolation Threshold
3.6. Sheet-to-Sheet Distance, Mean Overlap Distance and Network Topology
3.7. What Governs Thermal Conductivity?
4. Conclusions and Outlook
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CNT | Carbon Nanotube |
| DSC | Differential Scanning Calorimetry |
| EG | Expanded Graphite |
| GNP | Graphene Nano Platelet |
| H25 | Graphene nanoplatelet type, 25 µm wide and 15 nm thick |
| LHS | Latent Heat Storage |
| M25 | Graphene nanoplatelet type, 25 µm wide and 7 nm thick |
| M5 | Graphene nanoplatelet type, 5 µm wide and 7 nm thick |
| PA | Paraffin |
| PCC | Phase Change Composite |
| PCM | Phase Change Material |
| SEM | Scanning Electron Microscope |
| SHS | Sensible Heat Storage |
| TES | Thermal Energy Storage |
| THS | Thermal Heat Storage |
| 1 |
References
- Energy Consumption in Households. Available online: https://ec.europa.eu/eurostat/statistics-explained/index.php?title=Energy_consumption_in_households (accessed on 8 April 2023).
- Gasia, J.; Miró, L.; Cabeza, L.F. Review on System and Materials Requirements for High Temperature Thermal Energy Storage. Part 1: General Requirements. Renew. Sustain. Energy Rev. 2017, 75, 1320–1338. [Google Scholar] [CrossRef]
- Delta Energy & Environment Ltd. Evidence Gathering: Thermal Energy Storage (TES) Technologies; Delta Energy & Environment Ltd.: Edinburg, Schotland, 2016. [Google Scholar]
- Rathod, M.K.; Banerjee, J. Thermal Stability of Phase Change Materials Used in Latent Heat Energy Storage Systems: A Review. Renew. Sustain. Energy Rev. 2013, 18, 246–258. [Google Scholar] [CrossRef]
- Hasnain, S.M. Review on Sustainable Thermal Energy Storage Technologies, Part I: Heat Storage Materials and Techniques. Energy Convers. Manag. 1998, 39, 1127–1138. [Google Scholar] [CrossRef]
- Wang, X.; Li, W.; Luo, Z.; Wang, K.; Shah, S.P. A Critical Review on Phase Change Materials (PCM) for Sustainable and Energy Efficient Building: Design, Characteristic, Performance and Application. Energy Build. 2022, 260, 1–25. [Google Scholar] [CrossRef]
- Ben Romdhane, S.; Amamou, A.; Ben Khalifa, R.; Saïd, N.M.; Younsi, Z.; Jemni, A. A Review on Thermal Energy Storage Using Phase Change Materials in Passive Building Applications. J. Build. Eng. 2020, 32, 1–18. [Google Scholar] [CrossRef]
- Junaid, M.F.; Rehman, Z.u.; Čekon, M.; Čurpek, J.; Farooq, R.; Cui, H.; Khan, I. Inorganic Phase Change Materials in Thermal Energy Storage: A Review on Perspectives and Technological Advances in Building Applications. Energy Build. 2021, 252, 111443. [Google Scholar] [CrossRef]
- Reyez-Araiza, J.L.; Pineda-Piñón, J.; López-Romero, J.M.; Gasca-Tirado, J.R.; Contreras, M.A.; Correa, J.C.J.; Apátiga-Castro, L.M.; Rivera-Muñoz, E.M.; Velazquez-Castillo, R.R.; Pérez Bueno, J.D.J.; et al. Thermal Energy Storage by the Encapsulation of Phase Change Materials in Building Elements—A Review. Materials 2021, 14, 1420. [Google Scholar] [CrossRef]
- Joseph, M.; Sajith, V. Graphene Enhanced Paraffin Nanocomposite Based Hybrid Cooling System for Thermal Management of Electronics. Appl. Therm. Eng. 2019, 163, 114342. [Google Scholar] [CrossRef]
- Liu, C.; Xu, D.; Weng, J.; Zhou, S.; Li, W.; Wan, Y.; Jiang, S.; Zhou, D.; Wang, J.; Huang, Q. Phase Change Materials Application in Battery Thermal Management System: A Review. Materials 2020, 13, 4622. [Google Scholar] [CrossRef]
- Tahan Latibari, S.; Sadrameli, S.M. Carbon Based Material Included-Shaped Stabilized Phase Change Materials for Sunlight-Driven Energy Conversion and Storage: An Extensive Review. Sol. Energy 2018, 170, 1130–1161. [Google Scholar] [CrossRef]
- Abhat, A. Low Temperature Latent Heat Thermal Energy Storage: Heat Storage Materials. Sol. Energy 1983, 30, 313–332. [Google Scholar] [CrossRef]
- Lane, G.A. (Ed.) Solar Heat Storage: Volume I: Latent Heat Material; CRC Press: Boca Raton, FL, USA, 1983; ISBN 978-1-351-07675-3. [Google Scholar]
- Dincer, I.; Rosen, M.A. Thermal Energy Storage: Systems and Applications, 2nd ed.; John Wiley & Sons: Toronto, ON, Canada, 2002; ISBN 9780470747063. [Google Scholar]
- Gallo, A.B.; Simões-Moreira, J.R.; Costa, H.K.M.; Santos, M.M.; Moutinho dos Santos, E. Energy Storage in the Energy Transition Context: A Technology Review. Renew. Sustain. Energy Rev. 2016, 65, 800–822. [Google Scholar] [CrossRef]
- Yuan, Y.; Zhang, N.; Li, T.; Cao, X.; Long, W. Thermal Performance Enhancement of Palmitic-Stearic Acid by Adding Graphene Nanoplatelets and Expanded Graphite for Thermal Energy Storage: A Comparative Study. Energy 2016, 97, 488–497. [Google Scholar] [CrossRef]
- Zhang, N.; Yuan, Y.; Du, Y.; Cao, X.; Yuan, Y. Preparation and Properties of Palmitic-Stearic Acid Eutectic Mixture/Expanded Graphite Composite as Phase Change Material for Energy Storage. Energy 2014, 78, 950–956. [Google Scholar] [CrossRef]
- Dai, J.; Ma, F.; Fu, Z.; Li, C.; Jia, M.; Shi, K.; Wen, Y.; Wang, W. Applicability Assessment of Stearic Acid/Palmitic Acid Binary Eutectic Phase Change Material in Cooling Pavement. Renew. Energy 2021, 175, 748–759. [Google Scholar] [CrossRef]
- Cabeza, L.F.; Castell, A.; Barreneche, C.; De Gracia, A.; Fernández, A.I. Materials Used as PCM in Thermal Energy Storage in Buildings: A Review. Renew. Sustain. Energy Rev. 2011, 15, 1675–1695. [Google Scholar] [CrossRef]
- Farid, M.M.; Khudhair, A.M.; Razack, S.A.K.; Al-Hallaj, S. A Review on Phase Change Energy Storage: Materials and Applications. Energy Convers. Manag. 2004, 45, 1597–1615. [Google Scholar] [CrossRef]
- Zalba, B.; Marıín, J.M.; Cabeza, L.F.; Mehling, H. Review on Thermal Energy Storage with Phase Change: Materials, Heat Transfer Analysis and Applications. Appl. Therm. Eng. 2003, 23, 251–283. [Google Scholar] [CrossRef]
- Mehling, H.; Cabeza, L.F. Heat and Cold Storage with PCM: An up to Date Introduction to Basics and Applications, 1st ed.; Mewes, D., Mayinger, F., Eds.; Springer: Berlin, Germany, 2008; ISBN 9783540685562. [Google Scholar]
- Chen, C.; Wang, L.; Huang, Y. Morphology and Thermal Properties of Electrospun Fatty Acids/Polyethylene Terephthalate Composite Fibers as Novel Form-Stable Phase Change Materials. Sol. Energy Mater. Sol. Cells 2008, 92, 1382–1387. [Google Scholar] [CrossRef]
- Liu, H.; Awbi, H.B. Performance of Phase Change Material Boards under Natural Convection. Build. Environ. 2009, 44, 1788–1793. [Google Scholar] [CrossRef]
- Calabrese, L.; Brancato, V.; Palomba, V.; Frazzica, A.; Cabeza, L.F. Magnesium Sulphate-Silicone Foam Composites for Thermochemical Energy Storage: Assessment of Dehydration Behaviour and Mechanical Stability. Sol. Energy Mater. Sol. Cells 2019, 200, 109992. [Google Scholar] [CrossRef]
- Singh, P.; Sharma, R.K.; Ansu, A.K.; Goyal, R.; Sarı, A.; Tyagi, V.V. A Comprehensive Review on Development of Eutectic Organic Phase Change Materials and Their Composites for Low and Medium Range Thermal Energy Storage Applications. Sol. Energy Mater. Sol. Cells 2021, 223, 110955. [Google Scholar] [CrossRef]
- Lan, X.Z.; Tan, Z.C.; Yue, D.T.; Shi, Q.; Yang, C.G. Heat Storage Performance of Disodium Hydrogen Phosphate Dodecahydrate: Prevention of Phase Separation by Thickening and Gelling Methods. Chinese J. Chem. 2007, 25, 921–925. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, Y. Form-Stable Phase Change Material Based on Na2CO3·10H2O-Na2HPO4·12H2O Eutectic Hydrated Salt/Expanded Graphite Oxide Composite: The Influence of Chemical Structures of Expanded Graphite Oxide. Renew. Energy 2018, 115, 734–740. [Google Scholar] [CrossRef]
- Liu, Y.; Yang, Y. Preparation and Thermal Properties of Na2CO3·10H2O-Na2HPO4·12H2O Eutectic Hydrate Salt as a Novel Phase Change Material for Energy Storage. Appl. Therm. Eng. 2017, 112, 606–609. [Google Scholar] [CrossRef]
- Baetens, R.; Jelle, B.P.; Gustavsen, A. Phase Change Materials for Building Applications: A State-of-the-Art Review. Energy Build. 2010, 42, 1361–1368. [Google Scholar] [CrossRef]
- Tebaldi, M.L.; Belardi, R.M.; Montoro, S.R. Polymers with Nano-Encapsulated Functional Polymers: Encapsulated Phase Change Materials. In Encapsulated Phase Change Materials, 1st ed.; Elsevier Inc: Oxford, UK, 2016; ISBN 9780323394543. [Google Scholar]
- Kenisarin, M.M. Thermophysical Properties of Some Organic Phase Change Materials for Latent Heat Storage. A Review. Sol. Energy 2014, 107, 553–575. [Google Scholar] [CrossRef]
- Dirand, M.; Bouroukba, M.; Briard, A.J.; Chevallier, V.; Petitjean, D.; Corriou, J.P. Temperatures and Enthalpies of (Solid + Solid) and (Solid + Liquid) Transitions of n-Alkanes. J. Chem. Thermodyn. 2002, 34, 1255–1277. [Google Scholar] [CrossRef]
- Mandelkern, L.; Prasad, A.; Alamo, R.G.; Stack, G.M. Melting Temperature of the N-Alkanes and the Linear Polyethylenes. Macromolecules 1990, 23, 3696–3700. [Google Scholar] [CrossRef]
- Thomas, N.; Abiramalatha, T.; Bhat, V.; Varanattu, M.; Rao, S.; Wazir, S.; Lewis, L.; Balakrishnan, U.; Murki, S.; Mittal, J.; et al. Phase Changing Material for Therapeutic Hypothermia in Neonates with Hypoxic Ischemic Encephalopathy—A Multi-Centric Study. Indian Pediatr. 2018, 55, 201–205. [Google Scholar] [CrossRef]
- Ma, K.; Zhang, X.; Ji, J.; Han, L.; Ding, X.; Xie, W. Application and Research Progress of Phase Change Materials in Biomedical Field. Biomater. Sci. 2021, 9, 5762–5780. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Gao, H.; Hai, G.; Jia, D.; Xing, L.; Chen, S.; Cheng, P.; Han, M.; Dong, W.; Wang, G. Carbon Nanotube Bundles Assembled Flexible Hierarchical Framework Based Phase Change Material Composites for Thermal Energy Harvesting and Thermotherapy. Energy Storage Mater. 2020, 26, 129–137. [Google Scholar] [CrossRef]
- Xu, W.; Xu, L.; Jia, W.; Mao, X.; Liu, S.; Dong, H.; Zhang, H.; Zhang, Y. Nanomaterials Based on Phase Change Materials for Antibacterial Application. Biomater. Sci. 2022, 10, 6388–6398. [Google Scholar] [CrossRef]
- Zhang, N.; Yuan, Y.; Cao, X.; Du, Y.; Zhang, Z.; Gui, Y. Latent Heat Thermal Energy Storage Systems with Solid-Liquid Phase Change Materials: A Review. Adv. Eng. Mater. 2018, 20, 1–30. [Google Scholar] [CrossRef]
- Xiao, M.; Feng, B.; Gong, K. Preparation and Performance of Shape Stabilized Phase Change Thermal Storage Materials with High Thermal Conductivity. Energy Convers. Manag. 2002, 43, 103–108. [Google Scholar] [CrossRef]
- Ai, D.; Su, L.; Gao, Z.; Deng, C.; Dai, X. Study of ZrO2 Nanopowders Based Stearic Acid Phase Change Materials. Particuology 2010, 8, 394–397. [Google Scholar] [CrossRef]
- Zeng, J.L.; Cao, Z.; Yang, D.W.; Sun, L.X.; Zhang, L. Thermal Conductivity Enhancement of Ag Nanowires on an Organic Phase Change Material. J. Therm. Anal. Calorim. 2010, 101, 385–389. [Google Scholar] [CrossRef]
- Wu, Z.G.; Zhao, C.Y. Experimental Investigations of Porous Materials in High Temperature Thermal Energy Storage Systems. Sol. Energy 2011, 85, 1371–1380. [Google Scholar] [CrossRef]
- Mehrali, M.; Latibari, S.T.; Mehrali, M.; Metselaar, H.S.C.; Silakhori, M. Shape-Stabilized Phase Change Materials with High Thermal Conductivity Based on Paraffin/Graphene Oxide Composite. Energy Convers. Manag. 2013, 67, 275–282. [Google Scholar] [CrossRef]
- Allahbakhsh, A.; Arjmand, M. Graphene-Based Phase Change Composites for Energy Harvesting and Storage: State of the Art and Future Prospects. Carbon 2019, 148, 441–480. [Google Scholar] [CrossRef]
- Wang, J.; Xie, H.; Xin, Z. Thermal Properties of Paraffin Based Composites Containing Multi-Walled Carbon Nanotubes. Thermochim. Acta 2009, 488, 39–42. [Google Scholar] [CrossRef]
- Huang, X.; Zhi, C.; Lin, Y.; Bao, H.; Wu, G.; Jiang, P.; Mai, Y.W. Thermal Conductivity of Graphene-Based Polymer Nanocomposites. Mater. Sci. Eng. R 2020, 142, 100577. [Google Scholar] [CrossRef]
- Balandin, A.A. Thermal Properties of Graphene and Nanostructured Carbon Materials. Nat. Mater. 2011, 10, 569–581. [Google Scholar] [CrossRef] [PubMed]
- Ghosh, S.; Bao, W.; Nika, D.L.; Subrina, S.; Pokatilov, E.P.; Lau, C.N.; Balandin, A.A. Dimensional Crossover of Thermal Transport in Few-Layer Graphene. Nat. Mater. 2010, 9, 555–558. [Google Scholar] [CrossRef]
- Heremans, J.; Beetz, C.P., Jr. Thermal Conductivity and Thermopower of Vapor-Grown Graphite Fibers. Phys. Rev. B 1985, 32, 207–219. [Google Scholar] [CrossRef]
- Balandin, A.A.; Ghosh, S.; Bao, W.; Calizo, I.; Teweldebrhan, D.; Miao, F.; Lau, C.N. Superior Thermal Conductivity of Single-Layer Graphene. Nano Lett. 2008, 8, 902–907. [Google Scholar] [CrossRef]
- Warzoha, R.J.; Fleischer, A.S. Heat Flow at Nanoparticle Interfaces. Nano Energy 2014, 6, 137–158. [Google Scholar] [CrossRef]
- Pop, E.; Varshney, V.; Roy, A.K. Thermal Properties of Graphene: Fundamentals and Applications. MRS Bull. 2012, 37, 1273–1281. [Google Scholar] [CrossRef]
- Kargar, F.; Barani, Z.; Salgado, R.; Debnath, B.; Lewis, J.S.; Aytan, E.; Lake, R.K.; Balandin, A.A. Thermal Percolation Threshold and Thermal Properties of Composites with High Loading of Graphene and Boron Nitride Fillers. ACS Appl. Mater. Interfaces 2018, 10, 37555–37565. [Google Scholar] [CrossRef]
- Shtein, M.; Nadiv, R.; Buzaglo, M.; Kahil, K.; Regev, O. Thermally Conductive Graphene-Polymer Composites: Size, Percolation, and Synergy Effects. Chem. Mater. 2015, 27, 2100–2106. [Google Scholar] [CrossRef]
- Gu, J.; Xie, C.; Li, H.; Dang, J.; Geng, W.; Zhang, Q. Thermal Percolation Behaviour Fo Graphene Nanoplatelets/Polyphenylene Sulfide Thermal Conductivity Composites. Polym. Polym. Compos. 2014, 35, 1087–1092. [Google Scholar] [CrossRef]
- Halvaee, M.; Didehban, K.; Goodarzi, V.; Ghaffari, M.; Ehsani, M.; Saeb, M.R. Comparison of Pristine and Polyaniline-Grafted MWCNTs as Conductive Sensor Elements for Phase Change Materials: Thermal Conductivity Trend Analysis. J. Appl. Polym. Sci. 2017, 134, 1–10. [Google Scholar] [CrossRef]
- Li, Y.; Sun, K.; Kou, Y.; Liu, H.; Wang, L.; Yin, N.; Dong, H.; Shi, Q. One-Step Synthesis of Graphene-Based Composite Phase Change Materials with High Solar-Thermal Conversion Efficiency. Chem. Eng. J. 2022, 429, 1–9. [Google Scholar] [CrossRef]
- Sari, A.; Karaipekli, A. Thermal Conductivity and Latent Heat Thermal Energy Storage Characteristics of Paraffin/Expanded Graphite Composite as Phase Change Material. Appl. Therm. Eng. 2007, 27, 1271–1277. [Google Scholar] [CrossRef]
- Shi, J.-N.; Ger, M.-D.; Liu, Y.-M.; Fan, Y.-C.; Wen, N.-T.; Lin, C.-K.; Pu, N.-W. Improving the Thermal Conductivity and Shape-Stabilization of Phase Change Materials Using Nanographite Additives. Carbon 2013, 51, 365–372. [Google Scholar] [CrossRef]
- Tang, Y.; Alva, G.; Huang, X.; Su, D.; Liu, L.; Fang, G. Thermal Properties and Morphologies of MA-SA Eutectics/CNTs as Composite PCMs in Thermal Energy Storage. Energy Build. 2016, 127, 603–610. [Google Scholar] [CrossRef]
- Burger, N.; Laachachi, A.; Ferriol, M.; Lutz, M.; Toniazzo, V.; Ruch, D. Review of Thermal Conductivity in Composites: Mechanisms, Parameters and Theory. Prog. Polym. Sci. 2016, 61, 1–28. [Google Scholar] [CrossRef]
- Marconnet, A.M.; Yamamoto, N.; Panzer, M.A.; Wardle, B.L.; Goodson, K.E. Thermal Conduction in Aligned Carbon Nanotube-Polymer Nanocomposites with High Packing Density. ACS Nano 2011, 5, 4818–4825. [Google Scholar] [CrossRef]
- Huxtable, S.T.; Cahill, D.G.; Shenogin, S.; Xue, L.; Ozisik, R.; Barone, P.; Usrey, M.; Strano, M.S.; Siddons, G.; Shim, M.; et al. Interfacial Heat Flow in Carbon Nanotube Suspensions. Nat. Mater. 2003, 2, 731–734. [Google Scholar] [CrossRef]
- Paton, K.R.; Varrla, E.; Backes, C.; Smith, R.J.; Khan, U.; O’Neill, A.; Boland, C.; Lotya, M.; Istrate, O.M.; King, P.; et al. Scalable Production of Large Quantities of Defect-Free Few-Layer Graphene by Shear Exfoliation in Liquids. Nat. Mater. 2014, 13, 624–630. [Google Scholar] [CrossRef]
- Arapov, K.; Goryachev, A.; De With, G.; Friedrich, H. A Simple and Flexible Route to Large-Area Conductive Transparent Graphene Thin-Films. Synth. Met. 2015, 201, 67–75. [Google Scholar] [CrossRef]
- Müller, L.; Rubio-Pérez, G.; Bach, A.; Muñoz-Rujas, N.; Aguilar, F.; Worlitschek, J. Consistent DSC and TGA Methodology as Basis for the Measurement and Comparison of Thermo-Physical Properties of Phase Change Materials. Materials 2020, 13, 4486. [Google Scholar] [CrossRef] [PubMed]
- Ren, X.; Shen, H.; Yang, Y.; Yang, J. Study on the Properties of a Novel Shape-Stable Epoxy Resin Sealed Expanded Graphite/Paraffin Composite PCM and Its Application in Buildings. Phase Transitions 2019, 92, 581–594. [Google Scholar] [CrossRef]
- Su, W.; Li, Y.; Zhou, T.; Darkwa, J.; Kokogiannakis, G.; Li, Z. Microencapsulation of Paraffin with Poly (Urea Methacrylate) Shell for Solar Water Heater. Energies 2019, 12, 3406. [Google Scholar] [CrossRef]
- Warzoha, R.J.; Fleischer, A.S. Effect of Graphene Layer Thickness and Mechanical Compliance on Interfacial Heat Flow and Thermal Conduction in Solid-Liquid Phase Change Materials. ACS Appl. Mater. Interfaces 2014, 6, 12868–12876. [Google Scholar] [CrossRef]
- Temirel, M.; Hu, H.; Shabgard, H.; Boettcher, P.; McCarthy, M.; Sun, Y. Solidification of Additive-Enhanced Phase Change Materials in Spherical Enclosures with Convective Cooling. Appl. Therm. Eng. 2017, 111, 134–142. [Google Scholar] [CrossRef]
- Jeong, S.-G.; Jeon, J.; Chung, O.; Kim, S.; Kim, S. Evaluation of PCM/Diatomite Composites Using Exfoliated Graphite Nanoplatelets (XGnP) to Improve Thermal Properties. J. Therm. Anal. Calorim. 2013, 114, 689–698. [Google Scholar] [CrossRef]
- van Hazendonk, L.S.; Vonk, C.F.; van Grondelle, W.; Vonk, N.H.; Friedrich, H. Towards a Predictive Understanding of Direct Ink Writing of Graphene-Based Inks. Appl. Mater. Today 2024, 36, 102014. [Google Scholar] [CrossRef]
- Boomstra, M.; Geurts, B.; Lyulin, A. Molecular Weight Segregation and Thermal Conductivity of Polydisperse Wax–Graphene Nanocomposites. Polymers 2023, 15, 2175. [Google Scholar] [CrossRef]
- Paksoy, H.; Sahan, N. Thermally Enhanced Paraffin for Solar Applications. Energy Procedia 2012, 30, 350–352. [Google Scholar] [CrossRef]
- Onsager, L. The Effects of Shape on the Interaction of Colloidal Particles. Ann. N. Y. Acad. Sci. 1949, 51, 627–659. [Google Scholar] [CrossRef]
- Wensink, H.H.; Lekkerkerker, H.N.W. Phase Diagram of Hard Colloidal Platelets: A Theoretical Account. Mol. Phys. 2009, 107, 2111–2118. [Google Scholar] [CrossRef]



| Inorganic Material | Binary Material | Organic Material | |
|---|---|---|---|
| Examples | Salt hydrates (Na2SO4 10H2O, C2H3NaO2) Metals (Gallium, Bi49In21Pb18Sn12) | Inorganic–inorganic (NH2CONH2 +NH4NO3) Inorganic–organic (Mg(NO3)2 •6H2O + glutaric acid) Organic–organic (Palmitic acid–Stearic acid) | Paraffin Fatty acids Alcohol Ester Polyethylene glycol |
| Advantages | Non-flammable High heat of fusion (100–200 kJ/kg) Good thermal conductivity (0.5–1.5 W/mK) | Wide range of phase change temperatures Chemically and thermally stable High heat of fusion (100–230 kJ/kg) Little supercooling | Non-corrosive Chemically and thermally stable Little supercooling High heat of fusion (130–260 kJ/kg) Non-toxic |
| Disadvantages | Corrosive High supercooling Metastability (hysteresis) Thermal instability High density | Low thermal conductivity Expensive Limited data available on thermophysical properties | Low thermal conductivity Flammable Relatively large volume change (up to 15 %) |
| Graphene Source | Lateral Size/Diameter (μm) | Thickness (nm) | Surface Area (m2/g) | Aspect Ratio | Thermal Conductivity (W·m−1·K−1) |
|---|---|---|---|---|---|
| M5 | 5 | 7 | 150 | 625 | 3000 |
| H25 | 25 | 15 | 80 | 1667 | 3000 |
| M25 | 25 | 7 | 150 | 3125 | 3000 |
| Wt% | M5 | H25 | M25 |
|---|---|---|---|
| 2 | 89 | 38 | 18 |
| 5 | 43 | 12 | 0 |
| 8 | 12 | 0 | 0 |
| 10 | 0 | 0 | 0 |
| Filler | Theoretical | Experimental (2 wt%) | Calculated GNP Thickness (nm) | a Experimental | a Calculated GNP Thickness (nm) |
|---|---|---|---|---|---|
| H25 | 0.0018 | 0.009 | 72 | 0.025 | 199 |
| M25 | 0.00088 | 0.009 | 72 | 0.009 | 72 |
| M5 | 0.0044 | 0.009 | 14 | 0.009 | 72 |
| Filler wt% | Theoretical Sheet-to-Sheet Distance (µm) | Measured Sheet-to-Sheet Distance (µm) ± Stdev (µm) | Experimental Overlap Distance (µm) |
|---|---|---|---|
| PA-2-H25 | 10.901 | ||
| PA-5-H25 | 7.954 | 15.70 ± 14.69 | 1.27 |
| PA-8-H25 | 7.035 | ||
| PA-10-H25 | 6.274 | 12.48 ± 8.61 | −1.95 |
| PA-2-M25 | 8.354 | ||
| PA-5-M25 | 6.256 | 14.11 ± 12.95 | −0.32 |
| PA-8-M25 | 5.274 | ||
| PA-10-M25 | 4.909 | 11.48 ± 9.25 | −2.95 |
| PA-2-M5 | 2.888 | ||
| PA-5-M5 | 2.136 | 2.91 ± 0.98 | 0.02 |
| PA-8-M5 | 1.807 | ||
| PA-10-M5 | 1.691 | 1.66 ± 0.71 | −1.23 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wijkhuijs, L.E.A.; Schmit, P.; Schreur-Piet, I.; Huinink, H.; Tuinier, R.; Friedrich, H. Graphene Nanoplatelet Distribution Governs Thermal Conductivity and Stability of Paraffin-Based PCMs. Nanomaterials 2025, 15, 587. https://doi.org/10.3390/nano15080587
Wijkhuijs LEA, Schmit P, Schreur-Piet I, Huinink H, Tuinier R, Friedrich H. Graphene Nanoplatelet Distribution Governs Thermal Conductivity and Stability of Paraffin-Based PCMs. Nanomaterials. 2025; 15(8):587. https://doi.org/10.3390/nano15080587
Chicago/Turabian StyleWijkhuijs, Levina E. A., Pauline Schmit, Ingeborg Schreur-Piet, Henk Huinink, Remco Tuinier, and Heiner Friedrich. 2025. "Graphene Nanoplatelet Distribution Governs Thermal Conductivity and Stability of Paraffin-Based PCMs" Nanomaterials 15, no. 8: 587. https://doi.org/10.3390/nano15080587
APA StyleWijkhuijs, L. E. A., Schmit, P., Schreur-Piet, I., Huinink, H., Tuinier, R., & Friedrich, H. (2025). Graphene Nanoplatelet Distribution Governs Thermal Conductivity and Stability of Paraffin-Based PCMs. Nanomaterials, 15(8), 587. https://doi.org/10.3390/nano15080587









