Dielectric Metalens for Superoscillatory Focusing Based on High-Order Angular Bessel Function
Abstract
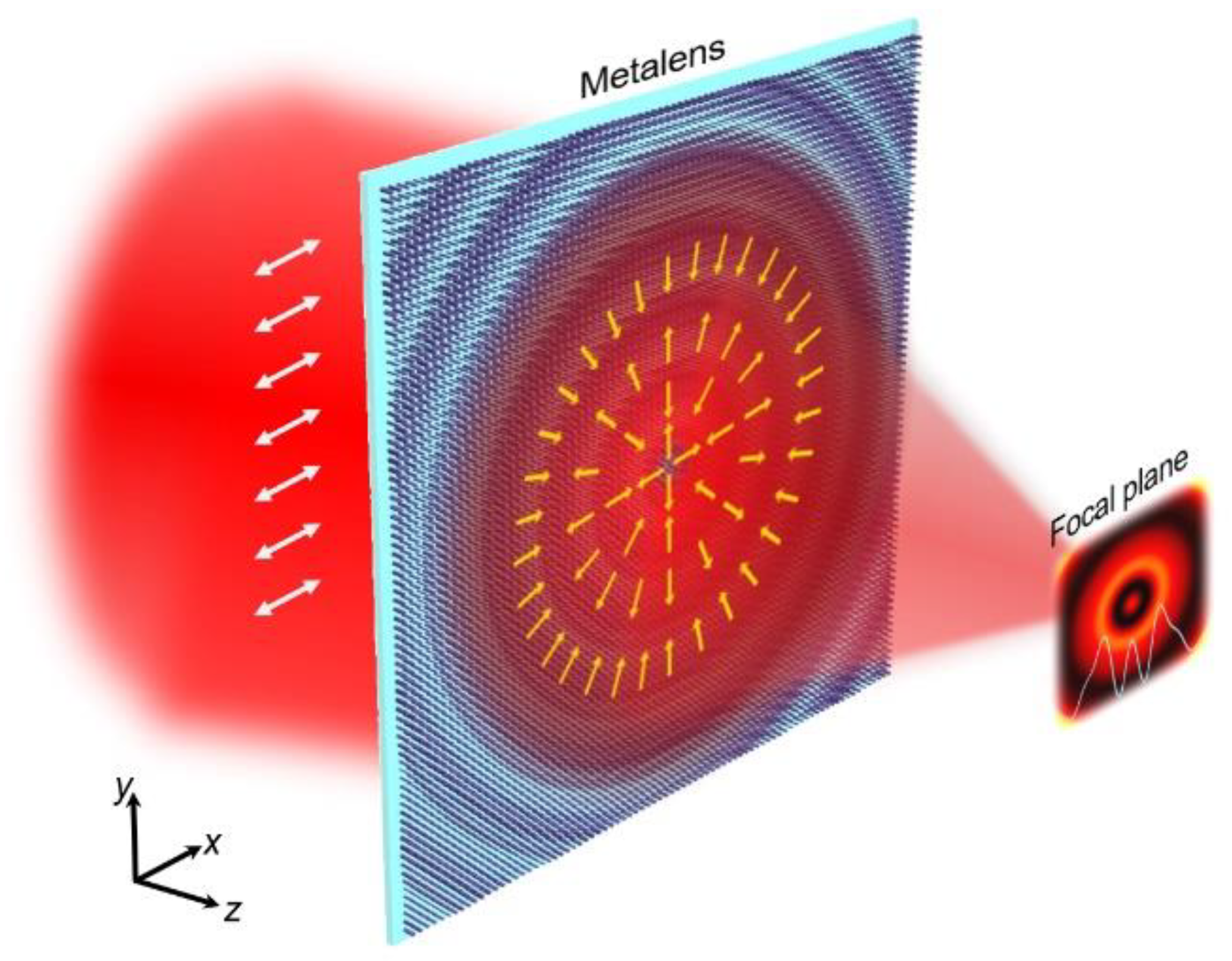
1. Introduction
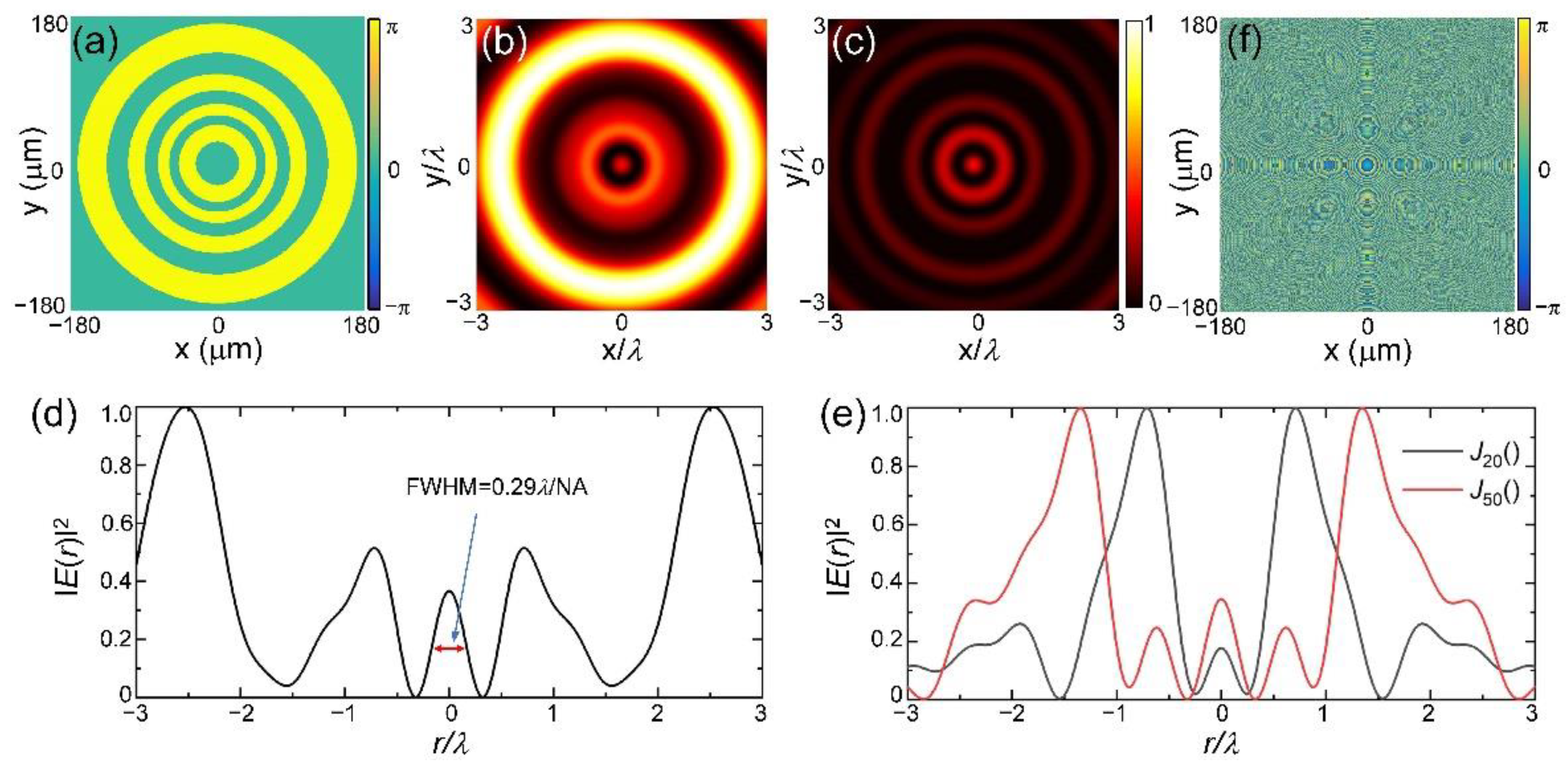
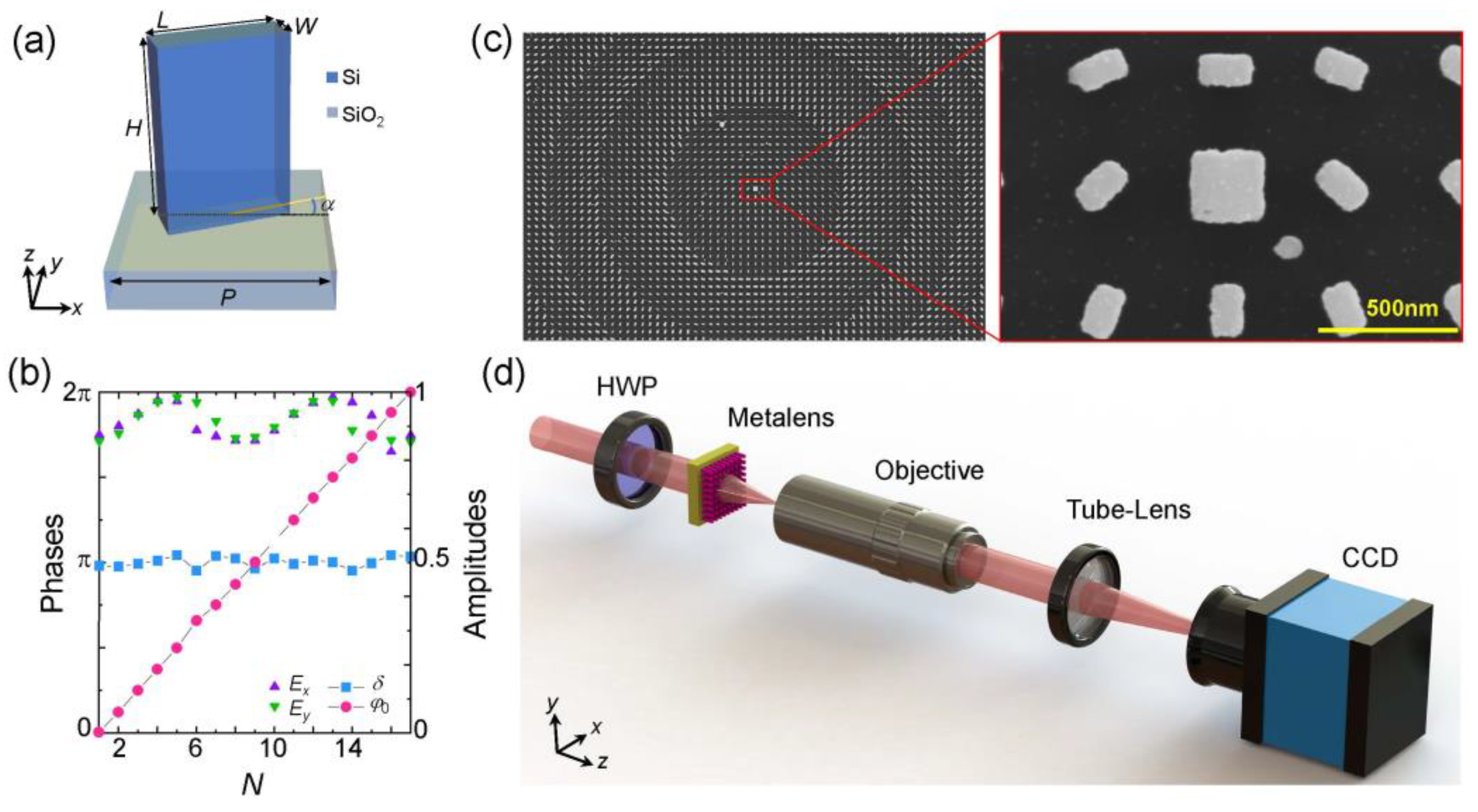
2. Theory and Method
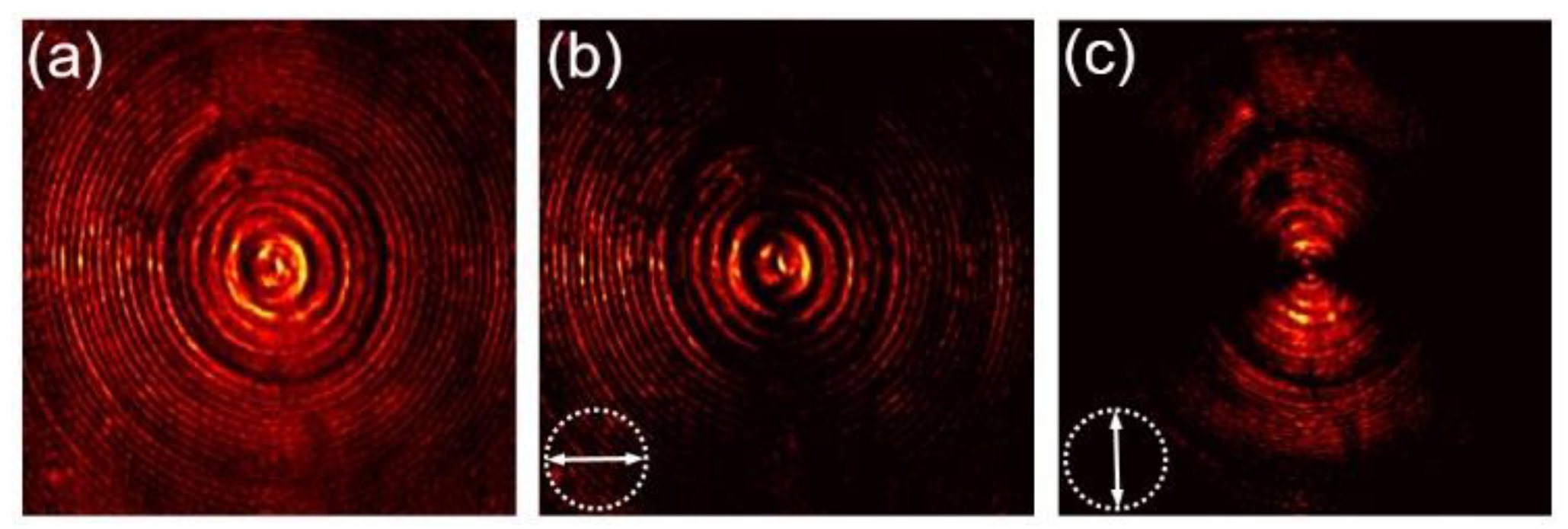
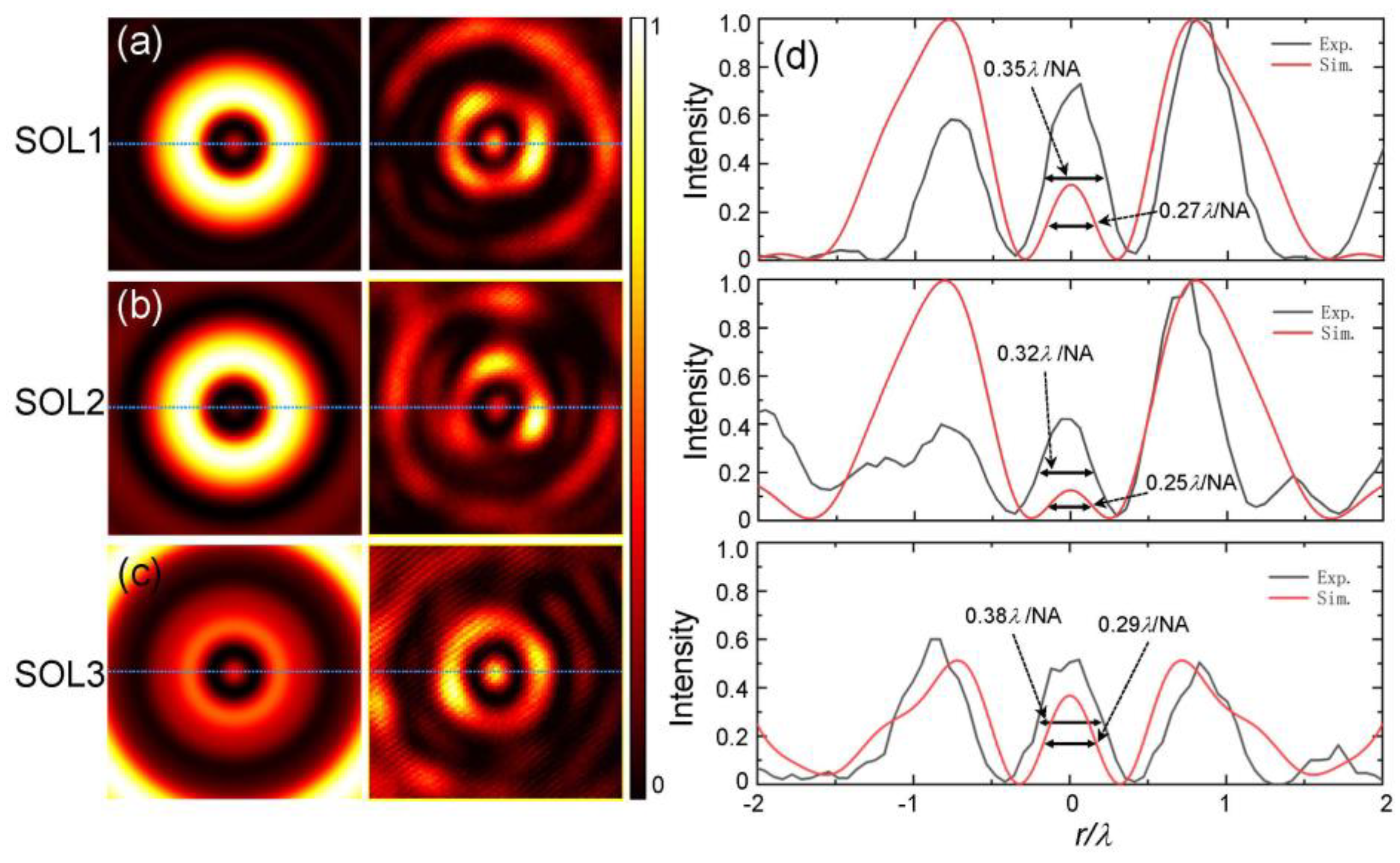
3. Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Abbe, E. Beiträge zur Theorie des Mikroskops und der Mikroskopischen Wahrnehmung. Arch. Für Mikrosk. Anat. 1873, 9, 413–468. [Google Scholar] [CrossRef]
- Buratto, S.K. Near-field scanning optical microscopy. Curr. Opin. Solid State Mater. Sci. 1996, 1, 485–492. [Google Scholar] [CrossRef]
- Fang, N.; Lee, H.; Sun, C.; Zhang, X. Sub-Diffraction-Limited Optical Imaging with a Silver Superlens. Science 2005, 308, 534–537. [Google Scholar] [CrossRef] [PubMed]
- Rust, M.J.; Bates, M.; Zhuang, X. Sub-diffraction-limit imaging by stochastic optical reconstruction microscopy (STORM). Nat. Methods 2006, 3, 793–796. [Google Scholar] [CrossRef]
- Taubner, T.; Korobkin, D.; Urzhumov, Y.; Shvets, G.; Hillenbrand, R. Near-Field Microscopy Through a SiC Superlens. Science 2006, 313, 1595. [Google Scholar] [CrossRef]
- Renz, M. Fluorescence microscopy-A historical and technical perspective. Cytom. Part A 2013, 83, 767–779. [Google Scholar] [CrossRef]
- Luk’Yanchuk, B.S.; Bekirov, A.R.; Wang, Z.B.; Minin, I.V.; Fedyanin, A.A. Optical Phenomena in Dielectric Spheres Several Light Wavelengths in Size: A Review. Phys. Wave Phenom. 2022, 30, 217–241. [Google Scholar] [CrossRef]
- Minin, I.V.; Minin, O.V. Control of focusing properties of diffraction elements. Sov. J. Quantum Electron. 1990, 20, 198–199. [Google Scholar] [CrossRef]
- Hell, S.W. Far-Field Optical Nanoscopy. Science 2007, 316, 1153–1158. [Google Scholar] [CrossRef]
- Berry, M.V.; Popescu, S. Evolution of quantum superoscillations and optical superresolution without evanescent waves. J. Phys. A Math. Gen. 2006, 39, 6965–6977. [Google Scholar] [CrossRef]
- Berry, M.; Zheludev, N.; Aharonov, Y.; Colombo, F.; Sabadini, I.; Struppa, D.C.; Tollaksen, J.; Rogers, E.T.F.; Qin, F.; Hong, M.; et al. Roadmap on superoscillations. J. Opt. 2019, 21, 053002. [Google Scholar] [CrossRef]
- Chen, G.; Wen, Z.-Q.; Qiu, C.-W. Superoscillation: From physics to optical applications. Light Sci. Appl. 2019, 8, 56. [Google Scholar] [CrossRef]
- Zheludev, N.I.; Yuan, G. Optical superoscillation technologies beyond the diffraction limit. Nat. Rev. Phys. 2022, 4, 16–32. [Google Scholar] [CrossRef]
- Huang, F.M.; Zheludev, N.I. Super-Resolution without Evanescent Waves. Nano Lett. 2009, 9, 1249–1254. [Google Scholar] [CrossRef] [PubMed]
- Ayas, S.; Cinar, G.; Ozkan, A.D.; Soran, Z.; Ekiz, O.; Kocaay, D.; Tomak, A.; Toren, P.; Kaya, Y.; Tunc, I.; et al. Label-Free Nanometer-Resolution Imaging of Biological Architectures through Surface Enhanced Raman Scattering. Sci. Rep. 2013, 3, 2624. [Google Scholar] [CrossRef] [PubMed]
- Qin, F.; Huang, K.; Wu, J.; Teng, J.; Qiu, C.-W.; Hong, M. A Supercritical Lens Optical Label-Free Microscopy: Sub-Diffraction Resolution and Ultra-Long Working Distance. Adv. Mater. 2017, 29, 1602721. [Google Scholar] [CrossRef]
- Huang, F.M.; Zheludev, N.; Chen, Y.; de Abajo, F.J.G. Focusing of light by a nanohole array. Appl. Phys. Lett. 2007, 90, 091119. [Google Scholar] [CrossRef]
- Wang, X.; Wang, T.; Kuang, C.; Hao, X.; Liu, X. Experimental verification of the far-field subwavelength focusing with multiple concentric nanorings. Appl. Phys. Lett. 2010, 97, 231105. [Google Scholar] [CrossRef]
- Rogers, E.; Lindberg, J.; Roy, T.; Savo, S.; Chad, J.; Dennis, M.R.; Zheludev, N. A super-oscillatory lens optical microscope for subwavelength imaging. Nat. Mater. 2012, 11, 432–435. [Google Scholar] [CrossRef]
- Liu, T.; Tan, J.; Liu, J.; Wang, H. Vectorial design of super-oscillatory lens. Opt. Express 2013, 21, 15090–15101. [Google Scholar] [CrossRef]
- Jiang, Y.; Li, X.; Gu, M. Generation of sub-diffraction-limited pure longitudinal magnetization by the inverse Faraday effect by tightly focusing an azimuthally polarized vortex beam. Opt. Lett. 2013, 38, 2957–2960. [Google Scholar] [CrossRef] [PubMed]
- Rogers, E.T.F.; Savo, S.; Lindberg, J.; Roy, T.; Dennis, M.R.; Zheludev, N.I. Super-oscillatory optical needle. Appl. Phys. Lett. 2013, 102, 031108. [Google Scholar] [CrossRef]
- Rogers, E.; Zheludev, N. Optical super-oscillations: Sub-wavelength light focusing and super-resolution imaging. J. Opt. 2013, 15, 094008. [Google Scholar] [CrossRef]
- Yu, Y.; Li, W.; Li, H.; Li, M.; Yuan, W. An Investigation of Influencing Factors on Practical Sub-Diffraction-Limit Focusing of Planar Super-Oscillation Lenses. Nanomaterials 2018, 8, 185. [Google Scholar] [CrossRef]
- Chen, Z.; Zhang, Y.; Xiao, M. Design of a superoscillatory lens for a polarized beam. J. Opt. Soc. Am. B 2015, 32, 1731–1735. [Google Scholar] [CrossRef]
- Yu, N.; Genevet, P.; Kats, M.A.; Aieta, F.; Tetienne, J.-P.; Capasso, F.; Gaburro, Z. Light Propagation with Phase Discontinuities: Generalized Laws of Reflection and Refraction. Science 2011, 334, 333–337. [Google Scholar] [CrossRef]
- Arbabi, E.; Arbabi, A.; Kamali, S.M.; Horie, Y.; Faraon, A. Controlling the sign of chromatic dispersion in diffractive optics with dielectric metasurfaces. Optica 2017, 4, 625–632. [Google Scholar] [CrossRef]
- Tang, D.; Wang, C.; Zhao, Z.; Wang, Y.; Pu, M.; Li, X.; Gao, P.; Luo, X. Ultrabroadband superoscillatory lens composed by plasmonic metasurfaces for subdiffraction light focusing. Laser Photonics Rev. 2015, 9, 713–719. [Google Scholar] [CrossRef]
- Chen, G.; Li, Y.; Wang, X.; Wen, Z.; Lin, F.; Dai, L.; Chen, L.; He, Y.; Liu, S. Super-Oscillation Far-Field Focusing Lens Based on Ultra-Thin Width-Varied Metallic Slit Array. IEEE Photonics Technol. Lett. 2016, 28, 335–338. [Google Scholar] [CrossRef]
- Yuan, G.H.; Rogers, E.T.; Zheludev, N.I. Achromatic super-oscillatory lenses with sub-wavelength focusing. Light Sci. Appl. 2017, 6, e17036. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, T.; Wang, Y.; Kong, W.; Zhang, J.; Huang, Y.; Wang, C.; Li, X.; Pu, M.; Luo, X. Achromatic Broadband Super-Resolution Imaging by Super-Oscillatory Metasurface. Laser Photonics Rev. 2018, 12, 1800064. [Google Scholar] [CrossRef]
- Qin, F.; Liu, B.; Zhu, L.; Lei, J.; Fang, W.; Hu, D.; Zhu, Y.; Ma, W.; Wang, B.; Shi, T.; et al. π-phase modulated monolayer supercritical lens. Nat. Commun. 2021, 12, 32. [Google Scholar] [CrossRef]
- Li, Y.; Fan, X.; Li, P.; Zhao, J. Subdiffraction Focusing Metalens Based on the Depletion of Bessel Beams. IEEE Photonics J. 2022, 14, 1–5. [Google Scholar] [CrossRef]
- Cheng, K.; Li, Z.; Wu, J.; Hu, Z.-D.; Wang, J. Super-resolution imaging based on radially polarized beam induced superoscillation using an all-dielectric metasurface. Opt. Express 2022, 30, 2780–2791. [Google Scholar] [CrossRef] [PubMed]
- Qin, F.; Huang, K.; Wu, J.; Jiao, J.; Luo, X.; Qiu, C.; Hong, M. Shaping a Subwavelength Needle with Ultra-long Focal Length by Focusing Azimuthally Polarized Light. Sci. Rep. 2015, 5, 9977. [Google Scholar] [CrossRef]
- Yuan, G.; Rogers, K.S.; Rogers, E.T.; Zheludev, N.I. Far-Field Superoscillatory Metamaterial Superlens. Phys. Rev. Appl. 2019, 11, 064016. [Google Scholar] [CrossRef]
- Liu, S.; Guo, Z.; Li, P.; Wei, B.; Zhao, J. Tightly autofocusing beams: An effective enhancement of longitudinally polarized fields. Opt. Lett. 2020, 45, 575–578. [Google Scholar] [CrossRef]
- Guo, Z.; Liu, S.; Li, P.; Wei, B.; Zhao, J. Tightly autofocusing beams along the spherical surface. Opt. Express 2022, 30, 26192–26200. [Google Scholar] [CrossRef]
- Quabis, S.; Dorn, R.; Eberler, M.; Glöckl, O.; Leuchs, G. Focusing light to a tighter spot. Opt. Commun. 2000, 179, 1–7. [Google Scholar] [CrossRef]
- Dorn, R.; Quabis, S.; Leuchs, G. Sharper Focus for a Radially Polarized Light Beam. Phys. Rev. Lett. 2003, 91, 233901. [Google Scholar] [CrossRef]
- Wang, H.; Shi, L.; Lukyanchuk, B.; Sheppard, C.; Chong, C.T. Creation of a needle of longitudinally polarized light in vacuum using binary optics. Nat. Photonics 2008, 2, 501–505. [Google Scholar] [CrossRef]
- Hao, X.; Kuang, C.; Wang, T.; Liu, X. Phase encoding for sharper focus of the azimuthally polarized beam. Opt. Lett. 2010, 35, 3928–3930. [Google Scholar] [CrossRef] [PubMed]
- Huang, K.; Ye, H.; Teng, J.; Yeo, S.P.; Luk’Yanchuk, B.; Qiu, C.-W. Optimization-free superoscillatory lens using phase and amplitude masks. Laser Photonics Rev. 2014, 8, 152–157. [Google Scholar] [CrossRef]
- Richards, B.; Wolf, E.; Gabor, D. Electromagnetic diffraction in optical systems, II. Structure of the image field in an aplanatic system. Proc. R. Soc. Lond. Ser. A Math. Phys. 1959, 253, 358–379. [Google Scholar] [CrossRef]
- Han, L.; Qi, S.; Liu, S.; Cheng, H.; Li, P.; Zhao, J. Tightly focused light field with controllable pure transverse polarization state at the focus. Opt. Lett. 2020, 45, 6034–6037. [Google Scholar] [CrossRef] [PubMed]
- Guo, X.; Li, P.; Zhong, J.; Liu, S.; Wei, B.; Zhu, W.; Qi, S.; Cheng, H.; Zhao, J. Tying Polarization-Switchable Optical Vortex Knots and Links via Holographic All-Dielectric Metasurfaces. Laser Photonics Rev. 2020, 14, 1900366. [Google Scholar] [CrossRef]
- Guo, X.; Zhong, J.; Li, B.; Qi, S.; Li, Y.; Li, P.; Wen, D.; Liu, S.; Wei, B.; Zhao, J. Full-Color Holographic Display and Encryption with Full-Polarization Degree of Freedom. Adv. Mater. 2022, 34, 2103192. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Fan, X.; Huang, Y.; Guo, X.; Zhou, L.; Li, P.; Zhao, J. Dielectric Metalens for Superoscillatory Focusing Based on High-Order Angular Bessel Function. Nanomaterials 2022, 12, 3485. https://doi.org/10.3390/nano12193485
Li Y, Fan X, Huang Y, Guo X, Zhou L, Li P, Zhao J. Dielectric Metalens for Superoscillatory Focusing Based on High-Order Angular Bessel Function. Nanomaterials. 2022; 12(19):3485. https://doi.org/10.3390/nano12193485
Chicago/Turabian StyleLi, Yu, Xinhao Fan, Yunfeng Huang, Xuyue Guo, Liang Zhou, Peng Li, and Jianlin Zhao. 2022. "Dielectric Metalens for Superoscillatory Focusing Based on High-Order Angular Bessel Function" Nanomaterials 12, no. 19: 3485. https://doi.org/10.3390/nano12193485
APA StyleLi, Y., Fan, X., Huang, Y., Guo, X., Zhou, L., Li, P., & Zhao, J. (2022). Dielectric Metalens for Superoscillatory Focusing Based on High-Order Angular Bessel Function. Nanomaterials, 12(19), 3485. https://doi.org/10.3390/nano12193485






