Development of a Two-Dimensional Hybrid Sediment-Transport Model
Abstract
1. Introduction
2. Numerical Model
2.1. Flow Model
2.2. Sediment-Transport Model
2.2.1. Suspended-Load Transport
2.2.2. Bed-Load Transport
2.2.3. Bed Changes and Sorting
3. Numerical Method
3.1. FVM Discretization
3.2. Edge-Gradient Evaluation
3.3. Multipoint Momentum Interpolation Correction
3.4. Solution Procedure
4. Examples and Application
4.1. Bed Degradation
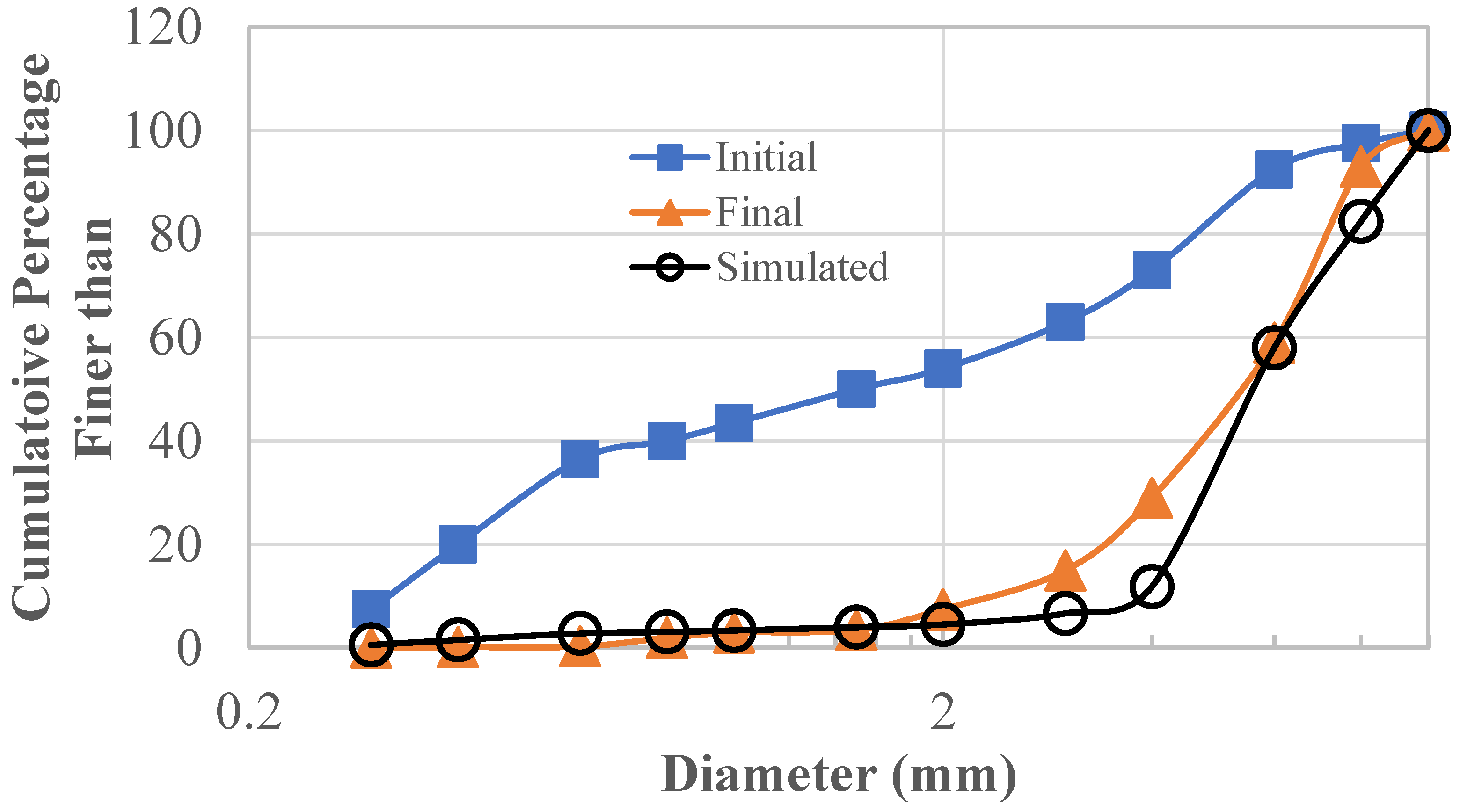
4.2. Bed Aggradation
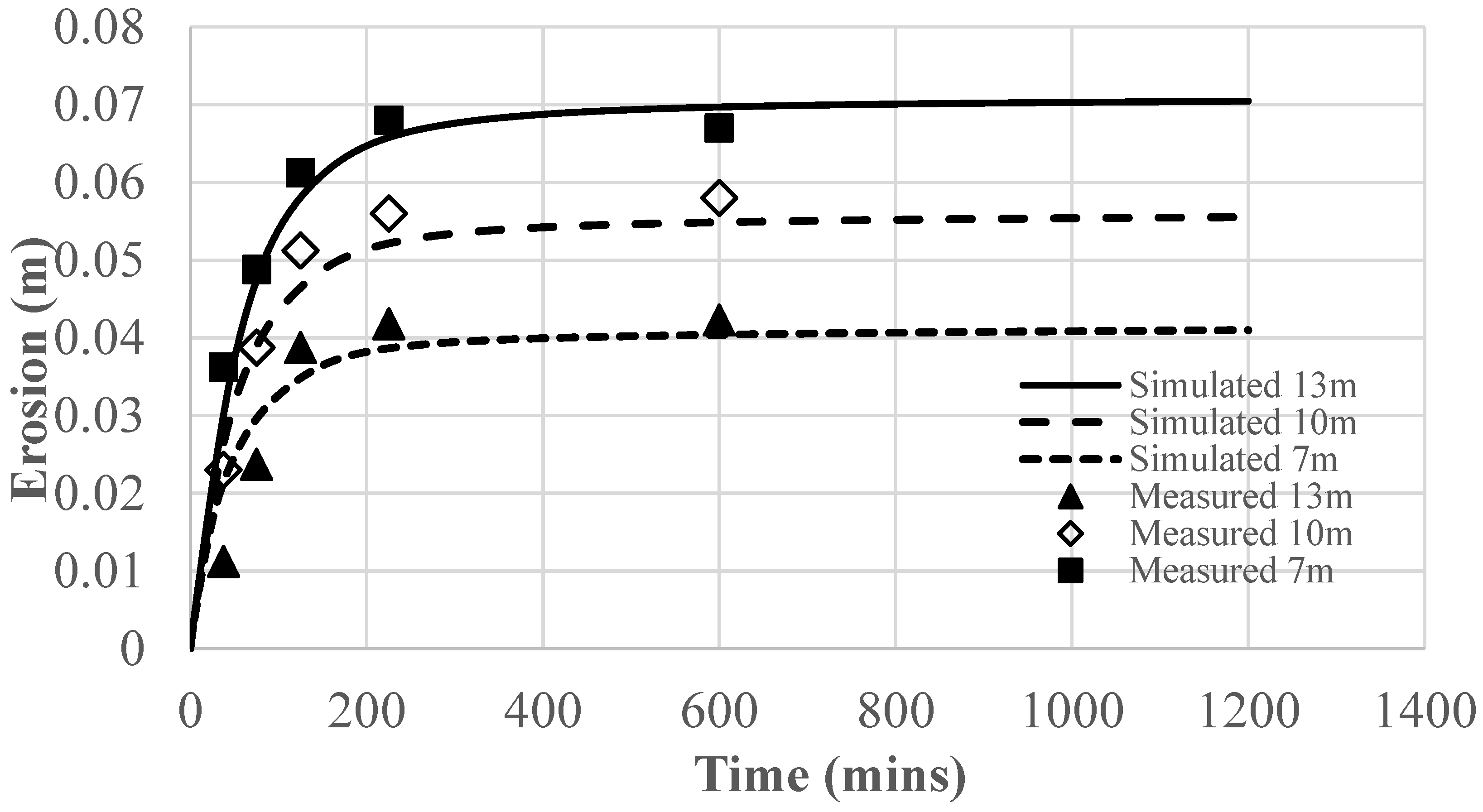
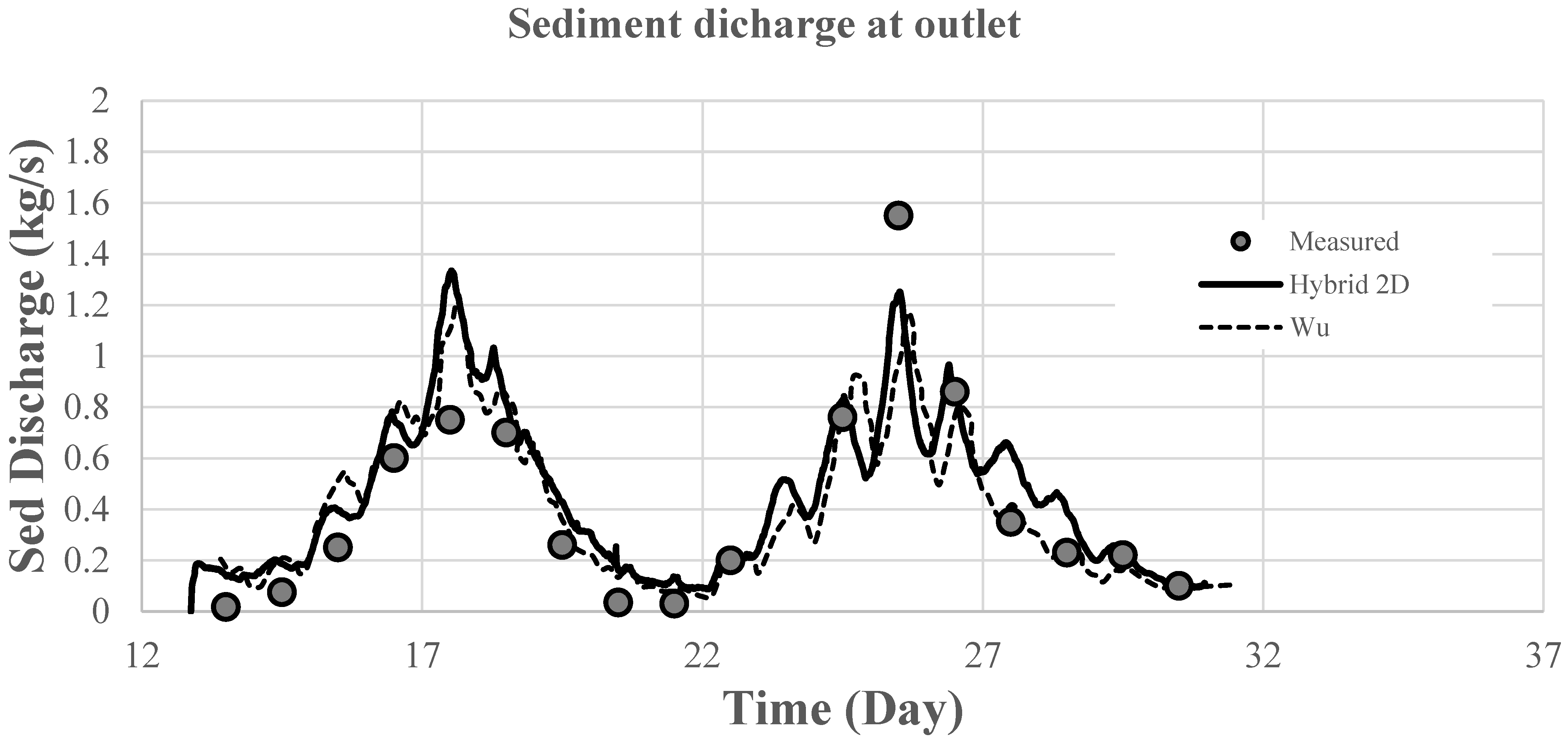
4.3. East Fork River
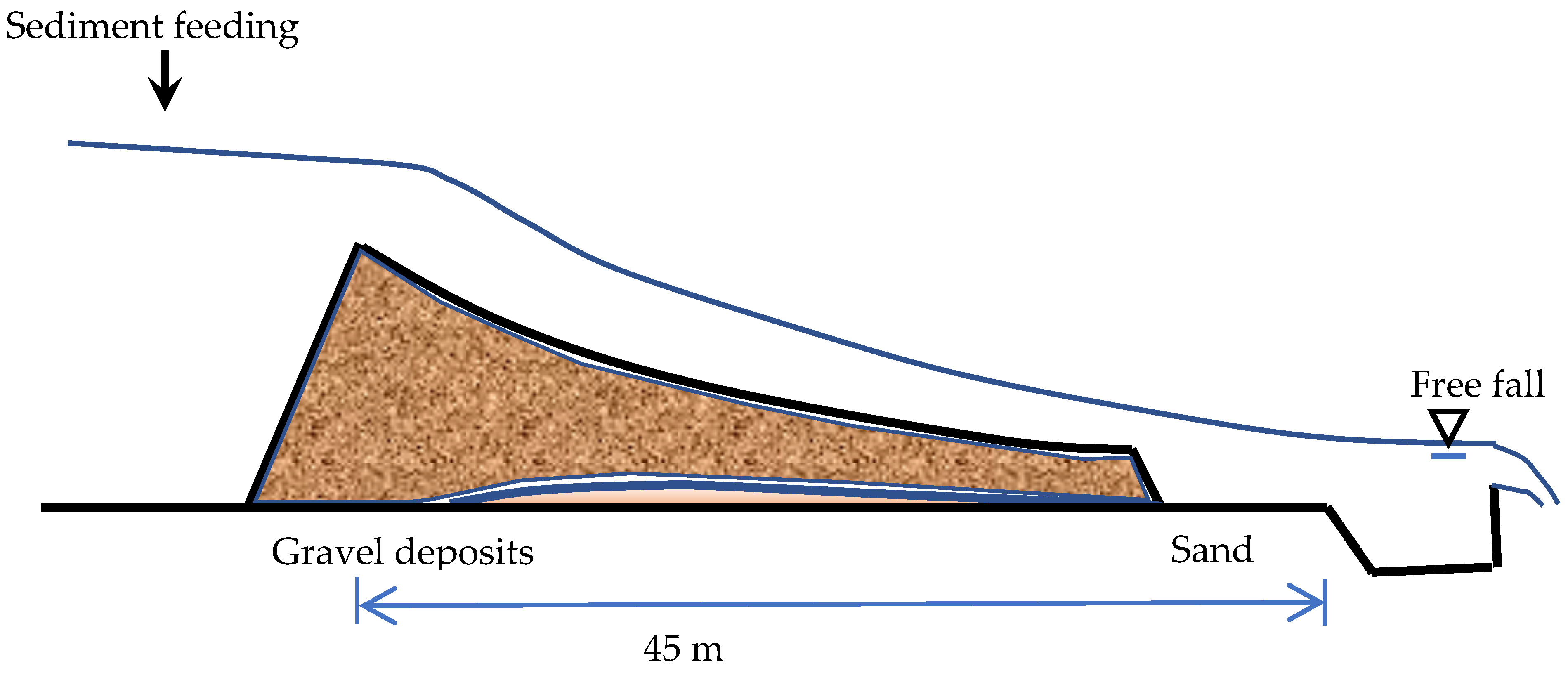
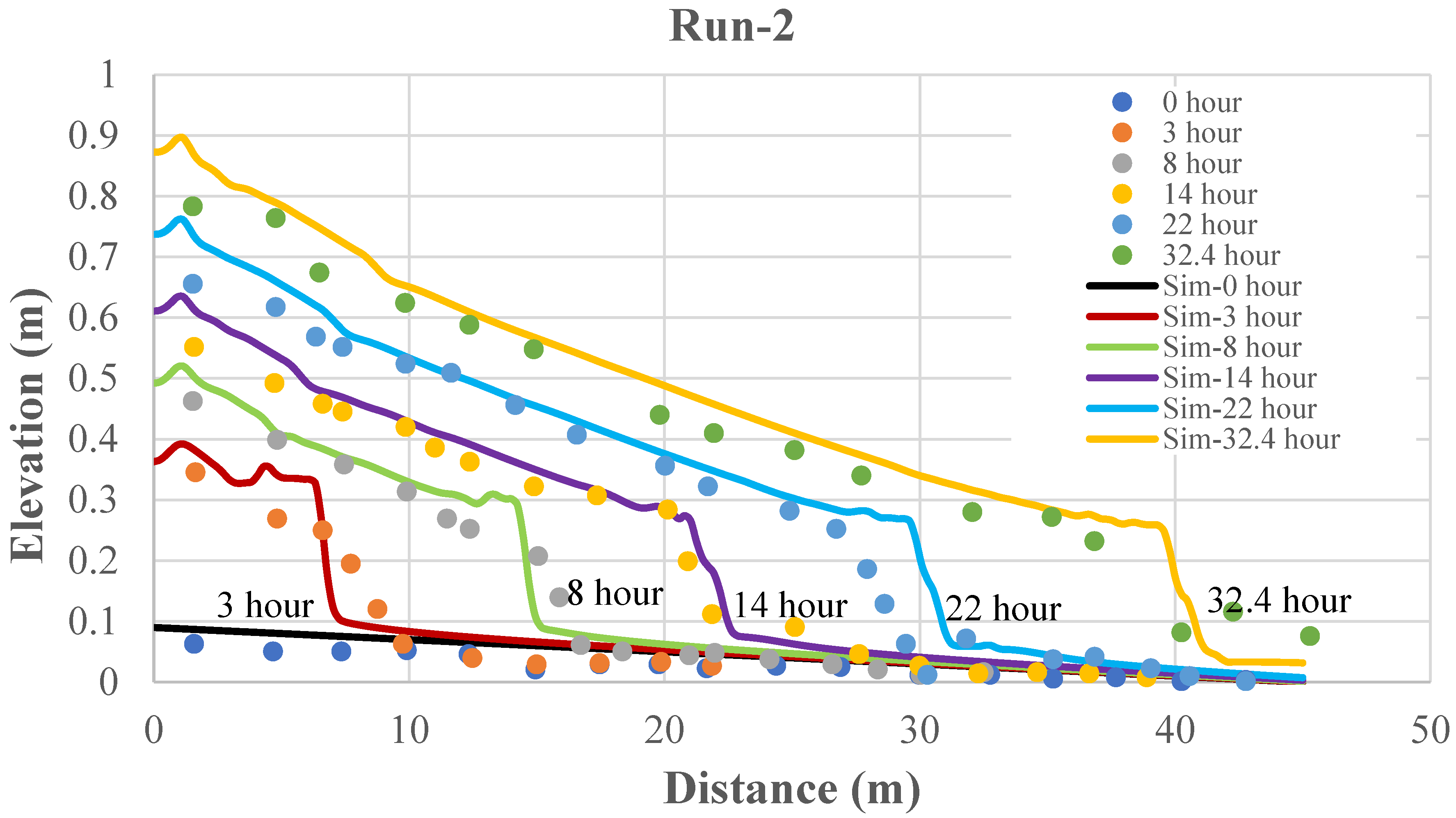
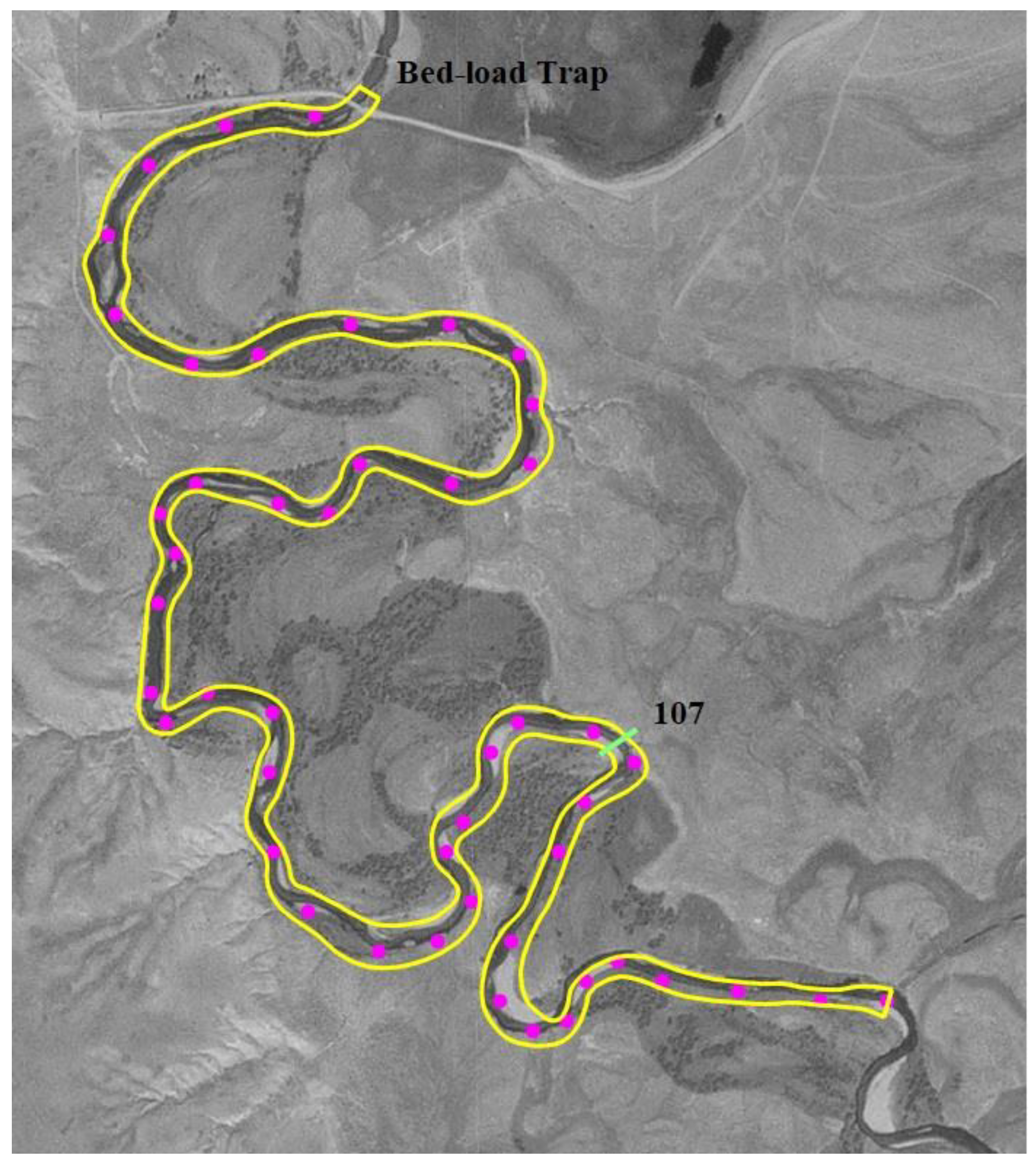
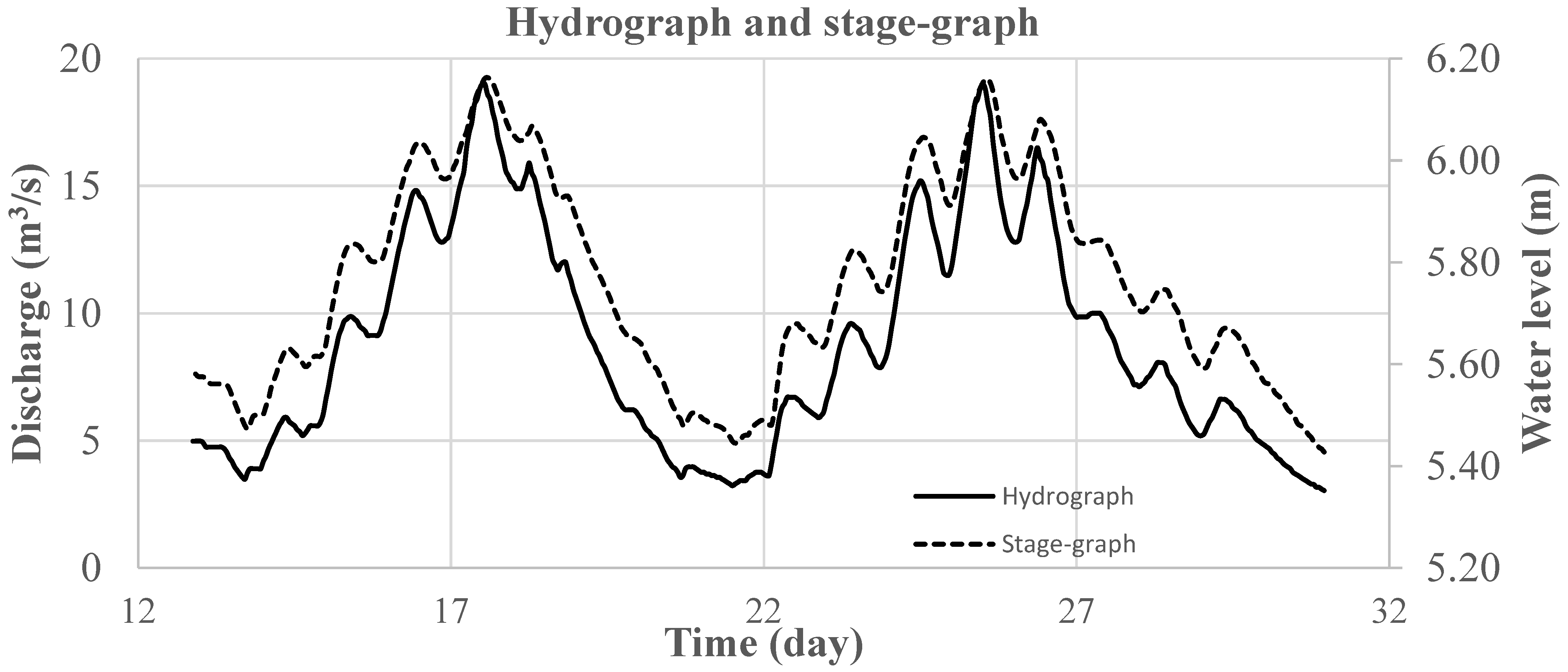
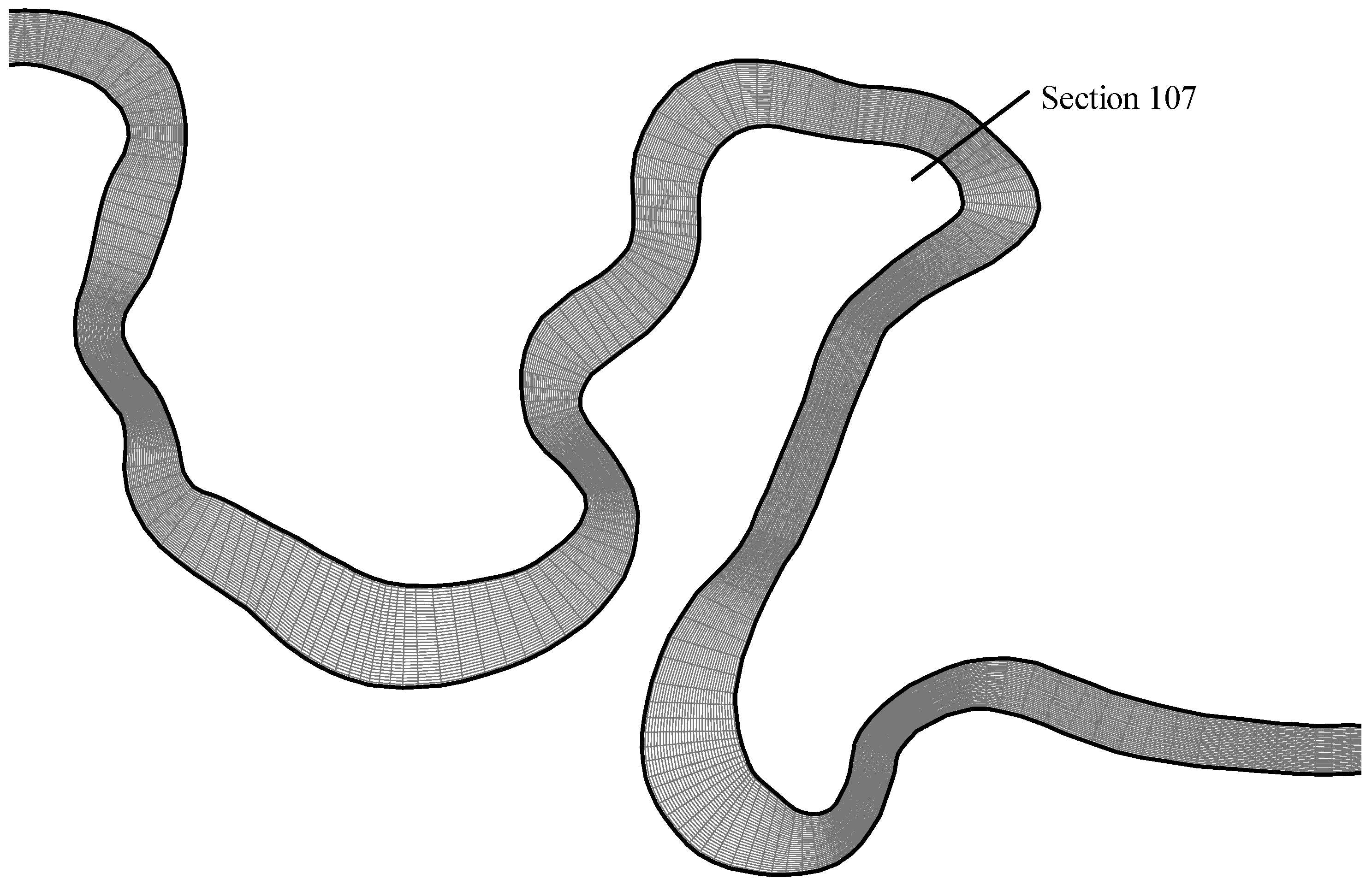
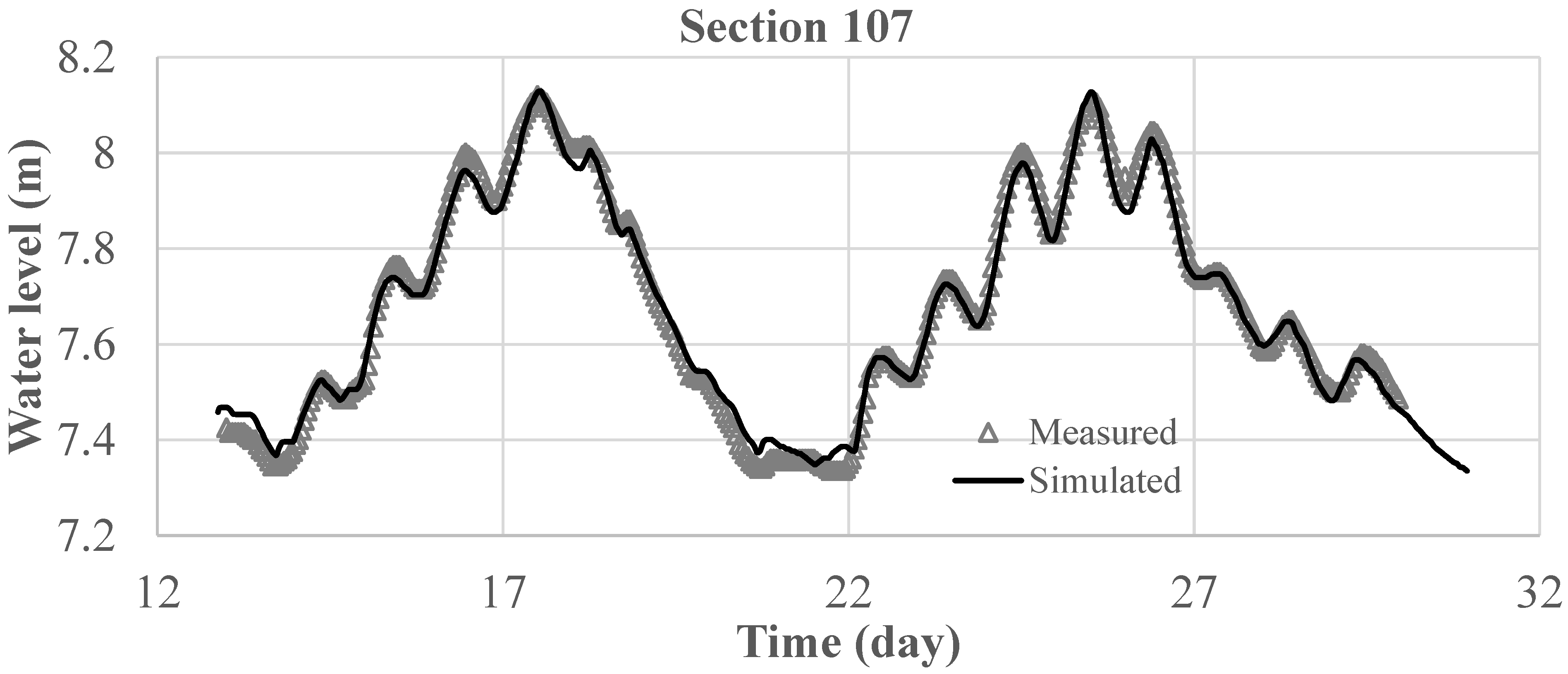
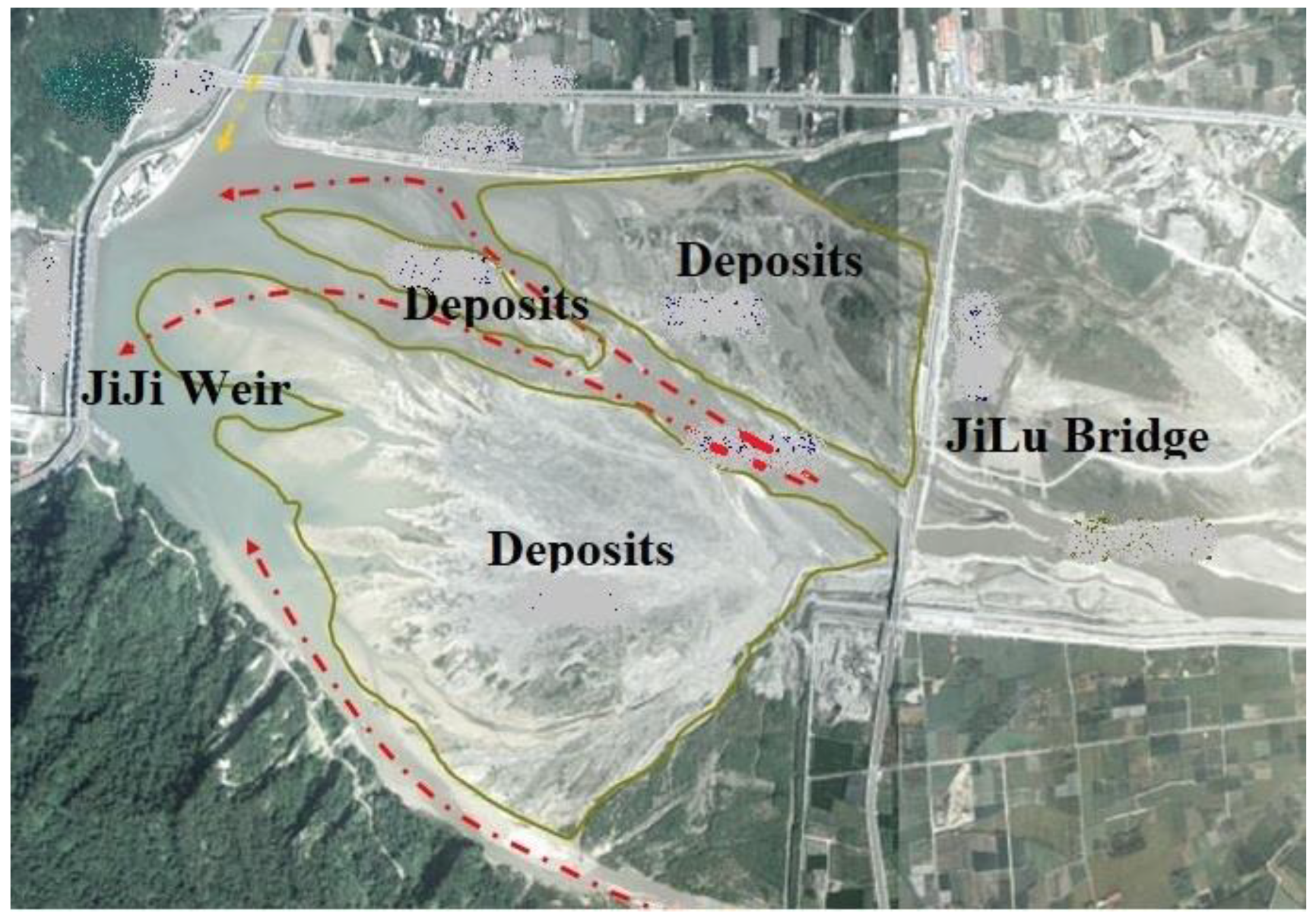
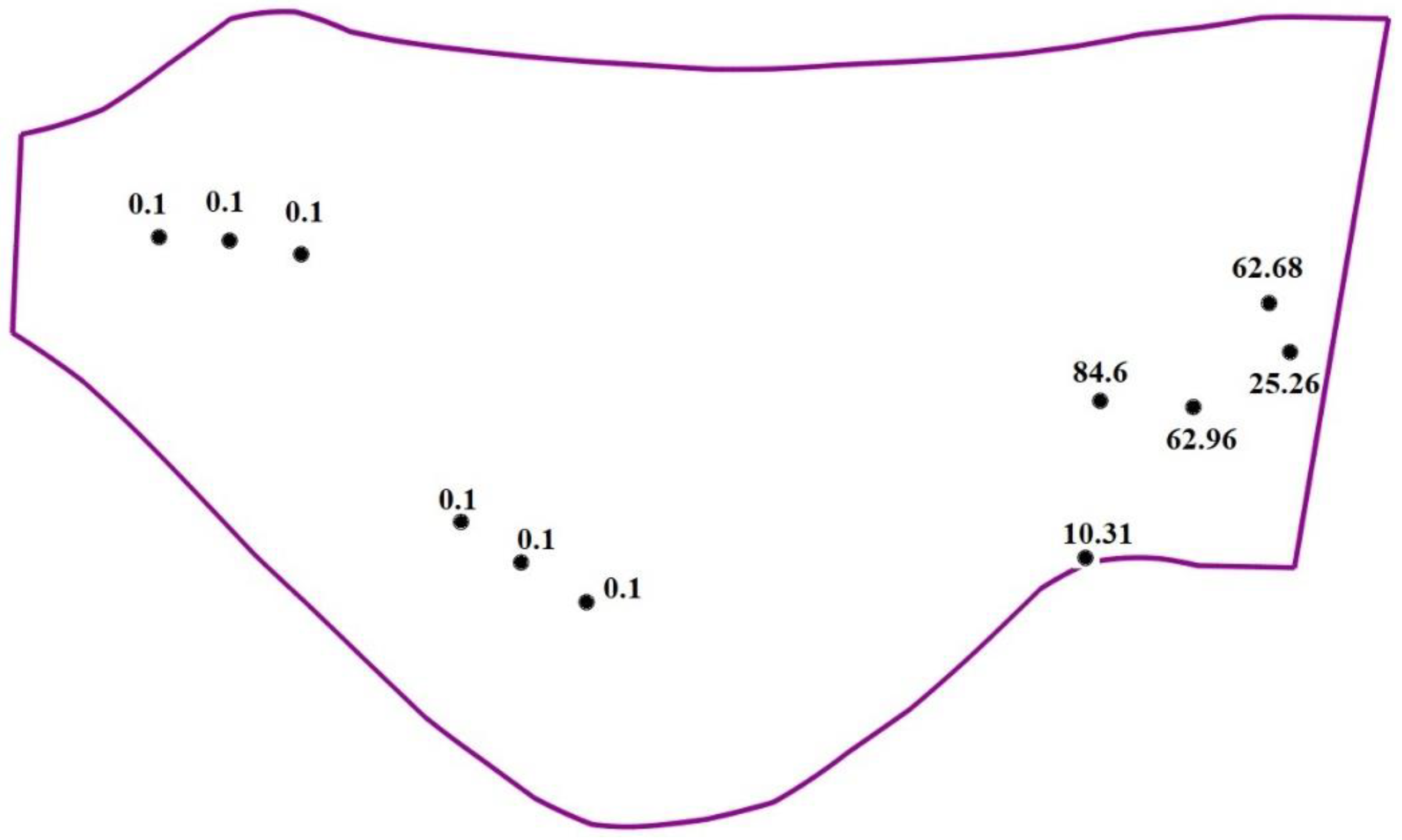
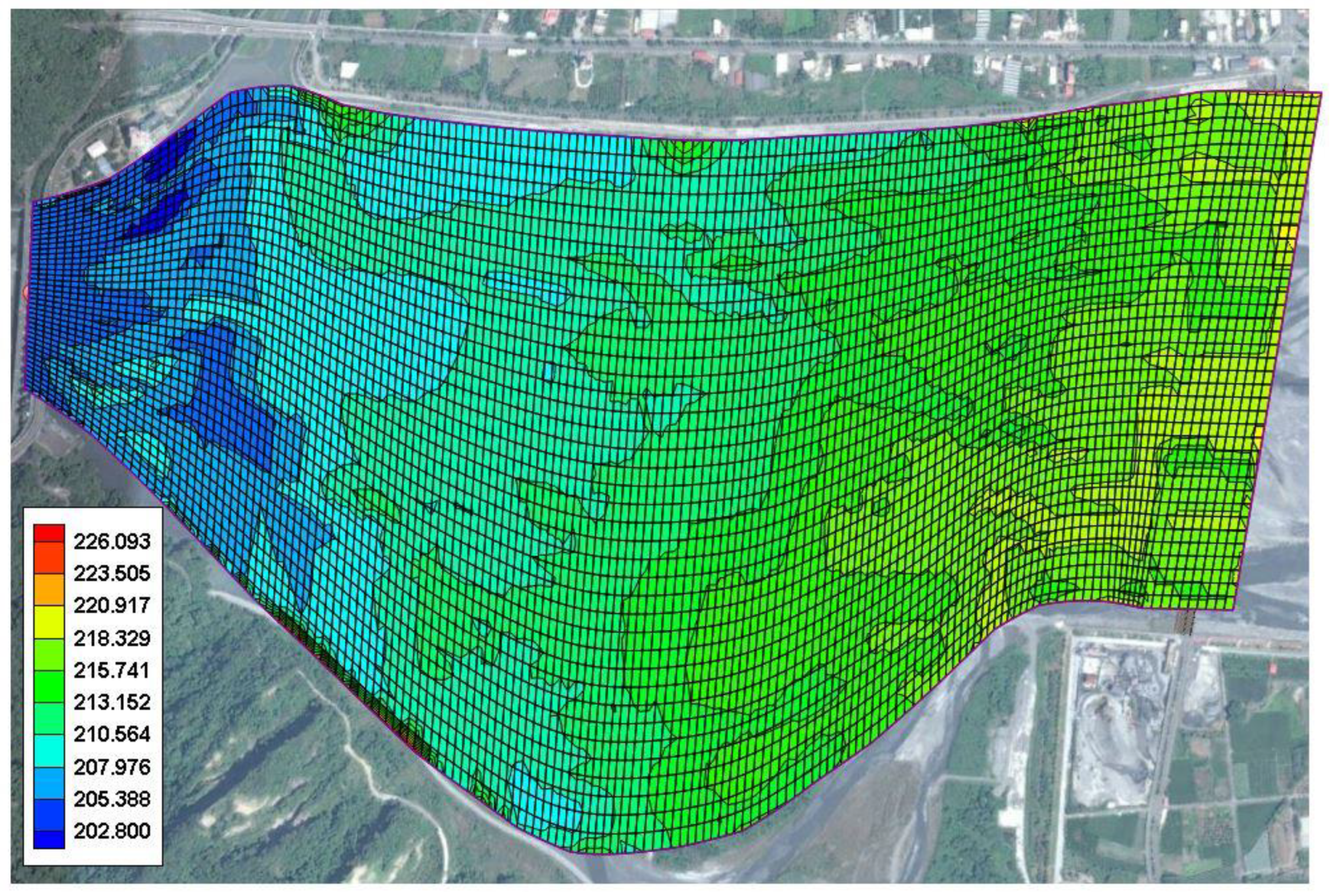
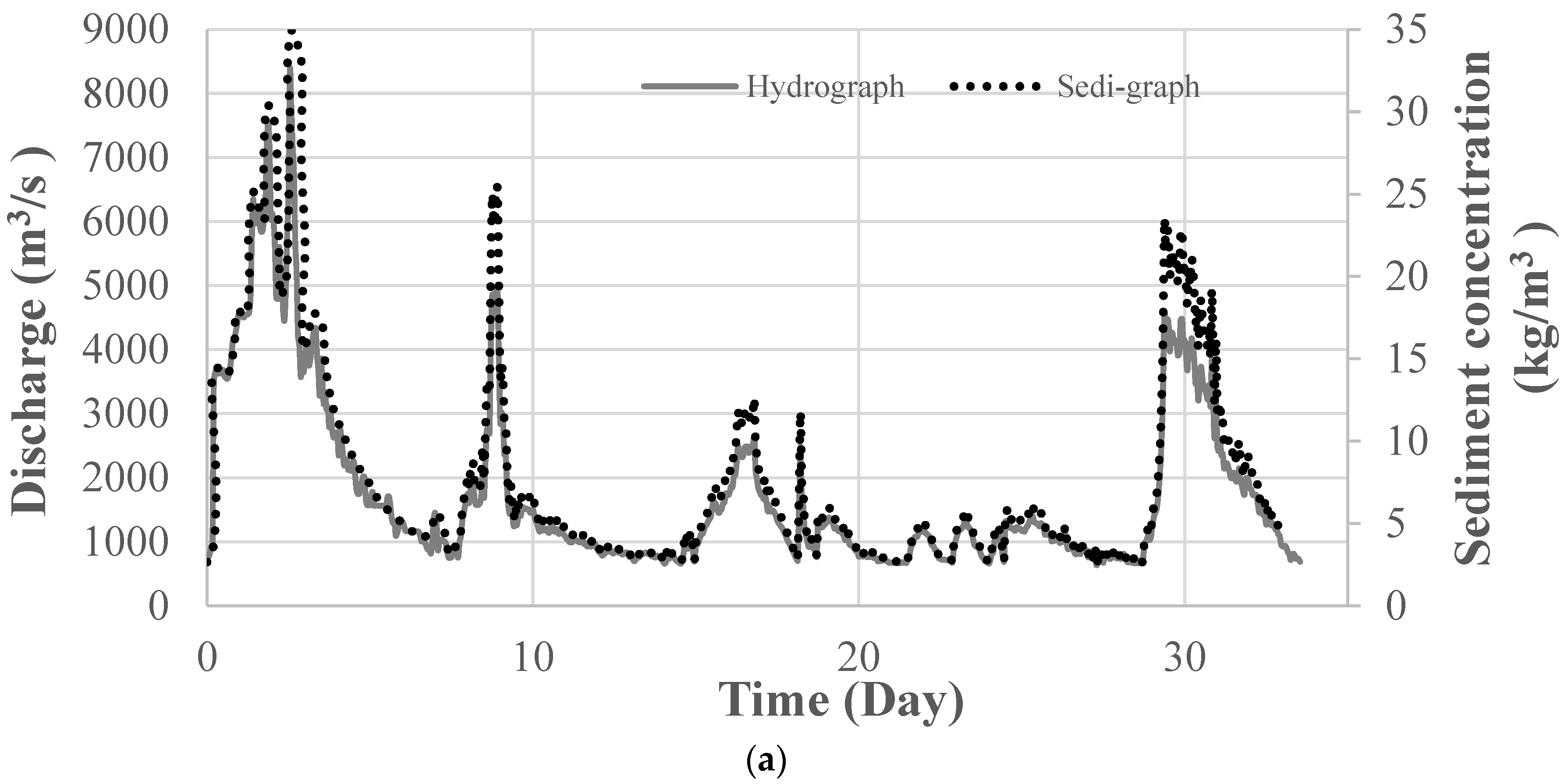
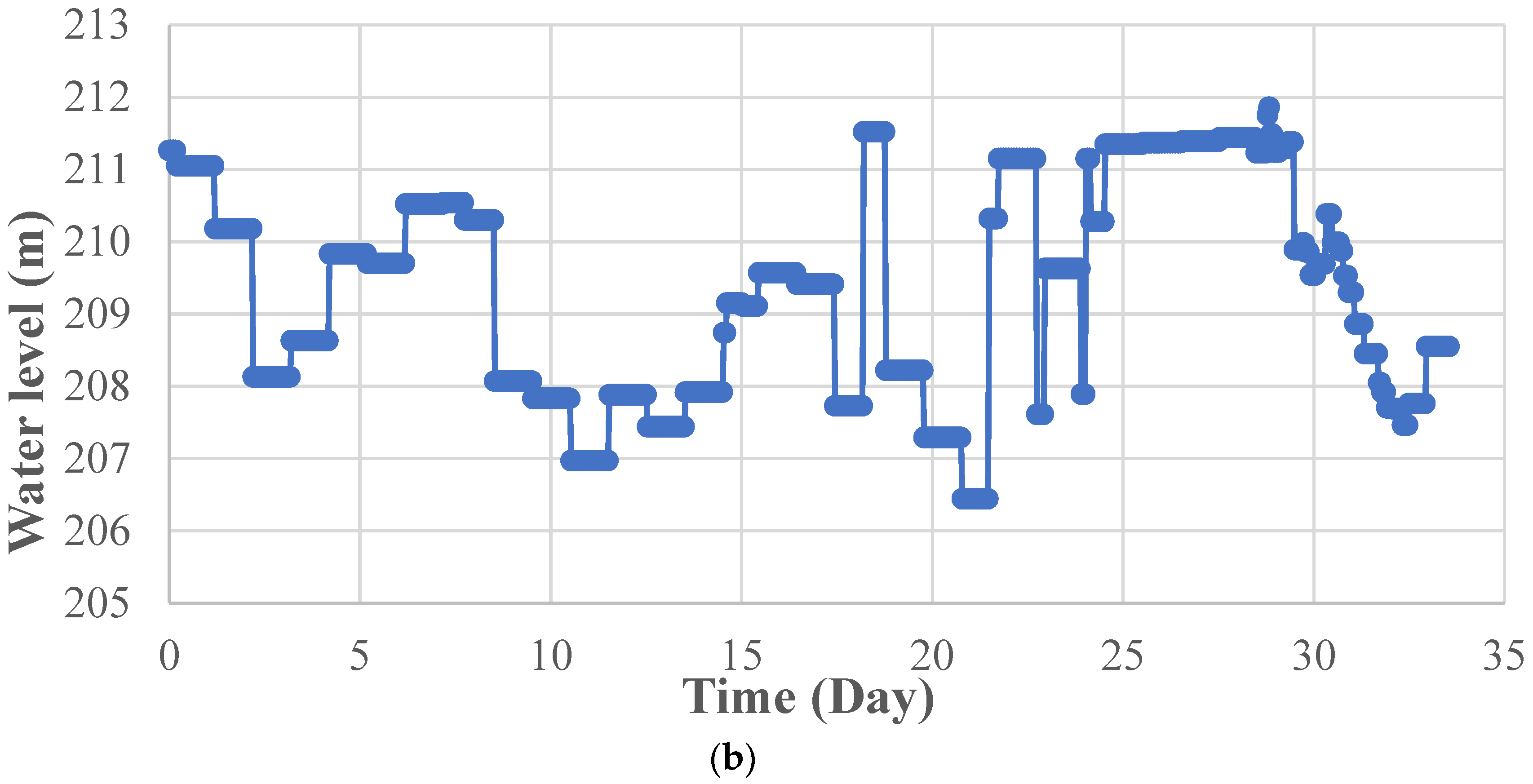
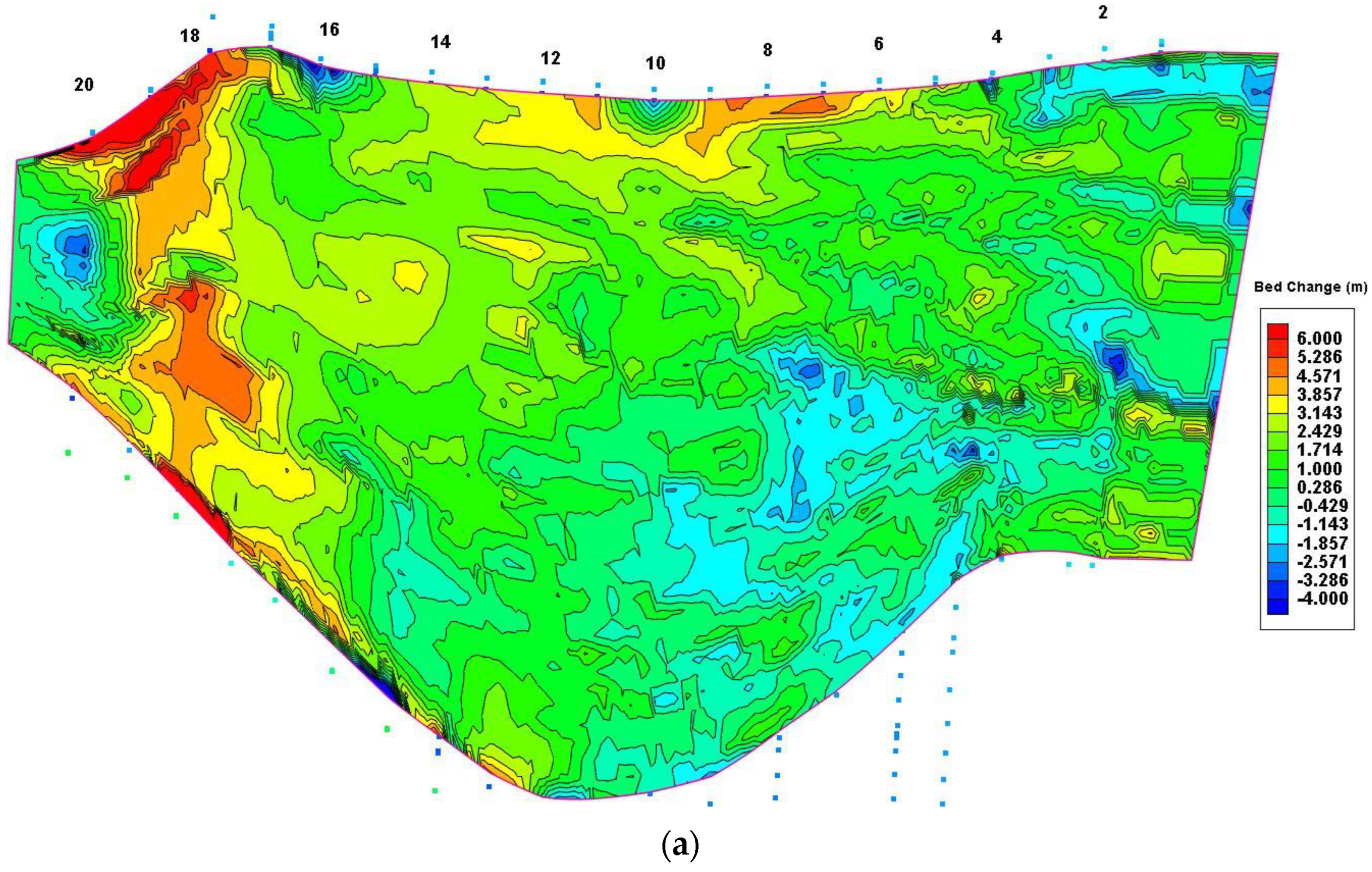
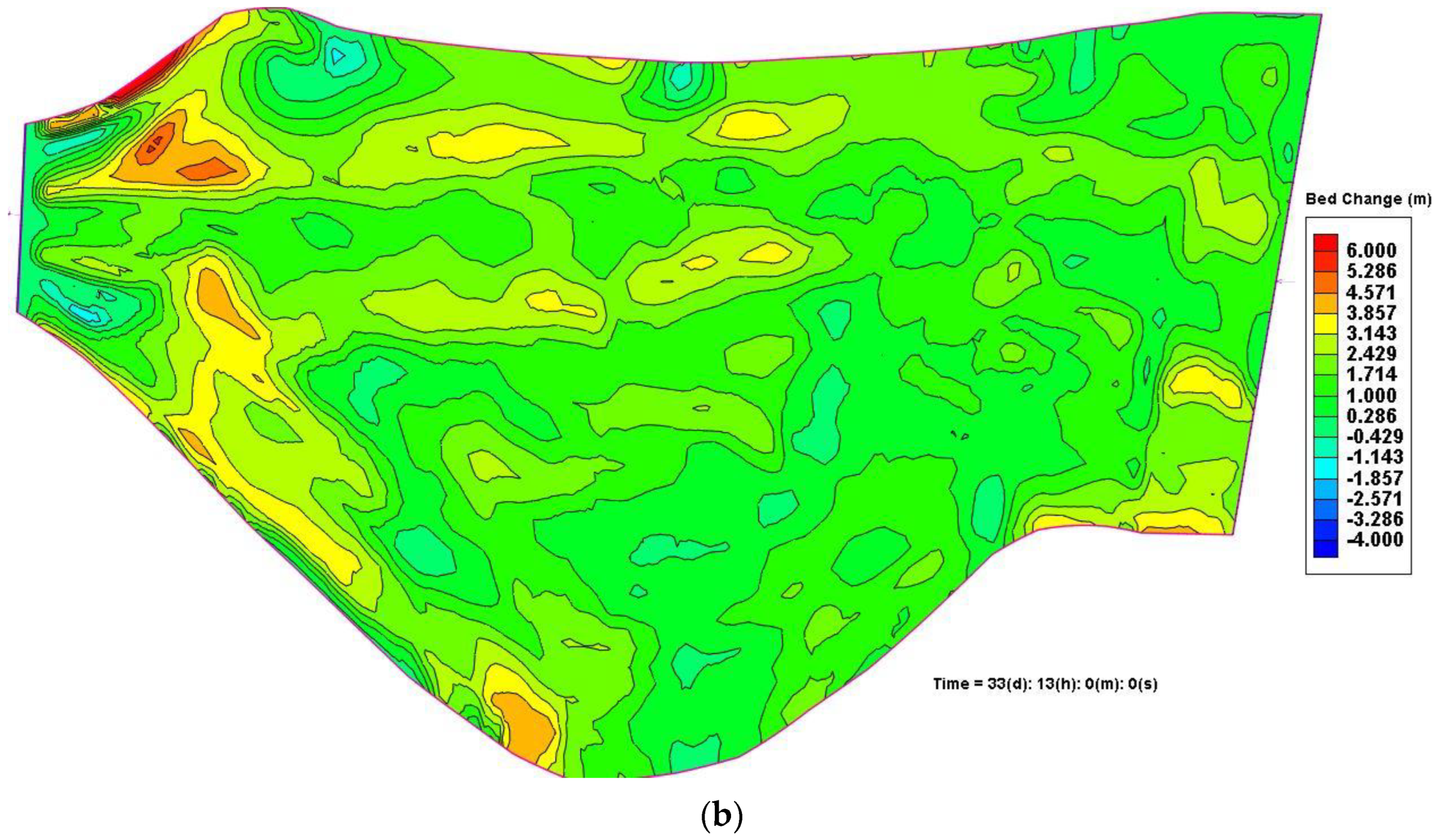
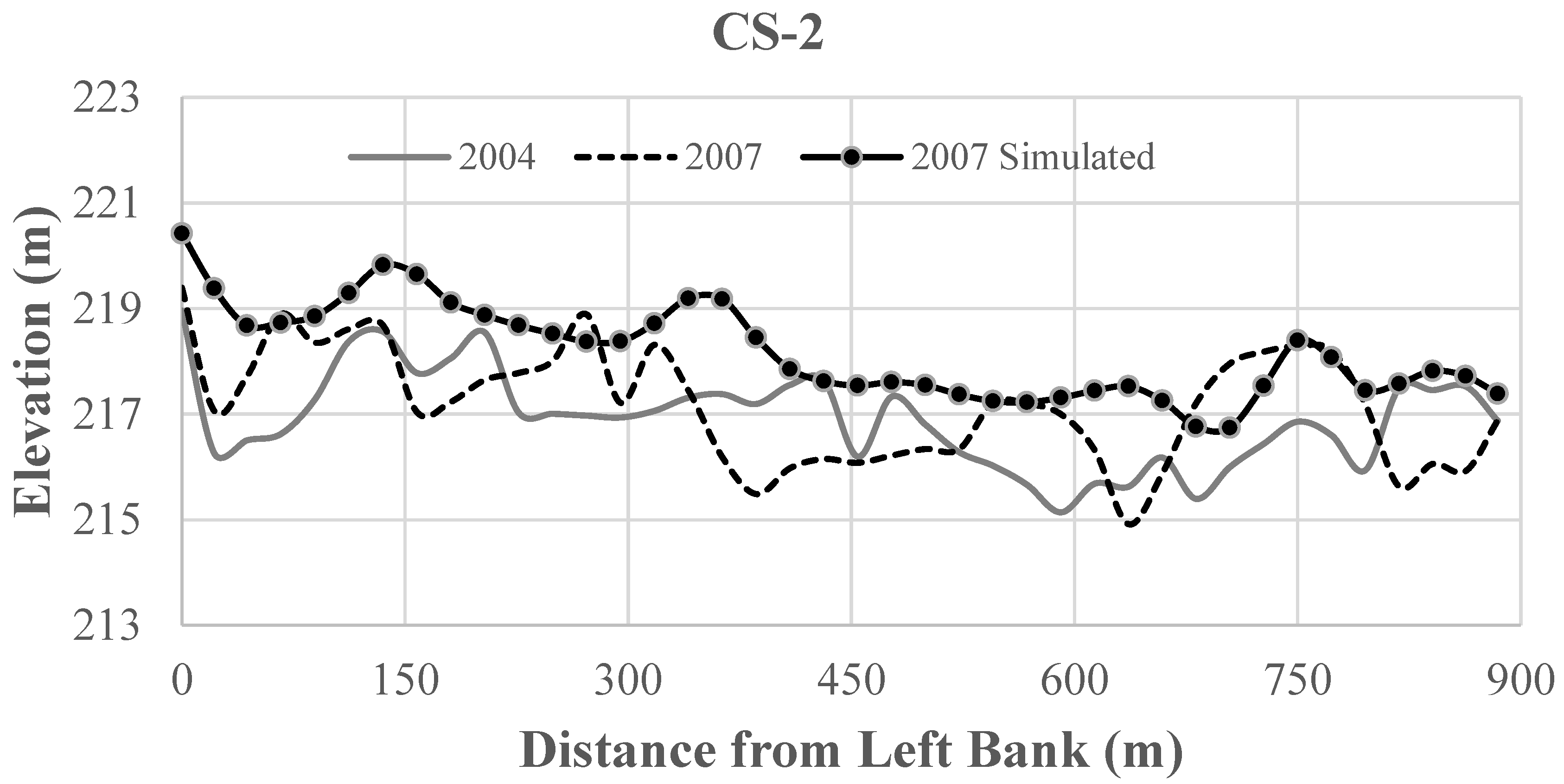
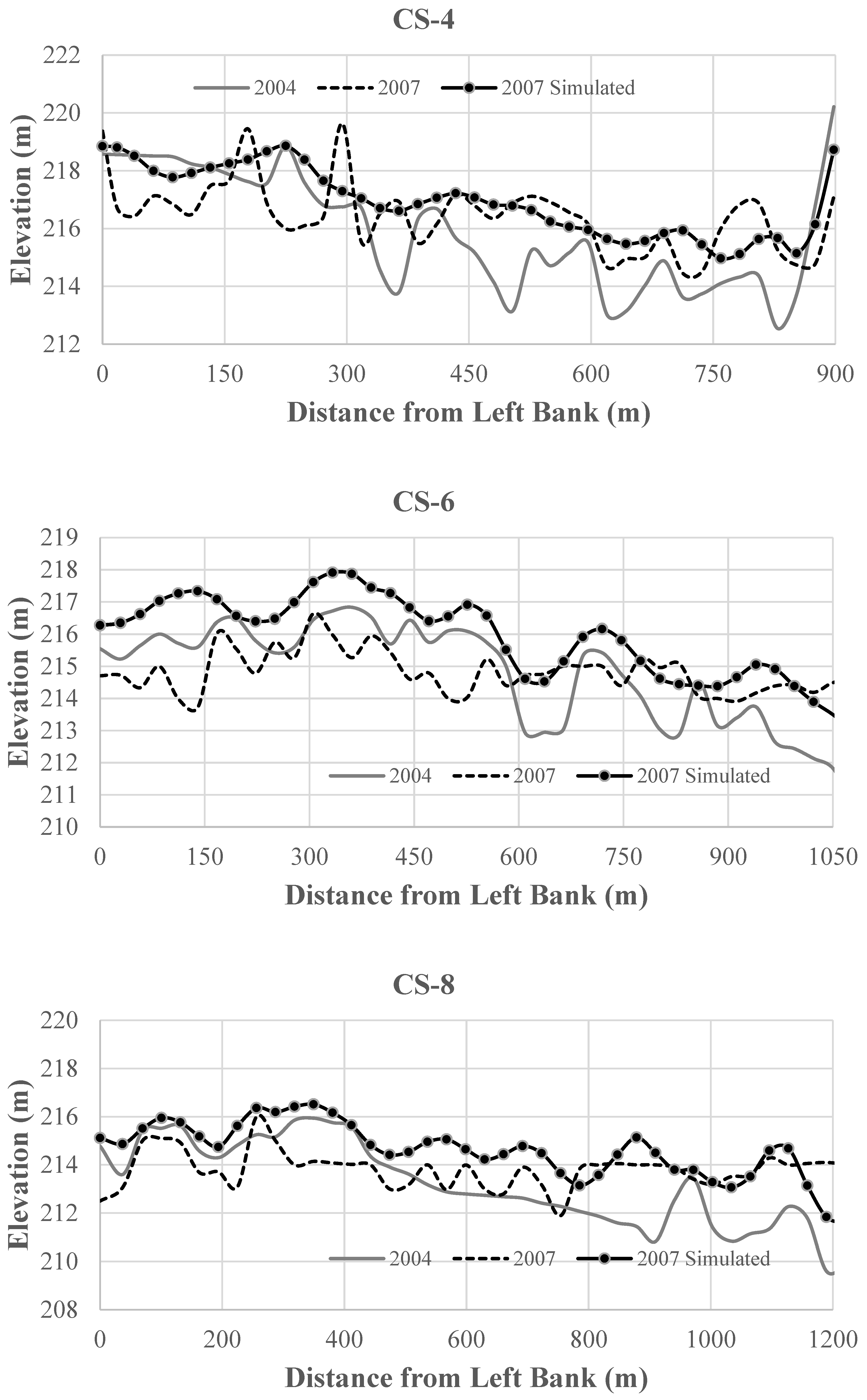
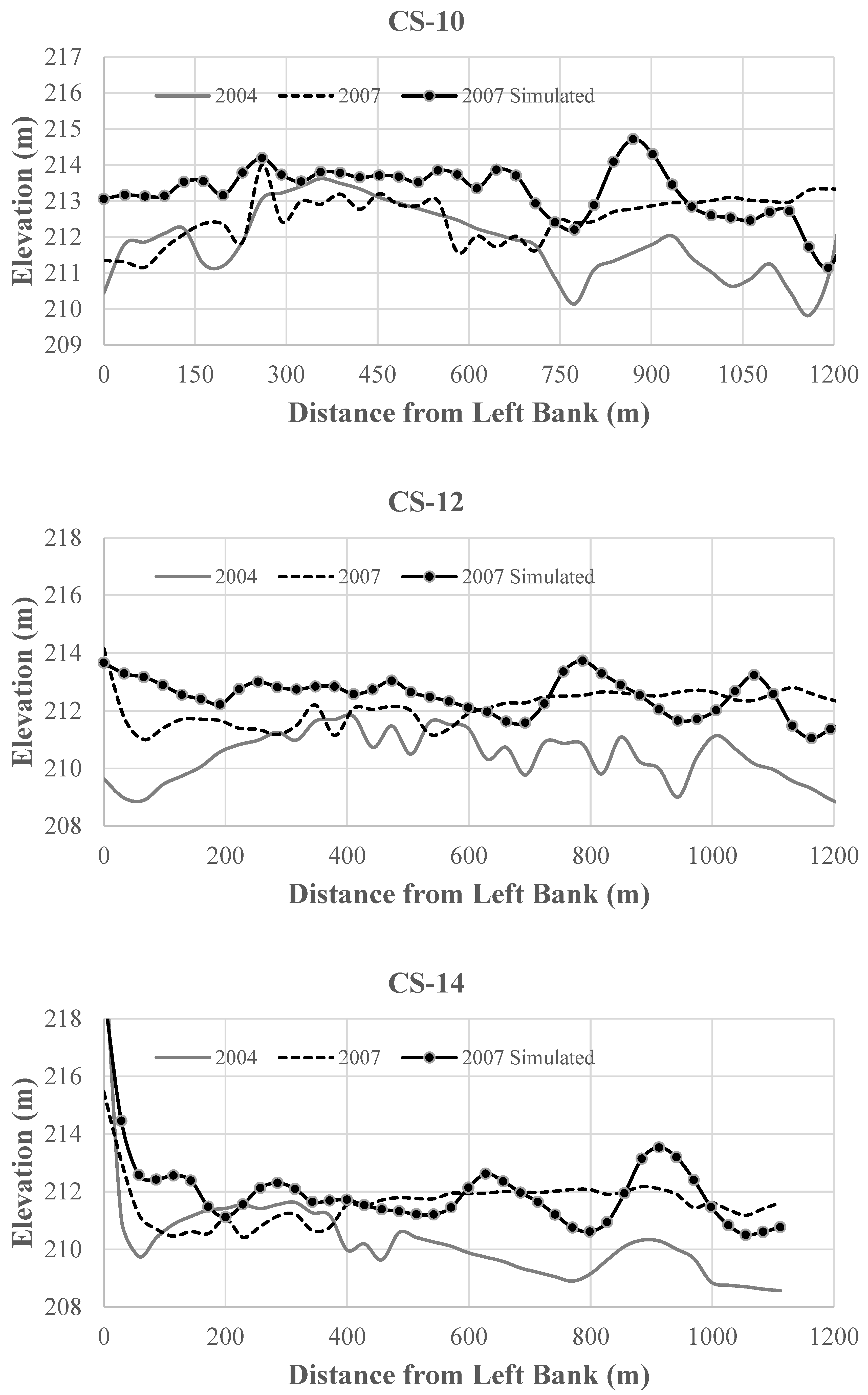
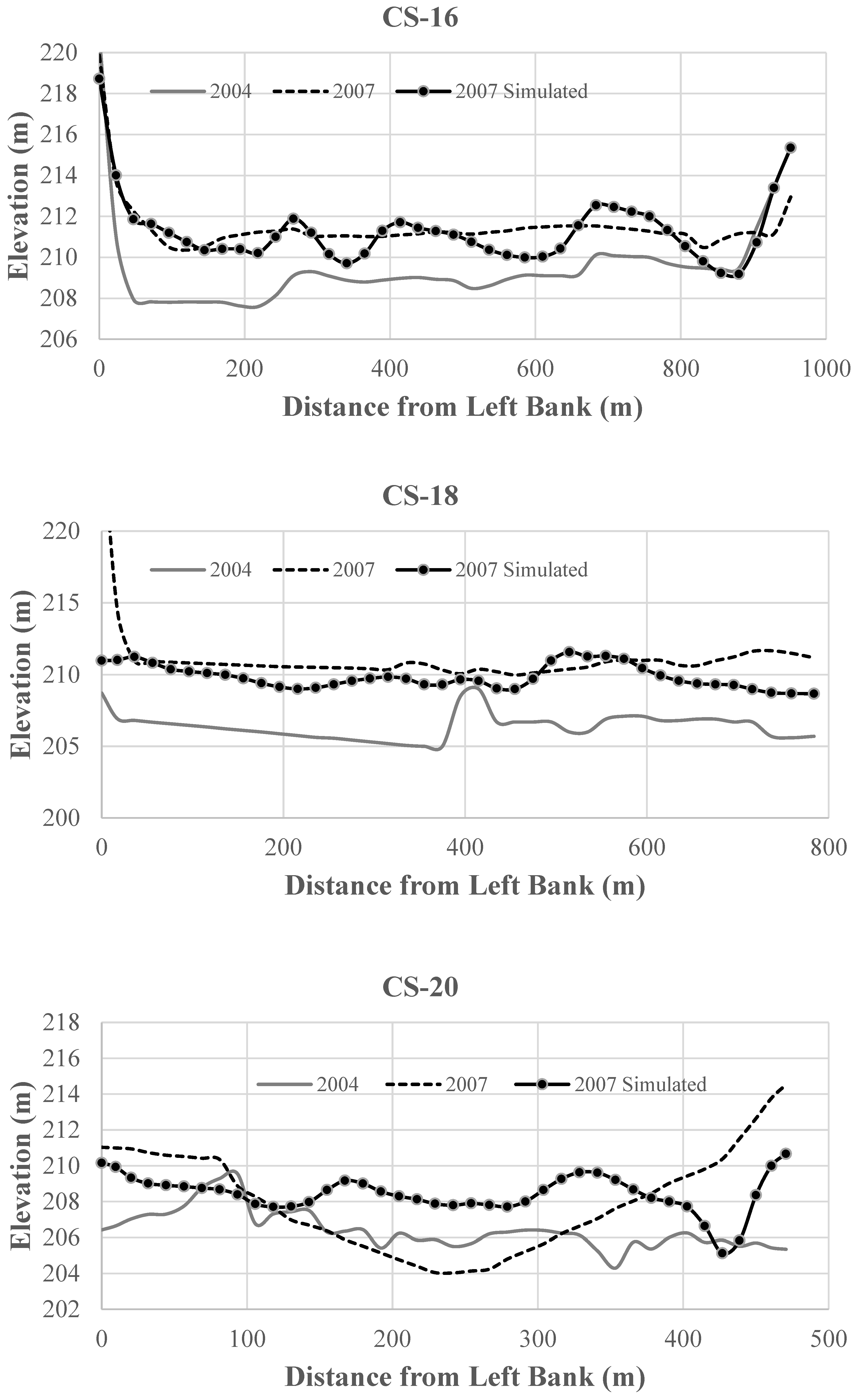
4.4. JiJi Reservoir
4.5. Discussion
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, Y.; Jia, Y.; Yeh, K.C.; Liao, C.T.; Wang, S.S.Y. Numerical Simulation of Sediment Transport Simulation of JiJi Weir Reservoir. In Proceedings of the EWRI World Environment & Water Resources Congress 2009, Kansas City, MI, USA, 17–21 May 2009. [Google Scholar]
- Yen, Y.H.; Chen, T.C.; Liu, G.X. Best Model for Operations of JiJi Weir. In Proceedings of the 3rd International Workshop on Sediment Bypass Tunnels, Taipei, Taiwan, 9–12 April 2019. [Google Scholar]
- Turner, R.E.; Baustian, J.J.; Swenson, E.M.; Spicer, J.S. Wetland Sedimentation from Hurricanes Katrina and Rita. Sci. (Am. Assoc. Adv. Sci.) 2006, 314, 449–452. [Google Scholar] [CrossRef] [PubMed]
- Liu, K.; Chen, Q.; Hu, K.L.; Xu, K.H.; Twilley, R.R. Modeling Hurricane-induced wetland-bay and bay-shelf sediment fluxes. Coast. Eng. 2018, 135, 77–90. [Google Scholar] [CrossRef]
- Zhang, Y.; Jia, Y.; Yeh, K.C.; Liao, C.T.; Wang, S.S.Y. Sediment Transport Simulation of Chuoshui Creek. In Proceedings of the EWRI World Environment & Water Resources Congress 2010, Providence, RI, USA, 16–20 May 2010. [Google Scholar]
- Jia, Y.; Zhang, Y.; Yeh, K.C.; Liao, C.T. Modeling River Morphodynamic Process Using a Depth-Averaged Computational Model and an Application to a Mountain River; IntechOpen: London, UK, 2019. [Google Scholar] [CrossRef]
- Ding, Y.; Frey, A.E.; Kim, S.; Permenter, R.E. Probabilistic shoreline change modeling and risk estimation of erosion. Costal Eng. 2018, 36, 1. [Google Scholar] [CrossRef]
- Dong, P.; Wu, X.Z. Application of a stochastic differential equation to the prediction of shoreline evolution. Stoch. Environ. Res. Risk. Assess 2013, 27, 1799–1814. [Google Scholar] [CrossRef]
- Ashida, K.; Michiue, M. An investigation of river bed degradation downstream of a dam. In Proceedings of the 14th Congress of the IAHR, Paris, France, 3 September 1971. [Google Scholar]
- Zhang, Y.; Jia, Y.; Yeh, K.C.; Liao, C.T. Erosion Control at Downstream of Reservoir Using in-Stream Weirs; IntechOpen: London, UK, 2022. [Google Scholar] [CrossRef]
- Hoffmans, G.; Pilarczyk, K. Local scour downstream of hydraulic structures. J. Hydraul. Eng. 1995, 121, 326–340. [Google Scholar] [CrossRef]
- Jia, Y.; Altinakar, M.; Guney, M.S. Three-dimensional Numerical Simulations Of Local Scouring Around Bridge Piers. J. Hydraul. Res. 2018, 56, 351–366. [Google Scholar] [CrossRef]
- Vandaele, K.; Poesen, J.; Govers, G.; van Wesemael, B. Geomorphic threshold conditions for ephemeral gully incision. Geomorphology 1996, 16, 161–173. [Google Scholar] [CrossRef]
- Roberts, M.E.; Burrows, R.M.; Thwaites, R.N.; Hamilton, D.P. Modelling classical gullies—A review. Geomorphlogy 2022, 407, 108216. [Google Scholar] [CrossRef]
- Spasojevic, M.; Holly, F.M., Jr. 2-D bed evolution in natural watercourses—New simulation approach. J. Waterw. Port Coast. Ocean. Eng. 1990, 116, 425–443. [Google Scholar] [CrossRef]
- Karki, S.; Nakagawa, H.; Zhang, Y. How Good Are 2D-Morphadynamic Models in Reproducing Bed Morphology Of Meandering Channels? Analyzing the Strengths And Limitations Of Three Popular 2D Models. In Proceedings of the 1st IAHR Young Professionals Congress, Virtual, 17–18 November 2020. [Google Scholar]
- Chao, X.; Jia, Y.; Shields, F.D., Jr.; Wang, S.S.Y.; Cooper, C.M. Three-Dimensional Numerical Modelling of Water Quality and Sediment-Associated Processes with Application to a Mississippi Delta Lake. J. Environ. Manag. 2010, 91, 1456–1466. [Google Scholar] [CrossRef]
- Zhang, R.J. River Dynamics; Industry Press: Beijing, China, 1961. (In Chinese) [Google Scholar]
- Wu, W. Depth-averaged two-dimensional numerical modeling of unsteady flow and non-uniform sediment transport in open channels. J. Hydraul. Eng. 2004, 130, 1013–1024. [Google Scholar] [CrossRef]
- Wu, W. Computational River Dynamics; Taylor & Francis Group: London, UK, 2007. [Google Scholar]
- Chauchat, J.; Cheng, Z.; Nagel, T.; Bonamy, C.; Hsu, T.J. SedFoam-2.0: 2 3-D two-phase flow numerical model for sediment transport. Geosci. Model Dev. 2017, 10, 4372–4392. [Google Scholar] [CrossRef]
- Dong, P.; Zhang, K. Two-phase flow modelling of sediment motions in oscillatory sheet flow. Coast. Eng. 1999, 36, 87–109. [Google Scholar] [CrossRef]
- Cheng, Z.; Hsu, T.J.; Calantoni, J. SedFoam: A multi-dimensional Eulerian two-phase model for sediment transport and its application to momentary bed failure. Coast. Eng. 2017, 119, 32–50. [Google Scholar] [CrossRef]
- Jia, Y.; Wang, S.S.Y. Numerical model for channel flow and morphological change studies. J. Hydraul. Eng. ASCE 1999, 125, 924–933. [Google Scholar] [CrossRef]
- Horvat, M.; Horvat, Z. Sediment transport and bed evolution model for complex river systems. Environ. Monit. Assess. 2020, 192, 242. [Google Scholar] [CrossRef] [PubMed]
- Lai, Y. A Two-Dimensional Depth-Averaged Sediment Transport Mobile-Bed Model with Polygonal Meshes. Water 2020, 12, 1032. [Google Scholar] [CrossRef]
- Wu, W.; Vieira, D.A.; Wang, S.S.Y. A 1-D numerical model for nonuniform sediment transport under unsteady flows in channel networks. J. Hydraulic Eng. ASCE 2004, 130, 914–923. [Google Scholar] [CrossRef]
- DHI. MIKE 11 Users’ Manual; Danish Hydraulic Institute: Horsholm, Denmark, 2005. [Google Scholar]
- Gibson, S.; Brunner, G.; Piper, S.; Jensen, M. Sediment Transport Computations in HEC-RAS. In Proceedings of the Eighth Federal Interagency Sedimentation Conference (8th FISC), Reno, NV, USA, 2–6 April 2006; pp. 57–64. [Google Scholar]
- Huang, J.; Greimann, B.P. GSTAR-1D, General Sediment Transport for Alluvial Rivers—One Dimension; Technical Service Center, U.S. Bureau of Reclamation: Denver, CO, USA, 2007.
- Jia, Y. CCHE3D Technical Manual, National Center for Computational Hydroscience and Engineering Technical Report; The University of Mississippi: Oxford, MS, USA, 2013. [Google Scholar]
- Deltares. Delft3D-FLOW. In Simulation of Multi-Dimensional Hydrodynamic Flow and Transport Phenomena, including Sediments–User Manual; Version 3.04, rev. 11114; Deltares: Delft, The Netherlands, 2010. [Google Scholar]
- Wu, W. CCHE2D Sediment Transport Model; Technical Report No. NCCHE-TR-2001-3, NCCHE; The University of Mississippi: Oxford, MS, USA, 2001. [Google Scholar]
- Papanicolaou, A.T.N.; Elhakeem, M.; Krallis, G.; Prakash, S.; Edinger, J. Sediment Transport Modeling Review-Current and Future Development. ASCE J. Hydraul. Eng. 2008, 134, 1–14. [Google Scholar] [CrossRef]
- Zhang, Y.; Jia, Y. Hybrid mesh generation using advancing reduction technique. Int. J. Numer. Methods Fluids 2015, 79, 514–535. [Google Scholar] [CrossRef]
- Zhang, Y.; Jia, Y.; Zhu, T.T. Edge Gradients Evaluation in 2D hybrid FVM Model. J. Hydraul. Res. 2015, 53, 423–439. [Google Scholar] [CrossRef]
- Julien, P.Y. Erosion and Deposition; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Cheng, N.S. Simplified settling velocity formula for sediment particle. J. Hydraulic Eng. ASCE 1997, 123, 149–152. [Google Scholar] [CrossRef]
- Einstein, H.A. The Bed-Load Function for Sediment Transportation in Open Channel Flows; Technical Bulletin No. 1026; U.S. Department of Agriculture, Soil Conservation Service: Washington, DC, USA, 1950.
- Bagnold, R.A. An Approach to the Sediment Transport Problem from General Physics; Professional Paper 422-J; USGS: Washington, DC, USA, 1966.
- Wu, W.; Wang, S.S.Y.; Jia, Y. Nonuniform sediment transport in alluvial rivers. J. Hydr. Res. IAHR 2000, 38, 427–434. [Google Scholar] [CrossRef]
- Wu, W.; Altinakar, M.; Wang, S.S.Y. Depth-average analysis of hysteresis between flow and sediment transport under unsteady conditions. Int. J. Sediment Res. 2006, 21, 101–112. [Google Scholar]
- Van Rijn, L.C. Sediment transport, part I: Bed-load transport. J. Hydraulic Eng. ASCE 1984, 110, 1431–1456. [Google Scholar] [CrossRef]
- Meyer-Peter, E.; Mueller, R. Formulas for bed-load transport. In Report on Second Meeting of IAHR; IAHR: Stockholm, Sweden, 1948; pp. 39–64. [Google Scholar]
- Engelund, F.; Hansen, E. A Monograph on Sediment Transport in Alluvial Streams; Teknisk Vorlag: Copenhagen, Denmark, 1967. [Google Scholar]
- Yalin, M.S. Mechanics of Sediment Transport; Pergamon Press: Oxford, UK, 1972. [Google Scholar]
- Galappatti, G.; Vreugdenhil, C.B. A depth-integrated model for suspended sediment transport. J. Hydr. Res. IAHR 1985, 23, 359–377. [Google Scholar] [CrossRef]
- Armanini, A.; di Silvio, G. Discussion on the paper ‘A depth-integrated model for suspended sediment transport’ by G. Galappatti and C.B. Vreugdenhil. J. Hydr. Res. 1986, 24, 437–441. [Google Scholar] [CrossRef]
- Karim, F.; Kennedy, J.F. IALLUVIAL: A Computer-Based Flow- and Sediment- Routing Model for Alluvial Streams and Its Application to the Missouri River; Technical Rep. No. 250; IIHR, Univ. of Iowa: Iowa City, IA, USA, 1982. [Google Scholar]
- Rhie, C.M.; Chow, W.L. Numerical study of the turbulent flow passed an airfoil with trailing edge separation. AIAA J. 1983, 21, 1525–1532. [Google Scholar] [CrossRef]
- Zhang, Y.; Jia, Y. Multi-point momentum interpolation correction on collocated meshes. J. Comput. Phys. 2021, 449, 110783. [Google Scholar] [CrossRef]
- Sleijpen, G.; Fokkema, D. BICGSTAB(L) For Linear Equations Involving Unsymmetric Matrices with Complex Spectrum. Electron. Trans. Numer. Anal. 1993, 1, 11–32. [Google Scholar]
- Seal, R.; Paola, C.; Parker, G.; Southard, J.B.; Wilcok, P.R. Experiments on downstream fining of gravel: I. Narrow-channel Runs. ASCE J. Hydraul. Eng. 1997, 123, 874–884. [Google Scholar] [CrossRef]
- Meade, R.H.; Myrick, R.M.; Emmett, W.W. Field data describing the movement and storage of sediment in the East Fork River. In Wyoming, Part II Bed Elevations, 1979; USGS Open-File Rep.; USGS: Denver, CO, USA, 1980; pp. 80–1190. [Google Scholar]
- Zhang, Y. CCHE-MESH 2D Structured and Unstructured Mesh Generator—Users’ Manual version 5.x; NCCHE-TR-2017-02; The University of Mississippi: Oxford, MS, USA, April 2017.

| Size (mm) | 0.25 | 0.35 | 0.5 | 0.7 | 0.9 | 1.25 | 1.75 | 2.5 | 3.5 | 5 | 7 | 9 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Lower Bound | 0.2 | 0.3 | 0.4 | 0.6 | 0.8 | 1 | 1.5 | 2 | 3 | 4 | 6 | 8 |
| Upper Bound | 0.3 | 0.4 | 0.6 | 0.8 | 1 | 1.5 | 2 | 3 | 4 | 6 | 8 | 10 |
| Fraction | 0.075 | 0.125 | 0.165 | 0.035 | 0.035 | 0.065 | 0.04 | 0.09 | 0.1 | 0.195 | 0.05 | 0.025 |
| Size (mm) | 0.125 | 0.25 | 0.5 | 1 | 2 | 4 | 8 | 16 | 32 | 64 |
|---|---|---|---|---|---|---|---|---|---|---|
| Fraction | 0.022 | 0.104 | 0.102 | 0.07 | 0.04 | 0.172 | 0.12 | 0.168 | 0.124 | 0.078 |
| Size (mm) | 0.088 | 0.177 | 0.354 | 0.707 | 1.41 | 2.83 | 5.66 | 11.3 | 32 |
|---|---|---|---|---|---|---|---|---|---|
| Fraction | 0.044 | 0.00038 | 0.02 | 0.478 | 0.233 | 0.145 | 0.093 | 0.02 | 0.01 |
| Size (mm) | 0.0272 | 0.297 | 2.38 | 9.52 | 152 | 457 |
|---|---|---|---|---|---|---|
| Lower Bound | 0.001 | 0.074 | 0.59 | 4.76 | 19.1 | 305 |
| Upper Bound | 0.074 | 0.59 | 4.76 | 19.1 | 305 | 610 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Al-Hamdan, M.; Wren, D. Development of a Two-Dimensional Hybrid Sediment-Transport Model. Appl. Sci. 2023, 13, 4940. https://doi.org/10.3390/app13084940
Zhang Y, Al-Hamdan M, Wren D. Development of a Two-Dimensional Hybrid Sediment-Transport Model. Applied Sciences. 2023; 13(8):4940. https://doi.org/10.3390/app13084940
Chicago/Turabian StyleZhang, Yaoxin, Mohammad Al-Hamdan, and Daniel Wren. 2023. "Development of a Two-Dimensional Hybrid Sediment-Transport Model" Applied Sciences 13, no. 8: 4940. https://doi.org/10.3390/app13084940
APA StyleZhang, Y., Al-Hamdan, M., & Wren, D. (2023). Development of a Two-Dimensional Hybrid Sediment-Transport Model. Applied Sciences, 13(8), 4940. https://doi.org/10.3390/app13084940










