Bilateral Asymmetry of Spatiotemporal Running Gait Parameters in U14 Athletes at Different Speeds
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Desing
2.2. Subjects
2.3. Procedures
Running Assessment
2.4. Statistical Analysis
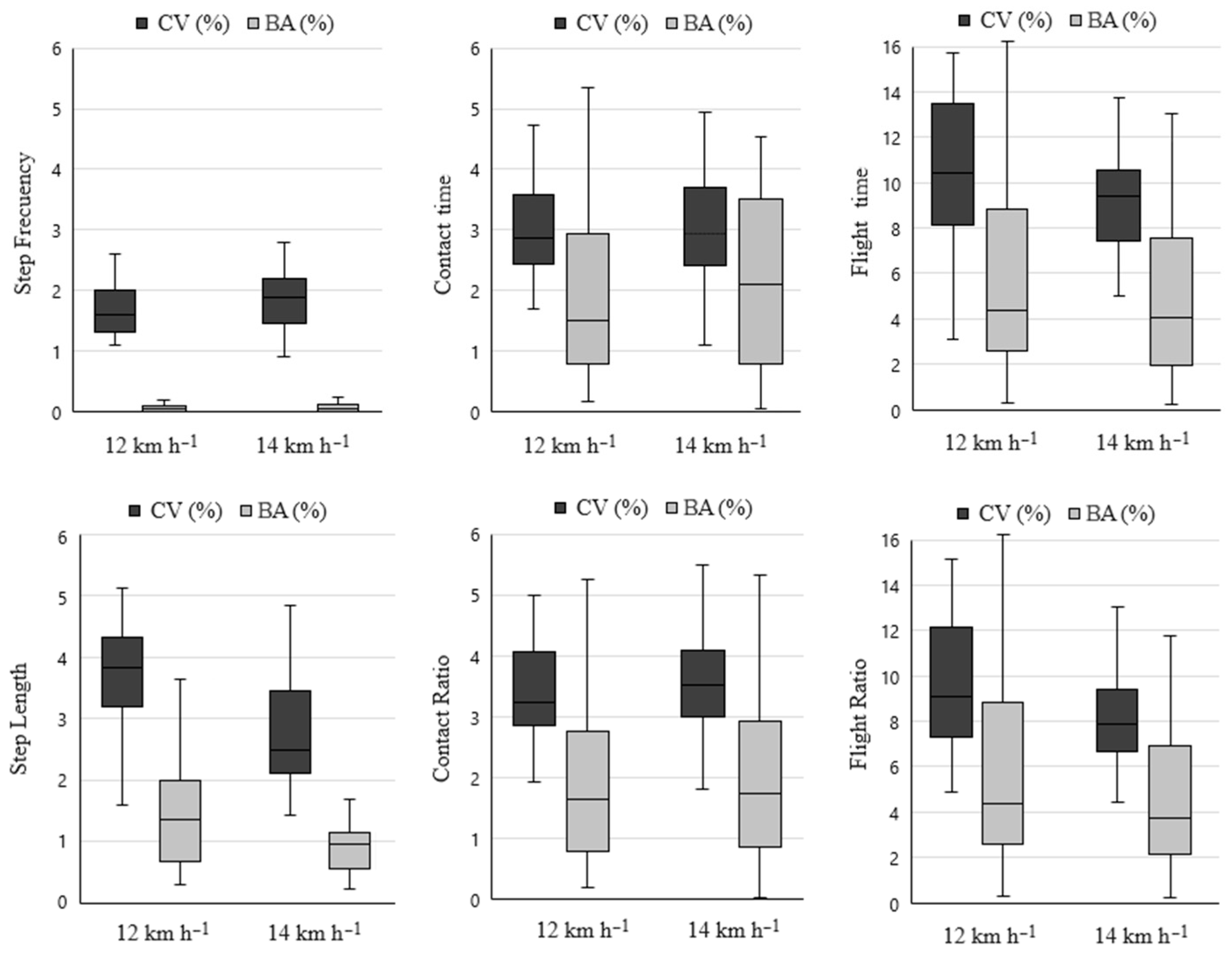
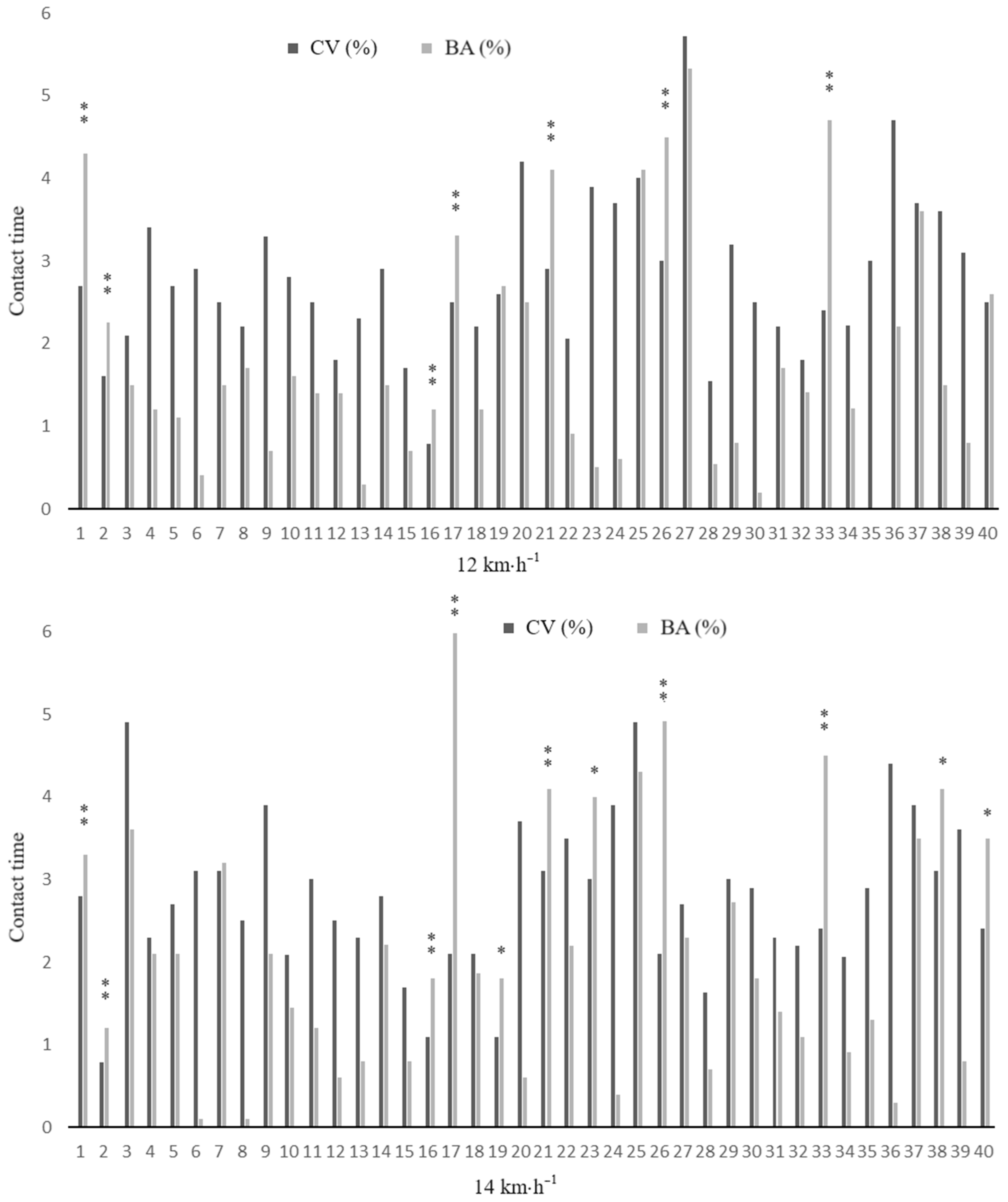
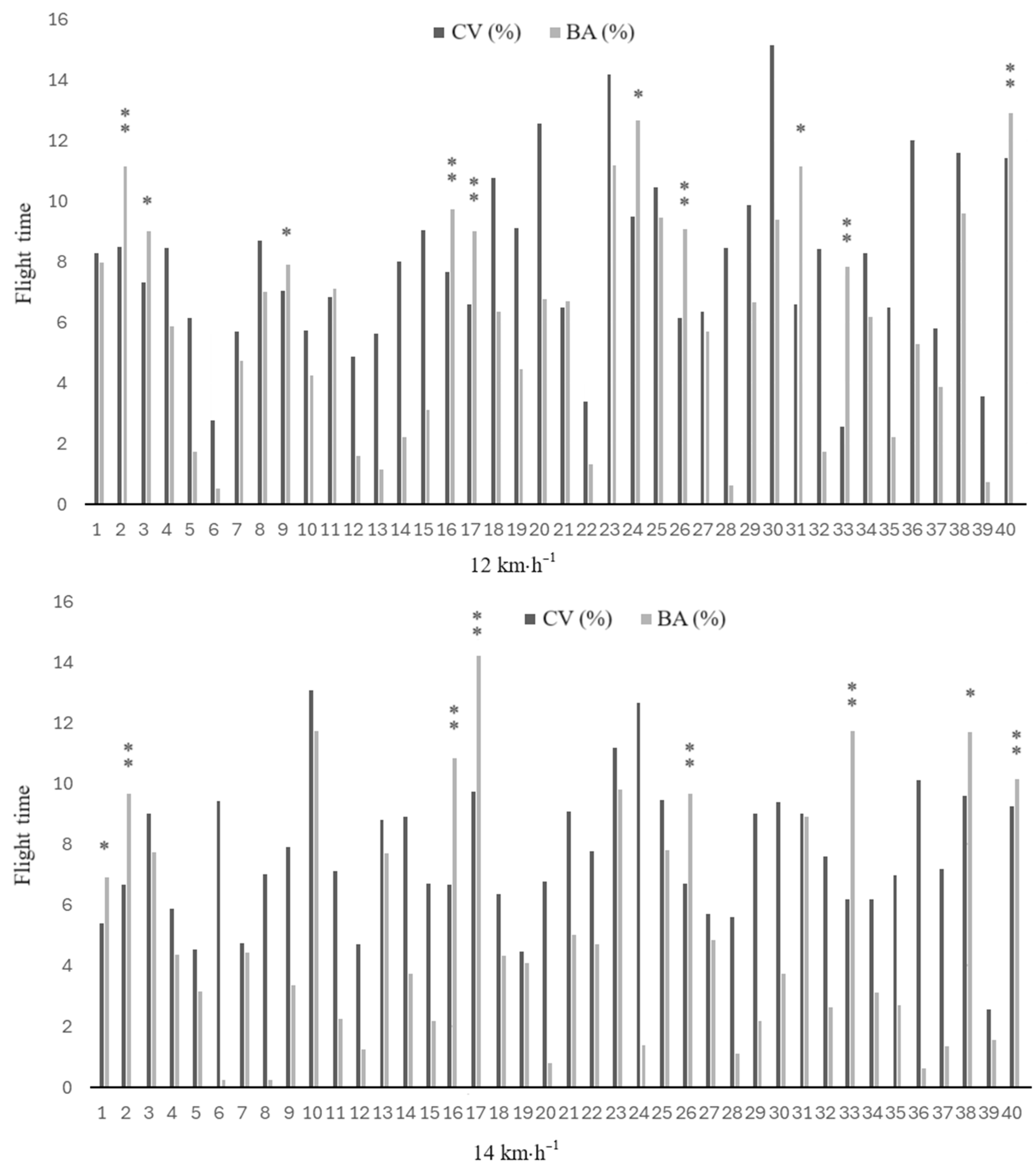
3. Results
4. Discussion
5. Practical Implications
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bishop, C.; Pereira, L.A.; Reis, V.P.; Read, P.; Turner, A.N.; Loturco, I. Comparing the Magnitude and Direction of Asymmetry during the Squat, Countermovement and Drop Jump Tests in Elite Youth Female Soccer Players. J. Sports Sci. 2020, 38, 1296–1303. [Google Scholar] [CrossRef] [PubMed]
- Mo, S.; Lau, F.O.Y.; Lok, A.K.Y.; Chan, Z.Y.S.; Zhang, J.H.; Shum, G.; Cheung, R.T.H. Bilateral Asymmetry of Running Gait in Competitive, Recreational and Novice Runners at Different Speeds. Hum. Mov. Sci. 2020, 71, 102600. [Google Scholar] [CrossRef]
- Trecroci, A.; Bongiovanni, T.; Cavaggioni, L.; Pasta, G.; Formenti, D.; Alberti, G. Agreement between Dribble and Change of Direction Deficits to Assess Directional Asymmetry in Young Elite Football Players. Symmetry 2020, 12, 787. [Google Scholar] [CrossRef]
- Ujaković, F.; Šarabon, N. Relationship between Asymmetries Measured on Different Levels in Elite Basketball Players. Symmetry 2021, 13, 1436. [Google Scholar] [CrossRef]
- Bishop, C.; Turner, A.; Read, P. Effects of Inter-Limb Asymmetries on Physical and Sports Performance: A Systematic Review. J. Sports Sci. 2018, 36, 1135–1144. [Google Scholar] [CrossRef]
- Chalmers, S.; Debenedictis, T.A.; Zacharia, A.; Townsley, S.; Gleeson, C.; Lynagh, M.; Townsley, A.; Fuller, J.T. Asymmetry during Functional Movement Screening and Injury Risk in Junior Football Players: A Replication Study. Scand. J. Med. Sci. Sports 2018, 28, 1281–1287. [Google Scholar] [CrossRef] [PubMed]
- Gilgen-Ammann, R.; Taube, W.; Wyss, T. Gait Asymmetry During 400- to 1000-m High-Intensity Track Running in Relation to Injury History. Int. J. Sports Physiol. Perform. 2017, 12, S2157–S2160. [Google Scholar] [CrossRef]
- Helme, M.; Tee, J.; Emmonds, S.; Low, C. Does Lower-Limb Asymmetry Increase Injury Risk in Sport? A Systematic Review. Phys. Ther. Sport 2021, 49, 204–213. [Google Scholar] [CrossRef]
- Keeley, D.W.; Plummer, H.A.; Oliver, G.D. Predicting Asymmetrical Lower Extremity Strength Deficits in College-Aged Men and Women Using Common Horizontal and Vertical Power Field Tests: A Possible Screening Mechanism. J. Strength Cond. Res. 2011, 25, 1632–1637. [Google Scholar] [CrossRef]
- Parkinson, A.O.; Apps, C.L.; Morris, J.G.; Barnett, C.T.; Lewis, M.G.C. The Calculation, Thresholds and Reporting of Inter-Limb Strength Asymmetry: A Systematic Review. J. Sports Sci. Med. 2021, 20, 594–617. [Google Scholar] [CrossRef]
- Bishop, C.; Read, P.; Chavda, S.; Turner, A. Asymmetries of the Lower Limb: The Calculation Conundrum in Strength Training and Conditioning. Strength Cond. J. 2016, 38, 27–32. [Google Scholar] [CrossRef]
- Kocak, U.Z.; Stiffler-Joachim, M.R.; Heiderscheit, B.C. Comparison of Eccentric Hamstring Strength and Asymmetry at Return-to-Sport after Hamstring Strain Injury among Those Who Did and Did Not Re-Injure. Phys. Ther. Sport 2023, 59, 25–29. [Google Scholar] [CrossRef] [PubMed]
- Loturco, I.; Pereira, L.A.; Kobal, R.; Cal Abad, C.C.; Fernandes, V.; Ramirez-Campillo, R.; Suchomel, T. Portable Force Plates: A Viable and Practical Alternative to Rapidly and Accurately Monitor Elite Sprint Performance. Sports 2018, 6, 61. [Google Scholar] [CrossRef] [PubMed]
- Bishop, C.; Turner, A.; Maloney, S.; Lake, J.; Loturco, I.; Bromley, T.; Read, P. Drop Jump Asymmetry Is Associated with Reduced Sprint and Change-of-Direction Speed Performance in Adult Female Soccer Players. Sports 2019, 7, 29. [Google Scholar] [CrossRef] [PubMed]
- Roso-Moliner, A.; Lozano, D.; Nobari, H.; Bishop, C.; Carton-Llorente, A.; Mainer-Pardos, E. Horizontal Jump Asymmetries Are Associated with Reduced Range of Motion and Vertical Jump Performance in Female Soccer Players. BMC Sports Sci. Med. Rehabil. 2023, 15, 80. [Google Scholar] [CrossRef] [PubMed]
- Girard, O.; Alsenoy, K.V.; Li, S.N.; Ryu, J.H.; Peeling, P. Constant Low-to-Moderate Mechanical Asymmetries during a Treadmill Graded Exercise Test. Eur. J. Sport Sci. 2022, 22, 530–538. [Google Scholar] [CrossRef] [PubMed]
- Girard, O.; Millet, G.P.; Micallef, J.P. Constant Low-to-Moderate Mechanical Asymmetries during 800-m Track Running. Front. Sports Act. Living 2024, 6, 1278454. [Google Scholar] [CrossRef] [PubMed]
- Mtibaa, K.; Zarrouk, N.; Ryu, J.H.; Racinais, S.; Girard, O. Mechanical Asymmetries Remain Low-to-Moderate during 30 Min of Self-Paced Treadmill Running. Front. Physiol. 2023, 14, 1289172. [Google Scholar] [CrossRef]
- Furlong, L.M.; Egginton, N.L. Kinetic Asymmetry during Running at Preferred and Nonpreferred Speeds. Med. Sci. Sports Exerc. 2018, 50, 1241–1248. [Google Scholar] [CrossRef]
- Liu, Q.; Chen, H.; Song, Y.; Alla, N.; Fekete, G.; Li, J.; Gu, Y. Running Velocity and Longitudinal Bending Stiffness Influence the Asymmetry of Kinematic Variables of the Lower Limb Joints. Bioengineering 2022, 9, 607. [Google Scholar] [CrossRef]
- Jiang, X.; Chen, H.; Sun, D.; Baker, J.S.; Gu, Y. Running Speed Does Not Influence the Asymmetry of Kinematic Variables of the Lower Limb Joints in Novice Runners. Acta Bioeng. Biomech. 2021, 23, 69–81. [Google Scholar]
- Giakas, G.; Baltzopoulos, V. Time and Frequency Domain Analysis of Ground Reaction Forces during Walking: An Investigation of Variability and Symmetry. Gait Posture 1997, 5, 189–197. [Google Scholar] [CrossRef]
- Exell, T.A.; Irwin, G.; Gittoes, M.J.; Kerwin, D.G. Implications of Intra-Limb Variability on Asymmetry Analyses. J. Sports Sci. 2012, 30, 403–409. [Google Scholar] [CrossRef]
- Korhonen, M.T.; Suominen, H.; Viitasalo, J.T.; Liikavainio, T.; Alen, M.; Mero, A.A. Variability and Symmetry of Force Platform Variables in Maximum-Speed Running in Young and Older Athletes. J. Appl. Biomech. 2010, 26, 357–366. [Google Scholar] [CrossRef]
- Kung, S.M.; Fink, P.W.; Legg, S.J.; Ali, A.; Shultz, S.P. Age-Dependent Variability in Spatiotemporal Gait Parameters and the Walk-to-Run Transition. Hum. Mov. Sci. 2019, 66, 600–606. [Google Scholar] [CrossRef]
- Komar, J.; Seifert, L.; Thouvarecq, R. What Variability Tells Us about Motor Expertise: Measurements and Perspectives from a Complex System Approach. Mov. Sport Sci.-Sci. Mot. 2015, 89, 65–77. [Google Scholar] [CrossRef]
- Eston, R. Anthropometry. In Kinanthropometry and Exercise Physiology Laboratory Manual: Tests, Procedures and Data, 3rd ed.; Routledge: Abingdon, UK, 2008; Volume 1. [Google Scholar]
- Mirwald, R.L.; Baxter-Jones, A.D.; Bailey, D.A.; Beunen, G.P. An Assessment of Maturity from Anthropometric Measurements. Med. Sci. Sports Exerc. 2002, 34, 689–694. [Google Scholar] [CrossRef]
- Lavcanska, V.; Taylor, N.F.; Schache, A.G. Familiarization to Treadmill Running in Young Unimpaired Adults. Hum. Mov. Sci. 2005, 24, 544–557. [Google Scholar] [CrossRef]
- Koldenhoven, R.M.; Hertel, J. Validation of a Wearable Sensor for Measuring Running Biomechanics. Digit. Biomark. 2018, 2, 74–78. [Google Scholar] [CrossRef]
- Koo, T.K.; Li, M.Y. A Guideline of Selecting and Reporting Intraclass Correlation Coefficients for Reliability Research. J. Chiropr. Med. 2016, 15, 155–163. [Google Scholar] [CrossRef]
- Bishop, C.; Read, P.; Lake, J.; Chavda, S.; Turner, A. Interlimb Asymmetries: Understanding How to Calculate Differences From Bilateral and Unilateral Tests. Strength Cond. J. 2018, 40, 1–6. [Google Scholar] [CrossRef]
- Hopkins, W.G.; Marshall, S.W.; Batterham, A.M.; Hanin, J. Progressive Statistics for Studies in Sports Medicine and Exercise Science. Med. Sci. Sports Exerc. 2009, 41, 3–13. [Google Scholar] [CrossRef]
- Turner, A.; Brazier, J.; Bishop, C.; Chavda, S.; Cree, J.; Read, P. Data Analysis for Strength and Conditioning Coaches: Using Excel to Analyze Reliability, Differences, and Relationships. Strength Cond. J. 2015, 37, 76–83. [Google Scholar] [CrossRef]
- Hollis, C.R.; Koldenhoven, R.M.; Resch, J.E.; Hertel, J. Running Biomechanics as Measured by Wearable Sensors: Effects of Speed and Surface. Sports Biomech. 2021, 20, 521–531. [Google Scholar] [CrossRef]
- Kozinc, Ž.; Smajla, D.; Šarabon, N. The Reliability of Wearable Commercial Sensors for Outdoor Assessment of Running Biomechanics: The Effect of Surface and Running Speed. Sports Biomech. 2022, 1–14. [Google Scholar] [CrossRef]
- García-Pinillos, F.; Latorre-Román, P.Á.; Soto-Hermoso, V.M.; Párraga-Montilla, J.A.; Pantoja-Vallejo, A.; Ramírez-Campillo, R.; Roche-Seruendo, L.E. Agreement between the Spatiotemporal Gait Parameters from Two Different Wearable Devices and High-Speed Video Analysis. PLoS ONE 2019, 14, e0222872. [Google Scholar] [CrossRef]
- DeJong, A.F.; Hertel, J. Validation of Foot-Strike Assessment Using Wearable Sensors During Running. J. Athl. Train. 2020, 55, 1307–1310. [Google Scholar] [CrossRef]
- Zifchock, R.A.; Davis, I.; Hamill, J. Kinetic Asymmetry in Female Runners with and without Retrospective Tibial Stress Fractures. J. Biomech. 2006, 39, 2792–2797. [Google Scholar] [CrossRef]
- Zifchock, R.A.; Davis, I.; Higginson, J.; Royer, T. The Symmetry Angle: A Novel, Robust Method of Quantifying Asymmetry. Gait Posture 2008, 27, 622–627. [Google Scholar] [CrossRef] [PubMed]
- Zifchock, R.A.; Davis, I.; Higginson, J.; McCaw, S.; Royer, T. Side-to-Side Differences in Overuse Running Injury Susceptibility: A Retrospective Study. Hum. Mov. Sci. 2008, 27, 888–902. [Google Scholar] [CrossRef] [PubMed]
- Haugen, T.; Danielsen, J.; McGhie, D.; Sandbakk, Ø.; Ettema, G. Kinematic Stride Cycle Asymmetry Is Not Associated with Sprint Performance and Injury Prevalence in Athletic Sprinters. Scand. J. Med. Sci. Sports 2018, 28, 1001–1008. [Google Scholar] [CrossRef]
- Malisoux, L.; Gette, P.; Delattre, N.; Urhausen, A.; Theisen, D. Gait Asymmetry in Spatiotemporal and Kinetic Variables Does Not Increase Running-Related Injury Risk in Lower Limbs: A Secondary Analysis of a Randomised Trial Including 800+ Recreational Runners. BMJ Open Sport Exerc. Med. 2024, 10, e001787. [Google Scholar] [CrossRef]
- Girard, O.; Morin, J.-B.; Ryu, J.; Read, P.; Townsend, N. Running Velocity Does Not Influence Lower Limb Mechanical Asymmetry. Front. Sports Act. Living 2019, 1, 36. [Google Scholar] [CrossRef]
- Ducharme, S.W.; Turner, D.S.; Pleuss, J.D.; Moore, C.C.; Schuna, J.M.; Tudor-Locke, C.; Aguiar, E.J. Using Cadence to Predict the Walk-to-Run Transition in Children and Adolescents: A Logistic Regression Approach. J. Sports Sci. 2021, 39, 1039–1045. [Google Scholar] [CrossRef]
- Blickhan, R. The Spring-Mass Model for Running and Hopping. J. Biomech. 1989, 22, 1217–1227. [Google Scholar] [CrossRef]
- Millet, G.Y.; Morin, J.B.; Degache, F.; Edouard, P.; Feasson, L.; Verney, J.; Oullion, R. Running from Paris to Beijing: Biomechanical and Physiological Consequences. Eur. J. Appl. Physiol. 2009, 107, 731–738. [Google Scholar] [CrossRef]
- van Oeveren, B.T.; de Ruiter, C.J.; Beek, P.J.; van Dieën, J.H. The Biomechanics of Running and Running Styles: A Synthesis. Sports Biomech. 2024, 23, 516–554. [Google Scholar] [CrossRef]
- Exell, T.; Irwin, G.; Gittoes, M.; Kerwin, D. Strength and Performance Asymmetry during Maximal Velocity Sprint Running. Scand. J. Med. Sci. Sport. 2017, 27, 1273–1282. [Google Scholar] [CrossRef]
- Van Hooren, B.; Fuller, J.T.; Buckley, J.D.; Miller, J.R.; Sewell, K.; Rao, G.; Barton, C.; Bishop, C.; Willy, R.W. Is Motorized Treadmill Running Biomechanically Comparable to Overground Running? A Systematic Review and Meta-Analysis of Cross-Over Studies. Sports Med. 2020, 50, 785–813. [Google Scholar] [CrossRef]

| Variable | ||
|---|---|---|
| Sex | Male | 18 (45%) |
| Female | 22 (55%) | |
| Age (years) | 12.2 ± 0.6 | |
| Height (cm) | 155.68 ± 7.02 | |
| Body Mass (kg) | 40.1 ± 7.0 | |
| BMI (kg/m2) | 17.4 ± 1.7 | |
| Maturity Offset (years) | −0.67 ± 1.30 | |
| Ankle dorsiflexion (°) | 45.0 ± 8.4 | |
| Leg length asymmetry (%) | 0.8 ± 0.8 |
| Speed | Variables | Leg | Mean (SD) | CV (%) | ICC (95% CI) | BA (%) | p-Value |
|---|---|---|---|---|---|---|---|
| 12 km·h−1 | SF (spm) | Right Left | 177.4 ± 7.3 177.6 ± 7.3 | 1.7 1.7 | 0.96 (0.93–0.98) 0.94 (0.92–0.97) | 0.1 | 0.712 |
| SL (cm) | Right Left | 111.7 ± 5.0 112.0 ± 4.9 | 3.7 3.9 | 0.88 (0.78–0.94) 0.86 (0.83–0.91) | 1.5 | 0.442 | |
| CT (ms) | Right Left | 248 ± 16 249 ± 15 | 3.0 2.9 | 0.92 (0.87–0.95) 0.92 (0.86–0.95) | 2.0 | 0.311 | |
| FT (ms) | Right Left | 92 ± 21 91 ± 21 | 10.2 10.7 | 0.98 (0.96–0.99) 0.96 (0.92–0.98) | 5.5 | 0.223 | |
| CR (%) | Right Left | 73.2 ± 5.6 73.5 ± 5.5 | 3.4 3.4 | 0.93 (0.88–0.96) 0.94 (0.89–0.97) | 2.1 | 0.428 | |
| FR (%) | Right Left | 26.8 ± 5.6 26.5 ± 5.5 | 9.6 9.9 | 0.78 (0.56–0.88) 0.79 (0.60–0.86) | 5.7 | 0.402 | |
| 14 km·h−1 | SF (spm) | Right Left | 181.5 ± 8.5 181.4 ± 8.5 | 1.9 1.9 | 0.94 (0.88–0.97) 0.94 (0.92–0.98) | 0.1 | 0.482 |
| SL (cm) | Right Left | 127.3 ± 6.1 127.2 ± 6.4 | 3.5 3.5 | 0.91 (0.85–0.95) 0.90 (0.83–0.98) | 1.0 | 0.575 | |
| CT (ms) | Right Left | 229 ± 14 229 ± 13 | 3.0 3.0 | 0.94 (0.88–0.97) 0.95 (0.93–0.98) | 2.0 | 0.980 | |
| FT (ms) | Right Left | 103 ± 21 103 ± 20 | 9.3 8.9 | 0.97 (0.94–0.99) 0.96 (0.93–0.98) | 4.8 | 0.990 | |
| CR (%) | Right Left | 69.3 ± 5.4 69.2 ± 4.9 | 3.5 3.5 | 0.93 (0.86–0.96) 0.93 (0.90–0.98) | 2.2 | 0.826 | |
| FR (%) | Right Left | 30.7 ± 5.4 30.8 ± 5.0 | 8.1 7.9 | 0.83 (0.70–0.91) 0.86 (0.63–0.96) | 5.2 | 0.888 |
| Variables | 12 km·h−1 | 14 km·h−1 | 12 vs. 14 km·h−1 (p-Value (ES)) | ||
|---|---|---|---|---|---|
| p-Value (ES) | CV | BA | |||
| SF (spm) | 177.5 ± 7.3 | 181.5 ± 8.5 | 0.013 * (0.6) | 0.177 (0.3) | 0.592 (0.3) |
| SL (cm) | 111.8 ± 5.0 | 127.3 ± 6.3 | <0.001 ** (3.0) | 0.349 (0.2) | 0.797 (0.1) |
| CT (ms) | 249 ± 16 | 229 ± 14 | <0.001 ** (1.3) | 0.928 (0.0) | 0.367 (0.2) |
| FT (ms) | 91 ± 21 | 103 ± 21 | <0.001 ** (0.5) | 0.003 * (0.4) | 0.817 (0.0) |
| CR (%) | 73.4 ± 5.6 | 69.3 ± 5.2 | <0.001 ** (0.7) | 0.576 (0.1) | 0.786 (0.1) |
| FR (%) | 26.7 ± 5.6 | 30.7 ± 5.3 | <0.001 ** (0.7) | 0.002 * (0.6) | 0.497 (0.2) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cartón-Llorente, A.; Cardiel-Sánchez, S.; Molina-Molina, A.; Ráfales-Perucha, A.; Rubio-Peirotén, A. Bilateral Asymmetry of Spatiotemporal Running Gait Parameters in U14 Athletes at Different Speeds. Sports 2024, 12, 117. https://doi.org/10.3390/sports12050117
Cartón-Llorente A, Cardiel-Sánchez S, Molina-Molina A, Ráfales-Perucha A, Rubio-Peirotén A. Bilateral Asymmetry of Spatiotemporal Running Gait Parameters in U14 Athletes at Different Speeds. Sports. 2024; 12(5):117. https://doi.org/10.3390/sports12050117
Chicago/Turabian StyleCartón-Llorente, Antonio, Silvia Cardiel-Sánchez, Alejandro Molina-Molina, Andrés Ráfales-Perucha, and Alberto Rubio-Peirotén. 2024. "Bilateral Asymmetry of Spatiotemporal Running Gait Parameters in U14 Athletes at Different Speeds" Sports 12, no. 5: 117. https://doi.org/10.3390/sports12050117
APA StyleCartón-Llorente, A., Cardiel-Sánchez, S., Molina-Molina, A., Ráfales-Perucha, A., & Rubio-Peirotén, A. (2024). Bilateral Asymmetry of Spatiotemporal Running Gait Parameters in U14 Athletes at Different Speeds. Sports, 12(5), 117. https://doi.org/10.3390/sports12050117









