On the Suspension Design of Paquitop, a Novel Service Robot for Home Assistance Applications
Abstract
:1. Introduction
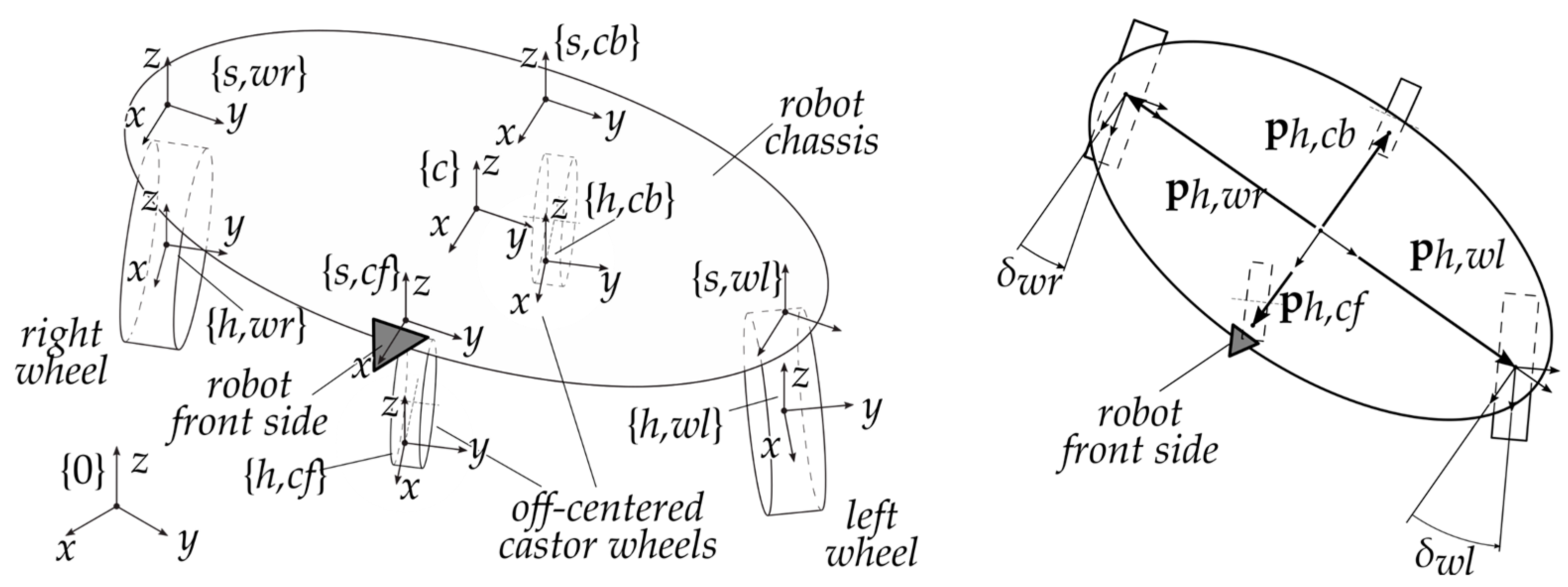
2. Platform Mobility
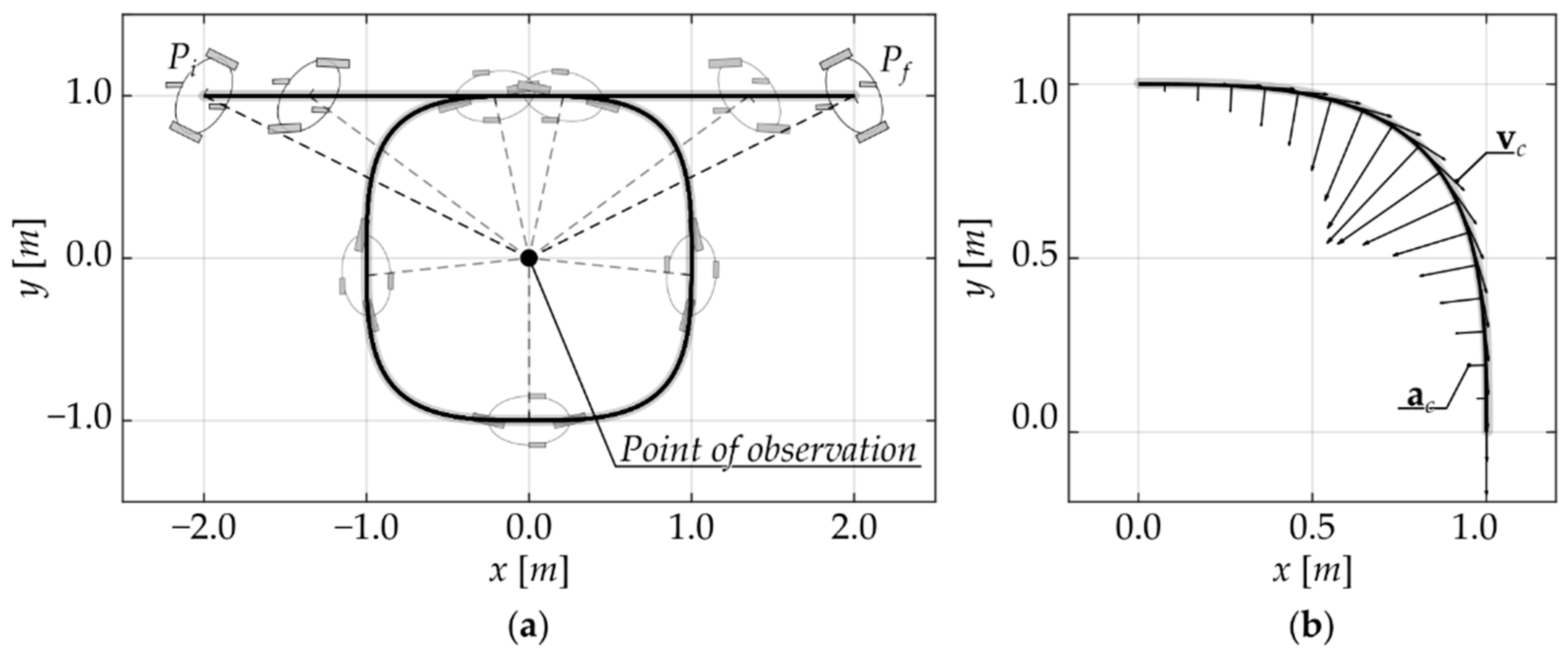
- Configuration I: adopting different steering angles , the robot exploits all its degrees of actuation to achieve a general motion in the plane.
- Configuration II: when the axes of the two driven wheels are parallel, but not or , the robot is able to translate while rotations are inhibited. Assuming Equation (2) imposes that the angular velocities and are equal. In this configuration, the platform is able to translate while maintaining a fixed direction of observation.
- Configuration III: when the steering angles of the driven wheel are zero, the mobility of the robot corresponds to the well-known scenario of a differential actuation. Equation (2) is fulfilled whatever angular velocity is provided. Consequently, the robot is able to exhibit an angular velocity but loses the possibility of owning a -directed velocity in .
- Configuration IV: when the steering angles are equal to , the configuration of the robot is very similar to Configuration II with driven axes parallel and not coincident. However, the platform exhibits improvedstatic and dynamic performance which enables high accelerations of the robot. Besides, a small variation to just one of the two wheels, or opposed small angles around , would also allow the robot to approach curved trajectories without relevant modifications to its ability to absorb higher accelerations in that direction.
2.1. Kinematics
2.2. Simplified Dynamics Model
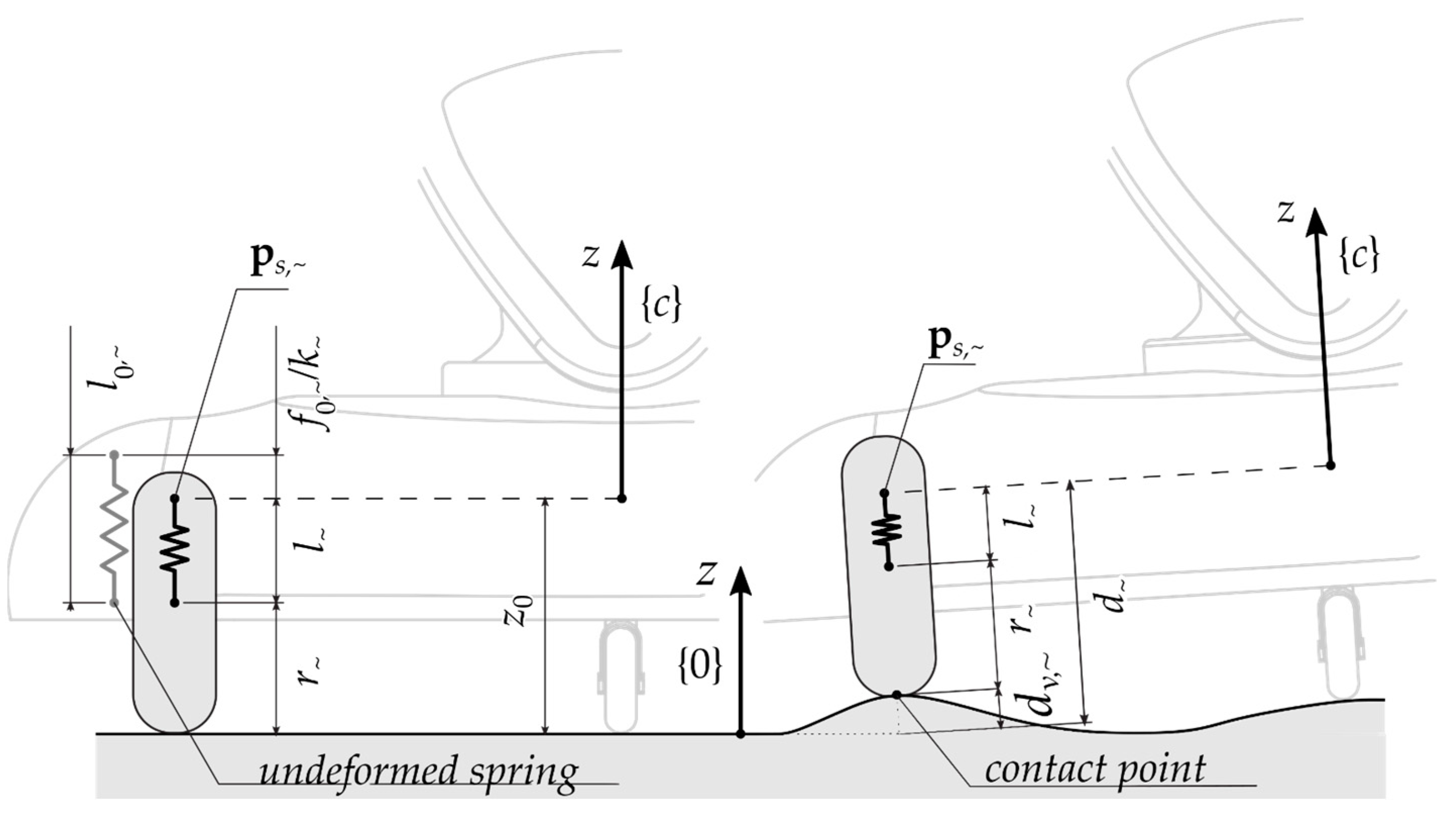
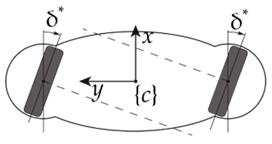
2.3. Castor Wheel’s Offset Effect
3. Functional Suspension Design
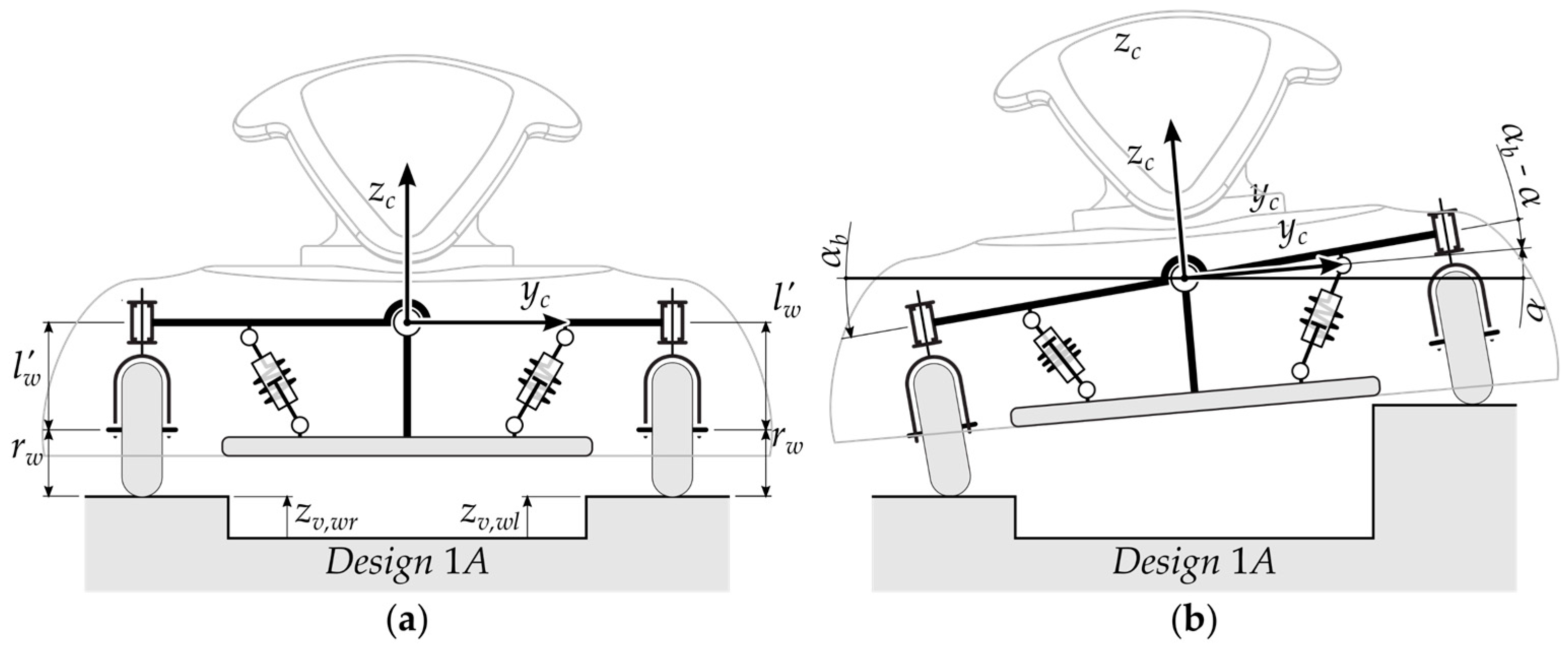
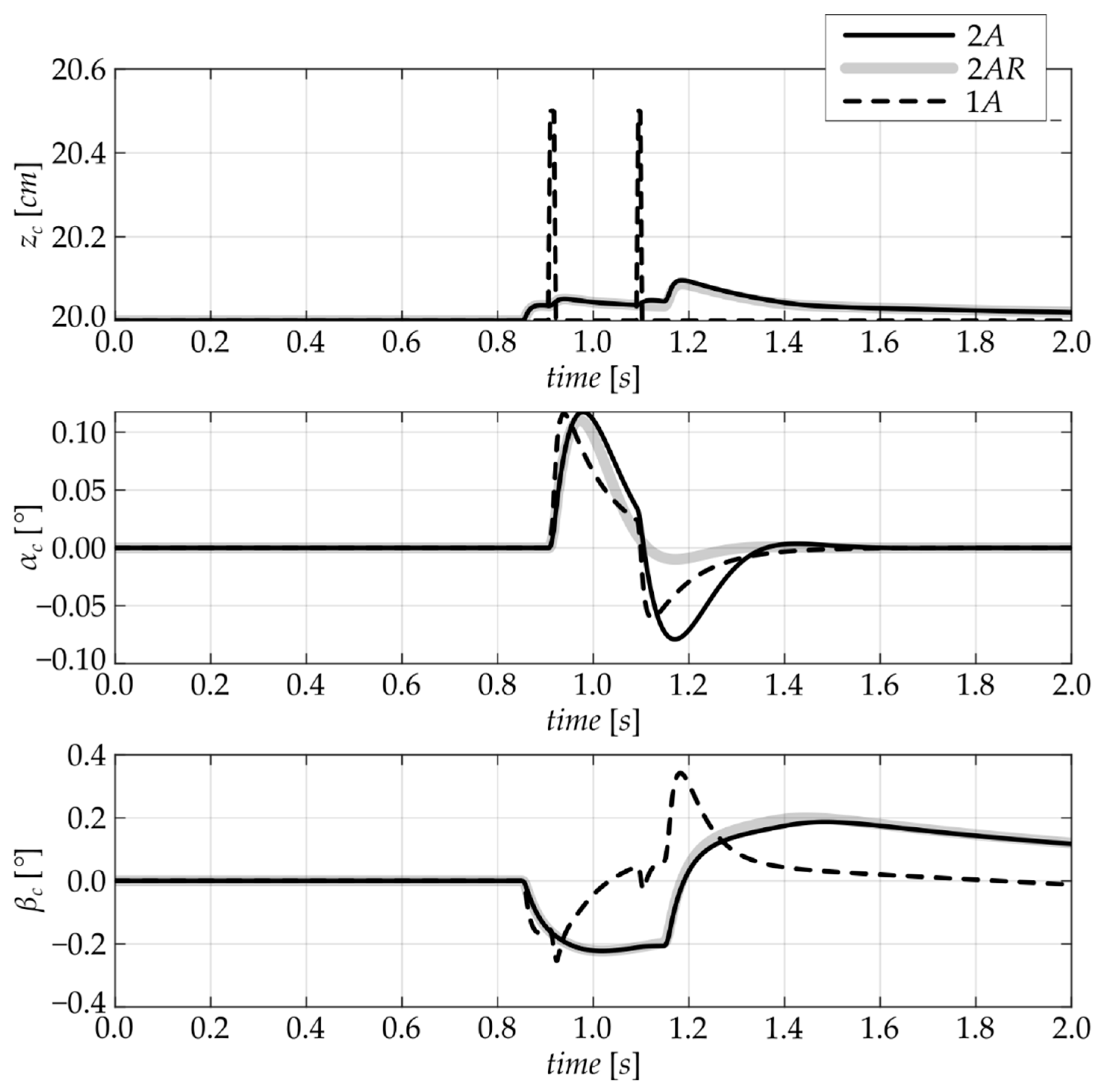
3.1. Two Arms Suspensions: Design: 2A
3.2. Suspension Design: 2AR, Configuration 2A with a Generic Anti-Rool Device
3.3. One Arm Suspensions: Design 1A
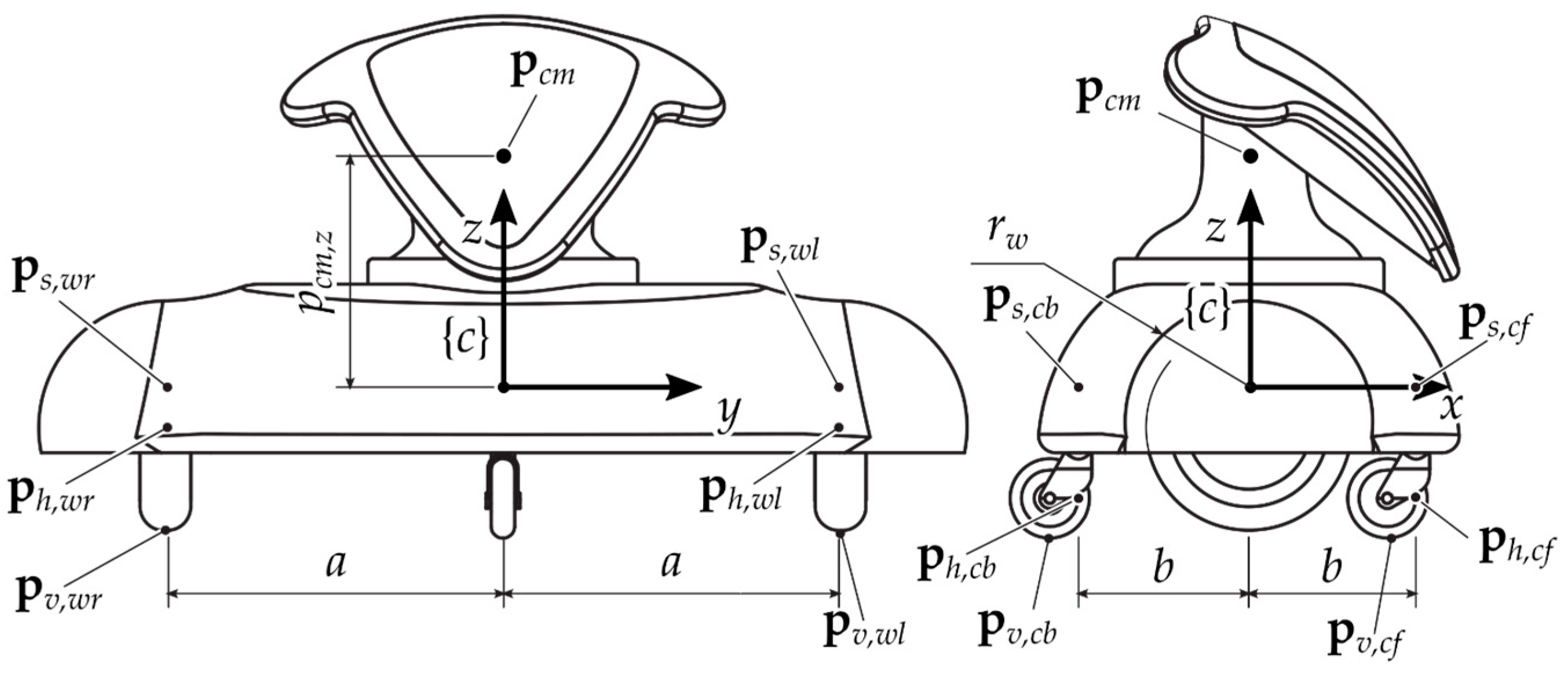
3.4. Parameter Selection
- Robot geometry: at this point of the design process, the geometry of the chassis is not precisely defined yet. The dimensions used here are hypothesized accordingly to the need of allowing the platform to work in a human-sized environment. Due to that, it is chosen , which provides the robot with a footprint similar to that of a person. The chassis has been supposed elliptical. The whole robot body was approximated by the prism built on such footprint, with a total eight of
- Robot mass and inertia: the inertial properties of the robot were estimated supposing a homogeneous mass distribution within the elliptic prism. With a mass , the principal inertia moments are: , , . Given the shape and the uniform mass distribution, the centre mass is elevated by with respect to the origin of r.f. .
- Robot suspension parameters: the analyses have been performed under the hypotheses of symmetrical left-right and front-back suspensions; therefore, it was considered:
- Adopting the dynamic optimization method proposed in [21], the suspension parameters for the design 2A are evaluated to optimize the pitch roll dynamics, as suggested by the non-axisymmetric aspect of the robot which will produce remarkable out of plane rotations. In this way, the parameters of the suspensions turn to be ; ; ; . Concerning the other configurations of the suspensions system, parameters of the same order of magnitude of the respective parameters for the 2A design were selected: ; ; . For the succeeding analyses, the suspensions were set up so that and . Such assumption is made because the passive wheels have been introduced as a simple solution for providing stability to a two-wheel platform without the need of complex self-balancing strategies. In this perspective, the additional contact points with the ground, provided by the passive wheels, become active only when the platform starts pitching, but no loads are applied on the castor wheels at zero velocity. The parameters are recapped in Table 2.
4. Simulation Results
4.1. Case Study 1: The Curved Path
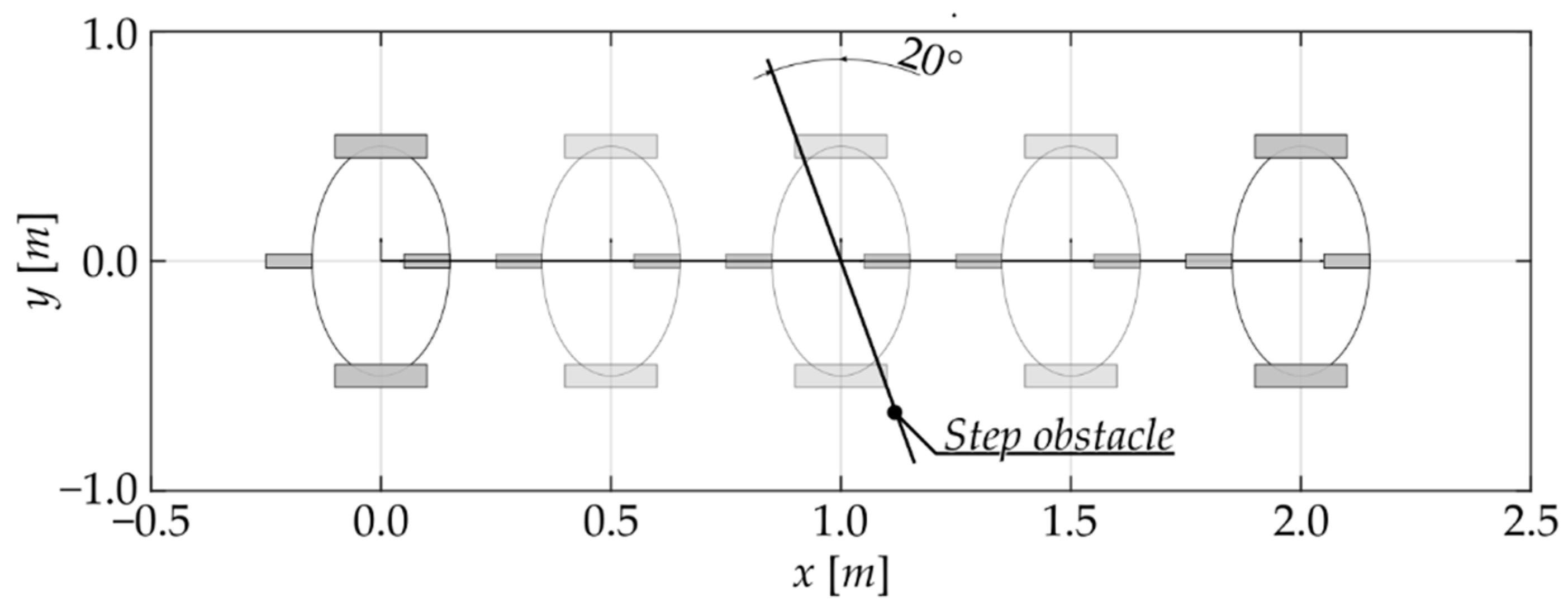
4.2. Case Study 2: Straight Path with an Obstacle
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Romero-Garcés, A.; Martínez-Cruz, J.; Inglés-Romero, J.F.; Vicente-Chicote, C.; Marfil, R.; Bandera, A. Measuring quality of service in a robotized comprehensive geriatric assessment scenario. Appl. Sci. 2020, 10, 6618. [Google Scholar]
- Yang, G.Z.; Nelson, B.J.; Murphy, R.R.; Choset, H.; Christensen, H.; Collins, S.H.; McNutt, M. Combating COVID-19—The role of robotics in managing public health and infectious diseases. Sci. Robot. 2020, 5589. [Google Scholar] [CrossRef] [Green Version]
- Rosolia, U.; Zhang, X.; Borrelli, F. Data-driven predictive control for autonomous systems. Annu. Rev. Control Robot. Auton. Syst. 2018, 1, 259–286. [Google Scholar] [CrossRef]
- Gia Luan, P.; Thinh, N.T. Real-time hybrid navigation system-based path planning and obstacle avoidance for mobile robots. Appl. Sci. 2020, 10, 3355. [Google Scholar] [CrossRef]
- Jung, J.W.; Park, J.S.; Kang, T.W.; Kang, J.G.; Kang, H.W. Mobile robot path planning using a laser range finder for environments with transparent obstacles. Appl. Sci. 2020, 10, 2799. [Google Scholar] [CrossRef] [Green Version]
- Aguiar, A.; Santos, F.; Sousa, A.J.; Santos, L. FAST-FUSION: An improved accuracy omnidirectional visual odometry system with sensor fusion and gpu optimization for embedded low cost hardware. Appl. Sci. 2019, 9, 5516. [Google Scholar] [CrossRef] [Green Version]
- Juel, W.K.; Haarslev, F.; Ram’ırez, E.R.; Marchetti, E.; Fischer, K.; Shaikh, D.; Manoonpong, P.; Hauch, C.; Bodenhagen, L.; Kruger, N. Smooth robot: Design for a novel modular welfare robot. J. Intell. Robot. Syst. 2020, 98, 19–37. [Google Scholar] [CrossRef]
- Ito, S.; Sugiura, S.; Masuda, Y.; Nohara, S.; Morita, R. Mechanism and control of a one-actuator mobile robot incorporating a torque limiter. J. Intell. Robot. Syst. 2020, 97, 431–448. [Google Scholar] [CrossRef]
- Rivera, Z.B.; De Simone, M.C.; Guida, D. Unmanned ground vehicle modelling in Gazebo/ROS-based environments. Machines 2019, 7, 42. [Google Scholar] [CrossRef] [Green Version]
- Vasiliev, A.; Dalyaev, I. Simulation Method for the Transport System of a Small-Sized Reconfigurable Mobile Robot. Machines 2021, 9, 8. [Google Scholar] [CrossRef]
- DeSantis, R.M. Modeling and path-tracking control of a mobile wheeled robot with a differential drive. Robotica 1995, 13, 401–410. [Google Scholar] [CrossRef]
- Chung, Y.; Park, C.; Harashima, F. A position control differential drive wheeled mobile robot. IEEE Trans. Ind. Electron. 2001, 48, 853–863. [Google Scholar] [CrossRef]
- Chwa, D. Tracking control of differential-drive wheeled mobile robots using a backstepping-like feed-back linearization. IEEE Trans. Syst. Man Cybern. Part A Syst. Hum. 2010, 40, 1285–1295. [Google Scholar] [CrossRef]
- Bruzzone, L.; Baggetta, M.; Nodehi, S.E.; Bilancia, P.; Fanghella, P. Functional Design of a Hybrid Leg-Wheel-Track Ground Mobile Robot. Machines 2021, 9, 10. [Google Scholar] [CrossRef]
- Korayem, M.H.; Ghariblu, H. Analysis of wheeled mobile flexible manipulator dynamic motions with maximum load carrying capacities. Robot. Auton. Syst. 2004, 48, 63–76. [Google Scholar] [CrossRef]
- White, G.D.; Bhatt, R.M.; Tang, C.P.; Krovi, V.N. Experimental evaluation of dynamic redundancy resolution in a nonholonomic wheeled mobile manipulator. IEEE/ASME Trans. Mechatron. 2009, 14, 349–357. [Google Scholar] [CrossRef]
- Dietrich, A.; Wimbock, T.; Albu-Schaffer, A.; Hirzinger, G. Reactive whole-body control: Dynamic mobile manipulation using a large number of actuated degrees of freedom. IEEE Robot. Autom. Mag. 2012, 19, 20–33. [Google Scholar] [CrossRef] [Green Version]
- Carbonari, L.; Botta, A.; Cavallone, P.; Quaglia, G. Functional design of a novel over-actuated mobile robotic platform for assistive tasks. In Proceedings of the International Conference on Robotics in Alpe-Adria Danube Region, Cham, Switzerland, 17–19 June 2020; pp. 380–389. [Google Scholar]
- Iagnemma, K.; Rzepniewski, A.; Dubowsky, S.; Schenker, P. Control of robotic vehicles with actively articulated suspensions in rough terrain. Auton. Robot. 2003, 14, 5–16. [Google Scholar] [CrossRef]
- Jiang, H.; Xu, G.; Zeng, W.; Gao, F.; Chong, K. Lateral stability of a mobile robot utilizing an active ad-justable suspension. Appl. Sci. 2019, 9, 4410. [Google Scholar] [CrossRef] [Green Version]
- Carbonari, L.; Botta, A.; Tagliavini, L.; Cavallone, P.; Quaglia, G. Dynamics characterization of paquitop, a novel platform for robotized domestic applications. In Proceedings of the IMECE International Mechanical Engineering Congress & Exposition, 16–19 November 2020. [Google Scholar]
- Tseng, H.E.; Hrovat, D. State of the art survey: Active and semi-active suspension control. Veh. Syst. Dyn. 2015, 53, 1034–1062. [Google Scholar] [CrossRef]



| Configuration I | Configuration II |
 |  |
| Configuration III | Configuration IV |
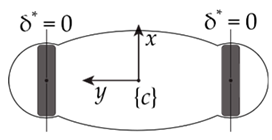 | 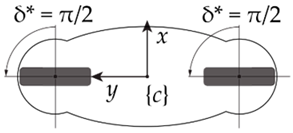 |
| Parameter | Value | Units | Parameter | Value | Units |
|---|---|---|---|---|---|
| 0.250 | |||||
| 0.150 | |||||
| 0.400 | |||||
| 0.015 | |||||
| 0.050 | |||||
| 0.040 | |||||
| 0.200 | m |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tagliavini, L.; Botta, A.; Cavallone, P.; Carbonari, L.; Quaglia, G. On the Suspension Design of Paquitop, a Novel Service Robot for Home Assistance Applications. Machines 2021, 9, 52. https://doi.org/10.3390/machines9030052
Tagliavini L, Botta A, Cavallone P, Carbonari L, Quaglia G. On the Suspension Design of Paquitop, a Novel Service Robot for Home Assistance Applications. Machines. 2021; 9(3):52. https://doi.org/10.3390/machines9030052
Chicago/Turabian StyleTagliavini, Luigi, Andrea Botta, Paride Cavallone, Luca Carbonari, and Giuseppe Quaglia. 2021. "On the Suspension Design of Paquitop, a Novel Service Robot for Home Assistance Applications" Machines 9, no. 3: 52. https://doi.org/10.3390/machines9030052
APA StyleTagliavini, L., Botta, A., Cavallone, P., Carbonari, L., & Quaglia, G. (2021). On the Suspension Design of Paquitop, a Novel Service Robot for Home Assistance Applications. Machines, 9(3), 52. https://doi.org/10.3390/machines9030052







