On the Harmonic Oscillator Model of Electron Delocalization (HOMED) Index and its Application to Heteroatomic π-Electron Systems
Abstract
:1. Introduction
2. Results and Discussion
2.1. Original HOMA Index (oHOMA)
2.2. Reformulated HOMA Index (rHOMA)
2.3. HOMED Index
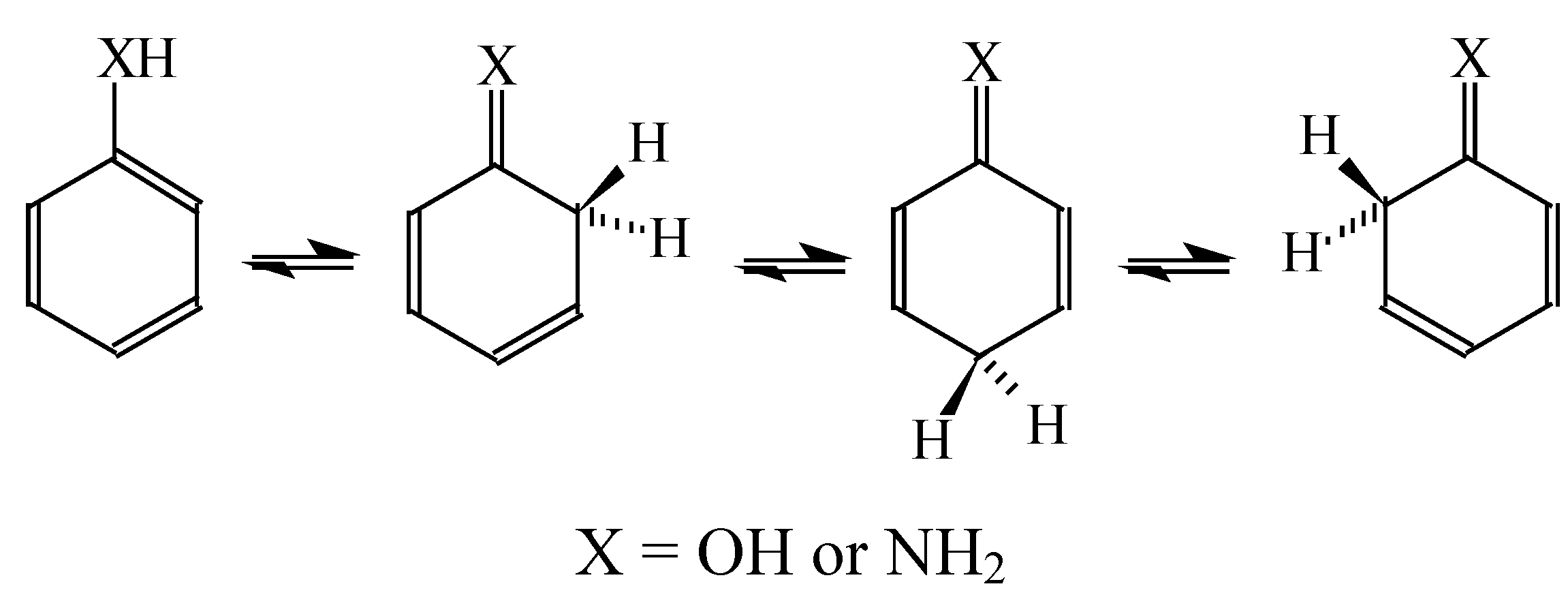
2.4. Non-aromatic π-electron Systems
2.5. Aromatic Systems
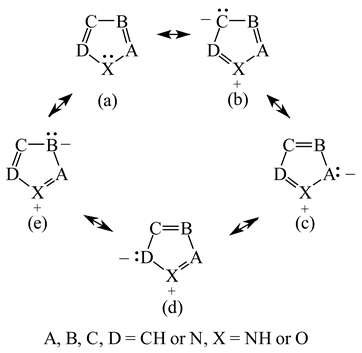
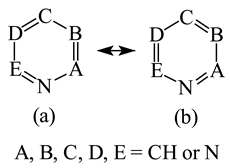
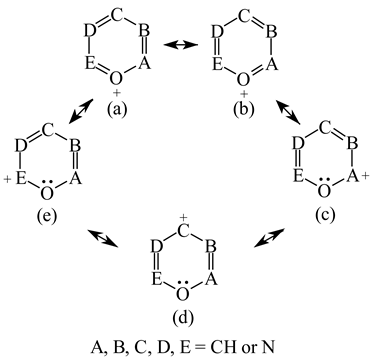
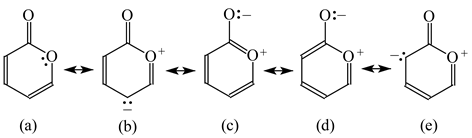

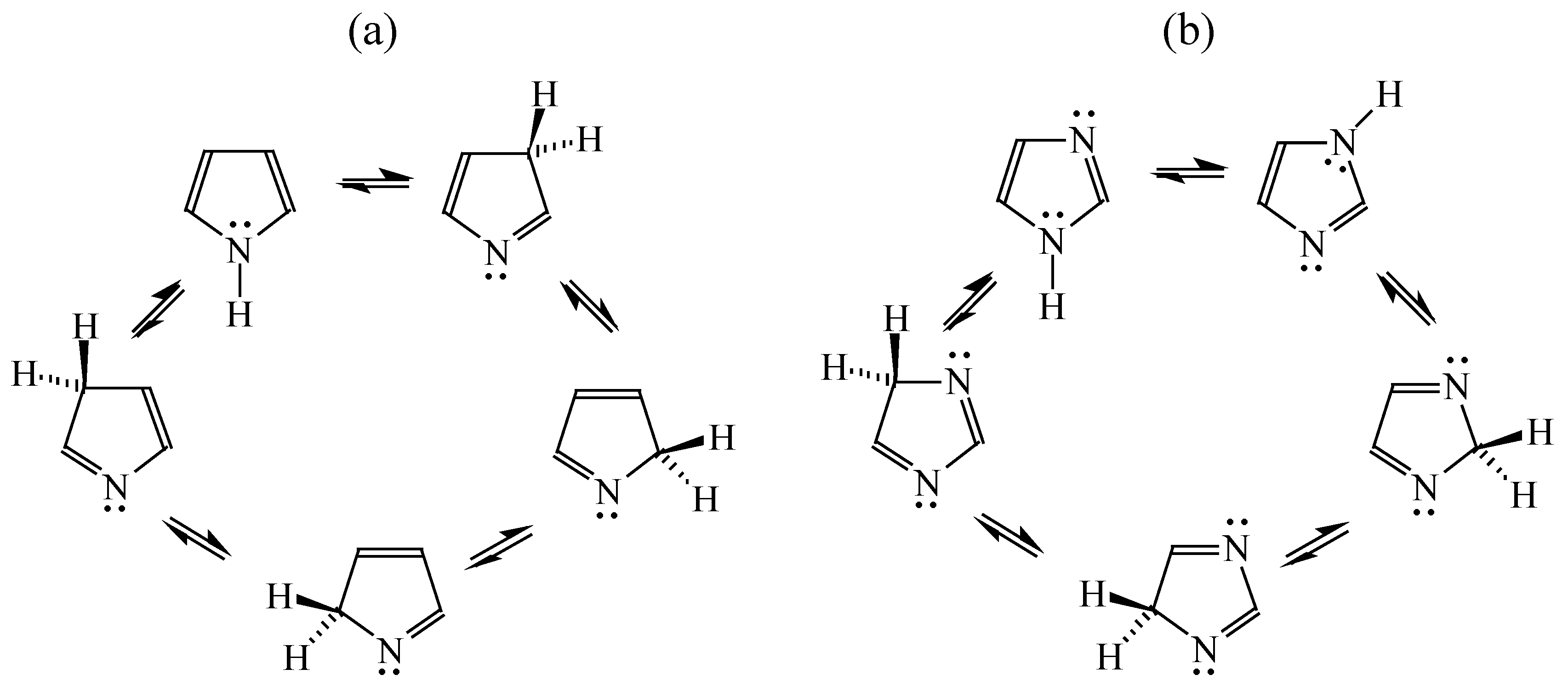
2.6. Tautomeric Systems
3. Computational Details
4. Conclusions
Acknowledgments
References and Notes
- Streiwieser, A. Molecular Orbital Theory for Organic Chemists; Wiley: New York, NY, USA, 1961. [Google Scholar]
- Dewar, M.J.S. The Molecular Orbital Theory of Organic Chemistry; McGraw-Hill: New York, NY, USA, 1969. [Google Scholar]
- Hess, B.A., Jr.; Schaad, L.J. Hueckel Molecular Orbital π-Resonance Energies. Heterocycles Containing Divalent Sulfur. J. Am. Chem. Soc. 1973, 95, 3907–3912. [Google Scholar] [CrossRef]
- Hehre, W.J.; Radom, L.; Schleyer, P.v.R.; Pople, J.A. Ab initio Molecular Theory; Wiley: New York, NY, USA, 1986. [Google Scholar]
- Bird, C.W. A New Aromaticity Index and Its Application to Five-Membered Ring Heterocycles. Tetrahedron 1985, 41, 1409–1414. [Google Scholar] [CrossRef]
- Bird, C.W. The Application of a New Aromaticity Index to Six-Membered Ring Heterocycles. Tetrahedron 1986, 42, 89–92. [Google Scholar] [CrossRef]
- Katritzky, A.R.; Barczyński, P.; Musumurra, G.; Pisano, D.; Szafran, M. Aromaticity as a Quantitative Concept. 1. A Statistical Demonstration of the Orthogonality of ‘Classical’ and ‘Magnetic’ Aromaticity in Five- and Six-Membered Heterocycles. J. Am. Chem. Soc. 1989, 11, 7–15. [Google Scholar] [CrossRef]
- Minkin, V.I.; Glukhovtsev, M.N.; Simkin, B.Y. Aromaticity and Antiaromaticity. Electronic and Structural Aspects; Wiley: New York, NY, USA, 1994. [Google Scholar]
- Schleyer, P.v.R.; Maerker, C.; Dransfield, A.; Jiao, H.; van Eikema Hommes, N.J.R. Nucleus-Independent Chemical Shifts: A Simple and EfficientAromaticity Probe. J. Am. Chem. Soc. 1996, 118, 6317–6318. [Google Scholar] [CrossRef] [PubMed]
- Schleyer, P.v.R.; Jiao, H. What is Aromaticity? Pure Appl. Chem. 1996, 68, 209–218. [Google Scholar] [CrossRef]
- Chen, Z.; Wannere, C.S.; Corminboeuf, C.; Puchta, R.; Schleyer, P.v.R. Nucleus-Independent Chemical Shifts (NICS) as Aromaticity Criterion. Chem. Rev. 2005, 105, 3842–3888. [Google Scholar] [CrossRef] [PubMed]
- Katritzky, A.R.; Jug, K.; Oniciu, D.C. Quantitative Measures of Aromaticity for Mono-, Bi-, and Tricyclic Penta- and Hexaatomic Heteroaromatic Ring Systems and Their Interrelations. Chem. Rev. 2001, 101, 1421–1450. [Google Scholar] [CrossRef] [PubMed]
- Krygowski, T.M.; Cyrański, M.K. Structural Aspects of Aromaticity. Chem. Rev. 2001, 101, 1385–1419, and references cited therein. [Google Scholar] [CrossRef]
- Randić, M. Aromaticity of Polycyclic Conjugated Hydrocarbons. Chem. Rev. 2003, 103, 3449–3606. [Google Scholar] [CrossRef]
- Balaban, A.T.; Oniciu, D.C.; Katritzky, A.R. Aromaticity as a Cornestone of Heterocyclic Chemistry. Chem. Rev. 2004, 104, 2777–2812. [Google Scholar] [CrossRef] [PubMed]
- Cyrański, M.K. Energetic Aspects of Cyclic Pi-Electron Delocalization: Evaluation of the Methods of Estimating Aromatic Stabilization Energies. Chem. Rev. 2005, 105, 3773–3811, and references cited therein. [Google Scholar] [CrossRef] [PubMed]
- Krygowski, T.M.; Stępień, B.T. Sigma- and Pi-Electron Delocalization: Focus on Substituent Effects. Chem. Rev. 2005, 105, 3482–3512, and references cited therein. [Google Scholar] [CrossRef] [PubMed]
- Raczyńska, E.D.; Kosińska, W.; Ośmiałowski, B.; Gawinecki, R. Tautomeric Equilibria in Relation to Pi-Electron Delocalization. Chem. Rev. 2005, 105, 3561–3612, and references cited therein. [Google Scholar] [CrossRef] [PubMed]
- Poater, J.; Duran, M.; Sola, M.; Silvi, B. Theoretical Evaluation of Electron Delocalization in Aromatic Molecules by Means of Atoms in Molecules (AIM) and Electron Localization Function (ELF) Topological Approaches. Chem. Rev. 2005, 105, 3911–3947. [Google Scholar] [CrossRef] [PubMed]
- Kruszewski, J.; Krygowski, T.M. Harmonic Oscillator Approach to the Definition of Aromaticity. Bull. Acad. Pol. Sci. Chim. 1972, 20, 907–915. [Google Scholar]
- Kruszewski, J.; Krygowski, T.M. Definition of Aromaticity Basing on the Harmonic Oscillator Model. Tetrahedron Lett. 1972, 3839–3842. [Google Scholar] [CrossRef]
- Krygowski, T.M.; Kruszewski, J. Bond Reactivity Index (BRI) in Terms of Simple Harmonic Oscillator Theory. Bull. Acad. Pol. Sci. Chim. 1973, 21, 409–412. [Google Scholar]
- Krygowski, T.M.; Kruszewski, J. Aromaticity of Thiophene, Pyrrole and Furan in Terms of Aromaticity Indices and Hammett ρ Constants. Bull. Acad. Pol. Sci. Chim. 1974, 22, 871–876. [Google Scholar]
- Krygowski, T.M. Crystallographic Studies of Inter- and Intramolecular Interactions Reflected in Aromatic Character of π-Electron Systems. J. Chem. Inform. Comput. Sci. 1993, 33, 70–78. [Google Scholar] [CrossRef]
- Jug, K.; Koester, A. Influence of σ and π Electrons on Aromaticity. J. Am. Chem. Soc. 1990, 112, 6772–6777. [Google Scholar] [CrossRef]
- Raczyńska, E.D. On n-π Electron Distribution in Simple Keto-Enol Tautomeric Systems: H3C−C(R)=OH2C=C(R)−OH and RH2CCH=ORHC=CH−OH. Pol. J. Chem. 2005, 79, 749–758. [Google Scholar]
- Raczyńska, E.D.; Ośmiałowski, B.; Gawinecki, R. Tautomeric Equilibria (1,5-Proton Shift) in Simple Heteroconjugated Systems HX−A=B−C=YX=A−B=C−YH in Relation to π Electron Delocalization. Pol. J. Chem. 2005, 79, 1093–1097. [Google Scholar]
- Raczyńska, E.D. On n-π Electron Distribution in Simple Acyclic Tetrad and Pentad Tautomeric Systems. Pol. J. Chem. 2005, 79, 1003–1013. [Google Scholar]
- Raczyńska, E.D.; Krygowski, T.M.; Zachara, J.E.; Ośmiałowski, B.; Gawinecki, R. Tautomeric Equilibria, H-Bonding and π-Electron Delocalization in o-Nitrosophenol. A B3LYP/6-311+G(2df,2p) Study. J. Phys. Org. Chem. 2005, 18, 892–897. [Google Scholar] [CrossRef]
- Osmialowski, B.; Raczyńska, E.D.; Krygowski, T.M. Tautomeric Equilibria and Pi Electron Delocalization for Some Monohydroxyarenes–Quantum Chemical Studies. J. Org. Chem. 2006, 71, 3727–3736. [Google Scholar] [CrossRef] [PubMed]
- Raczyńska, E.D.; Krygowski, T.M.; Duczmal, K.; Hallmann, M. On Geometry-Based HOMED (A Measure of Hyperconjugation, n-π, and π-π Conjugation) and HOMA Index (A Measure of Aromaticity). In Proceedings of the XVIII International Conference on Physical Organic Chemistry, Warsaw, Poland, 20–25 August 2006; p. 31. [Google Scholar]
- Parr, R.G.; Yang, W. Density Functional Theory of Atoms and Molecules; Oxford University Press: New York, NY, USA, 1989. [Google Scholar]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti Correlation-Energy Formula into a Functional of the Electron Density. Phys. Rev. 1988, B37, 785–789. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional Exchange-energy Approximation with Correct Asymptotic Behavior. Phys. Rev. 1988, A38, 3098–3100. [Google Scholar] [CrossRef]
- Jensen, F. Introduction to Computational Chemistry; John Wiley & Sons: New York, NY, USA, 1999. [Google Scholar]
- Cyrański, M.K.; Gilski, M.; Jaskólski, M.; Krygowski, T.M. On the Aromatic Character of the Heterocyclic Bases of DNA and RNA. J. Org. Chem. 2003, 68, 8607–8613. [Google Scholar] [CrossRef]
- Böhm, S.; Fiedler, P.; Exner, O. Analysis of the Ortho Effect. Acidity of 2-Substituted Benzoic Acids. New. J. Chem. 2004, 28, 67–74. [Google Scholar] [CrossRef]
- Fiedler, P.; Böhm, S.; Kulhánek, J.; Exner, O. Acidity of Ortho-Substituted Benzoic Acids: An Infrared and Theoretical Study of the Intramolecular Hydrogen Bonds. Org. Biomol. Chem. 2006, 4, 2003–2011. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.L.; Wannere, C.S.; Mo, Y.; Schleyer, P.v.R.; Bunz, U.H.F. 4n π Electron but Stable: N,N-Dihydrodiazapentacenes. J. Org. Chem. 2009, 74, 4343–4349. [Google Scholar] [CrossRef] [PubMed]
- Wodrich, M.D.; Wannere, C.S.; Mo, Y.; Jarowski, P.D.; Houk, K.N.; Schleyer, P.v.R. The Concept of Protobranching and Its Many Paradigm Shifting Implications for Energy Evaluations. Chem. Eur. J. 2007, 13, 7731–7744. [Google Scholar] [CrossRef] [PubMed]
- Madura, I.D.; Krygowski, T.M.; Cyrański, M.K. Structural Aspects of the Aromaticity of Cyclic π-Electron Systems with BN Bonds. Tetrahedron 1998, 54, 14913–14918. [Google Scholar] [CrossRef]
- Weinhold, F. Chemistry: A New Twist on Molecular Shape. Nature 2001, 411, 539–541. [Google Scholar] [CrossRef]
- Pophristic, V.; Goodman, L. Hyperconjugation not Steric Repulsion Leads to the Staggered Structure of Ethane. Nature 2001, 411, 565–568. [Google Scholar] [CrossRef] [PubMed]
- Pophristic, V.; Goodman, L. Origin of Staggered Conformational Preference in Methanol. J. Phys. Chem. A 2002, 106, 1642–1646. [Google Scholar] [CrossRef]
- Mó, Y.R.; Wu, W.; Song, L.C.; Lin, M.H.; Zhang, Q.; Gao, J.L. The Magnitude of Hyperconjugation in Ethane: A Perspective from Ab initio Valence Bond Theory. Angew. Chem. Int. Ed. 2004, 43, 1986–1990. [Google Scholar] [CrossRef]
- Fleischer, U.; Kutzelnigg, W.; Lazzeretti, P.; Mühlenkamp, V. IGLO Study of Benzene and Some of Ist Isomers and Related Molecules. Search for Evidence of the Ring Current Model. J. Am. Chem. Soc. 1994, 116, 5298–5306. [Google Scholar] [CrossRef]
- Steinmann, S.N.; Jana, D.F.; Wu, J.I.C.; Schleyer, P.v.R.; Mo, Y. Corminboeuf C. Direct Assessment of Electron Delocalization Using NMR Chemical Shifts. Angew. Chem. Int. Ed. 2009, 48, 9828–9833. [Google Scholar] [CrossRef]
- Carey, F.A.; Sundberg, R.J. Advanced Organic Chemistry, 3rd ed.; Plenum Press: New York, NY, USA, 1993. [Google Scholar]
- Möller, C.; Plesset, M.S. Note on an Approximation Treatment for Many-electron Systems. Phys. Rev. 1934, 46, 618–622. [Google Scholar] [CrossRef]
- Pople, J.A.; Brinkley, J.S.; Seeger, R. Theoretical Models Incorporating Electron Correlation. Int. J. Quantum. Chem. 1976, 10, 1–19. [Google Scholar] [CrossRef]
- March, J. Advanced Organic Chemistry, Reaction, Mechanism and Structure, 4th ed.; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Prasad, B.V.; Grover, G.; Uppal, P.; Kaur, D. N-Inversion and C–N Rotational Barriers in HX=CH–NH2 (X = N, P, As) Compounds: An Ab initio Study. Theochem-J. Mol. Struct. 1999, 458, 227–237. [Google Scholar] [CrossRef]
- Burk, P.; Schleyer, P.v.R. Why Are Carboxylic Acids Stronger Acids than Alcohols? The Electrostatic Theory of Siggel-Thomas Revisites. Theochem-J. Mol. Struct. 2000, 505, 161–167. [Google Scholar] [CrossRef]
- Mó, Y.; Schleyer, P.v.R.; Wu, W.; Menghai, L.; Zhang, Q.; Gao, J. Importance of Electrostatic Delocalization on the C−N Bond Rotation in HCX(NH2) (X = O, NH, CH2, S, and Se). J. Phys. Chem. A 2003, 107, 10011–10018. [Google Scholar] [CrossRef]
- Elguero, J.; Marzin, C.; Katritzky, A.R.; Linda, P. Advances in Heterocyclic Chemistry, Supplement 1; Katritzky, A.R., Boulton, A.J., Eds.; Academic Press: New York, NY, USA, 1976. [Google Scholar]
- Rappoport, Z. (Ed.) The Chemistry of Enols; Wiley: Chichester, UK, 1990. [Google Scholar]
- Rappoport, Z. (Ed.) The Chemistry of Phenols; John Wiley & Sons: Chichester, UK, 2003. [Google Scholar]
- Pozharskii, A.F.; Soldatenkov, A.T.; Katritzky, A.R. Heterocycles in Life and Society; Wiley: New York, NY, USA, 1997. [Google Scholar]
- Raczyńska, E.D.; Zientara, K.; Kolczyńska, K.; Stępniewski, T.M. Change of Tautomeric Equilibria, Intramolecular Interactions and π-Electron Delocalization when Going from Phenol to Uracil. Theochem-J. Mol. Struct. 2009, 894, 103–111. [Google Scholar] [CrossRef]
- Raczyńska, E.D.; Kolczyńska, K.; Stępniewski, T.M. Influence of N-Aza Group(s), Intramolecular Interactions and Ionization on Tautomeric Equilibria for Cytosine. In Proceedings of the 42nd IUPAC Congress, Chemistry Solution, Glasgow, UK, 2–7 August 2009. P202_021. [Google Scholar]
- Raczyńska, E.D.; Zientara, K.; Stępniewski, T.M.; Kolczyńska, K. Stability, Polarity, Intramole-cular Interactions and π-Electron Delocalization for all Eighteen Tautomers Rotamers of Uracil. DFT Studies in the Gas Phase. Collect. Czech. Chem. Commun. 2009, 74, 57–72. [Google Scholar] [CrossRef]
- Firsch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Montgomery, J.A., Jr.; Vreven, T.; Kudin, K.N.; Burant, J.C.; Millam, J.M.; Iyengar, S.S.; Tomasi, J.; Barone, V.; Mennucci, B.; Cossi, M.; Scalmani, G.; Rega, N.; Petersson, R.; Nakatsuji, H.; Hada, M.; Ehara, M.; Toyota, K.; Fukuda, R.; Hasegawa, J.; Ishida, M.; Nakajima, T.; Honda, Y.; Kitao, O.; Nakai, H.; Klene, M.; Li, X.; Knox, J.E.; Hratchian, H.P.; Cross, J.B.; Bakken, V.; Adamo, C.; Jaramillo, R.; Gomperts, R.; Stratmann, R.E.; Yazyev, O.; Austin, A.J.; Cammi, R.; Pomelli, C.; Ochterski, J.W.; Ayala, P.Y.; Morokuma, K.; Voth, G.A.; Salvador, P.; Dannenberg, J.J.; Zakrzewski, V.G.; Dapprich, S.; Daniels, A.D.; Strain, M.C.; Farkas, O.; Malick, D.K.; Rabuck, A.D.; Raghavachari, K.; Foresman, J.B.; Oritz, J.V.; Cui, Q.; Baboul, A.G.; Clifford, S.; Cioslowski, J.; Stefanov, B.B.; Liu, G.; Liashenko, A.; Piskorz, P.; Komaromi, I.; Martin, R.L.; Fox, D.J.; Keith, T.; Al-Laham, M.A.; Peng, C.Y.; Nanayakkara, A.; Challacombe, M.; Gill, P.M.W.; Johnson, B.; Chen, W.; Wong, M.W.; Gonzalez, C.; Pople, J.A. Gaussian-03, Revision E.01; Gaussian, Inc.: Wallingford, CT, USA, 2004. [Google Scholar]
- Mo, Y.; Schleyer, P.v.R. An Energetic Measure of Aromaticity and Antiaromaticity Based on the Pauling-Wheland Resonance Energies. Chem. Eur. J. 2006, 12, 2009–2020. [Google Scholar] [CrossRef] [PubMed]
| Formula | Rs(CC) | Formula | Rs(CN) | Formula | Rs(CO) |
|---|---|---|---|---|---|
| H3C−CH3 | 1.530 | H3C−NH2 | 1.466 | H3C−OH | 1.424 |
 | 1.532 | 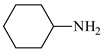 | 1.470 | 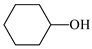 | 1.432 |
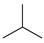 | 1.534 | 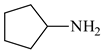 | 1.472 |  | 1.428 |
 | 1.508 |  | 1.472 | 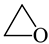 | 1.431 |
 | 1.554 | 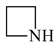 | 1.485 |  | 1.450 |
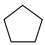 | 1.554 1.557 1.542 1.536 1.540 | 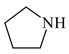 | 1.466 | 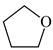 | 1.425 |
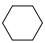 | 1.536 | 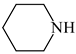 | 1.465 | 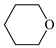 | 1.425 |
| Formula | Rd(CC) | Formula | Rd(CN) | Formula | Rd(CO) |
|---|---|---|---|---|---|
| H2C=CH2 | 1.329 | H2C=NH | 1.267 | H2C=O | 1.202 |
 | 1.291 | 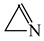 | 1.250 | 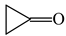 | 1.197 |
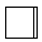 | 1.339 | 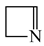 | 1.285 | 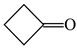 | 1.198 |
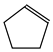 | 1.333 |  | 1.270 | 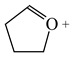 | 1.251 |
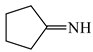 | 1.269 | 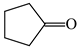 | 1.207 | ||
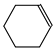 | 1.335 | 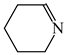 | 1.269 | 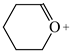 | 1.252 |
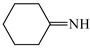 | 1.274 | 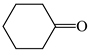 | 1.212 |
| Formula | Ro(CC) | Formula | Ro(CN) | Formula | Ro(CO) |
|---|---|---|---|---|---|
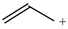 | 1.381 | 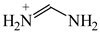 | 1.312 |  | 1.269 |
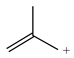 | 1.388 | 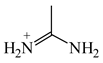 | 1.321 | 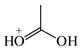 | 1.285 |
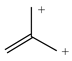 | 1.422 |  | 1.335 | 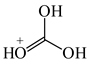 | 1.281 |
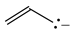 | 1.394 | 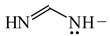 | 1.326 |  | 1.252 |
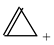 | 1.363 | 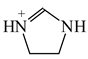 | 1.313 | 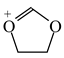 | 1.268 |
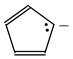 | 1.415 | 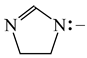 | 1.330 | 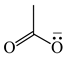 | 1.256 |
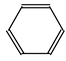 | 1.394 | 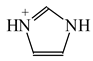 | 1.334 | 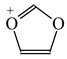 | 1.286 |
 | 1.396 | 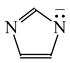 | 1.349 | 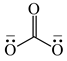 | 1.308 |
 | 1.408 | 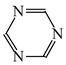 | 1.334 | 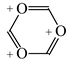 | 1.322 |
| Bond | Formula | Rs | Formula | Rd | Formula | Ro |
|---|---|---|---|---|---|---|
| CC | H3C−CH3 | 1.5300 | H2C=CH2 | 1.3288 | 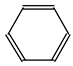 | 1.3943 |
| CN | H3C−NH2 | 1.4658 | H2C=NH | 1.2670 |  | 1.3342 |
| CO | H3C−OH | 1.4238 | H2C=O | 1.2017 | 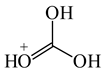 | 1.2811 |
| Bond | α2i | α3 | α5 | α7 | |||
|---|---|---|---|---|---|---|---|
| 2s+1d | 1s+2d | 3s+2d | 2s+3d | 4s+3d | 3s+4d | ||
| CC | 88.09 | 72.96 | 111.13 | 78.34 | 100.76 | 80.90 | 96.68 |
| CN | 91.60 | 73.90 | 113.85 | 81.98 | 103.77 | 84.52 | 99.97 |
| CO | 75.00 | 63.79 | 90.83 | 67.84 | 83.84 | 69.74 | 81.10 |
| Level | Rs | Rd | Ro | α | HOMED |
|---|---|---|---|---|---|
| B3LYP/6-31G(d) | 1.5301 | 1.3307 | 1.3965 | 90.18 | 0.319 |
| B3LYP/6-31G(d,p) | 1.5296 | 1.3299 | 1.3962 | 90.12 | 0.322 |
| B3LYP/6-31+G(d,p) | 1.5317 | 1.3339 | 1.3984 | 91.20 | 0.332 |
| B3LYP/6-31++G(d,p) | 1.5317 | 1.3339 | 1.3984 | 91.20 | 0.333 |
| B3LYP/6-311+G(d,p) | 1.5300 | 1.3288 | 1.3943 | 88.09 | 0.331 |
| B3LYP/6-311++G(d,p) | 1.5302 | 1.3288 | 1.3943 | 87.88 | 0.333 |
| B3LYP/6-311+G(3df,2p) | 1.5272 | 1.3247 | 1.3910 | 87.16 | 0.334 |
| B3LYP/6-311++G(3df,2p) | 1.5272 | 1.3248 | 1.3910 | 87.21 | 0.333 |
| B3LYP/cc-pVTZ | 1.5267 | 1.3241 | 1.3908 | 87.27 | 0.329 |
| B3LYP/aug-cc-pVTZ | 1.5267 | 1.3248 | 1.3911 | 87.78 | 0.333 |
| MP2/6-31G(d) | 1.5257 | 1.3361 | 1.3968 | 98.52 | 0.314 |
| MP2/6-311++G(d,p) | 1.5288 | 1.3392 | 1.4006 | 98.98 | 0.320 |
| HF/6-31G(d) | 1.5272 | 1.3170 | 1.3864 | 81.16 | 0.265 |
| HF/6-311++G(d,p) | 1.5268 | 1.3184 | 1.3864 | 82.18 | 0.270 |
| Formula | HOMED rHOMA | Formula | HOMED rHOMA | Formula | HOMED rHOMA |
|---|---|---|---|---|---|
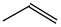 | 0.331 −1.030 |  | 0.225 −1.371 | 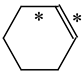 | 0.270 −1.217 |
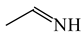 | 0.344 −0.751 |  | 0.095 −1.511 | 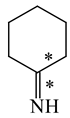 | 0.222 −1.150 |
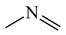 | 0.138 0.113 | 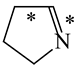 | 0.186 −1.237 |  | 0.180 −1.243 |
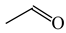 | 0.259 −0.978 |  | −0.029 −1.900 | 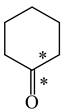 | 0.112 −1.538 |
| Formula | HOMED rHOMA | Formula | HOMED rHOMA | Formula | HOMED rHOMA |
|---|---|---|---|---|---|
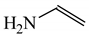 | 0.710 0.526 | 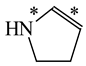 | 0.627 0.435 |  | 0.734 0.587 |
 | 0.716 0.559 |  | 0.642 0.485 | ||
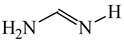 | 0.779 0.773 | 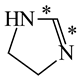 | 0.701 0.696 | 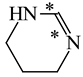 | 0.782 0.773 |
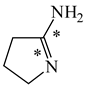 | 0.772 0.767 |  | 0.699 0.690 | ||
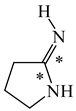 | 0.775 0.771 | 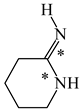 | 0.771 0.766 | ||
 | 0.787 0.745 | 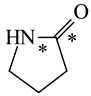 | 0.777 0.743 | 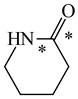 | 0.814 0.797 |
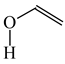 | 0.574 −0.174 | 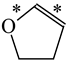 | 0.546 −0.253 |  | 0.591 −0.120 |
 | 0.599 −0.106 |  | 0.518 −0.305 | ||
 | 0.610 0.248 | 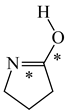 | 0.638 0.260 |  | 0.543 0.043 |
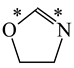 | 0.557 0.097 |  | 0.539 0.046 | ||
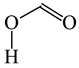 | 0.589 0.141 | 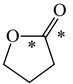 | 0.479 −0.135 | 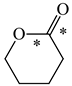 | 0.547 0.017 |
| Formula | HOMED rHOMA | Formula | HOMED rHOMA |
|---|---|---|---|
 | 0.627 0.173 | 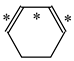 | 0.603 0.098 |
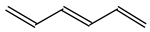 | 0.726 0.314 | 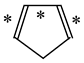 | 0.634 0.161 |
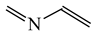 | 0.549 0.486 |  | 0.495 0.490 |
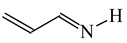 | 0.574 0.192 |  | 0.620 0.370 |
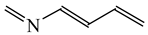 | 0.697 0.486 | 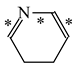 | 0.515 0.486 |
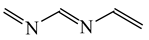 | 0.627 0.592 |  | 0.459 0.559 |
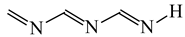 | 0.580 0.619 | 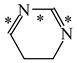 | 0.549 0.539 |
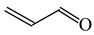 | 0.488 −0.030 |  | 0.651 0.235 |
| Formula | HOMED rHOMA | Formula | HOMED rHOMA |
|---|---|---|---|
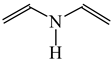 | 0.753 0.574 |  | 0.524 −0.299 |
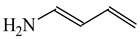 | 0.772 0.476 | 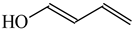 | 0.687 0.098 |
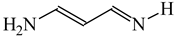 | 0.805 0.599 | 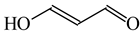 | 0.700 0.221 |
| Formula | HOMED rHOMA | Formula | HOMED rHOMA | Formula | HOMED rHOMA |
|---|---|---|---|---|---|
 | 0.921 0.854 | 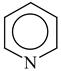 | 0.9997 0.995 | 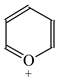 | 0.926 0.748 |
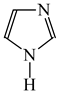 | 0.903 0.882 | 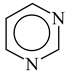 | 0.9995 0.999 |  | 0.893 0.699 |
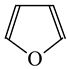 | 0.749 0.189 |  | 0.9999 0.996 | 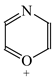 | 0.951 0.816 |
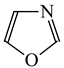 | 0.702 0.224 | 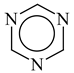 | 1 (by def.) 1.000 | 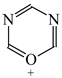 | 0.870 0.661 |
| Formula | HOMED rHOMA | Formula | HOMED rHOMA | Formula | HOMED rHOMA |
|---|---|---|---|---|---|
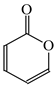 | 0.618 −0.052 | 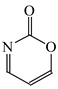 | 0.543 −0.122 | 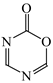 | 0.487 −0.169 |
 | 0.601 −0.044 |  | 0.557 −0.024 | ||
 | 0.666 −0.045 | 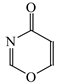 | 0.562 0.176 |  | 0.462 0.064 |
| Tautomer(s) | ΔG | HOMED rHOMA |
|---|---|---|
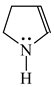 | 6.6 | 0.627 0.435 |
 | 0.0 | 0.186 −1.237 |
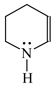 | 1.7 | 0.734 0.587 |
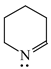 | 0.0 | 0.180 −1.243 |
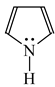 | 0.0 | 0.921 0.854 |
 | 16.6 | 0.413 −0.669 |
 | 14.9 | 0.398 −0.426 |
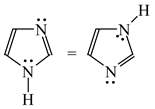 | 0.0 | 0.903 0.882 |
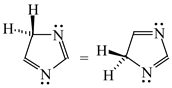 | 16.6 | 0.337 −0.203 |
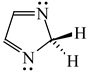 | 16.8 | 0.320 −0.081 |
| Tautomer | ΔG | HOMED rHOMA | Tautomer | ΔG | HOMED rHOMA |
|---|---|---|---|---|---|
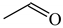 | 0.0 | 0.259 −0.978 | 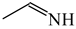 | 0.0 | 0.344 −0.751 |
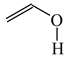 | 11.4 | 0.574 −0.174 | 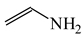 | 2.6 | 0.710 0.526 |
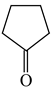 | 0.0 | −0.029 −1.900 | 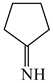 | 0.0 | 0.095 −1.511 |
 | 13.3 | 0.599 −0.106 |  | 2.1 | 0.716 0.559 |
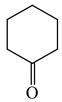 | 0.0 | 0.112 −1.538 |  | 0.0 | 0.222 −1.538 |
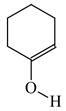 | 10.8 | 0.518 −0.305 |  | 1.8 | 0.642 0.485 |
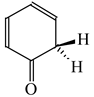 | 16.7 | 0.469 −0.749 | 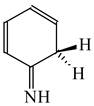 | 28.4 | 0.459 −0.795 |
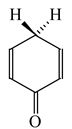 | 16.3 | 0.495 −0.667 | 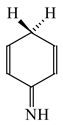 | 26.5 | 0.512 −0.624 |
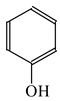 | 0.0 | 0.915 0.742 | 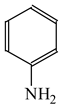 | 0.0 | 0.948 0.923 |
© 2010 by the authors; licensee MDPI, Basel, Switzerland. This article is an Open Access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Raczyńska, E.D.; Hallman, M.; Kolczyńska, K.; Stępniewski, T.M. On the Harmonic Oscillator Model of Electron Delocalization (HOMED) Index and its Application to Heteroatomic π-Electron Systems. Symmetry 2010, 2, 1485-1509. https://doi.org/10.3390/sym2031485
Raczyńska ED, Hallman M, Kolczyńska K, Stępniewski TM. On the Harmonic Oscillator Model of Electron Delocalization (HOMED) Index and its Application to Heteroatomic π-Electron Systems. Symmetry. 2010; 2(3):1485-1509. https://doi.org/10.3390/sym2031485
Chicago/Turabian StyleRaczyńska, Ewa D., Małgorzata Hallman, Katarzyna Kolczyńska, and Tomasz M. Stępniewski. 2010. "On the Harmonic Oscillator Model of Electron Delocalization (HOMED) Index and its Application to Heteroatomic π-Electron Systems" Symmetry 2, no. 3: 1485-1509. https://doi.org/10.3390/sym2031485
APA StyleRaczyńska, E. D., Hallman, M., Kolczyńska, K., & Stępniewski, T. M. (2010). On the Harmonic Oscillator Model of Electron Delocalization (HOMED) Index and its Application to Heteroatomic π-Electron Systems. Symmetry, 2(3), 1485-1509. https://doi.org/10.3390/sym2031485




