Measuring Device for More Precise Mistuning Identification of Integrated Bladed Discs
Abstract
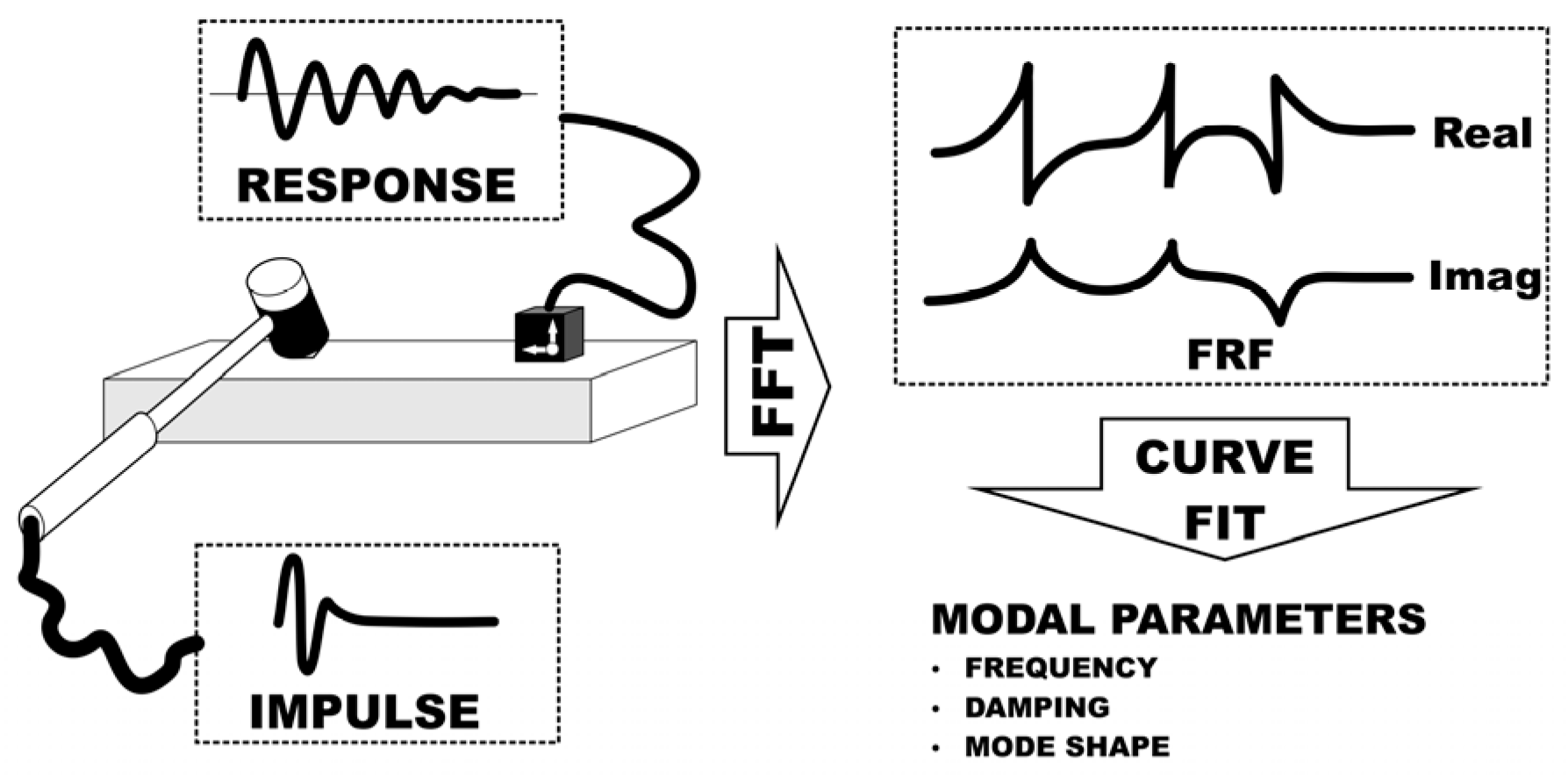
1. Introduction
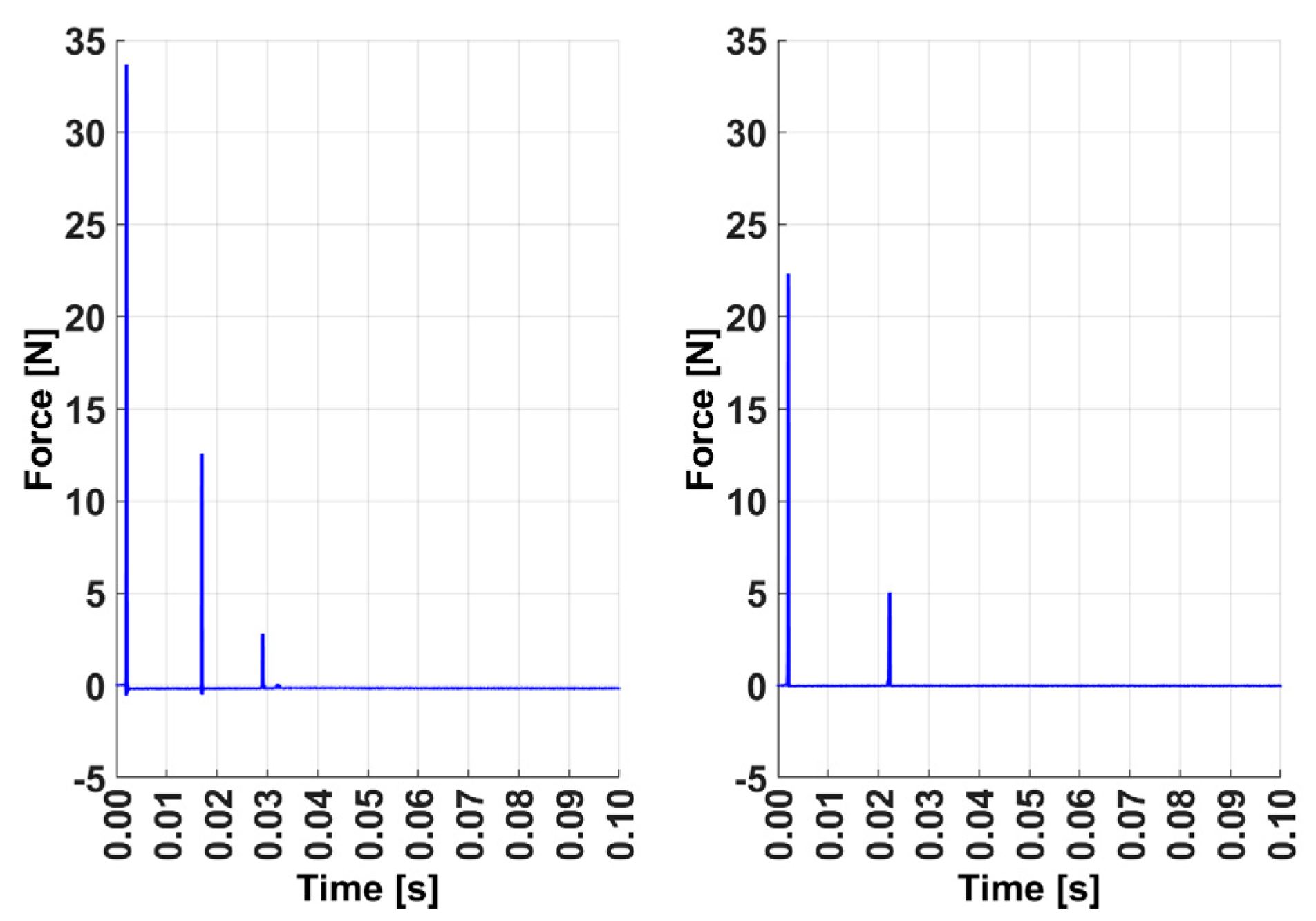
- Variability of strike force. Manual control of the modal hammer can cause variability in the strike force between individual measurements. If the strike force is not constant, it can affect the results of the analysis, especially in terms of accurate measurements of amplitudes and frequencies of the structure’s response.
- Incorrect strike angle. It is difficult to ensure that the strike is always performed at the required angle during manual operations. Changes in the angle can alter the excitation characteristics and lead to errors in the data, which may impact the accuracy of mode identification.
- Lack of repeatability. Manual control can lead to repeatability issues, meaning that each strike may not be exactly the same. Even small differences in position, angle, and strike force can cause changes in the dynamic responses of the measured structure.
- Introduction of unwanted influences. In addition to the strike force, manual operation may transfer vibrations from the hand or arm to the hammer. These transfers can affect the results of the analysis, as unwanted interferences may be recorded in the data instead of a pure structural response.
- Limited positioning accuracy. The placement of the hammer at different points on the structure (depending on the manual handling) can influence the characteristics of the excitation. Different areas of the structure may have different dynamic properties, resulting in varying responses to the same strike.
2. Materials and Methods
2.1. Inaccuracies in Manual Control of the Modal Hammer
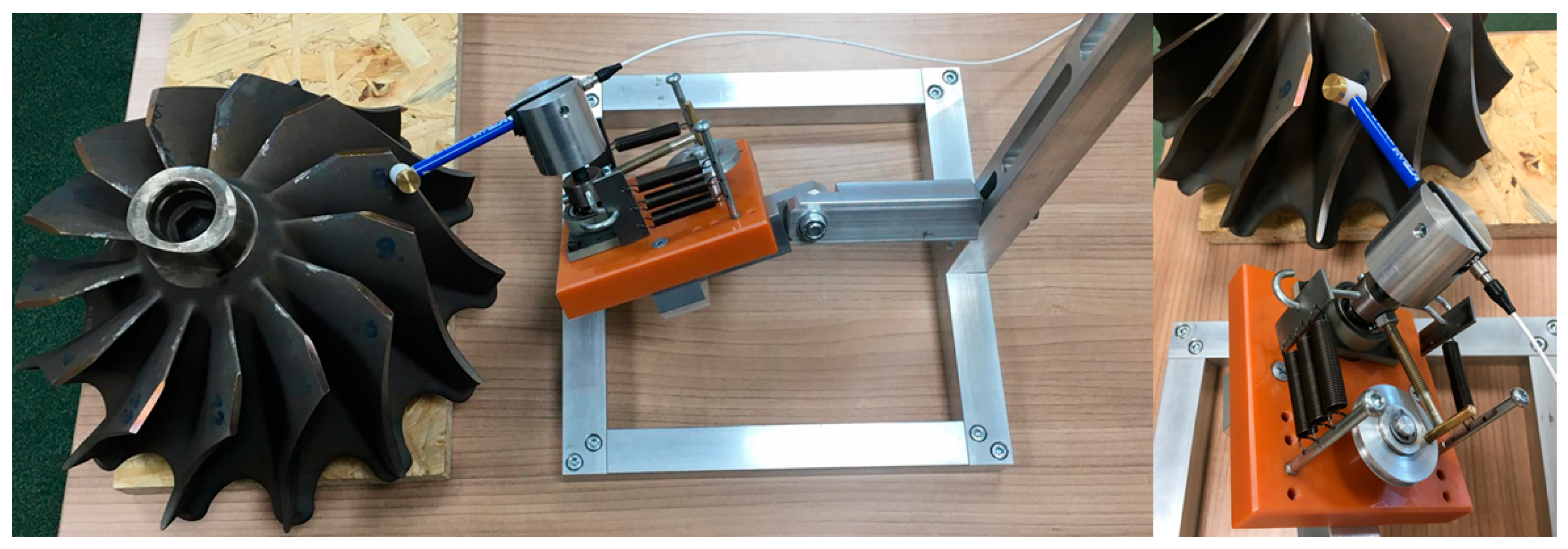
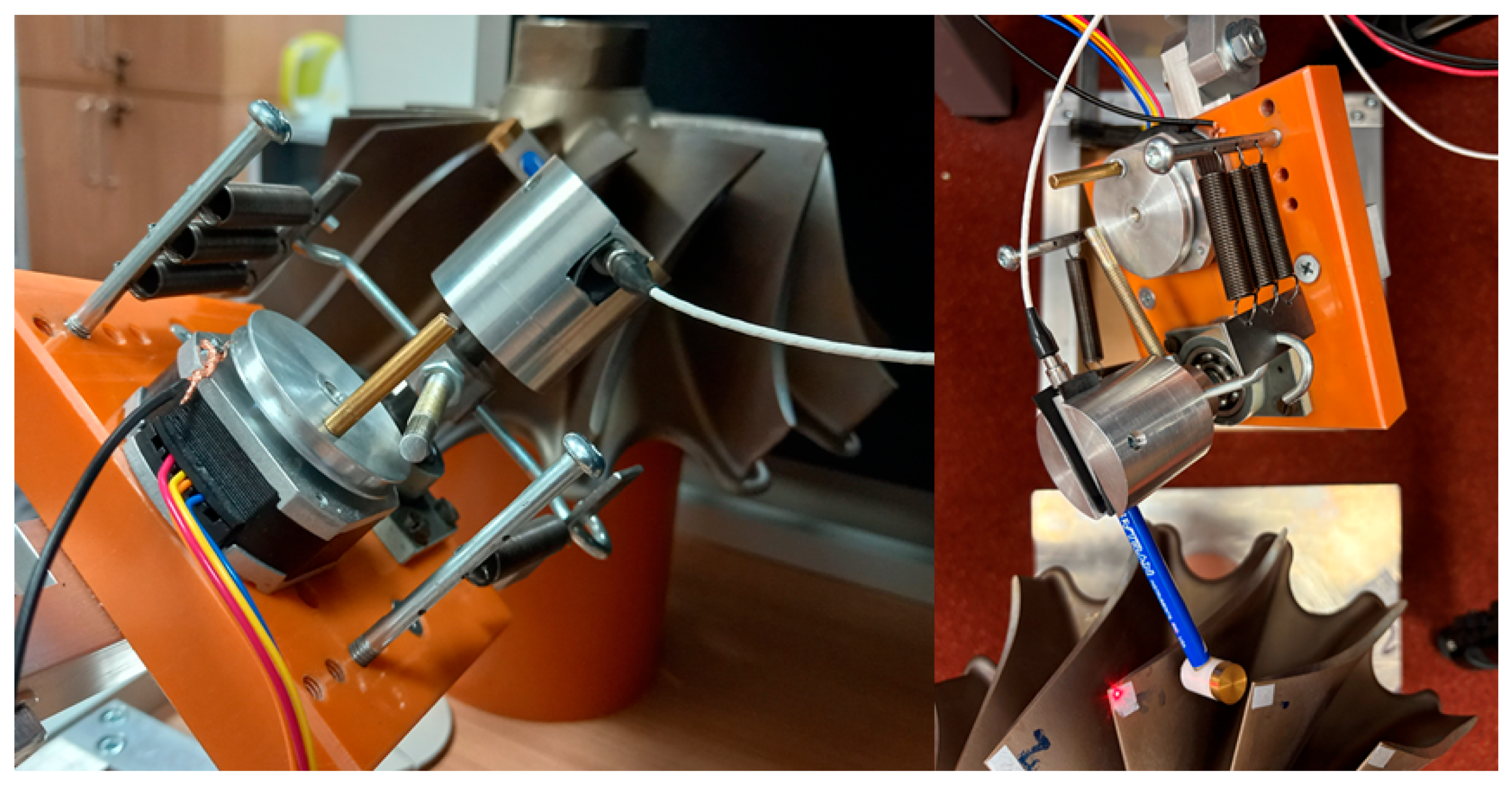
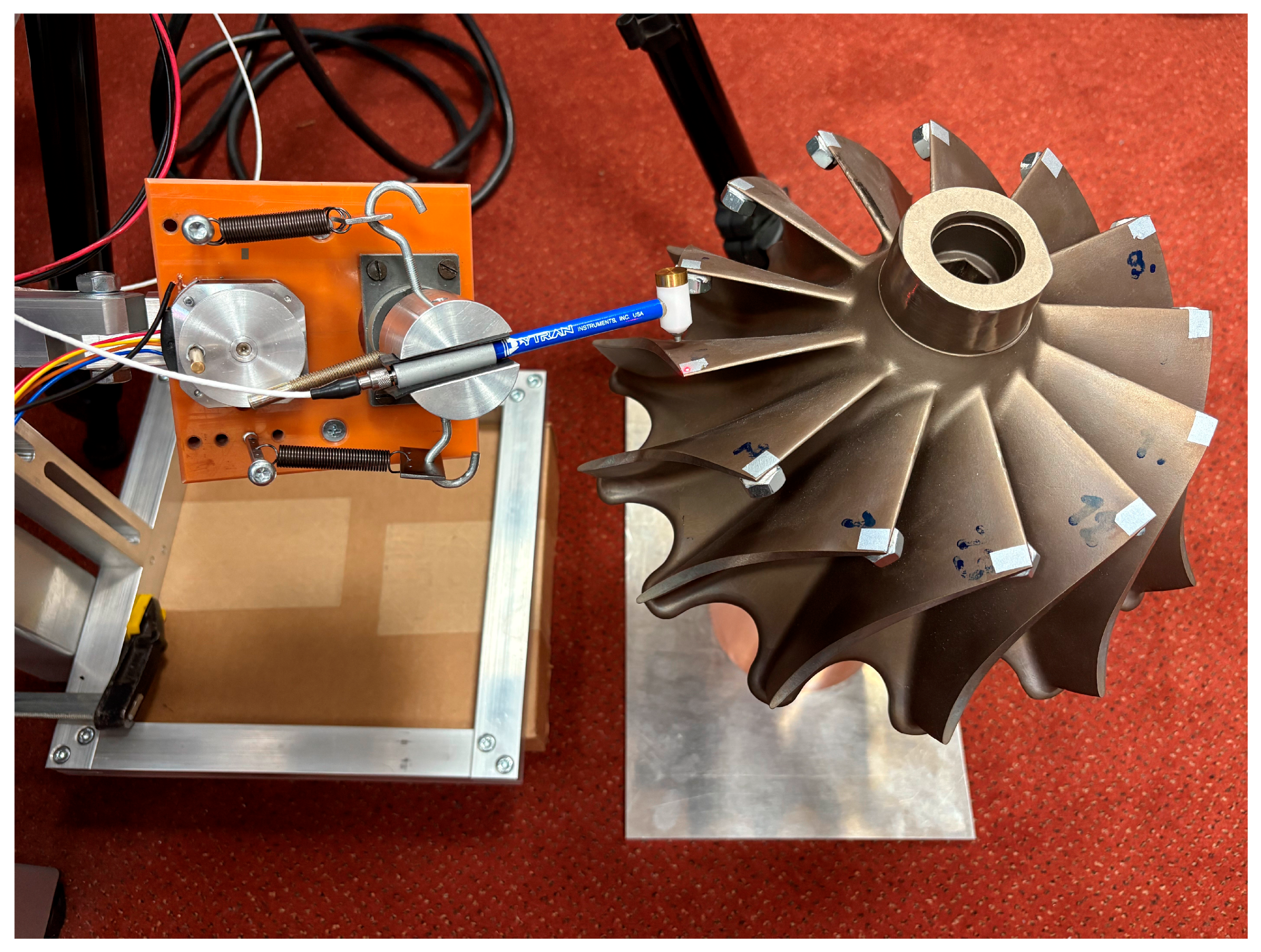
2.2. Measuring Device Replacing the Human Hand
3. Results
3.1. Computational Model of Tuned Turbine Wheel
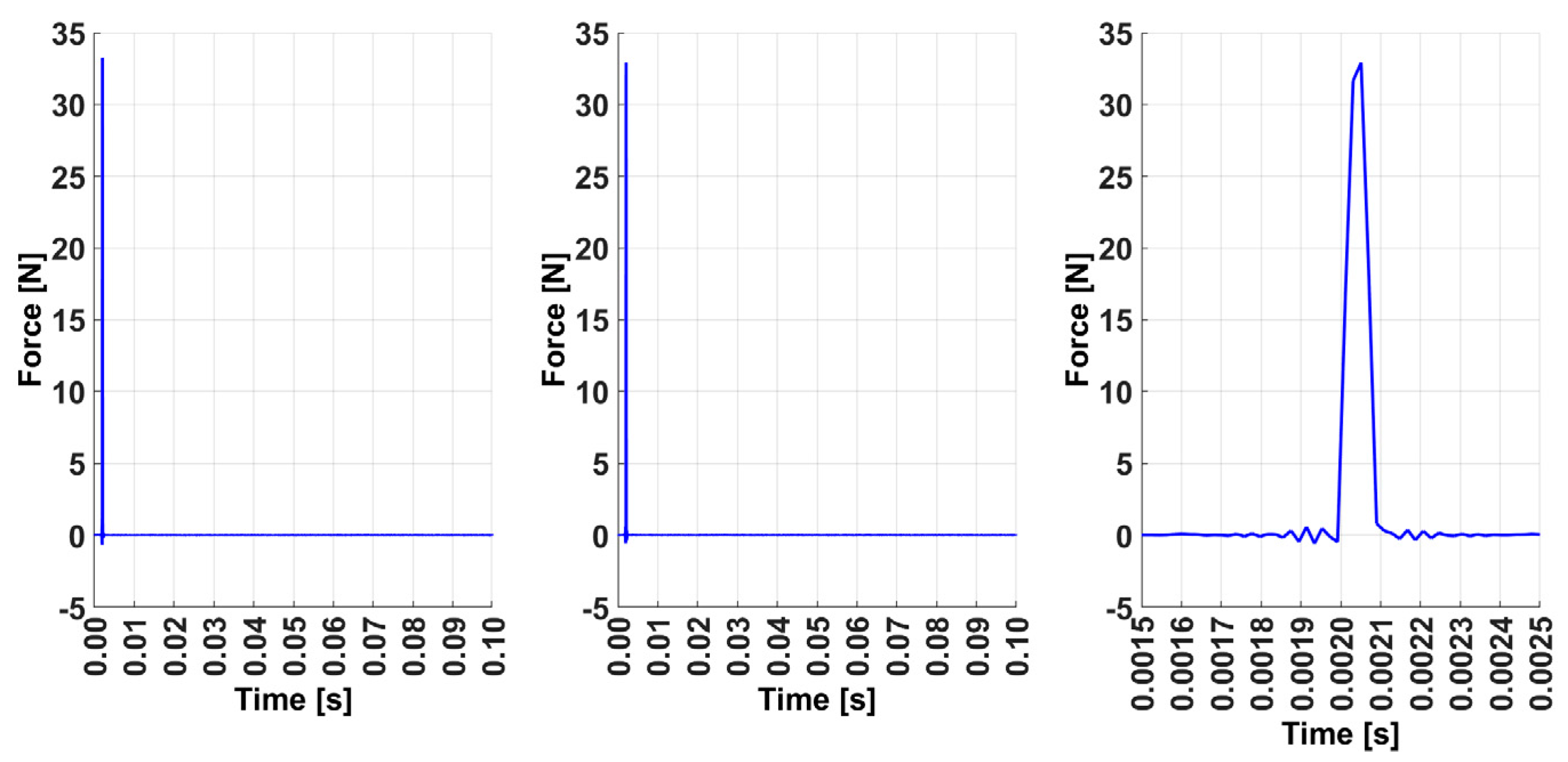
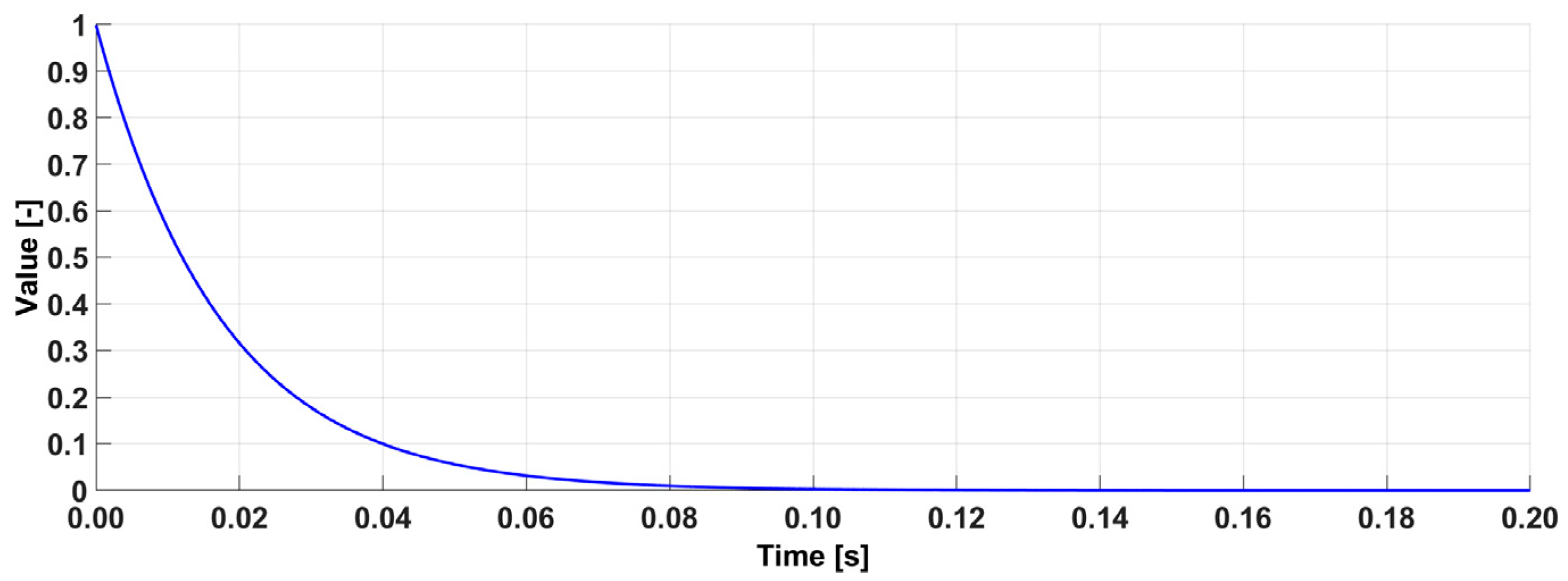
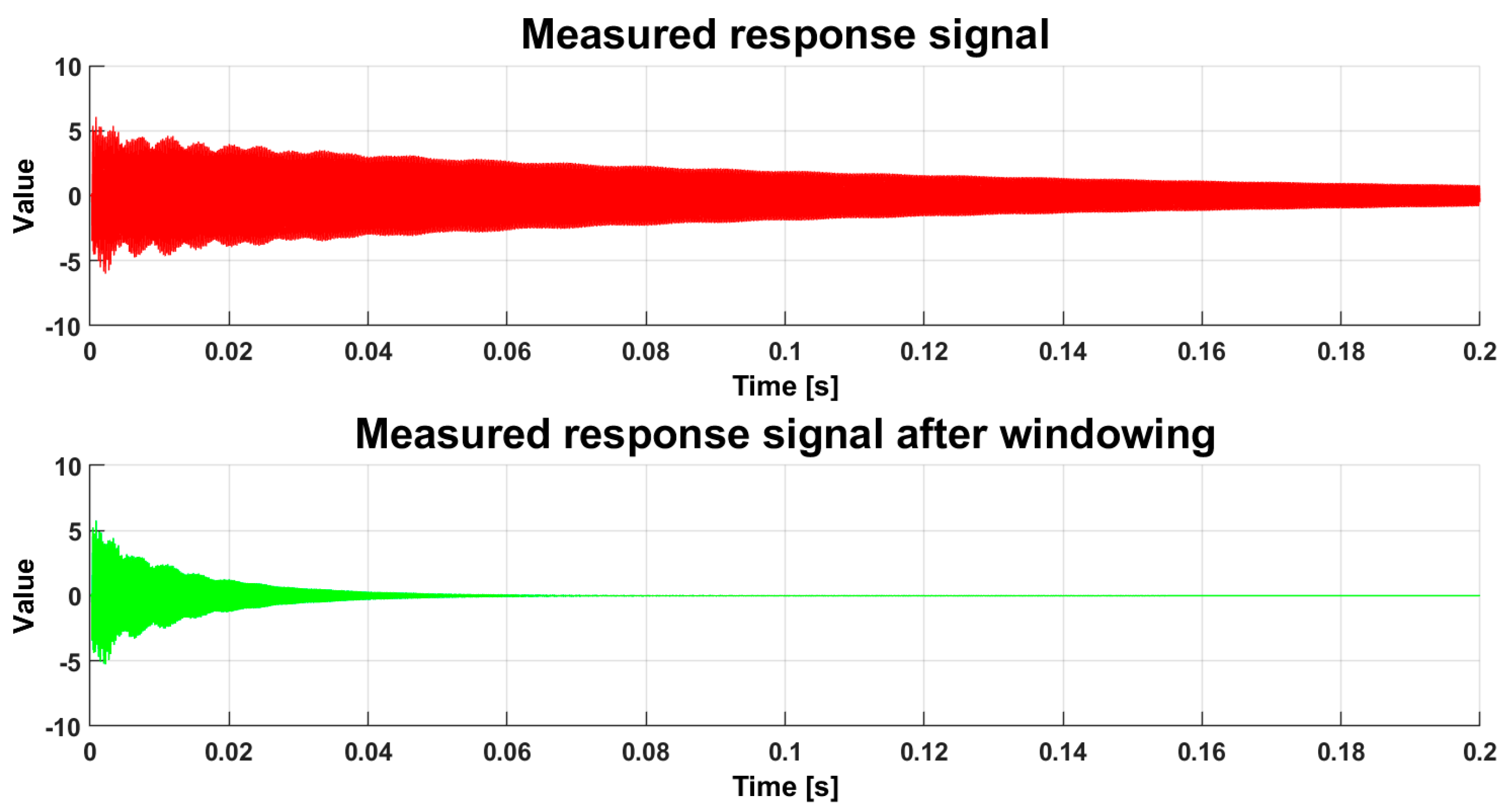
3.2. Measurement and Processing of Measured Data
4. Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FFT | Fast Fourier Transform |
| FRF | Frequency Response Function |
References
- Ewins, D.J. Modal Testing: Theory, Practice and Application, 2nd ed.; Wiley: Hoboken, NJ, USA, 2000; ISBN 978-0-863-80218-8. [Google Scholar]
- Zhao, J.; Experimental Modal Analysis Overview. Crystal Instruments. 2017. Available online: https://www.crystalinstruments.com/blog/2017/8/28/experimental-modal-analysis-overview (accessed on 28 January 2025).
- Feng, K.; Ji, J.C.; Ni, Q.; Beer, M. A review of vibration-based gear wear monitoring and prediction techniques. Mech. Syst. Signal Process. 2023, 182, 109605. [Google Scholar] [CrossRef]
- Qin, Y.; Tang, X.; Jia, T.; Duan, Z.; Zhang, J. Noise and vibration suppression in hybrid electric vehicles: State of the art and challenges. Renew. Sustain. Energy Rev. 2020, 124, 109782. [Google Scholar] [CrossRef]
- Moon, B.Y.; Cho, D.S. A study on vibration reduction by heat deformation of casting frame motor. J. Mech. Sci. Technol. 2022, 36, 1621–1630. [Google Scholar] [CrossRef]
- Qiang, L.; Jingxin, L.; Jing, M.; Dayong, G.; Jiaming, H. Structure design and vibration control of vibration reduction boring bar with variable damping. Int. J. Adv. Manuf. Technol. 2024, 134, 5301–5319. [Google Scholar] [CrossRef]
- You, X.; Feng, H.; Zhang, H.; Liu, P. Optimization analysis of vibration reduction for aeroengine multistage blade-disk system. J. Vibroeng. 2024, 26, 1547–1566. [Google Scholar] [CrossRef]
- Park, M.-H.; Yeo, S.; Choi, J.-H.; Lee, W.-J. Review of noise and vibration reduction technologies in marine machinery: Operational insights and engineering experience. Appl. Ocean Res. 2024, 152, 104195. [Google Scholar] [CrossRef]
- Haywood-Alexander, M.; Mills, R.S.; Champneys, M.D.; Jones, M.R.; Bonney, M.S. Full-scale modal testing of a Hawk T1A aircraft for benchmarking vibration-based methods. J. Sound Vib. 2024, 576, 118295. [Google Scholar] [CrossRef]
- Pais, T.; Silvestri, P. Full scale cruise ship dynamic identification using operational modal analysis on sea trial data measurements. Ocean Eng. 2024, 311, 118931. [Google Scholar] [CrossRef]
- Cai, J.; Ding, S.; Zhang, Q.; Liu, R.; Zeng, D.; Zhou, L. Broken Ice Circumferential Crack Estimation via Image Techniques. Ocean Engineering 2022, 259, 111735. [Google Scholar] [CrossRef]
- Zhi, S.; Wu, H.; Shen, H.; Wang, T.; Fu, H. Entropy-Aided Meshing-Order Modulation Analysis for Wind Turbine Planetary Gear Weak Fault Detection under Variable Rotational Speed. Entropy 2024, 26, 409. [Google Scholar] [CrossRef] [PubMed]
- Monka, P.; Monkova, K.; Uban, M.; Hruzik, L.; Vasina, M. Vibrodiagnostics as the tool of a tap wear monitoring. Procedia Struct. Integr. 2018, 13, 959–964. [Google Scholar] [CrossRef]
- Gatti, P.L. Experimental modal analysis. In Applied Structural and Mechanical Vibrations; CRC Press: London, UK, 1999. [Google Scholar]
- He, J.; Fu, Z.-F. Modal Analysis; Butterworth-Heinemann: Oxford, UK, 2001; ISBN 978-0-7506-5079-3. [Google Scholar] [CrossRef]
- Siemens. Modal Analysis: What Is Modal Analysis? 2025. Available online: https://www.sw.siemens.com/en-US/technology/modal-analysis/ (accessed on 21 February 2025).
- Píštěk, V.; Kučera, P.; Fomin, O.V.; Lovska, A. Effective Mistuning Identification Method of Integrated Bladed Discs of Marine Engine Turbochargers. J. Mar. Sci. Eng. 2020, 8, 379. [Google Scholar] [CrossRef]
- Ewins, D.J. Experimental Modal Analysis; Springer: London, UK, 2000; ISBN 978-1852334236. [Google Scholar]
- Blevins, R.D. Modal Testing: Theory, Practise and Application; Prentice Hall: Upper Saddle River, NJ, USA, 2001; ISBN 978-0750675991. [Google Scholar]
- Statham, G.E.M. Introduction to Experimental Modal Analysis and Testing; Prentice Hall: Upper Saddle River, NJ, USA, 2000; ISBN 978-0131048536. [Google Scholar]
- Shapiro, M.H. Vibration Testing: Theory and Practice; Wiley-Interscience: New York, NY, USA, 1993; ISBN 978-0471047890. [Google Scholar]
- Schenck, W.C.; Driscoll, G.B. Handbook of Experimental Structural Dynamics; ASME Press: New York, NY, USA, 1997; ISBN 978-0791802099. [Google Scholar]
- Kühhorn, A.; Beirow, B. Method for Determining Blade Mistuning on Integrally Manufactured Rotor Wheels. U.S. Patent 20,100,286,934 A1, 11 November 2010. [Google Scholar]




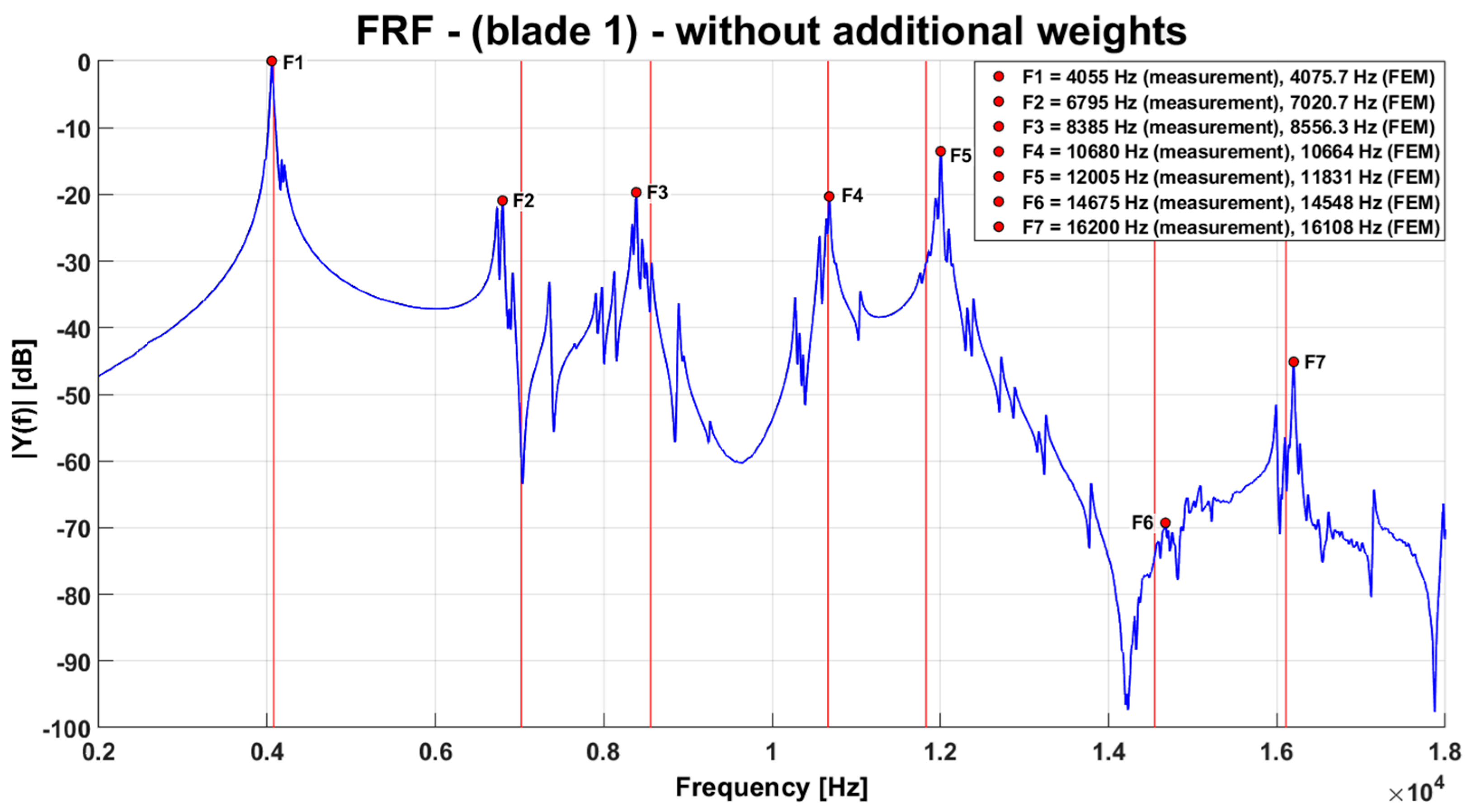
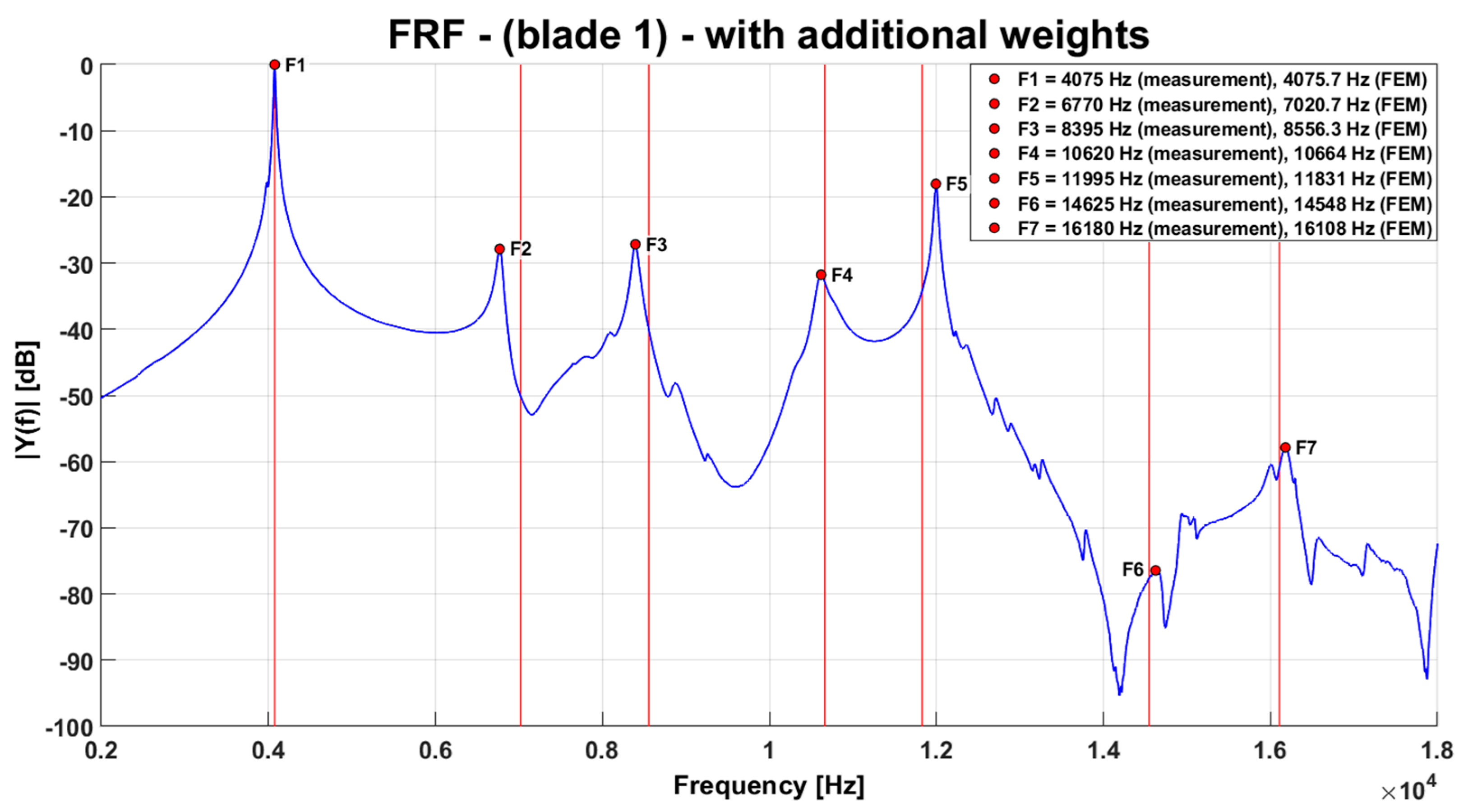
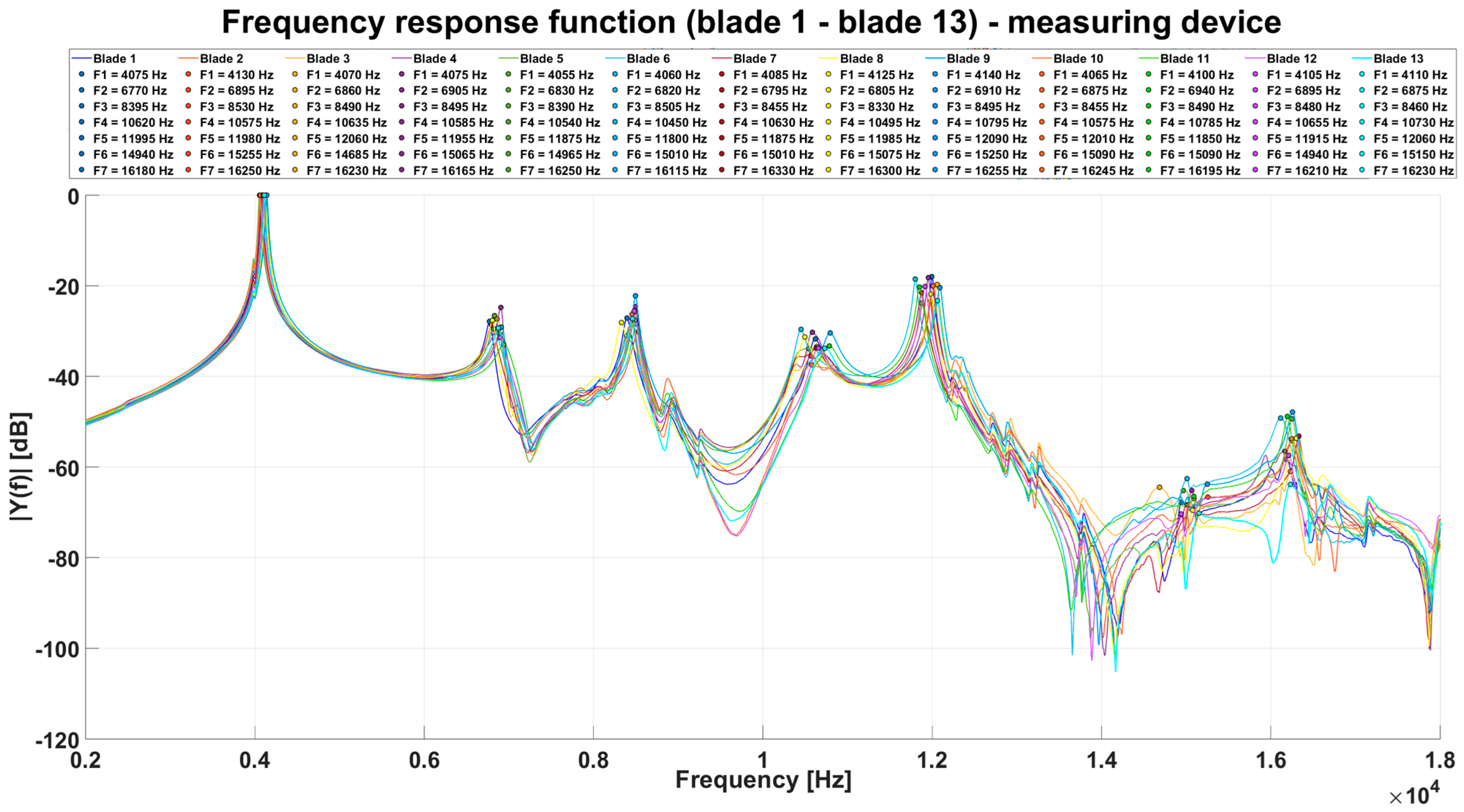
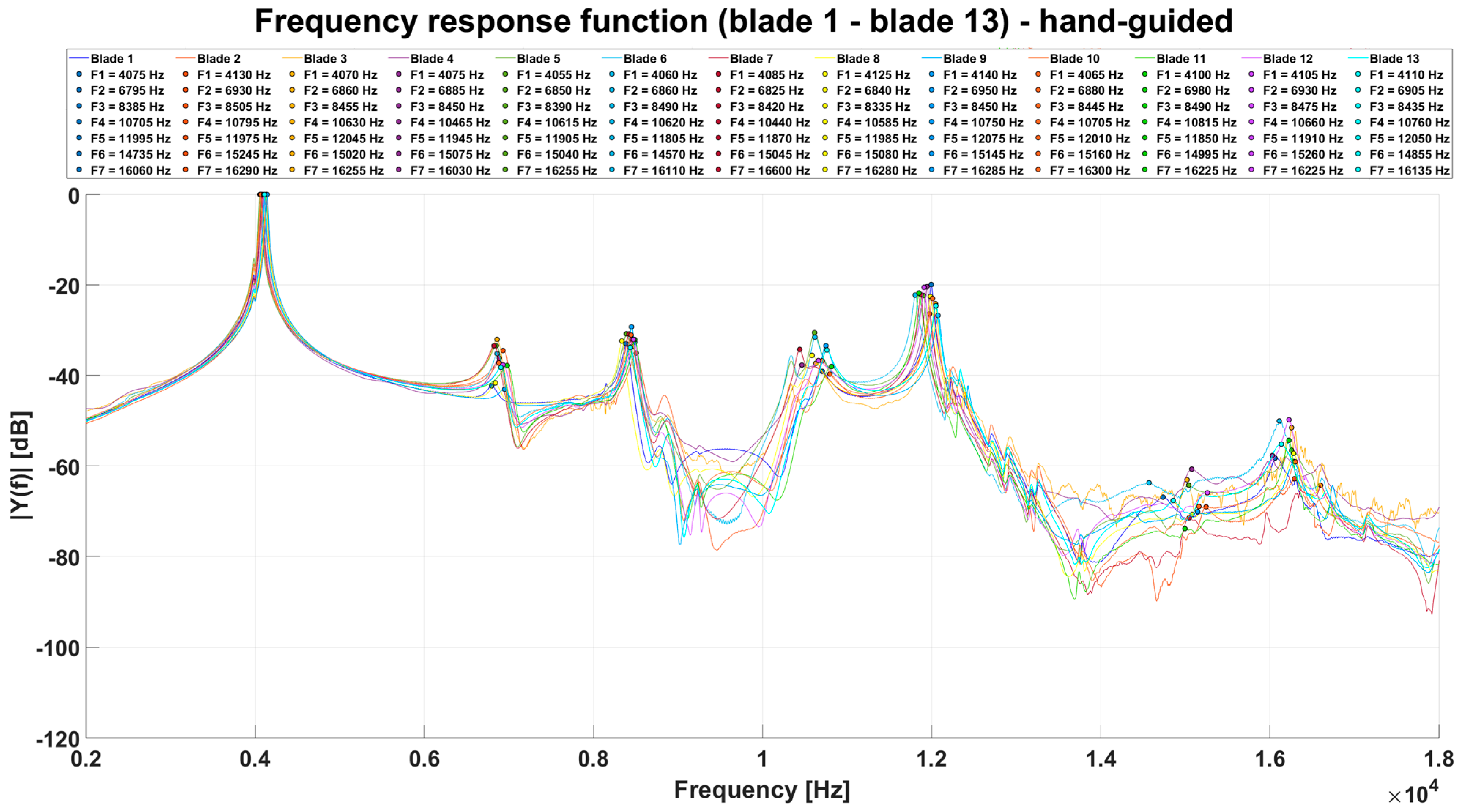
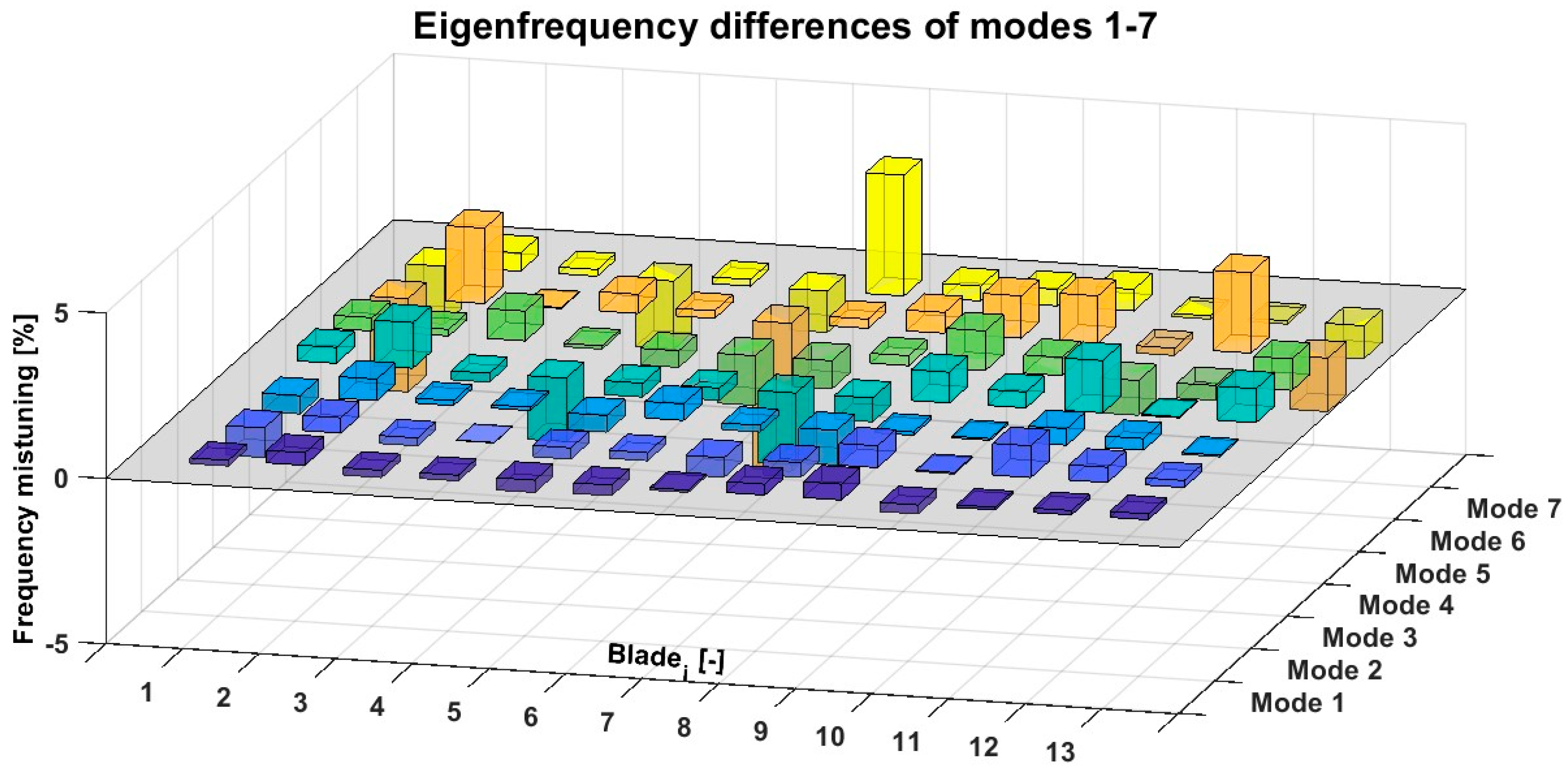
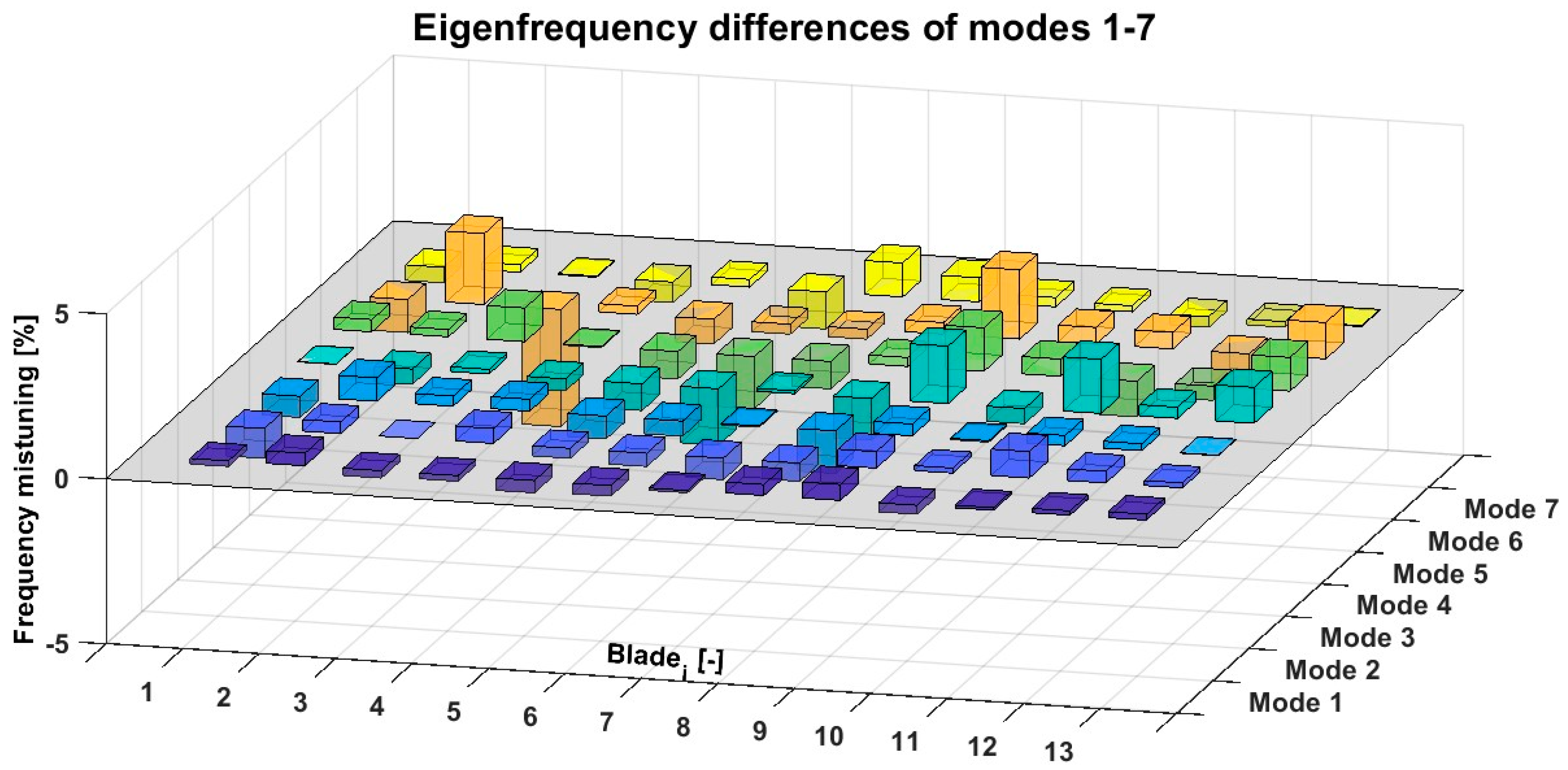
| Mode [-] | Frequency [Hz] |
|---|---|
| 1 | 4075.7 |
| 2 | 7020.7 |
| 3 | 8556.3 |
| 4 | 10,664.0 |
| 5 | 11,831.0 |
| 6 | 14,548.0 |
| 7 | 16,108.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kučera, O.; Píštěk, V.; Fomin, O.; Kučera, P.; Sagin, S. Measuring Device for More Precise Mistuning Identification of Integrated Bladed Discs. Symmetry 2025, 17, 717. https://doi.org/10.3390/sym17050717
Kučera O, Píštěk V, Fomin O, Kučera P, Sagin S. Measuring Device for More Precise Mistuning Identification of Integrated Bladed Discs. Symmetry. 2025; 17(5):717. https://doi.org/10.3390/sym17050717
Chicago/Turabian StyleKučera, Ondřej, Václav Píštěk, Oleksij Fomin, Pavel Kučera, and Sergii Sagin. 2025. "Measuring Device for More Precise Mistuning Identification of Integrated Bladed Discs" Symmetry 17, no. 5: 717. https://doi.org/10.3390/sym17050717
APA StyleKučera, O., Píštěk, V., Fomin, O., Kučera, P., & Sagin, S. (2025). Measuring Device for More Precise Mistuning Identification of Integrated Bladed Discs. Symmetry, 17(5), 717. https://doi.org/10.3390/sym17050717









