Nuclear Symmetry Energy Effects on Neutron Star Properties within Bogoliubov Quark–Meson Coupling Model
Abstract
:1. Introduction
2. Bogoliubov Quark Meson Coupling Model
3. Hadronic Matter
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Observation of Gravitational Waves from a Binary Neutron Star Inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [CrossRef] [PubMed]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. GW170817: Measurements of Neutron Star Radii and Equation of State. Phys. Rev. Lett. 2018, 121, 161101. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. Multi-messenger Observations of a Binary Neutron Star Merger*. Astrophys. J. Lett. 2017, 848, L12. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; et al. GW190425: Observation of a Compact Binary Coalescence with Total Mass ∼3.4 M⊙. Astrophys. J. Lett. 2020, 892, L3. [Google Scholar] [CrossRef]
- Demorest, P.; Pennucci, T.; Ransom, S.M.; Roberts, M.S.E.; Hessels, J.W.T. A two-solar-mass neutron star measured using Shapiro delay. Nature 2010, 467, 1081. [Google Scholar] [CrossRef]
- Fonseca, E.; Pennucci, T.T.; Ellis, J.A.; Stairs, I.H.; Nice, D.J.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Crowter, K.; Dolch, T.; et al. The NANOGrav Nine-year Data Set: Mass and Geometric Measurements of Binary Millisecond Pulsars. Astrophys. J. 2016, 832, 167. [Google Scholar] [CrossRef]
- Antoniadis, J.; Freire, P.C.C.; Wex, N.; Tauris, T.M.; Lynch, R.S.; van Kerkwijk, M.H.; Kramer, M.; Bassa, C.; Dhillon, V.S.; Driebe, T.; et al. A Massive Pulsar in a Compact Relativistic Binary. Science 2013, 340, 1233232. [Google Scholar] [CrossRef] [PubMed]
- Cromartie, H.T.; Fonseca, E.; Ransom, S.M.; Demorest, P.B.; Arzoumanian, Z.; Blumer, H.; Brook, P.R.; DeCesar, M.E.; Dolch, T.; Ellis, J.A.; et al. Relativistic Shapiro delay measurements of an extremely massive millisecond pulsar. Nat. Astron. 2020, 4, 72. [Google Scholar] [CrossRef]
- Fonseca, E.; Cromartie, H.T.; Pennucci, T.T.; Ray, P.S.; Kirichenko, A.Y.; Ransom, S.M.; Demorest, P.B.; Stairs, I.H.; Arzoumanian, Z.; Guillemot, L.; et al. Refined Mass and Geometric Measurements of the High-mass PSR J0740+6620. Astrophys. J. Lett. 2021, 915, L12. [Google Scholar] [CrossRef]
- Riley, T.E.; Watts, A.L.; Bogdanov, S.; Ray, P.S.; Ludlam, R.M.; Guillot, S.; Arzoumanian, Z.; Baker, C.L.; Bilous, A.V.; Chakrabarty, D.; et al. A NICER View of PSR J0030+0451: Millisecond Pulsar Parameter Estimation. Astrophys. J. Lett. 2019, 887, L21. [Google Scholar] [CrossRef]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Harding, A.K.; Ho, W.C.G.; Lattimer, J.M.; et al. PSR J0030+0451 Mass and Radius from NICER Data and Implications for the Properties of Neutron Star Matter. Astrophys. J. Lett. 2019, 887, L24. [Google Scholar] [CrossRef]
- Raaijmakers, G.; Riley, T.E.; Watts, A.L.; Greif, S.K.; Morsink, S.M.; Hebeler, K.; Schwenk, A.; Hinderer, T.; Nissanke, S.; Guillot, S.; et al. A NICER view of PSR J0030+0451: Implications for the dense matter equation of state. Astrophys. J. Lett. 2019, 887, L22. [Google Scholar] [CrossRef]
- Riley, T.E.; Watts, A.L.; Ray, P.S.; Bogdanov, S.; Guillot, S.; Morsink, S.M.; Bilous, A.V.; Arzoumanian, Z.; Choudhury, D.; Deneva, J.S.; et al. A NICER View of the Massive Pulsar PSR J0740+6620 Informed by Radio Timing and XMM-Newton Spectroscopy. Astrophys. J. Lett. 2021, 918, L27. [Google Scholar] [CrossRef]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Ho, W.C.G.; Lattimer, J.M.; Loewenstein, M.; et al. The Radius of PSR J0740+6620 from NICER and XMM-Newton Data. Astrophys. J. Lett. 2021, 918, L28. [Google Scholar] [CrossRef]
- Baldo, M.; Buballa, M.; Burgio, F.; Neumann, F.; Oertel, M.; Schulze, H.J. Neutron stars and the transition to color superconducting quark matter. Phys. Lett. B 2003, 562, 153–160. [Google Scholar] [CrossRef]
- Vidana, I.; Logoteta, D.; Providencia, C.; Polls, A.; Bombaci, I. Estimation of the effect of hyperonic three-body forces on the maximum mass of neutron stars. EPL 2011, 94, 11002. [Google Scholar] [CrossRef]
- Bednarek, I.; Haensel, P.; Zdunik, J.L.; Bejger, M.; Mańka, R. Hyperons in neutron-star cores and a 2 M⊙ pulsar. A&A 2012, 543, A157. [Google Scholar] [CrossRef]
- Weissenborn, S.; Chatterjee, D.; Schaffner-Bielich, J. Hyperons and massive neutron stars: Vector repulsion and SU(3) symmetry. Phys. Rev. C 2012, 85, 065802. [Google Scholar] [CrossRef]
- Weissenborn, S.; Chatterjee, D.; Schaffner-Bielich, J. Hyperons and massive neutron stars: Vector repulsion and strangeness. Nucl. Phys. A 2013, 914, 421–426. [Google Scholar] [CrossRef]
- Colucci, G.; Sedrakian, A. Equation of state of hypernuclear matter: Impact of hyperon-scalar-meson couplings. Phys. Rev. C 2013, 87, 055806. [Google Scholar] [CrossRef]
- Providência, C.; Rabhi, A. Interplay between the symmetry energy and the strangeness content of neutron stars. Phys. Rev. C 2013, 87, 055801. [Google Scholar] [CrossRef]
- van Dalen, E.; Colucci, G.; Sedrakian, A. Constraining hypernuclear density functional with λ hypernuclei and compact stars. Phys. Lett. B 2014, 734, 383–387. [Google Scholar] [CrossRef]
- Fortin, M.; Providência, C.; Raduta, A.R.; Gulminelli, F.; Zdunik, J.L.; Haensel, P.; Bejger, M. Neutron star radii and crusts: Uncertainties and unified equations of state. Phys. Rev. C 2016, 94, 035804. [Google Scholar] [CrossRef]
- Malik, T.; Providência, C. Bayesian inference of signatures of hyperons inside neutron stars. arXiv 2022, arXiv:2205.15843. [Google Scholar] [CrossRef]
- Bogolioubov, P.N. Sur un modèle à quarks quasi-indépendants. Ann. l’IHP Phys. Théorique 1968, 8, 163. [Google Scholar]
- Bohr, H.; Moszkowski, S.A.; Panda, P.K.; Providência, C.; da Providência, J. QMC approach based on the Bogoliubov independent quark model of the nucleon. Int. J. Mod. Phys. E 2016, 25, 1650007. [Google Scholar] [CrossRef]
- Panda, P.K.; Providência, C.; Moszkowski, S.A.; Bohr, H.; da Providência, J. Hyperonic stars within the Bogoliubov quark meson model for nuclear matter. Int. J. Mod. Phys. E 2019, 28, 1950034. [Google Scholar] [CrossRef]
- Rabhi, A.; Providência, C.; Moszkowski, S.A.; da Providência, J.A.; Bohr, H. Neutron stars within the Bogoliubov quark-meson coupling model. Phys. Rev. C 2021, 103, 035811. [Google Scholar] [CrossRef]
- Lattimer, J.M.; Lim, Y. Constraining the Symmetry Parameters of the Nuclear Interaction. Astrophys. J. 2013, 771, 51. [Google Scholar] [CrossRef]
- Oertel, M.; Hempel, M.; Klähn, T.; Typel, S. Equations of state for supernovae and compact stars. Rev. Mod. Phys. 2017, 89, 015007. [Google Scholar] [CrossRef]
- Adhikari, D.; Albataineh, H.; Androic, D.; Aniol, K.A.; Armstrong, D.S.; Averett, T.; Ayerbe Gayoso, C.; Barcus, S.K.; Bellini, V.; Beminiwattha, R.S.; et al. Accurate Determination of the Neutron Skin Thickness of 208Pb through Parity-Violation in Electron Scattering. Phys. Rev. Lett. 2021, 126, 172502. [Google Scholar] [CrossRef]
- Adhikari, D.; Albataineh, H.; Androic, D.; Aniol, K.A.; Armstrong, D.S.; Averett, T.; Ayerbe Gayoso, C.; Barcus, S.K.; Bellini, V.; Beminiwattha, R.S.; et al. Precision Determination of the Neutral Weak Form Factor of Ca48. Phys. Rev. Lett. 2022, 129, 042501. [Google Scholar] [CrossRef]
- Reinhard, P.G.; Roca-Maza, X.; Nazarewicz, W. Combined Theoretical Analysis of the Parity-Violating Asymmetry for Ca48 and Pb208. Phys. Rev. Lett. 2022, 129, 232501. [Google Scholar] [CrossRef] [PubMed]
- Mondal, C.; Gulminelli, F. Nucleonic metamodeling in light of multimessenger, PREX-II, and CREX data. Phys. Rev. C 2023, 107, 015801. [Google Scholar] [CrossRef]
- Reed, B.T.; Fattoyev, F.J.; Horowitz, C.J.; Piekarewicz, J. Density Dependence of the Symmetry Energy in the Post PREX-CREX Era. arXiv 2022, arXiv:2305.19376. [Google Scholar]
- Yakovlev, D.G.; Pethick, C.J. Neutron star cooling. Ann. Rev. Astron. Astrophys. 2004, 42, 169–210. [Google Scholar] [CrossRef]
- Horowitz, C.J.; Piekarewicz, J. Neutron star structure and the neutron radius of Pb-208. Phys. Rev. Lett. 2001, 86, 5647. [Google Scholar] [CrossRef] [PubMed]
- Carriere, J.; Horowitz, C.J.; Piekarewicz, J. Low mass neutron stars and the equation of state of dense matter. Astrophys. J. 2003, 593, 463–471. [Google Scholar] [CrossRef]
- Cavagnoli, R.; Menezes, D.P.; Providencia, C. Neutron star properties and the symmetry energy. Phys. Rev. C 2011, 84, 065810. [Google Scholar] [CrossRef]
- Gal, A.; Hungerford, E.V.; Millener, D.J. Strangeness in nuclear physics. Rev. Mod. Phys. 2016, 88, 035004. [Google Scholar] [CrossRef]
- Glashow, S.L. ϕω Mixing. Phys. Rev. Lett. 1963, 11, 48. [Google Scholar] [CrossRef]
- Bodmer, A.R. Collapsed Nuclei. Phys. Rev. D 1971, 4, 1601–1606. [Google Scholar] [CrossRef]
- Witten, E. Cosmic separation of phases. Phys. Rev. D 1984, 30, 272–285. [Google Scholar] [CrossRef]
- Stone, J.R.; Stone, N.J.; Moszkowski, S.A. Incompressibility in finite nuclei and nuclear matter. Phys. Rev. C 2014, 89, 044316. [Google Scholar] [CrossRef]
- Hebeler, K.; Lattimer, J.M.; Pethick, C.J.; Schwenk, A. Equation of state and neutron star properties constrained by nuclear physics and observation. Astrophys. J. 2013, 773, 11. [Google Scholar] [CrossRef]
- Providência, C.; Fortin, M.; Pais, H.; Rabhi, A. Hyperonic stars and the symmetry energy. arXiv 2018, arXiv:1811.00786. [Google Scholar] [CrossRef]
- Lattimer, J.M.; Prakash, M.; Pethick, C.J.; Haensel, P. Direct URCA process in neutron stars. Phys. Rev. Lett. 1991, 66, 2701–2704. [Google Scholar] [CrossRef]
- Tolman, R.C. Static Solutions of Einstein’s Field Equations for Spheres of Fluid. Phys. Rev. 1939, 55, 364–373. [Google Scholar] [CrossRef]
- Oppenheimer, J.R.; Volkoff, G.M. On Massive Neutron Cores. Phys. Rev. 1939, 55, 374–381. [Google Scholar] [CrossRef]
- Baym, G.; Pethick, C.; Sutherland, P. The ground state of matter at high densities: Equation of state and stellar models. Astrophys. J. 1971, 170, 299. [Google Scholar] [CrossRef]
- Pais, H.; Providência, C. Vlasov formalism for extended relativistic mean field models: The crust-core transition and the stellar matter equation of state. Phys. Rev. C 2016, 94, 015808. [Google Scholar] [CrossRef]
- Haensel, P.; Bejger, M.; Fortin, M.; Zdunik, L. Rotating neutron stars with exotic cores: Masses, radii, stability. Eur. Phys. J. A 2016, 52, 59. [Google Scholar] [CrossRef]
- De, S.; Finstad, D.; Lattimer, J.M.; Brown, D.A.; Berger, E.; Biwer, C.M. Tidal Deformabilities and Radii of Neutron Stars from the Observation of GW170817. Phys. Rev. Lett. 2018, 121, 091102. [Google Scholar] [CrossRef]
- Fattoyev, F.J.; Piekarewicz, J.; Horowitz, C.J. Neutron Skins and Neutron Stars in the Multimessenger Era. Phys. Rev. Lett. 2018, 120, 172702. [Google Scholar] [CrossRef]
- Malik, T.; Alam, N.; Fortin, M.; Providência, C.; Agrawal, B.K.; Jha, T.K.; Kumar, B.; Patra, S.K. GW170817: Constraining the nuclear matter equation of state from the neutron star tidal deformability. Phys. Rev. C 2018, 98, 035804. [Google Scholar] [CrossRef]
- Hinderer, T. Tidal Love Numbers of Neutron Stars. Astrophys. J. 2008, 677, 1216. [Google Scholar] [CrossRef]
- Abbott, B.P.; Abbott, R.; Abbott, T.D.; Acernese, F.; Ackley, K.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; Adya, V.B.; et al. Properties of the binary neutron star merger GW170817. Phys. Rev. X 2019, 9, 011001. [Google Scholar] [CrossRef]
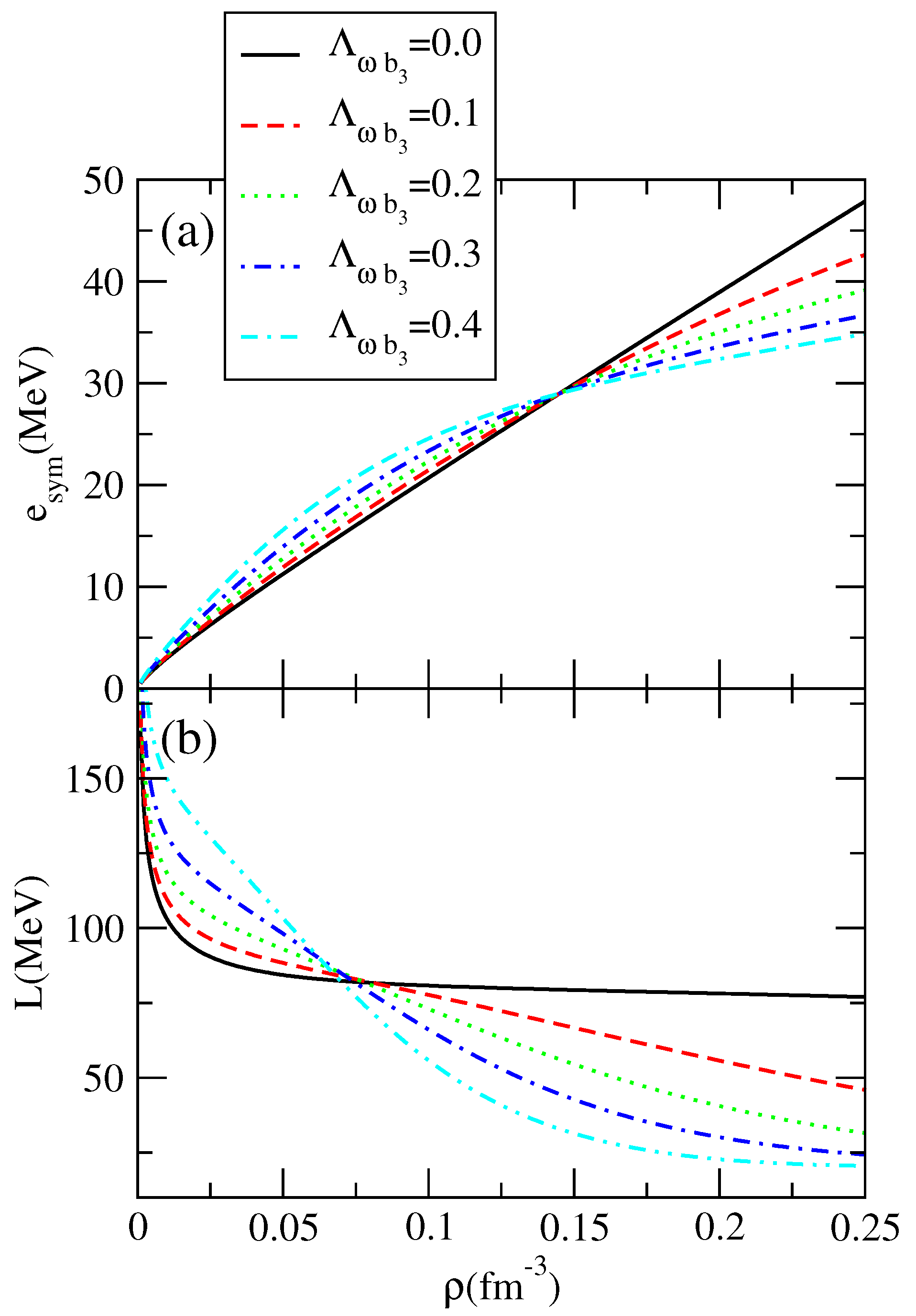
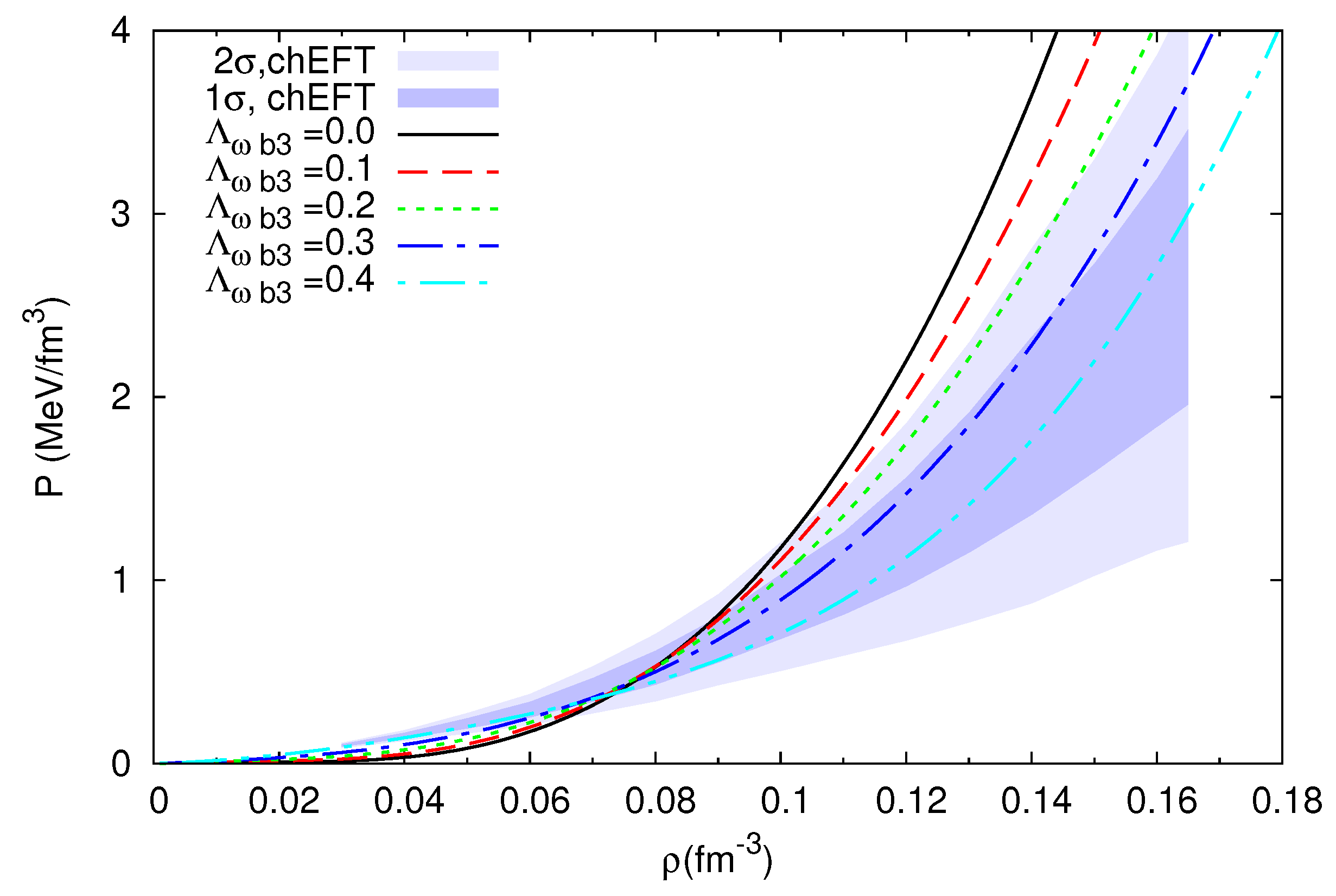
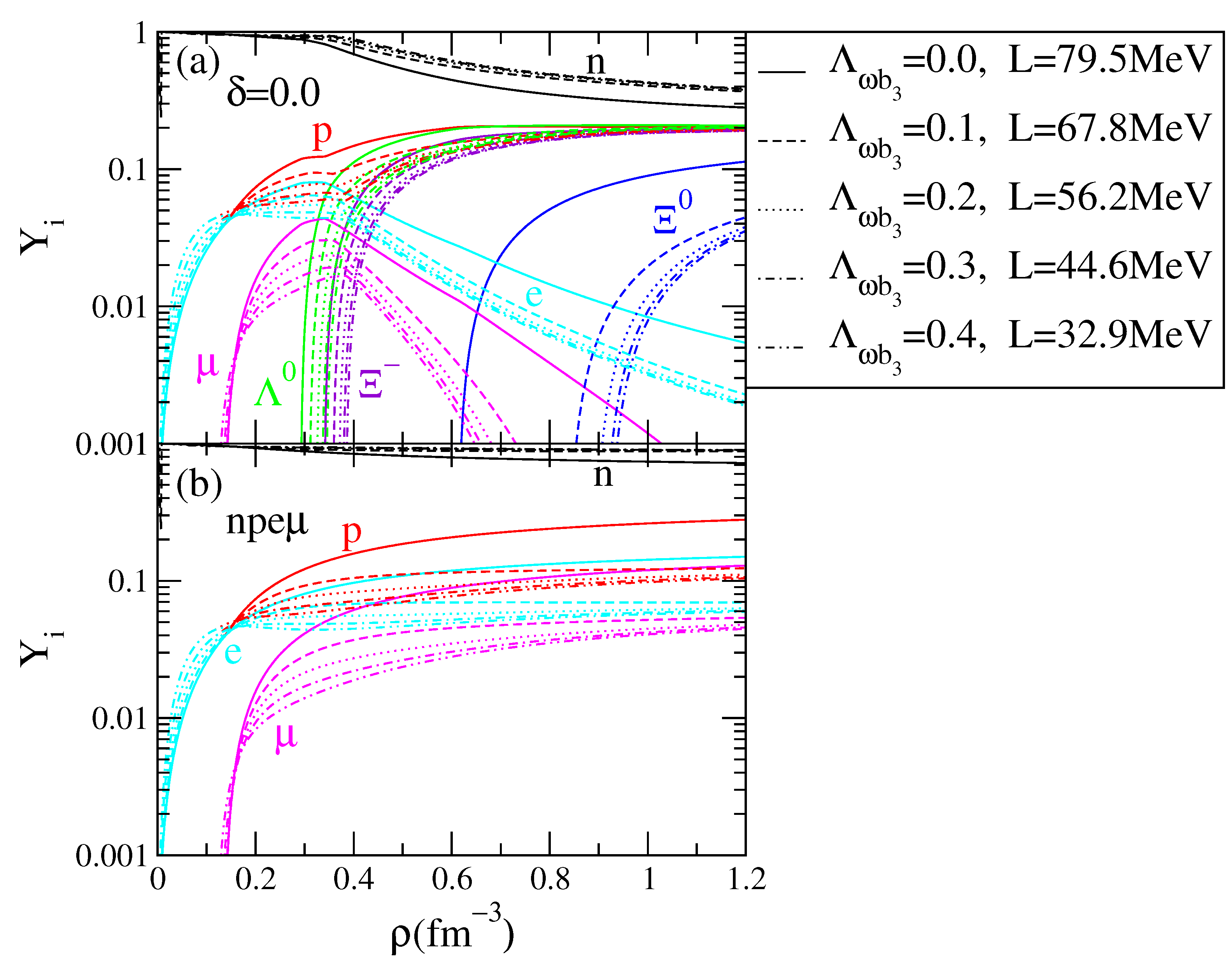
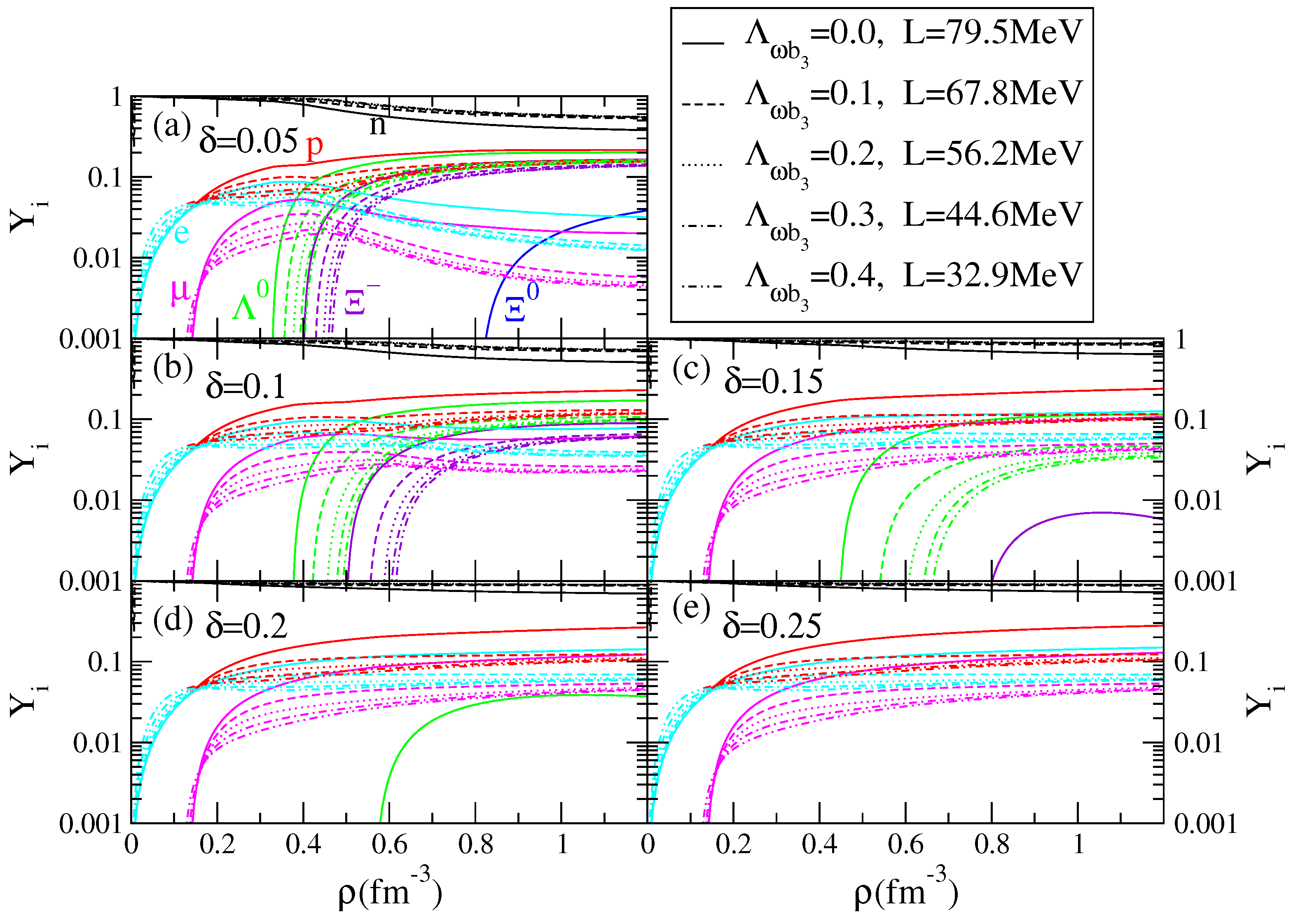
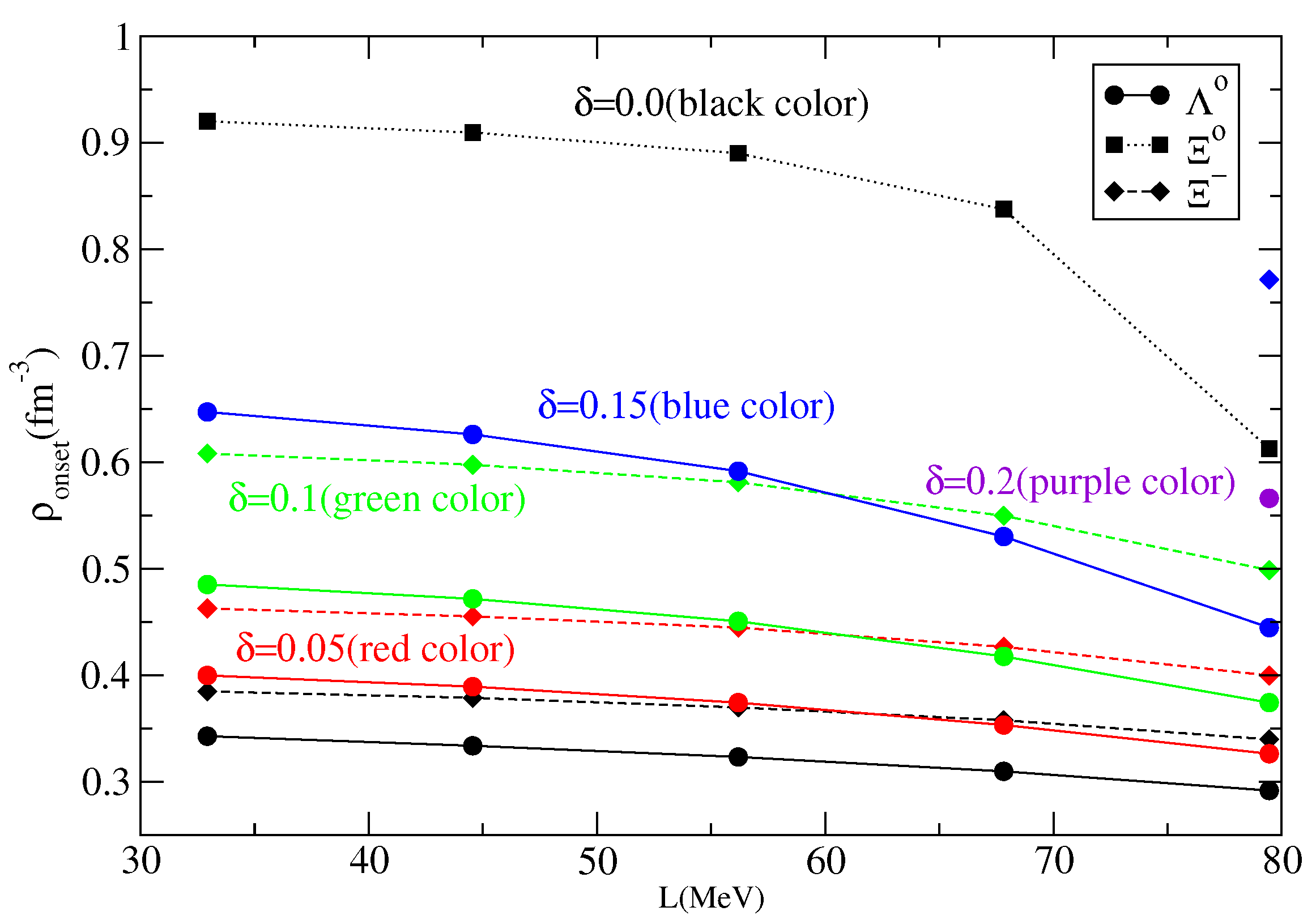
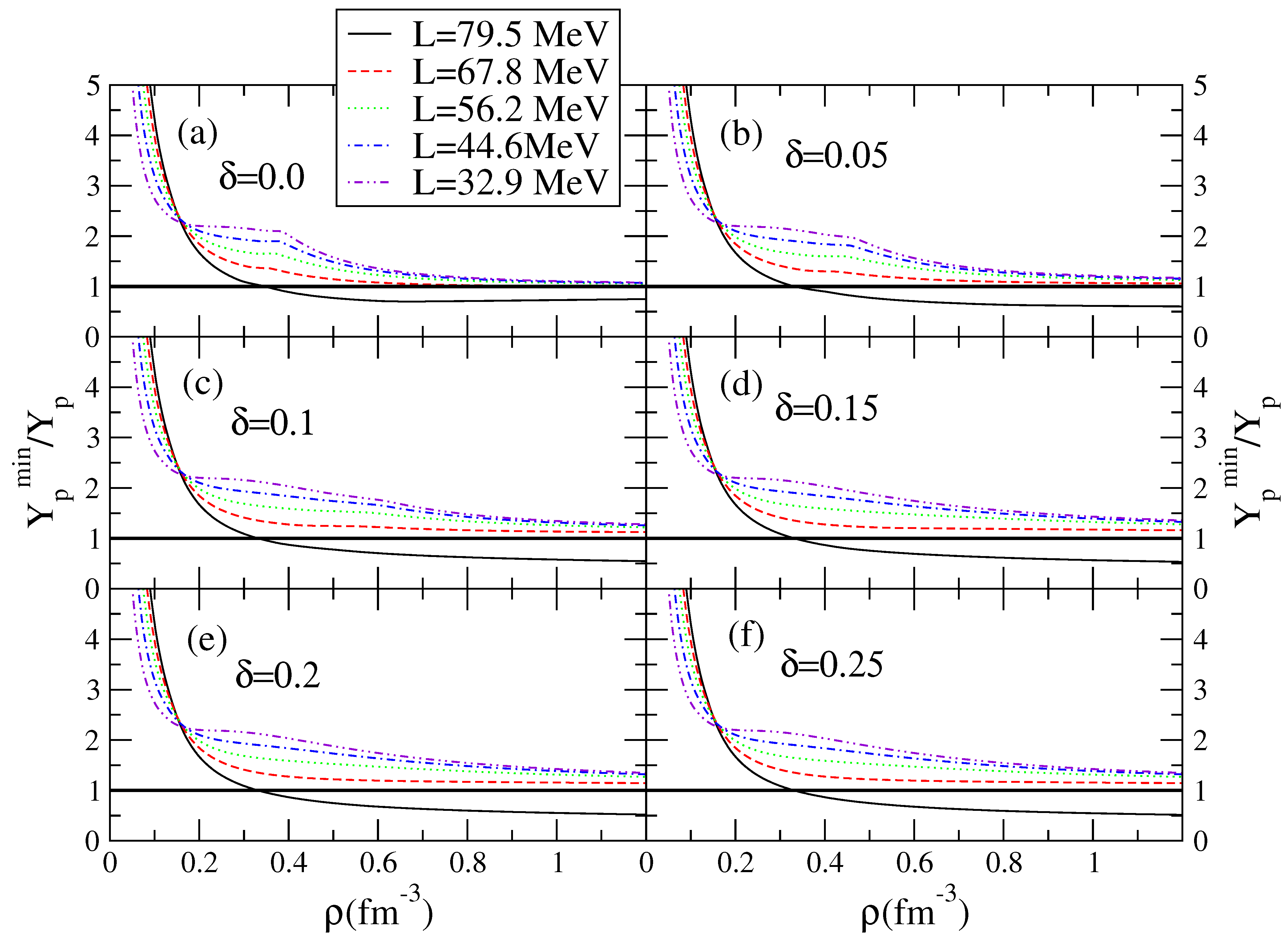
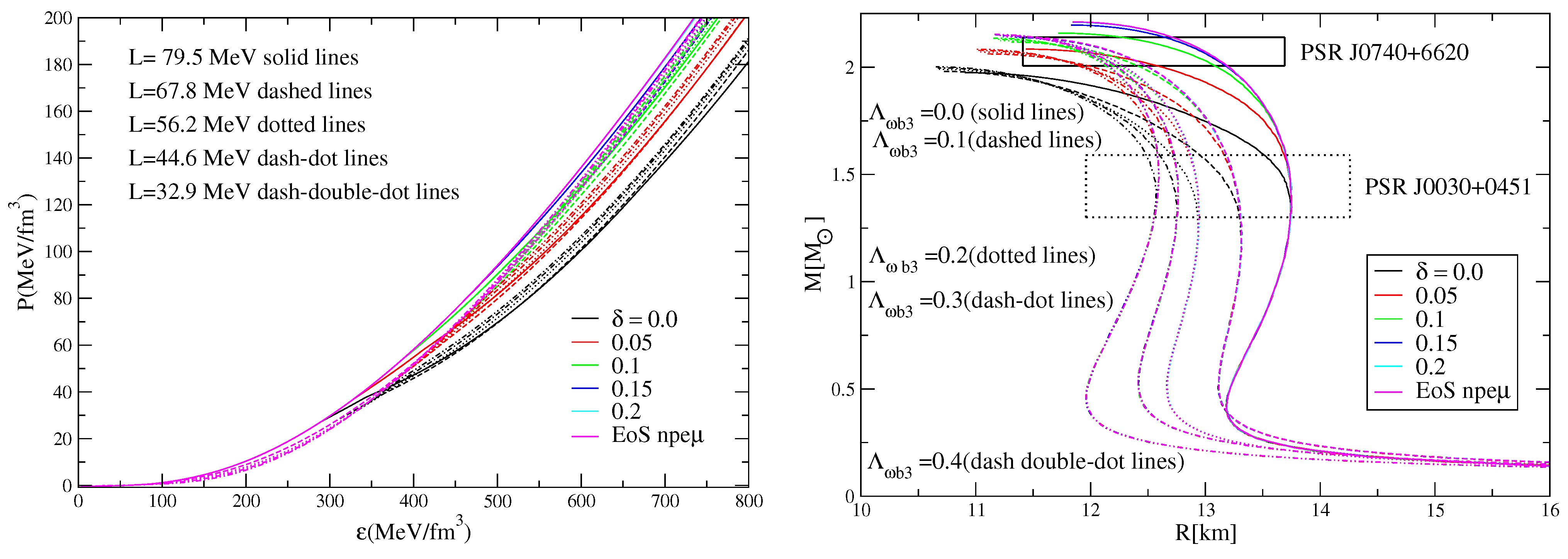

| L (MeV) | ||
|---|---|---|
| 3.953289 | 79.5 | |
| 4.23752 | 67.8 | |
| 4.593438 | 56.2 | |
| 5.057328 | 44.6 | |
| 5.697799 | 32.9 |
| L (MeV) | [fm] | [fm] | [fm] | ||
|---|---|---|---|---|---|
| 0.0 | 0.0 | 79.5 | 0.292 | 0.613 | 0.340 |
| 0.1 | 67.8 | 0.310 | 0.837 | 0.358 | |
| 0.2 | 56.2 | 0.323 | 0.890 | 0.370 | |
| 0.3 | 44.6 | 0.334 | 0.909 | 0.379 | |
| 0.4 | 32.9 | 0.343 | 0.920 | 0.385 | |
| 0.05 | 0.0 | 79.5 | 0.326 | - | 0.340 |
| 0.1 | 67.8 | 0.353 | - | 0.427 | |
| 0.2 | 56.2 | 0.374 | - | 0.445 | |
| 0.3 | 44.6 | 0.389 | - | 0.455 | |
| 0.4 | 32.9 | 0.400 | - | 0.463 | |
| 0.1 | 0.0 | 79.5 | 0.374 | - | 0.499 |
| 0.1 | 67.8 | 0.418 | - | 0.550 | |
| 0.2 | 56.2 | 0.4507 | - | 0.581 | |
| 0.3 | 44.6 | 0.472 | - | 0.598 | |
| 0.4 | 32.9 | 0.485 | - | 0.608 | |
| 0.15 | 0.0 | 79.5 | 0.445 | - | 0.771 |
| 0.1 | 67.8 | 0.530 | - | - | |
| 0.2 | 56.2 | 0.592 | - | - | |
| 0.3 | 44.6 | 0.626 | - | - | |
| 0.4 | 32.9 | 0.647 | - | - | |
| 0.2 | 0.0 | 79.5 | 0.566 | - | - |
| R [km] | [fm] | [km] | [km] | [km] | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.0 | 0.0 | 1.97 | 2.28 | 10.91 | 7.25 | 7.674 | 13.731 | 934.72 | 13.492 | 344.43 | - | - |
| 0.1 | 1.98 | 2.30 | 10.70 | 7.46 | 7.865 | 13.240 | 703.90 | 12.931 | 246.80 | - | - | |
| 0.2 | 1.99 | 2.32 | 10.66 | 7.40 | 7.821 | 12.907 | 615.35 | 12.691 | 227.53 | - | - | |
| 0.3 | 2.00 | 2.33 | 10.67 | 7.32 | 7.764 | 12.745 | 576.27 | 12.590 | 221.80 | - | - | |
| 0.4 | 2.00 | 2.34 | 10.65 | 7.26 | 7.720 | 12.568 | 555.19 | 12.478 | 219.74 | 10.80 | - | |
| 0.05 | 0.0 | 2.08 | 2.43 | 11.42 | 6.43 | 6.882 | 13.752 | 947.47 | 13.680 | 394.49 | 12.62 | 40.95 |
| 0.1 | 2.06 | 2.41 | 11.09 | 6.81 | 7.235 | 13.283 | 726.25 | 13.155 | 290.46 | 12.03 | 27.27 | |
| 0.2 | 2.07 | 2.43 | 11.04 | 6.75 | 7.197 | 12.936 | 631.51 | 12.855 | 257.87 | 11.95 | 27.76 | |
| 0.3 | 2.08 | 2.44 | 11.02 | 6.72 | 7.181 | 12.761 | 586.53 | 12.716 | 244.82 | 11.95 | 28.82 | |
| 0.4 | 2.08 | 2.45 | 11.00 | 6.66 | 7.135 | 12.582 | 561.35 | 12.580 | 239.44 | 11.93 | 29.79 | |
| 0.1 | 0.0 | 2.16 | 2.53 | 11.73 | 5.97 | 6.429 | 13.750 | 948.13 | 13.693 | 399.85 | 13.11 | 59.61 |
| 0.1 | 2.12 | 2.48 | 11.30 | 6.45 | 6.891 | 13.289 | 726.08 | 13.173 | 295.53 | 12.44 | 38.27 | |
| 0.2 | 2.12 | 2.50 | 11.21 | 6.44 | 6.896 | 12.937 | 631.23 | 12.864 | 260.34 | 12.27 | 36.18 | |
| 0.3 | 2.13 | 2.52 | 11.19 | 6.42 | 6.881 | 12.763 | 586.85 | 12.727 | 246.40 | 12.21 | 36.07 | |
| 0.4 | 2.14 | 2.53 | 11.15 | 6.40 | 6.866 | 12.586 | 561.33 | 12.580 | 239.44 | 12.15 | 36.34 | |
| 0.15 | 0.0 | 2.20 | 2.58 | 11.83 | 5.84 | 6.285 | 13.746 | 948.13 | 13.698 | 399.97 | 13.19 | 63.85 |
| 0.1 | 2.14 | 2.51 | 11.35 | 6.35 | 6.790 | 13.284 | 726.08 | 13.177 | 295.52 | 12.50 | 40.43 | |
| 0.2 | 2.14 | 2.53 | 11.24 | 6.41 | 6.842 | 12.939 | 631.23 | 12.864 | 260.34 | 12.30 | 37.36 | |
| 0.3 | 2.15 | 2.54 | 11.20 | 6.42 | 6.858 | 12.763 | 586.85 | 12.723 | 246.40 | 12.23 | 36.86 | |
| 0.4 | 2.15 | 2.55 | 11.16 | 6.39 | 6.844 | 12.581 | 561.33 | 12.584 | 239.44 | 12.17 | 36.94 | |
| 0.2 | 0.0 | 2.21 | 2.60 | 11.84 | 5.81 | 6.256 | 13.748 | 948.13 | 13.697 | 399.97 | 13.20 | 64.33 |
| 0.1 | 2.14 | 2.52 | 11.36 | 6.34 | 6.776 | 13.288 | 726.08 | 13.175 | 295.52 | 12.50 | 40.54 | |
| 0.2 | 2.14 | 2.53 | 11.24 | 6.41 | 6.843 | 12.939 | 631.23 | 12.866 | 260.34 | 12.30 | 37.37 | |
| 0.3 | 2.15 | 2.54 | 11.20 | 6.42 | 6.859 | 12.762 | 586.85 | 12.727 | 246.40 | 12.23 | 36.86 | |
| 0.4 | 2.15 | 2.55 | 11.15 | 6.40 | 6.844 | 12.585 | 561.33 | 12.579 | 239.44 | 12.17 | 36.94 | |
| npe | 0.0 | 2.21 | 2.60 | 11.84 | 5.84 | 6.272 | 13.746 | 948.13 | 13.696 | 399.97 | 13.20 | 64.33 |
| 0.1 | 2.14 | 2.52 | 11.36 | 6.36 | 6.790 | 13.283 | 726.08 | 13.177 | 295.52 | 12.50 | 40.54 | |
| 0.2 | 2.14 | 2.53 | 11.24 | 6.43 | 6.856 | 12.939 | 631.23 | 12.866 | 260.34 | 12.30 | 37.37 | |
| 0.3 | 2.15 | 2.54 | 11.20 | 6.42 | 6.859 | 12.762 | 586.85 | 12.727 | 246.40 | 12.23 | 36.86 | |
| 0.4 | 2.15 | 2.55 | 11.15 | 6.40 | 6.844 | 12.585 | 561.33 | 12.579 | 239.44 | 12.17 | 36.94 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boukari, O.; Rabhi, A. Nuclear Symmetry Energy Effects on Neutron Star Properties within Bogoliubov Quark–Meson Coupling Model. Symmetry 2023, 15, 1742. https://doi.org/10.3390/sym15091742
Boukari O, Rabhi A. Nuclear Symmetry Energy Effects on Neutron Star Properties within Bogoliubov Quark–Meson Coupling Model. Symmetry. 2023; 15(9):1742. https://doi.org/10.3390/sym15091742
Chicago/Turabian StyleBoukari, Olfa, and Aziz Rabhi. 2023. "Nuclear Symmetry Energy Effects on Neutron Star Properties within Bogoliubov Quark–Meson Coupling Model" Symmetry 15, no. 9: 1742. https://doi.org/10.3390/sym15091742
APA StyleBoukari, O., & Rabhi, A. (2023). Nuclear Symmetry Energy Effects on Neutron Star Properties within Bogoliubov Quark–Meson Coupling Model. Symmetry, 15(9), 1742. https://doi.org/10.3390/sym15091742






