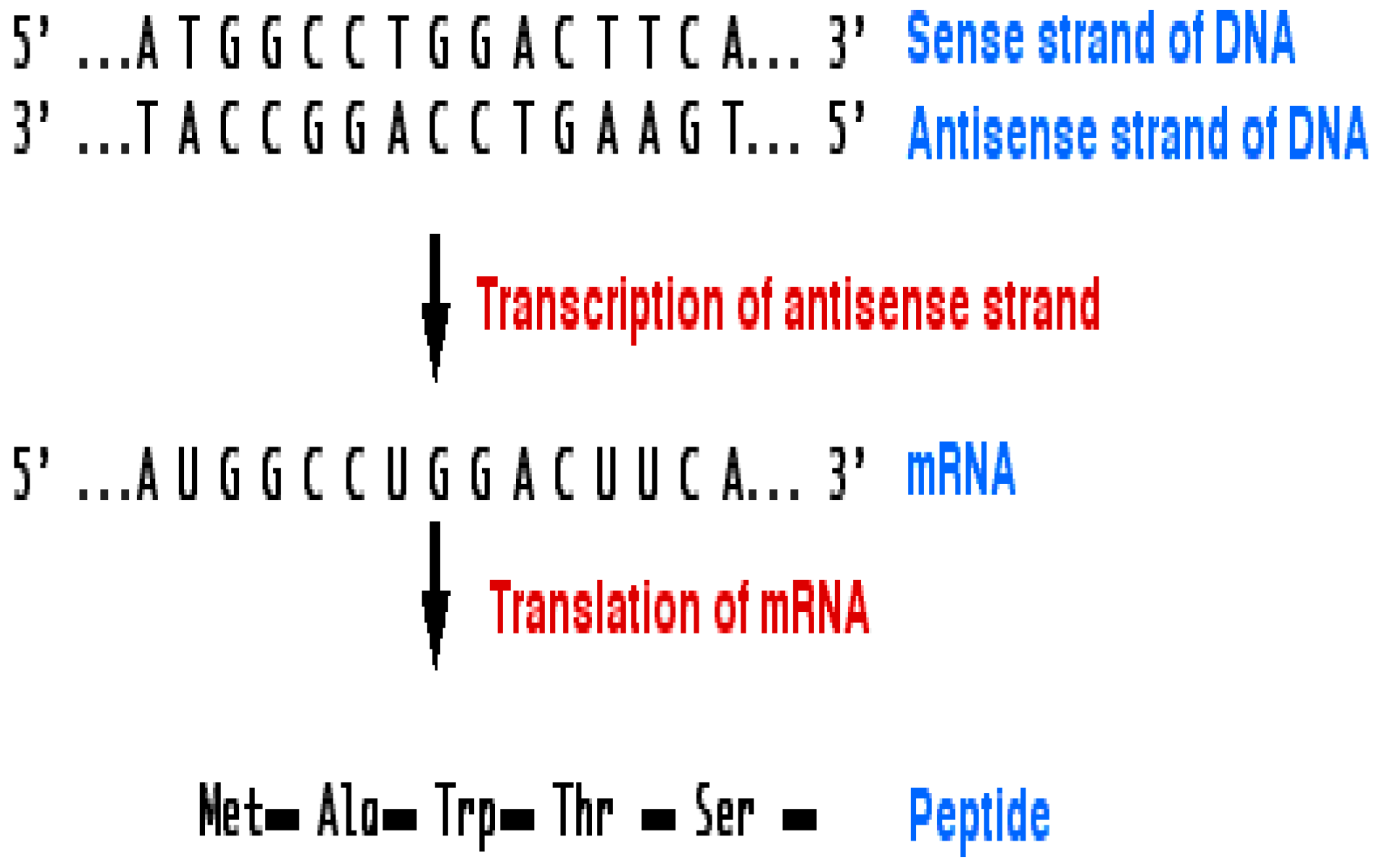
Mutations of Nucleic Acids via Matroidal Structures
Abstract
:1. Introduction
2. Basic Concepts on Matroid Theory
2.1. Matroid Theory
- (i)
- The members of T are called the independent sets of M and symbolised by IND (M).
- (ii)
- For any is said to be dependent if and is symbolised by D(M)
- (iii)
- A set in T that is maximal in the sense of inclusion is called a base of the matroid M and is symbolised by B(M)
- (iv)
- A minimal, in the sense of inclusion, dependent subset of E is called a circuit of the matroid M and is symbolised by C(M). The singleton circuit is called a loop. If {a,b} is a circuit, then a and b are said to be parallel.
- (v)
- The rank function of the matroid is a function , for .
- (vi)
- For each , the closure operator of a matrix M is defined as (A) = and (A) is called the closure of A in M. When there is confusion, we use the symbol . A is called a closed set if
2.2. Matroid and Matrices
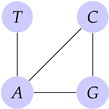
2.3. Matroids and Graph Theory
- (i)
- if A does not contain a cycle of G.
- (ii)
- B is a circuit of if B is a cycle of G.
- (iii)
- B is a base of if B is a spanning forest of G.
- (i)
- if and only if , equivalently, if and only if .
- (ii)
- if and only if
3. Matroidal Structure Induced by Topological Operators
- (i)
- .
- (ii)
- .
- (PCL1)
- (PCL2)
- If , then ;
- (PCL3)
- (PCL4)
- ;
- (PCL5)
- ;
- (PCL6)
- (PCL7)
- For any
- (i)
- By Definition 5, it is simple to verify this. = is a preclosure system on X.
- (ii)
- A preclosure operator that satisfies (PCL4) and (PCL5) is called a Kuratowski preclosure operator (KPO), which determines a supra topology on X.
- (iii)
- A matroid structure is defined by a preclosure operator that satisfies (PCL6), which we refer to as a matroidal preclosure operator (MPO) and is defined by
- (i)
- That is obvious
- (ii)
- If and and for every then Therefore, there exists a preopen set G where and so . By , since and for Therefore, and
- (iii)
- By the fact that for any subset A, we have that if since , therefore there exists where .
4. Mutations via Their Graph and Matroidal Structures
DNA Structure and Mutations
- (i)
- A mutation that exchanges one base for another is called substitution as in Figure 6.
- (ii)
- Insertion mutation occurs when extra base pairs are inserted as in Figure 7.
- (iii)
- If a section of DNA is lost or deleted, then the mutation is called a deletion as in Figure 8.

| Algorithm 1: Mutation via matroids and graphs. |
Input: A graph G = (V,E) from DNA stand. Output: The existence of mutation in DNA or not
|
| Tair Accession: | 1005028114. |
| GenBank Accession: | AF068299. |
| Sequence Length | 5277. |
| A | T | C | G | |
|---|---|---|---|---|
| A | 0 | 1859 | 0 | 0 |
| T | 1543 | 0 | 0 | 0 |
| C | 0 | 0 | 0 | 1019 |
| G | 0 | 0 | 856 | 0 |

5. Matroidal Structure of DNA via Matrices
| Algorithm 2: Mutation via matroids and matrices. |
Input: A matrix (aij) from DNA stand, where i indicates to row and j indicates to column. Output: the existence of mutation in DNA or not.
|
- (i)
- Consider the following DNA strand, and . By Algorithm 2, the matrix , where the row represents the first tape in wild type and the column represents the second tape in wild type , if , the structure for the matrix We observe that which means that all vectors of matroid are independent. Then there is no mutation in this DNA sequence.
- (ii)
- Consider the following DNA strand, and By Algorithm 2, the matrix if the structure for the matrix M2 is = . We observe that C() = which means that not all vectors of the matroid are independent. There is substitution mutation in this DNA strand.
6. A Similarity and Dissimilarity between the Sequences of DNA
- (i)
- A similarity = = 1; dissimilarity=.
- (ii)
- A similarity =; dissimilarity=.
7. Conclusions and Future Work
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhu, W.; Wang, S. Matroidal approaches to generalized rough sets based on relations. Int. J. Mach. Learn. Cybern. 2011, 2, 273–279. [Google Scholar] [CrossRef]
- Wang, S.; Zhu, W. Matroidal structure of covering-based rough sets through the upper approximation number. Int. J. Granular Comput. Rough Sets Intell. Syst. 2011, 2, 141–148. [Google Scholar] [CrossRef]
- Tang, J.; She, K.; Min, F.; Zhu, W. A matroidal approach to rough set theory. Theory Comput. Sci. 2013, 471, 1–11. [Google Scholar] [CrossRef]
- Oxley, J. Matroid Theory, 2nd ed.; Oxford University Press: New York, NY, USA, 2011. [Google Scholar]
- Whitney, H. On the abstract properties of linear dependence. Am. J. Math. 1935, 57, 509–533. [Google Scholar] [CrossRef]
- Oxley, J.G. Matroid Theory; Oxford University Press: New York, NY, USA, 1992. [Google Scholar]
- Atik, A.E. Approximation of simplicial complexes using matroids and rough sets. Soft Comput. 2023, 27, 2217–2229. [Google Scholar] [CrossRef]
- Atik, A.E.; Ali, M.E. Matroidal and Lattices Structures of Rough Sets and Some of Their Topological Characterizations. Inf. Sci. Lett. 2022, 11, 331–341. [Google Scholar]
- Atik, A.E.; Haroun, S. A topological representation of matroids using graphs. Int. J. Math. Comput. Sci. 2022, 17, 1079–1086. [Google Scholar]
- Bone, M.; Vernizzi, G.; Orland, H.; Zee, A. Topological classification of RNA structures. J. Mol. 2008, 379, 900–911. [Google Scholar] [CrossRef]
- Pervouchine, D.D. Circular exonic RNAs: When RNA structure meets topology. BBA Gene Regul. Mech. 2019, 1862, 194384. [Google Scholar] [CrossRef]
- Qiu, W.; Xin, H. Topological structure of closed circular DNA. J. Mol. Struct. Theochem 1998, 428, 35–39. [Google Scholar] [CrossRef]
- Silva-Santiago, E.; Pardo, J.P.; Hernandes-Munoz, R.; Aranda-Anzaldo, A. The nuclear higher-order structure defined by the set of topological relationships between DNA and the nuclear matrix is species-specific in hepatocytes. Gene 2017, 597, 40–48. [Google Scholar] [CrossRef] [PubMed]
- Adams, C.C.; Robert, D.F. Introduction to Topology: Pure and Applied; Pearson Prentice Hall: Upper Saddle River, NJ, USA, 2008. [Google Scholar]
- Bondy, J.A.; Murty, U.S.R. Graph Theory with Applications; Macmillan: London, UK, 1976; Volume 290. [Google Scholar]
- Diestel, R. Graph Theory, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Nada, S.I.; El-Atik, A.A.; Atef, M. New types of topological structure via graphes. Math. Methods Appl. Sci. 2018, 41, 5801–5810. [Google Scholar] [CrossRef]
- El-Sharkasy, M.M.; Badr, M.S. Modeling DNA and RNA mutation using mset and topology. Int. J. Biomath. 2018, 11, 18500584. [Google Scholar] [CrossRef]
- El-Atik, A.; Tashkandy, Y.; Jafari, S.; Nasef, A.A.; Emam, W.; Badr, M. Mutation of DNA and RNA sequences through the application of topological spaces. AIMS Math. 2023, 8, 19275–19296. [Google Scholar] [CrossRef]
- El-Bably, M.K.; Abu-Gdairi, R.; El-Gayar, M.A. Medical diagnosis for the problem of Chikungunya disease using soft rough sets. AIMS Math. 2023, 8, 9082–9105. [Google Scholar] [CrossRef]
- Hosny, A.R.; Abu-Gdairi, R.; El-Bably, M.K. Approximations by Ideal Minimal Structure with Chemical Application. Intell. Autom. Soft Comput. 2023, 36, 3073–3085. [Google Scholar] [CrossRef]
- Gioan, E. Complete graph drawings up to triangle mutations. Discret. Comput. Geom. 2022, 67, 985–1022. [Google Scholar] [CrossRef]
- Nieto, J.A.; Nieto-Marín, C.C.; Nieto-Marín, N.; Nieto-Marín, I. New mathematical tools for the study of the DNA structure. J. Appl. Math. Phys. 2021, 9, 1896–1903. [Google Scholar] [CrossRef]
- Bonin, J.; Oxley, J.G. Matroid Theory. Grad. Texts Math. 1996, 197, 234–260. [Google Scholar]
- Lai, H. Matroidal Theory; Higher Education Press: Beijing, China, 2001. [Google Scholar]
- Li, X.; Liu, S. Matroidal approaches to rough set theory via closure operator. Int. J. Approx. Reason. 2012, 53, 513–527. [Google Scholar] [CrossRef]
- Wang, Z.; Yanping, L. The relationships between degree rough sets and matroids. Anals Fuzzy Math. Inform. 2012, 12, 139–153. [Google Scholar]
- Nasef, A.A.; Jafari, S.; Caldas, M.; Latif, R.M.; Azzam, A.A. preclosure operator and its applications in general topology. J. Linear Topol. Algebra 2018, 7, 1–9. [Google Scholar]
- Crick, F.; Anderson, P.W. What mad pursuit: A personal view of scientific discovery. Phys. Today 1989, 17, 42–68. [Google Scholar] [CrossRef]
- Nirenberg, M.W.; Matthaei, J.H. The dependence of cell-free protein synthesis in E. coli upon naturally occurring or synthetic polyribonucleotides. Proc. Nat. Acad. Sci. USA 1961, 47, 1588–1602. [Google Scholar] [CrossRef]
- Blikstad, J.; Mukhopadhyay, S.; Nanongkai, D.; Tu, T.W. Fast Algorithms via Dynamic-Oracle Matroids. In Proceedings of the 55th Annual ACM Symposium on Theory of Computing, Orlando, FL, USA, 20–23 June 2023; pp. 1229–1242. [Google Scholar]
- Baiou, M.; Barahona, F. On some algorithmic aspects of hypergraphic matroids. Discret. Math. 2023, 346, 113222. [Google Scholar] [CrossRef]
- Available online: https://www.ncbi.nlm.nih.gov (accessed on 1 January 2023).
- Nieto, J.J.; Torres, A.; Georgiou, D.N.; Karakasidis, T.E. Fuzzy polynucleotide spaces and metrics. Bull. Math. Biol. 2006, 68, 703–725. [Google Scholar] [CrossRef]
- Georgiou, D.N.; Karakasidis, T.E.; Nieto, J.J.; Torres, A. A study of entropy clarity of genetic sequences using metric spaces and fuzzy sets. J. Theory Biol. 2010, 267, 95–105. [Google Scholar] [CrossRef]



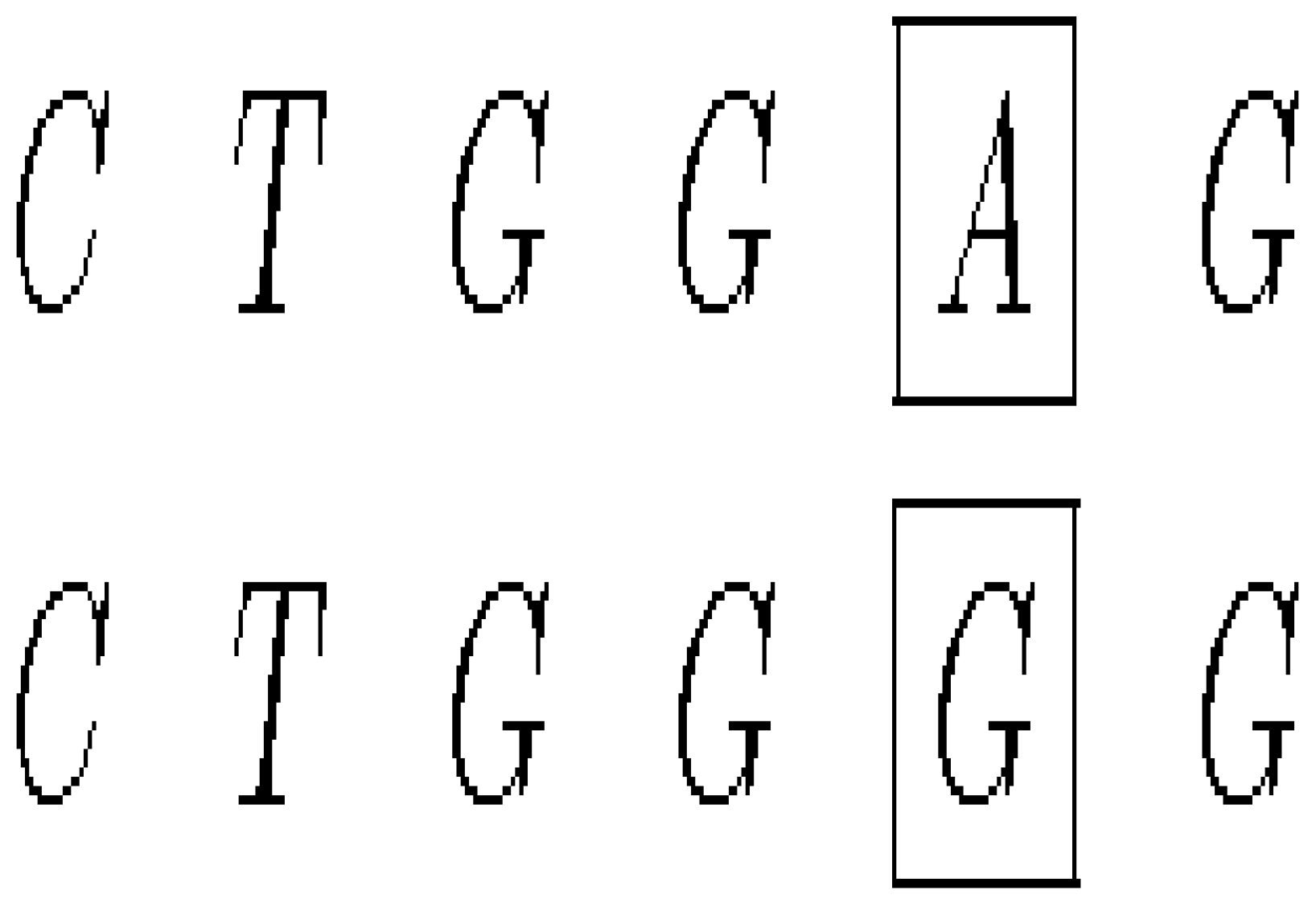
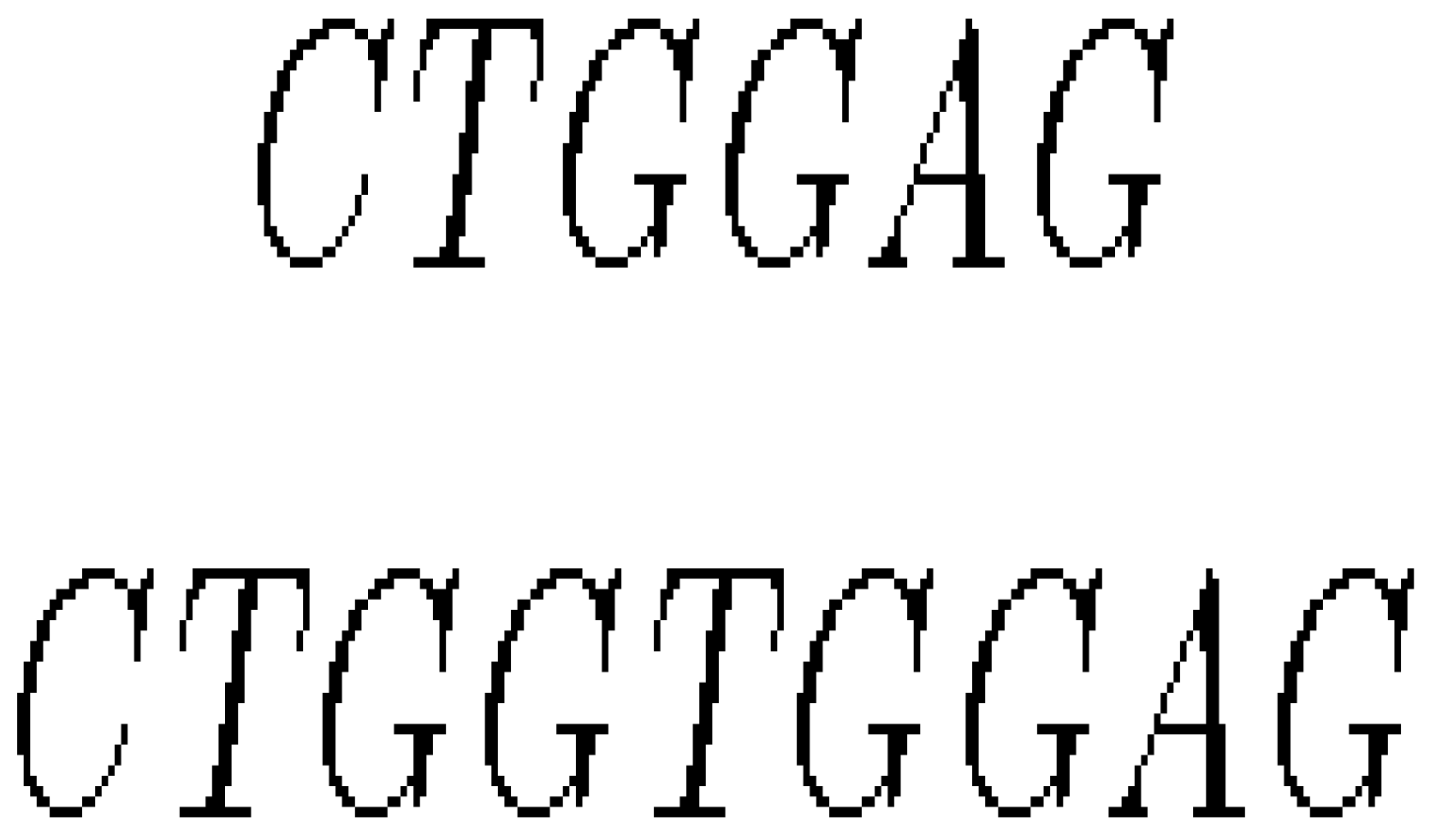
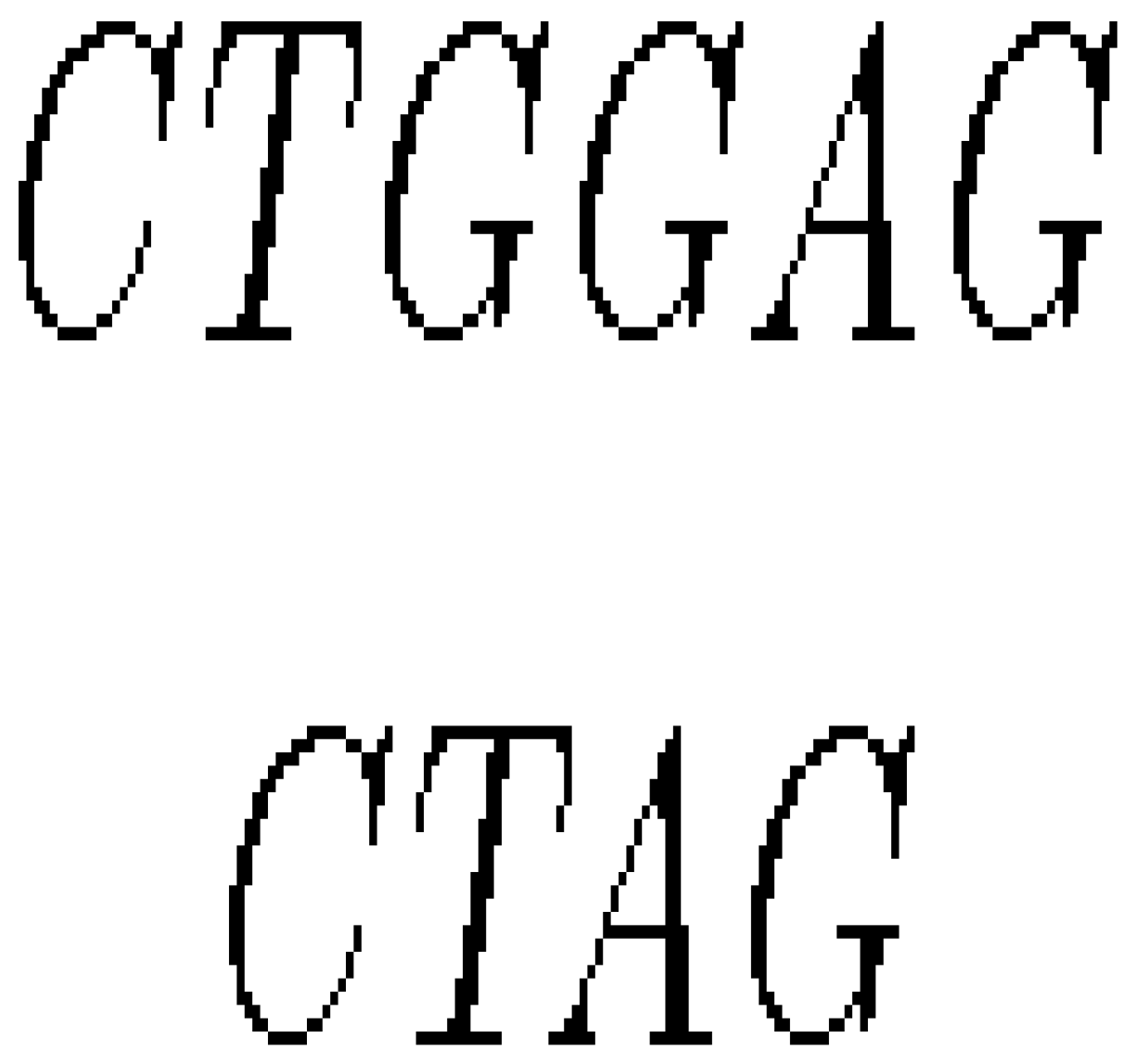

| A | T | C | G | |
|---|---|---|---|---|
| A | 203 | 1351 | 175 | 130 |
| T | 1091 | 154 | 124 | 171 |
| C | 130 | 202 | 47 | 633 |
| G | 118 | 149 | 510 | 78 |
| A | T | C | G | |
|---|---|---|---|---|
| A | 0 | 1859 | 0 | 0 |
| T | 1543 | 0 | 0 | 0 |
| C | 0 | 0 | 0 | 1019 |
| G | 0 | 0 | 856 | 0 |
| A | T | C | G | |
|---|---|---|---|---|
| A | 0 | 1 | 0 | 0 |
| T | 1 | 0 | 0 | 0 |
| C | 0 | 0 | 0 | 1 |
| G | 0 | 0 | 1 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Badr, M.; Abu-Gdairi, R.; Nasef, A.A. Mutations of Nucleic Acids via Matroidal Structures. Symmetry 2023, 15, 1741. https://doi.org/10.3390/sym15091741
Badr M, Abu-Gdairi R, Nasef AA. Mutations of Nucleic Acids via Matroidal Structures. Symmetry. 2023; 15(9):1741. https://doi.org/10.3390/sym15091741
Chicago/Turabian StyleBadr, M., Radwan Abu-Gdairi, and A. A. Nasef. 2023. "Mutations of Nucleic Acids via Matroidal Structures" Symmetry 15, no. 9: 1741. https://doi.org/10.3390/sym15091741
APA StyleBadr, M., Abu-Gdairi, R., & Nasef, A. A. (2023). Mutations of Nucleic Acids via Matroidal Structures. Symmetry, 15(9), 1741. https://doi.org/10.3390/sym15091741