New Efficient Computations with Symmetrical and Dynamic Analysis for Solving Higher-Order Fractional Partial Differential Equations

Abstract

Due to the rapid development of theoretical and computational techniques in the recent years, the role of nonlinearity in dynamical systems has attracted increasing interest and has been intensely investigated. A study of nonlinear waves in shallow water is presented in this paper. The classic form of the Korteweg–de Vries (KdV) equation is based on oceanography theory, shallow water waves in the sea, and internal ion-acoustic waves in plasma. A shallow fluid assumption is shown in the framework by a sequence of nonlinear fractional partial differential equations. Indeed, the primary purpose of this study is to use a semi-analytical technique based on Fractional Taylor Series to achieve numerical results for nonlinear fifth-order KdV models of non-integer order. Caputo is the operator used for dealing with fractional derivatives. The generated solutions of nonlinear fifth-order KdV models of non-integer order for modeling turbulence processes in the field of ocean engineering are compared analytically and numerically, to demonstrate the behaviors of several parameters of the current model. We verified the method’s convergence analysis and provided an error estimate by showing 2D and 3D graphs to further confirm its efficacy.

1. Introduction

Fractional differential equations (FDEs) are becoming increasingly popular in a variety of research and engineering applications. FDEs have piqued the interest of many scholars in the recent years due to their wide range of applications in the applied sciences. FDEs are ideal for representing a wide range of phenomena in electromagnetics, fluid mechanics, viscoelasticity, solid mechanics, biological population models, electrochemistry, and signal processing (see, for example, [1,2]).

In most circumstances, accurate solutions for FDEs are not accessible, hence, the use of various numerical approaches to suggest effective numerical solutions to tackle the various FDEs becomes important. Some FDEs are treated using wavelet approaches, as shown in [3,4]. A variety of studies, including [5,6,7], make use of operational matrix approaches. For some more numerical approaches that may be used to address various FDEs, see, for example [8,9,10].

The propagation of long waves, which is nonlinear in nature, in the environment is a physical phenomenon that has been summarized in a number of reviews, including those on the seas [11], stratified laboratory studies [12], and the earth’s atmosphere [13]. To explain the dispersion of these nonlinear long waves in a purely physical sense, many mathematical models have been developed. The majority of these models are derived from the well-known KdV equation, which is a generic model for studying weakly nonlinear long waves.

Studies showed that it originated by applying a multi-scale asymptotic procedure to the main Euler equations for incompressible and inviscid fluids, and it chiefly defined surface waves with small amplitudes and long wavelengths in shallow water [14] and internal waves in a shallow density-stratified fluid [15]. The KdV equation is derived by taking dispersion and nonlinearity of first-order only of a first-order perturbation expansion. However, in many circumstances, finer clarity is required when explaining physical processes. The effect of nonlinear and dispersive terms of higher order in physical systems, on the other hand, cannot be overlooked. In this situation, applying the perturbation procedure to the leading Euler equations while leaving second-order components out of the perturbation expansions result in the KdV-like equation of fifth-order. Marchant and Smyth [16,17] introduced the KdV-like equation of fifth-order in the background of propagating surface water waves to simulate more precisely the resonant flow of a fluid across the terrain. In [18], an equation of this sort was also created to investigate higher-order solitary-wave interactions.

The identical equation was obtained by the author in [19] to describe surface waves in shallow water exposed to a linear shear flow. The fifth-order KdV-like equation was developed for propagating internal waves in stratified media first by Koop and Butler [20] for a two-layer system, and later, by Lamb and Yan [21] for a continuous density stratification with no free surface and no fundamental shear flow. Pelinovsky et al. [22] then modified the same equation to incorporate a basic shear flow, but with no free surface. Furthermore, the fifth-order KdV-type equation was utilized to explain internal waves of modest amplitude in density-stratified fluids [23].

Many applications use Korteweg–de Vries (KdV)-type equations and their modifications. There are several forms of KdV equations. The third-order KdV equations are equations that represent the behavior of one-dimensional shallow water waves of modest but finite amplitude, for example, [24,25]. A modified third-order KdV problem was handled in [26] using the Petrov–Galerkin finite element technique. Some papers looked at fifth-order KdV equations. For example, the authors of [27] suggested a numerical method for dealing with fifth-order KdV equations. In [28], another strategy based on the decomposition method was used to handle the fifth-order KdV problem. In terms of seventh-order KdV equations, several explicit solutions of KdV–Burgers’ and Lax’s seventh-order KdV equations were published in [29].

Some writers looked at different sorts of fractional KdV equations.

A form of fractional KdV equation, for example, was handled using the fractional natural decomposition approach in [30]. The author of [31] used a Green’s function to solve the fractional KdV equation. For the time fractional KdV equation with a weak singularity solution, [32] employed the Galerkin technique. Many writers presented numerical solutions to time FPDEs with a second-order partial derivative, for example, [33,34,35]; however, numerical studies of time FPDEs with a third-order partial derivative remain insufficient. This piques our curiosity in researching such issues. The authors suggested a Petrov–Galerkin spectral approach for dealing with the linearized time fractional KdV problem in [36]. There are several ways for dealing with some fractional KdV equations, such as [37,38].

We will concentrate on getting a semi-analytic solution to the aforementioned problem in this paper. We will use a novel analytical approach for this aim. The authors of [39] pioneered this technique. Many scholars are interested in the Novel Analytical approach, which is a novel efficient method for solving partial differential equations. Some of these may be found in [40,41,42,43,44,45,46,47,48,49,50,51,52,53,54,55].

The remainder of the article is arranged as follows. Some important definitions of fractional calculus theory are given in Section 2. Section 3 is limited to suggesting a numerical technique for numerically treating the fractional fifth-order KdV problem using the Fractional Novel Analytical Method (FNAM). Section 4 discusses the investigation of the numerical problems by the above-mentioned method with the help of error graphs. Graphs for different values of alpha are also presented in this section. In addition, some estimates for truncation and global errors are included in this section. Finally, in Section 5, some findings are offered.

2. Preliminaries of Fractional Calculus

We introduce the basic definitions and properties of fractional calculus [39,40,41,42] in this section.

Definition 1.

A real function $\mathfrak{G}(\rho )$, $\rho >0$ is said to be in space ${\mathbb{C}}_{\upsilon }$, $\upsilon \in \mathbb{R}$ if ∃ a real number $p>\upsilon$ such that $\mathfrak{G}(\rho )={\rho }^p{\mathfrak{G}}_1(\rho )$ where ${\mathfrak{G}}_1(\rho )\in \mathbb{C}(0,+\infty )$ and it is said to be in the space ${\mathbb{C}}_{\upsilon }^{\aleph }$ if ${\mathfrak{G}}^{\aleph }\in {\mathbb{C}}_{\upsilon }$, $\aleph \in \mathbb{N}$.

Definition 2.

The Riemann–Liouville (RL) Fractional Integral (FI) operator of order α, of a function $\mathfrak{G}\in {\mathbb{C}}_{\upsilon }$, $\upsilon >-1$, is defined as:

$$J^{\alpha }\mathfrak{G}(\rho )={\displaystyle \frac{1}{\Gamma \left[\alpha \right]}}{\int }_0^{\rho }{(\rho -\varsigma )}^{\alpha -1}\mathfrak{G}(\varsigma )d\varsigma ,\alpha >0$$

$$J^0\mathfrak{G}(\rho )=\mathfrak{G}(\rho )$$

Many authors have studied recently different inequalities of RL-FI’s, for further details, see [39,40,41,42]. We need here some properties of the operator $J^{\alpha }$, which are as follows: for $\mathfrak{G}\in {\mathbb{C}}_{\upsilon }$, $\upsilon \ge -1$, $\alpha ,\beta \ge 0$

$$J^{\alpha }{J}^{\beta }\mathfrak{G}(\rho )=J^{\alpha +\beta }\mathfrak{G}(\rho )$$

$$J^{\alpha }{\rho }^n={\displaystyle \frac{\Gamma [n+1]}{\Gamma [n+\alpha +1]}}{\rho }^{\alpha +n}$$

Definition 3.

The Fractional Derivative (FD) of $\mathfrak{G}(\rho )$ in the Caputo sense [42] is defined as:

$$D^{\alpha }\mathfrak{G}(\rho )=J^{m-\alpha }{D}^m\mathfrak{G}(\rho )$$

for $m-1<\alpha \le m$, $m\in \mathbb{N}$, $\rho >0$ & $\mathfrak{G}\in {\mathbb{C}}_{-1}^m$. In Caputo FD, an ordinary derivative is estimated followed by an FI to attain the desired order of FD. The RL-FI operator is a linear operation, defined as:

$$J^{\alpha }\left(\sum _{\ell =1}^h{C}_{\ell }{\mathfrak{G}}_{\ell }(\rho )\right)=\sum _{\ell =1}^h{C}_{\ell }{J}^{\alpha }{\mathfrak{G}}_{\ell }(\rho )$$

where ${\left\{C_{\ell }\right\}}_{\ell }^h$ are constants. In this study, FD’s are considered in the Caputo sense.

3. Fractional Novel Analytical Method for Fifth-Order Fractional Korteweg–De Vries Equations

We will discuss the elementary concepts of constructing an FNAM for the fifth-order Fractional Korteweg–de Vries Equation (FKDVE) in this section. Consider the following general Fractional Order FKdvE:

$$D_{\eta }^{2\delta }\varphi (\rho ,\eta )=\mathcal{H}\left(D_{\eta }^{\delta }\varphi ,\varphi ,D_{\rho }^{\delta }\varphi ,D_{\rho }^{2\delta }\varphi ,D_{\rho }^{3\delta }\varphi ,D_{\rho }^{4\delta }\varphi ,D_{\rho }^{5\delta }\varphi ,\cdots \right)$$

(1)

with initial condition,

$$\varphi (\rho ,0)={\chi }_0(\rho ),D_{\eta }^{\delta }\varphi (\rho ,0)={\chi }_1(\rho ).$$

(2)

Taking the Fractional Integral (FI) on both sides of Equation (1) from 0 to $\eta$, we get

$$D_{\eta }^{\delta }\varphi (\rho ,\eta )={\chi }_1(\rho )+I_{\eta }^{\delta }\mathcal{H}\left[\varphi \right]$$

(3)

where $\mathcal{H}\left[\varphi \right]=\mathcal{H}\left(D_{\eta }^{\delta }\varphi ,\varphi ,D_{\rho }^{\delta }\varphi ,D_{\rho }^{2\delta }\varphi ,D_{\rho }^{3\delta }\varphi ,D_{\rho }^{4\delta }\varphi ,D_{\rho }^{5\delta }\varphi ,\cdots \right)$. Then, again taking the FI from 0 to $\eta$, on both sides of Equation (3), we obtain

$$\varphi (\rho ,\eta )={\chi }_0(\rho )+{\chi }_1(\rho ){\displaystyle \frac{{\eta }^{\delta }}{\Gamma (\delta +1)}}+I_{\eta }^{2\delta }\mathcal{H}\left[\phi \right].$$

(4)

For $\mathcal{H}\left[\varphi \right]$, the Fractional Taylor Series (FTS) is extended about $\eta =0$.

$$\mathcal{H}\left[\varphi \right]=\sum _{j=0}^{+\infty }{\displaystyle \frac{D_{\eta }^{j\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma [j\delta +1]}}{\eta }^{j\delta },\delta >0,$$

$$\mathcal{H}\left[\varphi \right]=\mathcal{H}\left[{\varphi }_0\right]+{\displaystyle \frac{D_{\eta }^{\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma [\delta +1]}}{\eta }^{\delta }+{\displaystyle \frac{D_{\eta }^{2\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma [2\delta +1]}}{\eta }^{2\delta }+{\displaystyle \frac{D_{\eta }^{3\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma [3\delta +1]}}{\eta }^{3\delta }+\cdots +{\displaystyle \frac{D_{\eta }^{j\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma [j\delta +1]}}{\eta }^{j\delta }+\cdots .$$

(5)

Substituting Equation (5) by Equation (4), we obtain

$$\varphi (\rho ,\eta )={\chi }_0(\rho )+{\chi }_1(\rho ){\displaystyle \frac{{\eta }^{\delta }}{\Gamma (\delta +1)}}+I_{\eta }^{2\delta }\left[\mathcal{H}\left[{\varphi }_0\right]+{\displaystyle \frac{D_{\eta }^{\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma [\delta +1]}}{\eta }^{\delta }+{\displaystyle \frac{D_{\eta }^{2\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma [2\delta +1]}}{\eta }^{2\delta }+\cdots +{\displaystyle \frac{D_{\eta }^{j\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma [j\delta +1]}}{\eta }^{j\delta }+\cdots \right]$$

$$\varphi (\rho ,\eta )={\chi }_0(\rho )+{\chi }_1(\rho ){\displaystyle \frac{{\eta }^{\delta }}{\Gamma (\delta +1)}}+{\displaystyle \frac{\mathcal{H}\left[{\varphi }_0\right]}{\Gamma (2\delta +1)}}{\eta }^{2\delta }+{\displaystyle \frac{D_{\eta }^{\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma (3\delta +1)}}{\eta }^{3\delta }+{\displaystyle \frac{D_{\eta }^{2\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma (4\delta +1)}}{\eta }^{4\delta }+\cdots +{\displaystyle \frac{D_{\eta }^{j\delta }\mathcal{H}\left[{\varphi }_0\right]}{\Gamma ((j+2)\delta +1)}}{\eta }^{(j+2)\delta }+\cdots$$

$$\varphi (\rho ,\eta )={\mathfrak{a}}_0+{\mathfrak{a}}_1{\displaystyle \frac{{\eta }^{\delta }}{\Gamma (\delta +1)}}+{\mathfrak{a}}_2{\displaystyle \frac{{\eta }^{2\delta }}{\Gamma (2\delta +1)}}+{\mathfrak{a}}_3{\displaystyle \frac{{\eta }^{3\delta }}{\Gamma (3\delta +1)}}+{\mathfrak{a}}_4{\displaystyle \frac{{\eta }^{4\delta }}{\Gamma (4\delta +1)}}+\cdots +{\mathfrak{a}}_j{\displaystyle \frac{{\eta }^{j\delta }}{\Gamma (j\delta +1)}}+\cdots ,$$

(6)

where

$$\begin{array}{cc}\hfill {\mathfrak{a}}_0& ={\chi }_0(\rho ),\hfill \\ \hfill {\mathfrak{a}}_1& ={\chi }_1(\rho ),\hfill \\ \hfill {\mathfrak{a}}_2& =\mathcal{H}\left[{\varphi }_0\right],\hfill \\ \hfill {\mathfrak{a}}_3& =D_{\eta }^{\delta }\mathcal{H}\left[{\varphi }_0\right],\hfill \\ \hfill {\mathfrak{a}}_4& =D_{\eta }^{2\delta }\mathcal{H}\left[{\varphi }_0\right],\hfill \\ \hfill ⋮\\ \hfill {\mathfrak{a}}_j& =D_{\eta }^{(j-2)\delta }\mathcal{H}\left[{\varphi }_0\right],\hfill \end{array}$$

such that the highest derivative of $\varphi$ is j. The endorsement of Equation (6) is to extend FTS for $\varphi$ about $\eta =0$. It means that

$$\begin{array}{cc}\hfill {\mathfrak{a}}_0& =\varphi (\rho ,0),\hfill \\ \hfill {\mathfrak{a}}_1& =D_{\eta }^{\delta }\varphi (\rho ,0),\hfill \\ \hfill {\mathfrak{a}}_2& =D_{\eta }^{2\delta }\varphi (\rho ,0),\hfill \\ \hfill {\mathfrak{a}}_3& =D_{\eta }^{3\delta }\varphi (\rho ,0),\hfill \\ \hfill {\mathfrak{a}}_4& =D_{\eta }^{4\delta }\varphi (\rho ,0),\hfill \\ \hfill ⋮\\ \hfill {\mathfrak{a}}_j& =D_{\eta }^{j\delta }\varphi (\rho ,0).\hfill \end{array}$$

So, we can obtain our desired numerical solution easily. Convergence of this method is discussed in [39,40,41].

4. Numerical Application

In this section, four problems based on fifth-order Fractional Korteweg–de Vries Equations are solved by using the proposed Fractional Novel Analytical Method.

Problem 1.

Consider the Nonlinear fifth-order Fractional Korteweg–de Vries Equation [43],

$$D_{\eta }^{\delta }\varphi (\rho ,\eta )=-{\displaystyle \frac{\partial \varphi (\rho ,\eta )}{\partial \rho }}-{\varphi }^2(\rho ,\eta ){\displaystyle \frac{{\partial }^2\varphi (\rho ,\eta )}{\partial {\rho }^2}}-{\displaystyle \frac{\partial \varphi (\rho ,\eta )}{\partial \rho }}{\displaystyle \frac{{\partial }^2\varphi (\rho ,\eta )}{\partial {\rho }^2}}+20{\varphi }^2(\rho ,\eta ){\displaystyle \frac{{\partial }^3\varphi (\rho ,\eta )}{\partial {\rho }^3}}-{\displaystyle \frac{{\partial }^5\varphi (\rho ,\eta )}{\partial {\rho }^5}},0<\delta \le 1$$

$$\varphi (\rho ,0)={\displaystyle \frac{1}{\rho }}.$$

By following the steps elaborated in the FNAM, we acquire the following series of solutions,

$$\varphi (\rho ,\eta )=\sum _{j=0}^{+\infty }{\displaystyle \frac{j!{\eta }^{j\delta }}{{\rho }^{j+1}\Gamma (j\delta +1)}}.$$

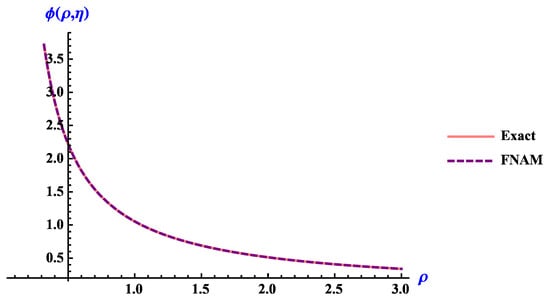
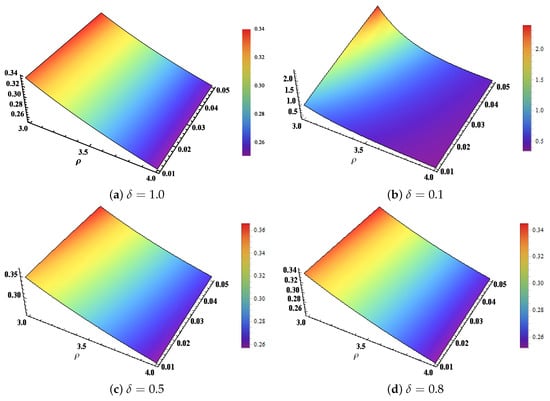
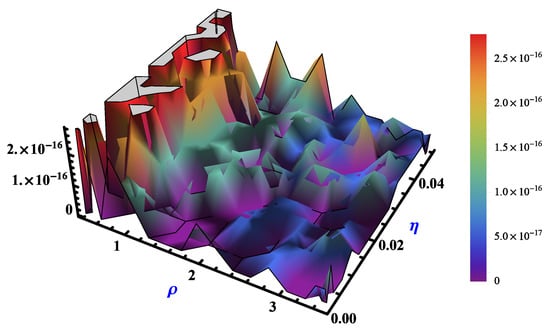
The Exact Solution of this problem at $\delta =1$ is $\varphi (\rho ,\eta )={\displaystyle \frac{1}{(\rho -\eta )}}$. In Figure 1, the comparison between exact and obtained solutions at $\eta =0.05$ are shown. 3-dimensional graphs of the obtained results at different values of δ are shown in Figure 2. The Absolute Error (AE) of the obtained solution is plotted in 3D in Figure 3 and a numerical comparison is given in

Table 1. Error comparison of $\varphi (\rho ,\eta )$ obtained by FNAM with solution obtained by Laplace Decomposition Method (LDM) from Ref. [43] at $\alpha =1$ for Problem 1.

$\mathit{\rho }$	0.5		1.0		1.5		2.0		2.5
$\mathit{\eta }$	FNAM	LDM	FNAM	LDM	FNAM	LDM	FNAM	LDM	FNAM	LDM
0.01	0.00	6.53 $\times {10}^{-09}$	0.00	2.13 $\times {10}^{-07}$	1.11 $\times {10}^{-16}$	1.65 $\times {10}^{-06}$	0.00	7.12 $\times {10}^{-06}$	5.55 $\times {10}^{-17}$	2.22 $\times {10}^{-05}$
0.02	8.88 $\times {10}^{-16}$	1.01 $\times {10}^{-10}$	0.00	3.26 $\times {10}^{-09}$	0.00	2.50 $\times {10}^{-08}$	0.00	1.06 $\times {10}^{-07}$	0.00	3.28 $\times {10}^{-07}$
0.03	7.72 $\times {10}^{-14}$	8.83 $\times {10}^{-12}$	2.22 $\times {10}^{-16}$	2.84 $\times {10}^{-10}$	0.00	2.17 $\times {10}^{-09}$	0.00	9.23 $\times {10}^{-09}$	5.55 $\times {10}^{-17}$	2.83 $\times {10}^{-08}$
0.04	1.86 $\times {10}^{-12}$	1.57 $\times {10}^{-12}$	4.44 $\times {10}^{-16}$	5.05 $\times {10}^{-11}$	0.00	13.85 $\times {10}^{-10}$	0.00	1.63 $\times {10}^{-09}$	5.55 $\times {10}^{-17}$	5.00 $\times {10}^{-09}$
0.05	2.22 $\times {10}^{-11}$	4.11 $\times {10}^{-13}$	5.10 $\times {10}^{-15}$	1.32 $\times {10}^{-11}$	1.11 $\times {10}^{-16}$	1.00 $\times {10}^{-10}$	1.11 $\times {10}^{-16}$	4.26 $\times {10}^{-10}$	5.55 $\times {10}^{-17}$	1.30 $\times {10}^{-09}$

.

Problem 2.

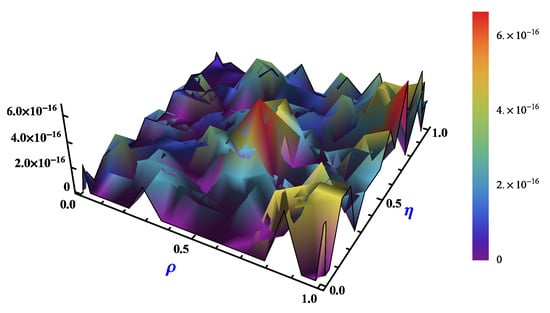
Consider the nonlinear fifth-order Fractional Korteweg–de Vries Equation [43],

$$D_{\eta }^{\delta }\varphi (\rho ,\eta )=-\varphi (\rho ,\eta ){\displaystyle \frac{\partial \varphi (\rho ,\eta )}{\partial \rho }}+\varphi (\rho ,\eta ){\displaystyle \frac{{\partial }^3\varphi (\rho ,\eta )}{\partial {\rho }^3}}-{\displaystyle \frac{{\partial }^5\varphi (\rho ,\eta )}{\partial {\rho }^5}},0<\delta \le 1,$$

$$\varphi (\rho ,0)=e^{\rho }.$$

By following the steps elaborated in the FNAM, we acquire the following series of solutions,

$$\varphi (\rho ,\eta )=\sum _{j=0}^{+\infty }{\displaystyle \frac{{(-1)}^j{e}^{\rho }{\eta }^{j\delta }}{\Gamma (j\delta +1)}}.$$

The exact solution of this problem at $\delta =1$ is $\varphi (\rho ,\eta )=e^{(\rho -\eta )}$. A comparison between exact and obtained solutions at $\eta =0.01$ is shown in Figure 4. The obtained solutions are plotted in 3D in Figure 5 at different values of δ. The 3D Absolute Error graph of the obtained result is shown in Figure 6 and a numerical comparison is given in

Table 2. Error comparison of $\varphi (\rho ,\eta )$ obtained by FNAM with solution obtained by Laplace Decomposition Method (LDM) from Ref. [43] at $\alpha =1$ for Problem 2.

$\mathit{\rho }$	0.5		1.0		1.5		2.0		2.5
$\mathit{\eta }$	FNAM	LDM	FNAM	LDM	FNAM	LDM	FNAM	LDM	FNAM	LDM
0.01	2.22 $\times {10}^{-16}$	1.37 $\times {10}^{-11}$	0.00	4.38 $\times {10}^{-11}$	0.00	3.32 $\times {10}^{-10}$	0.00	1.39 $\times {10}^{-09}$	3.55 $\times {10}^{-15}$	4.25 $\times {10}^{-09}$
0.02	0.00	2.26 $\times {10}^{-12}$	0.00	7.22 $\times {10}^{-11}$	8.88 $\times {10}^{-16}$	5.47 $\times {10}^{-10}$	0.00	2.30 $\times {10}^{-09}$	0.00	7.02 $\times {10}^{-09}$
0.03	0.00	3.72 $\times {10}^{-12}$	0.00	1.19 $\times {10}^{-10}$	8.88 $\times {10}^{-16}$	9.03 $\times {10}^{-10}$	0.00	3.79 $\times {10}^{-09}$	3.55 $\times {10}^{-15}$	1.15 $\times {10}^{-08}$
0.04	0.00	6.14 $\times {10}^{-12}$	4.44 $\times {10}^{-16}$	1.96 $\times {10}^{-10}$	8.88 $\times {10}^{-16}$	1.48 $\times {10}^{-09}$	0	6.26 $\times {10}^{-09}$	0.00	1.90 $\times {10}^{-08}$
0.05	0.00	1.01 $\times {10}^{-11}$	0.00	3.23 $\times {10}^{-10}$	0.00	2.45 $\times {10}^{-09}$	8.88 $\times {10}^{-16}$	1.03 $\times {10}^{-08}$	3.55 $\times {10}^{-15}$	3.14 $\times {10}^{-08}$

. The 2D plots of the obtained solutions at different values of δ are presented in Figure 7 at different δ values for $\eta =0.03$ and $\eta =0.05$.

Problem 3.

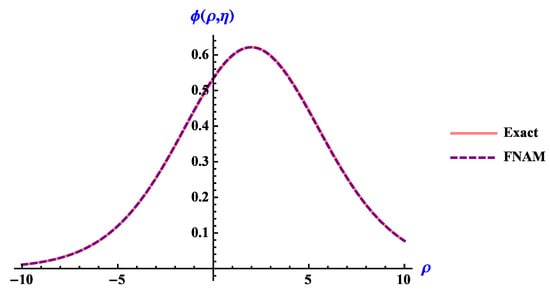
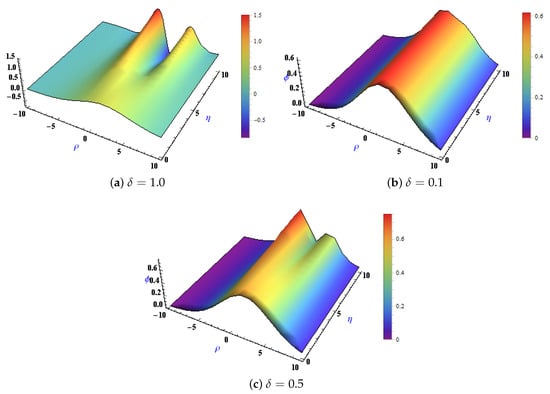
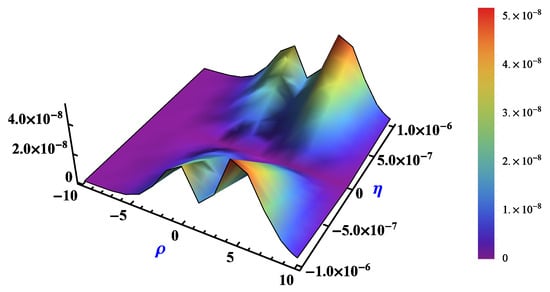
Consider the nonlinear fractional Kawahara Equation [43],

$$D_{\eta }^{\delta }\varphi (\rho ,\eta )=-\varphi (\rho ,\eta ){\displaystyle \frac{\partial \varphi (\rho ,\eta )}{\partial \rho }}-\varphi (\rho ,\eta ){\displaystyle \frac{{\partial }^3\varphi (\rho ,\eta )}{\partial {\rho }^3}}+{\displaystyle \frac{{\partial }^5\varphi (\rho ,\eta )}{\partial {\rho }^5}},0<\delta \le 1,$$

$$\varphi (\rho ,0)={\displaystyle \frac{105}{169}}sec{h}^4\left({\displaystyle \frac{1}{2\sqrt{13}}}(\rho -{\rho }_0)\right).$$

By following the steps elaborated in the FNAM, we acquire the following series of solutions,

$$\varphi (\rho ,\eta )={\displaystyle \frac{105}{169}}sec{h}^4\left({\displaystyle \frac{1}{2\sqrt{13}}}(\rho -{\rho }_0)\right)+{\displaystyle \frac{{\eta }^{\delta }}{\Gamma (\delta +1)}}\left(-{\displaystyle \frac{735sec{h}^6\left({\displaystyle \frac{1}{2\sqrt{13}}}(\rho -{\rho }_0)\right)tanh\left({\displaystyle \frac{1}{2\sqrt{13}}}(\rho -{\rho }_0)\right)}{2196\sqrt{13}}}-\cdots \right)+\cdots .$$

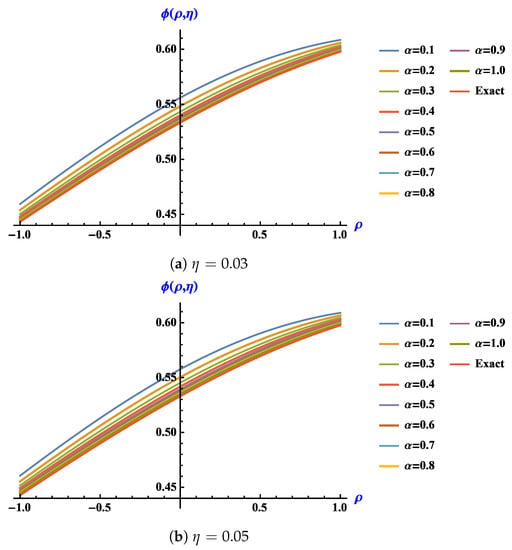
The exact solution of this problem at $\delta =1$ is $\varphi (\rho ,\eta )={\displaystyle \frac{105}{169}}sec{h}^4\left({\displaystyle \frac{1}{2\sqrt{13}}}(\rho -{\displaystyle \frac{36}{169}}\eta -{\rho }_0)\right)$. A comparison between exact and obtained solutions at $\eta =0.05$ and ${\rho }_0=2.0$ is plotted in Figure 8. 3D and 2D graphs of the obtained solutions at different values of δ are shown in Figure 9, Figure 10 and Figure 11, respectively. The 3D Absolute Error graph of the obtained result is shown in Figure 10 and a numerical comparison is given in

Table 3. Error comparison of $\varphi (\rho ,\eta )$ obtained by FNAM with solution obtained by Laplace Decomposition Method (LDM) from Ref. [43] at $\alpha =1$ for Problem 3.

$\mathit{\rho }$	0.5		1.0		1.5		2.0		2.5
$\mathit{\eta }$	FNAM	LDM	FNAM	LDM	FNAM	LDM	FNAM	LDM	FNAM	LDM
0.01	1.11 $\times {10}^{-16}$	1.11 $\times {10}^{-16}$	3.33 $\times {10}^{-16}$	0.00	2.22 $\times {10}^{-16}$	8.88 $\times {10}^{-16}$	0.00	2.66 $\times {10}^{-15}$	1.11 $\times {10}^{-16}$	8.44 $\times {10}^{-15}$
0.02	0.00	3.33 $\times {10}^{-16}$	0.00	0.00	0.00	7.77 $\times {10}^{-16}$	1.11 $\times {10}^{-16}$	2.55 $\times {10}^{-15}$	5.55 $\times {10}^{-16}$	6.66 $\times {10}^{-15}$
0.03	0.00	2.22 $\times {10}^{-16}$	3.33 $\times {10}^{-16}$	0.00	1.11 $\times {10}^{-16}$	2.22 $\times {10}^{-16}$	1.11 $\times {10}^{-16}$	7.77 $\times {10}^{-16}$	2.22 $\times {10}^{-16}$	3.89 $\times {10}^{-15}$
0.04	0.00	0.00	3.33 $\times {10}^{-16}$	1.11 $\times {10}^{-16}$	4.44 $\times {10}^{-16}$	1.11 $\times {10}^{-16}$	3.33 $\times {10}^{-16}$	2.22 $\times {10}^{-16}$	2.22 $\times {10}^{-16}$	2.22 $\times {10}^{-16}$
0.05	0.00	0.00	1.11 $\times {10}^{-16}$	5.55 $\times {10}^{-16}$	1.11 $\times {10}^{-16}$	4.44 $\times {10}^{-16}$	2.22 $\times {10}^{-16}$	1.44 $\times {10}^{-15}$	1.11 $\times {10}^{-16}$	3.77 $\times {10}^{-15}$

.

Problem 4.

Consider the nonlinear nonhomogeneous fifth-order Fractional Korteweg–de Vries Equation [43],

$$D_{\eta }^{\delta }\varphi (\rho ,\eta )-\varphi (\rho ,\eta ){\displaystyle \frac{\partial \varphi (\rho ,\eta )}{\partial \rho }}+{\displaystyle \frac{{\partial }^5\varphi (\rho ,\eta )}{\partial {\rho }^5}}=cos(\rho )+2\eta sin(\rho )+{\displaystyle \frac{{\rho }^2}{2}}sin(2\rho ),0<\delta \le 1,$$

$$\varphi (\rho ,0)=0.$$

By following the steps elaborated in the FNAM, we acquire the following series of solutions,

$$\varphi (\rho ,\eta )={\displaystyle \frac{{\eta }^{\delta }cos(\rho )}{\Gamma (\delta +1)}}.$$

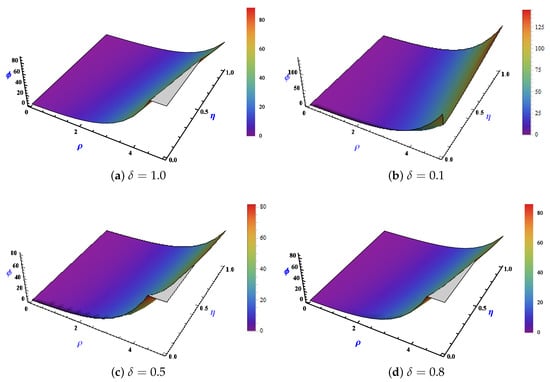
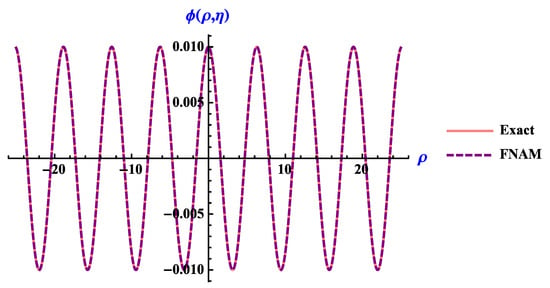
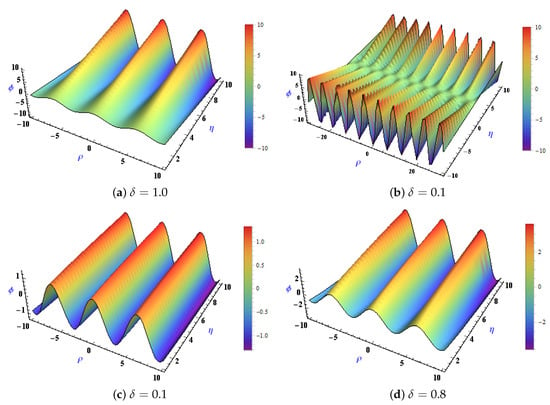
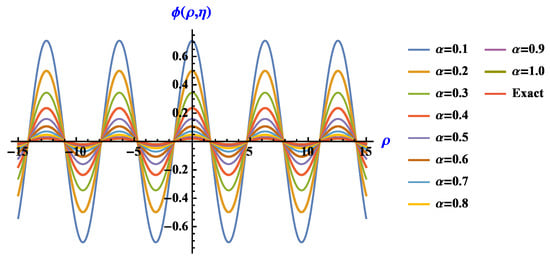
We canceled out the noise terms in the series solution of this equation. The exact solution of this problem at $\delta =1$ is $\varphi (\rho ,\eta )=\eta cos(\rho )$. A comparison between exact and obtained solutions is shown in Figure 12 at $\eta =0.01$. 3D and 2D graphs of the obtained solutions at different values of δ are plotted in Figure 13 and Figure 14, respectively.

5. Conclusions

The Fractional Novel Analytical Method (FNAM) is used to examine the numerical solutions of the fractional fifth-order KdV-like problem that naturally occur in maritime engineering. Caputo is the operator used for dealing with fractional derivatives. It is shown that the proposed method gives excellent results when applied to different nonlinear fifth-order KdV of non-integer order equations. The results obtained from the proposed method are more accurate and better than the results obtained from other methods, as shown in Table 1, Table 2 and Table 3. The solution of the nonlinear fractional fifth-order KdV differential equation converges to the solution of integer KdV differential equation, as shown in the figures. According to the convergence analysis, the error of the proposed method is reduced while increasing the number of iterations. We offer a specific numerical representation of the various solitary waves that may be formed from the resulting solution by altering the values of two model parameters. A broader examination of the provided solution can be gained by varying a greater number of parameters. The derived traveling wave solution and numerical drawings may be used to evaluate tsunami forecasts, atmospheric flows, storm surges, and flows around buildings.