Optimal Conformity Design of Tibial Insert Component Based on ISO Standard Wear Test Using Finite Element Analysis and Surrogate Model
Abstract
:1. Introduction
2. Materials and Methods
2.1. Modeling of TKA
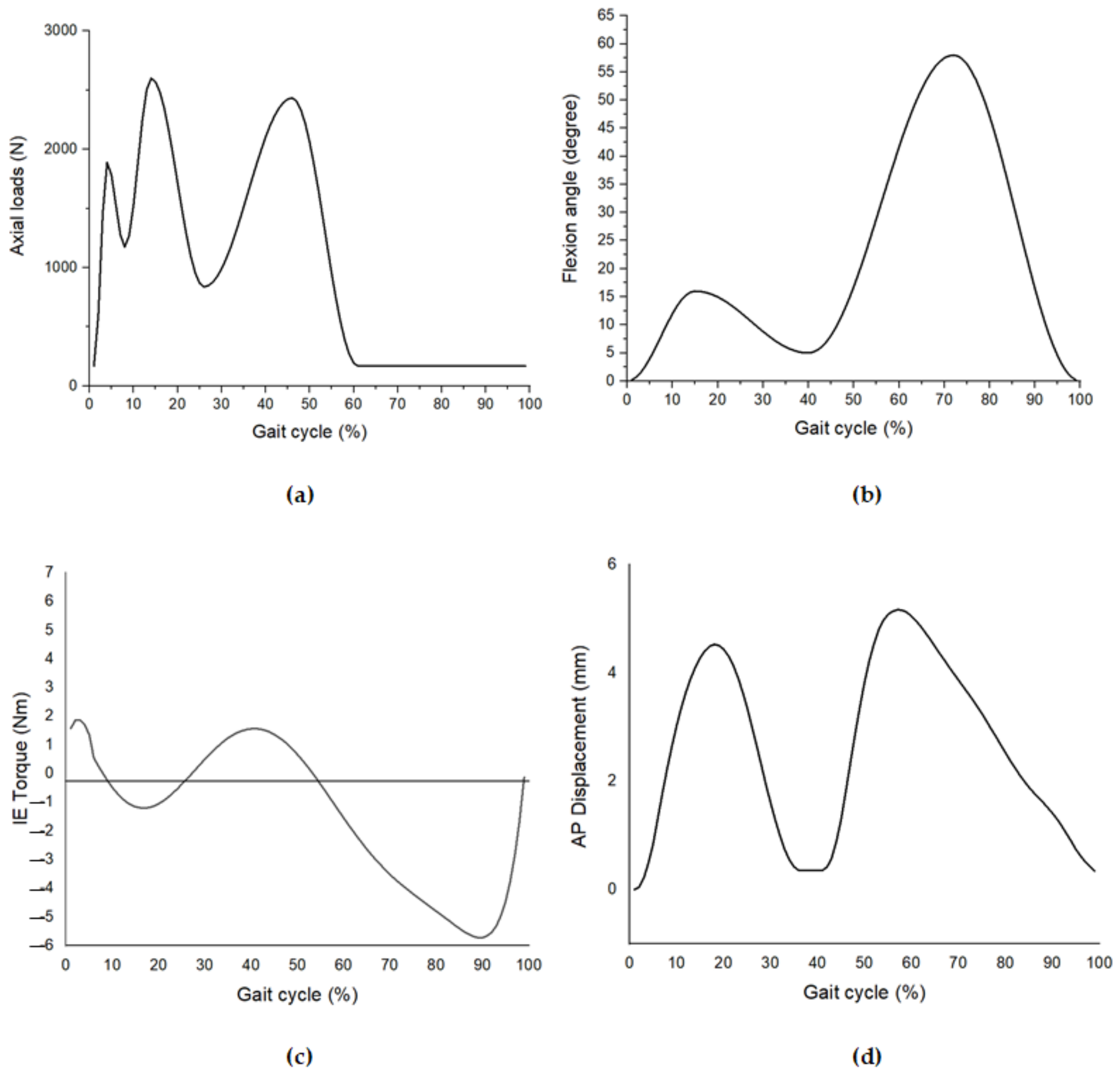
2.1.1. Finite Element (FE) Model
2.1.2. Material Properties and Boundary Conditions
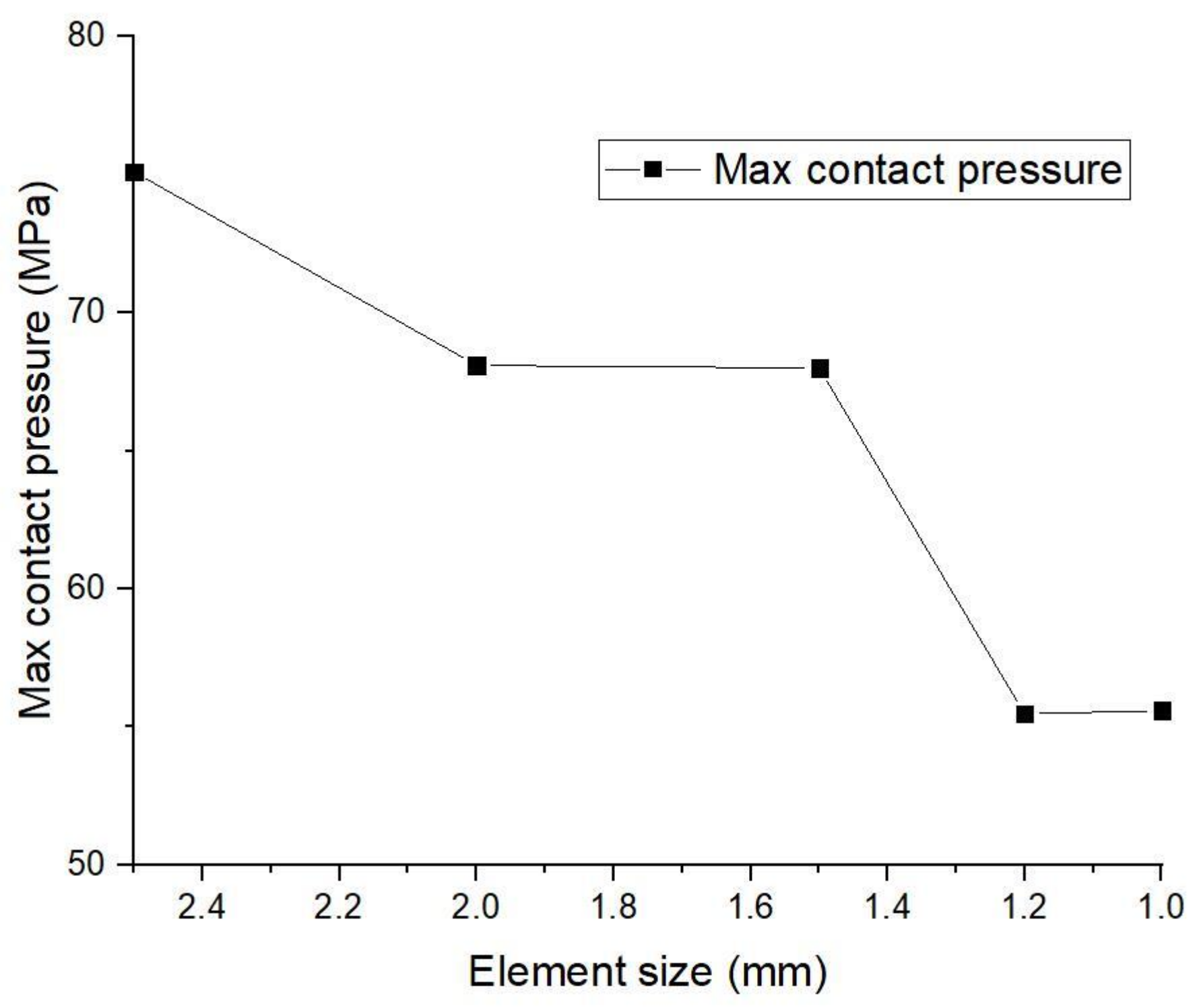
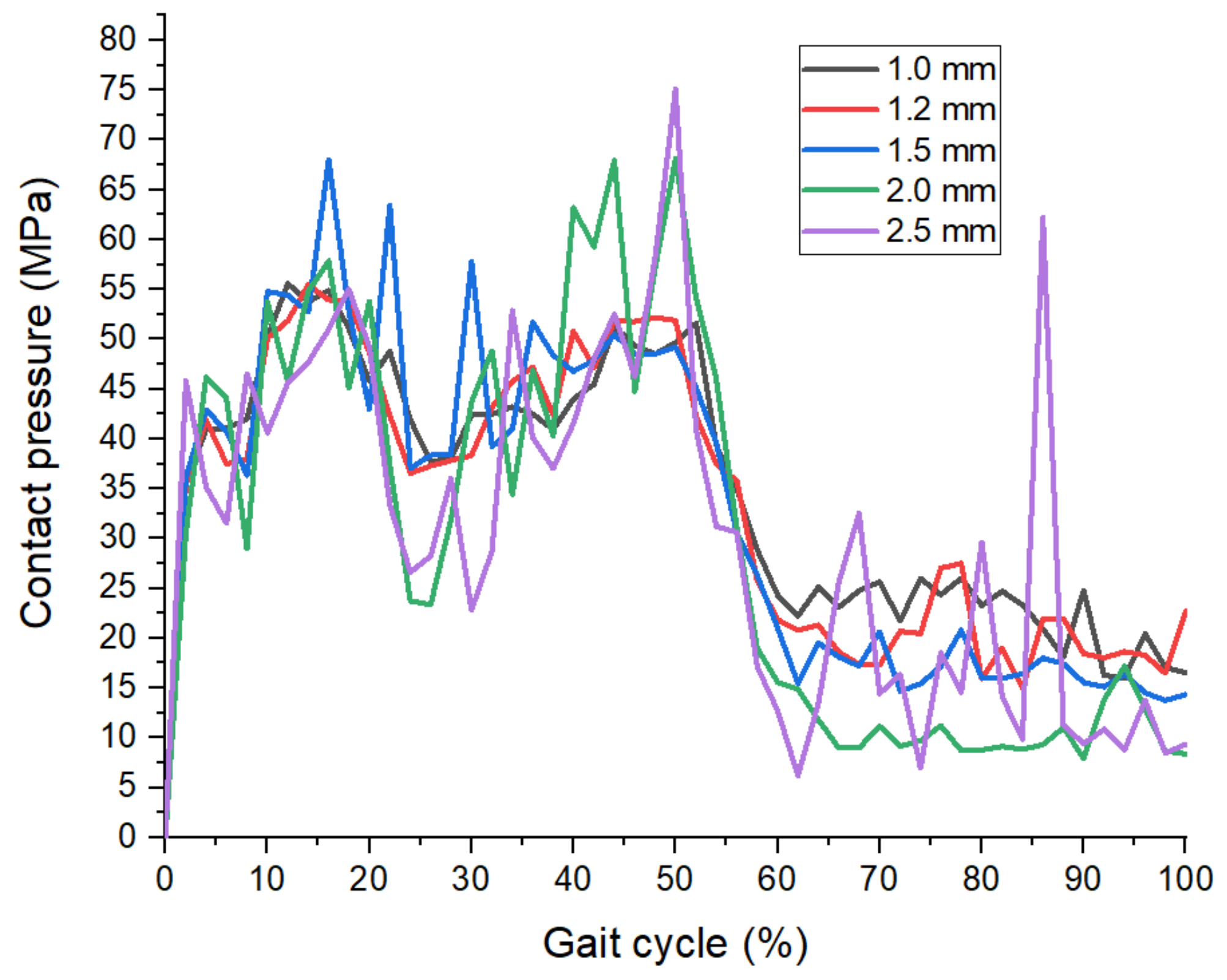
2.1.3. Mesh Convergence Test
2.2. Wear Equation and Computational
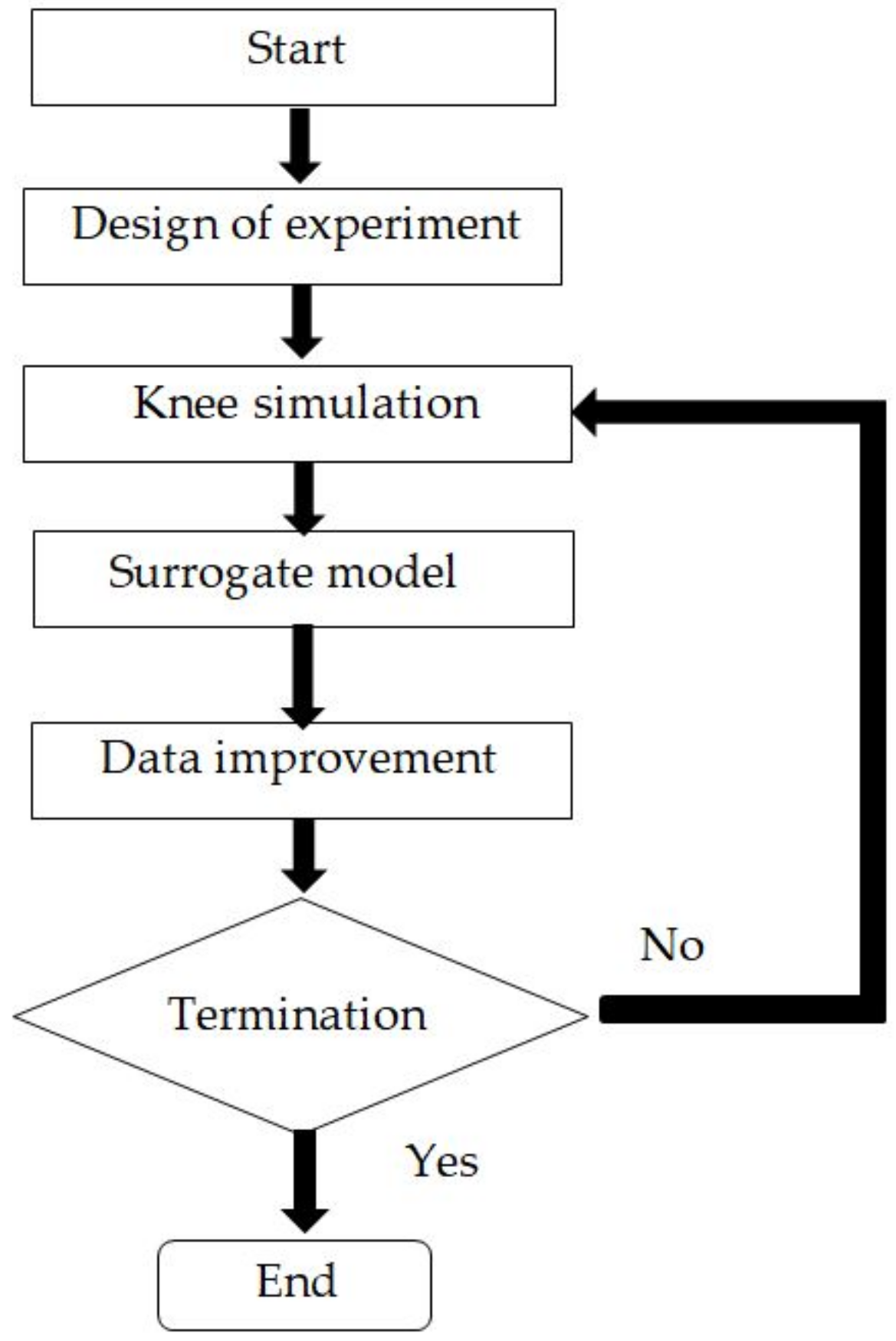
2.3. Design of Experiments (DOE)
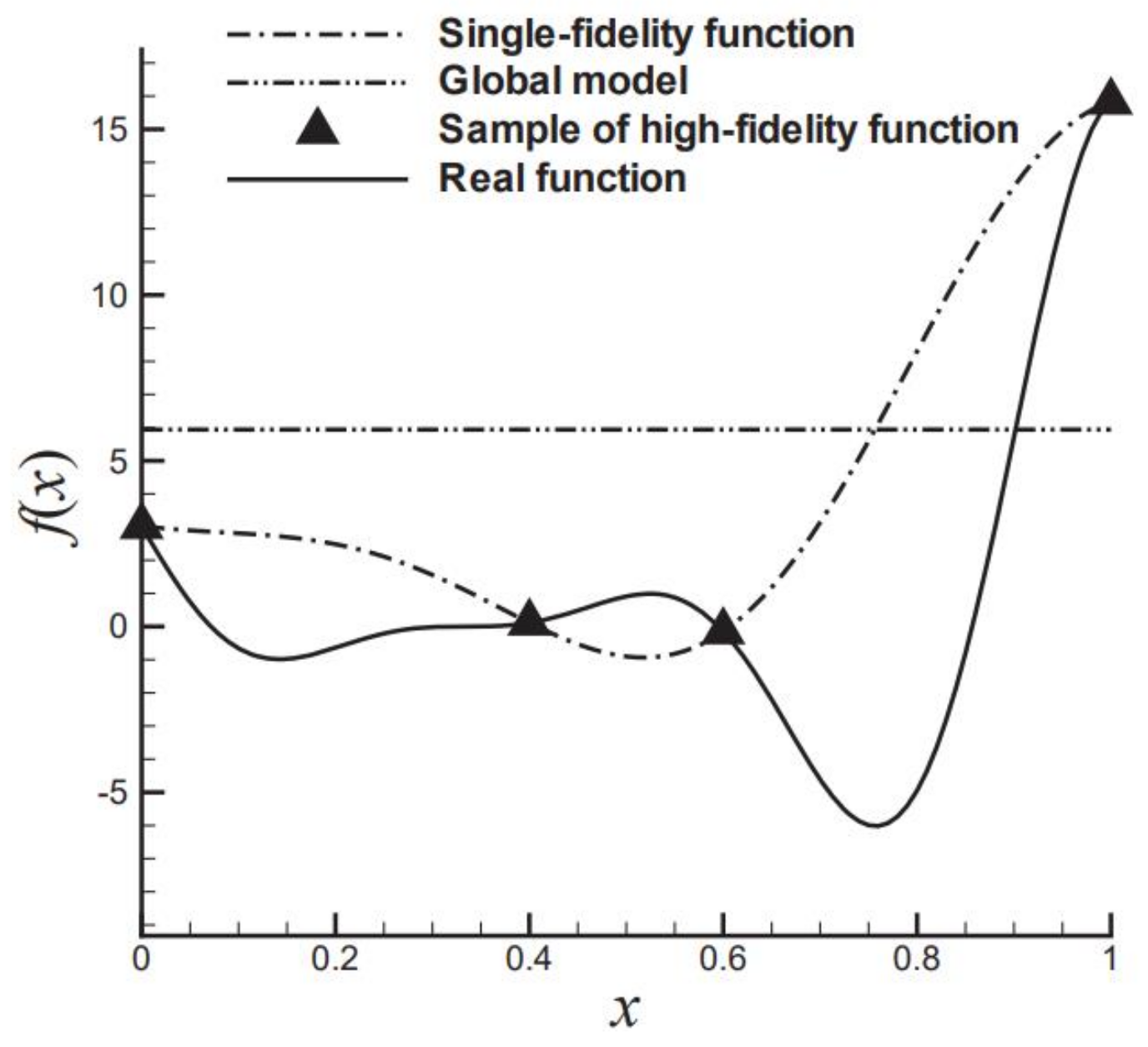
2.4. Surrogate Model Methods
Kriging Method
2.5. Efficient Global Optimization
2.6. Objective Function and Calculation Condition
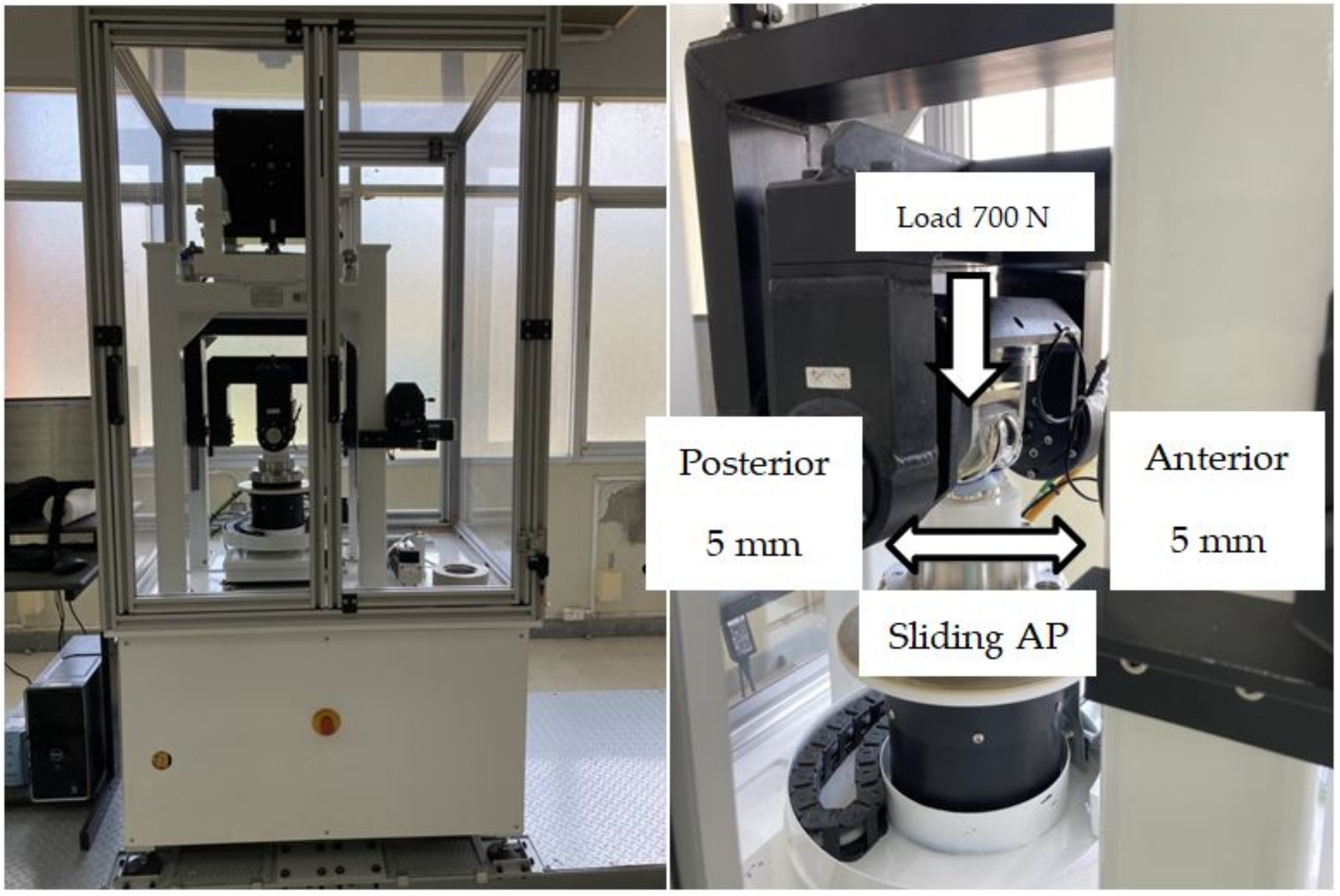
2.7. Experiment Test to Wear Test for Validation with Simulation
3. Results
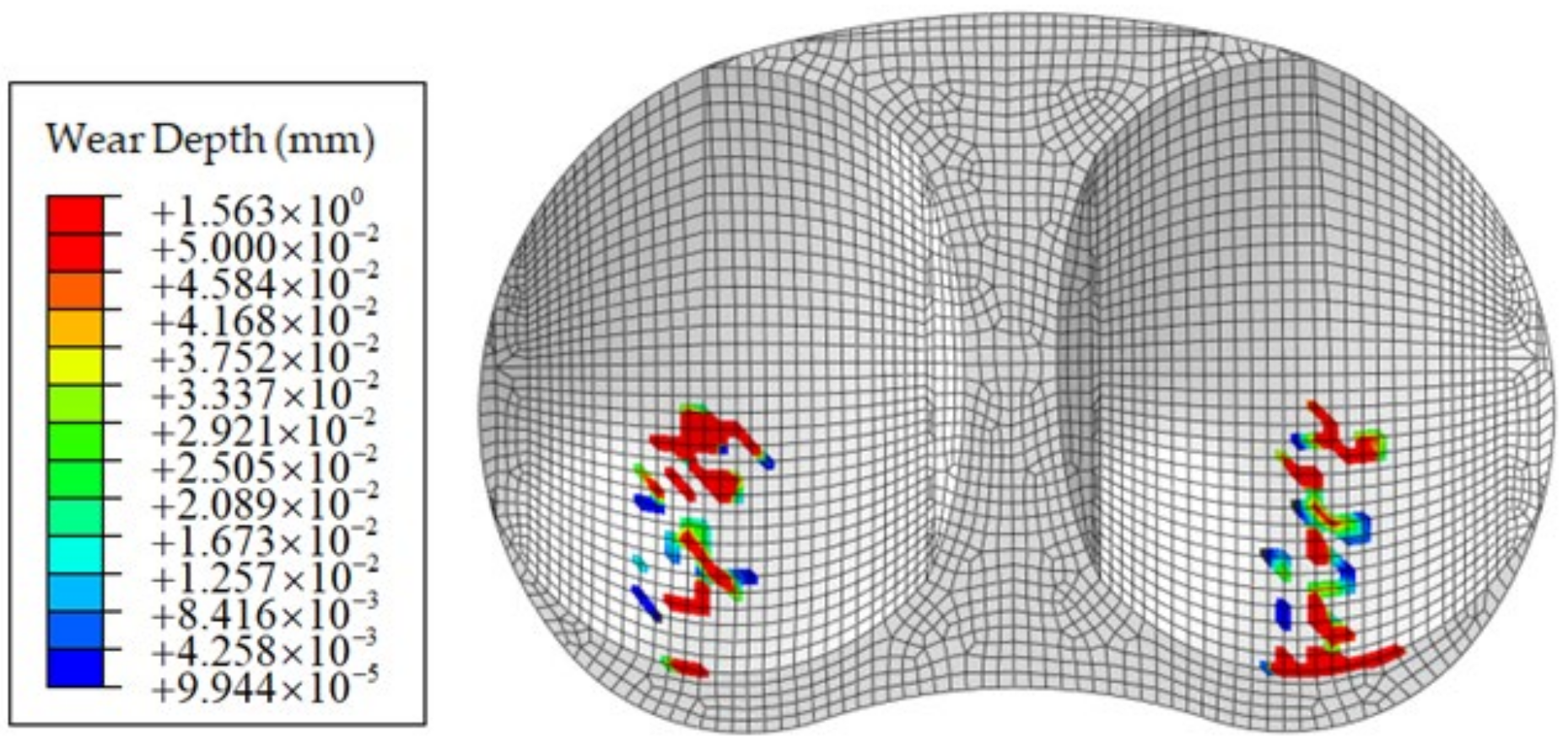
3.1. The Result of Simulations
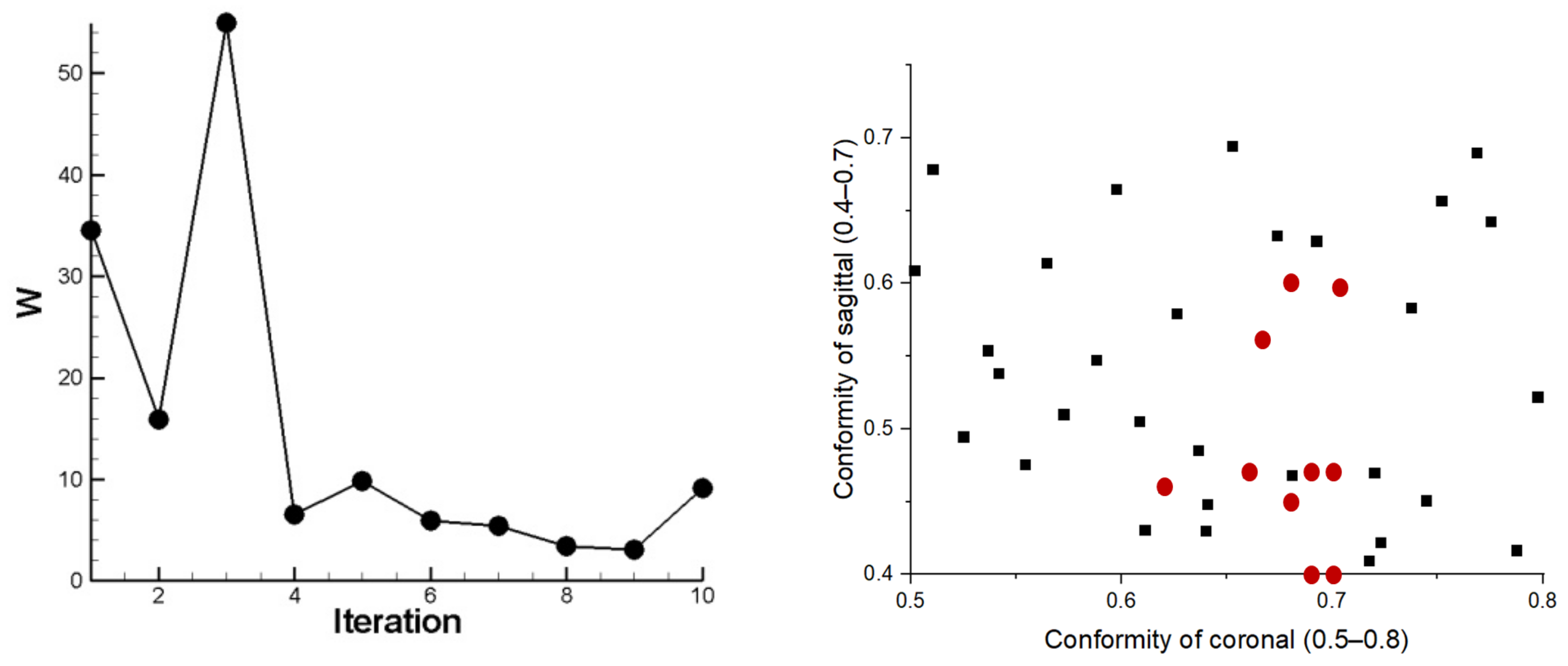
3.2. Minimization Problem of the Wear Volume Optimization
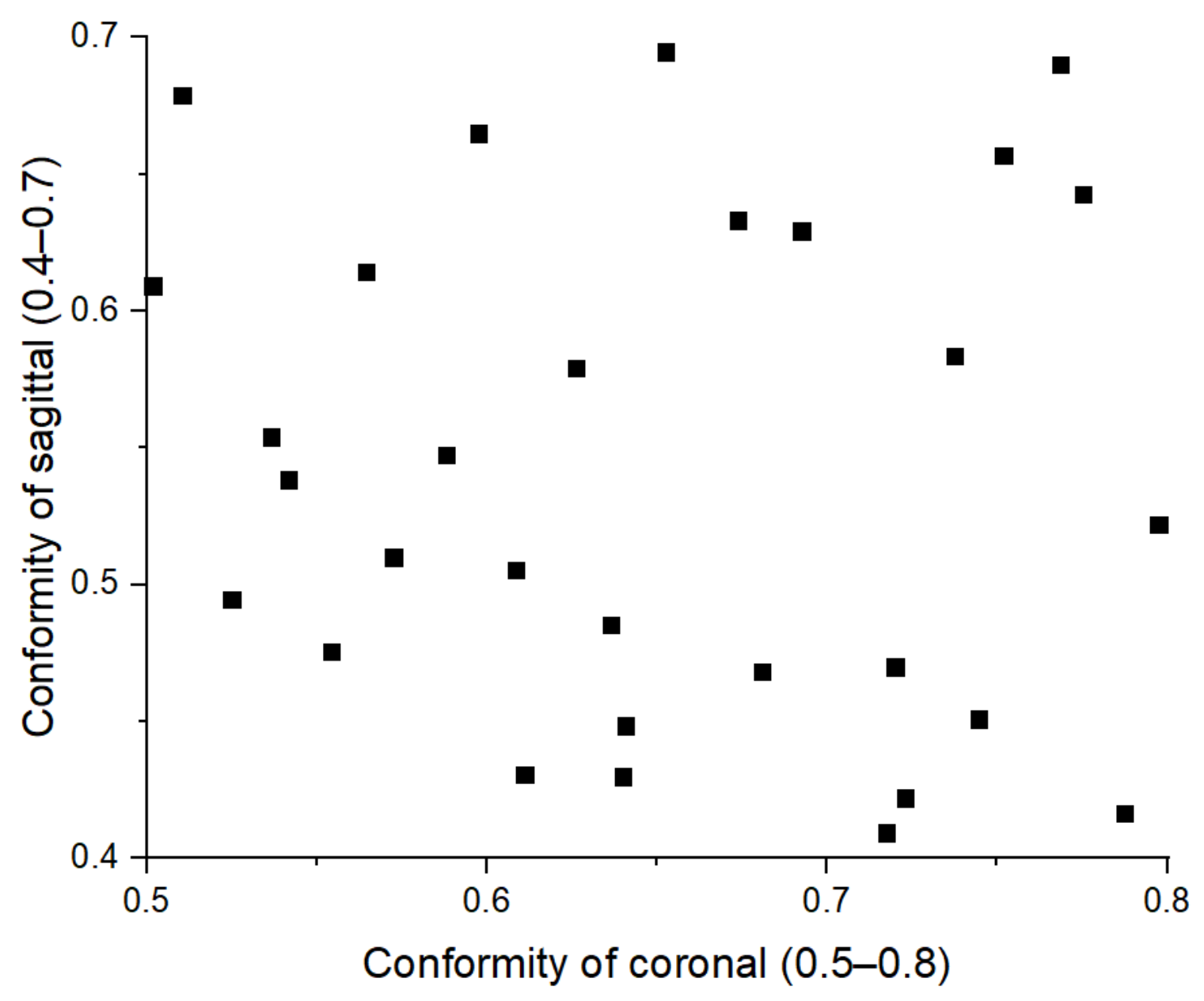
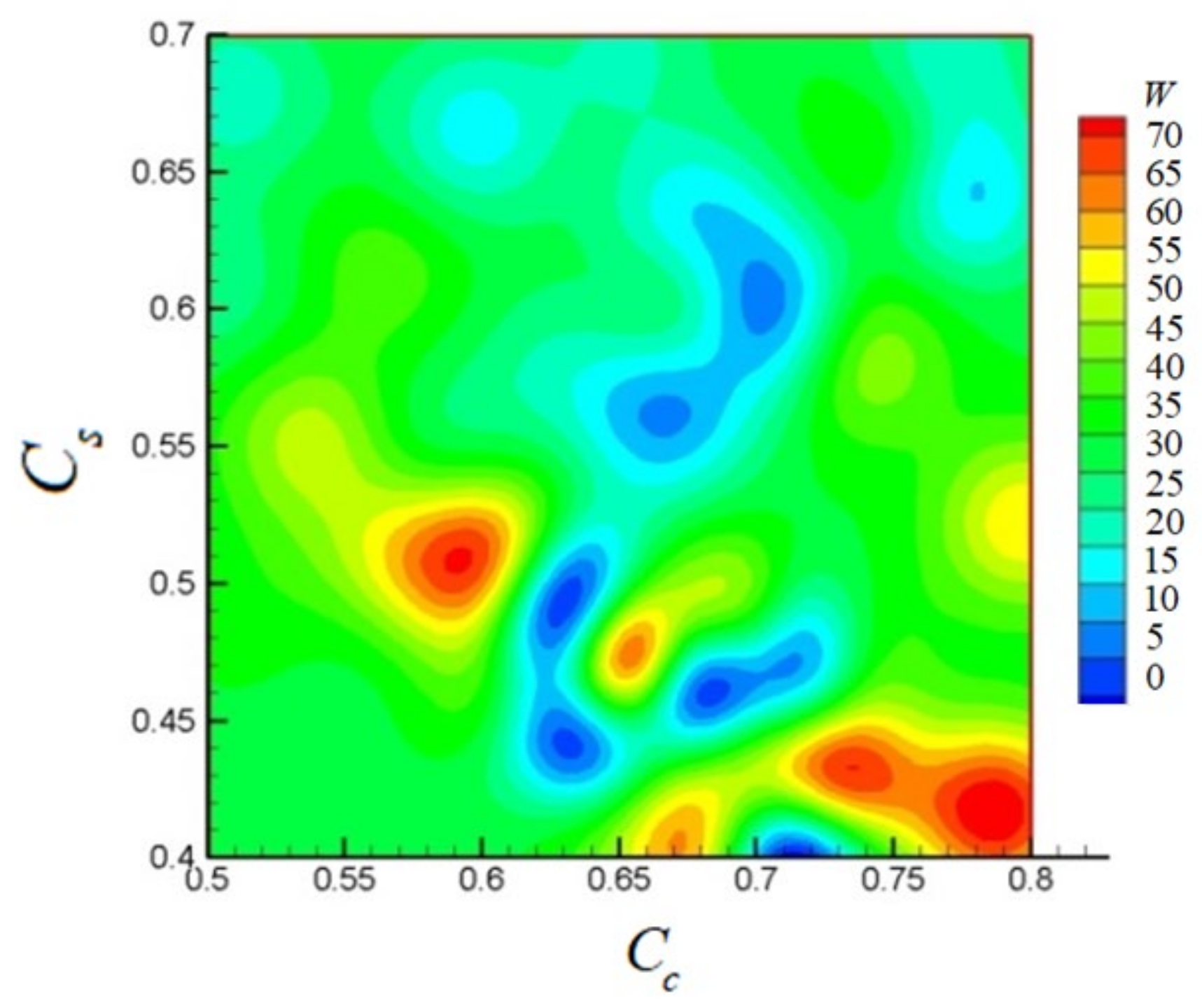
3.3. The Relationship Contour of the Design Tibial Insert to Wear Volume
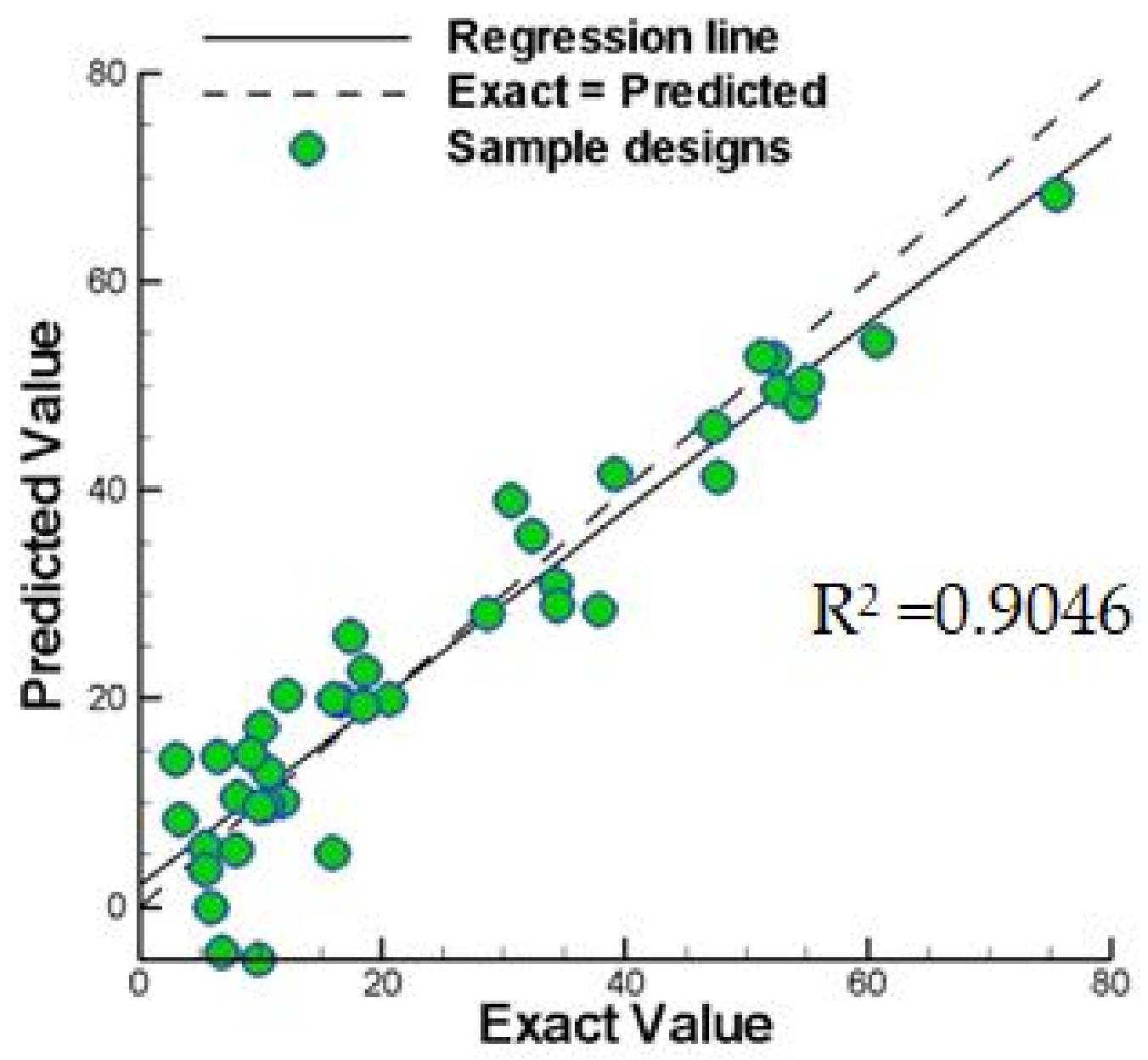
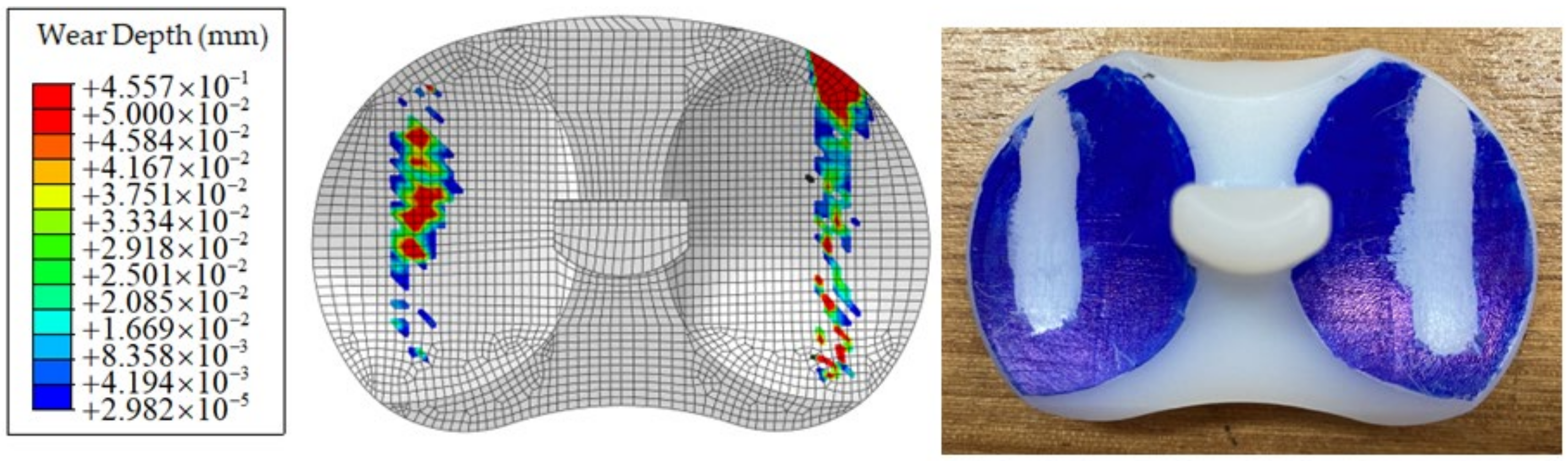
3.4. Result of Validation between Experimental and Simulation
4. Discussion
- 1.
- In consideration of the conformity of the prosthetic knee under the lowest wear volume, this research used the wear co-efficient referenced from the preceding research. It is tested using the ISO-14243 load, where it uses the actual wear coefficient from actual tests. However, this research had studied and found the wear co-efficient from a simplified study to recheck the accuracy of the simulation and the actual test using wear co-efficient trials to achieve the closest value to the actual test.
- 2.
- The prosthetic knee tests for this research used the specific testing machine certified with the ISO-14243. However, the study reduced the perimeters to two conditions: the axial loads and sliding anterior-posterior to recheck the accuracy of the simulation.
- 3.
- The model designed has the conformity value appropriate for production and is used to test, and the accuracy is validated by comparing it to the ISO-14243 standard.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Keeney, J.A.; Eunice, S.; Pashos, G.; Wright, R.W.; Clohisy, J.C. What is the evidence for total knee arthroplasty in young patients? A systematic review of the literature. Clin. Orthop. Relat. Res. 2010, 469, 574–583. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Long, M.; Riester, L.; Hunter, G. Nano-hardness measurements of oxidized Zr-2.5Nb and various orthopaedic materials. Trans. Soc. Biomater. 1998, 21, 528. [Google Scholar]
- Naudie, D.D.; Ammeen, D.J.; Engh, G.A.; Rorabeck, C.H. Wear and osteolysis around total knee arthroplasty. J. Am. Acad. Orthop. Surg. 2007, 64, 15–53. [Google Scholar] [CrossRef] [PubMed]
- Vince, K.G. Why knees fail. J. Arthroplast. 2003, 18, 39–44. [Google Scholar] [CrossRef]
- Laskin, R.S. The Genesis total knee prosthesis: A 10-year followup study. Clin. Orthop. Relat. Res. 2001, 388, 95–102. [Google Scholar] [CrossRef] [PubMed]
- Harsha, A.P.; Joyce, T.J. Comparative wear tests of ultra-high molecular weight polyethylene and cross-linked polyethylene. J. Eng. Med. 2013, 227, 600.e8. [Google Scholar] [CrossRef] [PubMed]
- Collier, J.P.; Currier, B.H.; Kennedy, F.E.; Currier, J.H.; Timmins, G.; Jackson, S.K.; Brewer, R.L. Comparison of cross-linked polyethylene materials for orthopedic applications. Clin. Orthop. Relat. Res. 2003, 414, 289–304. [Google Scholar] [CrossRef] [PubMed]
- Villa, T.; Migliavacca, F.; Gastaldi, D.; Colombo, M.; Pietrabissa, R. Contact stresses and fatigue life in a knee prosthesis: Comparison between in vitro measurements and computational simulations. J. Biomech. 2004, 37, 45–53. [Google Scholar] [CrossRef]
- Abdelgaied, A.; Brockett, C.L.; Liu, F.; Jennings, L.M.; Jin, Z.; Fisher, J. The effect of insert conformity and material on total knee replacement wear. Proc. Inst. Mech. Eng. Med. 2014, 228, 98–106. [Google Scholar] [CrossRef] [Green Version]
- D’Lima, D.D.; Chen, P.C.; Colwell, C.W., Jr. Polyethylene contact stresses, articular congruity, and knee alignment. Clin. Orthop. Relat. Res. 2001, 392, 232–238. [Google Scholar] [CrossRef]
- Luger, E.; Sathasivam, S.; Walker, P.S. Inherent differences in the laxity and stability between the intact knee and total knee replacements. Knee 1997, 4, 7–14. [Google Scholar] [CrossRef]
- Brockett, C.L.; Carbone, S.; Fisher, J.; Jennings, L.M. Influence of conformity on the wear of total knee replacement: An experimental study. J. Eng. Med. 2018, 232, 127–134. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Abdelgaied, A.; Liu, F.; Brockett, C.; Jennings, L.; Fisher, J.; Jin, Z. Computational wear prediction of artificial knee joints based on a new wear law and formulation. J. Biomech. 2011, 44, 1108–1116. [Google Scholar] [CrossRef]
- Osano, K.; Nagamine, R.; Todo, M.; Kawasaki, M. The Effect of Malrotation of Tibial Component of Total Knee Arthroplasty on Tibial Insert during High Flexion Using a Finite Element Analysis. Sci. World J. 2014, 2014, 695028. [Google Scholar] [CrossRef]
- Knight, L.A.; Pal, S.; Coleman, J.C.; Bronson, F.; Haider, H.; Levine, D.L.; Taylor, M.; Rullkoetter, P.J. Comparison of long-term numerical and experimental total knee replacement wear during simulated gait loading. J. Biomech. 2007, 40, 1550–1558. [Google Scholar] [CrossRef] [PubMed]
- Forrester, A.I.J.; Sóbester, A.; Keane, A.J. Engineering Design via Surrogate Modelling A Practical Guide; John Wiley & Sons Ltd.: Chichester, UK, 2008. [Google Scholar]
- Lin, Y.-C.; Haftka, R.T.; Queipo, N.V.; Fregly, B.J. Two-Dimensional Surrogate Contact Modeling for Computationally Efficient Dynamic Simulation of Total Knee Replacements. J. Biomech. Eng. 2009, 131, 041010-1–041010-8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Eskinazi, I.; Fregly, B.J. Surrogate modeling of deformable joint contact using artificial neural networks. Med. Eng. Phys. 2015, 37, 885–891. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lin, Y.-C.; Haftka, R.T.; Queipo, N.V.; Fregly, B.J. Surrogate articular contact models for computationally efficient multibody dynamic simulations. Med Eng. Phys. 2010, 32, 584–594. [Google Scholar] [CrossRef] [PubMed]
- Phombut, C.; Rooppakhun, S. The Design of Total Knee Arthroplasty (TKA) Prosthesis Based on Thai Morphology Data Using Reverse Engineering Technique; Technical Report; Suranaree University of Technology: Nakhon Ratchasima, Thailand, 2021. [Google Scholar]
- Petrović Savić, S.; Adamović, D.; Devedžić, G.; Ristić, B.; Matić, A. Contact Stress Generation on the UHMWPE Tibial Insert. Tribol. Ind. 2014, 36, 354–360. [Google Scholar]
- Koh, Y.-G.; Lee, J.-A.; Kang, K.-T. Prediction of Wear on Tibial Inserts Made of UHMWPE, PEEK, and CFR-PEEK in Total Knee Arthroplasty Using Finite-Element Analysis. Lubricants 2019, 7, 30. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.-H.; Zhang, W.; Song, D.-Y.; Li, H.; Dong, X.; Zhang, M.; Zhao, F.; Jin, Z.-M.; Cheng, C.-K. The impact of variations in input directions according to ISO 14243 on wearing of knee prostheses. PLoS ONE 2018, 13, e0206496. [Google Scholar] [CrossRef] [Green Version]
- Archard, J.F. Contact and Rubbing of Flat Surfaces. J. Appl. Phys. 1953, 24, 981. [Google Scholar] [CrossRef]
- Matheron, G. Principles of Geostatistics. Econ. Geol. 1963, 58, 1246–1266. [Google Scholar] [CrossRef]
- Donald, R.J.; Schonlau, M.; Welch, W.J. Efficient Global Optimization of Expensive BlackBox Functions. J. Glob. Optim. 1998, 13, 455–492. [Google Scholar]
- Afzal, A.; Kim, K.Y.; Seo, J.W. Effects of Latin hypercube sampling on surrogate modeling and optimization. Int. J. Fluid Mach. Syst. 2017, 10, 240–253. [Google Scholar] [CrossRef]
- Bingham, J.S.; Bukowski, B.R.; Wyles, C.C.; Pareek, A.; Berry, D.J.; Abdel, M.P. Rotating-Hinge Revision Total Knee Arthroplasty for Treatment of Severe Arthrofibrosis. J. Arthroplast. 2019, 34, S271–S276. [Google Scholar] [CrossRef]
- Fisher, J.; Jennings, L.M.; Galvin, A.L.; Jin, Z.M.; Stone, M.H.; Ingham, E. Polyethylene Wear in Total Knees. Clin. Orthop. Relat. Res. 2010, 468, 12–18. [Google Scholar] [CrossRef] [Green Version]
- Netter, J.; Hermida, J.; Flores-Hernandez, C.; Steklov, N.; Kester, M.; D’Limar, D.D. Prediction of Wear in Crosslinked Polyethylene Unicompartmental Knee Arthroplasty. Lubricants 2015, 3, 381–393. [Google Scholar] [CrossRef]
- Innocenti, B.; Labey, L.; Kamali, A.; Pascale, W.; Pianigiani, S. Development and Validation of a Wear Model to Predict Polyethylene Wear in a Total Knee Arthroplasty: A finite element analysis. Lubricants 2014, 2, 193–205. [Google Scholar] [CrossRef] [Green Version]
- Koh, Y.-G.; Jung, K.-H.; Hong, H.-T.; Kim, K.-M.; Kang, K.-T. Optimal Design of Patient-Specific Total Knee Arthroplasty for Improvement in Wear Performance. J. Clin. Med. 2019, 8, 2023. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Srinivas, G.R.; Deb, A.; Kumar, M.N. A Study on Polyethylene Stresses in MobileBearing and Fixed-Bearing Total Knee Arthroplasty (TKA) using Explicit Finite Element Analysis. J. Long-Term Eff. Med. Implant. 2013, 23, 275–283. [Google Scholar] [CrossRef]
- Abdelgaied, A.; Brockett, C.L.; Liu, F.; Jennings, L.M.; Fisher, J.; Jin, Z. Quantification of the effect of crossshear and applied nominal contact pressure on the wear of moderately cross-linked polyethylene. J. Eng. Med. 2012, 227, 18–26. [Google Scholar] [CrossRef] [Green Version]
- Galvin, A.; Kang, L.; Tipper, J.; Stone, M.; Ingham, E.; Jin, Z.; Fisher, J. Wear of crosslinked polyethylene under different tribological conditions. J. Mater. Sci. Mater. Med. 2006, 17, 235–243. [Google Scholar] [CrossRef] [PubMed]
- Brockett, C.L.; Jennings, L.M.; Hardaker, C.; FisherWear, J. Wear of moderately cross-linked polyethylene in fixed-bearing total knee replacements. J. Eng. Med. 2012, 226, 529–535. [Google Scholar] [CrossRef]
- Galvin, A.L.; Kang, L.; Udofia, I.; Jennings, L.M.; McEwen, H.M.J.; Jin, Z.; Fisher, J. Effect of conformity and contact stress on wear in fixed-bearing. J. Biomech. 2009, 42, 1898–1902. [Google Scholar] [CrossRef] [PubMed]
- Wasielewski, R.C. The causes of insert backside wear in total knee arthroplasty. Clin. Orthop. Relat. Res. 2002, 404, 232–246. [Google Scholar] [CrossRef] [PubMed]
- Ettinger, M.; Zoch, J.M.; Becher, C.; Hurschler, C.; Stukenborg-Colsman, C.; Claassen, L.; Ostermeier, S.; Calliess, T. In vitro kinematics of fixed versus mobile-bearing in unicondylar knee arthroplasty. Arch. Orthop. Trauma Surg. 2015, 135, 871–877. [Google Scholar] [CrossRef]
- Ardestani, M.M.; Moazen, M.; Jin, Z. Contribution of geometric design parameters to knee implant performance: Conflicting impact of conformity on kinematics and contact mechanics. Knee 2015, 22, 217–224. [Google Scholar] [CrossRef]



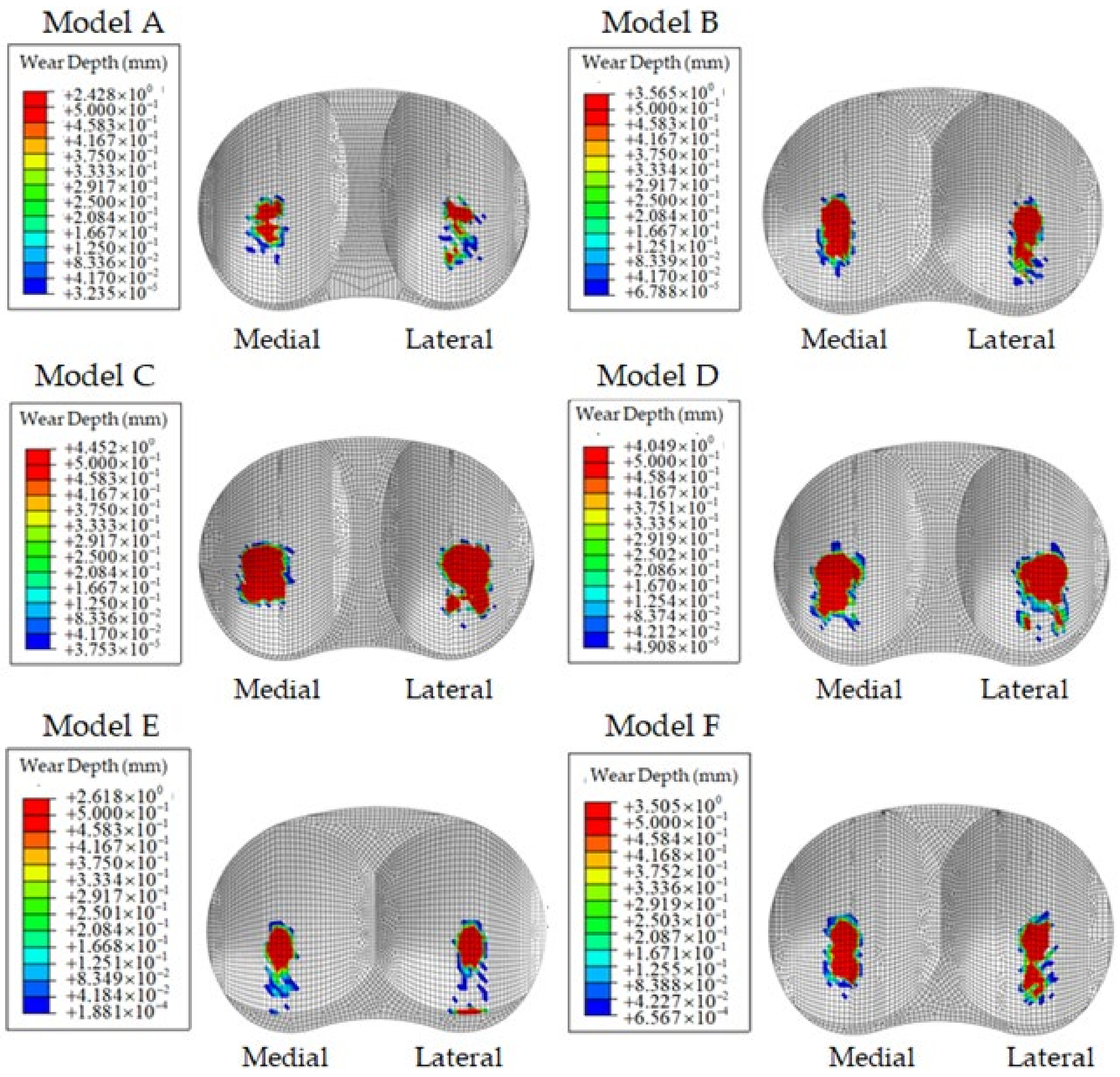

| Model | Conformity of Coronal | Conformity of Sagittal | Wear Volume of Million Cycles |
|---|---|---|---|
| A | 0.71 | 0.41 | 12.13 |
| B | 0.52 | 0.49 | 34.38 |
| C | 0.79 | 0.42 | 75.58 |
| D | 0.79 | 0.52 | 54.51 |
| E | 0.5 | 0.61 | 20.63 |
| F | 0.55 | 0.48 | 32.4 |
| Conformity of Coronal (0.5–0.8) | Conformity of Sagittal (0.4–0.7) | Wear Volume (mm3) in 1 million Cycles |
|---|---|---|
| 0.69 | 0.4 | 34.53 |
| 0.68 | 0.6 | 15.88 |
| 0.66 | 0.47 | 54.98 |
| 0.7 | 0.47 | 6.54 |
| 0.62 | 0.46 | 9.85 |
| 0.69 | 0.47 | 5.93 |
| 0.68 | 0.45 | 5.42 |
| 0.66 | 0.56 | 3.37 |
| 0.7 | 0.59 | 3.07 |
| 0.7 | 0.4 | 9.12 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Takian, W.; Rooppakhun, S.; Ariyarit, A.; Sucharitpwatskul, S. Optimal Conformity Design of Tibial Insert Component Based on ISO Standard Wear Test Using Finite Element Analysis and Surrogate Model. Symmetry 2021, 13, 2377. https://doi.org/10.3390/sym13122377
Takian W, Rooppakhun S, Ariyarit A, Sucharitpwatskul S. Optimal Conformity Design of Tibial Insert Component Based on ISO Standard Wear Test Using Finite Element Analysis and Surrogate Model. Symmetry. 2021; 13(12):2377. https://doi.org/10.3390/sym13122377
Chicago/Turabian StyleTakian, Wisanupong, Supakit Rooppakhun, Atthaphon Ariyarit, and Sedthawatt Sucharitpwatskul. 2021. "Optimal Conformity Design of Tibial Insert Component Based on ISO Standard Wear Test Using Finite Element Analysis and Surrogate Model" Symmetry 13, no. 12: 2377. https://doi.org/10.3390/sym13122377
APA StyleTakian, W., Rooppakhun, S., Ariyarit, A., & Sucharitpwatskul, S. (2021). Optimal Conformity Design of Tibial Insert Component Based on ISO Standard Wear Test Using Finite Element Analysis and Surrogate Model. Symmetry, 13(12), 2377. https://doi.org/10.3390/sym13122377







