Some Results on Various Cancellative CA-Groupoids and Variant CA-Groupoids

Abstract

Cyclic associativity can be regarded as a kind of variation symmetry, and cyclic associative groupoid (CA-groupoid) is a generalization of commutative semigroup. In this paper, the various cancellation properties of CA-groupoids, including cancellation, quasi-cancellation and power cancellation, are studied. The relationships among cancellative CA-groupoids, quasi-cancellative CA-groupoids and power cancellative CA-groupoids are found out. Moreover, the concept of variant CA-groupoid is proposed firstly, some examples are presented. It is shown that the structure of variant CA-groupoid is very interesting, and the construction methods and decomposition theorem of variant CA-groupoids are established.

1. Introduction

An algebraic structure is called a groupoid, if it is well-defined regarding an operation on it. A groupoid satisfying the “cyclic associative law” (that is, x(yz) = y(zx)) is called a cyclic associative groupoid, or simply CA-groupoid [1,2].

In fact, as early as 1946, when Byrne [3] studied axiomatization of Boolean algebra, he mentioned the following operation law: (xy)z = (yz)x. Obviously, its dual form is as follows: z(yx) = x(zy), this is the cyclic associative law mentioned above. In 1954, Sholander [4] mentioned Byrne’s paper [3], and used the term of “cyclic associative law” to express the operation law: (ab)c = (bc)a. This is the first literature we know to use the term “cyclic associative law”. At the same time, Hosszu also used the term of “cyclic associative law” in the study of functional equation (see [5] and the introduction and explanation by Maksa [6]). Later, Kleinfeld [7] and Behn [8,9] studied the rings satisfying the cyclic associative law, and Iqbal et al. [10] studied the AG-groupoids satisfying the cyclic associative law. It is on the basis of these researches that we start to systematically study the groupoids satisfying the cyclic binding law (CA-groupoids) in [1,2], in order to provide a common basis for the research of related algebraic systems.

As a continuation of [1,2], this paper focuses on various cancellation properties of CA- groupoids and a special class of CA-groupoids. In many algebraic systems (such as semigroups, commutative semigroups and AG-groupoids), the cancellation, quasi-cancellation and power cancellation properties have important research value (see [11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26]). In 1957, Takayuki Tamura studied commutative non-potent Archimedean semigroups with cancellative law (see [11]), cancellability is applied to semigroups. Since then, various cancellative laws have been put forward and applied to various algebraic systems, and a series of valuable conclusions have been drawn. The rise of these properties makes an irreplaceable contribution to the development of algebra.

Semigroup with the identity is named monoid, the research of monoid is gradually deepening (see [24,27]). In addition, AG-group is an AG-groupoid with the left identity and inverses (see [28,29,30,31,32]). Through these papers, we know that the identity is a powerful tool for solving algebraic problems. Therefore, we naturally consider CA-groupoids with unit element. However, our study finds that CA-groupoids with unit element degenerate into commutative monoids, and a CA- groupoid with quasi right unit element (i.e., there exists e, if x ≠ e, then xe = x; and ee ≠ e) maybe not a semigroup. Moreover, this kind of CA-groupoids (with quasi right unit element) not only has very interesting properties, but also promotes the study of algebraic structures such as rings and semirings (some examples are presented in Section 5). Therefore, this paper studies it in depth, and we call them variant CA-groupoids.

At last, the content of this paper as follows: in Section 2, we introduce some basic concepts and cancellative properties on semigroup and AG-groupoid; in Section 3, we give the definitions of cancellative CA-groupoids, left cancellative CA-groupoids, right cancellative CA-groupoids and weak cancellative CA-groupoids, and discuss the relationships about them; in Section 4, we give the definitions of several quasi-cancellative CA-groupoids and power cancellative CA-groupoids, and analyze the relationships about several types cancellative CA-groupoids; in Section 5, we propose the new notion of variant CA-groupoid and some interesting examples, moreover, we prove the structure theorem and construction method of variant CA-groupoids.

2. Preliminaries

This paper mainly studies some special types of CA-groupoids. In this section some notions and results on semigroups and CA-groupoids are given. A groupoid (S, *) is a non-empty set S together with a binary operation *. Traditionally, the * operator is omitted without confusion, and (S, *) is abbreviated to S. For a groupoid S, an element a∈S is called to be left cancellative (respectively right cancellative) if for all x, y∈S, ax = ay implies x = y (xa = ya implies x = y); an element is called to be cancellative if it is both left and right cancellative. A groupoid S satisfying the associative law is called a semigroup. A monoid S is a semigroup with an identity element.

Definition 1.

[1] Let S be a groupoid. If for all a, b, c∈S, a(bc) = c(ab), then S is called a cyclic associative groupoid (or shortly CA-groupoid).

Proposition 1.

[1] If S is a CA-groupoid, then, for any a, b, c, d, x, y∈S:

(1) (ab)(cd) = (da)(cb);

(2) (ab)((cd)(xy)) = (da)((cb)(xy)).

Proposition 2.

[1] Every commutative semigroup is a CA-groupoid. Assume that (S, ·) is a CA-groupoid, if S is commutative, then S is a commutative semigroup.

Proposition 3.

[1] Let S be a CA-groupoid. (1) If S have a left identity element, that is, there exists e∈S such that ea = a for all a∈S, then S is a commutative semigroup (thus, S is a commutative monoid). (2) If e∈S is a left identity element in S, then e is an identity element in S. (3) If e∈S is a right identity element in S, that is, ae = a for all a∈S, then e is an identity element in S. (4) If S have a right identity element, then S is a commutative semigroup (thus, S is a commutative monoid).

Proposition 4.

[1] Let S be a CA-groupoid. If for all a∈S, a² = a, then S is commutative (thus, S is a commutative semigroup).

Proposition 5.

[1] Let S₁, S₂ be two CA-groupoids. Then the direct product S₁× S₂ is a CA-groupoid.

Definition 2.

[2] An element a of a CA-groupoid S is called locally associative if satisfied:

a(aa) = (aa)a.

S is called a locally associative CA-groupoid, if all elements in S are locally associative.

Definition 3.

[2] Let S be a groupoid. If for all a, b, c∈S:

a(bc) = (ab)c, a(bc) = c(ab),

then S is called a cyclic associative semigroup (shortly, CA-semigroup).

Definition 4.

[18] Let S be a semigroup. S is called a separative semigroup, if for any x, y$\in$S:

(i) x² = xy and y² = yx imply x = y;

(ii) x² = yx and y² = xy imply x = y.

A semigroup S is called quasi-separative if for alla, b$\in$S, x² = xy = y² imply x = y.

3. Cancellation Properties of CA-Groupoids

Definition 5.

Assume that S is a CA-groupoid. If every element of S is left cancellative (right cancellative, cancellative), then S is called a left cancellative (right cancellative, cancellative) CA-groupoid.

Example 1.

Let S = {$\overline{0}$,$\overline{1}$,$\overline{2}$,$\overline{3}$,$\overline{4}$}.For all x, y$\in$S, the operation* on S is defined asx*y =$\overline{x + y}$= x + y (mod 5), see

Table 1. The operation * on S.

*	$\overline{0}$	$\overline{1}$	$\overline{2}$	$\overline{3}$	$\overline{4}$
$\overline{\mathbf{0}}$	$\overline{0}$	$\overline{1}$	$\overline{2}$	$\overline{3}$	$\overline{4}$
$\overline{\mathbf{1}}$	$\overline{1}$	$\overline{2}$	$\overline{3}$	$\overline{4}$	$\overline{0}$
$\overline{\mathbf{2}}$	$\overline{2}$	$\overline{3}$	$\overline{4}$	$\overline{0}$	$\overline{1}$
$\overline{\mathbf{3}}$	$\overline{3}$	$\overline{4}$	$\overline{0}$	$\overline{1}$	$\overline{2}$
$\overline{\mathbf{4}}$	$\overline{4}$	$\overline{0}$	$\overline{1}$	$\overline{2}$	$\overline{3}$

. Then, (S, *) is a cancellative CA-groupoid.

Definition 6.

Assume that S is a CA-groupoid. Let x$\in$S, if for any y, z$\in$S, xy = xz and yx = zx imply y = z, then x iscalled to be weak cancellative.If all elements in S are weak cancellative, then S iscalled a weak cancellative CA-groupoid.

Obviously, for a CA-groupoid S and any x∈S, if x is a left (or right) cancellative, then x is weak cancellative.

Example 2.

Let S = {1, 2, 3, 4}. The operation * on S is defined as

Table 2. The operation * on S.

*	1	2	3	4
1	4	3	2	1
2	3	1	4	2
3	2	4	1	3
4	1	2	3	4

. Then, (S, *) is a weak cancellative CA-groupoid.

Theorem 1.

Let S be a CA-groupoid. Then, for any element a$, b\in$S:

(1) 

if a is left cancellative, then a is right cancellative, thus a is cancellative;

(2) 

if a and b are left cancellative, then ab is right cancellative;

(3) 

if a is right cancellative and b is left cancellative, then ab is right cancellative;

(4) 

if ab is right cancellative, then ab = ba;

(5) 

if ab is cancellative, then b is cancellative;

(6) 

if ab is cancellative, then a and b are cancellative;

(7) 

if a and ab are right cancellative, and b is left cancellative, then a is cancellative;

(8) 

if a and ab are right cancellative, and b is left cancellative, then ab is cancellative.

Proof. 

Suppose that (S, *) is a CA-groupoid and a, b∈S.

(1) Assume that a is a left cancellative element. If (∀x, y∈S) x*a = y*a, then (by cyclic association):

a*(a*x) = x*(a*a) = a*(x*a) = a*(y*a) = a*(a*y).

From this, applying left cancellation property of a, a*x = a*y. From this, applying left cancellation property of a one time, we get that x = y. Therefore, a is a right cancellative element in S, so a is a cancellative element in S.

(2) Suppose that a and b are left cancellative. If (∀x, y∈S) x*ab = y*ab, then:

a*(b*x) = x*(a*b) = x*(ab) = y*(ab) = y*(a*b) = b*(y*a) = a*(b*y).

Since a is left cancellative, so b*x = b*y. Moreover, from this and b is left cancellative, we get that x = y. Therefore, ab is a right cancellative.

(3) Assume that a is right cancellative and b is left cancellative. If (∀x, y∈S) x*ab = y*ab, then:

b*(x*a) = a*(b*x) = x*(a*b) = x*(ab) = y*(ab) = y*(a*b) = b*(y*a).

Since b is left cancellative, so x*a = y*a. Moreover, from this and a is right cancellative, we get that x = y. Therefore, ab is a right cancellative.

(4) Suppose that ab is right cancellative. Since:

ab*ab = b*(ab*a) = a*(b*ab) = a*(b*ba) = ba*ab

Since ab is right cancellative, we get that ab = ba.

(5) Assume that ab is cancellative. If b*x = b*y, x, y∈S, then:

x*ab = b*(x*a) = a*(b*x) = a*(b*y) = y*ab

Since ab is cancellative, so x = y. This means that b is left cancellative. Applying (1), we get that b is cancellative.

(6) Assume that ab is cancellative. Using (5), we know that b is cancellative. Moreover, since ab is cancellative, so ab is right cancellative, applying (4) we get that ba = ab. Thus, ba is cancellative, using (5) again, a is cancellative.

(7) Suppose that a and ab are right cancellative, and b is left cancellative. If a*x = a*y, x, y∈S, then (applying Proposition 1 (1)):

b*(xa*ab) = b*(bx*aa) = b*(ab*ax) = b*(ab*ay) = b*(ya*ab).

Since b is left cancellative, so xa*ab = ya*ab. Using the condition that ab is right cancellative, it follows that xa = ya. Since a is right cancellative, thus, x = y. Hence, a is left cancellative. Therefore, a is cancellative

(8) Suppose that a and ab are right cancellative, and b is left cancellative. If ab*x = ab*y, x, y∈S, then:

b*(xa*ab) = ab*(b*xa) = ab*(a*bx) = ab*(x*ab) = ab*(ab*x) = ab*(ab*y) =

ab*(y*ab) = ab*(a*by) = ab*(b*ya) = b*(ya*ab).

Since b is left cancellative, so xa*ab = ya*ab. Using the condition that ab is right cancellative, it follows that xa = ya. Since a is right cancellative, thus, x = y. This means that ab is left cancellative. From this and ab is right cancellative, we know that ab is cancellative. □

Applying Theorem 1 we can get the following corollaries.

Corollary 1.

Let S be a CA-groupoid. Then the following asserts are equivalent:

(1) 

S is a left cancellative CA-groupoid;

(2) 

S is a right cancellative CA-groupoid;

(3) 

S is a cancellative and commutative semigroup;

(4) 

S is a cancellative CA-groupoid.

Proof. 

(1) ⇒ (2): It follows Theorem 1 (1).

(2) ⇒ (3): For any a, b∈S, then ab∈S. Since S is right cancellative, then ab is right cancellative. Applying Theorem 1 (4), ab = ba. This means that S is commutative. By Proposition 2, we know that S is a commutative semigroup. Moreover, since S is right cancellative, so S is left cancellative. Thus, S is a cancellative and commutative semigroup.

(3) ⇒ (4): Obviously.

(4) ⇒ (1): It follows from Definition 5. □

Corollary 2.

Let S be a CA-groupoid. If there exists a cancellative element in S, then the set H = {a∈S: ais cancellative} is a sub CA-groupoid of S.

Proof. 

By the condition that there exists a cancellative element in S, we know that H is not empty.

For any a, b∈H, then a and b are left and right cancellative. Applying Theorem 1 (2), we know that ab is right cancellative. By Theorem 1 (8), ab is cancellative. Thus ab∈H. It follows that H is a sub CA-groupoid of S. □

Corollary 3.

Let S be a CA-groupoid. If there exists a non-cancellative element in S, then the set K = {a∈S: a is non-cancellative} is a sub CA-groupoid of S.

Proof. 

Obviously, K is non-empty. For any a, b∈K, then a and b are non-cancellative. By Theorem 1 (5), we know that ab is non-cancellative. Thus ab∈K. It follows that K is a sub CA-groupoid of S. □

The following example shows that a weak cancellative element maybe not a left (or right) cancellative element.

Example 3.

Let S = {1, 2, 3, 4, 5}, and the operation * on S is defined as

Table 3. The operation * on S.

*	1	2	3	4	5
1	1	1	1	1	1
2	1	1	2	1	2
3	1	1	4	2	2
4	1	1	2	1	2
5	1	1	1	1	1

, then S is a CA-groupoid. It is easy to verify that 3 is weak cancellative, but 3 is not left (right) cancellative.

Open Problem 1 (to prove or give a counterexample): Is any weak cancellative CA-groupoid necessarily cancellative?

Theorem 2.

Let S be a CA-groupoid and a, b, c∈S. Define on S the relation ~ as:

$$a~b\iff a \mathit{and} b \mathit{are} \mathit{both} \mathit{cancellative} \mathit{or} \mathit{non}-\mathit{cancellative}.$$

Then ~ is an equivalence relation.

Proof. 

Suppose that a is a cancellative element (or non-cancellative element) of CA-groupoid S. Then a~a. This means that ~ is reflexive.

Suppose a~b. If a and b are cancellative, then b~a; if a and b are non-cancellative, then b~a. Thus ~ is symmetric.

Next, suppose that a~b and b~c. If a and b are cancellative, from b~c we know that c is cancellative, thus a and c are cancellative, i.e., a~c; if a and b are non-cancellative, from b~c we know that c is non-cancellative, thus a and c are non-cancellative, i.e., a~c. Thus ~ is transitive.

Therefore, ~ is an equivalence relation. □

Example 4.

Let S = {1, 2, 3, 4} and the operation * on S is defined as

Table 4. The operation * on S.

*	1	2	3	4
1	1	2	4	3
2	2	1	3	4
3	3	4	4	3
4	4	3	3	4

, then S is a CA-groupoid. Obviously, 1 and 2 are cancellative, 3 and 4 are non-cancellative. H = {1, 2} is a sub CA-groupoid of S.

Theorem 3.

Let S₁, S₂ are CA-groupoids, then the direct product S₁×S₂ of S₁ and S₂ is a CA-groupoid. If a$\in$S₁, b$\in$S₂, a and b are cancellative, then(a, b)$\in$S₁ × S₂ is cancellative.

Proof. 

Suppose that S₁ and S₂ are CA-groupoids. By Proposition 5, S₁ × S₂ is a CA-groupoid. Let a$\in$S₁, b$\in$S₂, a and b be cancellative. For any$\left(x_1,x_2\right),\left(y_1,y_2\right)\in S_1\times S_2$, if $\left(a,b\right)\ast \left(x_1,x_2\right) = \left(a,b\right)\ast \left(y_1,y_2\right)$, then:

(ax₁, bx₂) = (ay₁, by₂)

ax₁ = ay₁, bx₂ = by₂

x₁ = y₁, x₂ = y₂. (since a and b are cancellative)

(x₁, x₂) = (y₁, y₂).

hence, (a, b) is cancellative. □

4. Separability and Quasi-Cancellability of CA-Groupoids

Definition 7.

Let S be a CA-groupoid. (1) S is called to be left (right) separative, for all x, y$\in$S, if x² = xy and y² = yx (x² = yx and y² = xy) imply x = y. (2) S is called to be separative, if it is both left and right separative. (3) S is called to be quasi-separative, if for all x, y$\in$S, x² = xy = y² implies x = y.

Example 5.

Let S = {1, 2, 3, 4}. The operation * on S is defined as

Table 5. The operation * on S.

*	1	2	3	4
1	4	2	1	1
2	2	2	2	2
3	1	2	3	4
4	1	2	4	4

. Then (S, *) is a separative CA-groupoid.

Example 6.

Let S = {1, 2, 3, 4}. The operation * on S is defined as

Table 6. The operation * on S.

*	1	2	3	4
1	4	3	2	1
2	3	2	3	2
3	2	3	2	3
4	1	2	3	4

. Then (S, *) is a quasi-separative CA-groupoid.

Theorem 4.

Let S be a CA-groupoid. Then the following asserts are equivalent:

(1) 

S is separative;

(2) 

S is left separative;

(3) 

S is right separative;

(4) 

S is quasi-separative.

Proof. 

Obviously, (1)$\Rightarrow$(2), by Definition 7.

(2)$\Rightarrow$(3): Suppose that S is left separative. For any x, y$\in$S, if x² = yx and y² = xy, then (by Proposition 1 (1)):

(xy)² = (xy)(xy) = (xy) y² = (xy)(yy) = (yx)(yy) = x²(yy) = (xx)(yy) = (yx)(yx) = (xy)(yx);

(yx)² = (yx)(yx) = (xy)(yx) = (xx)(yy) = x²y² = (yx)(xy).

Since S is left separative, by Definition 7 we have xy = yx. From this, using x² = yx and y² = xy, we get that x² = xy and y² = yx. Applying the condition that S is left separative, by Definition 7 again, we have x = y. This means that S is right separative.

(3)$\Rightarrow$(4): Suppose that S is right separative. For any x, y$\in$S, if x² = xy = y², then (by Proposition 1 (1)):

(xy) ² = (xy)(xy) = x² (xy) = (xx)(xy) = (yx)(xx) = (yx)x² = (yx)(xy);

(yx) ² = (yx)(yx) = (xy)(yx).

Since S is right separative, by Definition 7 we have xy = yx. From this, using x² = xy = y², we get that x² = yx and y² = xy. Applying the condition that S is right separative, by Definition 7 again, we have x = y. This means that S is quasi-separative.

(4)$\Rightarrow$(1) Suppose that S is quasi-separative. For any x, y$\in$S, then (by Proposition 1 (1)):

(xy)² = (xy)(xy) = (yx)(xy) = (yy)(xx) = y²x²;

(yx)² = (yx)(yx) = (xy)(yx) = (xx)(yy) = x²y².

Moreover,

[(yx)(xy)]² = [(yx)(xy)] [(yx)(xy)] = [(yx)(xy)] [(yy)(xx)] = [(yx)(xy)] (y²x²) = [(yx)(xy)] (xy)² =

[(yx)(xy)] [(xy)(xy)] = [(xy)(yx)] [(xy)(xy)] = [(xx)(yy)] [(xy)(xy)] = (x²y²) [(xy)(xy)] =

(yx)² [(xy)(xy)] = [(yx)(yx)] [(xy)(xy)] = [(xy)(yx)] [(xy)(yx)] = [(xy)(yx)]².

If x² = xy and y² = yx, then

(y⁴)² = (y²y²)² = [(yx)(yx)]²= [(xy)(yx)]²= [(yx)(xy)]²= (y²x²)²= (y²x²)(y²x²) = (x²y²)(y²x²) = (x²x²)(y²y²) =x⁴y⁴;

(x⁴)² = (x²x²)² = [(xy)(xy)]²= [(yx)(xy)]²= [(yy)(xx)]²= (y²x²)²= (y²x²)(y²x²) = (x²y²)(y²x²) = (x²x²)(y²y²) =x⁴y⁴.

From this, applying the condition that S is quasi-separative, we get that x⁴=y⁴. Thus,

(xy)² = (x²)² = x⁴ = y⁴ = (y²)² = (yx)² = (yx)(yx) = (xy)(yx).

That is, (xy)² = (xy)(yx) = (yx)². Since S is quasi-separative, by Definition 7 we have xy = yx. From this, using x² = xy and y² = yx, we have x² = xy = y². Applying the condition that S is quasi-separative, by Definition 7 again, we have x = y. This means that S is left separative. □

Similarly, we can prove that S is right separative. Therefore, S is separative by Definition 7.

Proposition 6.

Let S be a CA-groupoid. If S is cancellative, then S is separative.

Proof. 

Assume that S is cancellative. For any x, y$\in$S, if x² = xy = y², then xx = xy and xy = yy. Using cancellability of S, we have x = y. This means that S is separative.

Similarly, we can prove that S is separative when S is left (or right) cancellative. □

The following example shows that a separative CA-groupoid maybe not a left (or right) cancellative CA-groupoid.

Example 7.

Let S = {1,2,3,4}. The operation * on S is defined as

Table 7. The operation * on S.

*	1	2	3	4
1	1	2	3	1
2	1	4	3	2
3	3	3	3	3
4	1	2	3	4

. Then (S, *) is a separative CA- groupoid, but S isn’t cancellative, since 1*1 = 2*1, 1$\ne$2.

Definition 8.

Let S be a CA-groupoid. S is called a CA-band, if for all a$\in$S, aa = a; S is called CA-3-band, if for all a$\in$S, a*aa = aa*a = a.

Definition 9.

Let S be a CA-groupoid. S is called to be left(right) quasi-cancellative, for all x, y$\in$S, if x = xy and y² = yx (x = yx and y² = xy) imply x = y. S is called quasi-cancellative, if it is both left and right quasi- cancellative.

Example 8.

Let S = {1,2,3,4}. The operation * on S is defined as

Table 8. The operation * on S.

*	1	2	3	4
1	1	1	1	1
2	1	2	1	1
3	1	1	3	4
4	1	1	4	3

. Then (S, *) is a quasi-cancellative CA-groupoid.

Theorem 5.

Let S be a CA-groupoid. If S is left quasi-cancellative, then S is right quasi-cancellative.

Proof. 

Suppose that S is left quasi-cancellative. For any x, y$\in$S, if x = yx and y² = xy, then (by Proposition 1 (1)):

x² = (yx)(yx) = (xy)(yx) = (xx)(yy) = x²y²;

(y²)² = y²y² = (xy)(xy) = (yx)(xy) = (yy)(xx) = y²x².

From this, applying the condition that S is left quasi-cancellative, we get that x² = y². Thus:

xy = y² = x² = (yx)(yx) = (xy)(yx);

(yx)² = (yx)(yx) = x(yx) = x(xy) = (yx)(xy).

From this, applying the condition that S is left quasi-cancellative and Definition 9 again, we get that xy = yx. Hence, using the condition that x = yx and y² = xy, we have x = xy and y² = yx, applying the definition of left quasi-cancellative, we get that x = y. Therefore, S is right quasi-cancellative. □

Open Problem 2 (to prove or give a counterexample): Is any right quasi-cancellative CA-groupoid necessarily left quasi-cancellative?

Theorem 6.

The following asserts are true:

(1)

Every CA-band is quasi-cancellative.

(2)

Every CA-3-band is quasi-cancellative.

(3)

Everyquasi-separativeCA-groupoid is quasi-cancellative;

(4)

Every separative(or left-, right-separative) CA-groupoid is quasi-cancellative.

Proof. 

(1) Let S be a CA-band. For any x, y$\in$S, if x = xy and y² = yx, then (by Definition 8) x = x², y = y². It follows that:

x = x² = (xy)(xy) = (yx)(xy) = y²(xy) = y(xy) = yx = y² = y.

This means that S is left quasi-cancellative. Applying Theorem 5, we know that S is right quasi- cancellative. Hence, S is quasi-cancellative.

(2) Let S be a CA-3-band. For any x, y$\in$S, if x = xy and y² = yx, then (by Definition 8) x = xx² = x²x, y = yy² = y²y. Furthermore:

y² = yx = y(xy) = y(yx) = yy² = y,

x = xy = x(yy²) = y²(xy) = y²x = yx = y² = y.

Thus, S is left quasi-cancellative. Applying Theorem 5, we get that S is right quasi-cancellative. Hence, S is quasi-cancellative.

(3) Let S be a quasi-separative CA-groupoid. For any x, y$\in$S, if x = xy and y² = yx, then:

x² = xx = x(xy) = y(xx) = x(yx) = xy² = x(yy) = y(xy) = yx = y²

That is, y² = yx = x². By Definition 7 we have x = y. This means that S is left quasi-cancellative. Applying Theorem 5, we get that S is right quasi-cancellative. Hence, S is quasi-cancellative.

(4) It follows from (3) and Theorem 4. □

Example 9.

Let S = {1,2,3,4,5}. Theoperation* on S is defined as

Table 9. The operation * on S.

*	1	2	3	4	5
1	3	3	5	3	3
2	3	3	5	3	3
3	4	4	3	5	5
4	3	3	5	3	3
5	3	3	5	3	3

. Then (S, *) is a quasi-cancellative CA-groupoid, S isn’t separative, because 2*2 = 2*4 = 3, 4*4 = 4*2 = 3, but 2$\ne$4.

Definition 10.

Let (S, *) be a CA-groupoid. S is called to be power-cancellative, if for all x, y$\in$S, x² = y² implies x = y.

Example 10.

Let S = {1,2,3,4,5}. The operation * on S is defined as

Table 10. The operation * on S.

*	1	2	3	4	5
1	1	1	1	4	5
2	1	2	1	4	5
3	1	1	3	4	5
4	4	4	4	5	1
5	5	5	5	1	4

. Then (S,*) is a power- cancellative CA-groupoid, S isn’t cancellative, because 1*2 = 1*3, but 2$\ne$3.

Example 11.

Let S = {1,2,3,4}. The operation * on S is defined as

Table 11. The operation * on S.

*	1	2	3	4
1	1	2	3	4
2	2	1	4	3
3	3	4	2	1
4	4	3	1	2

. Then (S, *) is a cancellative CA-groupoid, S isn’t power-cancellative, because 1² = 2² = 1, but 1$\ne$2.

Theorem 7.

Let S be a CA-groupoid. If S is power-cancellative, then:

(1) 

S is commutative, and S is a commutative semigroup.

(2) 

S is separative.

Proof. 

(1) Suppose that S is power-cancellative. For any x, y$\in$S, since (by Proposition 1 (1)):

(xy)² = (xy)(xy) = (yx)(xy) = (yy)(xx) = y²x²;

(yx)² = (yx)(yx) = (xy)(yx) = (xx)(yy) = x²y².

Moreover,

[(yx)(xy)]² = [(yx)(xy)] [(yx)(xy)] = [(yx)(xy)] [(yy)(xx)] = [(yx)(xy)] (y²x²) = [(yx)(xy)] (xy)² =

[(yx)(xy)] [(xy)(xy)] = [(xy)(yx)] [(xy)(xy)] = [(xx)(yy)] [(xy)(xy)] = (x²y²) [(xy)(xy)] =

(yx)² [(xy)(xy)] = [(yx)(yx)] [(xy)(xy)] = [(xy)(yx)] [(xy)(yx)] = [(xy)(yx)]²

Applying the condition that S is power-cancellative, we get that (yx)(xy) = (xy)(yx). Thus:

(xy)² = y²x² = (yx)(xy) = (xy)(yx) = x²y² = (yx)².

By Definition 10, we have xy = yx. This means that S is commutative, and S is a commutative semigroup (by Proposition 2).

(2) Assume that S is power-cancellative. For any x, y$\in$S, if x² = xy = y², then (by Definition 10), x = y. This means that S is quasi-separative. Applying Theorem 4, we know that S is separative. □

5. Variant CA-Groupoids

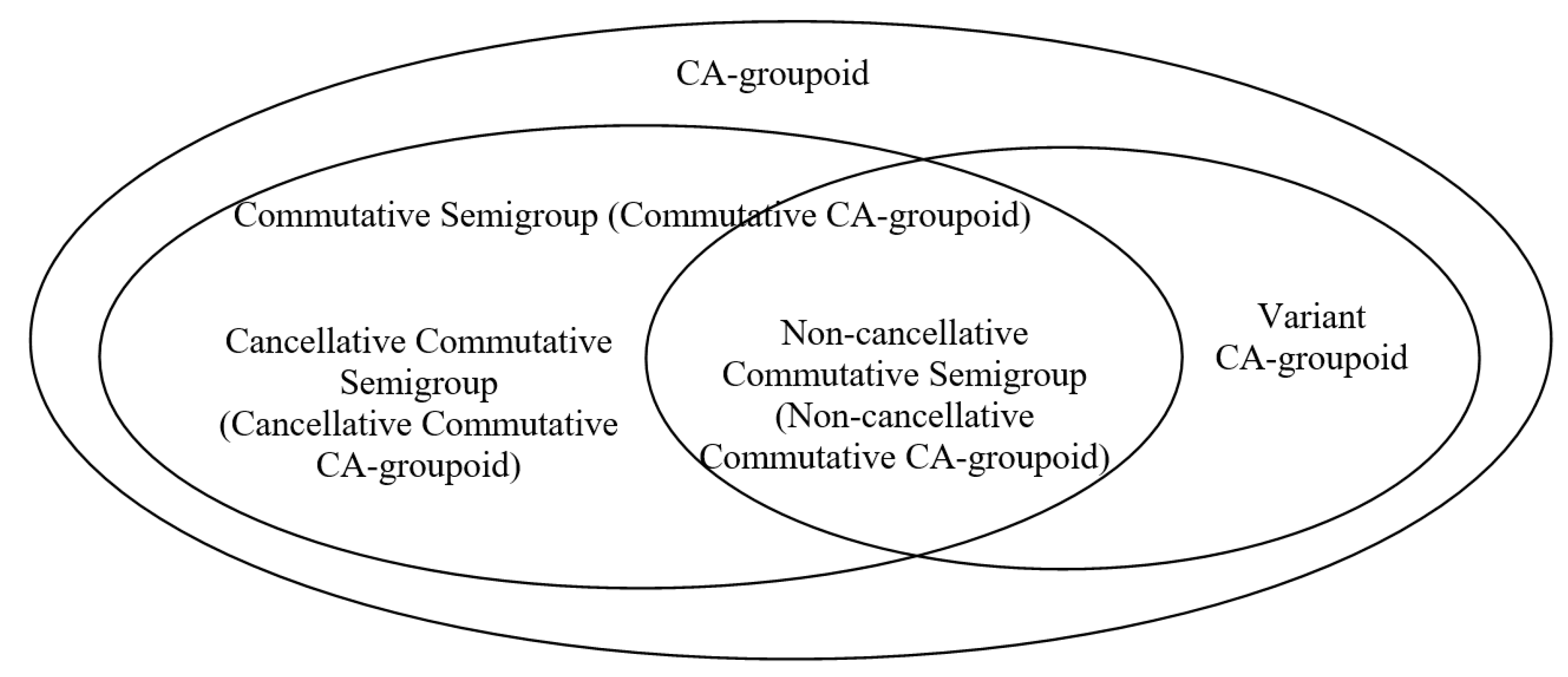
In this section, we focus on a special class of CA-groupoids, which are called variant CA-groupoids. The reasons why we want to discuss this kind of CA-groupoids are that: (1) it is closely related to the generalized unit element (i.e., quasi right unit element), and it is the closest to the commutative semigroup (see Example 12 and Example 13 below); (2) this kind of CA-groupoids has many interesting properties, and it can constructed from any commutative semigroup, please refer to the following Theorem 9; (3) the research this kind of CA-groupoids is of great significance to study some special rings and semirings. See literature [7,8,9] and Example 14 and Example 15 below.

Definition 11.

Let (S, *) be a CA-groupoid. S is called a variant CA-groupoid, if exist e$\in$S, such that for all x$\in$S−{e}, xe = x and e²$\ne$e. Where, e is called a quasi-right unite element of S.

Example 12.

Let S = {1, 2, 3, 4, 5}, The operation * on S is defined as

Table 12. The operation * on S.

*	1	2	3	4	5
1	3	3	3	4	4
2	2	3	3	4	4
3	3	3	3	4	4
4	4	4	4	4	4
5	5	4	4	4	4

, then (S, *) is a variant CA-groupoid and 1 is a quasi-right unit element in S. Obviously, S isn’t commutative.

Looking at the above example carefully, we find that: (1) the element 1 as a quasi-right unit element of S, does not appear in the operation table; (2) in the operation table, the first row is the same as the third row; (3) if we change the first row of the operation table to {1, 2, 3, 4, 5}, we will get a commutative semigroup (S, +) (as shown in

Table 13. A Commutative semigroup (S, +) corresponding to (S, *).

+	1	2	3	4	5
1	1	2	3	4	5
2	2	3	3	4	4
3	3	3	3	4	4
4	4	4	4	4	4
5	5	4	4	4	4

). These are all interesting phenomena. Later, we will analyze the characteristics of variant CA-groupoids.

Example 13.

Let S = {1, 2, 3, 4, 5}, The operation * on S is defined as

Table 14. The operation * on S.

*	1	2	3	4	5
1	1	1	1	1	1
2	1	4	2	3	2
3	1	2	3	4	3
4	1	3	4	2	4
5	1	2	3	4	3

, then (S, *) is a variant CA-groupoid and 5 is a quasi-right unit element in S. Obviously, S is commutative.

If we change the last row of the operation table to {1, 2, 3, 4, 5}, we will get a commutative semigroup (S, +) (as shown in

Table 15. A Commutative semigroup (S, +) corresponding to (S, *).

+	1	2	3	4	5
1	1	1	1	1	1
2	1	4	2	3	2
3	1	2	3	4	3
4	1	3	4	2	4
5	1	2	3	4	5

).

Example 14.

Let:

$$S=\left\{\left(\begin{array}{cc}a& 0\\ 0& 0\end{array}\right):aisaintegralnumber\right\}{\displaystyle \cup \left\{\left(\begin{array}{cc}1& 0\\ 0& 1\end{array}\right),\left(\begin{array}{cc}1& 0\\ 0& -1\end{array}\right)\right\}}.$$

Define the operation * on S is the common matrix multiplication, then (S, *) is a variant CA-groupoid and$\left(\begin{array}{cc}1& 0\\ 0& -1\end{array}\right)$is a quasi-right unit element in S. Moreover, we define the addition operation + on S as following: for any x, y∈S, denote S₁ =$\left\{\left(\begin{array}{cc}a& 0\\ 0& 0\end{array}\right):aisaintegralnumber\right\}$, S₂ =$\left\{\left(\begin{array}{cc}1& 0\\ 0& 1\end{array}\right),\left(\begin{array}{cc}1& 0\\ 0& -1\end{array}\right)\right\}$,

(1) 

if x, y∈ S₁, x + y is common matrix addition;

(2) 

if x∈S₁ and y∈S₂, x + y =$\left(\begin{array}{cc}a+1& 0\\ 0& 0\end{array}\right)$, where y =$\left(\begin{array}{cc}a& 0\\ 0& 0\end{array}\right)$;

(3) 

if x∈ S₂ and y∈S₁, x + y = y + x (see (2));

(4) 

if x = y∈S₂, x + y =$\left(\begin{array}{cc}0& 0\\ 0& 0\end{array}\right)$;

(5) 

if x,y∈S₂ and x ≠ y, x + y =$\left(\begin{array}{cc}2& 0\\ 0& 0\end{array}\right)$.

Then (S, +) is a commutative group, and (S; +, *) is a ring, that is, (x + y)*z = x*z + y*z and z*(x + y) = z*x + z*y, for any x, y, z∈S.

Example 15.

Let S = {1, 2, 3, 4, 5, 6}, The operation * on S is defined as

Table 16. The operation * on S.

*	1	2	3	4	5	6
1	3	3	3	4	4	6
2	2	3	3	4	4	6
3	3	3	3	4	4	6
4	4	4	4	4	4	6
5	5	4	4	4	4	6
6	6	6	6	6	6	6

, then (S, *) is a variant CA-groupoid and 1 is a quasi-right unit element in S. Obviously, S is not commutative.

Moreover, we define the addition operation + on S as

Table 17. A Commutative monoid (S, +).

+	1	2	3	4	5	6
1	1	1	1	1	1	1
2	1	2	3	3	3	2
3	1	3	3	3	3	3
4	1	3	3	4	5	4
5	1	4	3	5	5	5
6	1	2	3	4	5	6

or

Table 18. Another Commutative monoid (S, +).

+	1	2	3	4	5	6
1	1	2	1	4	5	1
2	2	2	2	4	5	2
3	1	2	3	4	5	3
4	4	4	4	4	5	4
5	5	5	5	5	4	5
6	1	2	3	4	5	6

, then (S, +) is a commutative semigroup with unite 6. We can verify that (x + y)*z = x*z + y*z for any x, y, z in S, so (S; +, *) is a semiring (for the theory of semirings, please see the monograph [33,34,35]).

Theorem 8.

Let S be a variant CA-groupoid:

(1) 

If e is aquasi-rightunit element of S and ee = a, a$\in$S, then ex = ax for all x$\in$S.

(2) 

The quasi-right unite elementis unique in S.

Proof. 

(1) Let e be a quasi-right unit element of S and ee = a, a$\in$S. By Definition 11, we know that a ≠ e. For any x∈S, if x = e, then ex = ee = a = ae = ax; if x ≠ e, then (by Definition 11):

ex = e*xe = e*ex = x*ee = xa = xe*ae = e*(xe*a) = a*(e*xe) = a*(e*ex) = a*(x*ee) = a*xa = a*ax

= x*aa = x*(a*ee) = x*(e*ae) = ae*xe = ax.

hence, ex = ax for all x∈S.

(2) Suppose that s and t are quasi-right unit elements of S, s$\ne$t. From Definition 11 we know that ss$\ne$s and tt$\ne$t. Since:

s = st = st*ts = s*(st*t) = t*(s*st) = t*(t*ss) = t*(s*ts) = t*st = ts = t.

This means that the quasi-right unit element is unique in S. □

Obviously, let S = {a} and (S, *) is a CA-groupoid, then S isn’t a variant CA-groupoid. Let S = {a, b} and (S, *) is a variant CA-groupoid, denote the quasi-right unit element e = a (or b), then for any x, y∈S, we have xy = b (or a).

Through the study of the variant CA-groupoid, we give the following construction method, that is to say, on the basis of a commutative semigroup, a variant CA-groupoid is formed by adding an element which does not intersect with it, and a variant CA-groupoid can also be decomposed to obtain a commutative semigroup and an independent element.

Theorem 9.

The following asserts are true:

(1) 

Let S be a variant CA-groupoid and e is the quasi-right unite element on S, then S₁ = S−{e} is a commutative semigroup.

(2) 

Let S be a commutative monoid with unit element e and a is an element such that {a}${{\displaystyle \cap }}^{\text{}}$S = $\varnothing$, then S₂ = S${{\displaystyle \cup }}^{\text{}}${a} is a variant CA-groupoid if define xa = x, ax = ex, aa = e, for all x$\in$S.

Proof. 

(1) Suppose that S is a variant CA-groupoid and e is the quasi-right unit element of S, if $\exists$x, y∈ S₁ = S−{e} such that xy = e, then for all a∈S−{e}, a*xy = ae = a, so we have:

ee = e*xy = y*ex = x*ye = xy = e.

This conclusion contradicts Definition 11. Hence, for all x, y$\in$S−{e}, xy$\ne$e, in other words, S−{e} is closed, that is, S−{e} is a sub CA-groupoid of S. Moreover, for all x, y∈ S₁ = S−{e}, applying Theorem 8 (1), ex = (ee)x, and:

xy = x*ye = e*xy = y*ex = y*(ee*x) = x*(y*ee) = x*(e*ye) = ye*xe = yx

hence S−{e} is commutative, then S−{e} is a commutative semigroup (by Proposition 2).

(2) On the other hand, suppose that S is commutative monoid with unit element e. Let a be an element such that {a}${{\displaystyle \cap }}^{\text{}}$S = $\varnothing$, denote S₂ = S${{\displaystyle \cup }}^{\text{}}${a}. Define a new binary operation • on S₂:

for any x, y∈ S₂, if x, y∈ S, then x• y = x*y; if x∈ S, then x•a = x, a•x = e•x, a•a = e.

Obviously, (S₂, •) is a groupoid. For all x, y, z$\in$S, by the definition of operation • we have:

x•yz = x*yz = z*xy = z•xy,

a•aa = a•aa,

x•aa = xe = ex = ax = a•xa,

a•xa = ax = bx = xe = x•ee = e•ex = a•ax,

a•ax = e•ex = e•xe = xe•e = x•ee = xe = x•aa,

y•ax = ax•y = ex•y = xy = x•ya,

x•ya = xy = e•xy = a•xy,

a•xy = (a•xa)•ya = ya•(a•xa) = y•ax.

thus, (S₂, •) is a variant CA-groupoid with the quasi-right unit element a. □

Applying Definition 11 and Definition 9 we can easy to verify that the following proposition is true.

Proposition 7.

(1) If S is a variant CA-groupoid, then S isn’t cancellative. (2) If S is a cancellative CA-groupoid, then S isn’t a variant CA-groupoid.

From Theorem 9, Proposition 7, Examples 12~15, we have Figure 1.

Theorem 10.

Let S be a variant CA-groupoid and e be a quasi-right unit element of S. Denote a = ee, b = aa. Then the following asserts are true:

(1)

If b = a, then {e, a} is a variant sub CA-groupoid of S;

(2)

If b$\ne$a, then {e, a, b} is a variant sub CA-groupoid of S.

Proof. 

(1) Suppose b = a. For the set {e, a}, since (by Theorem 8):

ee = a, aa = b = a, ae = a, ea = ee*a = aa = a.

It follows that {e, a} is closed on the operation *. Thus, {e, a} is a variant sub CA-groupoid with quasi-right unit element e.

(2) Assume b$\ne$a. By Theorem 9 (1), for all x, y∈$S-\left\{e\right\}$, xy = yx. For the set {e, a, b}, since (by Theorem 8):

ee = a, aa = b, ae = a, ea = ee*a = aa = b;

eb = e*aa = a*ea = a*ae = aa = b, be = b;

ba = ab = ee*aa = a*(ee*a) = a*(a*ee) = a*(e*ae) = ae*ae = aa = b.

Thus, {e, a, b} is closed about *, so {e, a, b} is a variant sub CA-groupoid of S. □

Theorem 11.

Let (S₁, *₁) and (S₂, *₂) be two variant CA-groupoids, e₁ and e₂ are quasi-right unit elements of (S₁, *₁) and (S₂, *₂), S₁${{\displaystyle \cap }}^{\text{}}$S₂ = {e} (e = e₁ = e₂). Denote S = S₁${{\displaystyle \cup }}^{\text{}}$S₂, and define the operation * on S as follows:

(i) if a, b$\in$S₁, then a*b = a*₁b;

(ii) if a, b$\in$S₂, then a*b = a*₂b;

(iii) if a$\in$S₁-{e}, b$\in$S₂-{e}, then a*b = b;

(iv) if a$\in$S₂-{e}, b$\in$S₁-{e}, then a*b = a.

Then (S, *) is a variant CA-groupoid with the quasi-right unite e.

Proof. 

It is only necessary to prove that the cyclic associative law hold in (S, *), that is, a*(b*c) = c*(a*b) for all a, b, c$\in$S. We will discuss the following situations separately:

(1)

If a, b, c$\in$S₁, or a, b, c$\in$S₂, then a*(b*c) = c*(a*b);

(2)

If a$\in$S₁-{e}, b$\in$S₂-{e} and c$\in$S₂-{e}, then a*(b*c) = b*c = c*b = c*(a*b);

(3)

If a$\in$S₂-{e}, b$\in$S₁-{e} and c$\in$S₂-{e}, then a*(b*c) = a*c = c*a = c*(a*b);

(4)

If a$\in$S₂-{e}, b$\in$S₂-{e} and c$\in$S₁-{e}, then a*(b*c) = a*b = c*(a*b);

(5)

If a$\in$S₁-{e}, b$\in$S₁-{e} and c$\in$S₂-{e}, then a*(b*c) = a*c = c = c*(a*b);

(6)

If a$\in$S₁-{e}, b$\in$S₂-{e} and c$\in$S₁-{e}, then a*(b*c) = a*b = c*(a*b);

(7)

If a$\in$S₂-{e}, b$\in$S₁-{e} and c$\in$S₁-{e}, then a*(b*c) = a = a*b = c*(a*b).

Then (S, *) is a variant CA-groupoid and e is the quasi-right unit element. □

Example 16.

Let S₁ = {1, 2, 3, 4} and S₂ = {1, 5, 6, 7}. Define operations *₁ and *₂ on S₁, S₂ as following

Table 19. The operation *₁ on S_1.

*1	1	2	3	4
1	2	2	4	4
2	2	2	4	4
3	3	4	4	4
4	4	4	4	4

and

Table 20. The operation *₂ on S_2.

*2	1	5	6	7
1	5	5	7	7
5	5	5	7	7
6	6	7	5	5
7	7	7	5	5

. Then S = S₁${{\displaystyle \cup }}^{\text{}}$S₂ ={1, 2, 3, 4, 5, 6}, and (S, *) is a variant CA-groupoid with the operation * in

Table 21. The operation * on S.

*	1	2	3	4	5	6	7
1	2	2	4	4	5	6	7
2	2	2	4	4	5	6	7
3	3	4	4	4	5	6	7
4	4	4	4	4	5	6	7
5	5	5	5	5	5	7	7
6	6	6	6	6	7	5	5
7	7	7	7	7	7	5	5

.

Similar to Theorem 11, we can get another constructer method as following proposition (the proof is omitted).

Proposition 8.

Let (S₁, *₁) and (S₂, *₂) be two variant CA-groupoids, e₁ and e₂ are variant unit elements of (S₁, *₁) and (S₂, *₂), S₁${{\displaystyle \cap }}^{\text{}}$S₂ =$\varnothing$and S₂ is commutative. Denote S = S₁${{\displaystyle \cup }}^{\text{}}$S₂, and define the operation * in S as follows:

(1) 

if a, b$\in$S₁, then a*b = a*₁b;

(2) 

if a, b$\in$S₂, then a*b = a*₂b;

(3) 

if a$\in$S₁, b$\in$S₂, then a*b = b;

(4) 

if a$\in$S₂, b$\in$S₁, then a*b = a.

Then (S, *) is a variant CA-groupoid with the quasi-right unite e₁.

Example 17.

Let S₁ = {1, 2, 3, 4} and S₂ = {5, 6, 7, 8}. Define operations *₁ and *₂ on S₁, S₂ as following

Table 22. The operation *₁ on S_1.

*1	1	2	3	4
1	3	2	2	4
2	2	2	2	4
3	3	2	2	4
4	4	4	4	4

and

Table 23. The operation *₂ on S_2.

*2	5	6	7	8
5	8	6	7	8
6	6	6	6	6
7	7	6	6	7
8	8	6	7	8

. Then S = S₁${{\displaystyle \cup }}^{\text{}}$S₂ ={1, 2, 3, 4, 5, 6, 7, 8}, and (S, *) is a variant CA-groupoid with the operation * in

Table 24. The operation * on S.

*	1	2	3	4	5	6	7	8
1	3	2	2	4	5	6	7	8
2	2	2	2	4	5	6	7	8
3	3	2	2	4	5	6	7	8
4	4	4	4	2	5	6	7	8
5	5	5	5	5	8	6	7	8
6	6	6	6	6	6	6	6	6
7	7	7	7	7	7	6	6	7
8	8	8	8	8	8	6	7	8

.

Theorem 12.

Let S₁ be a variant CA-groupoid with order n (n ≥ 2 and n is an even number) and the quasi- right unit element e₁$\in$S₁, let S₂ be a variant CA-groupoid with order 2 and the quasi-right unit element e₂$\in$S₂. If S = S₁${{\displaystyle \cup }}^{\text{}}$S₂ and S₁${{\displaystyle \cap }}^{\text{}}$S₂ =$\varnothing$, then S is a variant CA-groupoid, when it such that any of the following conditions:

(1) 

for thevariantCA-groupoid S, thequasi-rightunit element e = e₁, and e₂*e₁ = e₂, and for all x$\in$S, x*(e₂* e₂) = (e₂* e₂)*x = e₂* e₂, x* e₂ = e₂* e₂, e₂*x = e₂* e₂ (x$\ne$e₁);

(2) 

for thevariantCA-groupoid S, thequasi-rightunit element e = e₁ and for all x$\in$S, x*(e₂* e₂) = (e₂* e₂)*x = e₂* e₂, x* e₂ = e₂*x = e₂.

Proof. 

(1) Suppose that S is constructed according to the method described in (1), then for all x, y, z$\in$S₁, x*yz = z*xy = y*zx, and:

x*ye₂ = x*e ₂ e₂ = e₂ e₂, e₂*xy = e₂ e ₂ (xy ≠ e₁)

$$\begin{array}{c}\mathit{y}\ast {\mathit{e}}_{\mathbf{2}}\mathit{x} = \{\begin{array}{c}\mathit{y}\ast {\mathit{e}}_{\mathbf{2}}{\mathit{e}}_{\mathbf{1}} = \mathit{y}{\mathit{e}}_{\mathbf{2}} = {\mathit{e}}_{\mathbf{2}}{\mathit{e}}_{\mathbf{2}}\begin{array}{cc}& \mathit{x} = {\mathit{e}}_{\mathbf{1}}\end{array}\\ \mathit{y}\ast {\mathit{e}}_{\mathbf{2}}{\mathit{e}}_{\mathbf{2}} = {\mathit{e}}_{\mathbf{2}}{\mathit{e}}_{\mathbf{2}}\begin{array}{cc}& \mathit{x}\ne {\mathit{e}}_{\mathbf{1}}\end{array}\end{array}\end{array}$$

That is, x*ye₂ = e₂*xy = y*e₂x. Denote e₂ e₂ = b, then:

x*yb = xb = b, b*xy = b, y*bx = yb = b. That is, x*yb = b*xy = y*bx.

x*e₂e₂ = xb = b, e₂*xe₂ = e₂*e₂ e₂ = b,

$$\begin{array}{c}{\mathit{e}}_{\mathbf{2}}\ast {\mathit{e}}_{\mathbf{2}}\mathit{x} = \{\begin{array}{c}{\mathit{e}}_{\mathbf{2}}\ast {\mathit{e}}_{\mathbf{2}}{\mathit{e}}_{\mathbf{1}} = {\mathit{e}}_{\mathbf{2}}{\mathit{e}}_{\mathbf{2}} = \mathit{b}\begin{array}{cc}& \mathit{x} = {\mathit{e}}_{\mathbf{1}}\end{array}\\ {\mathit{e}}_{\mathbf{2}}\ast {\mathit{e}}_{\mathbf{2}}{\mathit{e}}_{\mathbf{2}} = \mathit{b}\begin{array}{cc}& \mathit{x}\ne {\mathit{e}}_{\mathbf{1}}\end{array}\end{array}\end{array}$$

Thus, x*e₂e₂ = e₂*xe₂ = e₂*e₂x. And:

x*be₂ = x*e₂ b = xb = b, b*xe₂ = b*e₂ e₂ = b = e₂ b = e₂*bx, e₂*xb = e₂ b = b,

$$\begin{array}{c}\begin{array}{c}\mathit{b}\ast {\mathit{e}}_{\mathbf{2}}\mathit{x} = \{\begin{array}{c}\mathit{b}\ast {\mathit{e}}_{\mathbf{2}}{\mathit{e}}_{\mathbf{1}} = \mathit{b}{\mathit{e}}_{\mathbf{2}} = \mathit{b}\begin{array}{cc}& \mathit{x} = {\mathit{e}}_{\mathbf{1}}\end{array}\\ \mathit{b}\ast {\mathit{e}}_{\mathbf{2}}{\mathit{e}}_{\mathbf{2}} = \mathit{b}\begin{array}{cc}& \mathit{x}\ne {\mathit{e}}_{\mathbf{1}}\end{array}\end{array}\end{array}\end{array}$$

It follows that x*be₂ = e₂*xb = b*e₂x, and x*e₂b = b*xe₂ = e₂*bx. Obviously, x*bb = b*xb = b*bx. Hence, S is a variant CA-groupoid.

(2) Suppose that S is constructed according to the method described in (2), then for all x, y, z$\in$S₁, x*yz = z*xy = y*zx, and:

x*ye₂ = xe₂ = e₂, e₂*xy = e₂, y*e₂x = ye₂ = e₂.

Then x*ye₂ = e₂*xy = y*e₂x. Assume e₂e₂ = b, then:

x*yb = xb = b, b*xy = b, y*bx = yb = b.

That is, x*yb = b*xy = y*bx. And:

x*e₂ e₂ = xb = b, e₂*xe₂ = e₂*e₂ x = e₂ e₂ = b.

Thus, x*e₂e₂ = e₂*xe₂ = e₂*e₂x. Moreover:

x*be₂ = x*e₂ b = xb = b, b*xe₂ = be₂ = b = e₂b = e₂*bx, e₂*xb = e₂ b = b = be₂ = b*e₂x.

It follows that x*be₂ = e₂*xb = b*e₂x, and x*e₂b = b*xe₂ = e₂*bx. Obviously, x*bb = b*xb = b*bx. Hence, S is a variant CA-groupoid. □

6. Conclusions

In the paper, we mainly study various cancellabilities of CA-groupoids and the structural properties of a special kind of CA-groupoids (variant CA-groupoids). Firstly, we investigate some cancellabilities of CA-groupoids, including left (right) cancellation, weak cancellation, left (right) quasi-cancellation and left (right) separation, and analyze the relationships among them. Secondly, from the view of quasi-right unit element, we introduce the new notion of variant CA-groupoid, illustrate the close connections among variant CA-groupoid with commutative semigroup, ring and semiring by some examples; discuss deeply the characteristics of variant CA-groupoid, and establish its structure theorem and construction methods. This paper obtains many conclusions, some important results as follows:

(1)

Every left cancellative element in CA-groupoid is right cancellative (see Theorem 1);

(2)

For a CA-groupoid, it is left cancellative if and only if it is right cancellative (see Theorem 1 and Corollary 1);

(3)

For a CA-groupoid, it is left separative if and only if it is right separative, and if and only if it is quasi-separative (see Theorem 4 and Corollary 1);

(4)

Every left quasi-cancellative CA-groupoid is right quasi-cancellative (see Theorem 5); every power cancellative CA-groupoid is separative (see Theorem 7);

(5)

For a variant CA-groupoid, its quasi-right unit element is unique;

(6)

A variant CA-groupoid can be decomposed into the quasi-right unit element and a commutative CA-groupoid; starting from any commutative semigroup, one can construct a variant CA-groupoid (see Theorem 9);

(7)

There are many ways to construct a new variant CA-groupoid from the existing variant CA-groupoids (see Theorems 11 and 12).

As a direction of future research, we will discuss the structural characteristics of CA-rings, CA-semirings and related algebraic systems (see [36,37,38,39]).