Flow Symmetry and Heat Transfer Characteristics of Winglet Vortex Generators Arranged in Common Flow up Configuration
Abstract
1. Introduction
2. Physical Model, Methods and Formulations
3. Results and Discussions
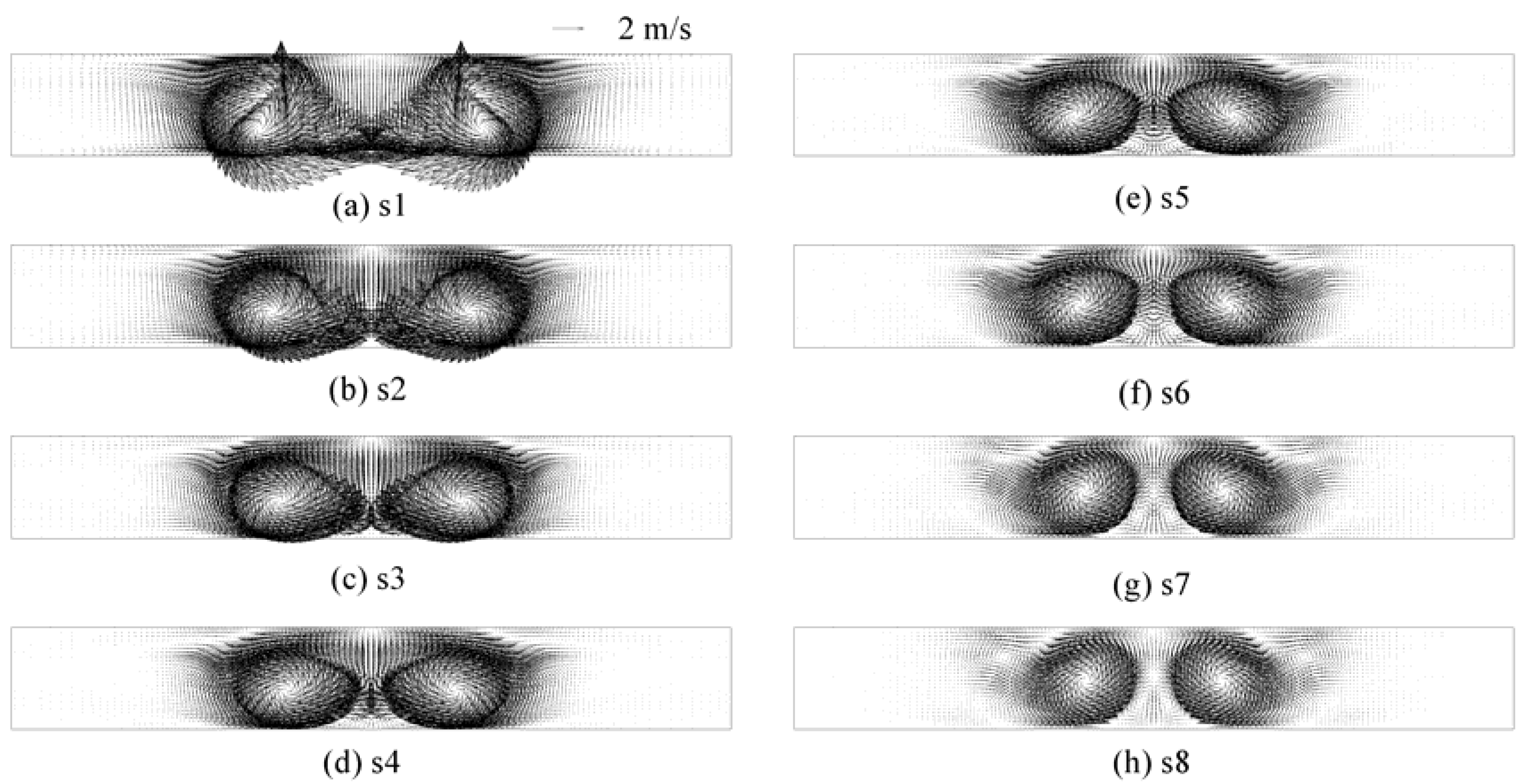
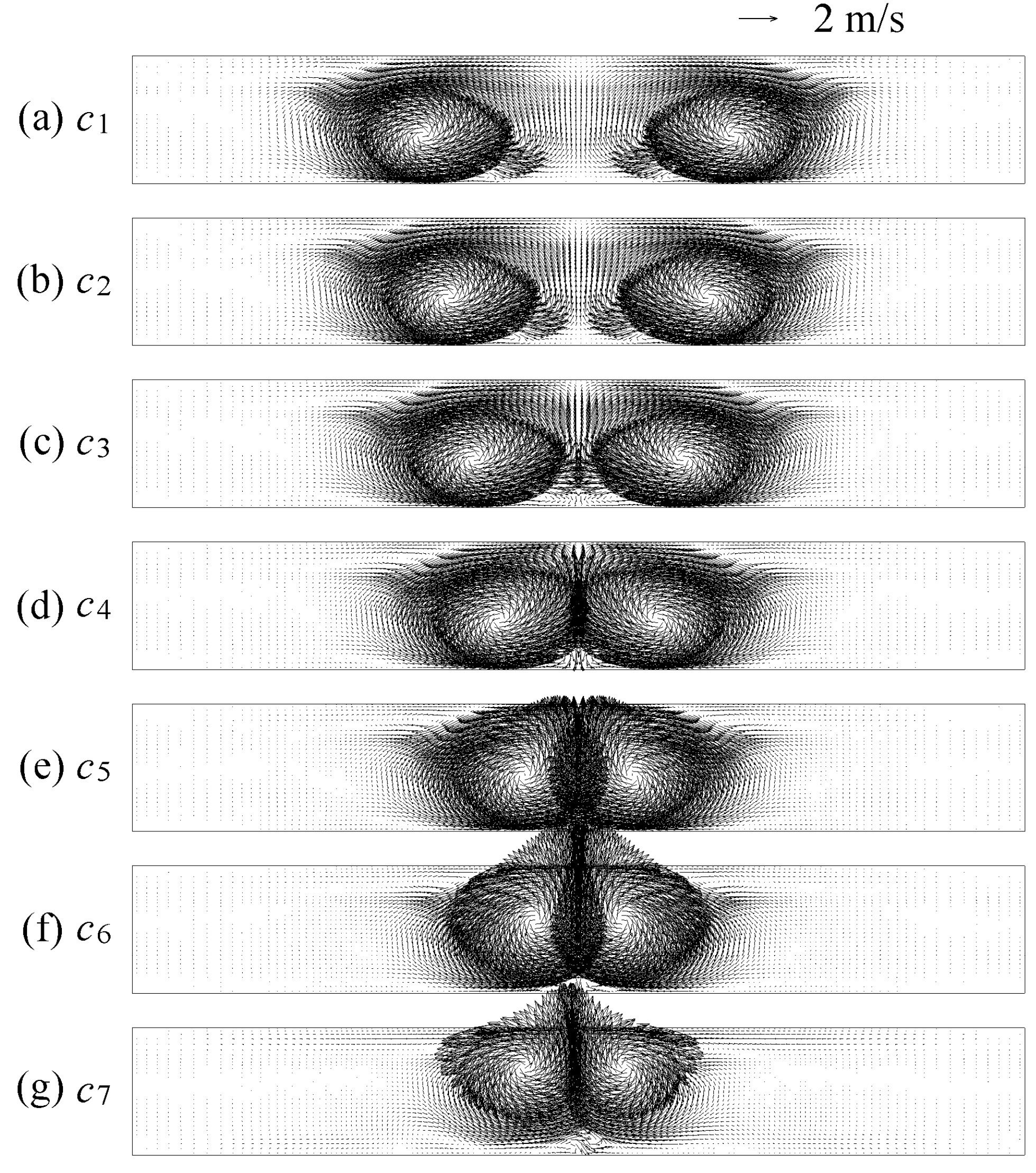
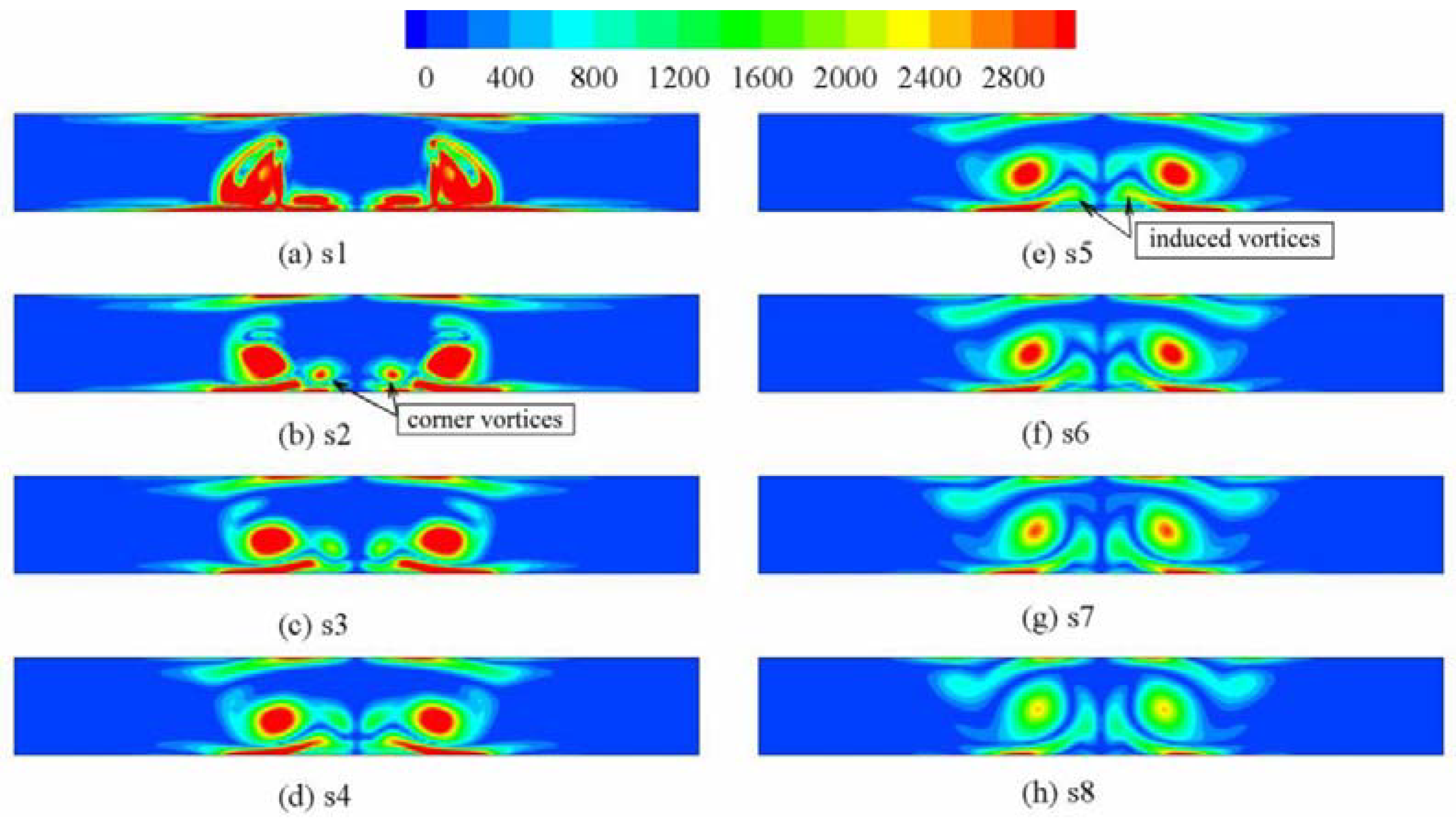
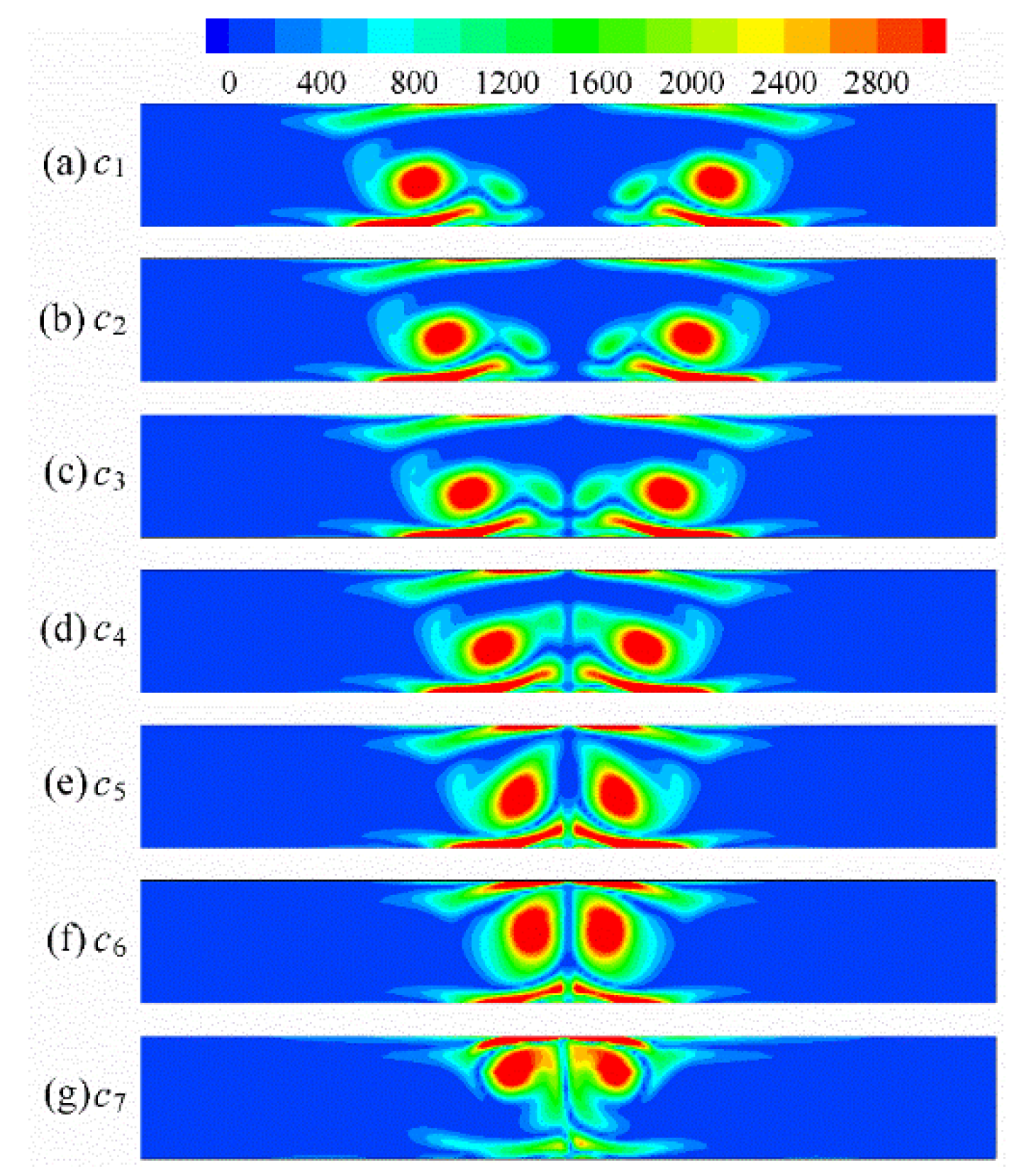
3.1. Symmetrical Vortex Structure and Vortex Intensity
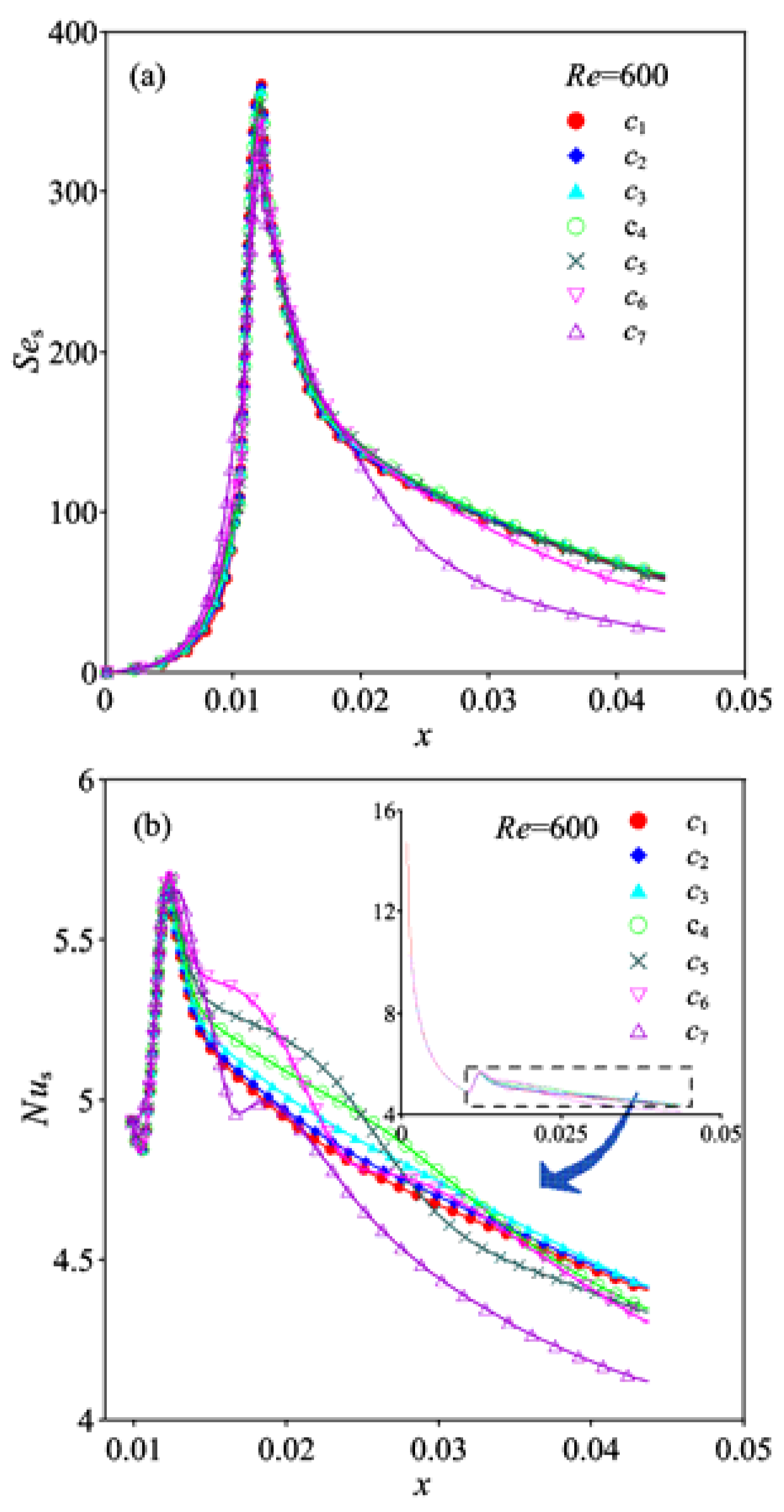
3.2. Distributions of the Span-Average Values of Ses and Nus
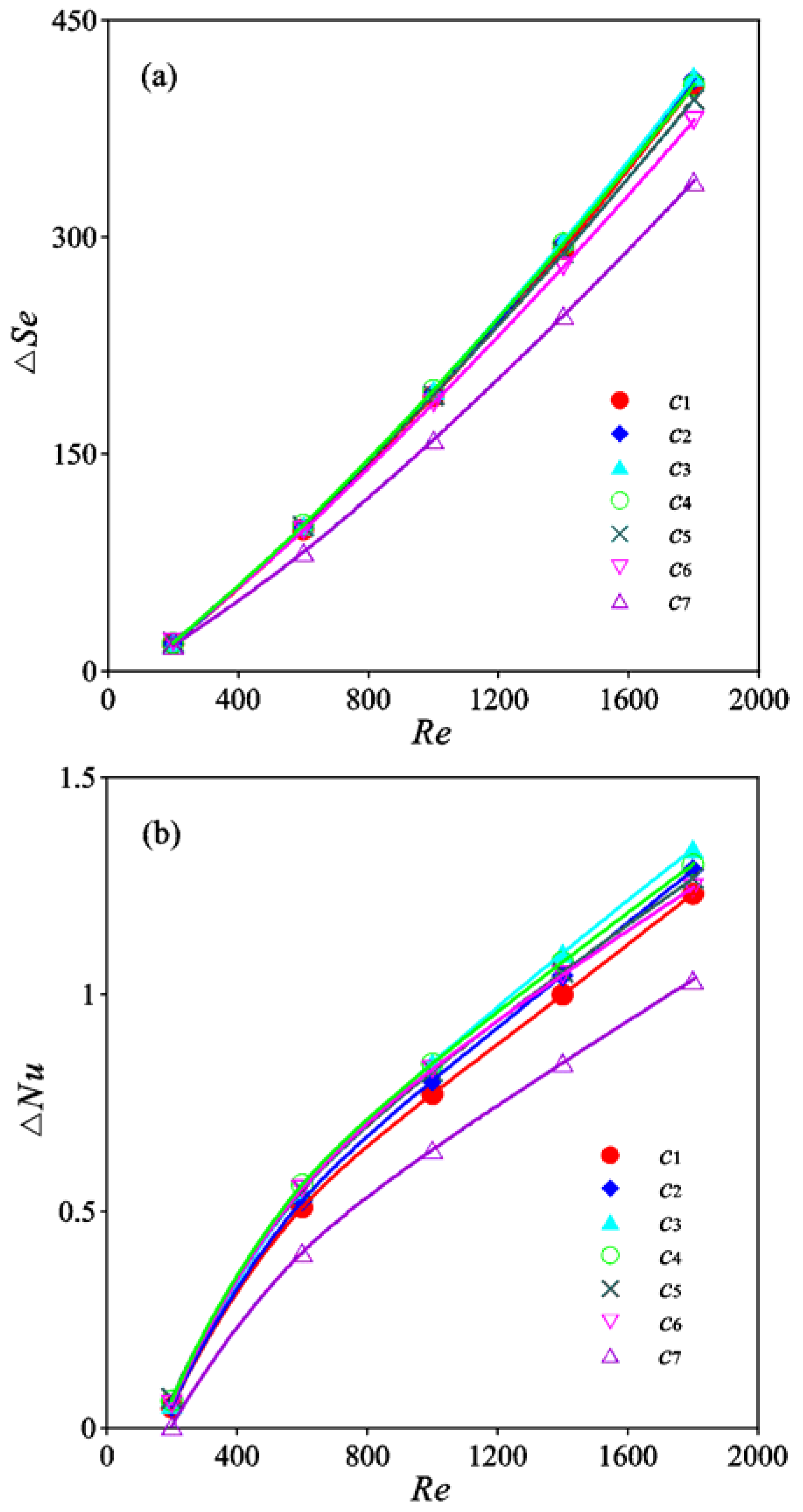
3.3. Distributions of Se, Nu, f and JF
4. Conclusions
- (1)
- The distribution of the longitudinal vortices of the winglet VGs pair has a perfect flow symmetry on the cross-section, and the symmetrical vortex structure is affected by the vortex interaction. The largest vortex intensity of c3 is approximately 21.4% larger than that of c7.
- (2)
- The transverse pitch of the winglet VGs strongly affects the vortex interaction and heat transfer performance, while the difference in the friction factor is slight. The increment of Nu for c3 is approximately 29.2% larger than that for c7. Thus, thermal performance can be strongly enhanced by optimizing the transverse pitch and the interaction between the longitudinal vortices.
- (3)
- An optimal transverse pitch exists for the studied configuration of winglet VGs in terms of the highest thermal performance factor. JF for the transverse pitch c3 is the largest and is approximately 5.0% larger than that for c7.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Cross-section area (m2) |
| B | width of simulation domain (m) |
| cp | specific heat at constant pressure (J/(kg·K)) |
| dh | hydraulic diameter (m) |
| D | location of VG from inlet (m) |
| f | friction factor (−) |
| tp | fin spacing |
| H | vortex generator height (m) |
| JF | surface goodness factor (−) |
| L | length of simulation domain (m) |
| Nu | Nusselt number (−) |
| Nus | span-average Nusselt number (−) |
| p | pressure (Pa) |
| Re | Reynolds number (−) |
| S | heat transfer area (m2) |
| Se | secondary flow intensity (−) |
| Ses | bulk secondary flow intensity at position x (−) |
| T | temperature (K) |
| T0 | constant temperature at inlet (K) |
| Ts | bulk temperature at position x (K) |
| Tw | fin surface temperature (K) |
| Us | characteristic velocity of secondary flow (m/s) |
| u, v, w | component of velocity (m/s) |
| u0 | constant average velocity at inlet (m/s) |
| um | average velocity (m/s) |
| Greek letters | |
| θ | angle of attack of VG (°) |
| λ | thermal conductivity (W/(m·K)) |
| μ | viscosity (kg/(m·s)) |
| ρ | density (kg/m3) |
| ωn | vorticity along main flow direction (1/s) |
References
- Tao, W.Q. Numerical Heat Transfer, 2nd ed.; Xi’an Jiaotong University Press: Xi’an, China, 2001. [Google Scholar]
- Joardar, A.; Jacobi, A.M. Impact of leading edge delta-wing vortex generators on the thermal performance of a flat tube, louvered-fin compact heat exchanger. Int. J. Heat Mass Transf. 2005, 48, 1480–1493. [Google Scholar] [CrossRef]
- Joardar, A.; Jacobi, A.M. Heat transfer enhancement by winglet-type vortex generator arrays in compact plain-fin-and-tube heat exchangers. Int. J. Refrig. 2008, 31, 87–97. [Google Scholar] [CrossRef]
- Joardar, A.; Jacobi, A.M. A Numerical Study of Flow and Heat Transfer Enhancement Using an Array of Delta-Winglet Vortex Generators in a Fin-and-Tube Heat Exchanger. J. Heat Transf. 2007, 129, 1156–1167. [Google Scholar] [CrossRef]
- Jacobi, A.M.; Shah, R.K. Heat transfer surface enhancement through the use of longitudinal vortices: A review of recent progress. Exp. Therm. Fluid Sci. 1995, 11, 295–309. [Google Scholar] [CrossRef]
- Biswas, G.; Deb, P.; Biswas, S. Generation of longitudinal streamwise vortices—A device for improving heat exchanger design. Heat Transf. 1994, 116, 588–597. [Google Scholar] [CrossRef]
- Fiebig, M. Vortices and heat transfer. J. Appl. Math. Mech. 1977, 77, 3–18. [Google Scholar] [CrossRef]
- Fiebig, M. Vortex generators for compact heat exchangers. Enhanc. Heat Transf. 1995, 2, 43–61. [Google Scholar] [CrossRef]
- Song, K.W.; Wang, Y.; Zhang, Q.; Wang, L.B.; Liu, Y.J. Numerical study of the fin efficiency and a modified fin efficiency formula for flat tube bank fin heat exchanger. Int. J. Heat Mass Transf. 2011, 54, 2661–2672. [Google Scholar] [CrossRef]
- Liu, S.; Wang, L.; Fan, J.; Zhang, Y.; Dong, Y.; Song, K. Tube transverse pitch effect on heat/mass transfer characteristics of flat tube bank fin mounted with vortex generators. J. Heat Transf. 2008, 130, 064502. [Google Scholar] [CrossRef]
- Song, K.W.; Wang, L.B. Relationship between heat transfer intensity and absolute vorticity flux intensity in flat tube bank fin channels with Vortex Generators. Prog. Comput. Fluid Dyn. 2008, 8, 496–502. [Google Scholar] [CrossRef]
- Wu, J.M.; Tao, W.Q. Numerical study on laminar convection heat transfer in a channel with longitudinal vortex generator part B: Parametric study of major influences factors. Int. J. Heat Mass Transf. 2008, 51, 3683–3692. [Google Scholar] [CrossRef]
- Min, C.H.; Qi, C.Y.; Kong, X.F.; Dong, J.F. Experimental study of rectangular channel with modified rectangular longitudinal vortex generators. Int. J. Heat Mass Transf. 2010, 53, 3023–3029. [Google Scholar] [CrossRef]
- Tian, L.T.; He, Y.L.; Lei, Y.G.; Tao, W.Q. Numerical study of fluid flow and heat transfer in a flat-plate channel with longitudinal vortex generators by applying field synergy principle analysis. Int. Commun. Heat Mass Transf. 2009, 36, 111–120. [Google Scholar] [CrossRef]
- Wu, J.M.; Tao, W.Q. Impact of delta winglet vortex generators on the performance of a novel fin-tube surface with two rows of tubes in different diameters. Energy Convers. Manag. 2011, 52, 2895–2901. [Google Scholar] [CrossRef]
- Li, M.J.; Zhang, H.; Zhang, J.; Mu, T.; Tian, E.; Dan, D.; Zhang, X.D.; Tao, W.Q. Experimental and numerical study and comparison of performance for wavy fin and a plain fin with radiantly arranged winglets around each tube in fin and-tube heat exchangers. Appl. Therm. Eng. 2018, 133, 298–307. [Google Scholar] [CrossRef]
- Naik, H.; Tiwari, S. Effect of winglet location on performance of fin-tube heat exchangers with inline tube arrangement. Int. J. Heat Mass Transf. 2018, 125, 248–261. [Google Scholar] [CrossRef]
- Song, K.W.; Xi, Z.P.; Su, M.; Wang, L.B. Effect of geometric size of curved delta winglet vortex generators and tube pitch on heat transfer characteristics of fin-tube heat exchanger. Exp. Therm. Fluid Sci. 2017, 82, 8–18. [Google Scholar] [CrossRef]
- Song, K.W.; Tagawa, T.; Chen, Z.H.; Zhang, Q. Heat transfer characteristics of concave and convex curved vortex generators in the channel of plate heat exchanger under laminar flow. Int. J. Therm. Sci. 2019, 137, 215–228. [Google Scholar] [CrossRef]
- Lu, G.F.; Zhai, X.Q. Effects of curved vortex generators on the air-side performance of fin-and-tube heat exchangers. Int. J. Therm. Sci. 2019, 136, 509–518. [Google Scholar] [CrossRef]
- Han, H.; Wang, S.; Sun, W.L.; Li, Y.; Wang, S. Numerical study of thermal and flow characteristics for a fin-and-tube heat exchanger with arc winglet type vortex generators. Int. J. Refrig. 2019, 98, 61–69. [Google Scholar] [CrossRef]
- Lu, G.F.; Zhai, X.Q. Analysis on heat transfer and pressure drop of fin-and-oval-tube heat exchangers with tear-drop delta vortex generators. Int. J. Heat Mass Transf. 2018, 127, 1054–1063. [Google Scholar] [CrossRef]
- Sarangi, S.K.; Mishra, D.P. Effect of winglet location on heat transfer of a fin-and-tube heat exchanger. Appl. Therm. Eng. 2017, 116, 528–540. [Google Scholar] [CrossRef]
- Song, K.W.; Wang, L.B. The effectiveness of secondary flow produced by vortex generators mounted on both surfaces of the fin to enhance heat transfer in a flat tube bank fin heat exchanger. ASME J. Heat Transf. 2013, 135, 041902. [Google Scholar] [CrossRef]
- Song, K.W.; Liu, S.; Wang, L.B. Interaction of counter rotating longitudinal vortices and the effect on fluid flow and heat transfer. Int. J. Heat Mass Transf. 2016, 93, 349–360. [Google Scholar] [CrossRef]
- Song, K.W.; Hu, W.L.; Liu, S.; Wang, L.B. Quantitative relationship between secondary flow intensity and heat transfer intensity in flat-tube-and-fin air heat exchanger with vortex generators. Appl. Therm. Eng. 2016, 103, 1064–1070. [Google Scholar] [CrossRef]
- Song, K.W.; Wang, L.B. Effects of longitudinal vortex interaction on periodically developed flow and heat transfer of fin-and-tube heat exchanger. Int. J. Therm. Sci. 2016, 109, 206–216. [Google Scholar] [CrossRef]
- Song, K.W.; Tagawa, T. The optimal arrangement of vortex generators for best heat transfer enhancement in flat-tube-fin heat exchanger. Int. J. Therm. Sci. 2018, 132, 355–367. [Google Scholar] [CrossRef]
- Song, K.W.; Shi, W.N.; Wu, X.; Wang, L.B. Characteristics of flow symmetry and heat transfer of winglet pair in common flow down configuration. Symmetry 2020, 12, 209. [Google Scholar] [CrossRef]
- Luo, C.; Wu, S.; Song, K.W.; Hua, L.; Wang, L.B. Thermo-hydraulic performance optimization of wavy fin heat exchanger by combining delta winglet vortex generators. Appl. Therm. Eng. 2019, 163, 114343. [Google Scholar] [CrossRef]

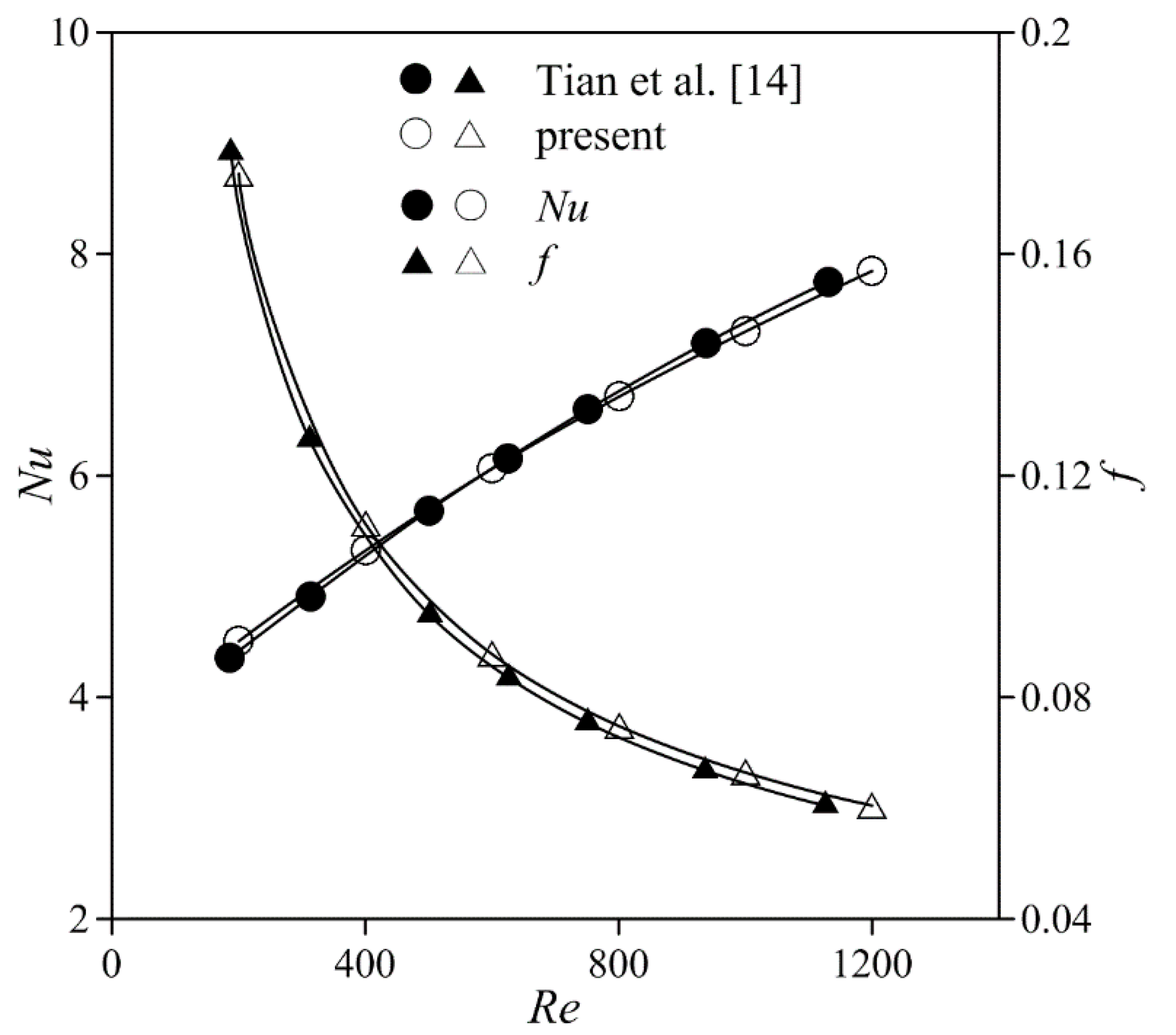


| No. | Grid (x × y × z) | Nu | Relative Error | f | Relative Error |
|---|---|---|---|---|---|
| 1 | 140 × 118 × 26 | 6.738 | 0.18% | 5.864 × 10−2 | 0.14% |
| 2 | 194 × 142 × 32 | 6.726 | - | 5.856 × 10−2 | - |
| 3 | 234 × 168 × 37 | 6.739 | 0.19% | 5.896 × 10−2 | 0.68% |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, K.; Wang, L.; Hu, Y.; Liu, Q. Flow Symmetry and Heat Transfer Characteristics of Winglet Vortex Generators Arranged in Common Flow up Configuration. Symmetry 2020, 12, 247. https://doi.org/10.3390/sym12020247
Song K, Wang L, Hu Y, Liu Q. Flow Symmetry and Heat Transfer Characteristics of Winglet Vortex Generators Arranged in Common Flow up Configuration. Symmetry. 2020; 12(2):247. https://doi.org/10.3390/sym12020247
Chicago/Turabian StyleSong, Kewei, Lu Wang, Yajun Hu, and Qi Liu. 2020. "Flow Symmetry and Heat Transfer Characteristics of Winglet Vortex Generators Arranged in Common Flow up Configuration" Symmetry 12, no. 2: 247. https://doi.org/10.3390/sym12020247
APA StyleSong, K., Wang, L., Hu, Y., & Liu, Q. (2020). Flow Symmetry and Heat Transfer Characteristics of Winglet Vortex Generators Arranged in Common Flow up Configuration. Symmetry, 12(2), 247. https://doi.org/10.3390/sym12020247




