Numerical Investigation of Forced Convective Heat Transfer and Performance Evaluation Criterion of Al2O3/Water Nanofluid Flow inside an Axisymmetric Microchannel
Abstract
1. Introduction
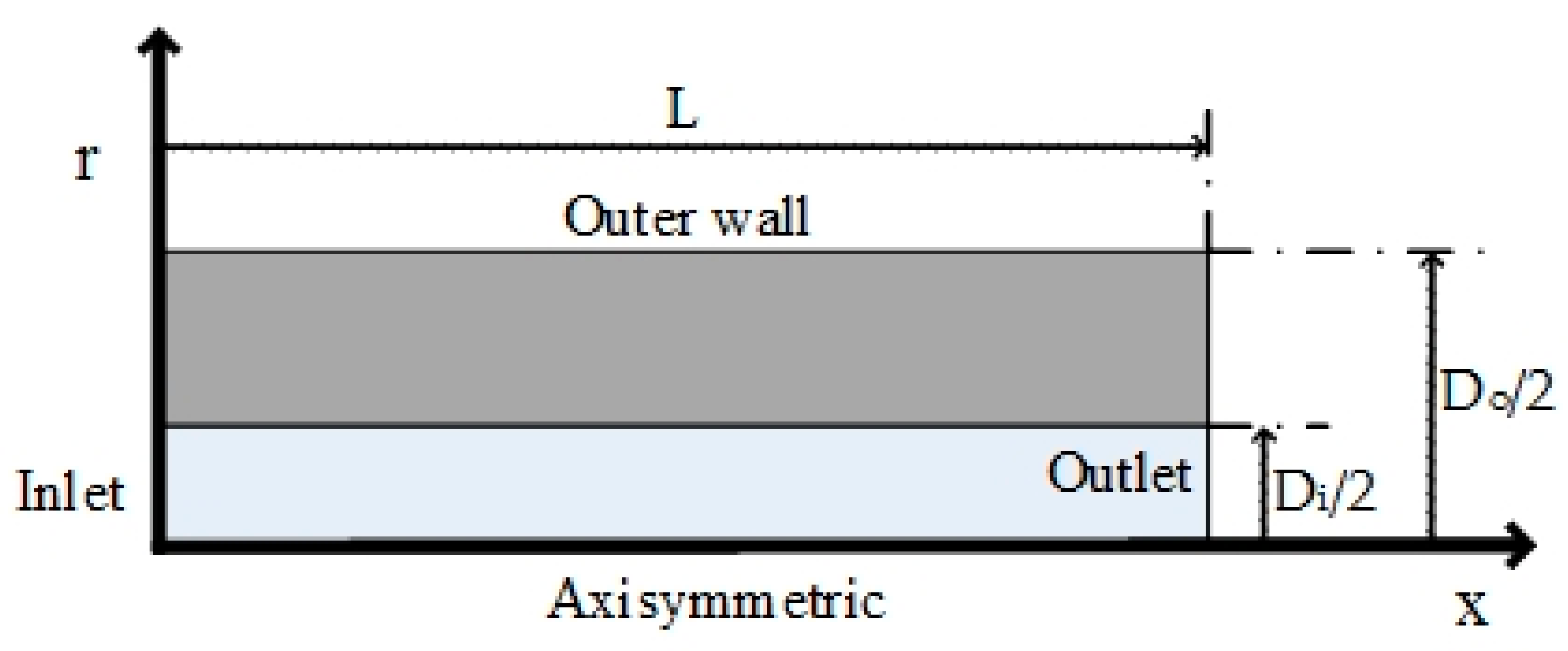
2. Numerical Model
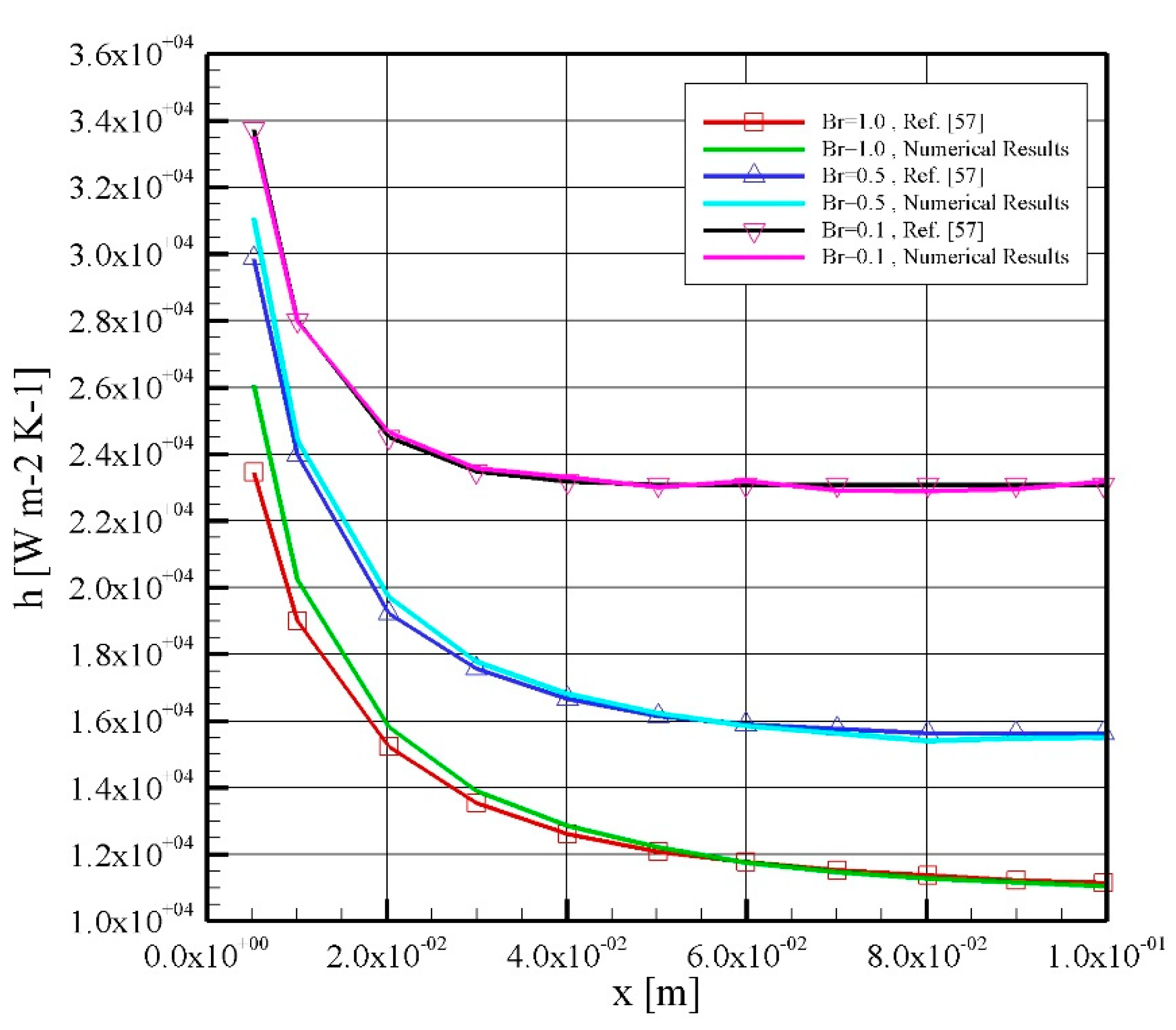
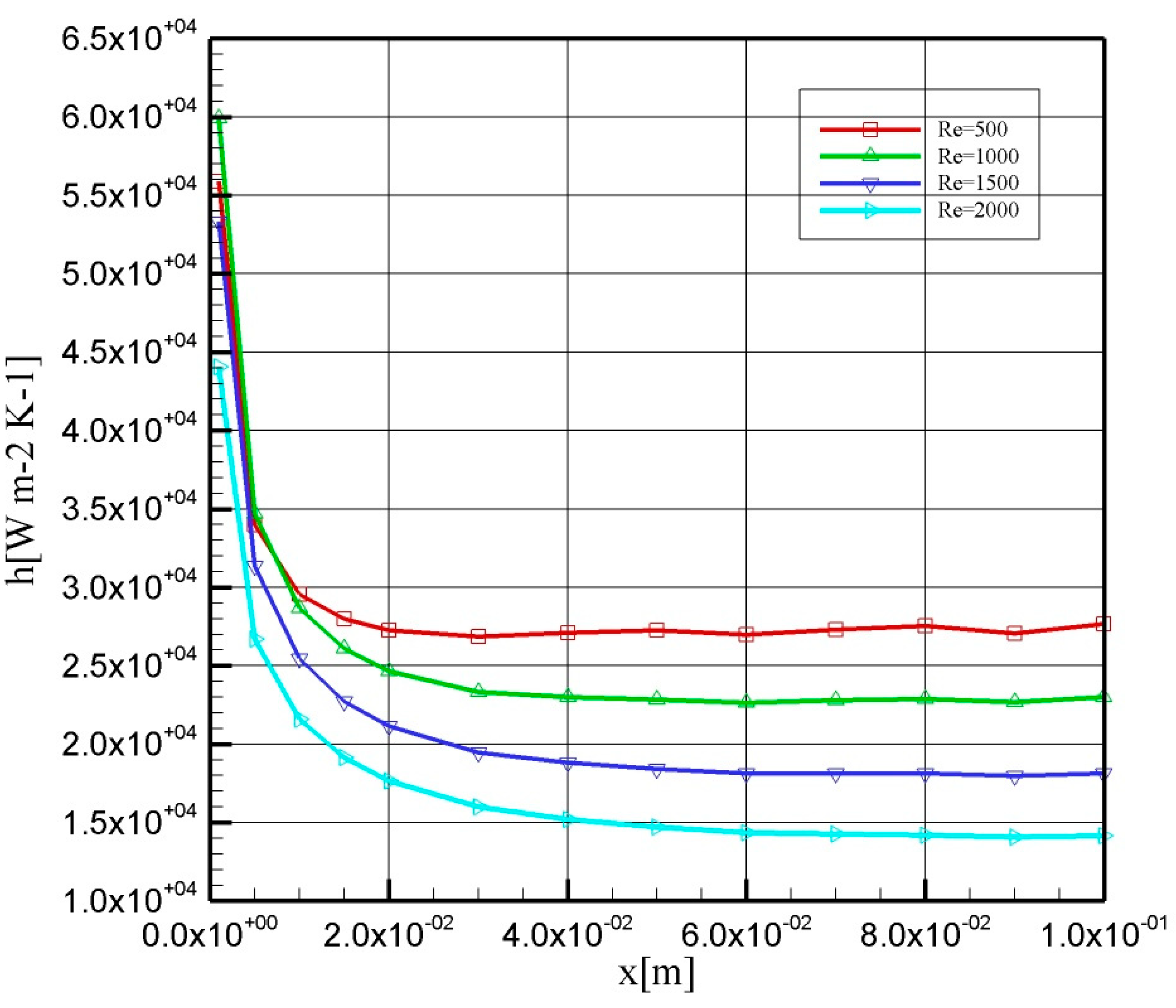
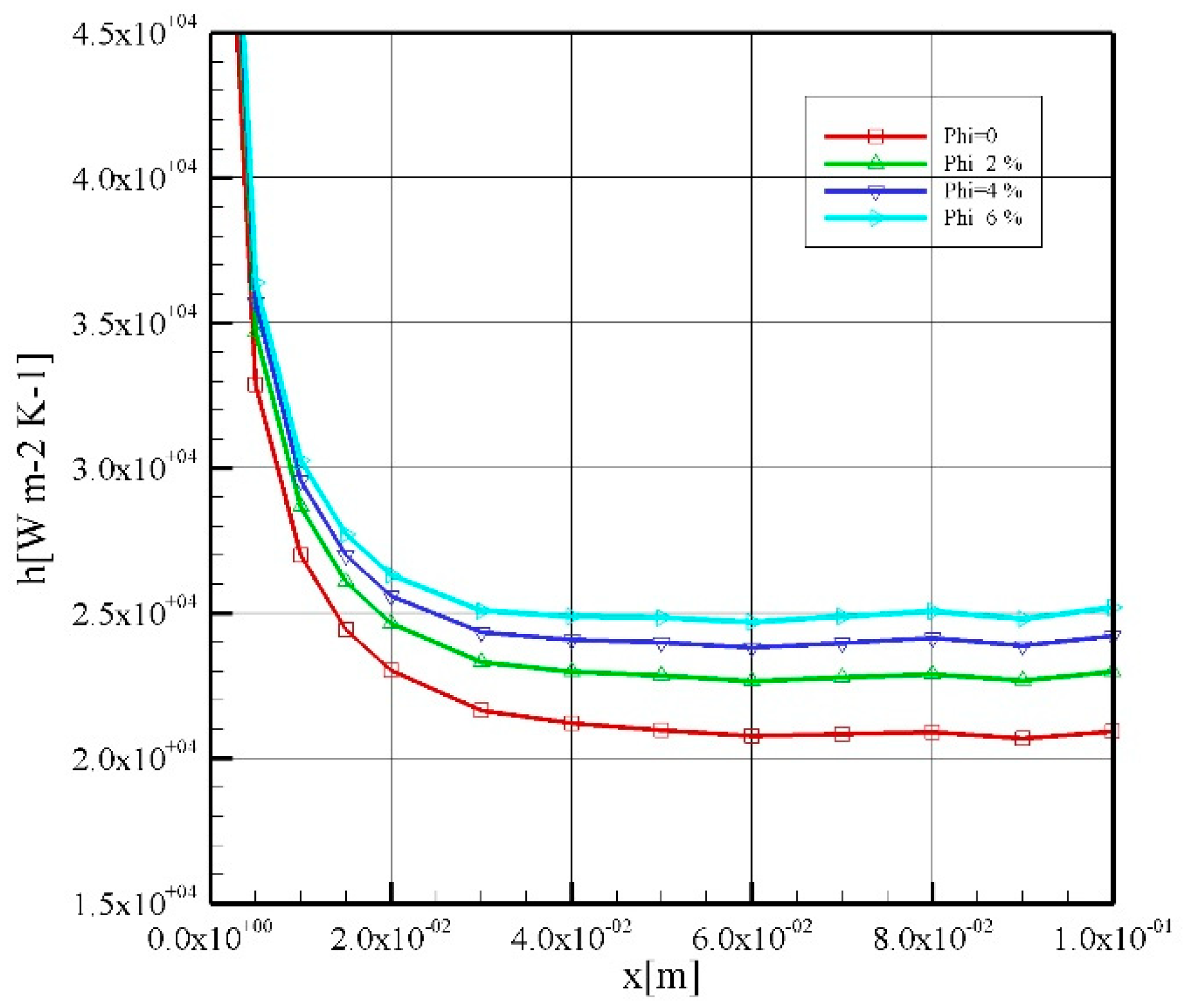
3. Results and Discussion
4. Conclusions
- Owing to the severe impact of viscous dissipation on the temperature of the wall and fluid, in contrast with the macro-scale problem, as the Reynolds number increases, the convective heat transfer coefficient reduces. Therefore, the viscous dissipation effect that is induced by shear stresses cannot be ignored in microchannels. Furthermore, PEC reduces as the Reynolds number increases, which is the result of higher pressure losses in high values of Reynolds numbers.
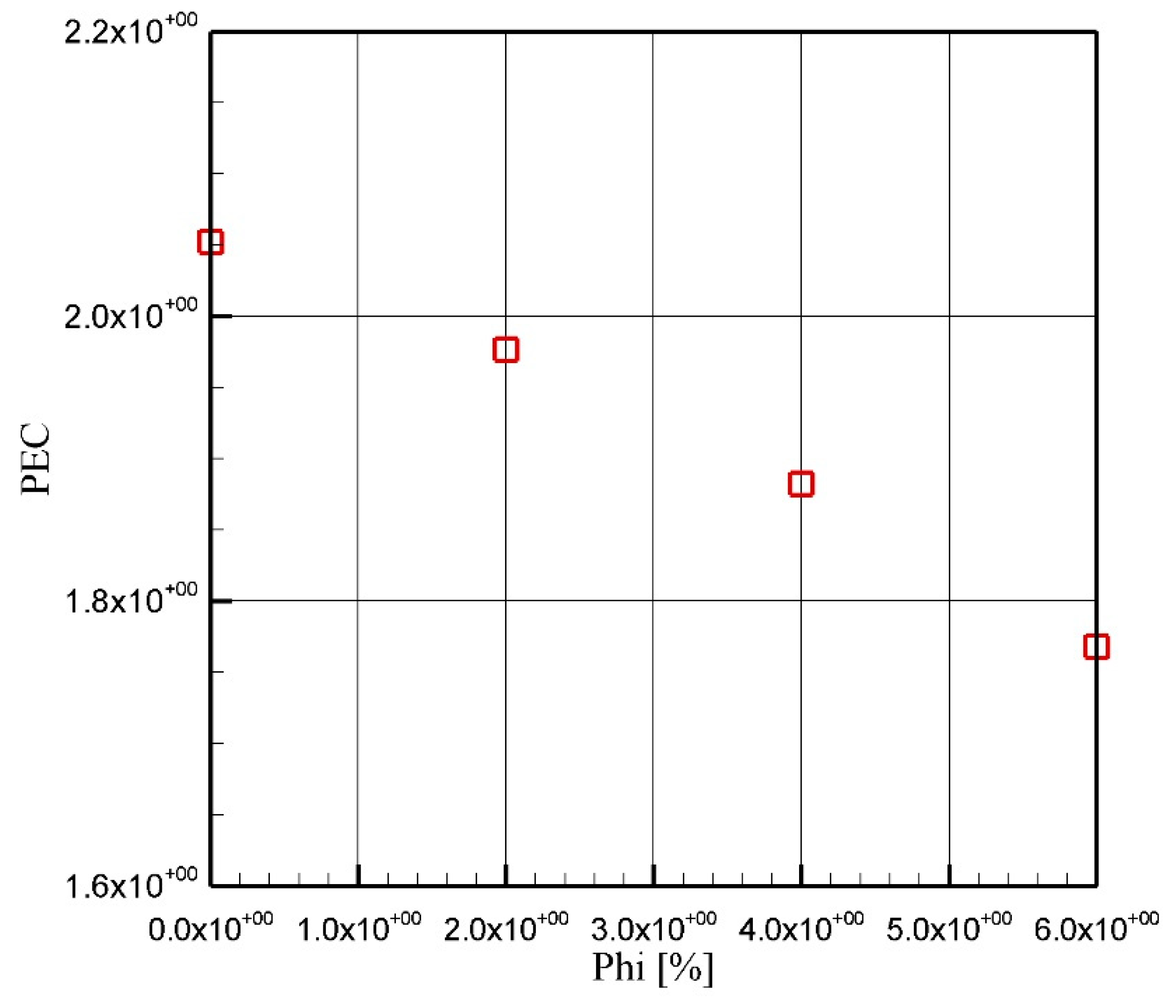
- Augmentation in the volume fraction of a nanoparticle can increase the heat transfer coefficient, which is in expense of a lower PEC, although the variation of the PEC is not great. The PEC reduces from 2.05 for pure water to 1.77 for a volume fraction of 6%.
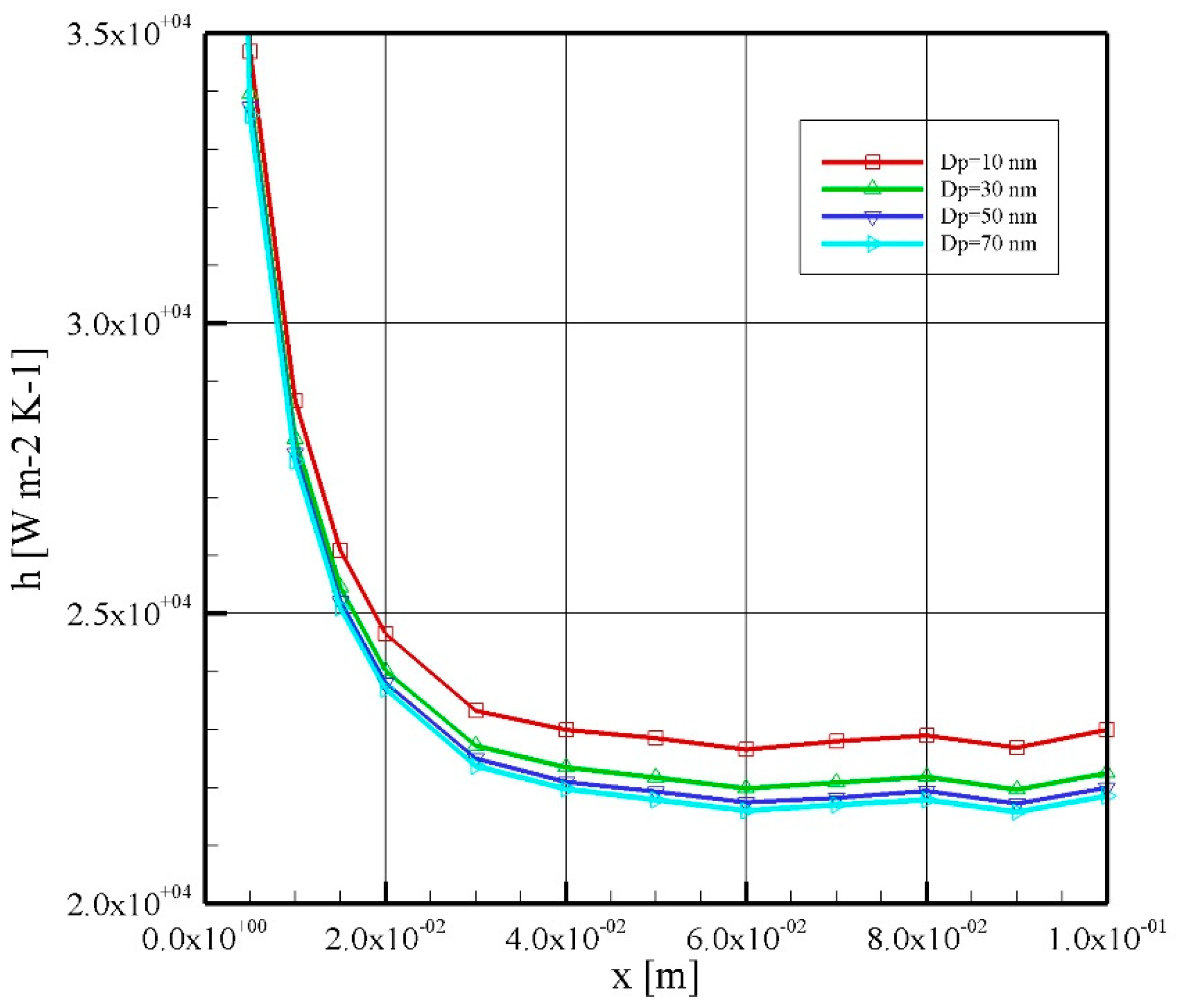
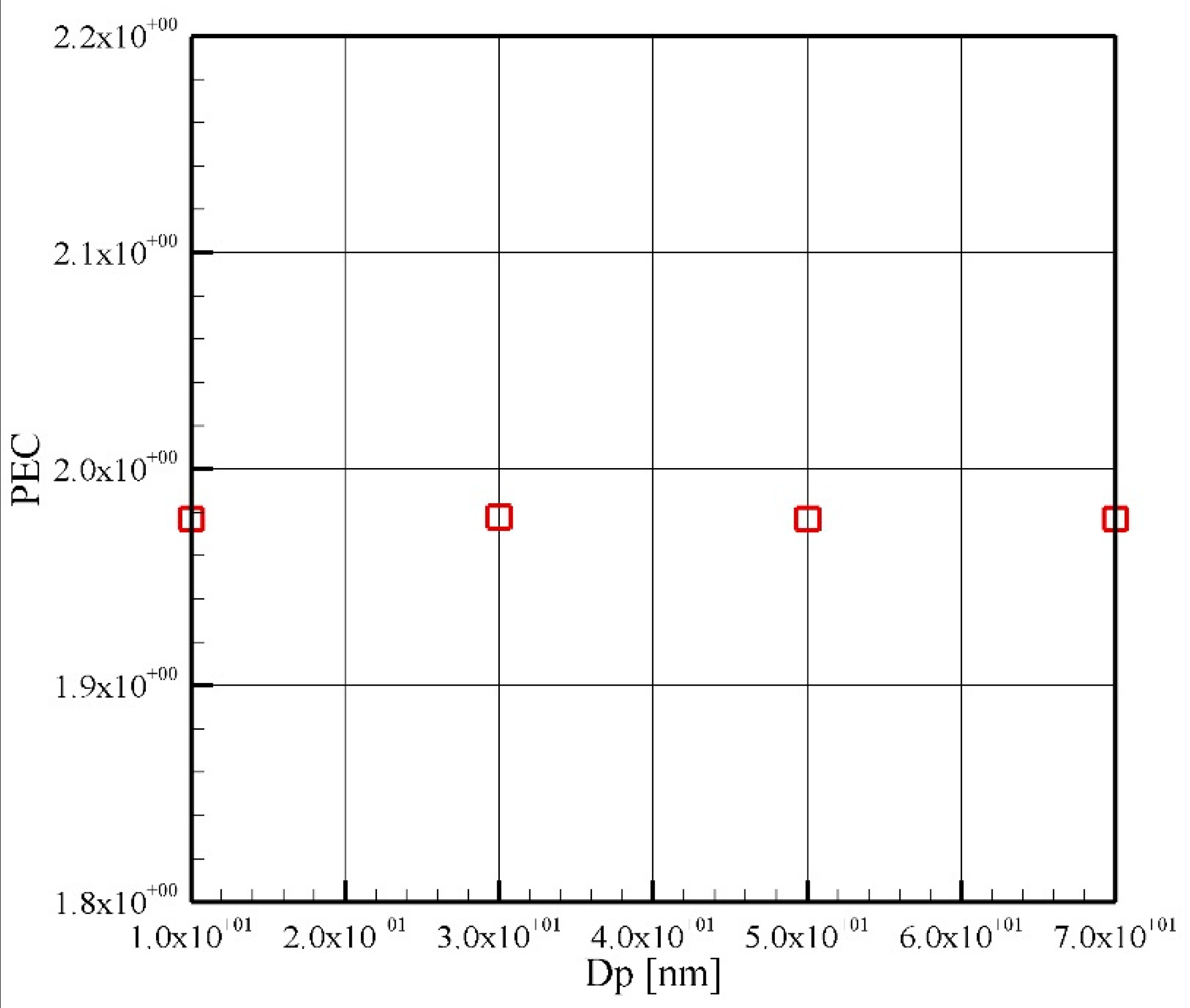
- Increasing the diameter of the nanoparticle can decrease heat transfer coefficient. Furthermore, it was observed that variations of the diameter of the nanoparticle have no effect on PEC for four different studied nanoparticle diameters and PEC equal to 1.98.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Br | Brinkman number |
| cp | Specific heat at constant pressure, J/kg K |
| D | Diameter, m |
| h | Heat transfer coefficient, W/m2 K |
| k | Thermal conductivity, W/m K |
| kb | Boltzmann constant, J/K |
| L | Length, m |
| lb | Mean free path, m |
| Mass flow rate, kg/s | |
| Pr | Prandtl number |
| q | Heat flux, W/m2 |
| Re | Reynolds number |
| r | Spatial coordinates in radial direction, m |
| T | Temperature, K |
| um | Mean entrance velocity of nanofluid, m/s |
| v | Velocity, m/s |
| Volumetric flow rate, m3/s | |
| x | Axial Direction, m |
| PEC | Performance Evaluation Criterion |
| Greek symbols | |
| Φ | Particle volume fraction,% |
| μ | Viscosity, Pa.s |
| ρ | Density, kg/m3 |
| Pumping power, W | |
| ΔP | Pressure drop, Pa |
| Subscripts | |
| b | Bulk |
| bf | Base fluid |
| eff | Effective |
| f | Fluid |
| i | Inner |
| o | Outer |
| p | Particle |
| s | Solid |
| w | Wall |
References
- Ramezanizadeh, M.; Alhuyi Nazari, M.; Ahmadi, M.H.; Lorenzini, G.; Pop, I. A review on the applications of intelligence methods in predicting thermal conductivity of nanofluids. J. Therm. Anal. Calorim. 2019, 138, 827–843. [Google Scholar] [CrossRef]
- Ramezanizadeh, M.; Ahmadi, M.H.; Nazari, M.A.; Sadeghzadeh, M.; Chen, L. A review on the utilized machine learning approaches for modeling the dynamic viscosity of nanofluids. Renew. Sustain. Energy Rev. 2019, 114, 109345. [Google Scholar] [CrossRef]
- Ramezanizadeh, M.; Alhuyi Nazari, M.; Hossein Ahmadi, M.; Chen, L. A review on the approaches applied for cooling fuel cells. Int. J. Heat Mass Transf. 2019, 139, 517–525. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Ramezanizadeh, M.; Nazari, M.A.; Lorenzini, G.; Kumar, R.; Jilte, R. Applications of nanofluids in geothermal: A review. Math. Model. Eng. Probl. 2018, 5, 281–285. [Google Scholar] [CrossRef]
- Abdollahzadeh Jamalabadi, M.; Ghasemi, M.; Alamian, R.; Wongwises, S.; Afrand, M.; Shadloo, M. Modeling of Subcooled Flow Boiling with Nanoparticles under the Influence of a Magnetic Field. Symmetry 2019, 11, 1275. [Google Scholar] [CrossRef]
- Abdollahzadeh Jamalabadi, M.Y.; Alamian, R.; Yan, W.-M.; Li, L.K.B.; Leveneur, S.; Safdari Shadloo, M. Effects of Nanoparticle Enhanced Lubricant Films in Thermal Design of Plain Journal Bearings at High Reynolds Numbers. Symmetry 2019, 11, 1353. [Google Scholar] [CrossRef]
- Karimipour, A.; D’Orazio, A.; Shadloo, M.S. The effects of different nano particles of Al2O3 and Ag on the MHD nano fluid flow and heat transfer in a microchannel including slip velocity and temperature jump. Phys. E Low-Dimens. Syst. Nanostruct. 2017, 86, 146–153. [Google Scholar] [CrossRef]
- Safaei, M.R.; Safdari Shadloo, M.; Goodarzi, M.S.; Hadjadj, A.; Goshayeshi, H.R.; Afrand, M.; Kazi, S.N. A survey on experimental and numerical studies of convection heat transfer of nanofluids inside closed conduits. Adv. Mech. Eng. 2016, 8, 168781401667356. [Google Scholar] [CrossRef]
- Tso, C.P.; Hor, C.H.; Chen, G.M.; Kok, C.K. Heat induction by viscous dissipation subjected to symmetric and asymmetric boundary conditions on a small oscillating flow in a microchannel. Symmetry 2018, 10, 499. [Google Scholar] [CrossRef]
- Li, Z.; He, Y.-L.; Tang, G.-H.; Tao, W.-Q. Experimental and numerical studies of liquid flow and heat transfer in microtubes. Int. J. Heat Mass Transf. 2007, 50, 3447–3460. [Google Scholar] [CrossRef]
- Lee, S.; Choi, S.U.-S.; Li, S.; Eastman, J.A. Measuring Thermal Conductivity of Fluids Containing Oxide Nanoparticles. J. Heat Transf. 1999, 121, 280. [Google Scholar] [CrossRef]
- Eastman, J.A.; Choi, S.U.S.; Li, S.; Yu, W.; Thompson, L.J. Anomalously increased effective thermal conductivities of ethylene glycol-based nanofluids containing copper nanoparticles. Appl. Phys. Lett. 2001, 78, 718–720. [Google Scholar] [CrossRef]
- Halelfadl, S.; Adham, A.M.; Mohd-Ghazali, N.; Maré, T.; Estellé, P.; Ahmad, R. Optimization of thermal performances and pressure drop of rectangular microchannel heat sink using aqueous carbon nanotubes based nanofluid. Appl. Therm. Eng. 2014, 62, 492–499. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Mirlohi, A.; Alhuyi Nazari, M.; Ghasempour, R. A review of thermal conductivity of various nanofluids. J. Mol. Liq. 2018, 265, 181–188. [Google Scholar] [CrossRef]
- Jalali, E.; Akbari, O.A.; Sarafraz, M.M.; Abbas, T.; Safaei, M.R. Heat transfer of oil/MWCNT nanofluid jet injection inside a rectangular microchannel. Symmetry 2019, 11, 757. [Google Scholar] [CrossRef]
- Safaei, M.R.; Togun, H.; Vafai, K.; Kazi, S.N.; Badarudin, A. Investigation of Heat Transfer Enhancement in a Forward-Facing Contracting Channel Using FMWCNT Nanofluids. Numer. Heat Transf. Part A Appl. 2014, 66, 1321–1340. [Google Scholar] [CrossRef]
- Behnampour, A.; Akbari, O.A.; Safaei, M.R.; Ghavami, M.; Marzban, A.; Sheikh Shabani, G.A.; Zarringhalam, M.; Mashayekhi, R. Analysis of heat transfer and nanofluid fluid flow in microchannels with trapezoidal, rectangular and triangular shaped ribs. Phys. E Low-Dimens. Syst. Nanostruct. 2017, 91, 15–31. [Google Scholar] [CrossRef]
- Tian, C.; Maleki, A.; Motie, S.; Yavarinasab, A.; Afrand, M. Generation expansion planning by considering wind resource in a competitive environment. J. Therm. Anal. Calorim. 2019. [Google Scholar] [CrossRef]
- Zhang, W.; Maleki, A.; Rosen, M.A. A heuristic-based approach for optimizing a small independent solar and wind hybrid power scheme incorporating load forecasting. J. Clean. Prod. 2019, 241, 117920. [Google Scholar] [CrossRef]
- Motie, S.; Keynia, F.; Ranjbar, M.R.; Maleki, A. Generation expansion planning by considering energy-efficiency programs in a competitive environment. Int. J. Electr. Power Energy Syst. 2016, 80, 109–118. [Google Scholar] [CrossRef]
- Li, J.; Mohammadi, A.; Maleki, A. Techno-economic analysis of new integrated system of humid air turbine, organic Rankine cycle, and parabolic trough collector. J. Therm. Anal. Calorim. 2019. [Google Scholar] [CrossRef]
- Maleki, A.; Rosen, M.A. Design of a cost-effective on-grid hybrid wind–hydrogen based CHP system using a modified heuristic approach. Int. J. Hydrogen Energy 2017, 42, 15973–15989. [Google Scholar] [CrossRef]
- Celata, G.; Cumo, M.; Marconi, V.; McPhail, S.J.; Zummo, G. Microtube liquid single-phase heat transfer in laminar flow. Int. J. Heat Mass Transf. 2006, 49, 3538–3546. [Google Scholar] [CrossRef]
- Bergman, T.L.; Incropera, F.P. Fundamentals of Heat and Mass Transfer; Wiley: Hoboken, NJ, USA, 2011; ISBN 9780470501979. [Google Scholar]
- Mital, M. Analytical analysis of heat transfer and pumping power of laminar nanofluid developing flow in microchannels. Appl. Therm. Eng. 2013, 50, 429–436. [Google Scholar] [CrossRef]
- Faghri, M.; Sparrow, E.M. Simultaneous Wall and Fluid Axial Conduction in Laminar Pipe-Flow Heat Transfer. J. Heat Transf. 1980, 102, 58–63. [Google Scholar] [CrossRef]
- Nonino, C.; Savino, S.; Del Giudice, S.; Mansutti, L. Conjugate forced convection and heat conduction in circular microchannels. Int. J. Heat Fluid Flow 2009, 30, 823–830. [Google Scholar] [CrossRef]
- El Bécaye Maïga, S.; Tam Nguyen, C.; Galanis, N.; Roy, G.; Maré, T.; Coqueux, M. Heat transfer enhancement in turbulent tube flow using Al2O3 nanoparticle suspension. Int. J. Numer. Methods Heat Fluid Flow 2006, 16, 275–292. [Google Scholar] [CrossRef]
- Akbari, M.; Behzadmehr, A. Developing mixed convection of a nanofluid in a horizontal tube with uniform heat flux. Int. J. Numer. Methods Heat Fluid Flow 2007, 17, 566–586. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Alhuyi Nazari, M.; Ghasempour, R.; Madah, H.; Shafii, M.B.; Ahmadi, M.A. Thermal conductivity ratio prediction of Al2O3/water nanofluid by applying connectionist methods. Colloids Surf. A Physicochem. Eng. Asp. 2018, 541, 154–164. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Ahmadi, M.A.; Nazari, M.A.; Mahian, O.; Ghasempour, R. A proposed model to predict thermal conductivity ratio of Al2O3/EG nanofluid by applying least squares support vector machine (LSSVM) and genetic algorithm as a connectionist approach. J. Therm. Anal. Calorim. 2018. [Google Scholar] [CrossRef]
- Ramezanizadeh, M.; Ahmadi, M.A.; Ahmadi, M.H.; Alhuyi Nazari, M. Rigorous smart model for predicting dynamic viscosity of Al2O3/water nanofluid. J. Therm. Anal. Calorim. 2019, 137, 307–316. [Google Scholar] [CrossRef]
- Ramezanizadeh, M.; Alhuyi Nazari, M. Modeling thermal conductivity of Ag/water nanofluid by applying a mathematical correlation and artificial neural network. Int. J. Low-Carbon Technol. 2019, 14, 468–474. [Google Scholar] [CrossRef]
- Hemmat Esfe, M.; Naderi, A.; Akbari, M.; Afrand, M.; Karimipour, A. Evaluation of thermal conductivity of COOH-functionalized MWCNTs/water via temperature and solid volume fraction by using experimental data and ANN methods. J. Therm. Anal. Calorim. 2015, 121, 1273–1278. [Google Scholar] [CrossRef]
- Komeilibirjandi, A.; Raffiee, A.H.; Maleki, A.; Alhuyi Nazari, M.; Safdari Shadloo, M. Thermal conductivity prediction of nanofluids containing CuO nanoparticles by using correlation and artificial neural network. J. Therm. Anal. Calorim. 2019. [Google Scholar] [CrossRef]
- Bagherzadeh, S.A.; Sulgani, M.T.; Nikkhah, V.; Bahrami, M.; Karimipour, A.; Jiang, Y. Minimize pressure drop and maximize heat transfer coefficient by the new proposed multi-objective optimization/statistical model composed of “ANN + Genetic Algorithm” based on empirical data of CuO/paraffin nanofluid in a pipe. Phys. A Stat. Mech. Its Appl. 2019, 527, 121056. [Google Scholar] [CrossRef]
- Hemmat Esfe, M.; Wongwises, S.; Naderi, A.; Asadi, A.; Safaei, M.R.; Rostamian, H.; Dahari, M.; Karimipour, A. Thermal conductivity of Cu/TiO2–water/EG hybrid nanofluid: Experimental data and modeling using artificial neural network and correlation. Int. Commun. Heat Mass Transf. 2015, 66, 100–104. [Google Scholar] [CrossRef]
- Koo, J.; Kleinstreuer, C. A new thermal conductivity model for nanofluids. J. Nanopart. Res. 2004, 6, 577–588. [Google Scholar] [CrossRef]
- Chon, C.H.; Kihm, K.D.; Lee, S.P.; Choi, S.U.S. Empirical correlation finding the role of temperature and particle size for nanofluid (Al2O3) thermal conductivity enhancement. Appl. Phys. Lett. 2005, 87, 153107. [Google Scholar] [CrossRef]
- Ben Mansour, R.; Galanis, N.; Nguyen, C.T. Effect of uncertainties in physical properties on forced convection heat transfer with nanofluids. Appl. Therm. Eng. 2007, 27, 240–249. [Google Scholar] [CrossRef]
- Li, J.; Peterson, G.; Cheng, P. Three-dimensional analysis of heat transfer in a micro-heat sink with single phase flow. Int. J. Heat Mass Transf. 2004, 47, 4215–4231. [Google Scholar] [CrossRef]
- Lelea, D. Effects of temperature dependent thermal conductivity on Nu number behavior in micro-tubes. Int. Commun. Heat Mass Transf. 2010, 37, 245–249. [Google Scholar] [CrossRef]
- Tso, C.P.; Mahulikar, S.P. Experimental verification of the role of Brinkman number in microchannels using local parameters. Int. J. Heat Mass Transf. 2000, 43, 1837–1849. [Google Scholar] [CrossRef]
- Tso, C.P.; Mahulikar, S.P. The role of the Brinkman number in analysing flow transitions in microchannels. Int. J. Heat Mass Transf. 1999, 42, 1813–1833. [Google Scholar] [CrossRef]
- Tso, C.P.; Mahulikar, S.P. The use of the Brinkman number for single phase forced convective heat transfer in microchannels. Int. J. Heat Mass Transf. 1998, 41, 1759–1769. [Google Scholar] [CrossRef]
- Morini, G.L. Scaling Effects for Liquid Flows in Microchannels. Heat Transf. Eng. 2006, 27, 64–73. [Google Scholar] [CrossRef]
- Safaei, M.R.; Mahian, O.; Garoosi, F.; Hooman, K.; Karimipour, A.; Kazi, S.N.; Gharehkhani, S. Investigation of micro- and nanosized particle erosion in a 90° pipe bend using a two-phase discrete phase model. Sci. World J. 2014, 2014, 740578. [Google Scholar] [CrossRef]
- Lin, C. Application of the Symmetric Model to the Design Optimization of Fan Outlet Grills. Symmetry 2019, 11, 959. [Google Scholar] [CrossRef]
- Chen, C.-W.; Lu, Y.-F. Computational Fluid Dynamics Study of Water Entry Impact Forces of an Airborne-Launched, Axisymmetric, Disk-Type Autonomous Underwater Hovering Vehicle. Symmetry 2019, 11, 1100. [Google Scholar] [CrossRef]
- Qin, Y. Pavement surface maximum temperature increases linearly with solar absorption and reciprocal thermal inertial. Int. J. Heat Mass Transf. 2016, 97, 391–399. [Google Scholar] [CrossRef]
- Qin, Y.; Tan, K.; Meng, D.; Li, F. Theory and procedure for measuring the solar reflectance of urban prototypes. Energy Build. 2016, 126, 44–50. [Google Scholar] [CrossRef]
- Versteeg, H.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method; Prentice Hall: Upper Saddle River, NJ, USA, 2007. [Google Scholar]
- Patankar, S. Numerical Heat Transfer and Fluid Flow; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Perry, R.H.; Green, D.W. Perry’s Chemical Engineers’ Handbook; McGraw-Hill: New York, NY, USA, 2008; ISBN 9780071422949. [Google Scholar]
- Batchelor, G.K. The effect of Brownian motion on the bulk stress in a suspension of spherical particles. J. Fluid Mech. 1977, 83, 97–117. [Google Scholar] [CrossRef]
- Roy, G.; Gherasim, I.; Nadeau, F.; Poitras, G.; Nguyen, C.T. Heat transfer performance and hydrodynamic behavior of turbulent nanofluid radial flows. Int. J. Therm. Sci. 2012, 58, 120–129. [Google Scholar] [CrossRef]
- Lelea, D.; Nisulescu, C. The micro-tube heat transfer and fluid flow of water based Al2O3 nanofluid with viscous dissipation. Int. Commun. Heat Mass Transf. 2011, 38, 704–710. [Google Scholar] [CrossRef]
- Jung, J.Y.; Oh, H.S.; Kwak, H.Y. Forced convective heat transfer of nanofluids in microchannels. Int. J. Heat Mass Transf. 2009, 52, 466–472. [Google Scholar] [CrossRef]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Irandoost Shahrestani, M.; Maleki, A.; Safdari Shadloo, M.; Tlili, I. Numerical Investigation of Forced Convective Heat Transfer and Performance Evaluation Criterion of Al2O3/Water Nanofluid Flow inside an Axisymmetric Microchannel. Symmetry 2020, 12, 120. https://doi.org/10.3390/sym12010120
Irandoost Shahrestani M, Maleki A, Safdari Shadloo M, Tlili I. Numerical Investigation of Forced Convective Heat Transfer and Performance Evaluation Criterion of Al2O3/Water Nanofluid Flow inside an Axisymmetric Microchannel. Symmetry. 2020; 12(1):120. https://doi.org/10.3390/sym12010120
Chicago/Turabian StyleIrandoost Shahrestani, Misagh, Akbar Maleki, Mostafa Safdari Shadloo, and Iskander Tlili. 2020. "Numerical Investigation of Forced Convective Heat Transfer and Performance Evaluation Criterion of Al2O3/Water Nanofluid Flow inside an Axisymmetric Microchannel" Symmetry 12, no. 1: 120. https://doi.org/10.3390/sym12010120
APA StyleIrandoost Shahrestani, M., Maleki, A., Safdari Shadloo, M., & Tlili, I. (2020). Numerical Investigation of Forced Convective Heat Transfer and Performance Evaluation Criterion of Al2O3/Water Nanofluid Flow inside an Axisymmetric Microchannel. Symmetry, 12(1), 120. https://doi.org/10.3390/sym12010120







