A New Method for MAGDM Based on Improved TOPSIS and a Novel Pythagorean Fuzzy Soft Entropy

Abstract

A decision-making environment is full of uncertainty and complexity. Existing tools include fuzzy sets, soft sets, intuitionistic fuzzy sets, Pythagorean fuzzy sets (PFSs) and so on. Compared with intuitionistic fuzzy sets (IFSs), PFSs proposed by Yager have advantages in handling vagueness in the real world and possess good symmetry. The entropy measure is the most widespread form of uncertainty measure. In this paper, we improve the technique for order preference by similarity to an ideal solution (TOPSIS) method to better deal with multiple-attribute group decision making (MAGDM) problems based on Pythagorean fuzzy soft sets (PFSSs). To better determine the weights of attributes, we firstly define a novel Pythagorean fuzzy soft entropy which is more reasonable and valid. Meanwhile the entropy has good symmetry. Entropy for PFSSs which is used to determine the subjective weights of attributes is also defined. Then we introduce a measure to calculate integrated weights by combining objective weights and subjective weights. Based on the integrated weights, the TOPSIS method is generalized and improved to solve the MAGDM problem. A distance measure taking into account the characteristics of Pythagorean fuzzy numbers (PFNs) is used to calculate distance between alternatives and ideal solutions. Finally, the proposed MAGDM method is applied in the case of selecting a missile position. Compared with other methods, it is shown that the proposed method can rank alternatives more reasonably and have higher distinguishability.

1. Introduction

Decision making is a common problem which occurs in almost every field. But the environment of decision making is full of uncertainty and complexity. Zadeh [1] first proposed the very influential theory of fuzzy sets in 1965. The theory breaks through the traditional cantor set’s limits by assigning each element a value between 0 and 1 as a single membership [2]. Intuitionistic fuzzy sets [3] was firstly proposed by Atanassov in 1986, which is an extension of Zadeh’s fuzzy sets. An intuitionistic fuzzy set is distinguished from a fuzzy set by adding a hesitance index. It has three parameters which are membership function, non-membership function and hesitance index (intuitionistic fuzzy index). These three parameters can respectively be used to describe the states of support, opposite, and neutrality in human cognition [4,5]. Soft set theory [6], introduced by Molodtsov from a parametrization perspective in 1999, has been considered as a valid tool for modeling uncertainties [7]. In some senses, fuzzy sets can be considered as a special case of Molodtsov’s soft sets. Yager [8,9] proposed Pythagorean fuzzy set recently.

Pythagorean fuzzy sets (PFSs) theory is a generalization of the intuitionistic fuzzy sets theory and has good symmetry. It allows the sum of the membership degree and non-membership degree to be larger than one but restrict that their square sum is equal to or less than one. The ability of PFSs to model such uncertainty of decision-making is much stronger than intuitionistic fuzzy sets. So PFSs theory is a more powerful tool for expressing uncertain information when making decisions. It is characterized by four parameters which are membership degree, non-membership degree, strength of commitment about membership, and direction of commitment [10]. Li [10] also proposed some novel distance measures for PFSs and Pythagorean fuzzy numbers (PFNs).

Multiple-attribute group decision making (MAGDM) aims to determine an optimal alternative from a set of feasible alternatives [11]. Multiple attributes and a group of people are the problems’ characteristics. MAGDM problems occur frequently in the real world. The process of MAGDM is full of fuzziness and uncertainty.

C.L. Hwang and K. Yoon firstly introduced technique for order preference by similarity to an ideal solution (TOPSIS) [12] method in 1981. This method effectively solves the problem of ranking alternatives. Then, the fuzzy set theory and TOPSIS method are often combined to deal with multiple criteria decision-making (MCDM) problems. Chen [13] solved supplier selection problem by using linguistic values and fuzzy TOPSIS method. Pawel Ziemba [14] applied the multi-criteria decision analysis (MCDA) method and fuzzy TOPSIS method to solve online comparison problems with uncertain and certain criteria. Intuitionistic fuzzy sets theory also has a good combination with the TOPSIS method. Chen [15] proposed a multiple attributes decision making method based on the TOPSIS method and the similarity measures between intuitionistic fuzzy sets. P. Muthukumara [16] proposed a novel similarity measure and a new weighted similarity measure on intuitionistic fuzzy soft sets (IFSSs).

Entropy measure [17,18] and its complementary concept knowledge measure [19] are effective ways to determine the weights vector of attributes when making decisions. Rodger considered the decision-maker’s intrinsic state and solved the decision-making problem by entropy principles [20]. Harish [21] proposed intuitionistic fuzzy entropy-based method to deal with MCDM problems with unknown criteria weights. Szmidt’s intuitionistic fuzzy entropy [22] measure is widely used in related studies. But it has some flaws.

Pythagorean fuzzy sets are also developed to solve multiple attributes decision-making (MADM) problems [23]. To fuse information, Li [24] proposed Pythagorean fuzzy Hamy mean (PFHM) operator, weighted Pythagorean fuzzy dual Hamy mean (WPFDHM) operator and so on to deal with MAGDM problems. Xue [11] solved a railway project investment decision-making problem by Pythagorean fuzzy LINMAP method based on the entropy theory. But their entropy definition does not accord with reality and fails to describe the maximum degree of fuzziness in PFSs objectively. Zhang [25] extended TOPSIS to multiple-attributes decision making with Pythagorean fuzzy sets. However, their weight vector of the attributes is directly given by the committee and the distance between two PFNs defined by them is also directly an extension of the distance between intuitionistic fuzzy numbers. Their distance measure considered the difference between the membership degrees, the non-membership degrees, and the degrees of hesitancy, but ignores the influence of the directions of Pythagorean fuzzy numbers. This may lead to unreasonable results in some cases [10]. Moreover, to our knowledge, there is little work on Pythagorean fuzzy soft sets.

To settle the problem of MAGDM and enrich the study of PFSSs, following the pioneering studies of the above people, we redefine entropy for Pythagorean fuzzy soft sets, introduce a novel Pythagorean fuzzy entropy and extend it to Pythagorean fuzzy soft entropy. Based on that, we then propose a new method for MAGDM based on improved TOPSIS and a novel PFS entropy. When calculating the distance between PFNs, we use the distance measure proposed by Li [10] to avoid unreasonable results sometimes.

The rest of this article is organized as follows. In Section 2, we recall some basic definitions and formulas of IFSs, PFSs, soft sets etc. In Section 3, we analyze some definitions of Pythagorean fuzzy entropy and related measures, and point out their flaws. Based on that, we introduce a novel Pythagorean fuzzy entropy definition and propose new entropy measures for Pythagorean fuzzy sets and Pythagorean fuzzy soft sets. In Section 4, we introduce a measure calculating objective attribute weights based on our entropy measure. Then, we introduce a measure calculating integrated weights which combines objective weights and subjective weights of attributes. In Section 5, we explain our MAGDM method based on the novel Pythagorean fuzzy soft entropy step by step. In Section 6, the proposed multiple attributes group decision-making method based on PFSSs is applied in the case of selecting missile position as the proposed method’s illustrative example. The results show the effectiveness of the method. In the last Section, conclusions are given.

2. Preliminaries

Zadeh firstly introduced the concept of fuzzy set in 1965. It was defined as follows.

Definition 1.

Let$X$be a universe ofdiscourse, A fuzzy set$A$in the$X$is an object having the form [1]

$$A=\{\langle x,{\mu }_A(x)\rangle |x\in X\}$$

(1)

where ${\mu }_A(x):X\to [0,1]$ is the degree of membership for x with respect to A.

Definition 2.

An Atanassov’s intuitionistic fuzzy set A over the universe$U$can be defined as follows:$A=\{\left(x,{\mu }_A(x),{\nu }_A(x)\right):x\in U\}$, where${\mu }_A(x)$:$U\to \left[0,1\right],$${\nu }_A(x)$:$U\to \left[0,1\right]$with the property$0\le$${\mu }_A(x)+{\nu }_A(x)$$\le 1,\forall x\in U$. Among of them, the values${\mu }_A(x)$and${\nu }_A(x)$respectively represent the degree of membership and the non-membership of$x$to$A$. ${\pi }_A(x)=1-({\mu }_A(x)+{\nu }_A(x))$is called the intuitionistic fuzzy index or hesitance index [3,26].

Definition 3.

(Soft set) A pair$\left(F,E\right)$is called a soft set(over$U$) if and only if F is a mapping of E into the set of all subsets of the set$U$ [6].

Definition 4.

(Fuzzy soft sets) Let$U$be an initial universe set and E be the set of parameters. Let$F\left(U\right)$denotes the fuzzy power set of the initial universe set$U$. Let$A\subset E$. A pair$\left(F,A\right)$is called a soft set over$U$, where$F$is a mapping given by$F:A\to F\left(U\right)$ [27].

Definition 5.

(Pythagorean fuzzy set [8,9]) Let $X$ be a universe of discourse, a Pythagorean fuzzy set in $X$ can be given by

$$P=\{\langle x,{\mu }_p(x),{\nu }_P(x)\rangle |x\in X\}$$

(2)

where ${\mu }_p:X\to [0,1]$ represents the degree of membership and ${\nu }_p:X\to [0,1]$ represents the degree of non-membership of the element $x\in X$ to the Pythagorean fuzzy set $P$, respectively, with the condition that $0\le {({\mu }_p(x))}^2+{({\nu }_p(x))}^2\le 1$. The degree of hesitancy ${\pi }_p(x)=\sqrt{1-{({\mu }_p(x))}^2-{({\nu }_p(x))}^2}$.

Definition 6.

(Intuitionistic fuzzy soft sets.) Let$U$be an initial universe set and$E$be a set of parameters. Let$I{F}^U$represent the collection of all intuitionistic fuzzy subsets of$U$. Let$A\subset E$. A pair$\left(F,A\right)$is called intuitionistic fuzzy soft sets (IFSSs) over$U$, where$F$is a mapping given by$F$:$A\to I{F}^U$ [28].

Then, we can easily expand intuitionistic fuzzy soft sets to Pythagorean fuzzy soft sets.

(Pythagorean fuzzy soft sets [28].) Let $U$ be an initial universe set and $E$ be a set of parameters. Let $P{F}^U$ represent the collection of all Pythagorean fuzzy subsets of $U$. Let $A\subset E$. A pair $\left(F,A\right)$ is called Pythagorean fuzzy soft sets (PFSSs) over $U$, where $F$ is a mapping given by $F$: $A\to P{F}^U$.

Definition 7.

(Entropy on Pythagorean Fuzzy Soft Sets [29].) Let $U=\{x_1,x_2,\cdots ,x_m\}$ be a universe of discourse. Let $E=\{e_1,e_2,\cdots e_n\}$ be a set of parameters. $PF\left(U\right)$ means the set of all Pythagorean fuzzy soft set over $U$. Let $A\subseteq E$. A pair $\left(F,A\right)$ is a Pythagorean fuzzy soft set over the universe of discourse $U$, where $F$ is a mapping given by $F:A\to PF\left(U\right)$.

Definition 8.

The operations on PFNs defined by Zhang and Xu [25] are shown as below:

$$\begin{array}{l}\left(1\right){\beta }^{\mathrm{c}}=P({\nu }_{\beta },{\mu }_{\beta });\\ \left(2\right){\beta }_1\oplus {\beta }_2=P\left(\sqrt{{\mu }_{{\beta }_1}^2+{\mu }_{{\beta }_2}^2-{\mu }_{{\beta }_1}^2{\mu }_{{\beta }_2}^2},{\nu }_{{\beta }_1}{\nu }_{{\beta }_2}\right);\\ \left(3\right){\beta }_1\otimes {\beta }_2=P\left({\mu }_{{\beta }_1}{\mu }_{{\beta }_2},\sqrt{{\nu }_{{\beta }_1}^2+{\nu }_{{\beta }_2}^2-{\nu }_{{\beta }_1}^2{\nu }_{{\beta }_2}^2}\right);\\ \left(4\right)\lambda \beta =P(\sqrt{1-{(1-{\mu }_{\beta }^2)}^{\lambda }},{({\nu }_{\beta })}^{\lambda }),\lambda >0;\\ \left(5\right){\beta }^{\lambda }=P({({\mu }_{\beta })}^{\lambda },\sqrt{1-{(1-{\nu }_{\beta }^2)}^{\lambda }}),\lambda >0.\end{array}$$

Definition 9.

(Zhang and Xu’s distance between PFSs [25].) Inspired by the distance between IFSs, Zhang and Xu calculate the distance between Pythagorean fuzzy sets as follows:

$$d(p(x_i),p(x_j))=\frac{1}{2}\left(\left|{\mu }_p{(x_i)}^2-{\mu }_p{(x_j)}^2\right|+\left|{\nu }_p{(x_i)}^2-{\nu }_p{(x_j)}^2\right|+\left|{\pi }_p{(x_i)}^2-{\pi }_p{(x_j)}^2\right|\right)$$

(3)

Definition 10.

(Li’s distance between PFSs [10].) Li and Zeng [10] take into account the characteristics of PFNs parameters and then systematically proposed a series of distance measures of PFNs and PFSs.

Let$p_1$and$p_2$be two PFNs, Li [10] define the normalized generalized distance between them as follows:

$$D_G\left(P_1,P_2\right)=[\frac{1}{4n}{\displaystyle \sum _{i=1}^n({\left|{\mu }_{p_1}\left(x_i\right)-{\mu }_{p_2}\left(x_i\right)\right|}^{\lambda }+{\left|{\nu }_{p_1}\left(x_i\right)-{\nu }_{p_2}\left(x_i\right)\right|}^{\lambda }}+{\left|r_{p_1}\left(x_i\right)-r_{p_2}\left(x_i\right)\right|}^{\lambda }+{\left|d_{p_1}\left(x_i\right)-d_{p_2}\left(x_i\right)\right|}^{\lambda }){]}^{1/\lambda }$$

(4)

where $\lambda \ge 1$.

If decision makers have different preferences on the four parameters, the weighted distance measure between them can be defined as follows [10]:

$$\begin{array}{l}{D}_{\omega G}\left(P_1,P_2\right)=[{\displaystyle \sum _{i=1}^n({\omega }_1{\left|{\mu }_{p_1}\left(x_i\right)-{\mu }_{p_2}\left(x_i\right)\right|}^{\lambda }}+{\omega }_2{\left|{\nu }_{p_1}\left(x_i\right)-{\nu }_{p_2}\left(x_i\right)\right|}^{\lambda }\\ +{\omega }_3{\left|r_{p_1}\left(x_i\right)-r_{p_2}\left(x_i\right)\right|}^{\lambda }+{\omega }_4{\left|d_{p_1}\left(x_i\right)-d_{p_2}\left(x_i\right)\right|}^{\lambda }){]}^{1/\lambda }\end{array}$$

(5)

Definition 11.

For the purpose of comparing the PFNs, Zhang and Xu [25] proposed a score function of the PFN. Let $\beta =P\left({\mu }_{\beta },{\nu }_{\beta }\right)$ be a PFN. The score function of $\beta$ is defined as:

$$s(\beta )={({\mu }_{\beta })}^2-{({\nu }_{\beta })}^2$$

(6)

The higher the score, the larger the PFN.

Definition 12.

Yager [9] also gave a way to compare Pythagorean membership grade. He utilized the characteristic of polar coordinates and put forward two new parameters to represent PFN, $p=\left(r_p,d_p\right)$. $r_p$ is called the strength of $p$ and $d_p$ is called the direction of the $r_p$. The relationships between the four parameters ${\mu }_p,{\nu }_p,r_p,d_p,{\theta }_p$ are as follows:

$${\mu }_p=r_p\cos ({\theta }_p),{\nu }_p=r_p\sin ({\theta }_p),where{d}_p=1-2{\theta }_p/\pi$$

The functions are established through the Takagi–Sugeno approach and can be expressed as below:

$$F\left(r,d\right)=\frac{1}{2}+r(d-\frac{1}{2})$$

And because $d=1-\frac{2\theta }{\pi }$, the function can also be expressed as:

$$F\left(r,\theta \right)=\frac{1}{2}+r\left(\frac{1}{2}-\frac{2\theta }{\pi }\right)$$

To compare PFNs, Yager [9] proposed a formula as follows:

$$V\left(p\right)=\frac{1}{2}+r_p\left(d_p-\frac{1}{2}\right)=\frac{1}{2}+r_p\left(\frac{1}{2}-\frac{2{\theta }_p}{\pi }\right)$$

(7)

Li and Zeng [10] compare several laws [9,25,30] of comparing PFNs and find that the Yager’s method is more credible. Their laws [10] of comparing two PFNs are as follows:

$$\begin{array}{l}\mathrm{Let}{p}_1=\left({\mu }_{p_1},{\nu }_{p_1}\right)\mathrm{and}{p}_2=\left({\mu }_{p_2},{\nu }_{p_2}\right)\mathrm{be}\mathrm{two}\mathrm{PFVs},\mathrm{then}\left(1\right)\mathrm{If}V\left(p_1\right)>V\left(p_2\right),\mathrm{then}{p}_1\succ p_2;\\ \left(2\right)\mathrm{If}V\left(p_1\right)=V\left(p_2\right),\mathrm{then}{p}_1\sim p_2.\end{array}$$

Definition 13.

Yager [9] proposed the weighted averaging aggregation operator to aggregate PFNs. PFNs consist of $p_1,p_2,\dots ,p_n$. Each $p_i=\left({\mu }_{pi},{\nu }_{pi}\right)$ is associated with an importance weight ${\omega }_i\in [0,1]\left(i=1,2,\dots ,n\right)$ and ${\displaystyle \sum _{\mathrm{i}=1}^n{\omega }_i}=1$, the Pythagorean fuzzy weighted average is defined as below:

$$C(p_1,p_{2,}\cdots ,p_n)=({\displaystyle \sum _{i=1}^n{\omega }_i{\mu }_{pi}},{\displaystyle \sum _{i=1}^n{\omega }_i{\nu }_{pi}})$$

(8)

3. Pythagorean Fuzzy Entropy and Pythagorean Fuzzy Soft (PFS) Entropy

Entropy measure is the most widespread form of uncertainty measures. Xue [11] popularized the concept of entropy for intuitionistic fuzzy sets [22].

Definition 14.

Let$PFSs(X)$denote the set of all PFSs in$X$. A crispfunction$E:PFE\left(X\right)\to [0,1]$is said to be an entropy on$PFSs(X)$, if it satisfies the following properties [11,22,31].

(D1) 

$E_P(\beta )=0\mathrm{if}\mathrm{and}\mathrm{only}\mathrm{if}\left(\mathrm{iff}\right)\beta \mathrm{is}a\mathrm{crisp}\mathrm{set};$

(D2) 

$E_P\left(\beta \right)=1$ iff ${\mu }_{\beta }\left(x_i\right)={\nu }_{\beta }\left(x_i\right)\mathrm{for}\forall x_i\in X;$

(D3) 

$E_p({\beta }_1)\le E_p({\beta }_2)\mathrm{if}{\beta }_1\mathrm{is}\mathrm{less}\mathrm{fuzzy}\mathrm{than}{\beta }_2,\mathrm{i}.\mathrm{e}.{\nu }_{{\beta }_1}\left(x_i\right)\ge {\nu }_{{\beta }_2}\left(x_i\right)\mathrm{and}{\mu }_{{\beta }_1}(x_i)\le {\mu }_{{\beta }_2}(x_i)\mathrm{for}{\mu }_{{\beta }_2}(x_i)\le {\nu }_{{\beta }_2}\left(x_i\right),\forall x_i\in X,$$\mathrm{or}{\nu }_{{\beta }_1}\left(x_i\right)\le {\nu }_{{\beta }_2}\left(x_i\right)\mathrm{and}{\mu }_{{\beta }_1}(x_i)\ge {\mu }_{{\beta }_2}(x_i)\mathrm{for}{\mu }_{{\beta }_2}(x_i)\ge {\nu }_{{\beta }_2}\left(x_i\right),\forall x_i\in X;$

(D4) 

$E_P\left(\beta \right){=E}_P\left({\beta }^C\right).$

Xue and Xu [11] then presented and proved a definition for Pythagorean fuzzy entropy as follows:

$$E_p(\beta )=\frac{1}{n}{\displaystyle \sum _{i=1}^n[1-({\mu }_{\beta }^2(x_i)+{\nu }_{\beta }^2(x_i))\left|{\mu }_{\beta }^2(x_i)-{\nu }_{\beta }^2(x_i)\right|]}$$

(9)

However, the property (D2) in Xue’s Definition 14 does not accord with reality and fails to describe the maximum degree of fuzziness in PFSs objectively. There are several reasons for that. Firstly, only when ${\mu }_{\beta }\left(x\right)={\nu }_{\beta }\left(x\right)=0$, we know nothing about the universe of discourse [32]. It is very obvious that we know more in the case of ${\mu }_{\beta }\left(x\right)={\nu }_{\beta }\left(x\right)\ne 0$ than in the case of ${\mu }_{\beta }\left(x\right)={\nu }_{\beta }\left(x\right)=0$. Secondly, hesitancy degree or intuitionism has been ignored in the (D2). In fact, even if membership is equal to non-membership, but when membership and non-membership increase in the meantime, it means that we know more about the universe of discourse and entropy should decrease in that case. But this situation will not happen according to the property (D2). Thirdly, IFSs and PFSs entropy measure should become the maximum value when ${\mu }_{\beta }\left(x\right)={\nu }_{\beta }\left(x\right)=0$. Bustince [33] call this situation as IFSs completely intuitionistic. In conclusion, we think unreasonable condition in Definition 14 should be revised and changed. Inspired by the paper [11,22,29,32], we give our Pythagorean fuzzy entropy definition and entropy measure as Definitions 15 and 16. It is obvious that our definition and measure have good symmetry.

Definition 15.

(A novel Pythagorean fuzzy entropy definition.) Let$PFSs(X)$denote the set of all PFSs in the universe of discourse$X$. A crispfunction$E:PFE\left(X\right)\to [0,1]$is said to be a novel entropy on$PFSs(X)$ [11,22,29,32], if it satisfies the following properties:

(P1) 

$E_P(\beta )=0\mathrm{if}\mathrm{and}\mathrm{only}\mathrm{if}\left(\mathrm{iff}\right)\beta \mathrm{is}\mathrm{a}\mathrm{crisp}\mathrm{set};$

(P2) 

$E_P\left(\beta \right)=1\mathrm{if}\mathrm{and}\mathrm{only}\mathrm{if}$${\mu }_{\beta }\left(x_i\right)={\nu }_{\beta }\left(x_i\right)=0$$\mathrm{for}\forall x_i\in X;$

(P3) 

$$\begin{gathered} E_p({\beta }_1)\le E_p({\beta }_2)\mathrm{if}{\beta }_1\mathrm{is}\mathrm{less}\mathrm{fuzzy}\mathrm{than}{\beta }_2,\mathrm{i}.\mathrm{e}.{\nu }_{{\beta }_1}\left(x_i\right)\ge {\nu }_{{\beta }_2}\left(x_i\right)\mathrm{and}{\mu }_{{\beta }_1}(x_i)\le {\mu }_{{\beta }_2}(x_i)\mathrm{for}{\mu }_{{\beta }_2}(x_i)\le {\nu }_{{\beta }_2}\left(x_i\right),\forall x_i\in X, \\ \mathrm{or}{\nu }_{{\beta }_1}\left(x_i\right)\le {\nu }_{{\beta }_2}\left(x_i\right)\mathrm{and}{\mu }_{{\beta }_1}(x_i)\ge {\mu }_{{\beta }_2}(x_i)\mathrm{for}{\mu }_{{\beta }_2}(x_i)\ge {\nu }_{{\beta }_2}\left(x_i\right),\forall x_i\in X; \end{gathered}$$

(P4) 

$E_P\left(\beta \right){=E}_P\left({\beta }^C\right).$

Definition 16.

(A novel Pythagorean fuzzy entropy.) Let $P=\{\langle x,{\mu }_{\beta }(x),{\mu }_{\beta }(x)\rangle |x\in X\}$ be a PFSs in the universe of discourse $X=\{x_1,x_2,\cdots x_n\}$. ${\beta }_{\mathrm{i}}$$\left(\mathrm{i}=1,2,\cdots ,n\right)$ be a separate element from $\beta$, then the novel Pythagorean fuzzy entropy $E_{PF}(\beta )$ is defined as follows:

$$E_{PF}(\beta )=\frac{1}{2n}{\displaystyle \sum _{i=1}^n\left(2-{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)-\left|{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)\right|\right)}$$

(10)

Lemma 1.

$E_{PF}(\beta )$satisfies all properties in Definition 15.

Proof (P1):

$E_{PF}\left(\beta \right)=0\iff E_{PF}\left({\beta }_i\right)=0\iff 2-{\mu }_{\beta }\left(x_i\right)-{\nu }_{\beta }\left(x_i\right)-\left|{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)\right|=0$ when ${\mu }_{\beta }\left(x_i\right)\ge {\nu }_{\beta }(x_i),\left|{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)\right|=$${\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)$, then $2-{\mu }_{\beta }\left(x_i\right)-{\nu }_{\beta }\left(x_i\right)-\left|{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)\right|=2-{\mu }_{\beta }\left(x_i\right)-{\nu }_{\beta }\left(x_i\right)-\left({\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)\right)=2-2{\mu }_{\beta }\left(x_i\right)=0$ iff ${\mu }_{\beta }\left(x_i\right)=1,{\nu }_{\beta }\left(x_i\right)=0$. In a similar way, when ${\mu }_{\beta }\left(x_i\right)\ge {\nu }_{\beta }(x_i)$, we can get ${\mu }_{\beta }\left(x_i\right)=0,{\nu }_{\beta }\left(x_i\right)=1$. So $E_P(\beta )=0\mathrm{if}\mathrm{and}\mathrm{only}\mathrm{if}\left(\mathrm{iff}\right)\beta \mathrm{is}a\mathrm{crisp}\mathrm{set};$ □

Proof (P2):

$E_{PF}\left(\beta \right)=1\iff E_{PF}\left({\beta }_i\right)=1\iff$$2-{\mu }_{\beta }\left(x_i\right)-{\nu }_{\beta }\left(x_i\right)-\left|{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)\right|=2$, So ${\mu }_{\beta }\left(x_i\right)+{\nu }_{\beta }\left(x_i\right)+\left|{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)\right|=0$. $\because {\mu }_{\beta }\left(x_i\right)\ge 0,{\nu }_{\beta }\left(x_i\right)\ge 0$$\left|{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)\right|\ge 0$, Then we can get ${\mu }_{\beta }\left(x_i\right)=0,{\nu }_{\beta }\left(x_i\right)=0$. □

Proof (P3):

When ${\nu }_{{\beta }_1}\left(x_i\right)\ge {\nu }_{{\beta }_2}\left(x_i\right)\mathrm{and}{\mu }_{{\beta }_1}(x_i)\le {\mu }_{{\beta }_2}(x_i)\mathrm{for}{\mu }_{{\beta }_2}(x_i)\le {\nu }_{{\beta }_2}\left(x_i\right),\forall x_i\in X$, we can have $0\le {\mu }_{{\beta }_1}(x_i)\le {\mu }_{{\beta }_2}(x_i)\le {\nu }_{{\beta }_2}\left(x_i\right)\le {\nu }_{{\beta }_1}\left(x_i\right)\le 1$, ${\mu }_{{\beta }_1}\left(x_i\right)+{\nu }_{{\beta }_1}\left(x_i\right)+\left|{\mu }_{{\beta }_1}(x_i)-{\nu }_{{\beta }_1}(x_i)\right|={\mu }_{{\beta }_1}\left(x_i\right)+{\nu }_{{\beta }_1}\left(x_i\right)+{\nu }_{{\beta }_1}(x_i)-{\mu }_{{\beta }_1}(x_i)=$$2{\nu }_{{\beta }_1}(x_i)$. ${\mu }_{{\beta }_2}\left(x_i\right)+{\nu }_{{\beta }_2}\left(x_i\right)+\left|{\mu }_{{\beta }_2}(x_i)-{\nu }_{{\beta }_2}(x_i)\right|={\mu }_{{\beta }_2}\left(x_i\right)+{\nu }_{{\beta }_2}\left(x_i\right)+{\nu }_{{\beta }_2}(x_i)-{\mu }_{{\beta }_2}(x_i)=$$2{\nu }_{{\beta }_2}(x_i)$.

Since ${\nu }_{{\beta }_2}(x_i)\le {\nu }_{{\beta }_1}(x_i)$, we can have $2-2{\nu }_{{\beta }_1}(x_i)\le 2-2{\nu }_{{\beta }_2}(x_i)$. Then $2-{\mu }_{{\beta }_1}\left(x_i\right)-{\nu }_{{\beta }_1}\left(x_i\right)-\left|{\mu }_{{\beta }_1}(x_i)-{\nu }_{{\beta }_1}(x_i)\right|$$\le 2-{\mu }_{{\beta }_2}\left(x_i\right)-{\nu }_{{\beta }_2}\left(x_i\right)-\left|{\mu }_{{\beta }_2}(x_i)-{\nu }_{{\beta }_2}(x_i)\right|$ So $E_{PF}({\beta }_1)\le E_{PF}({\beta }_2)$. When ${\nu }_{{\beta }_1}\left(x_i\right)\le {\nu }_{{\beta }_2}\left(x_i\right)\mathrm{and}{\mu }_{{\beta }_1}(x_i)\ge {\mu }_{{\beta }_2}(x_i)$ $\mathrm{for}{\mu }_{{\beta }_2}(x_i)\ge {\nu }_{{\beta }_2}\left(x_i\right),\forall x_i\in X$. In a similar way, We have $E_{PF}({\beta }_1)\le E_{PF}({\beta }_2)$. □

Proof (P4):

$E_{PF}(\beta )$$=\frac{1}{2n}{\displaystyle \sum _{i=1}^n\left(2-{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)-\left|{\mu }_{\beta }(x_i)-{\nu }_{\beta }(x_i)\right|\right)}$$=\frac{1}{2n}{\displaystyle \sum _{i=1}^n\left(2-{\nu }_{\beta }(x_i)-{\mu }_{\beta }(x_i)-\left|{\nu }_{\beta }(x_i)-{\mu }_{\beta }(x_i)\right|\right)}$$=E_{PF}({\beta }^{\mathrm{C}})$. □

Definition 17.

(Entropy on Pythagorean Fuzzy Soft Sets): Let$U=\{x_1,x_2,\cdots ,x_m\}$denote a universe of discourse. Let$E=\{e_1,e_2,\cdots e_n\}$denote a set of parameters.$\left(F,E\right)$and$\left(F,e_j\right)\left(j=1,2\cdots ,n\right)$are the Pythagorean fuzzy soft sets. Let,

$$H\left(F,E\right)=\frac{1}{n}{\displaystyle \sum _{i=1}^{n}H\left(F,e_j\right)}$$

(11)

$$H\left(F,e_j\right)=\frac{1}{2m}{\displaystyle \sum _{i=1}^m(2-{\mu }_{F(e_j)}\left(x_i\right)}-{\nu }_{F(e_j)}\left(x_i\right)-\left|{\mu }_{F(e_j)}\left(x_i\right)-{\nu }_{F(e_j)}\left(x_i\right)\right|)$$

(12)

We can call $H\left(F,E\right)$ as the entropy on PFSSs. According to Definition 16 and the proof process of Lemma 1, it is obvious that we can prove $H\left(F,E\right)$ is entropy on the Pythagorean fuzzy soft sets. Due to the limitation of space, it is omitted here.

4. Integrated Weight of Attributes Based on the PFS Entropy

Existing uncertainty measure are mostly defined based on entropy. When the entropy of an attribute becomes smaller, it means the uncertainty decreases and the evaluation information under this attribute is more reliable and certain. So this attribute is more important for decision making and should be given a greater weight. The entropy weight method avoids the secondary uncertainty brought by expert weighting model. We adopt a method combining the above two methods. Firstly, we determine the objective attribute weight based on Pythagorean fuzzy soft entropy. Then we adjust the objective attribute weights to reflect the subjective preferences of decision makers. At last, we get the integrated weight of attributes.

For an MADM problem, let $U_i\left(i=1,2,\cdots m\right)$ be the alternatives. The performance of the alternative $U_i$ is assessed across a set of attributes $\{e_1,e_2,\cdots e_n\}$. The decision makers give the subjective weights vector $\lambda =\{{\lambda }_1,{\lambda }_2,\cdots {\lambda }_n\}$, the process of calculating the objective attribute weights and the integrated weights of attributes are as follows.

Definition 18.

The objective weight${\rho }_j$of attribute$e_j$is [18]:

$${\rho }_j=\frac{1-H(F,e_j)}{{\displaystyle \sum _{j=1}^n(1-H(F,e_j))}},j=1,2,\cdots ,n$$

(13)

where

$$H\left(F,e_j\right)=\frac{1}{2m}{\displaystyle \sum _{i=1}^m(2-{\mu }_{F(e_j)}\left(U_i\right)-{\nu }_{F(e_j)}\left(U_i\right)}-\left|{\mu }_{F(e_j)}\left(U_i\right)-{\nu }_{F(e_j)}\left(U_i\right)\right|)$$

The integrated weight of attribute $e_j$ is defined as follows:

$${\omega }_j=\frac{{\rho }_j{\lambda }_j}{{\displaystyle \sum _{j=1}^n{\rho }_j{\lambda }_j}},j=1,2,\cdots ,n$$

(14)

5. Multiple-Attribute Group Decision Making (MAGDM) Method Based on the Novel Pythagorean Fuzzy Soft Entropy

Suppose that there are $l$ experts participating in the decision-making process. Let $\{U_1,U_2,\cdots ,U_m\}$ be the alternatives and $\{e_1,e_2,\cdots e_n\}$ be the attribute set. The attributes are independent of each other. Because every expert has different knowledge structure and they are not familiar with every attributes, they usually give evaluation values only for certain attributes. The evaluation values are given by Pythagorean fuzzy numbers $<{\mu }_{F\left(e_j\right)}^{\left(k\right)}\left(U_i\right),{\nu }_{F\left(e_j\right)}^{\left(k\right)}\left(U_i\right)>$$\left(1\le k\le l,1\le i\le m,1\le j\le n\right)$. It means that the kth expert give $<{\mu }_{F\left(e_j\right)}^{\left(k\right)}\left(U_i\right),{\nu }_{F\left(e_j\right)}^{\left(k\right)}\left(U_i\right)>$ as the evaluation value of ith alternative under jth attribute. The decision group gives the subjective weights vector of attributes $\lambda =\{{\lambda }_1,{\lambda }_2,\cdots {\lambda }_n\}$.

Step 1.

Suppose that there are $p_j(1\le p_j\le k)$ experts whose weight vector is $\theta =\{{\theta }_1,{\theta }_2,\cdots {\theta }_{p_j}\}$ giving evaluation values under jth attribute. By the formula (8), we now obtain the overall evaluation value as follows.

$$<{\mu }_{ij},{\nu }_{ij}>=<{\displaystyle \sum _{q=1}^{p_j}{\theta }_q}{\mu }_{F\left(e_j\right)}^{\left(k\right)}\left(U_i\right),{\displaystyle \sum _{q=1}^{p_j}{\theta }_q}{\nu }_{F\left(e_j\right)}^{\left(k\right)}\left(U_i\right)>$$

Step 2.

Let $\{U_1,U_2,\cdots ,U_m\}$ be the universe of discourse and $\{e_1,e_2,\cdots e_n\}$ be a set of parameters. We can establish a binary table form of PFSSs $\left(F,E\right)$ as

Table 1. The binary table form of Pythagorean fuzzy soft sets (PFSSs).

	e1	e2	…	en
$U_1$	$<{\mu }_{11},{\nu }_{11}>$	$<{\mu }_{12},{\nu }_{12}>$	…	$<{\mu }_{1n},{\nu }_{1n}>$
$U_2$	$<{\mu }_{21},{\nu }_{21}>$	$<{\mu }_{22},{\nu }_{22}>$	…	$<{\mu }_{2n},{\nu }_{2n}>$
…	…	…	…	…
$U_m$	$<{\mu }_{m1},{\nu }_{m1}>$	$<{\mu }_{m2},{\nu }_{m2}>$	…	$<{\mu }_{mn},{\nu }_{mn}>$

.

Step 3.

Calculate fuzzy entropy $H\left(F,e_j\right)$ of different attributes on Pythagorean fuzzy soft sets by utilizing Equation (12).

Step 4.

Obtain the objective weight ${\rho }_j$ and integrated weight ${\omega }_j$ of attribute by utilizing Equation (13) and Equation (14).

Step 5.

Determine alternatives’ positive ideal solution (PIS) $R^{+}$ and negative ideal solution (NIS) $R^{-}$ in Pythagorean fuzzy model for synthetic judgement as follows:

$$R^{+}=\{\langle {\mu }_1^{+},{\nu }_1^{+}\rangle ,\langle {\mu }_2^{+},{\nu }_2^{+}\rangle ,\cdots ,\langle {\mu }_n^{+},{\nu }_n^{+}\rangle \}$$

(15)

$$R^{-}=\{\langle {\mu }_1^{-},{\nu }_1^{-}\rangle ,\langle {\mu }_2^{-},{\nu }_2^{-}\rangle ,\cdots ,\langle {\mu }_n^{-},{\nu }_n^{-}\rangle \}$$

(16)

For benefit attributes: where ${\mu }_j^{+}=\underset{1\le i\le m}{\max }\{{\mu }_{ij}\},{\nu }_j^{+}=\underset{1\le i\le m}{\min }\{{\nu }_{ij}\}(j=1,2,\cdots ,n),{\mu }_j^{-}=\underset{1\le i\le m}{\min }\{{\mu }_{ij}\},{\nu }_j^{-}=\underset{1\le i\le m}{\max }\{{\nu }_{ij}\}(j=1,2,\cdots ,n)$

For cost attributes: where ${\mu }_j^{+}=\underset{1\le i\le m}{\min }\{{\mu }_{ij}\},{\nu }_j^{-}=\underset{1\le i\le m}{\max }\{{\nu }_{ij}\}(j=1,2,\cdots ,n),{\mu }_j^{-}=\underset{1\le i\le m}{\max }\{{\mu }_{ij}\},{\nu }_j^{+}=\underset{1\le i\le m}{\min }\{{\nu }_{ij}\}(j=1,2,\cdots ,n)$

Zhang [25] determine PIS and NIS for each attribute according to score function of each element ($<{\mu }_{ij},{\nu }_{ij}>$). But their score function are pointed out that the comparison result is sometimes unreasonable [10,30]. And our method also has another advantage which possesses higher distinguish degree. Because the distance between alternatives and PIS or NIS will be larger with our method in same distance measure. It is obvious that any elements in our PIS will be better than [25] under several laws of comparing PFNs [9,25,30].

Step 6.

Calculate the weighted Pythagorean fuzzy distance $D\left(U_i,R^{+}\right)$ between alternative $U_i$ and positive ideal solution(PIS) $R^{+}$ and the weighted Pythagorean fuzzy distance $D\left(U_i,R^{-}\right)$ between alternative $U_i$ and negative ideal solution (NIS) $R^{-}$. We think the four parameters are equal here.

$$D\left(U_i,R^{+}\right)=\frac{1}{4}{\displaystyle \sum _{j=1}^n{\omega }_j}(\left|{\mu }_{ij}-{\mu }_j^{+}\right|+\left|{\nu }_{ij}-{\nu }_j^{+}\right|+\left|r_{ij}-r_j^{+}\right|+{\left|d_{ij}-d_j^{+}\right|}^{\lambda })$$

(17)

$$D\left(U_i,R^{-}\right)=\frac{1}{4}{\displaystyle \sum _{j=1}^n{\omega }_j}(\left|{\mu }_{ij}-{\mu }_j^{-}\right|+\left|{\nu }_{ij}-{\nu }_j^{-}\right|+\left|r_{ij}-r_j^{-}\right|+{\left|d_{ij}-d_j^{-}\right|}^{\lambda })$$

(18)

Step 7.

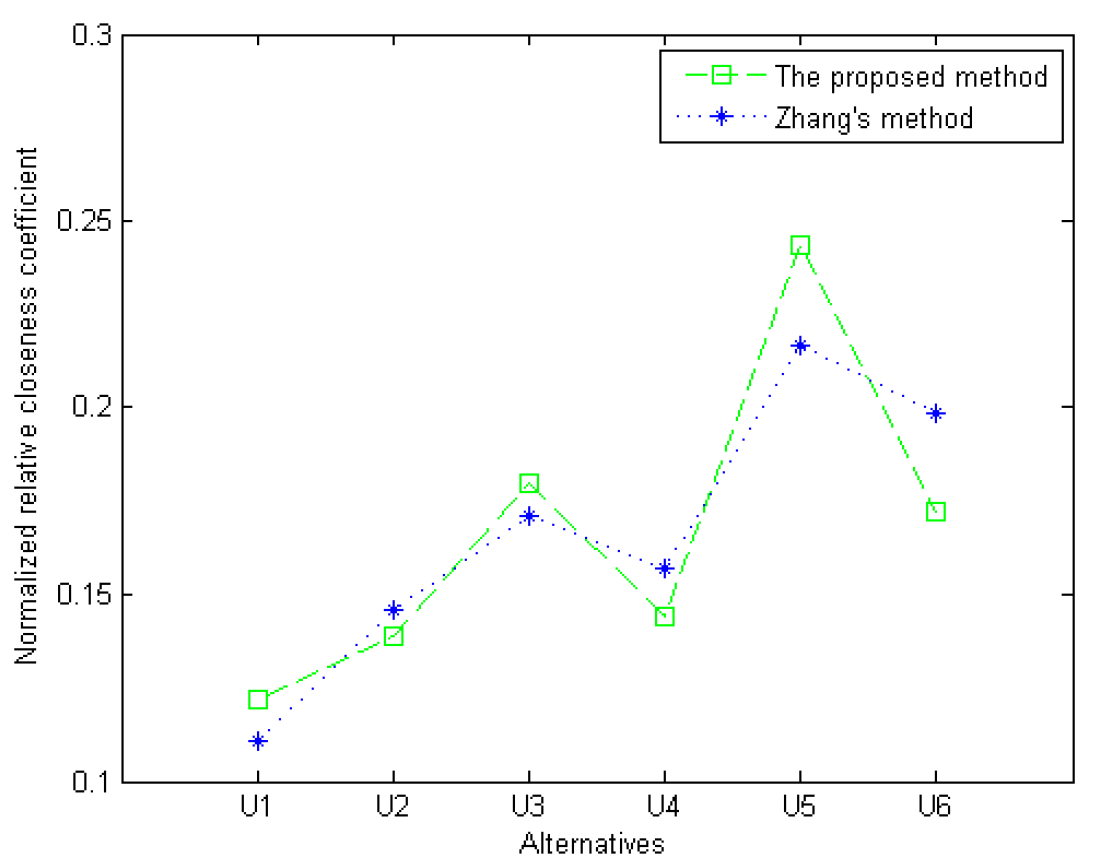
Calculate the relative closeness coefficient [34] $C_i$ to the Pythagorean ideal solution.

$$C_i=\frac{D\left(U_i,R^{-}\right)}{D\left(U_i,R^{+}\right)+D\left(U_i,R^{-}\right)}$$

(19)

Step 8.

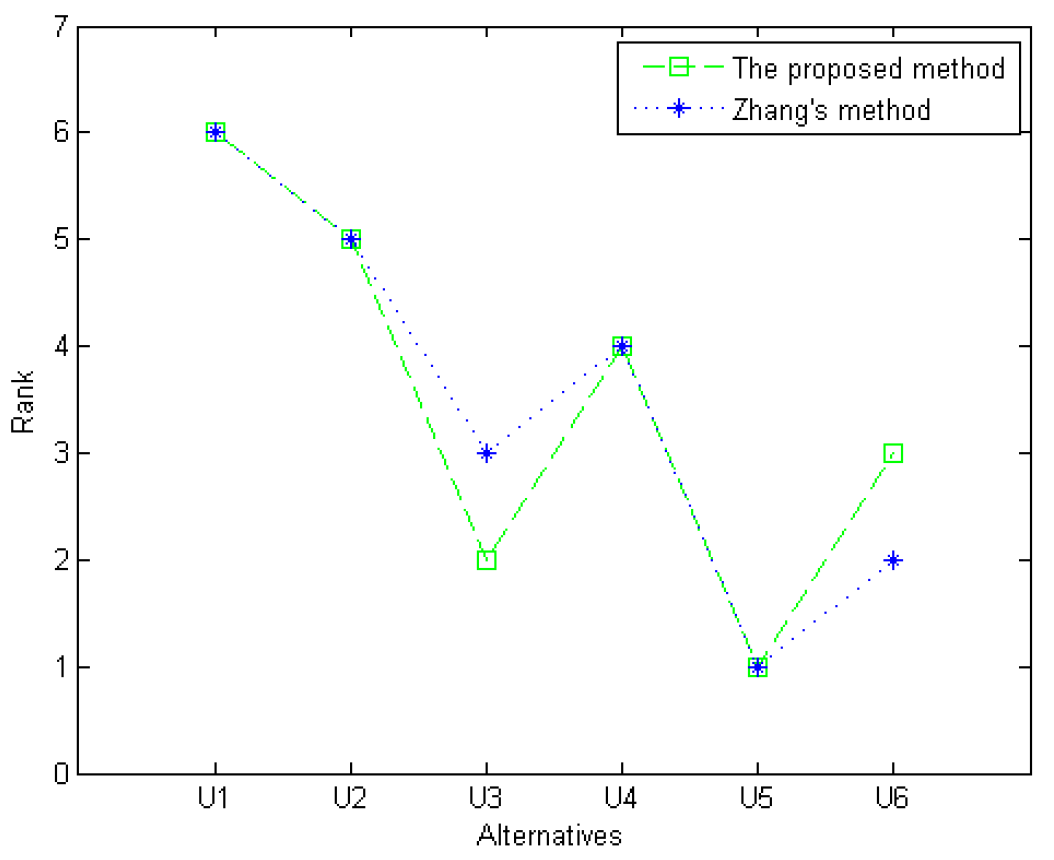
Rank the alternatives according to the above relative closeness coefficient $C_i$. The lager the $C_i$ is, the better the alternative $U_i$ is.

6. Illustrative Example

We have proposed a MAGDM method based on the novel PFS entropy measure. In this section, the method will be used in selecting a missile position. Assuming that in the process of making a battle plan, staff officers need to select a place as missile position. The primary factors which they considered are the following:

$e_1$: The operational intensions of superiors

$e_2$: The geological conditions of positions

$e_3$: The efficiency of firepower exertion

$e_4$: Maneuverability

$e_5$: Battlefield viability

Through a wide screening and comparison, six places $\{U_1,U_2,U_3,U_4,U_5,U_6\}$ are preliminarily selected as alternatives. To make a better decision, three experts are invited to give their PFNs evaluation values to the alternatives according to collected information, data, and their experiences. Expert A is familiar with $\{e_1,e_2,e_3\}$, Expert B is familiar with $\{e_2,e_3,e_4\}$, Expert C is familiar with $\{e_3,e_4,e_5\}$. Their evaluation values are in the

Table 2. The Pythagorean fuzzy numbers (PFNs) evaluation values of three experts.

2.1. The PFNs evaluation values of expert A
	$U_1$	$U_2$	$U_3$	$U_4$	$U_5$	$U_6$
$e_1$	<0.5, 0.8>	<0.7, 0.6>	<0.6, 0.3>	<0.4, 0.7>	<0.5, 0.2>	<0.8, 0.6>
$e_2$	<0.2, 0.3>	<0.5, 0.3>	<0.8, 0.4>	<0.7, 0.5>	<0.6, 0.2>	<0.7, 0.4>
$e_3$	<0.7, 0.2>	<0.8, 0.2>	<0.6, 0.6>	<0.8, 0.4>	<0.7, 0.6>	<0.4, 0.5>
2.2. The PFNs evaluation values of expert B
	$U_1$	$U_2$	$U_3$	$U_4$	$U_5$	$U_6$
$e_2$	<0.3, 0.6>	<0.7, 0.6>	<0.6, 0.3>	<0.8, 0.4>	<0.7, 0.3>	<0.4, 0.2>
$e_3$	<0.2, 0.7>	<0.6, 0.3>	<0.7, 0.6>	<0.6, 0.6>	<0.8, 0.1>	<0.7, 0.4>
$e_4$	<0.6, 0.5>	<0.3, 0.8>	<0.5, 0.7>	<0.5, 0.3>	<0.9, 0.2>	<0.7, 0.6>
2.3. The PFNs evaluation values of expert C
	$U_1$	$U_2$	$U_3$	$U_4$	$U_5$	$U_6$
$e_3$	<0.2, 0.8>	<0.7, 0.4>	<0.8, 0.2>	<0.6, 0.3>	<0.6, 0.5>	<0.8, 0.2>
$e_4$	<0.3, 0.6>	<0.1, 0.9>	<0.4, 0.6>	<0.3, 0.8>	<0.7, 0.2>	<0.9, 0.0>
$e_5$	<0.8, 0.3>	<0.6, 0.3>	<0.7, 0.6>	<0.6, 0.4>	<0.8, 0.4>	<0.3, 0.7>

.

Step 1.

Now we consider a simple condition. The three experts’ weight vector is equal. By the Formula (8), we can obtain the overall evaluation values.

Step 2.

We establish a binary table form of PFSSs $\left(F,E\right)$ as

Table 3. The overall evaluation values of three experts.

	${\mathit{e}}_1$	${\mathit{e}}_2$	${\mathit{e}}_3$	${\mathit{e}}_4$	${\mathit{e}}_5$
$U_1$	<0.5, 0.8>	<0.25, 0.45>	<0.37, 0.57>	<0.45, 0.55>	<0.8, 0.3>
$U_2$	<0.7, 0.6>	<0.6, 0.45>	<0.7, 0.3>	<0.2, 0.85>	<0.6, 0.3>
$U_3$	<0.6, 0.3>	<0.7, 0.35>	<0.7, 0.47>	<0.45, 0.65>	<0.7, 0.6>
$U_4$	<0.4, 0.7>	<0.7, 0.5>	<0.67, 0.43>	<0.4, 0.55>	<0.6, 0.4>
$U_5$	<0.5, 0.2>	<0.75, 0.25>	<0.7, 0.4>	<0.8, 0.2>	<0.8, 0.4>
$U_6$	<0.8, 0.6>	<0.55, 0.3>	<0.63, 0.37>	<0.8, 0.3>	<0.3, 0.7>

according to the overall evaluation values.

Step 3.

Calculate fuzzy entropy $H\left(F,e_j\right)$ by utilizing Equation (12)

$$H(F,e_1)=0.317,H(F,e_2)=0.375,H(F,e_3)=0.338,H(F,e_4)=0.3,H(F,e_5)=0.3$$

Step 4.

Experts give their subjective weights vector $\lambda =\{0.24,0.17,0.18,0.23,0.18\}$ after careful consideration and discussion. Calculate the objective weight and integrated weight of the attribute by utilizing Equations (13) and (14). The results of calculating are shown in

Table 4. The weights of attributes.

	$\mathit{\lambda }$	$\mathit{\rho }$	$\mathit{\omega }$
$e_1$	0.24	0.2027	0.2449
$e_2$	0.17	0.1855	0.1588
$e_3$	0.18	0.1964	0.1780
$e_4$	0.23	0.2077	0.2347
$e_5$	0.18	0.2077	0.1837

.

Step 5.

Determine the alternatives’ positive ideal solution $R^{+}$ and negative ideal solution $R^{-}$ by utilizing Equations (15) and (16).

$$R^{+}=\{<0.8,0.2>,<0.750.25>,<0.70.3>,<0.8,0.2>,<0.8,0.3>\}$$

$$R^{-}=\{<0.4,0.8>,<0.25,0.5>,<0.37,0.57>,<0.2,0.85>,<0.3,0.7>\}$$

Step 6.

Calculate the weighted distance $D\left(U_i,R^{+}\right)$ and $D\left(U_i,R^{-}\right)$ by utilizing Equations (17) and (18).

$$D\left(U_1,R^{+}\right)=0.2061,D\left(U_1,R^{-}\right)=0.1433,D\left(U_2,R^{+}\right)=0.1713,D\left(U_2,R^{-}\right)=0.1507$$

$$D\left(U_3,R^{+}\right)=0.1602,D\left(U_3,R^{-}\right)=0.2455,D\left(U_4,R^{+}\right)=0.1969,D\left(U_4,R^{-}\right)=0.1851$$

$$D\left(U_5,R^{+}\right)=0.0629,D\left(U_5,R^{-}\right)=0.2884,D\left(U_6,R^{+}\right)=0.1442,D\left(U_6,R^{-}\right)=0.1985$$

Step 7.

Calculate the relative closeness coefficient $C_i$ to the Pythagorean ideal solution by utilizing Equation (19).

$$C_1=0.4101,C_2=0.4680,C_3=0.6051,C_4=0.4846,C_5=0.8210,C_6=0.5792.$$

According to the principle of “the lager the relative closeness coefficient is, the better the alternative is”. So the missile position alternatives are ranked as $U_5\succ U_3\succ U_6\succ U_4\succ U_2\succ U_1$, $U_5$ is selected as the best missile position among the alternatives.

Zhang [25] extended TOPSIS to multiple criteria decision making with Pythagorean fuzzy sets. They determine PIS and NIS according to the following Formula (20) and (21) based on the score function Equation (6) and they defined.

$$R^{+}=\{e_j,\underset{i}{max}\langle s(e_j(U_i))\rangle |j=1,2,\cdots n\}$$

(20)

$$R^{-}=\{e_j,\underset{i}{min}\langle s(e_j(U_i))\rangle |j=1,2,\cdots n\}$$

(21)

They calculate distance by the Formula (3). And their weight vector of attributes is directly given by the experts. To compare the methods, we can see the Table 2 as a decision matrix in this case. If we take their approach, the results are as follows:

$$C_1=0.3718,C_2=0.4882,C_3=0.5731,C_4=0.5268,C_5=0.7251,C_6=0.6648$$

The alternatives are ranked as $U_5\succ U_6\succ U_3\succ U_4\succ U_2\succ U_1$. The ranking results of two methods are similar (shown in Figure 1). The best alternative is the same. But the ranking order between $U_6$ and $U_3$ is different. $U_3\succ U_6$ for our method, whereas it is $U_6\succ U_3$ for Zhang’s method.

To show the difference of ranking results of two methods, we normalize the relative closeness coefficient for comparison’s sake. The normalized relative closeness coefficient $S_i$ is calculated by the following Formula (22). The computed results are shown in

Table 5. Comparison table of normalized relative closeness coefficient.

	${\mathit{U}}_1$	${\mathit{U}}_2$	${\mathit{U}}_3$	${\mathit{U}}_4$	${\mathit{U}}_5$	${\mathit{U}}_6$
Zhang’s method	0.1110	0.1457	0.1711	0.1573	0.2165	0.1985
The proposed method	0.1218	0.1390	0.1797	0.1439	0.2438	0.1720

and Figure 2 for a visual expression.

$$S_i=\frac{C_i}{{\displaystyle \sum _{i=1}^6{C}_i}}$$

(22)

From Figure 2, we can see that the green line is more steeper. This means that the degrees of difference between alternatives are larger by our method.

We calculate the distinguishability $K_i$ between neighboring alternatives by Formula (23) to analyze the evaluation differences between the two methods quantitatively.

$$K_i=\frac{\left|C_i-C_{i+1}\right|}{\frac{1}{6}{\displaystyle \sum _{j=1}^6{C}_j}}\times 100\%(i=1,2,3,4,5)$$

(23)

Table 6. Comparison table of the distinguishability between neighboring alternatives.

	${\mathit{K}}_1$	${\mathit{K}}_2$	${\mathit{K}}_3$	${\mathit{K}}_4$	${\mathit{K}}_5$	Mean Value
Zhang’s method	20.85	15.21	8.29	35.52	10.80	18.13
The proposed method	10.31	24.42	21.47	59.93	43.08	31.84

shows the results of the distinguishability between neighboring alternatives by two methods and their mean values. It means that the evaluation of alternatives and the effectiveness of decision making are better if the distinguishability values are larger. By our method, the mean value of $K_i$ is higher. So the proposed method has better distinguishability in evaluation results.

Furthermore, the Pythagorean fuzzy soft entropy measure proposed by us is another reason for better distinguishability. If we extend Xue’s Pythagorean Fuzzy entropy measure to Pythagorean fuzzy soft entropy measure, we may obtain unreasonable results especially in the case of ${\mu }_{\beta }\left(x\right)={\nu }_{\beta }\left(x\right)$. The property (D2) in Xue’s Pythagorean fuzzy entropy Definition 14 does not accord with reality to some extent and fails to describe the maximum degree of fuzziness in PFSs objectively. The analysis has been shown in Section 3.

7. Conclusions

To solve multiple attributes group decision-making problem, we improved the TOPSIS method to better deal with the MAGDM problems with Pythagorean fuzzy soft sets. In this process, we proposed a novel PFS entropy to better determine the weights of attributes. Results of an example and analysis of comparison with other methods shows the proposed method has higher reliability and better distinguishability in evaluation results. The main contributions of this paper are summarized as below:

(1)

We combined PFSs and soft sets which have advantages in handling vague and uncertain information.

(2)

In most cases, experts may only be familiar with some particular attributes. We considered this situation and introduced a method to aggregate evaluation information.

(3)

We redefined PF entropy and proposed novel PF and PFS entropy measures which are more reasonable and valid.

(4)

To better determine the weights of attributes, we used PFS entropy to obtain objective weights. Then we combined objective weights and experts’ subjective weights which includes decision makers’ subjective intention to obtain integrated weights.

(5)

To better apply the TOPSIS method in PFSSs, we introduced more reasonable ways of determining positive ideal solutions, negative ideal solutions and calculating distances between PFNs, etc.