Limit Analysis of Progressive Asymmetrical Collapse Failure of Tunnels in Inclined Rock Stratum
Abstract
:1. Introduction
2. Methods
2.1. Theoretical Basis
2.1.1. Upper Bound Theorem of Limit Analysis
2.1.2. Nonlinear Hoek–Brown Yield Criterion
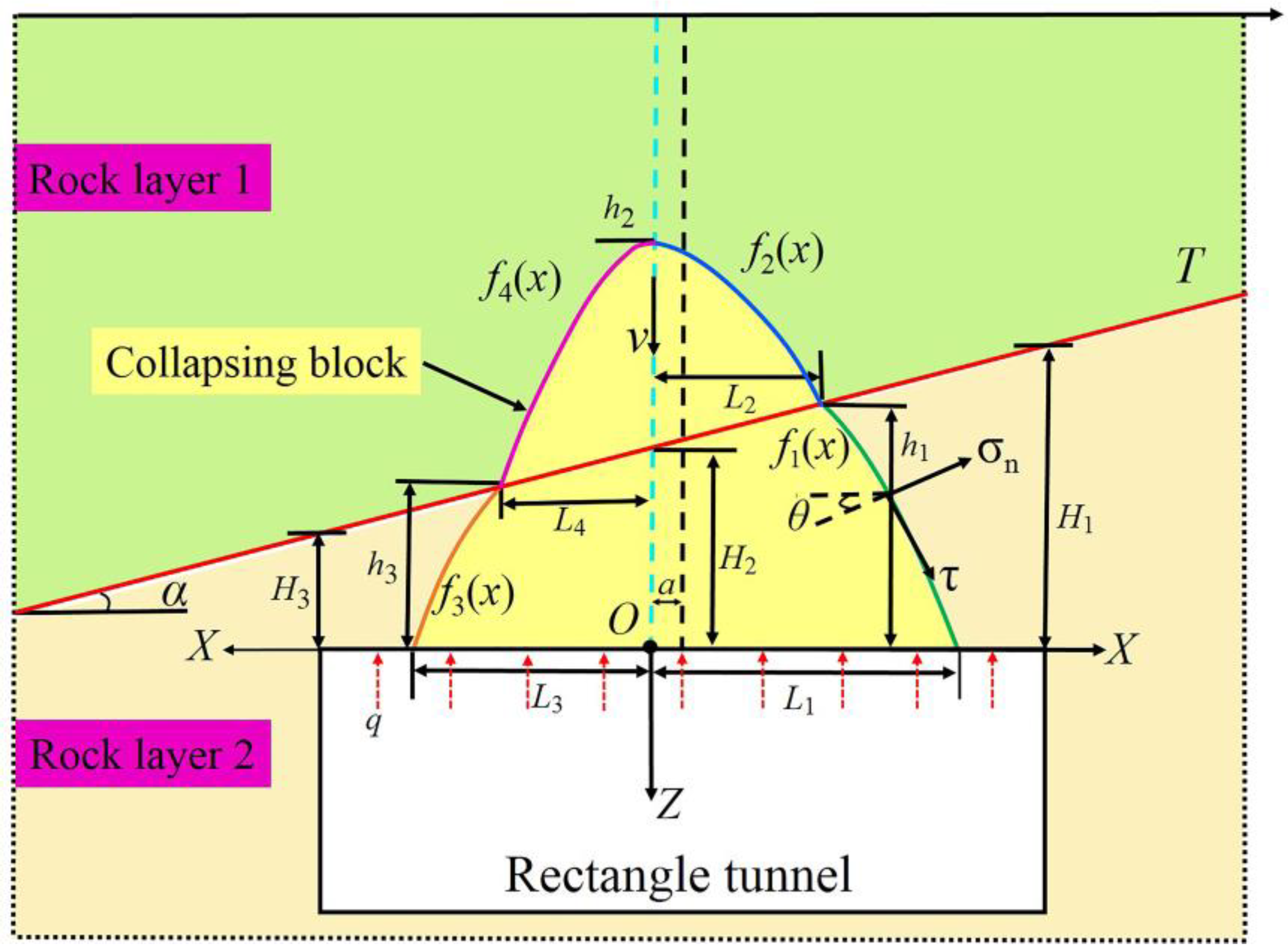
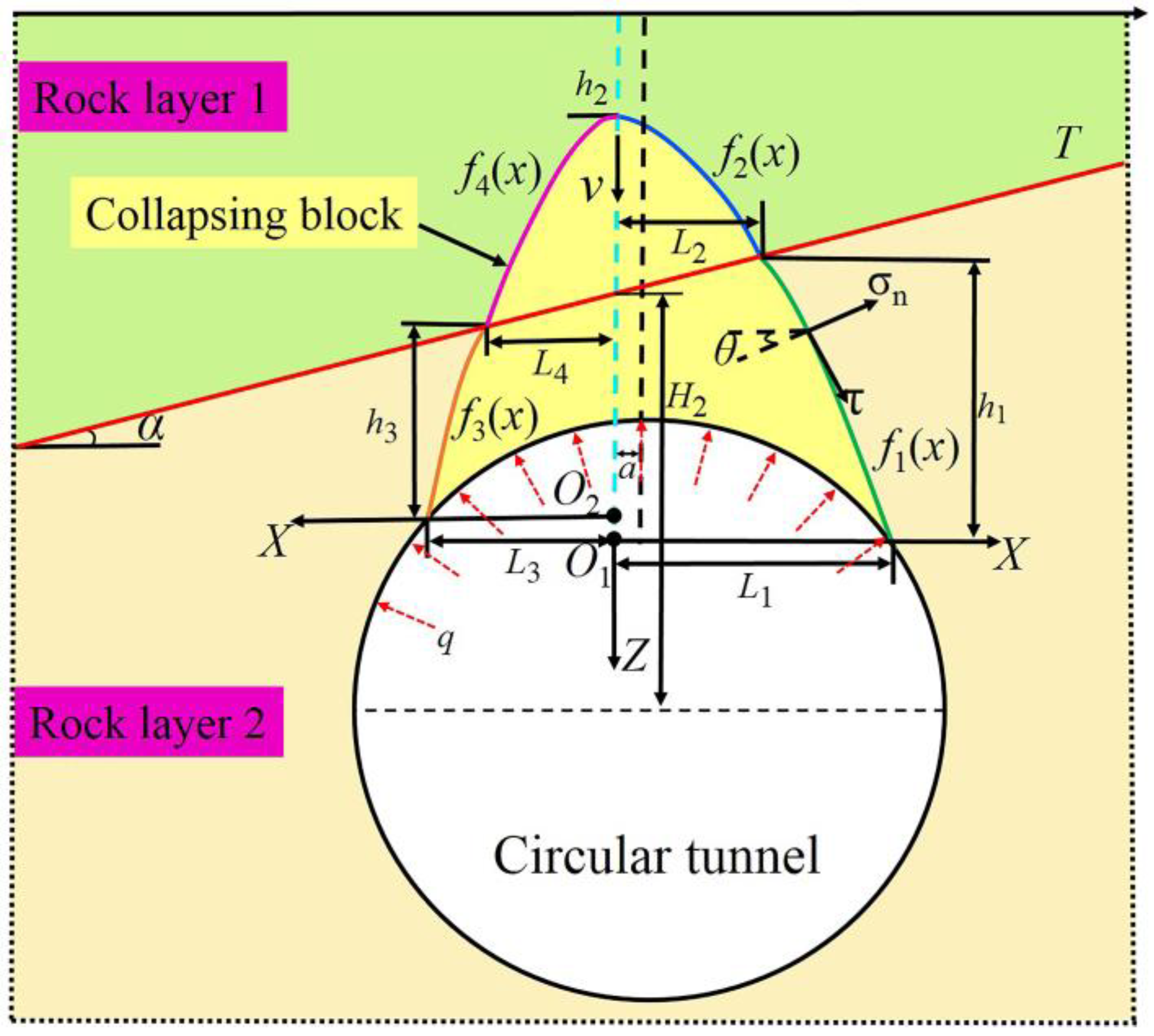
2.2. Progressive Asymmetrical Collapse Mechanism in Inclined Rock Stratum
3. Upper Bound Analysis of Progressive Asymmetrical Collapsing Block
3.1. Upper Bound Analysis of Rectangular Tunnels
3.2. Upper Bound Analysis of Circular Tunnels
4. Results and Discussion
4.1. Rationale Verification
4.1.1. Rectangular Tunnels
4.1.2. Circular Tunnels
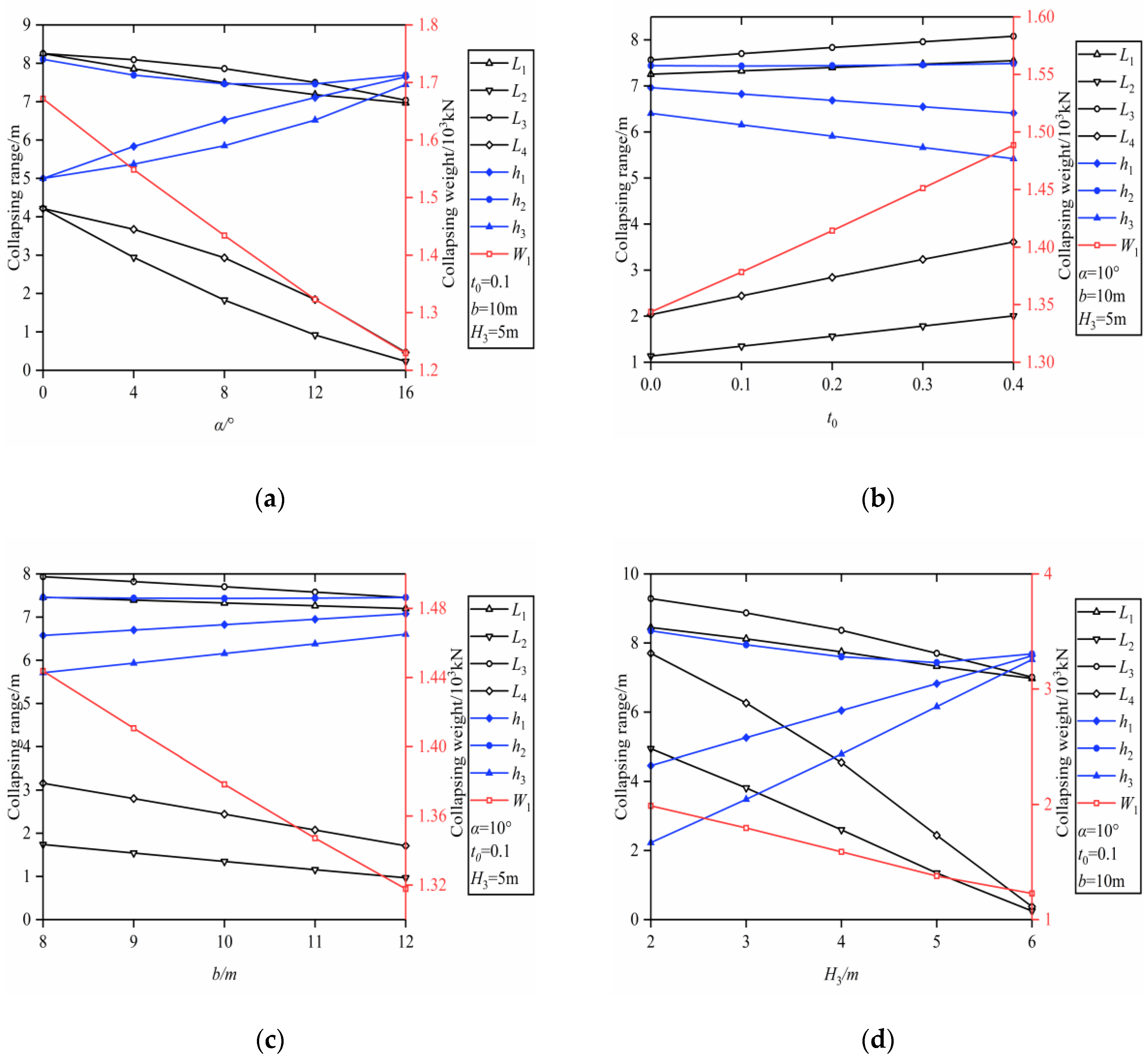
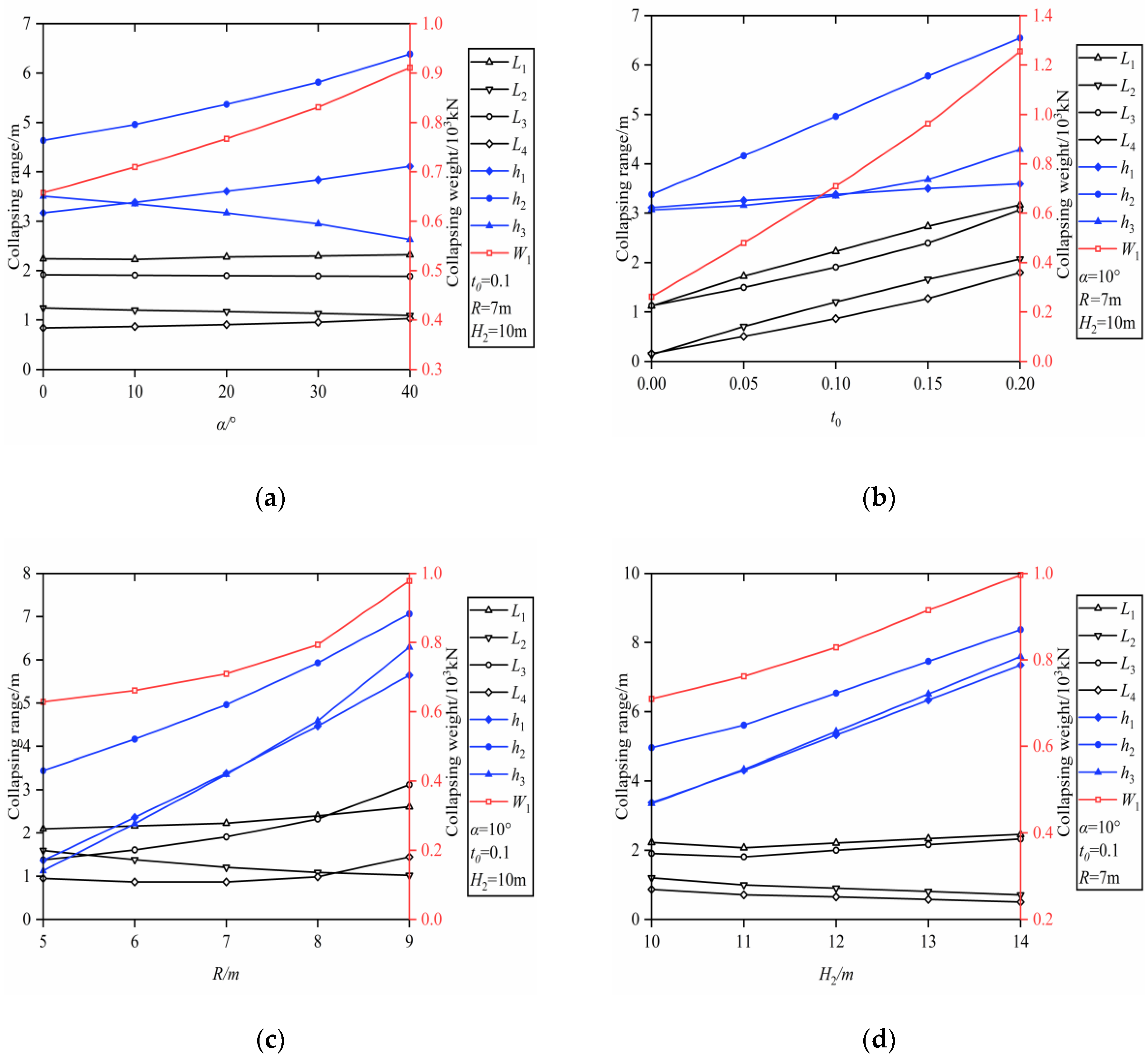
4.2. Sensitivity Analysis
4.2.1. Cases with Rectangular Tunnels
4.2.2. Cases with Circular Tunnels
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Derivation of the Total Rate of Energy Dissipation
Appendix B. Calculation of the Range and Total Weight of the Collapsing Block
References
- Lee, C.; Wu, B.; Chen, H.; Chiang, K. Tunnel stability and arching effects during tunneling in soft clayey soil. Tunn. Undergr. Space Technol. 2006, 21, 119–132. [Google Scholar] [CrossRef]
- Mollon, G.; Dias, D.; Soubra, A.-H. Face stability analysis of circular tunnels driven by a pressurized shield. J. Geotech. Geoenvironmental Eng. 2009, 136, 215–229. [Google Scholar] [CrossRef]
- Zhang, C.; Han, K. Collapsed Shape of Shallow Unlined Tunnels Based on Functional Catastrophe Theory. Math. Probl. Eng. 2015, 2015, 1–13. [Google Scholar] [CrossRef]
- Han, W.; Wang, G.; Liu, C.; Luan, H.; Wang, K. Time-Dependent Behavior of a Circular Symmetrical Tunnel Supported with Rockbolts. Symmetry 2018, 10, 381. [Google Scholar] [CrossRef]
- Liu, X.; Fang, Q.; Zhou, Q.; Liu, Y. Predicting Ground Settlement Due to Symmetrical Tunneling through an Energy Conservation Method. Symmetry 2018, 10, 186. [Google Scholar] [CrossRef]
- Min, B.; Zhang, X.; Zhang, C.; Gong, Y.; Yuan, T. Mechanical Behavior of Double-Arch Tunnels under the Effect of Voids on the Top of the Middle Wall. Symmetry 2018, 10, 703. [Google Scholar] [CrossRef]
- Mussa, M.H.; Mutalib, A.A.; Hamid, R.; Raman, S.N. Blast Damage Assessment of Symmetrical Box-Shaped Underground Tunnel According to Peak Particle Velocity (PPV) and Single Degree of Freedom (SDOF) Criteria. Symmetry 2018, 10, 158. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, L.; Xu, W.; Fu, Y.; Feng, J. Flat-plate roof collapse of shallow caverns and protective measures: A case study of Longyou ancient siltstone caverns. Nat. Hazards 2014, 76, 191–213. [Google Scholar] [CrossRef]
- Qin, C.; Chian, S.C.; Yang, X.; Du, D. 2D and 3D limit analysis of progressive collapse mechanism for deep-buried tunnels under the condition of varying water table. Int. J. Rock Mech. Min. Sci. 2015, 80, 255–264. [Google Scholar] [CrossRef]
- Monia, C.; Antonio, P.; Nicola, S. Reconstruction of the Geotechnical Model Considering Random Parameters Distributions. Eng. Geol. Soc. Territ. 2015, 2, 1347–1351. [Google Scholar]
- Vu-Bac, N.; Lahmer, T.; Zhuang, X.; Nguyen-Thoi, T.; Rabczuk, T. A software framework for probabilistic sensitivity analysis for computationally expensive models. Adv. Eng. Softw. 2016, 100, 19–31. [Google Scholar] [CrossRef]
- Pasculli, A.; Calista, M.; Sciarra, N.; Antonio, P.; Monia, C.; Nicola, S. Variability of local stress states resulting from the application of Monte Carlo and finite difference methods to the stability study of a selected slope. Eng. Geol. 2018, 245, 370–389. [Google Scholar] [CrossRef]
- Fraldi, M.; Guarracino, F. Limit analysis of collapse mechanisms in cavities and tunnels according to the Hoek–Brown failure criterion. Int. J. Rock Mech. Min. Sci. 2009, 46, 665–673. [Google Scholar] [CrossRef]
- Fraldi, M.; Guarracino, F. Analytical solutions for collapse mechanisms in tunnels with arbitrary cross sections. Int. J. Solids Struct. 2010, 47, 216–223. [Google Scholar] [CrossRef]
- Huang, F.; Yang, X. Upper bound limit analysis of collapse shape for circular tunnel subjected to pore pressure based on the Hoek–Brown failure criterion. Tunn. Undergr. Space Technol. 2011, 26, 614–618. [Google Scholar] [CrossRef]
- Li, T.; Yang, X. Limit analysis of failure mechanism of tunnel roof collapse considering variable detaching velocity along yield surface. Int. J. Rock Mech. Min. Sci. 2017, 100, 229–237. [Google Scholar] [CrossRef]
- Yang, X.; Huang, F. Collapse mechanism of shallow tunnel based on nonlinear Hoek–Brown failure criterion. Tunn. Undergr. Space Technol. 2011, 26, 686–691. [Google Scholar] [CrossRef]
- Yang, X.; Huang, F. Three-dimensional failure mechanism of a rectangular cavity in a Hoek–Brown rock medium. Int. J. Rock Mech. Min. Sci. 2013, 61, 189–195. [Google Scholar] [CrossRef]
- Qin, C.; Yang, X.; Pan, Q.; Sun, Z.; Wang, L.; Miao, T. Upper bound analysis of progressive failure mechanism of tunnel roofs in partly weathered stratified Hoek–Brown rock masses. Int. J. Rock Mech. Min. Sci. 2015, 74, 157–162. [Google Scholar] [CrossRef]
- Qin, C.; Chian, S.C. 2D and 3D stability analysis of tunnel roof collapse in stratified rock: A kinematic approach. Int. J. Rock Mech. Min. Sci. 2017, 100, 269–277. [Google Scholar] [CrossRef]
- Yang, X.L.; Zhou, T.; Li, W.T. Reliability analysis of tunnel roof in layered Hoek-Brown rock masses. Comput. Geotech. 2018, 104, 302–309. [Google Scholar] [CrossRef]
- Chen, W. Limit Analysis and Soil Plast. Amsterdam; Elsevier: Amsterdam, The Netherlands, 1975. [Google Scholar]
- Huang, M.; Li, S.; Yu, J.; Tan, J.Q.W. Continuous field based upper bound analysis for three-dimensional tunnel face stability in undrained clay. Comput. Geotech. 2018, 94, 207–213. [Google Scholar] [CrossRef]
- Hoek, E.; Brown, E.T. Underground Excavations in Rock; Institute of Mining and Metallurgy: London, UK, 1980. [Google Scholar]
- Hoek, E. Empirical strength criterion for rock masses. J. Geotech. Eng. Div. ASCE 1980, 106, 1013–1035. [Google Scholar]
- Hoek, E.; Carranza-Torres, C.T.; Corkum, B. Hoek-Brown failure criterion-edition. In Proceedings of the 5th North American Rock Mechanics Symposium, Toronto, ON, Canada, 31 May–1 June 2002; pp. 267–273. [Google Scholar]
- Hoek, E.; Brown, E.T. Practical Estimates of Rock Mass Strength. Int. J. Rock Mech. Min. Sci. 1997, 34, 1165–1186. [Google Scholar] [CrossRef]
| A1 | B1 | σc1 /MPa | γ1 /kN·m−3 | L1 /m | L2 /m | L3 /m | L4 /m | h1 /m | h2 /m | h3 /m | W1 /103 kN |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.1 | 0.8 | 8 | 20 | 1.88 | 0.37 | 1.92 | 0.44 | 6.65 | 6.75 | 6.51 | 0.34 |
| 0.2 | 0.8 | 8 | 20 | 3.72 | 0.69 | 3.85 | 0.92 | 6.71 | 6.94 | 6.43 | 0.67 |
| 0.3 | 0.8 | 8 | 20 | 5.53 | 0.98 | 5.78 | 1.49 | 6.76 | 7.14 | 6.32 | 1.01 |
| 0.4 | 0.8 | 8 | 20 | 7.33 | 1.35 | 7.70 | 2.44 | 6.82 | 7.43 | 6.16 | 1.38 |
| 0.5 | 0.8 | 8 | 20 | 9.16 | 2.23 | 9.98 | 5.41 | 6.98 | 8.23 | 5.63 | 2.11 |
| 0.4 | 0.76 | 8 | 20 | 8.80 | 2.42 | 9.23 | 4.49 | 7.01 | 8.42 | 5.79 | 1.94 |
| 0.4 | 0.77 | 8 | 20 | 8.40 | 2.05 | 8.83 | 3.88 | 6.95 | 8.06 | 5.90 | 1.76 |
| 0.4 | 0.78 | 8 | 20 | 8.03 | 1.75 | 8.44 | 3.33 | 6.90 | 7.78 | 6.00 | 1.61 |
| 0.4 | 0.79 | 8 | 20 | 7.67 | 1.53 | 8.07 | 2.85 | 6.86 | 7.58 | 6.09 | 1.48 |
| 0.4 | 0.8 | 7 | 20 | 6.02 | 0.17 | 6.07 | 0.29 | 6.62 | 6.65 | 6.54 | 0.92 |
| 0.4 | 0.8 | 8.5 | 20 | 7.85 | 1.86 | 8.22 | 3.18 | 6.91 | 7.88 | 6.03 | 1.58 |
| 0.4 | 0.8 | 9 | 20 | 8.32 | 2.32 | 8.63 | 3.77 | 7.00 | 8.32 | 5.92 | 1.76 |
| 0.4 | 0.8 | 10 | 20 | 9.10 | 3.12 | 9.29 | 4.71 | 7.14 | 9.16 | 5.76 | 2.10 |
| 0.4 | 0.8 | 8 | 19 | 7.84 | 1.85 | 8.31 | 3.32 | 6.91 | 7.87 | 6.00 | 1.59 |
| 0.4 | 0.8 | 8 | 21 | 6.86 | 0.91 | 7.10 | 1.59 | 6.75 | 7.09 | 6.31 | 1.22 |
| 0.4 | 0.8 | 8 | 22 | 6.43 | 0.53 | 6.55 | 0.86 | 6.68 | 6.84 | 6.43 | 1.11 |
| 0.4 | 0.8 | 8 | 23 | 6.07 | 0.22 | 6.10 | 0.33 | 6.63 | 6.67 | 6.53 | 1.04 |
| A2 | B2 | σc2 /MPa | γ2 /kN·m−3 | L1 /m | L2 /m | L3 /m | L4 /m | h1 /m | h2 /m | h3 /m | W1 /103 kN |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.28 | 0.7 | 10 | 21 | 7.32 | 1.43 | 7.67 | 2.64 | 6.84 | 7.57 | 6.12 | 1.40 |
| 0.32 | 0.7 | 10 | 21 | 7.33 | 1.30 | 7.73 | 2.33 | 6.82 | 7.34 | 6.18 | 1.37 |
| 0.34 | 0.7 | 10 | 21 | 7.34 | 1.27 | 7.76 | 2.27 | 6.81 | 7.28 | 6.19 | 1.36 |
| 0.36 | 0.7 | 10 | 21 | 7.34 | 1.26 | 7.78 | 2.23 | 6.81 | 7.23 | 6.19 | 1.36 |
| 0.3 | 0.4 | 10 | 21 | 7.41 | 1.26 | 8.44 | 2.81 | 6.81 | 6.81 | 6.09 | 1.41 |
| 0.3 | 0.5 | 10 | 21 | 7.40 | 1.25 | 8.29 | 2.64 | 6.81 | 6.84 | 6.12 | 1.40 |
| 0.3 | 0.6 | 10 | 21 | 7.38 | 1.24 | 8.01 | 2.34 | 6.80 | 6.98 | 6.17 | 1.37 |
| 0.3 | 0.65 | 10 | 21 | 7.36 | 1.24 | 7.86 | 2.26 | 6.81 | 7.13 | 6.19 | 1.36 |
| 0.3 | 0.7 | 8 | 21 | 6.98 | 1.03 | 7.04 | 1.56 | 6.77 | 7.23 | 6.31 | 1.21 |
| 0.3 | 0.7 | 9 | 21 | 7.13 | 1.17 | 7.31 | 1.91 | 6.79 | 7.31 | 6.25 | 1.28 |
| 0.3 | 0.7 | 11 | 21 | 7.57 | 1.58 | 8.28 | 3.22 | 6.87 | 7.60 | 6.02 | 1.53 |
| 0.3 | 0.7 | 12 | 21 | 7.89 | 1.89 | 9.05 | 4.28 | 6.92 | 7.83 | 5.83 | 1.76 |
| 0.3 | 0.7 | 10 | 22 | 7.32 | 1.35 | 7.68 | 2.45 | 6.83 | 7.45 | 6.16 | 1.43 |
| 0.3 | 0.7 | 10 | 23 | 7.31 | 1.36 | 7.66 | 2.45 | 6.83 | 7.46 | 6.16 | 1.48 |
| 0.3 | 0.7 | 10 | 24 | 7.30 | 1.36 | 7.63 | 2.45 | 6.83 | 7.48 | 6.15 | 1.53 |
| 0.3 | 0.7 | 10 | 25 | 7.29 | 1.37 | 7.61 | 2.46 | 6.83 | 7.50 | 6.15 | 1.58 |
| A1 | B1 | σc1 /MPa | γ1 /kN·m−3 | L1 /m | L2 /m | L3 /m | L4 /m | h1 /m | h2 /m | h3 /m | W2 /103 kN |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.10 | 0.8 | 18 | 18 | 2.23 | 1.20 | 1.91 | 0.87 | 3.38 | 4.96 | 3.35 | 0.71 |
| 0.11 | 0.8 | 18 | 18 | 2.36 | 1.22 | 2.04 | 0.90 | 3.41 | 5.03 | 3.40 | 0.74 |
| 0.12 | 0.8 | 18 | 18 | 2.50 | 1.23 | 2.24 | 0.96 | 3.45 | 5.11 | 3.48 | 0.79 |
| 0.13 | 0.8 | 18 | 18 | 2.59 | 1.23 | 2.42 | 1.02 | 3.48 | 5.16 | 3.56 | 0.83 |
| 0.14 | 0.8 | 18 | 18 | 2.74 | 1.25 | 2.62 | 1.09 | 3.52 | 5.25 | 3.65 | 0.87 |
| 0.10 | 0.72 | 18 | 18 | 2.88 | 1.29 | 2.88 | 1.23 | 3.57 | 5.43 | 3.77 | 0.94 |
| 0.10 | 0.74 | 18 | 18 | 2.71 | 1.26 | 2.55 | 1.08 | 3.52 | 5.29 | 3.61 | 0.86 |
| 0.10 | 0.76 | 18 | 18 | 2.55 | 1.24 | 2.32 | 0.99 | 3.47 | 5.17 | 3.51 | 0.81 |
| 0.10 | 0.78 | 18 | 18 | 2.40 | 1.22 | 2.10 | 0.92 | 3.43 | 5.07 | 3.42 | 0.76 |
| 0.10 | 0.8 | 17 | 18 | 1.97 | 0.99 | 1.52 | 0.55 | 3.29 | 4.56 | 3.26 | 0.57 |
| 0.10 | 0.8 | 19 | 18 | 2.03 | 1.04 | 1.61 | 0.63 | 3.31 | 4.66 | 3.28 | 0.60 |
| 0.10 | 0.8 | 20 | 18 | 2.11 | 1.10 | 1.71 | 0.70 | 3.34 | 4.76 | 3.30 | 0.64 |
| 0.10 | 0.8 | 21 | 18 | 2.18 | 1.15 | 1.81 | 0.78 | 3.36 | 4.87 | 3.33 | 0.67 |
| 0.10 | 0.8 | 18 | 17 | 2.29 | 1.23 | 1.98 | 0.92 | 3.40 | 5.02 | 3.37 | 0.72 |
| 0.10 | 0.8 | 18 | 19 | 2.23 | 1.19 | 1.85 | 0.82 | 3.38 | 4.95 | 3.33 | 0.70 |
| 0.10 | 0.8 | 18 | 20 | 2.20 | 1.18 | 1.79 | 0.78 | 3.37 | 4.91 | 3.32 | 0.69 |
| 0.10 | 0.8 | 18 | 21 | 2.18 | 1.16 | 1.74 | 0.74 | 3.36 | 4.88 | 3.31 | 0.68 |
| A2 | B2 | σc2 /MPa | γ2 /kN·m−3 | L1 /m | L2 /m | L3 /m | L4 /m | h1 /m | h2 /m | h3 /m | W2 /103 kN |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.2 | 0.9 | 10 | 25 | 1.90 | 1.08 | 1.61 | 0.76 | 3.29 | 6.83 | 3.26 | 0.66 |
| 0.3 | 0.9 | 10 | 25 | 2.14 | 1.14 | 1.80 | 0.81 | 3.35 | 5.84 | 3.32 | 0.69 |
| 0.4 | 0.9 | 10 | 25 | 2.25 | 1.19 | 1.87 | 0.85 | 3.38 | 5.34 | 3.34 | 0.71 |
| 0.6 | 0.9 | 10 | 25 | 2.29 | 1.23 | 1.93 | 0.88 | 3.40 | 4.76 | 3.36 | 0.71 |
| 0.5 | 0.5 | 10 | 25 | 2.41 | 1.34 | 2.02 | 0.96 | 3.45 | 3.65 | 3.38 | 0.724 |
| 0.5 | 0.6 | 10 | 25 | 2.41 | 1.33 | 2.01 | 0.96 | 3.45 | 3.72 | 3.38 | 0.723 |
| 0.5 | 0.7 | 10 | 25 | 2.38 | 1.31 | 2.00 | 0.94 | 3.44 | 3.91 | 3.37 | 0.723 |
| 0.5 | 0.8 | 10 | 25 | 2.33 | 1.27 | 1.97 | 0.92 | 3.42 | 4.32 | 3.37 | 0.720 |
| 0.5 | 0.9 | 21 | 25 | 2.01 | 0.99 | 1.69 | 0.66 | 3.30 | 4.64 | 3.30 | 0.61 |
| 0.5 | 0.9 | 22 | 25 | 2.07 | 1.04 | 1.73 | 0.70 | 3.32 | 4.71 | 3.31 | 0.63 |
| 0.5 | 0.9 | 23 | 25 | 2.13 | 1.09 | 1.78 | 0.75 | 3.34 | 4.79 | 3.32 | 0.66 |
| 0.5 | 0.9 | 24 | 25 | 2.19 | 1.15 | 1.84 | 0.80 | 3.36 | 4.88 | 3.34 | 0.68 |
| 0.5 | 0.9 | 10 | 21 | 2.52 | 1.44 | 2.17 | 1.11 | 3.50 | 5.41 | 3.42 | 0.715 |
| 0.5 | 0.9 | 10 | 22 | 2.45 | 1.38 | 2.09 | 1.04 | 3.46 | 5.29 | 3.40 | 0.714 |
| 0.5 | 0.9 | 10 | 23 | 2.38 | 1.32 | 2.02 | 0.97 | 3.44 | 5.18 | 3.38 | 0.713 |
| 0.5 | 0.9 | 10 | 24 | 2.32 | 1.26 | 1.96 | 0.92 | 3.41 | 5.08 | 3.36 | 0.712 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lyu, C.; Zeng, Z.; Dong, Y. Limit Analysis of Progressive Asymmetrical Collapse Failure of Tunnels in Inclined Rock Stratum. Symmetry 2019, 11, 904. https://doi.org/10.3390/sym11070904
Lyu C, Zeng Z, Dong Y. Limit Analysis of Progressive Asymmetrical Collapse Failure of Tunnels in Inclined Rock Stratum. Symmetry. 2019; 11(7):904. https://doi.org/10.3390/sym11070904
Chicago/Turabian StyleLyu, Cheng, Zhengqiang Zeng, and Yucang Dong. 2019. "Limit Analysis of Progressive Asymmetrical Collapse Failure of Tunnels in Inclined Rock Stratum" Symmetry 11, no. 7: 904. https://doi.org/10.3390/sym11070904
APA StyleLyu, C., Zeng, Z., & Dong, Y. (2019). Limit Analysis of Progressive Asymmetrical Collapse Failure of Tunnels in Inclined Rock Stratum. Symmetry, 11(7), 904. https://doi.org/10.3390/sym11070904




