Observing System Simulation Experiment to Reproduce Kelvin Wave in the Venus Atmosphere
Abstract

1. Introduction
2. Experiments
3. Results
3.1. Zonal Mean Field
3.2. Time Evolution
3.3. Composite Means
3.4. Requirements for the Reproduction of Kelvin-Type Waves
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Time/Altitude | h1 | h2 | h4 | h6 | h12 | h24 |
|---|---|---|---|---|---|---|
| 30 km | z30l90h1 | z30l90h2 | z30l90h4 | z30l90h6 | z30l90h12 | z30l90h24 |
| 40 km | z40l90h1 | z40l90h2 | z40l90h4 | z40l90h6 | z40l90h12 | z40l90h24 |
| 50 km | z50l90h1 | z50l90h2 | z50l90h4 | z50l90h6 | z50l90h12 | z50l90h24 |
| 60 km | z60l90h1 | z60l90h2 | z60l90h4 | z60l90h6 | z60l90h12 | z60l90h24 |
| 70 km | z70l90h1 | z70l90h2 | z70l90h4 | z70l90h6 | z70l90h12 | z70l90h24 |
| others | frf | ym-dat | - | - | - | - |

Appendix B

References
- Sánchez-Lavega, A.; Lebonnois, S.; Imamura, T.; Read, P.L.; Luz, D. The Atmospheric Dynamics of Venus. Space Sci. Rev. 2017, 212, 1541–1616. [Google Scholar] [CrossRef]
- Read, P.L.; Lebonnois, S. Superrotation on Venus, on Titan, and Elsewhere. Ann. Rev. Earth Planet. Sci. 2018, 46, 175–202. [Google Scholar] [CrossRef]
- Belton, M.J.S.; Smith, G.R.; Schubert, G.; Del Genio, A.D. Cloud patterns, waves and convection in the Venus atmosphere. J. Atmos. Sci. 1976, 33, 1394–1417. [Google Scholar] [CrossRef][Green Version]
- Del Genio, A.D.; Rossow, W.B. Planetary-scale waves and the cyclic nature of cloud top dynamics on Venus. J. Atmos. Sci. 1990, 47, 293–318. [Google Scholar] [CrossRef]
- Kouyama, T.; Imamura, T.; Nakamura, M.; Satoh, T.; Futaana, Y. Horizontal structure of planetary-scale waves at the cloud top of Venus deduced from Galileo SSI images with an improved cloud-tracking technique. Planet. Space Sci. 2012, 60, 207–216. [Google Scholar] [CrossRef]
- Khatuntsev, I.V.; Patsaeva, M.V.; Titov, D.V.; Ignatiev, N.I.; Turin, A.V.; Limaye, S.S.; Markiewicz, W.J.; Almeida, M.; Roatsch, T.; Moissl, R. Cloud level winds from the Venus Express Monitoring Camera imaging. Icarus 2013, 226, 140–158. [Google Scholar] [CrossRef]
- Peralta, J.; Sánchez-Lavega, A.; López-Valverde, M.A.; Luz, D.; Machado, P. Venus’s major cloud feature as an equatorially trapped wave distorted by the wind. Geophys. Res. Lett. 2015, 42, 705–711. [Google Scholar] [CrossRef]
- Hosouchi, M.; Kouyama, T.; Iwagami, N.; Ohtsuki, S.; Takagi, M. Wave signature in the Venus dayside cloud layer at 58–64 km observed by ground-based infrared spectroscopy. Icarus 2012, 220, 552–560. [Google Scholar] [CrossRef]
- Gierasch, P.J. Meridional circulation and maintenance of the Venus atmospheric rotation. J. Atmos. Sci. 1975, 32, 1038–1044. [Google Scholar] [CrossRef]
- Matsuda, Y. Dynamics of the four-day circulation in the Venus atmosphere. J. Meteorol. Soc. Jpn. 1980, 58, 443–470. [Google Scholar] [CrossRef]
- Sugimoto, N.; Takagi, M.; Matsuda, Y. Baroclinic instability in the Venus atmosphere simulated by GCM. J. Geophys. Res. 2014, 119, 1950–1968. [Google Scholar] [CrossRef]
- Ohfuchi, W.; Nakamura, H.; Yoshioka, M.K.; Enomoto, T.; Takaya, K.; Peng, X.; Yamane, S.; Nishimura, T.; Kurihara, Y.; Ninomiya, K. 10-km Mesh Meso-scale Resolving Simulations of the Global Atmosphere on the Earth Simulator, -Preliminary Outcomes of AFES (AGCM for the Earth Simulator). J. Earth Simulator 2004, 1, 8–34. [Google Scholar]
- Sugimoto, N.; Takagi, M.; Matsuda, Y. Waves in a Venus general circulation model. Geophys. Res. Lett. 2014, 41, 7461–7467. [Google Scholar] [CrossRef]
- Sugimoto, N.; Takagi, M.; Matsuda, Y. Fully developed super-rotation driven by the mean meridional circulation in a Venus GCM. Geophys. Res. Lett. 2019, 46, 1776–1784. [Google Scholar] [CrossRef]
- Ando, H.; Sugimoto, N.; Takagi, M.; Kashimura, H.; Imamura, T.; Matsuda, Y. The puzzling Venusian polar atmospheric structure reproduced by a general circulation model. Nat. Commun. 2016, 7, 10398. [Google Scholar] [CrossRef] [PubMed]
- Takagi, M.; Sugimoto, N.; Ando, H.; Matsuda, Y. Three dimensional structures of thermal tides simulated by a Venus GCM. J. Geophys. Res. Planets 2018, 123, 335–352. [Google Scholar] [CrossRef]
- Ando, H.; Takagi, M.; Fukuhara, T.; Imamura, T.; Sugimoto, N.; Sagawa, H.; Noguchi, K.; Tellmann, S.; Pätzold, M.; Häusler, B.; et al. Local time dependence of the thermal structure in the Venusian equatorial upper atmosphere: Comparison of Akatsuki radio occultation measurements and GCM results. J. Geophys. Res. Planets 2018, 123, 2970–2980. [Google Scholar] [CrossRef]
- Kashimura, H.; Sugimoto, N.; Takagi, M.; Matsuda, Y.; Ohfuchi, W.; Enomoto, T.; Nakajima, K.; Ishiwatari, M.; Sato, T.M.; Hashimoto, G.L.; et al. Planetary-scale streak structure reproduced in a Venus atmospheric simulation. Nat. Commun. 2019, 10, 23. [Google Scholar] [CrossRef]
- Yamamoto, M.; Takahashi, M. Venusian middle-atmospheric dynamics in the presence of a strong planetary-scale 5.5-day wave. Icarus 2012, 217, 702–713. [Google Scholar] [CrossRef]
- Crisp, D.; McMuldroch, S.; Stephens, S.K.; Sinton, W.M.; Ragent, B.; Hodapp, K.W.; Probst, R.G.; Doyle, L.R.; Allen, D.A.; Elias, J. Ground-Based Near-Infrared Imaging Observations of Venus During the Galileo Encounter. Science 1991, 253, 1538–1541. [Google Scholar] [CrossRef]
- Kalnay, E.; Kanamitsu, M.; Kistler, R.; Collins, W.; Deaven, D.; Gandin, L.; Iredell, M.; Saha, S.; White, G.; Woollen, J.; et al. The NCEP/NCAR 40-Year Reanalysis Project. Bull. Am. Meteorol. Soc. 1996, 77, 437–471. [Google Scholar] [CrossRef]
- Sugimoto, N.; Yamazaki, A.; Kouyama, T.; Kashimura, H.; Enomoto, T.; Takagi, M. Development of an ensemble Kalman filter data assimilation system for the Venusian atmosphere. Sci. Rep. 2017, 7, 9321. [Google Scholar] [CrossRef] [PubMed]
- Sugimoto, N.; Kouyama, T.; Takagi, M. Impact of data assimilation on thermal tides in the case of Venus Express wind observation. Geophys. Res. Lett. 2019, 46, 4573–4580. [Google Scholar] [CrossRef]
- Sugimoto, N.; Abe, M.; Kikuchi, Y.; Hosono, A.; Ando, H.; Takagi, M.; Garate-Lopez, I.; Lebonnois, S.; Ao, C. Observing system simulation experiment for radio occultation measurements of the Venus atmosphere among small satellites. J. Jpn. Soc. Civ. Eng. Ser. A2 (Appl. Mech.) 2019, 75, 477–486. [Google Scholar] [CrossRef]
- Tomasko, M.G.; Doose, L.R.; Smith, P.H.; Odell, A.P. Measurements of the flux of sunlight in the atmosphere of Venus. J. Geophys. Res. 1980, 85, 8167–8186. [Google Scholar] [CrossRef]
- Crisp, D. Radiative forcing of the Venus mesosphere: I. solar fluxes and heating rates. Icarus 1986, 67, 484–514. [Google Scholar] [CrossRef]
- Miyoshi, T.; Yamane, S. Local ensemble transform Kalman filtering with an AGCM at a T159/L48 resolution. Mon. Weather Rev. 2007, 135, 3841–3861. [Google Scholar] [CrossRef]
- Kouyama, T.; Imamura, T.; Nakamura, M.; Satoh, T.; Futaana, Y. Long-term variation in the cloud-tracked zonal velocities at the cloud top of Venus deduced from Venus Express VMC images. J. Geophys. Res. 2013, 118, 37–46. [Google Scholar] [CrossRef]
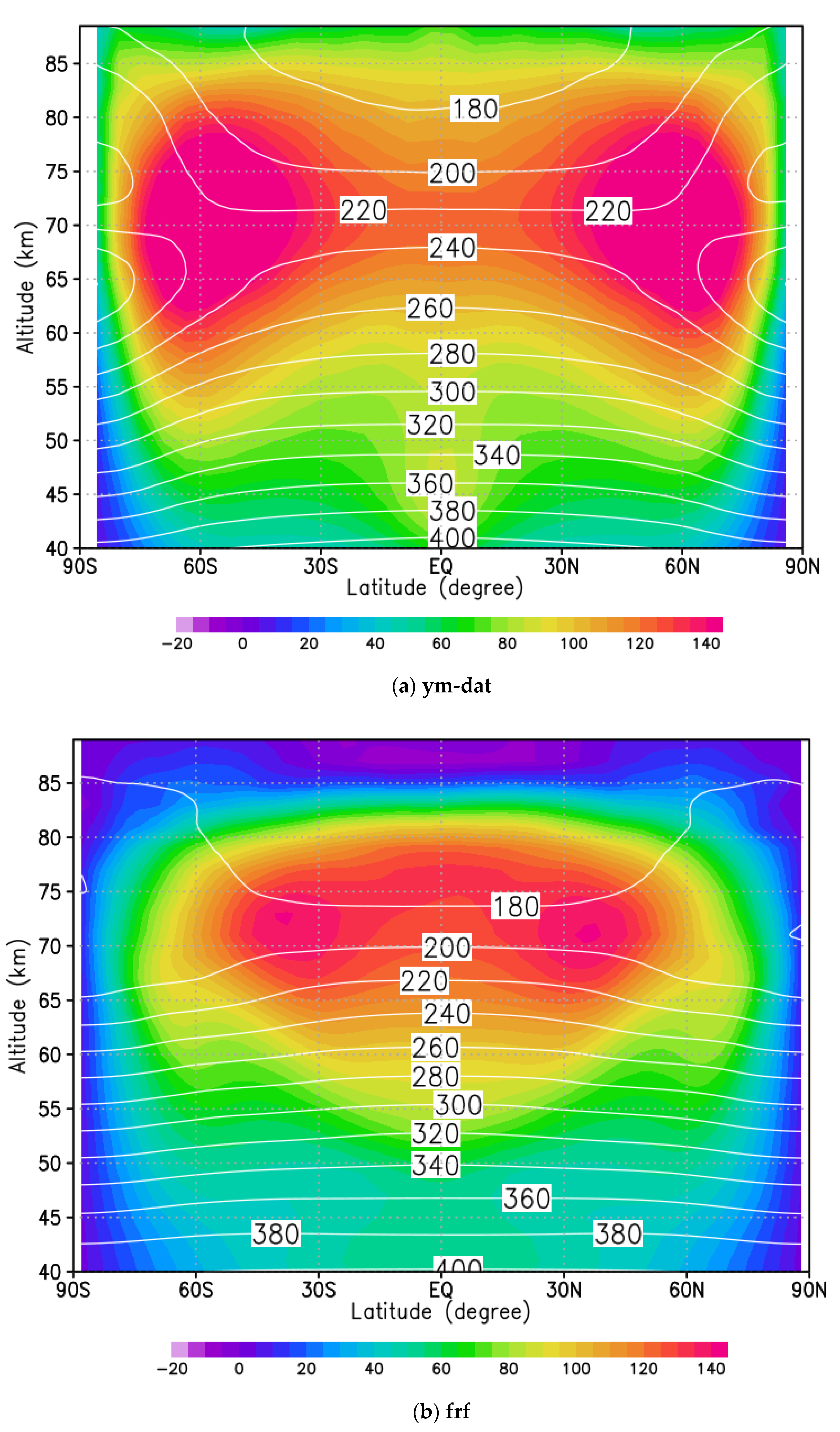
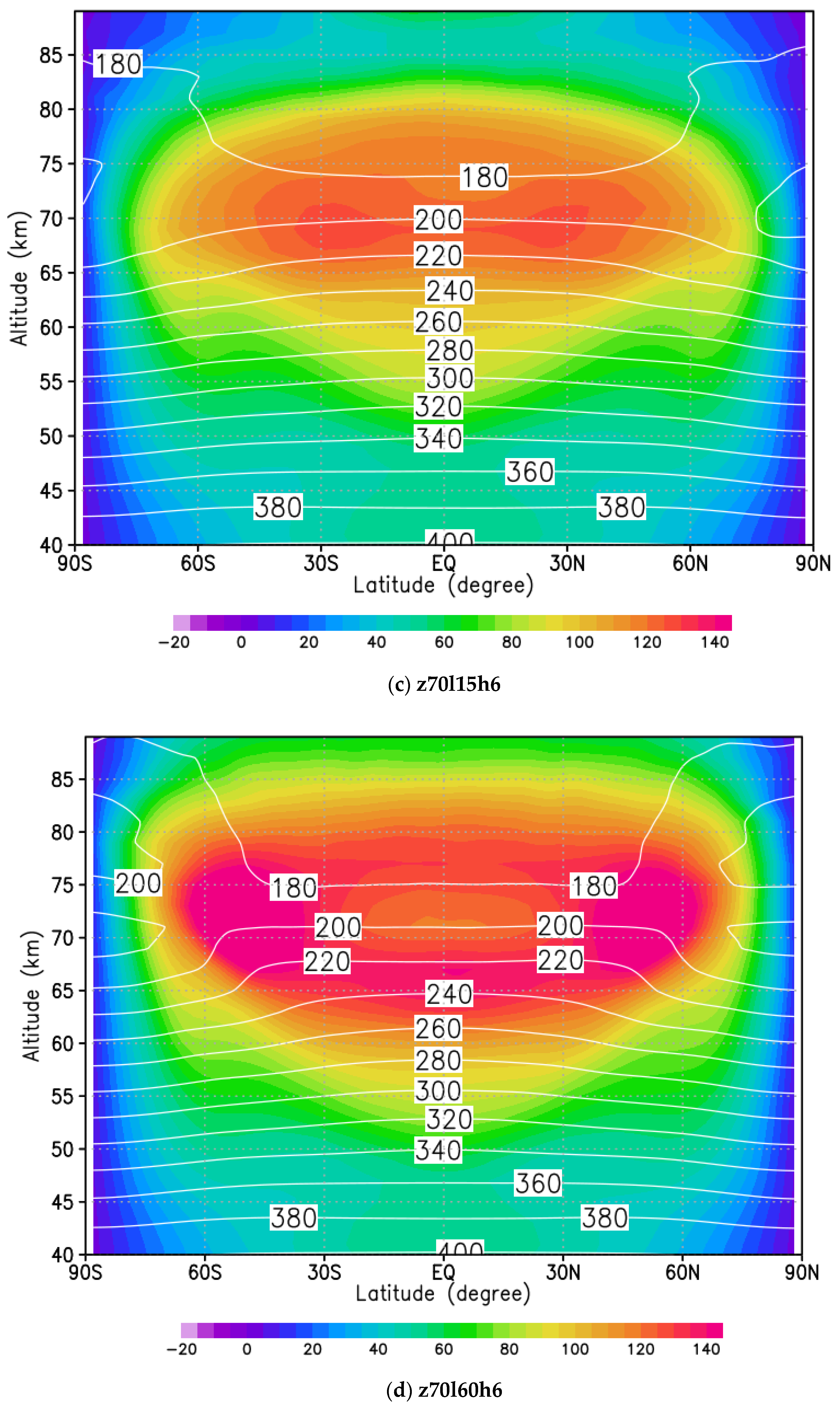
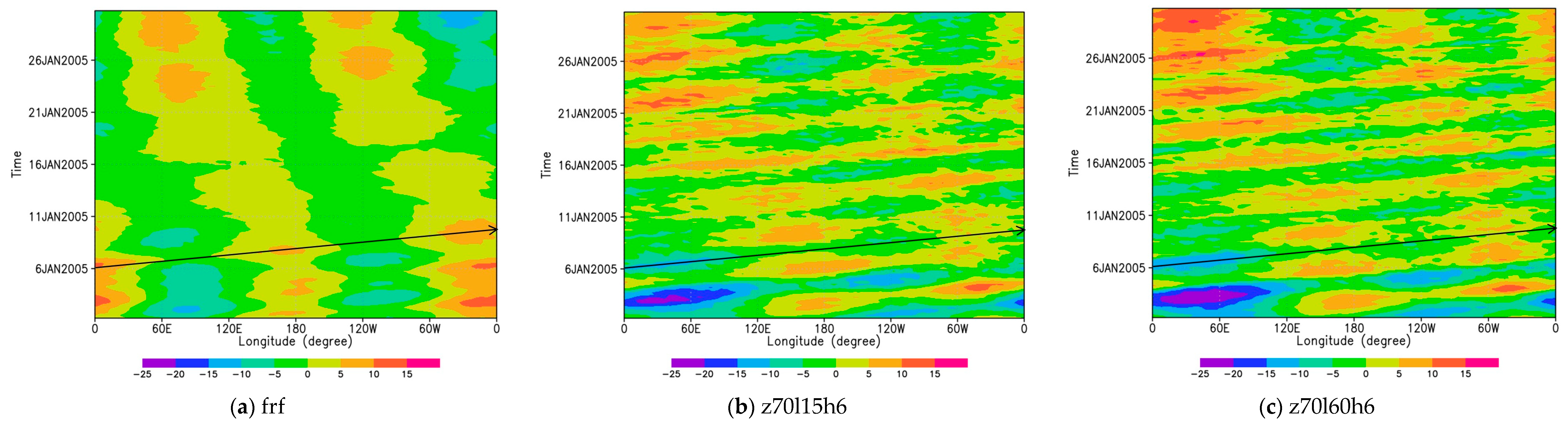

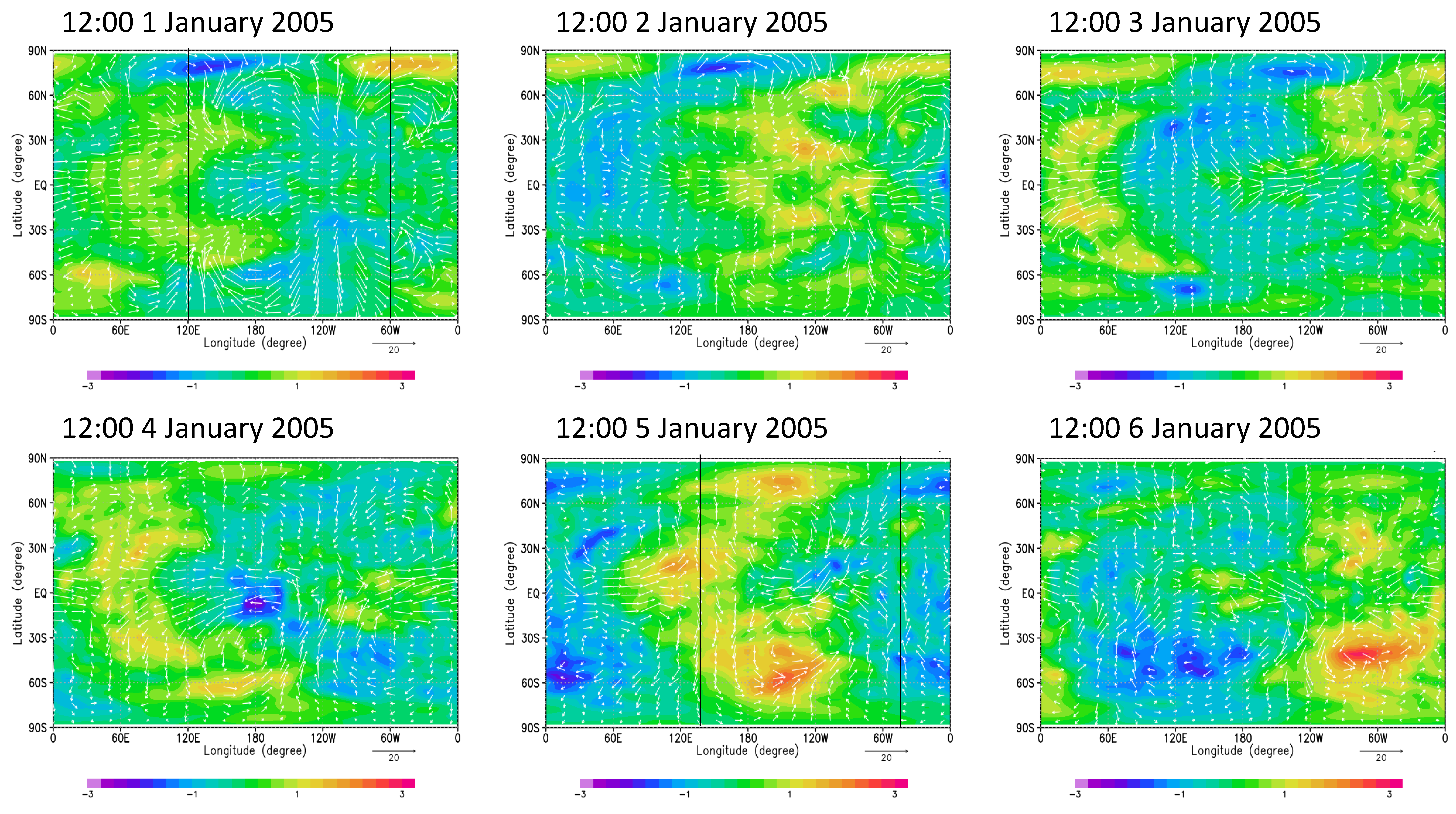
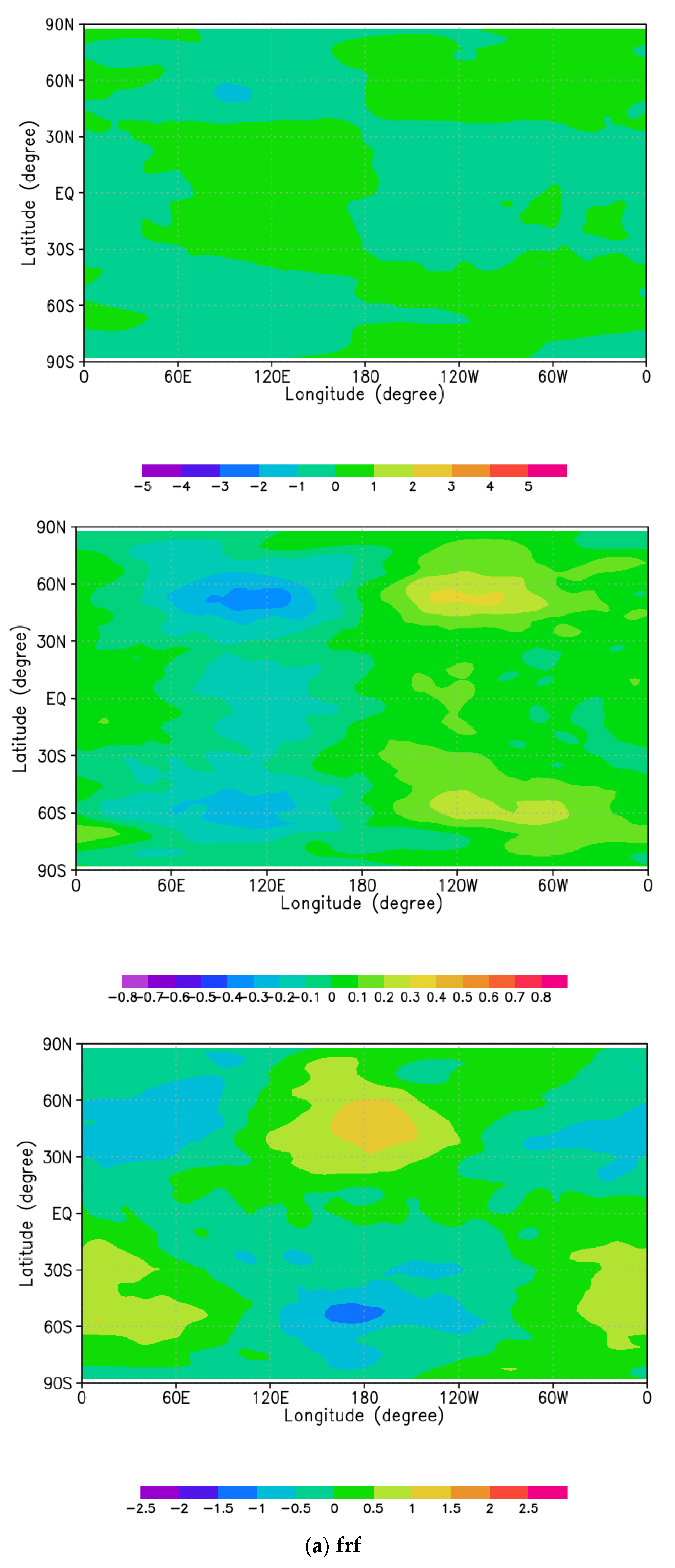
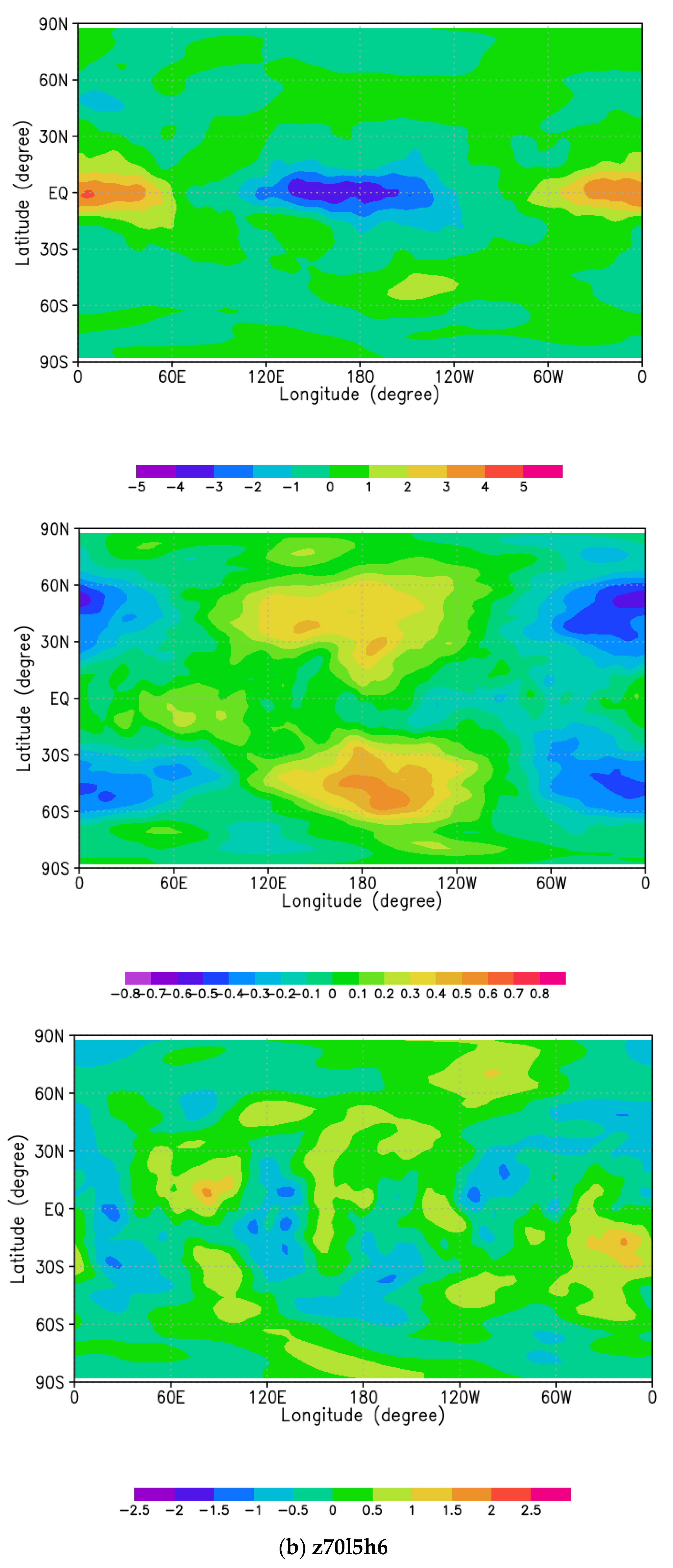


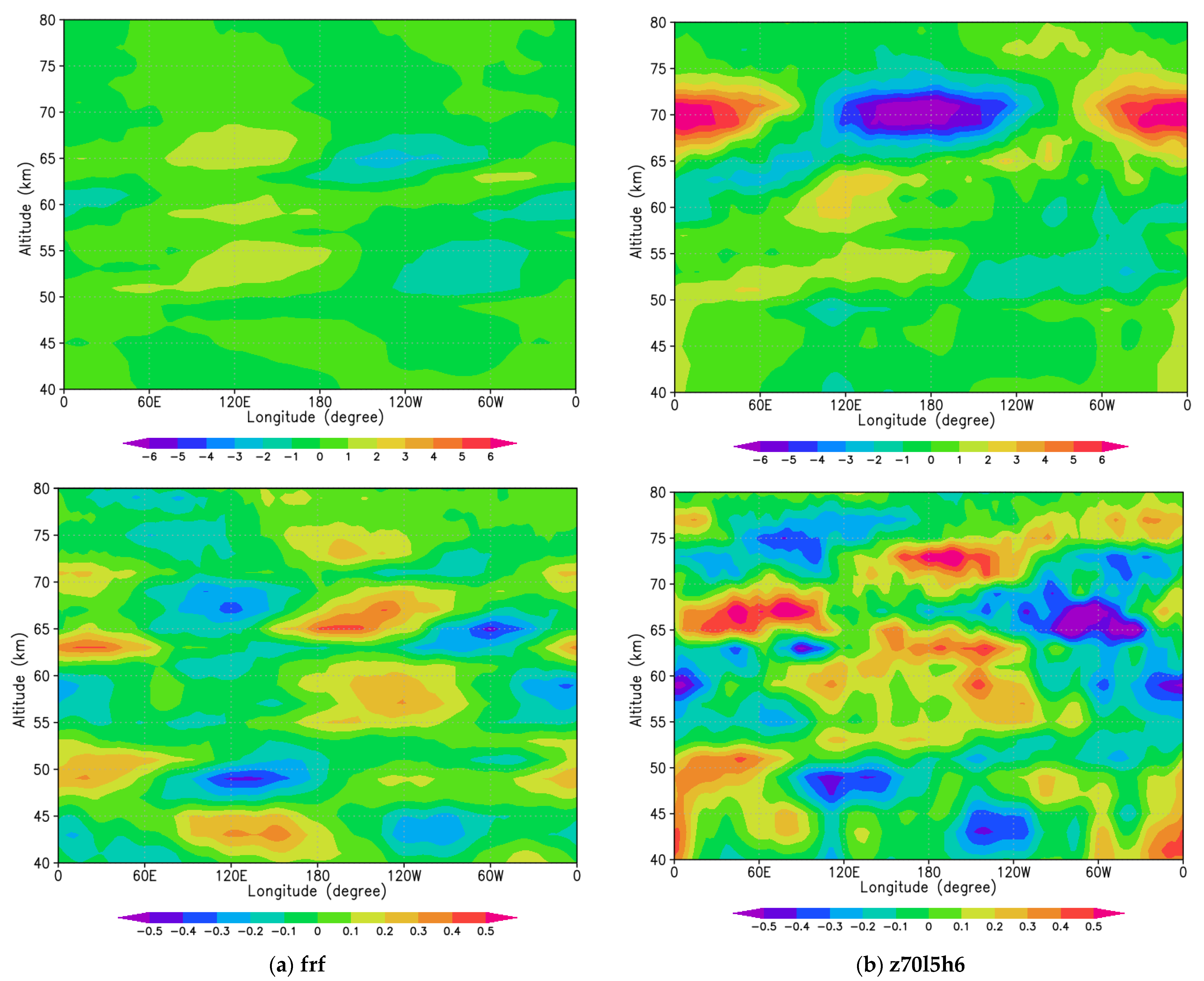


| Time/Latitude | h6 | h12 | h24 |
|---|---|---|---|
| l5 | z70l5h6 | z70l5h12 | z70l5h24 |
| l15 | z70l15h6 | z70l15h12 | z70l15h24 |
| l30 | z70l30h6 | z70l30h12 | z70l30h24 |
| l60 | z70l60h6 | z70l60h12 | z70l60h24 |
| l90 | z70l90h6 | z70l90h12 | z70l90h24 |
| others | frf | ym-dat |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sugimoto, N.; Fujisawa, Y.; Shirasaka, M.; Hosono, A.; Abe, M.; Ando, H.; Takagi, M.; Yamamoto, M. Observing System Simulation Experiment to Reproduce Kelvin Wave in the Venus Atmosphere. Atmosphere 2021, 12, 14. https://doi.org/10.3390/atmos12010014
Sugimoto N, Fujisawa Y, Shirasaka M, Hosono A, Abe M, Ando H, Takagi M, Yamamoto M. Observing System Simulation Experiment to Reproduce Kelvin Wave in the Venus Atmosphere. Atmosphere. 2021; 12(1):14. https://doi.org/10.3390/atmos12010014
Chicago/Turabian StyleSugimoto, Norihiko, Yukiko Fujisawa, Mimo Shirasaka, Asako Hosono, Mirai Abe, Hiroki Ando, Masahiro Takagi, and Masaru Yamamoto. 2021. "Observing System Simulation Experiment to Reproduce Kelvin Wave in the Venus Atmosphere" Atmosphere 12, no. 1: 14. https://doi.org/10.3390/atmos12010014
APA StyleSugimoto, N., Fujisawa, Y., Shirasaka, M., Hosono, A., Abe, M., Ando, H., Takagi, M., & Yamamoto, M. (2021). Observing System Simulation Experiment to Reproduce Kelvin Wave in the Venus Atmosphere. Atmosphere, 12(1), 14. https://doi.org/10.3390/atmos12010014





