A Wind Study of Venus’s Cloud Top: New Doppler Velocimetry Observations †
Abstract
1. Introduction
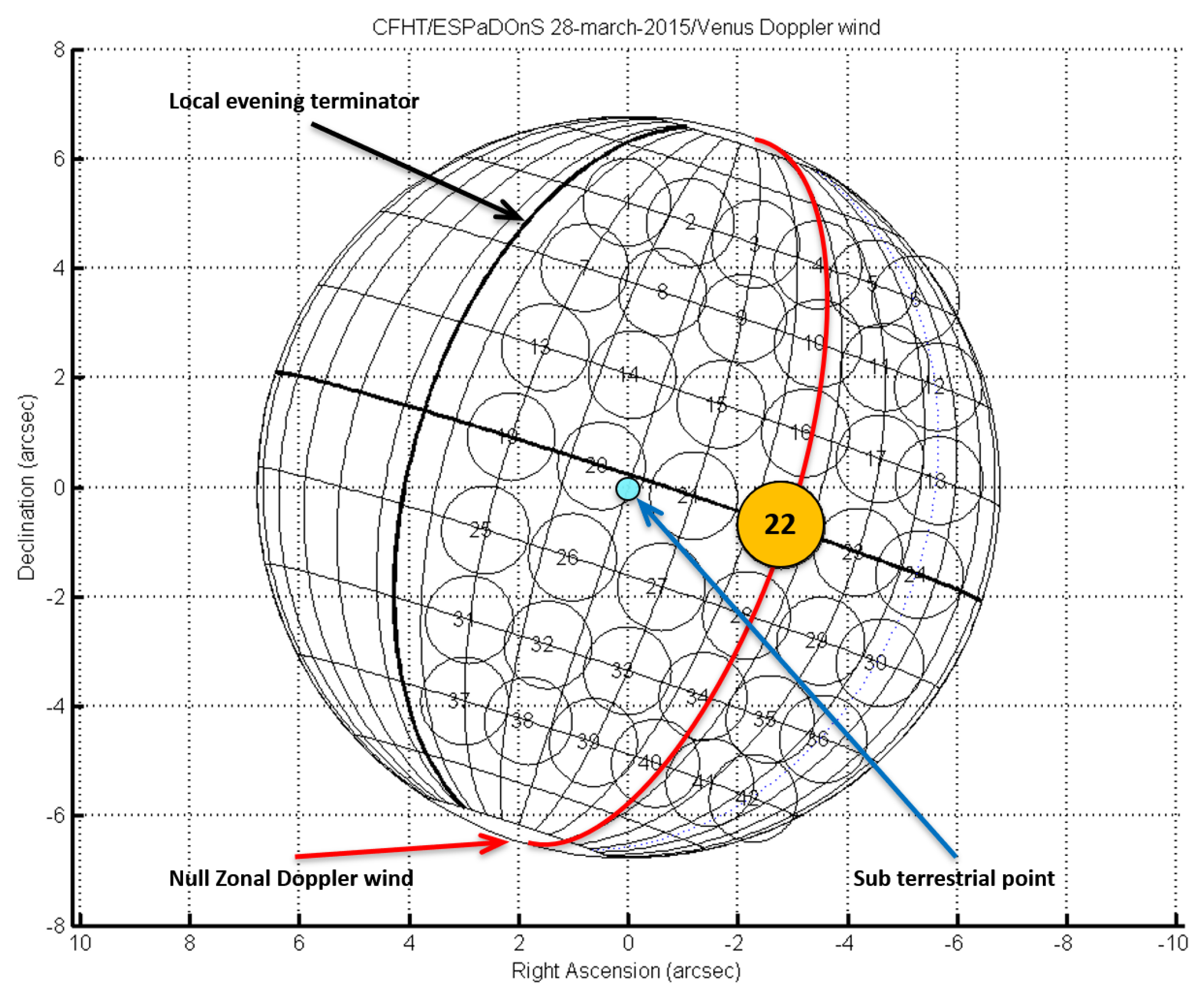
2. Observations
3. Doppler Velocimetry Technique
3.1. Summary
3.2. Altitude Probed
3.3. Measurement Errors
3.4. Young Effect
4. Results
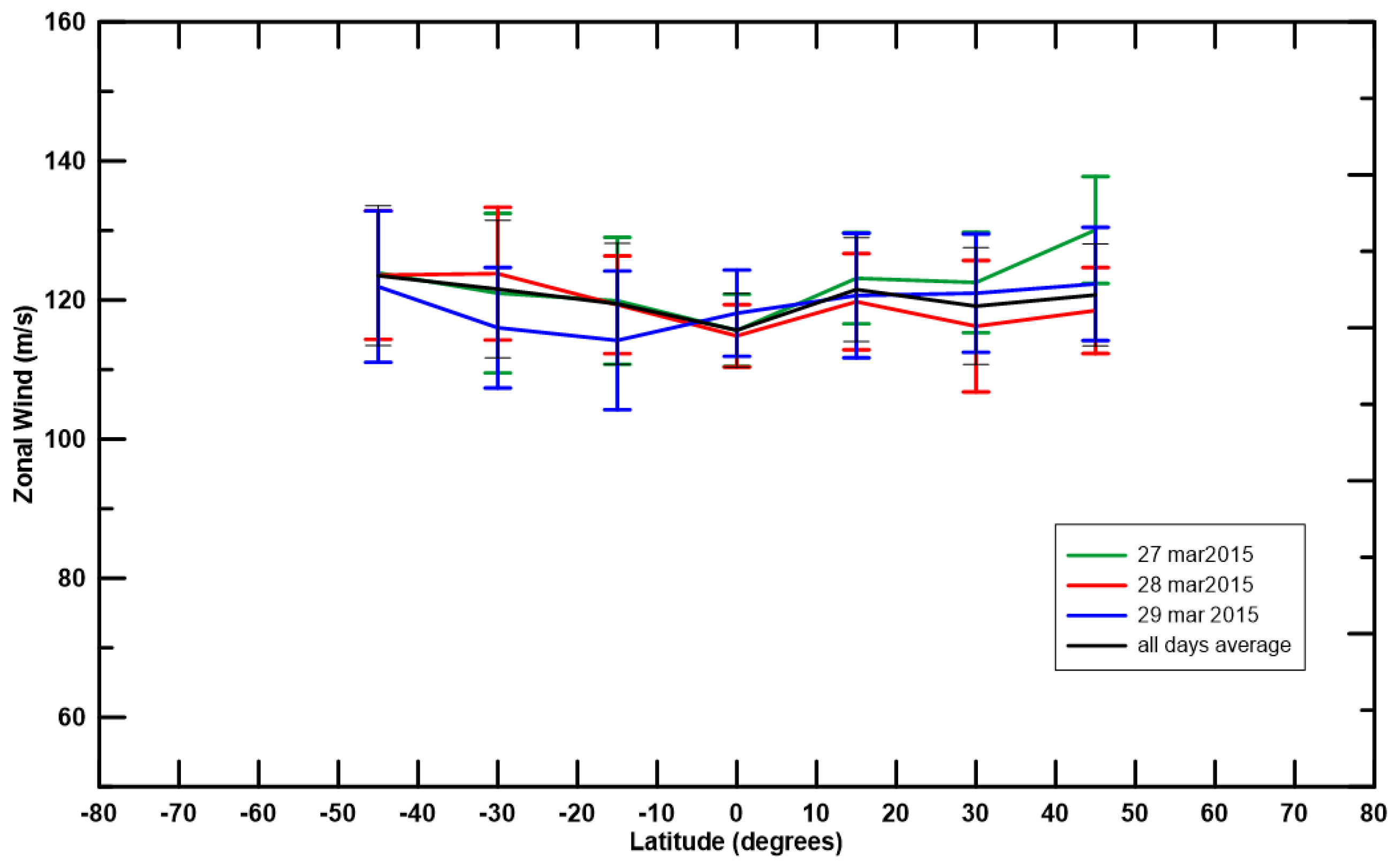
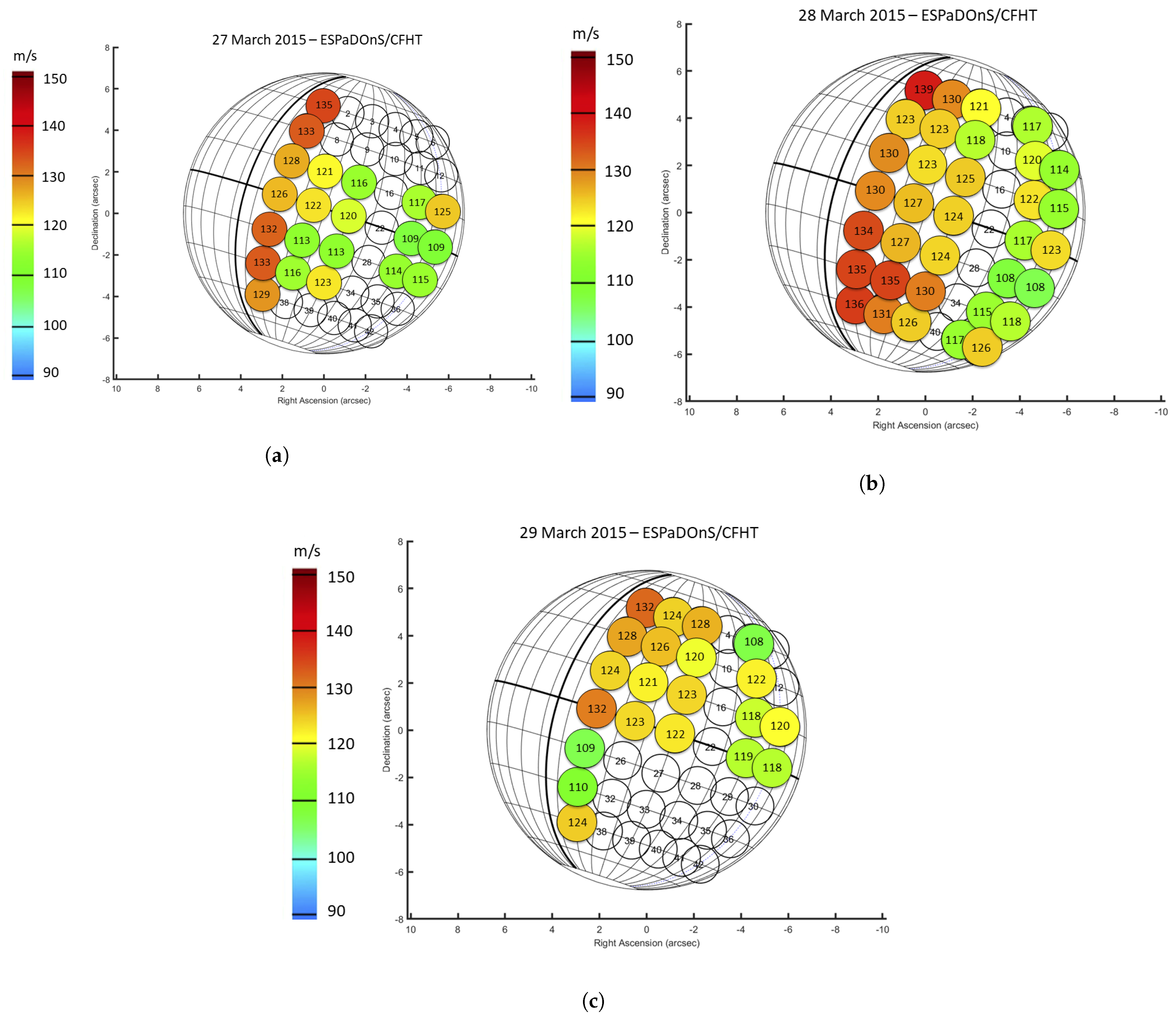
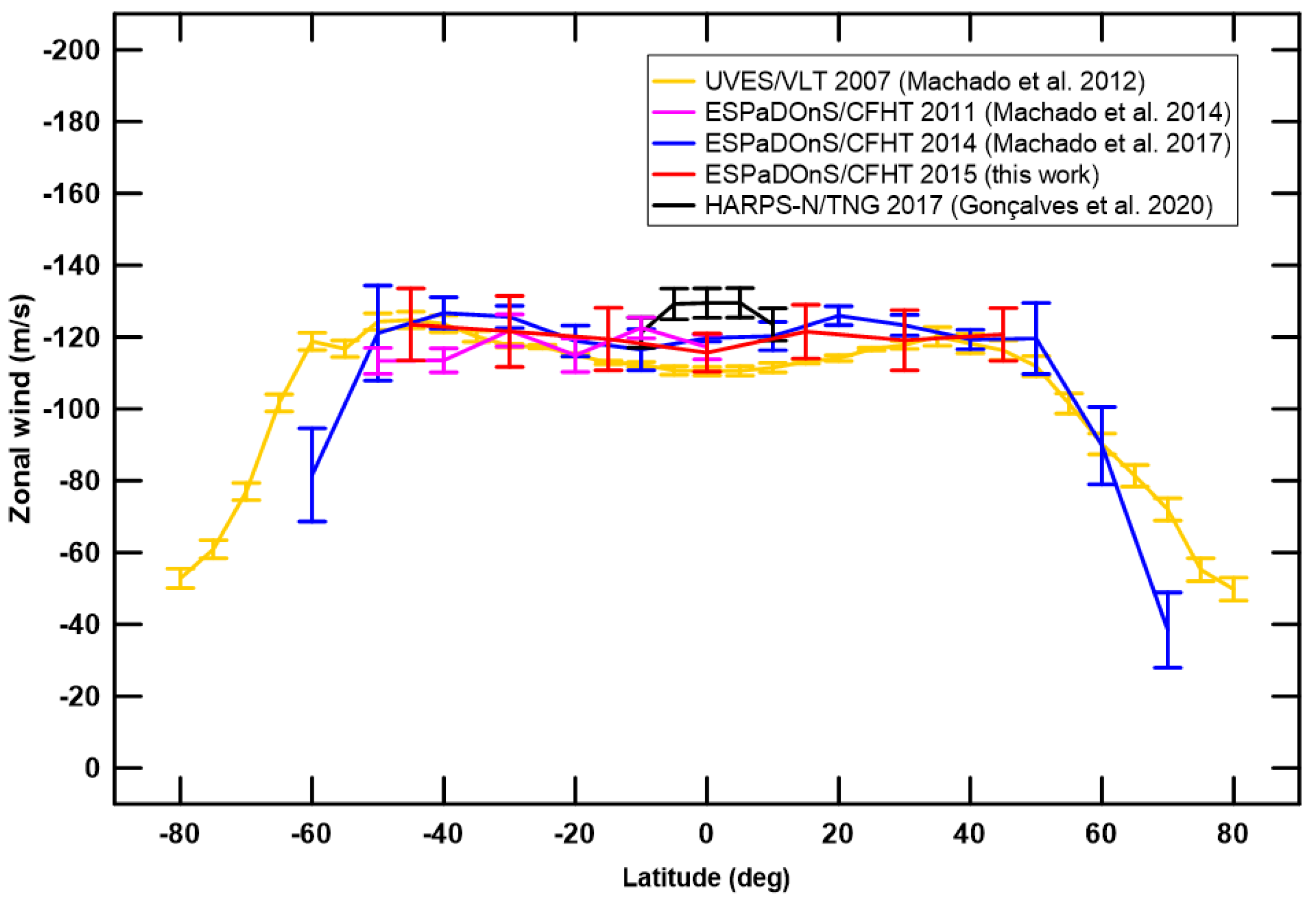
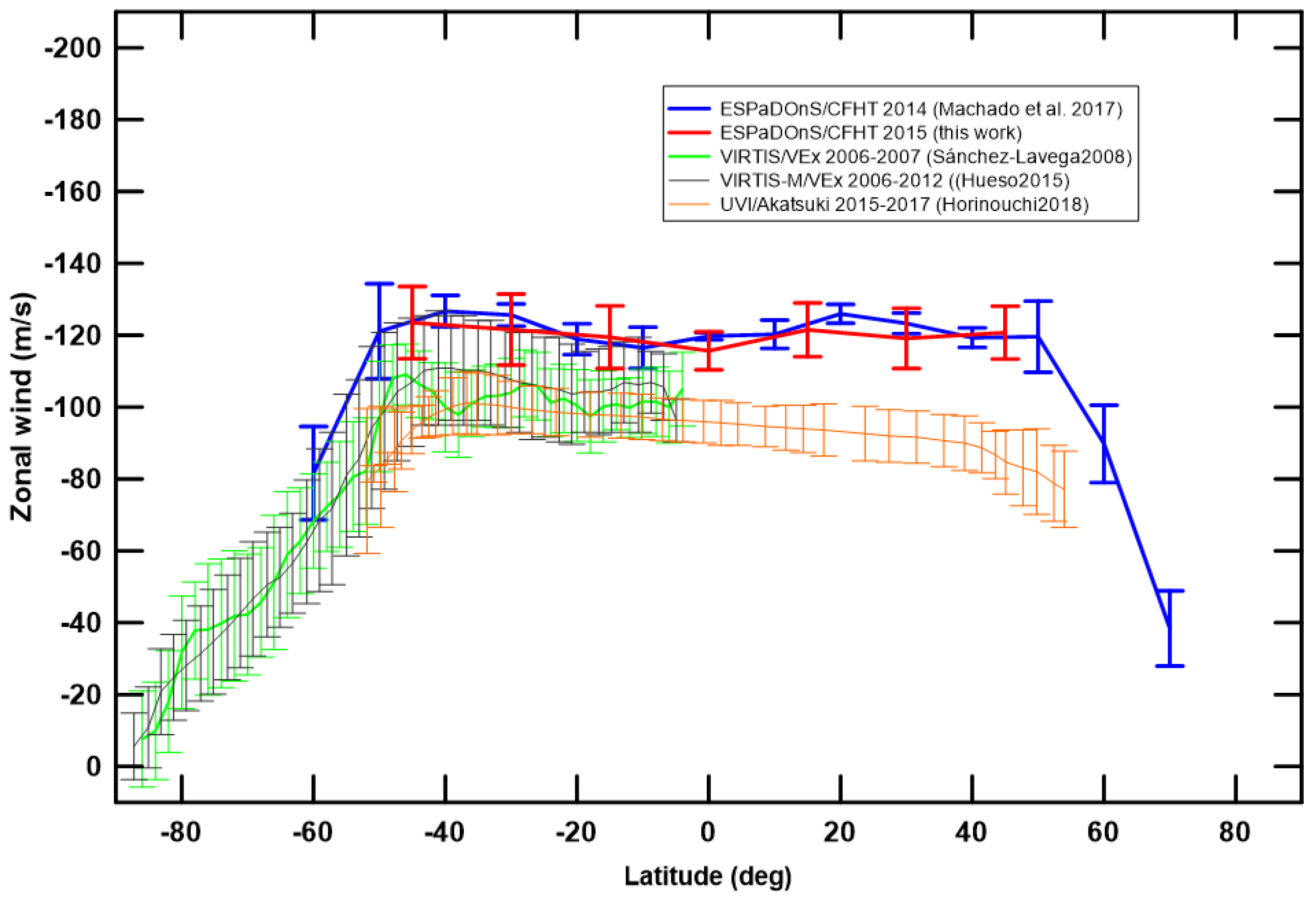
4.1. Zonal Wind
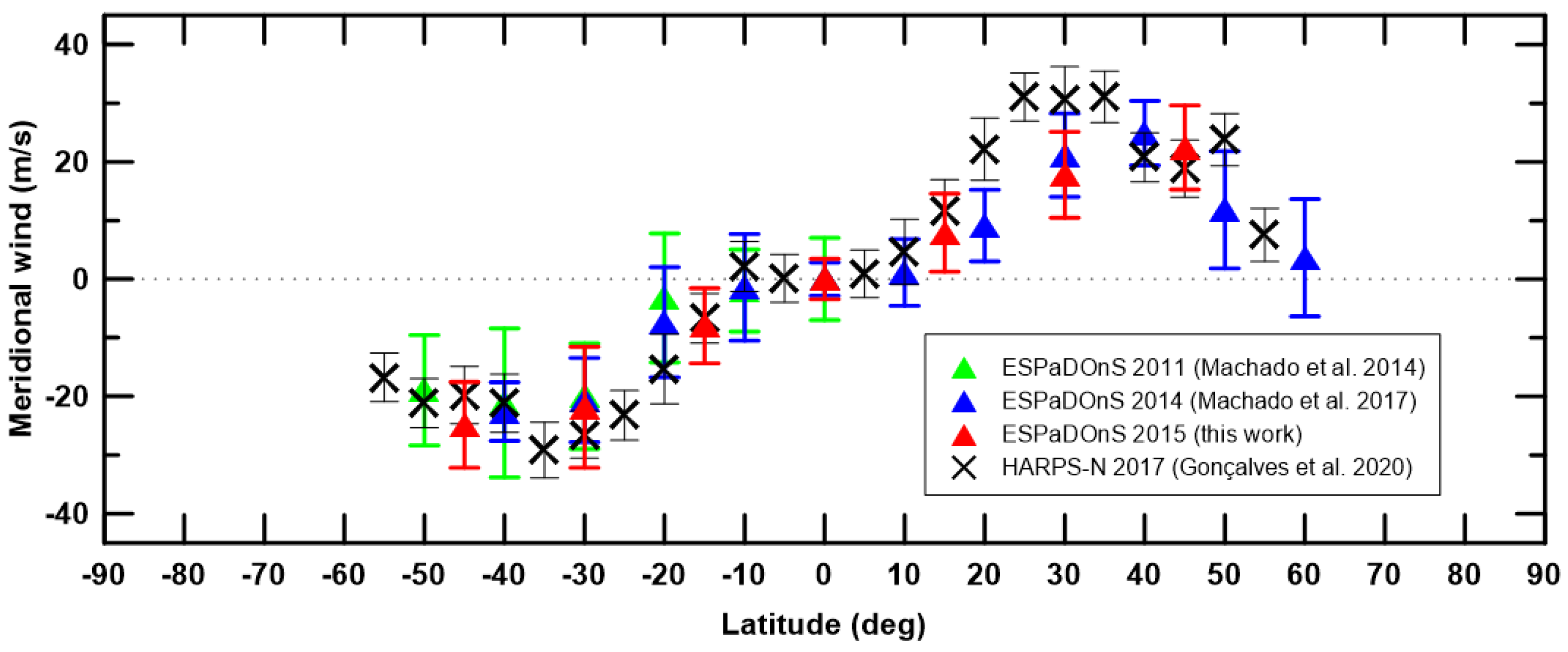
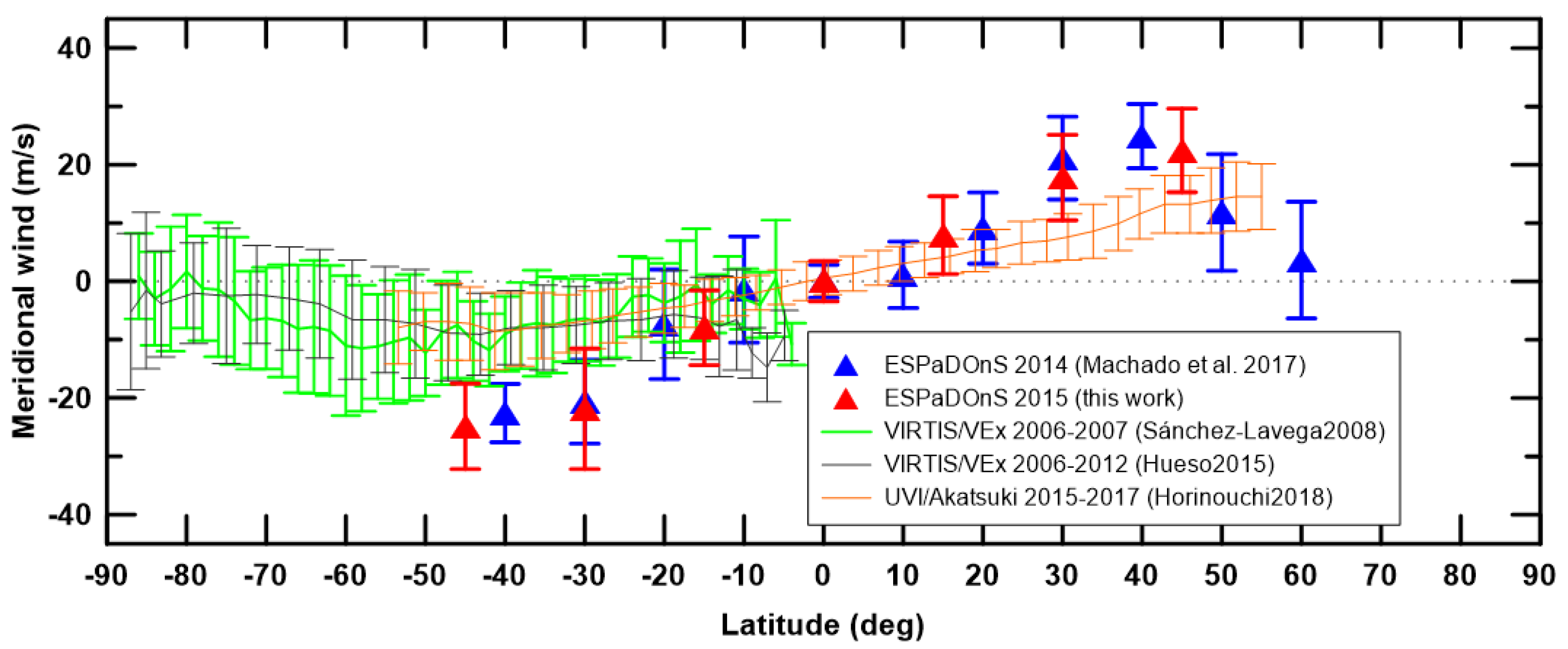
4.2. Meridional Wind
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Svedhem, H.; Titov, D.; Taylor, F.; Witasse, O. Venus as a more Earth-like planet. Nature 2007, 450, 629–632. [Google Scholar]
- Gierasch, P.J.; Goody, R.M.; Young, R.E.; Crisp, D.; Edwards, C.; Kahn, R.; Rider, D.; Del Genio, A.; Greeley, R.; Hou, A.; et al. The General Circulation of the Venus Atmosphere: An Assessment. Venus II: Geology, Geophysics, Atmosphere, and Solar Wind Environment; Tucson, A.Z., Bougher, S.W., Hunten, D.M., Phillips, R.J., Eds.; University of Arizona Press: Tucson, AZ, USA, 1997; pp. 459–500. [Google Scholar]
- Schubert, G.; Bougher, S.; Covey, C.; Del Genio, A.; Grossman, A.; Hollingsworth, J.; Limaye, S.; Young, R. Venus Atmosphere Dynamics: A Continuing Enigma, in Exploring Venus as a Terrestrial Planet; Esposito, L.W., Stofan, E.R., Cravens, T.E., Eds.; American Geophysical Union: Washington, DC, USA, 2007; pp. 101–120. [Google Scholar]
- Sánchez-Lavega, A.; Lebonnois, S.; Imamura, T.; Read, P.; Luz, D. The Atmospheric Dynamics of Venus. Space Sci. Rev. 2017, 212, 1541–1616. [Google Scholar]
- Read, P.L.; Lebonnois, S. Superrotation on Venus, on Titan, and Elsewhere. Annu. Rev. Earth Planet. Sci. 2018, 46, 175–202. [Google Scholar]
- Nakamura, M.; Imamura, T.; Ishii, N.; Abe, T.; Kawakatsu, Y.; Hirose, C.; Satoh, T.; Suzuki, M.; Ueno, M.; Yamazaki, A.; et al. AKATSUKI returns to Venus. Earth Planets Space 2016, 68, 75. [Google Scholar] [CrossRef]
- Sánchez-Lavega, A.; Hueso, R.; Piccioni, G.; Drossart, P.; Peralta, J.; Pérez-Hoyos, S.; Wilson, C.F.; Taylor, F.W.; Baines, K.H.; Luz, D.; et al. Variable winds on Venus mapped in three dimension. Geophys. Res. Lett. 2008, 35, 13204. [Google Scholar]
- Machado, P.; Widemann, T.; Luz, D.; Peralta, J. Wind circulation regimes at Venus’ cloud tops: Ground-based Doppler velocimetry using CFHT/ESPaDOnS and comparison with simultaneous cloud tracking measurements using VEx/VIRTIS in February 2011. Icarus 2014, 243, 249–263. [Google Scholar]
- Hueso, R.; Peralta, J.; Garate-Lopez, I.; Bados, T.V.; Sánchez-Lavega, A. Six years of Venus winds at the upper cloud level from UV, visible and near infrared observations from VIRTIS on Venus Express. Planet. Space Sci. 2015, 113–114, 78–99. [Google Scholar]
- Machado, P.; Widemann, T.; Peralta, J.; Gonçalves, R.; Donati, J.; Luz, D. Venus cloud-tracked and Doppler velocimetry winds from CFHT/ESPaDOnS and Venus Express/VIRTIS in April 2014. Icarus 2017, 285, 8–26. [Google Scholar]
- Horinouchi, T.; Kouyama, T.; Lee, Y.J.; Murakami, S.; Ogohara, K.; Takagi, M.; Imamura, T.; Nakajima, K.; Peralta, J.; Yamazaki, A. Mean winds at the cloud top of Venus obtained from two-wavelength UV imaging by Akatsuk. Earth Planets Space 2018, 70, 10. [Google Scholar]
- Gonçalves, R.; Machado, P.; Widemann, T.; Peralta, J.; Watanabe, S.; Yamazaki, A.; Satoh, T.; Takagi, M.; Ogohara, K.; Lee, Y.J.; et al. Venus’ cloud top wind study: Coordinated Akatsuki/UVI with cloud tracking and TNG/HARPS-N with Doppler velocimetry observations. Icarus 2020, 335, 113418. [Google Scholar] [CrossRef]
- Limaye, S. Venus Atmospheric Circulation: Known and unknown. J. Geophys. Res. Planets 2007, 112, E04S09. [Google Scholar]
- Lebonnois, S.; Lee, C.; Yamamoto, M.; Dawson, J.; Lewis, S.R.; Mendonca, J.; Read, P.; Parish, H.F.; Schubert, G.; Bengtsson, L.; et al. Models of Venus Atmosphere. In Towards Understanding the Climate of Venus. ISSI Scientific Report Series; Springer: New York, NY, USA, 2013; Volume 11, p. 129. ISBN 978-1-4614-5063-4. [Google Scholar]
- Horinouchi, T.; Hayashi, Y.Y.; Watanabe, S.; Yamada, M.; Yamazaki, A.; Kouyama, T.; Taguchi, M.; Fukuhara, T.; Takagi, M.; Ogohara, K.; et al. How waves and turbulence maintain the super-rotation of Venus’ atmosphere. Science 2020, 368, 405–409. [Google Scholar] [PubMed]
- Gierasch, P. Meridional circulation and the maintenance of the Venus atmospheric rotation. J. Atmos. Sci. 1995, 32, 1038. [Google Scholar]
- Luz, D.; Berry, D.L.; Piccioni, G.; Drossart, P.; Politi, R.; Wilson, C.F.; Erard, S.; Nuccilli, F. Venus’s Southern Polar Vortex Reveals Precessing Circulation. Science 2011, 332, 577–580. [Google Scholar]
- Garate-Lopez, I.; Hueso, R.; Sanchez-Lavega, A.; Peralta, J.; Piccioni, G.; Drossart, P. A chaotic long-lived vortex at the southern pole of Venus. Nat. Geosci. 2013, 6, 254–255. [Google Scholar]
- Moissl, R.; Khatuntsev, I.; Limaye, S.S.; Titov, D.V.; Markiewicz, W.J.; Ignatiev, N.I.; Roatsch, T.; Matz, K.D.; Jaumann, R.; Almeida, M.; et al. Venus cloud top winds from tracking UV features in Venus Monitoring Camera. J. Geophys. Res. 2009, 114, E00B31. [Google Scholar]
- Kouyama, T.; Imamura, T.; Nakamura, M.; Satoh, T.; Futaana, Y. Long-term variation in the cloud-tracked zonal velocities at the cloud top of Venus deduced from Venus Express VMC images. J. Geophys. Res. Planets 2013, 118, 37–46. [Google Scholar] [CrossRef]
- Khatuntsev, I.V.; Patsaeva, M.V.; Titov, D.V.; Ignatiev, N.I.; Turin, A.V.; Limaye, S.S.; Markiewicz, W.J.; Almeida, M.; Roatsch, T.; Moissl, R. Cloud level winds from the Venus Express Monitoring Camera imaging. Icarus 2013, 226, 140–158. [Google Scholar]
- Patsaeva, M.V.; Khatuntsev, I.V.; Patsaev, D.V.; Titov, D.V.; Ignatiev, N.I.; Markiewicz, W.J.; Rodin, A.V. The relationship between mesoscale circulation and cloud morphology at the upper cloud level of Venus from VMC/Venus Express. Planet Space Sci. 2015, 113, 100–108. [Google Scholar]
- Gaulme, P.; Schmider, F.-X.; Widemann, T.; Gonçalves, I.; Ariste, A.-L.; Gelly, B. Atmospheric circulation of Venus measured with visible imaging spectroscopy at the THEMIS observatory, Astron. Astrophys 2019, 627, A82. [Google Scholar]
- Takagi, M.; Sugimoto, N.; Ando, H.; Matsuda, Y. Three-Dimensional Structures of Thermal Tides Simulated by a Venus GCM. J. Geophys. Res. 2018, 123, 335–352. [Google Scholar] [CrossRef]
- Widemann, T.; Lellouch, E.; Donati, J.F. Venus Doppler winds at cloud tops observed with ESPaDOnS at CFHT. Planet. Space Sci. 2008, 56, 1320–1334. [Google Scholar] [CrossRef]
- Machado, P.; Luz, D.; Widemann, T.; Lellouch, E.; Witasse, O. Characterizing the atmospheric dynamics of Venus from ground-based Doppler velocimetry. Icarus 2012, 221, 248–261. [Google Scholar] [CrossRef]
- Kawabata, K.; Coffeen, D.; Hansen, J.; Lane, W.; Sato, M.; Travis, L. Cloud and haze properties from Pioneer Venus polarimetry. J. Geophys. Res. 1980, 85, 8129–8140. [Google Scholar] [CrossRef]
- Ignatiev, N.I.; Titov, D.V.; Piccioni, G.; Drossart, P.; Markiewicz, W.J.; Cottini, V.; Roatsch, T.; Almeida, M.; Manoel, N. Altimetry of the Venus cloud tops from the Venus Express observations. J. Geophys. Res. 2009, 114, E00B43. [Google Scholar] [CrossRef]
- Hansen, J.; Hovenier, J. Interpretation of the polarization of Venus. J. Atmos. Sci. 1974, 31, 1137–1160. [Google Scholar] [CrossRef]
- Fedorova, A.; Marcq, E.; Luginin, M.; Korablev, O.; Bertaux, J.L.; Montmessin, F. Variations of water vapor and cloud top altitude in the Venus’ mesosphere from SPICAV/VEx observations. Icarus 2016, 275, 143–162. [Google Scholar] [CrossRef]
- Widemann, T.; Lellouch, E.; Campargue, A. New wind measurements in Venus lower mesosphere from visible spectroscopy. Planet. Space Sci. 2007, 55, 1741–1756. [Google Scholar] [CrossRef]
- Young, A. Is the Four-Day “Rotation” of Venus Illusory? Icarus 1975, 24, 1–10. [Google Scholar] [CrossRef]
- Gaulme, P.; Schmider, F.-X.; Gonçalves, I. Measuring planetary atmospheric dynamics with Doppler spectroscopy. Astron. Astrophys. 2018, 617, A41. [Google Scholar] [CrossRef]
- Allen, C.W. Astrophysical Quantities; Athlone Press: London, UK, 1973. [Google Scholar]
- Cottini, V.; Ignatiev, N.I.; Piccioni, G.; Drossart, P.; Grassi, D.; Markiewicz, W.J. Water vapor near the cloud tops of Venus from Venus Express/VIRTIS dayside data. Icarus 2012, 217, 561–569. [Google Scholar] [CrossRef]
- Gilli, G.; Lebonnois, S.; González-Galindo, F.; López-Valverde, M.A.; Stolzenbach, A.; Lefèvre, F.; Chaufray, J.Y.; Lott, F. Thermal structure of the upper atmosphere of Venus simulated by a ground-to-thermosphere GCM. Icarus 2017, 281, 55–72. [Google Scholar] [CrossRef]

| (1) | (2) | (3) | (4) | (5) | (6) | (7) |
|---|---|---|---|---|---|---|
| Date | UT | Phase Angle | Ill. Fraction | Ang. Diam. | Ob-lon/lat | Airmass |
| () | (%) | (″) | () | |||
| 27 March | 20:00–00:52 | 54.0 | 79.4 | 13.5 | 22.4/−1.88 | 2.5–1.0 |
| 28 March | 19:31–00:55 | 54.4 | 79.1 | 13.6 | 25.0/−1.93 | 3.4–1.0 |
| 29 March | 19:32–00:04 | 54.8 | 78.8 | 13.7 | 27.7/−1.98 | 3.4–1.0 |
| (1) | (2) | (3) | (4) | (5) |
|---|---|---|---|---|
| Date | Loc. | Time Span (UT) | Points Order | Exp. Repetition |
| March 27 | ||||
| Equator | 20:00–20:18 | 23-21-20-19-23-24-22 | 3 x | |
| N lat 15 | 20:21–20:41 | 22-16-15-14-13-17-18-22 | 3 x | |
| S lat 15 | 20:42–20:59 | 28-22-28-27-26-25-22 | 3 x | |
| = −25 (HPA) | 22:59–23:20 | 22-16-10-4-22-28-34-40-22 | 3 x | |
| = 20 N | 23:22–23:39 | 22-19-19-13-7-1-22 | 3 x | |
| = 20 S | 23:47–00:10 | 22-25-31-22-31-37-37-22-22 | 3 x | |
| S lat 15 | 00:12–00:33 | 22-28-27-22-26-25-29-30-22 | 3 x | |
| S lat 30 | 00:34–00:51 | 22-34-22-33-32-31-22 | 3 x | |
| March 28 | ||||
| Equator | 19:31–19:49 | 22-22-21-20-19-23-24-24 | 3 x | |
| S lat 15 | 19:51–20:09 | 22-28-27-26-25-29-30-22 | 3 x | |
| S lat 30 | 20:10–20:28 | 22-34-33-32-31-35-36-22 | 3 x | |
| S lat 45 | 20:29–20:46 | 22-22-40-39-38-37-41-42-22 | 3 x | |
| = 20 | 20:47–23:08 | 22-19-13-7-1-25-31-37-22 | 3 x | |
| = −25 (HPA) | 23:08-23:26 | 16-22-10-4-28-34-40-22 | 3 x | |
| N lat 15 | 23:35–23:54 | 22-13-14-15-16-17-18-22 | 3 x | |
| N lat 30 | 23:55–00:13 | 7-8-22-9-10-11-12 | 3 x | |
| N lat 45 | 00:14–00:30 | 22-1-2-3-4-5-[6] | 3 x | |
| Equator | 00:32–00:30 | 22-21-20-19-22-[22]-[22] | 3 x | |
| March 29 | ||||
| = 20 | 19:32–19:54 | 22-19-13-13-7-1-25-31-37-22 | 3 x | |
| = −25 (HPA) | 19:56–20:15 | 22-16-10-4-22-28-34-40-22 | 3 x | |
| Equator | 20:17–22:42 | 22-21-22-22-21-20-19-23-24 | 3 x | |
| N lat 15 | 22:43–23:01 | 22-16-22-15-14-13-17-18 | 3 x | |
| N lat 30 | 23:02–23:22 | 22-10-22-9-8-7-11-12-22 | 3 x | |
| N lat 45 | 23:33–00:03 | 22-4-3-2-1-5-22-[22]-[22]-[22] | 3 x |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gonçalves, R.; Machado, P.; Widemann, T.; Brasil, F.; Ribeiro, J. A Wind Study of Venus’s Cloud Top: New Doppler Velocimetry Observations. Atmosphere 2021, 12, 2. https://doi.org/10.3390/atmos12010002
Gonçalves R, Machado P, Widemann T, Brasil F, Ribeiro J. A Wind Study of Venus’s Cloud Top: New Doppler Velocimetry Observations. Atmosphere. 2021; 12(1):2. https://doi.org/10.3390/atmos12010002
Chicago/Turabian StyleGonçalves, Ruben, Pedro Machado, Thomas Widemann, Francisco Brasil, and José Ribeiro. 2021. "A Wind Study of Venus’s Cloud Top: New Doppler Velocimetry Observations" Atmosphere 12, no. 1: 2. https://doi.org/10.3390/atmos12010002
APA StyleGonçalves, R., Machado, P., Widemann, T., Brasil, F., & Ribeiro, J. (2021). A Wind Study of Venus’s Cloud Top: New Doppler Velocimetry Observations. Atmosphere, 12(1), 2. https://doi.org/10.3390/atmos12010002






