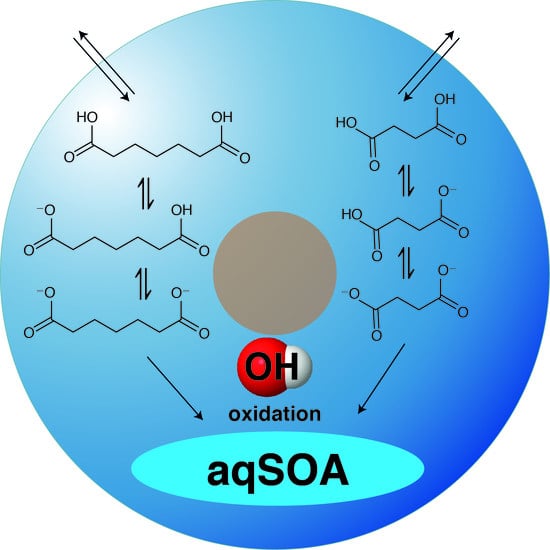
pH- and Temperature-Dependent Kinetics of the Oxidation Reactions of OH with Succinic and Pimelic Acid in Aqueous Solution

Abstract

Rate constants for the aqueous-phase reactions of the hydroxyl radical with the dicarboxylic acids, succinic acid and pimelic acid were determined using the relative rate technique over the temperature range 287 K ≤ T ≤ 318 K and at pH = 2.0, 4.6 or 4.9 and 8.0. OH radicals were generated by H₂O₂ laser flash photolysis while thiocyanate was used as a competitor. The pH values were adjusted to obtain the different speciation of the dicarboxylic acids. The following Arrhenius expressions were determined (in units of L mol⁻¹ s⁻¹):  succinic acid, k(T, AH₂) (2.1 ± 0.1) × 10¹⁰ exp[(−1530 ± 250 K)/T], k(T, AH⁻) (1.8 ± 0.1) × 10¹⁰ exp[(−1070 ± 370 K)/T], k(T, A²⁻) (2.9 ± 0.2) × 10¹¹ exp[(−1830 ± 350 K)/T] and pimelic acid, k(T, AH₂) (7.3 ± 0.2) × 10¹⁰ exp[(−1040 ± 140 K)/T], k(T, AH⁻) (1.8 ± 0.1) × 10¹¹ exp[(−1200 ± 240 K)/T], k(T, A²⁻) (1.4 ± 0.1) × 10¹² exp[(−1830 ± 110 K)/T]. A general OH radical reactivity trend for dicarboxylic acids was found as k(AH₂) < k(AH⁻) < k(A²⁻). By using the pH and temperature dependent rate constants, source and sinking processes in the tropospheric aqueous phase can be described precisely.

1. Introduction

Dicarboxylic acids such as oxalic, succinic, and pimelic acid are important trace constituents in the troposphere where they exist predominately in the particle phase as demonstrated by the Henry’s Law constant Hcp = (2–4.1) × 10⁷ mol m⁻³ Pa⁻¹ for succinic acid and Hcp = 8.1 × 10⁷ mol m⁻³ Pa⁻¹ for pimelic acid [1,2]. Dicarboxylic acids are ubiquitous and have been found in remote and polluted areas as well as in pristine areas such as the Arctic and Antarctic region [3,4,5,6,7,8]. These compounds reach the pristine areas through long-range transport or originate from the photochemical oxidation of volatile organic compounds (VOCs) (e.g., isoprene), biogenic unsaturated fatty acids emitted from the surface ocean [4], or biomass burning or vehicle emissions [3,4]. Organic acids and diacids can contribute to the acidity of cloud water [9,10], lead to the secondary organic aerosol (SOA) formation by fostering accretion reactions such as hemiacetal formation or esterification [11]), and catalyze the oxidation of SO₂ [12] and the hydrolysis of SO₃ [13]. The oxidation of dicarboxylic acid generally lead either to more functionalized (substituted) acids or to the degradation by shortening the carbon chain [7,11,14,15,16,17]. The aqueous-phase oxidation kinetics of carboxylic acids and dicarboxylic acid towards hydroxyl radicals have been investigated in several studies in the past [14,17,18,19,20,21,22,23,24]. These investigations clearly show an influence of the speciation of the dicarboxylic acid on the OH radical rate constant, a systematic investigation of the monoanionic form of the carboxylic acid is still missing. This motivates further investigations of the temperature- and pH-dependent of hydroxyl radical reaction with succinic acid (C₄) and pimelic acid (C₇) studied here, since (i) these DCAs have been identified in ambient aerosol particles [4,25,26] and (ii) the pH in the tropospheric aqueous phases varies between −2.5 and +9.35 [9]. The results were obtained by using the laser flash photolysis of hydrogen peroxide to generate the OH radicals, which react with a competitor SCN⁻. This relative rate technique is suitable over large temperature ranges and can be applied at different pH values. Dicarboxylic acids have two acid-base equilibria, so the pH value was chosen in such a way to first separately investigate the fully protonated and the fully deprotonated forms, and then determine the rate constant of the singly deprotonated form in order to characterize their atmospheric lifetime.

2. Experiments

2.1. Materials

The following chemicals were used as received: hydrogen peroxide (≥30% in water, Chemsolute), KSCN (≥99.0%, Chemsolute), Succinic acid (≥99.0%, Sigma-Aldrich), Pimelic acid (≥98.0%, Fluka), HClO₄ (70–72%, J. T. Baker Analyzed), NaOH (1 mol L⁻¹ in water, Fluka). All solutions were freshly prepared using Milli-Q grade water (18 MΩ cm, Millipore, Billerica, MA).

2.2. Methods

The second order OH radical rate constants of the reaction with organic acids were determined by applying the thiocyanate competition kinetics method [27,28,29] using a temperature-controlled laser flash photolysis long path absorption (LP-LPA) setup [28,29].

2.2.1. Experimental Setup

Briefly, the setup includes an excimer laser (Compex 201, Lambda-Physik) operating with a 20 ns pulse and a frequency of f = 4 Hz at λ = 248 nm as photolysis light source, a cuboid reaction flow cell (28 mL volume) with a photolysis path length of 3.5 cm, and a White cell mirror configuration to increase the optical path length of the analytical light to d = 48 cm. Two continuous-wave lasers at λ = 407 nm (Radius 405, Coherent) or λ = 561 nm (LCX-561, Oxxius) were used as analyzing light sources. A photodiode (S1336-44BQ, Hamamatsu) was applied as detector. For spectrometric measurements, a double-beam spectrometer (Lambda 900, Perkin Elmer) was applied and the pH value of the measurement solution was measured with a pH-electrode (N 5900 A, SI Analytics).

2.2.2. Competition Kinetics Method

In a competition reaction of the OH radical between the organic and the reference reactant thiocyanate an absorbing radical (SCN)₂⁻ is formed in reactions (R-3) to (R-5). The photolysis of hydrogen peroxide (R-1) (c(H₂O₂) = 2 · 10⁻⁴ mol L⁻¹) by a laser pulse at λ = 248 nm leads to the formation of OH radicals.

H₂O₂ + hν (248 nm) → 2 OH

(R-1)

OH + R-H → R + H₂O

(R-2)

OH + SCN⁻ ⇄ SCNOH⁻

(R-3)

SCNOH⁻ ⇄ SCN + OH⁻

(R-4)

SCN + SCN⁻ ⇄ (SCN)₂⁻

(R-5)

(SCN)₂⁻ + (SCN)₂⁻ ⇄ (SCN)₂ + 2 SCN⁻

(R-6)

Further details of the thiocyanate reference reactant in the competition kinetics method can be found in Herrmann et al. [14] and the references therein. The OH concentration can be given in a range of c(OH) = 1 · 10⁻⁷–1 · 10⁻⁶ mol L⁻¹. The change of the absorption (cf. Figure S1 in the Supplementary Materials), due to the formation of (SCN)₂⁻ radicals, was monitored at 407 or 561 nm (ε_{407 nm} = 3425 mol L⁻¹ cm⁻¹ and ε_{561 nm} = 3315 L mol⁻¹ cm⁻¹) [14]. Each absorbance-time profile in Figure S1 was obtained by averaging 8 × 32 single traces. The absorbance error was determined by using the standard deviation and the Student’s t-distribution. The OH radical reaction rate constants of the dicarboxylic acids (k_RH) can be derived, by using Equation (1).

$$\frac{{\mathrm{A}[(\mathrm{SCN})}_2^{-}{]}_{0,\mathrm{X}}}{{\mathrm{A}[(\mathrm{SCN})}_2^{-}{]}_{\mathrm{X}}}=1+\frac{{\mathrm{k}}_{\mathrm{RH}}[\mathrm{RH}]}{{\mathrm{k}}_0{[\mathrm{SCN}}^{-}]}$$

(1)

The expressions A[(SCN)₂⁻]_0,x and A[(SCN)₂⁻]_x corresponds to the maximum absorption of the (SCN)₂⁻ in the absence and in the presence of the organic reactant. The concentration of the organic reactant (c(RH) = 2.5 · 10⁻⁵ –4.0 · 10⁻⁴ mol L⁻¹) is increased stepwise, while the reference reactant concentration (c(SCN⁻) = 2.0 · 10⁻⁵ mol L⁻¹) is constant in the series of five single measurement. Both concentrations, that of the organic reactant and that of the reference reactant, are at least 10 times higher than the concentration of the OH radicals in order to fulfil the conditions of the pseudo-first order. The ratios of A[(SCN)₂⁻]_0,x/A[(SCN)₂⁻]_x were plotted against the corresponding ratios of [RH]/[SCN⁻] (cf. Figure S2 in the Supplementary Materials) and from the resulting slope the second order rate constant can be calculated by using the temperature-dependent reference rate constants (k₀) by Zhu et al. [27]. The reported errors of the rate constant represent the statistical error for a 95% confidence interval.

3. Results and Discussion

In the present study, the pH- and T-dependencies of the second order rate constants of the reactions of OH with the organic diacids succinic (C₄ dicarboxylic acid) and pimelic acid (C₇ dicarboxylic acid) were determined.

Table 1. Observed OH radical second order rate constants of the reaction with studied dicarboxylic acids at different temperatures.

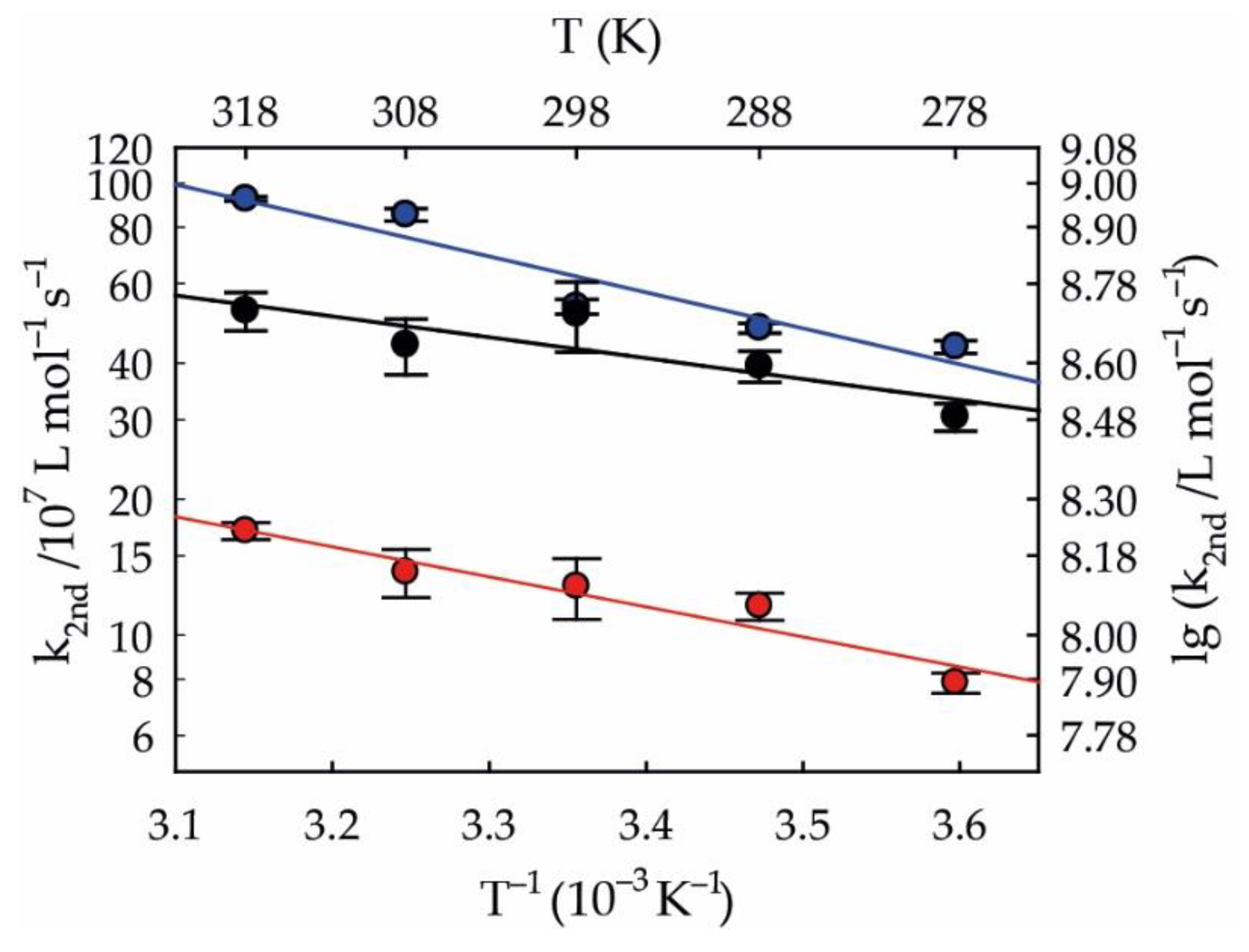
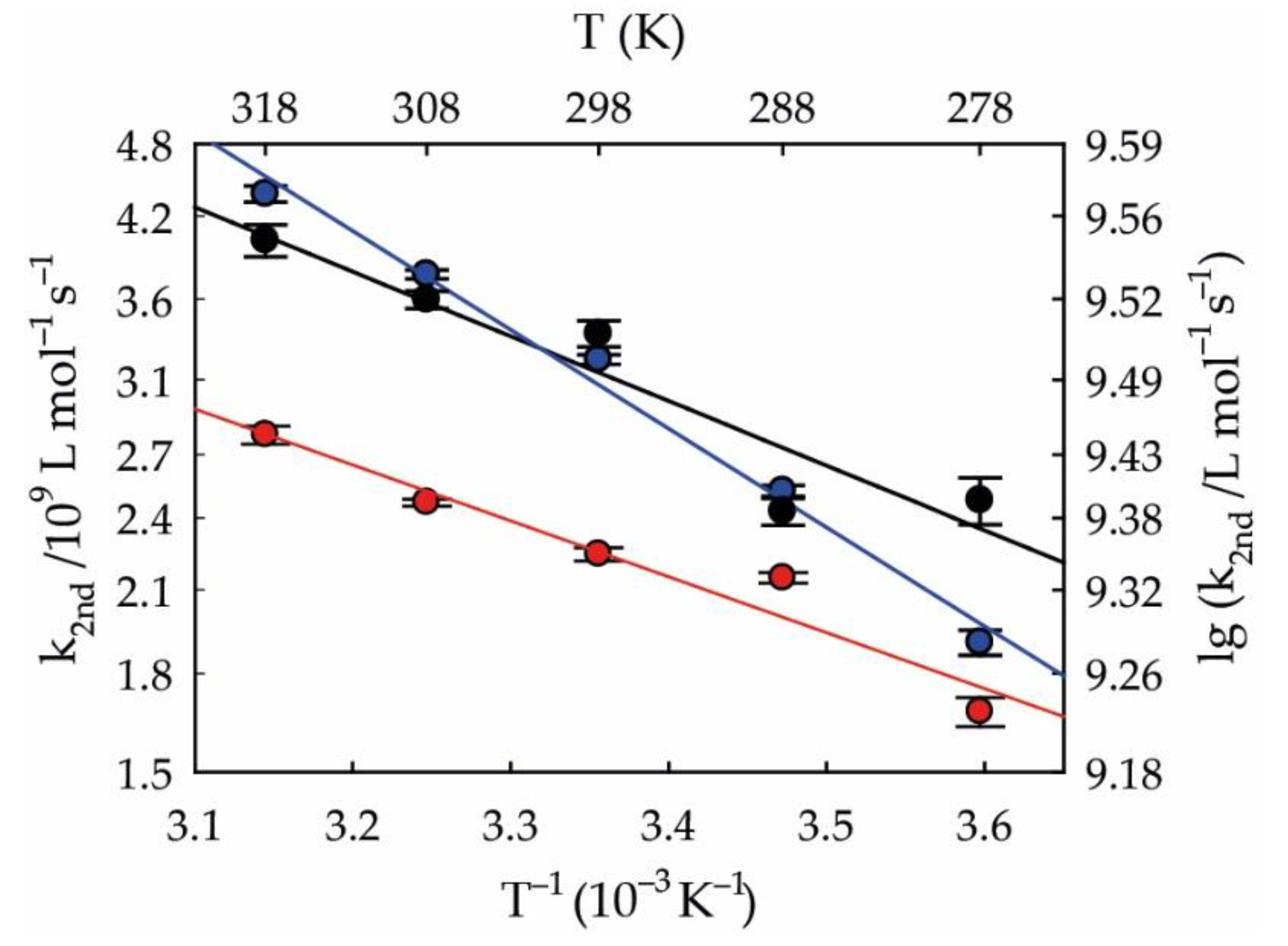
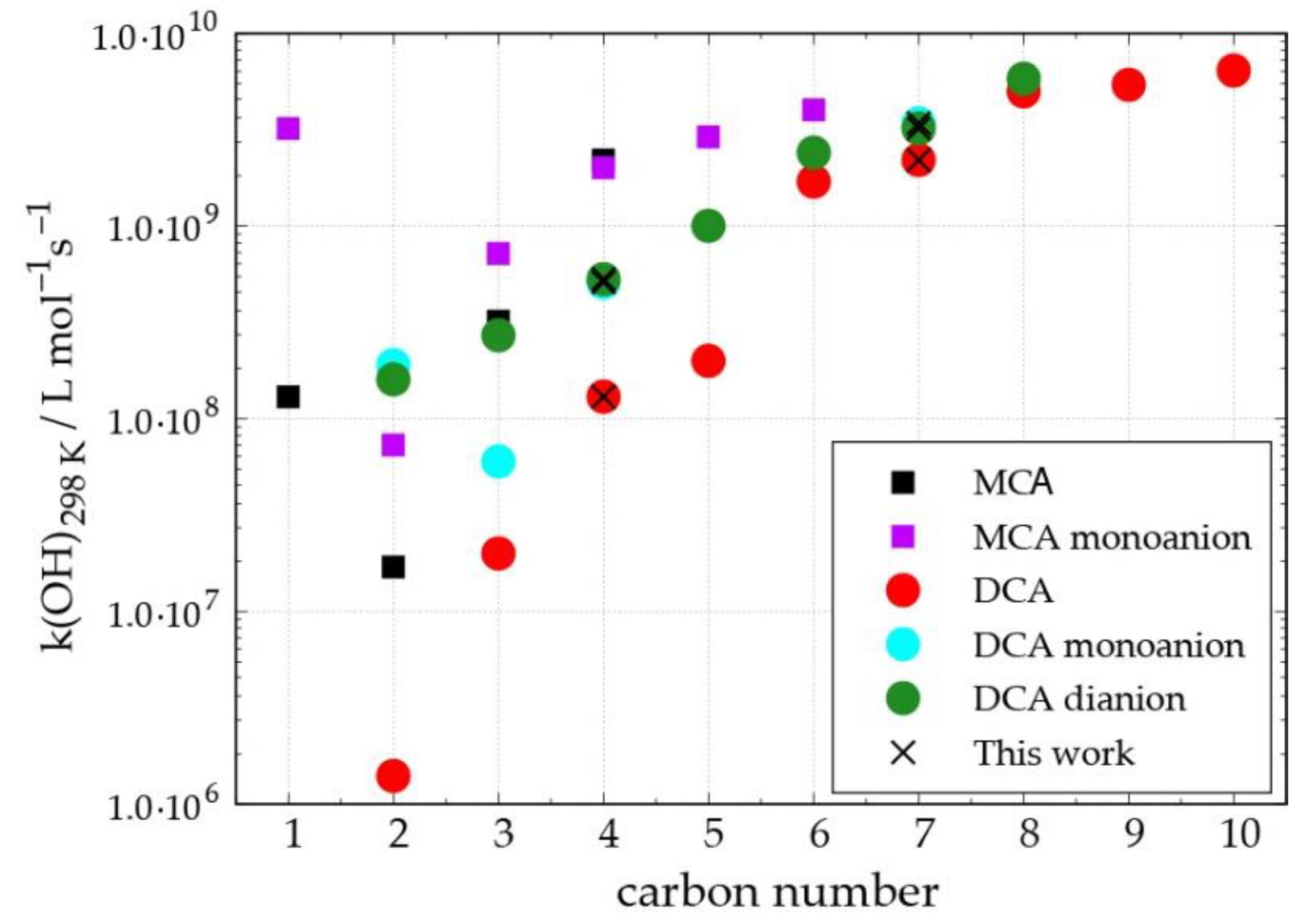
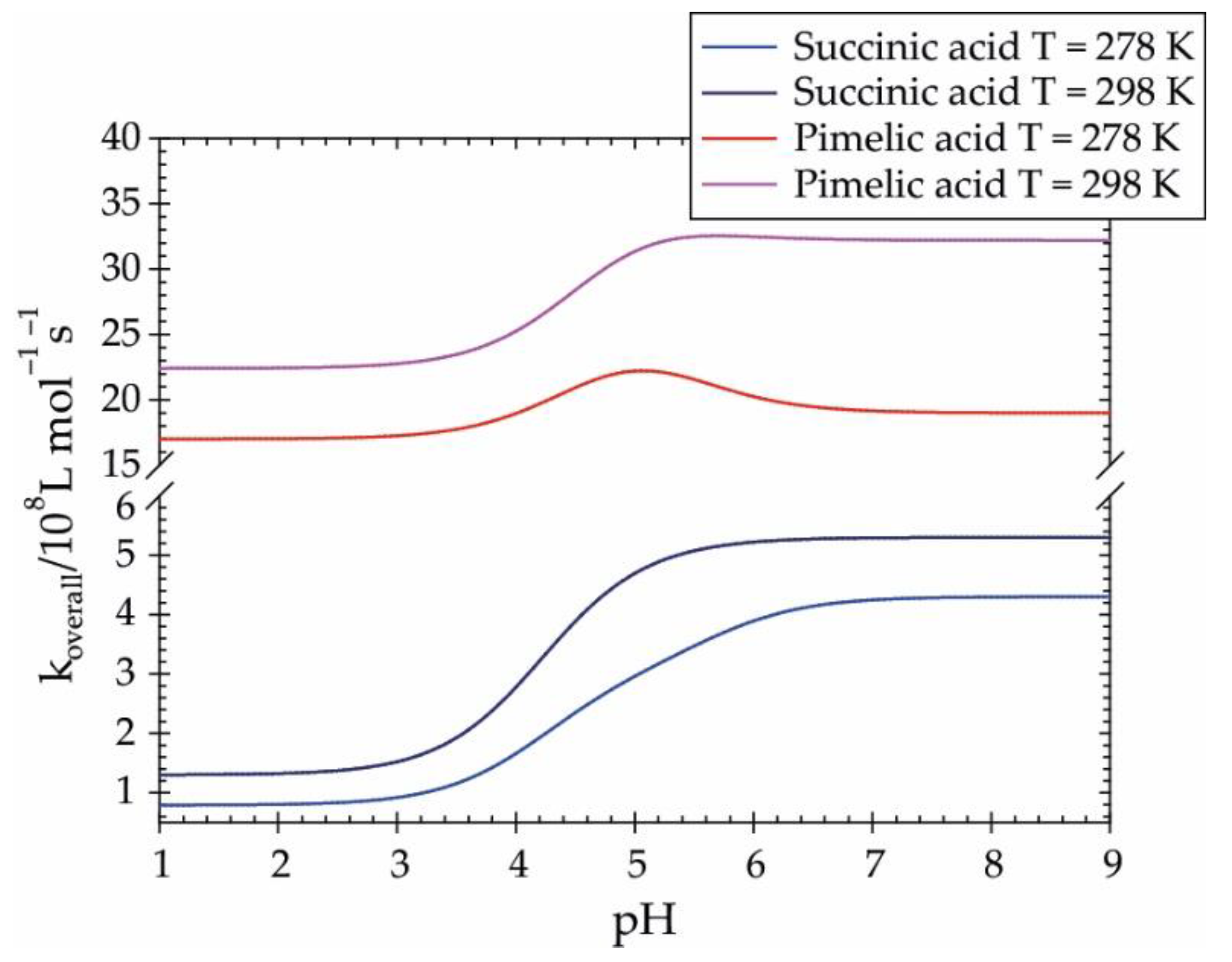
Compounds and pH Values	k(278 K)/L mol−1 s−1	k(288 K)/L mol−1 s−1	k(298 K)/L mol−1 s−1	k(308 K)/L mol−1 s−1	k(318 K)/L mol−1 s−1
Succinic acid
pH = 2	(7.9 ± 0.4) · 107	(1.2 ± 0.1) · 108	(1.3 ± 0.2) · 108	(1.4 ± 0.4) · 108	(1.7 ± 0.1) · 108
pH = 8-	(4.3 ± 0.1) · 108	(4.8 ± 0.1) · 108	(5.3 ± 0.2) · 108	(8.5 ± 0.3) · 108	(9.2 ± 0.1) · 108
pH = 4.9	(2.9 ± 0.1) · 108	(3.6 ± 0.1) · 108	(4.6 ± 0.3) · 108	(4.5 ± 0.3) · 108	(3.6 ± 0.1) · 108
Pimelic acid
pH = 2	(1.7 ± 0.1) · 109	(2.2 ± 0.1) · 109	(2.2 ± 0.1) · 109	(2.5 ± 0.1) · 109	(2.8 ± 0.1) · 109
pH = 8-	(1.9 ± 0.1) · 109	(2.5 ± 0.1) · 109	(3.2 ± 0.1) · 109	(3.8 ± 0.1) · 109	(4.4 ± 0.1) · 109
pH = 4.61	(2.1 ± 0.1) · 109	(2.3 ± 0.1) · 109	(2.8 ± 0.1) · 109	(3.2 ± 0.1) · 109	(3.5 ± 0.1) · 109

contains the determined OH radical rate constants at three different pH values (pH = 2.0, 4.6 or 4.9, and 8) and five different temperatures (287 K ≤ T ≤ 318 K).

The pK_a values of the diacids are available for succinic acid as pK_a,1 = 4.207 and pK_a,2 = 5.363 and for pimelic acid as pK_a,1 = 4.49 and pK_a,2 = 5.43 at ionic strength zero [30].

The ratio of the different forms AH₂, AH⁻, and A²⁻ as a function of the pH value shown in Figure 1 (top) for succinic acid and in Figure 1 (bottom) for pimelic acid can be calculated. The maximum of the monoanionic dicarboxylic acid form AH⁻ is present at pH 4.9 for both the investigated organic diacids. Following the acid–base equilibria in Figure 1, the OH radical rate constant measurement of k(obs), was performed close to the pH of the maximum occurrence of the AH⁻ form. To derive the rate constants of the monoanionic form, k(AH⁻), the observed rate constant k(obs) has to be corrected for the contribution χ of the fully protonated form AH₂ at pH = 2 as well as by the fully deprotonated form A²⁻ at pH = 8 using the following equation (Equation (2)). The term χ describes the percentage ratio of speciation calculated from the acid–base equilibria.

$${\mathrm{k}(\mathrm{obs})=\mathrm{k}(\mathrm{AH}}^{-})\cdot {\mathsf{\chi }(\mathrm{AH}}^{-}{)+\mathrm{k}(\mathrm{A}}^{2-})\cdot {\mathsf{\chi }(\mathrm{A}}^{2-}{)+\mathrm{k}(\mathrm{AH}}_2)\cdot {\mathsf{\chi }(\mathrm{AH}}_2{)/\mathrm{L}\mathrm{mol}}^{-1}{\mathrm{s}}^{-1}$$

(2)

In addition, the T-dependency of the pK_a value treated by Equation (3), derived from the van’t Hoff equation [28] (Equation (4)) shows only a small influence of the temperature on the pK_a value (

Table 2. Calculated pK_a values and radical rate constants of the monoanionic dicarboxylic acids AH⁻ at different temperatures.

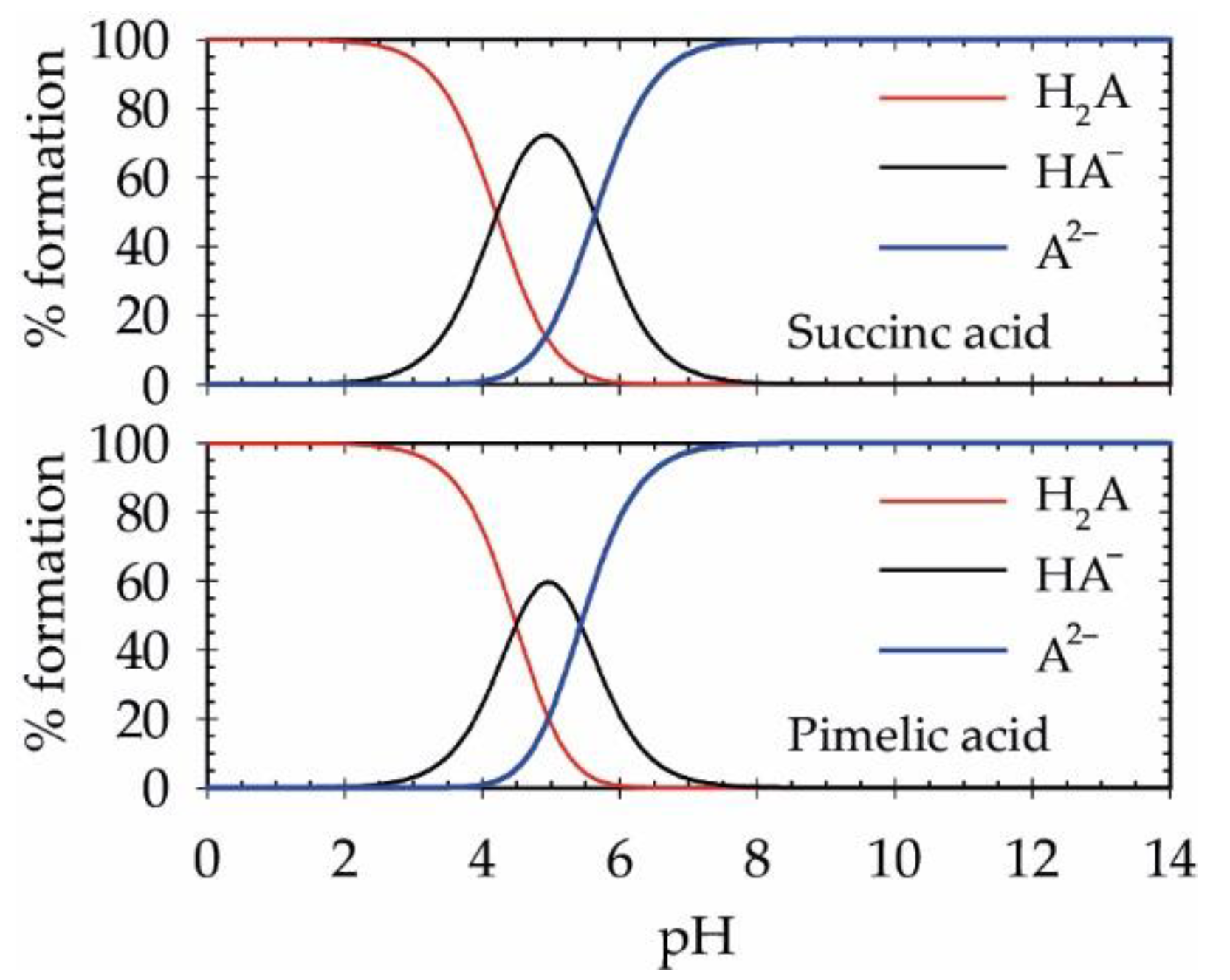
Compounds, pKa Values and Rate Constants	278 K	288 K	298 K	308 K	318 K
Succinic acid
pKa,1	4.243	4.224	4.207	4.191	4.176
pKa,2	5.634	5.635	5.636	5.637	5.638
k2nd(AH−)/L mol−1 s−1	(3.0 ± 0.1) · 108	(3.9 ± 0.1) · 108	(5.1 ± 0.1) · 108	(4.4 ± 0.1) · 108	(5.3 ± 0.1) · 108
Pimelic acid
pKa,1	4.474	4.482	4.490	4.497	4.504
pKa,2	5.383	5.407	5.430	5.451	5.471
k2nd(AH−)/L mol−1 s−1	(2.5 ± 0.1) · 109	(2.4 ± 0.1) · 109	(3.4 ± 0.1) · 109	(3.6 ± 0.1) · 109	(4.0 ± 0.1) · 109

).

$${\mathrm{pK}}_{\mathrm{a}}{=\mathrm{pK}}_{\mathrm{a}}^{\circ }+\frac{{\mathsf{\Delta }}_{\mathrm{R}}{\mathrm{H}}^{\mathrm{o}}}{\mathrm{R}\ln 10}\left(\frac{1}{\mathrm{T}}-\frac{1}{{\mathrm{T}}^{\mathrm{o}}}\right)$$

(3)

$${\left(\frac{{\partial }_{}\mathrm{lnK}}{{\partial }_{}\mathrm{T}}\right)}_{\mathrm{p}}=\frac{{\mathsf{\Delta }}_{\mathrm{R}}{\mathrm{H}}^{\mathrm{o}}}{{\mathrm{RT}}^2}$$

(4)

The standard enthalpy ∆_RH° at T = 298.15 K for succinic acid can be given ∆_RH° = −2845.12 J mol⁻¹ at pK_a,1 = 4.207 and ∆_RH° = 167.36 J mol⁻¹ at pK_a,2 = 5.636 as well as for pimelic acid given with ∆_RH° = 1255.2 J mol⁻¹ at pK_a,1 = 4.49 and ∆_RH° = 3765.6 J mol⁻¹ at pK_a,2 = 5.43 [30]. In order to calculate the rate constant for the reaction of OH with the monoanion form as exactly as possible from the observed rate constant at pH = 4.9 and 4.6 the T-dependent pK_a values given in Table 2 were used.

The UV spectra of the dicarboxylic acids were measured to clarify a possible influence of the internal absorption effect [28] on the measured rate constants. Since the obtained molar attenuation coefficient at the excitation wavelength λ = 248 nm is lower than ε_{248 nm} < 1 L mol⁻¹ cm⁻¹, this effect can be neglected for the used measurement conditions.

Briefly, the diffusion limit rate constants of the OH radical reaction were calculated by using a method based on the Smoluchowski equation [31]. Further details of this method can be found elsewhere [32,33,34]. The obtained values (unit/L mol⁻¹ s⁻¹) k_diff.(278 K) = 0.7 × 10¹⁰, k_diff.(288 K) = 1.0 × 10¹⁰, k_diff.(298 K) = 1.4 × 10¹⁰, k_diff.(308 K) = 1.7 × 10¹⁰, and k_diff.(318 K) = 2.2 × 10¹⁰ show that the measured rate constants are far away from the diffusion limitation and hence are chemically controlled.

The temperature-dependency of these radical rate constants can be described by an Arrhenius type equation [35] (Equation (5)), from this the pre-exponential factor, A, and the activation energy, E_A, are derived. The parameters R and T describe the gas constant and the temperature.

$$\mathrm{k}(\mathrm{T})=\mathrm{A}\cdot \exp \left(\raisebox{1ex}{$-{\mathrm{E}}_{\mathrm{A}}$}\!\left/ \!\raisebox{-1ex}{$\mathrm{RT}$}\right.\right){/\mathrm{L}\mathrm{mol}}^{-1}{\mathrm{s}}^{-1}$$

(5)

The T-dependent rate constants of the OH radical reaction with succinic acid were determined and are depicted in Figure 2.

The derived Arrhenius expressions are shown in Equation (6) for the protonated succinic acid (pH = 2), in Equation (6) for the monoanionic form (pH = 4.9), and in Equation (8) for the dianionic form (pH = 8.0).

$${\mathrm{k}(\mathrm{T},\mathrm{AH}}_2)=(2.1\pm 0.1)\cdot {10}^{10}\cdot \exp \left(\frac{-1530\pm 250\mathrm{K}}{\mathrm{T}}\right){/\mathrm{L}\mathrm{mol}}^{-1}{\mathrm{s}}^{-1}$$

(6)

$${\mathrm{k}(\mathrm{T},\mathrm{AH}}^{-})=(1.8\pm 0.1)\cdot {10}^{10}\cdot \exp \left(\frac{-1070\pm 370\mathrm{K}}{\mathrm{T}}\right){/\mathrm{L}\mathrm{mol}}^{-1}{\mathrm{s}}^{-1}$$

(7)

$${\mathrm{k}(\mathrm{T},\mathrm{A}}^{2-})=(2.9\pm 0.2)\cdot {10}^{11}\cdot \exp \left(\frac{-1830\pm 350\mathrm{K}}{\mathrm{T}}\right){/\mathrm{L}\mathrm{mol}}^{-1}{\mathrm{s}}^{-1}$$

(8)

The temperature-dependency of the rate constants for the OH radical reaction with succinic acid can be described in the following order: AH₂ < AH⁻ < A²⁻. Under alkaline conditions, the reaction is more T-dependent than under acidic conditions as it can be seen in the Arrhenius plot and the derived Arrhenius expressions.

The obtained Arrhenius plot of pimelic acid oxidation by OH radicals at pH = 2.0, pH = 4.6 and pH = 8 is shown in Figure 3. From the Arrhenius plot (Figure 3) the following T-dependencies are obtained:

$${\mathrm{k}(\mathrm{T},\mathrm{AH}}_2)=(7.3\pm 0.2)\cdot {10}^{10}\cdot \exp \left(\frac{-1040\pm 140\mathrm{K}}{\mathrm{T}}\right){/\mathrm{L}\mathrm{mol}}^{-1}{\mathrm{s}}^{-1}$$

(9)

$${\mathrm{k}(\mathrm{T},\mathrm{AH}}^{-})=(1.8\pm 0.1)\cdot {10}^{11}\cdot \exp \left(\frac{-1200\pm 240\mathrm{K}}{\mathrm{T}}\right){/\mathrm{L}\mathrm{mol}}^{-1}{\mathrm{s}}^{-1}$$

(10)

$${\mathrm{k}(\mathrm{T},\mathrm{A}}^{2-})=(1.4\pm 0.1)\cdot {10}^{12}\cdot \exp \left(\frac{-1830\pm 110\mathrm{K}}{\mathrm{T}}\right){/\mathrm{L}\mathrm{mol}}^{-1}{\mathrm{s}}^{-1}$$

(11)

Similar to the above-mentioned succinic acid the T dependency of the OH radical reaction of pimelic acid at pH = 8 is strongly dependent on T. The monoanionic form of both acids exhibits a similar T-dependency like the fully protonated acid with a faster reaction rate.

3.1. Oxidation Mechanism

The general reaction mechanism of OH radicals with organic compounds (dicarboxylic acids) in the aqueous media can be described by an H atom abstraction mechanism. The OH radical attack occurs predominantly at the weakest C-H bond of the molecule [29,36,37,38]. In general hydrogen bond dissociation energies (BDEs) of a dicarboxylic acid show the following trend: CH < CH₂ < CH₃ < OH [39]. The BDE of the C-H bond was estimated to be 395 kJ mol⁻¹ [37] and 397.8 ± 5.1 kJ mol⁻¹ [40] for succinic acid. An alkyl radical is formed after the H atom abstraction which reacts in the presence of O₂ to a peroxyl radical [41]. Depending on the molecule structure, the derived peroxyl radical can recombine to either form a tetroxide or react further in a unimolecular decay process [42]. In the case of succinic acid (C₄ dicarboxylic acid), the oxidation leads to the formation of malic acid and oxaloacetic acid via the Russell mechanism [16,43], oxaloacetic acid and hydrogen peroxide via Bennett and Summers mechanism [44], or by the fragmentation of the tetroxide which results in oxygen and an alkoxy radical [42]. These alkoxy radicals can react in a subsequent step to a CO₂⁻ radical and 3-oxopropanoic acid [15]. A second possibility is the intramolecular H-shift of the alkoxy radical, which leads in a subsequent reaction to oxaloacetic acid and a hydroperoxyl radical (HO₂) [42]. The alkoxy radical might also react with another organic molecule and undergo an H atom abstraction reaction to form an alkyl radical and malic acid, while the contribution for this process is small. Hence, this reaction plays a role only in highly concentrated solutions, as they can occur in aerosols. In addition, the tetroxide decays by disproportionation in the solvent cage to yield 3-hydroxypropionic acid proposed by Alterie et al. [11]. In a study using aerosol flow tube experiments from Chan et al. [17] it was reported that the oxidation products of succinic acid are mainly C₄-compounds, but fragmentation species such as oxomalonic acid (C₃) and glyoxylic acid (C₂) were also found.

Similarly, pimelic acid oxidation by OH radicals takes place at the α-position and leads to the main reaction products 2-hydroxyheptanedioic acid and 2-oxoheptanedioic acid via the Russell mechanism and chain shortening products such as 6-oxohexanoic acid.

3.2. Activation Parameters

The Gibbs free energy of activation, enthalpy of activation, and entropy of activation were derived as follows: the Gibbs free energy of activation, ΔG^‡, was calculated using the Eyring equation [35] (Equation (12)). The parameter k_B and h depict the Boltzmann’s constant and Planck’s constant

$$\mathrm{k}(\mathrm{T})=\frac{{\mathrm{k}}_{\mathrm{B}}\mathrm{T}}{\mathrm{h}}\cdot \mathrm{e}\left(\raisebox{1ex}{$-{\mathsf{\Delta }\mathrm{G}}^{‡}$}\!\left/ \!\raisebox{-1ex}{$\mathrm{RT}$}\right.\right){/\mathrm{L}\mathrm{mol}}^{-1}{\mathrm{s}}^{-1}$$

(12)

The enthalpy of activation, ΔH^‡, is related to the activation energy, E_A, as follows in Equation (13)

$${\mathsf{\Delta }\mathrm{H}}^{‡}{=\mathrm{E}}_{\mathrm{A}}-{\mathrm{RT}/\mathrm{kJ}\mathrm{mol}}^{-1}$$

(13)

The entropy of activation, ΔS^‡, was derived from the pre-exponential factor, A, based on Equation (14)

$${\mathsf{\Delta }\mathrm{S}}^{‡}=\mathrm{R}\left(\ln \mathrm{A}-\ln \left(\frac{{\mathrm{k}}_{\mathrm{B}}\mathrm{T}}{\mathrm{h}}\right)-1\right){/\mathrm{J}\mathrm{K}}^{-1}{\mathrm{mol}}^{-1}$$

(14)

The activation parameters derived for the investigated OH radical oxidation reactions are summarized in

Table 3. Activation parameters of the studied OH reactions in aqueous solution. Arrhenius pre-exponential factor, A, and activation energy, E_A, Gibbs free energy, ΔG^‡, enthalpy, ΔH^‡, and entropy, ΔS^‡, of activation.

Compounds	A/L mol−1 s−1	EA/kJ mol−1	ΔH‡/kJ mol−1	ΔS‡/J K−1 mol−1	ΔG‡/kJ mol−1
Succinic acid
Diacid	(2.1 ± 0.1) · 1010	13 ± 2	10 ± 2	−(56 ± 3)	27 ± 7
Monoanion	(1.5 ± 0.1) · 1010	9 ± 3	7 ± 3	−(57 ± 4)	24 ± 11
Dianion	(2.9 ± 0.2) · 1011	15 ± 3	13 ± 3	−(34 ± 2)	23 ± 7
Pimelic acid
Diacid	(7.3 ± 0.2) · 1010	9 ± 1	6 ± 1	−(45 ± 1)	20 ± 4
Monoanion	(1.8 ± 0.1) · 1011	10 ± 2	8 ± 2	−(38 ± 2)	19 ± 6
Dianion	(1.4 ± 0.1) · 1012	15 ± 1	13 ± 1	−(21 ± 1)	19 ± 2

.

The value of the activation energy, E_A, slightly increase by changing the pH value as well as the derived pre-exponential factors, A, increases. Positive values for enthalpy, ∆H^‡, in the range from 6 to 10 kJ mol⁻¹ for acidic conditions and 13 kJ mol⁻¹ for alkaline conditions were obtained. For both reactants, the negative values of the entropy, ΔS^‡, increase to less negative values by increasing the pH, while the pH-dependence of pimelic acid is stronger than that of succinic acid. In contrast to ∆H^‡, the calculated values for the free Gibbs enthalpy, ΔG^‡, do not show a strong pH-dependency. The monoanionic form of succinic acid has a smaller pre-exponential factor and a lower activation energy than the fully protonated or deprotonated form. In contrast, the monoanionic speciation of pimelic acid lies exactly between these two forms, which might be explained by the larger contribution of the H atom abstraction. The obtained values of the activation parameters are comparable with the literature values for the H-atom abstraction reactions of short-chain carbon compounds such as acids, dicarboxylic acids, diols, and ketones [14,28,29,45].

The obtained room temperature (T = 298 K) rate constants from the present study are compared with the available literature data in

Table 4. Second-order kinetic rate constants for the reactions of the studied dicarboxylic acids with OH in aqueous solution. Bold printing corresponds to data from the present study.

Compounds	kOH/L mol−1 s−1	Remarks	Reference
Succinic acid
AH2	1.0 · 108 a	pH = 2, T = not reported/room temperature, PR-comp. kinetics, ref. thymine (slope 0.023)	[46]
	1.33 · 108 a	pH = 2.0–2.2, T = 303.15 K, Fenton type-comp. kinetics, ref. methanol (slope 0.097)	[24]
	(3.1 ± 0.3) · 108 a	pH = 1.5–10, T = 298.25 K, PR-build-up kinetics at λ = 250–350 nm, (pKa 4.16, 5.61)	[47]
	(1.1 ± 0.1) · 108 a, b (1.06 ± 0.1) · 108 c	pH = 1.5, T = 298.15 K, PR-comp. kinetics, ref. SCN− (slope 0.0089), T-dependent measurement, (pKa 4.16, 5.61)	[19]d
	1.1 · 108	Estimation method	[48]
	7.7 · 107	Estimation method	[49]
	(1.3 ± 0.2) · 108 b	pH = 2, T = 298.15 K	This work
AH−	2.0 · 108	Estimation method	[49]
	(5.1 ± 0.1) · 108 b	pH = 4.9, T = 298.15 K	This work
A2−	(5.3 ± 0.5) · 108 a	pH = 9, borax buffer, T = 293.15 K, FP-comp. kinetics, ref. 4-ferrocenyl-butanoate (slope 0.049)	[21]
	(5.0 ± 0.5) · 108 a, b (4.8 ± 0.48) · 108 c	pH = 7.8, T = 298.15 K, LFP-comp. kinetics, ref. SCN- (slope 0.0403), T-dependent measurement, (pKa 4.16, 5.61)	[19]
	4.6 · 108	Estimation method	[49]
	(5.3 ± 0.2) · 108 b	pH = 8, T = 298.15 K	This work
Pimelic acid
AH2	2.4 · 109 a 3.5 · 109 e	pH = 2, T = not reported/room temperature, PR-comp. kinetics, ref. thymine (slope 0.558)	[46]
	(2.4 ± 0.7) · 109	pH = 2, T = 298.15 K, LFP-build-up kinetics at λ = 244 nm	[29]
	4.3 · 109	Estimation method	[48]
	1.6 · 109	Estimation method	[49]
	(2.2 ± 0.1) · 109 b	pH = 2, T = 298.15 K	This work
AH−	2.3 · 109	Estimation method	[49]
	(3.4 ± 0.1) · 109 b	pH = 4.6, T = 298.15 K	This work
A2−	(2.9 ± 0.8) · 109	pH = 9, T = 298.15 K, LFP-build-up kinetics at λ = 244 nm	[29]
	3.0 · 109	Estimation method	[49]
	(3.2 ± 0.1) · 109 b	pH = 8, T = 298.15 K	This work

LFP—laser flash photolysis, FP—Flash photolysis, PR—pulse radiolysis, ^a Reported in the publication, ^b reference constant k(OH + SCN⁻) = 1.24 · 10¹⁰ L mol⁻¹ s⁻¹ [50], ^c reference constant k(OH + SCN⁻) = 1.19 · 10¹⁰ L mol⁻¹ s⁻¹ [27], ^d in the original publication by Ervens et al. [19] the values of the rate constant of the fully protonated and fully deprotonated form of succinic in Data Table 11 of Ervens et al., [19] were confused with each other, while the expressions in the abstract are correct. ^e NIST Solution Kinetics Database [51].

.

Most of the rate constants for succinic acid were measured by relative-rate techniques, with the exception of the study by Cabelli and Bielski [47]. In this study, the authors used the product build-up kinetic method at λ = 250–330 nm and reported a pH-independent rate constant k(298 K) = (3.1 ± 0.3) · 10⁸ L mol⁻¹ s⁻¹. These findings are in contradiction to the observed rate constants for succinic acid and other carboxylic acids [9,14,20,21,22,29,46,52]. Ervens et al. also described the oxidation of succinic acid by OH radicals, but in this study the monoanionic form was not investigated [19]. It should be mentioned that a source of uncertainty in the rate constants could be the use of different reference constants (cf. Table 4). For example, in the study by Ervens et al. [19] a slightly larger reference constant was used. Correcting the given rate constants for succinic acid with the reference constant used in this study by Zhu et al. [27] results in smaller rate constants k(AH₂) = (1.06 ± 0.1) · 10⁸ L mol⁻¹ s⁻¹ and k(A²⁻) = (4.8 ± 0.48) · 10⁸ L mol⁻¹ s⁻¹. Chan et al. [17] reported an OH radical rate constant k = (7.4 ± 2.4) · 10⁷ L mol⁻¹ s⁻¹ by analyzing succinic acid decomposition with DART mass spectrometry in their aerosol flow tube experiments. Under the conditions used, most succinic acid should be present in the fully protonated form with small contributions of the monoanionic form and fits quiet well to the received rate constants from this study and reported rate constants in Table 4 [17]. However, the OH radical rate constants of succinic acid obtained from this study agree well with the reported values for the low pH range (cf.

Table 5. Atmospheric aqueous-phase lifetimes calculated for urban and remote areas.

Compounds	Cloud Case Urban	Cloud Case Remote	Aerosol Case Urban	Aerosol Case Remote
	[OH] = 3.5 · 10−15 /mol L−1	[OH] = 2.2 · 10−14 /mol L−1	[OH] = 4.4 · 10−13 /mol L−1	[OH] = 3.0 · 10−12 /mol L−1
Succinic acid
AH2	25.4–41.9 d	4.0–6.7 d	4.9–8.0 h	43–70 min
AH−	6.2–7.7 d	23.8–29.4 h	72–88 min	11–13 min
A2−	6.5–11 d	24.8–42.1 h	74–126 min	11–19 min
Pimelic acid
AH2	36.1–46.7 h	5.7–7.4 h	17–22 min	2–3 min
AH−	24.8–41.8 h	3.9–6.6 h	12–20 min	2–3 min
A2−	24.8–41.8 h	3.9–6.6 h	12–20 min	2–3 min

). Minakata et al. [48] or Doussin and Monod [49] developed two prediction methods for OH radical rate constants in aqueous solution based on structure activity relationships (SAR) (see more details in the Supplementary Materials). The obtained results for pH = 2 are consistent with the rate constant measured in this study. The method by Doussin and Monod [49] also reflects the pH effect on the rate constant for both acids, since it includes different group increments for carboxyl-/carboxylate group. The results of the OH radical reaction with pimelic acid are comparable with measurements using a build-up kinetic method [29] as well as relative-rate technique [46].

In the following Figure 4, the second-order OH radical rate constants are plotted against the carbon chain length for the different speciation of various mono- and dicarboxylic acids (cf. Tables S1 and S2). As expected, a significant increase in the reaction rate is shown in Figure 4 by increasing chain length of the dicarboxylic acids. This is because the length of the alkene backbone influences the number of abstractable hydrogen atoms and so the probability of an effective collision increases. Furthermore, the steric hindrance by the terminal carboxyl groups also contributes to these results, since the reaction rate of the OH radical with the carboxylic acid group is lower compared to the CH₂ group. This behavior is expressed in the estimation methods by the different fragments of the respective functional group [48,49].

The pH dependence of the OH radical reaction of dicarboxylic acids could be explained by the following effects: (i) the increasing contribution of an electron transfer reaction between the carboxylate group and the OH radical, (ii) an increased attraction between the carboxylate group and the electrophilic OH radical by electrostatic interactions, (iii) the reduction of the bond dissociation energy (BDE) of the C-H bond by deprotonation of the carboxyl function, and (iv) the formation of a ring-shaped molecular structure by intramolecular interactions of the carboxylate group with the CH₂ group. The formation of a ring-shaped molecular structure can be neglected, since significantly greater effects can be seen with the shorter dicarboxylic acid. Even the influence of the deprotonation of the carboxyl function on the BDE of the adjacent C-H bond cannot explain the change by one order of magnitude, since the change of the BDE is too small. According to the data summarized in Hoffmann et al. [37], the change in BDE must be more than 10 kJ mol⁻¹ in order for the OH radical rate constant to increase by more than one order of magnitude. Hence, the increase of the OH radical rate constant could be explained by a greater attraction leading to an increase in the number of molecular collisions or, more likely, by the contribution of an electron transfer reaction. If the carboxyl group is deprotonated, the electron transfer reaction can take place, this reaction pathway is inhibited by the protonation of the carboxyl group and the H atom abstraction from the OH group is a very slow process due to the higher BDE, which leads to a reduction of the rate constant. This should be clarified by further studies using ab initio theoretical methods.

4. Atmospheric Implications

It was the main objective of the present study to clarify the influence of the pH and temperature on the rate constant for the reactions of OH with succinic and pimelic acid in their respective three forms.

The performed kinetic measurements allow us to estimate the lifetime of the studied dicarboxylic acids in the atmospheric aqueous phase. Atmospheric aqueous phase lifetimes (τ) were calculated in this context by the following simple equation (Equation (15)).

$$\mathsf{\tau }={(\mathrm{k}\cdot {[\mathrm{OH}]}_0)}^{-1}/\mathrm{s}$$

(15)

The following OH model concentrations were used for different scenarios: an urban cloud case of 3.5 · 10⁻¹⁵ mol L⁻¹, a remote cloud case of 2.2 · 10⁻¹⁴ mol L⁻¹, an urban aerosol case of 4.4 · 10⁻¹³ mol L⁻¹, and a remote aerosol case of 3.0 · 10⁻¹² mol L⁻¹ [14]. Based on the assumption that the OH radicals present reacts exclusively with the dicarboxylic acid, the following lifetimes in the aqueous phase were obtained, using the rate constants at T = 278 K and T = 298 K to illustrate the atmospheric conditions (cf. Table 5).

Depending on the scenario and the used temperature, the calculated atmospheric lifetimes can range from a few minutes to a few days. The lifetime in aerosols is, as expected, considerably shorter than in clouds (Table 5). The tropospheric water phase can occur at both low and very high pH values. Both concentrated deliquescent particles and diluted cloud droplets can have a wide pH range from strongly acidic to slightly alkaline [9]. Therefore, all three species of the dicarboxylic acids AH₂, AH⁻, and A²⁻ can play a role in the tropospheric oxidation process of the investigated DCAs (cf. Figure 1). In urban and continental aerosol particles with a lower pH from zero to five mainly the fully protonated form AH₂ is present. The monoanionic form of both dicarboxylic acid occurs mainly in moderate tropospheric pH regimes from 3.0 to 6.5 as found in cloud droplets or rain. Finally, the fully deprotonated form could be present in marine aerosols, haze, and fog with elevated pH values up to nine.

Figure 5 shows the overall rate constant for T = 278 K and 298 K as function of the pH. The overall rate constant was calculated by using the Equation (2), the acid–base equilibrium contributions, and the individual rate constants of the respective speciation of the dicarboxylic acids. The OH radical oxidation generally results in further oxygenated compounds, which then can undergo further subsequent accretion reactions such as aldol, acetal, and ester formation. The before-mentioned accretion processes can become more important when droplet evaporation occurs [9,53], due to the presence of more oxygenated dicarboxylic acid or smaller dicarboxylic acids such as malic acid and oxaloacetic acid from the OH oxidation process and the water loss in the droplet. In addition, the lifetime of an aerosol particle can be estimated at about 5 days, whereas the lifetime of a cloud droplet is shorter than 18 h. This means that the tropospheric lifetime of dicarboxylic acids corresponds more to the lifetime of the aerosol phase. In addition, because of the different reactivity of dicarboxylic acids, succinic acid has a longer lifetime than pimelic acid, which explains the findings of Teich et al. [7]. Since pH dependence occurs during the oxidation of dicarboxylic acids, pH under cloud droplet conditions plays an important role, whereas due to the high acidity in aerosol particles the pH influence plays a minor role.

5. Conclusions

Within the present study, the pH- and temperature-dependent oxidation reactions of succinic acid and pimelic acid were investigated to determine the OH radical rate constants. The temperature-dependency of the acid-base equilibria of the dicarboxylic acids shows no significant influence of the ratio of the different species AH₂, AH⁻, or A²⁻. Moreover, the internal absorption effect of the used compounds can also be neglected, since the molar attenuation coefficient is too small for a significant contribution under the used conditions. The internal absorption effect can occur when using the competition kinetics, leading to a virtual increase of the measured rate constant, but this effect can be neglected for the two dicarboxylic acids under the conditions used. The following behavior of the OH radical rate constants was observed: the rate constant increases with increasing pH, due to the deprotonation of the carboxylic acid group of the molecule and thus the possible contribution of an electron transfer reaction increases. This effect is much stronger with small dicarboxylic acids than with long-chain dicarboxylic acids. The results obtained were compared with SAR prediction tools and revealed a very good agreement between the measured and the estimated values. Furthermore, the tropospheric lifetime of dicarboxylic acids was estimated to range from a few minutes under aerosol conditions to several hours under cloud conditions. This oxidation reaction by OH radicals can act as a sink for dicarboxylic acids, as well as a source of smaller dicarboxylic acids or multifunctional compounds.