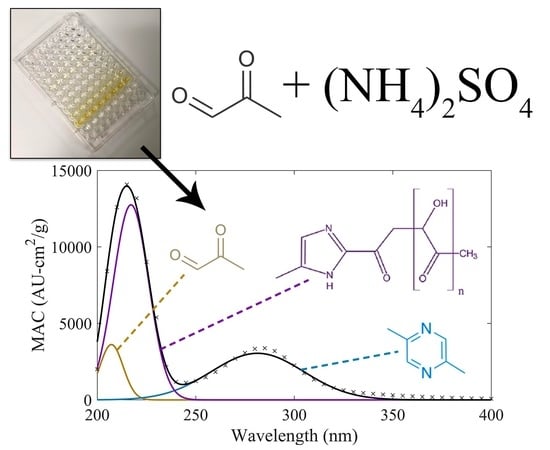
Modeling of Carbonyl/Ammonium Sulfate Aqueous Brown Carbon Chemistry via UV/Vis Spectral Decomposition
Abstract
1. Introduction
2. Materials and Methods
2.1. Sample Collection
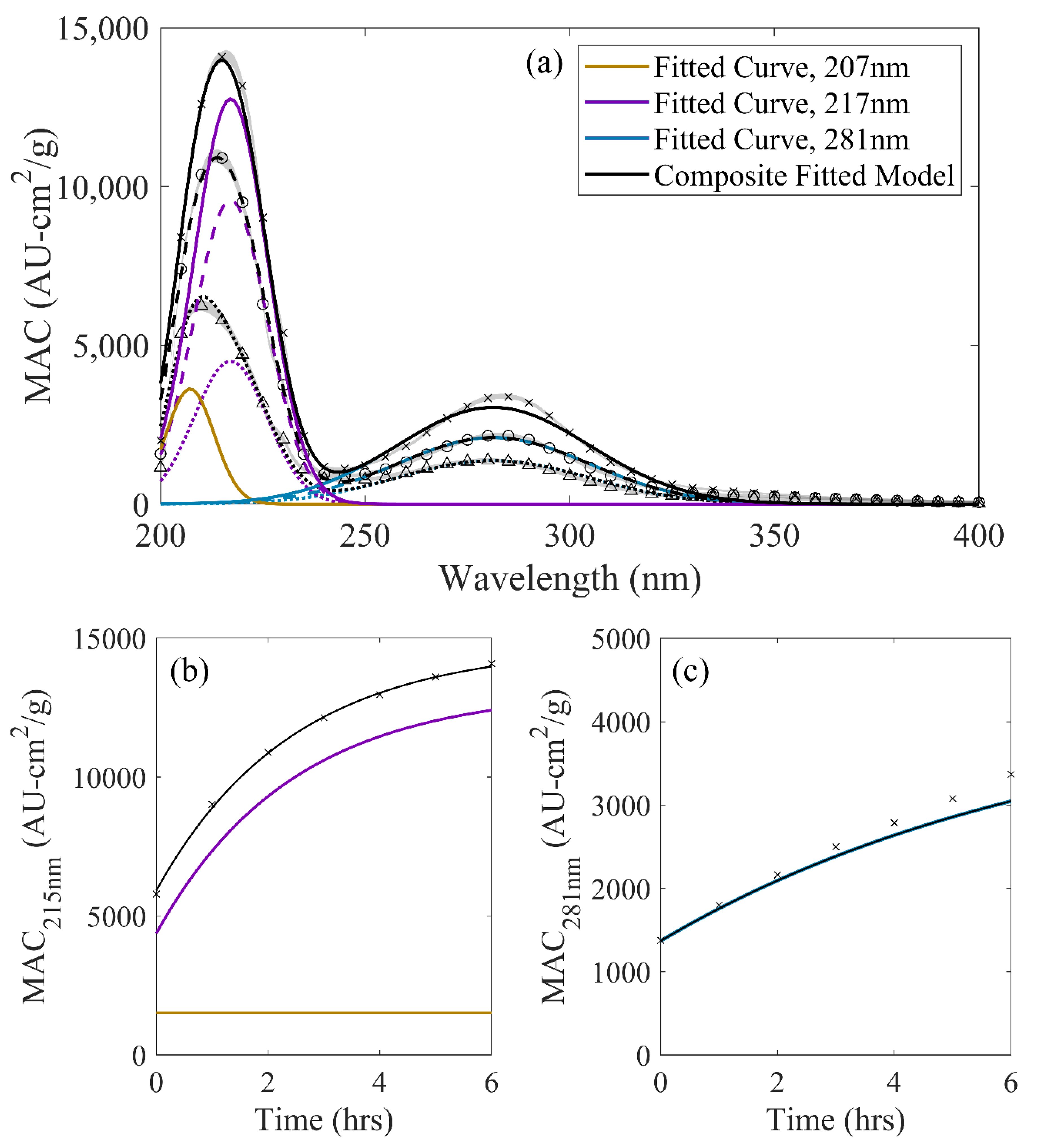
2.2. Modeling Approach
3. Results
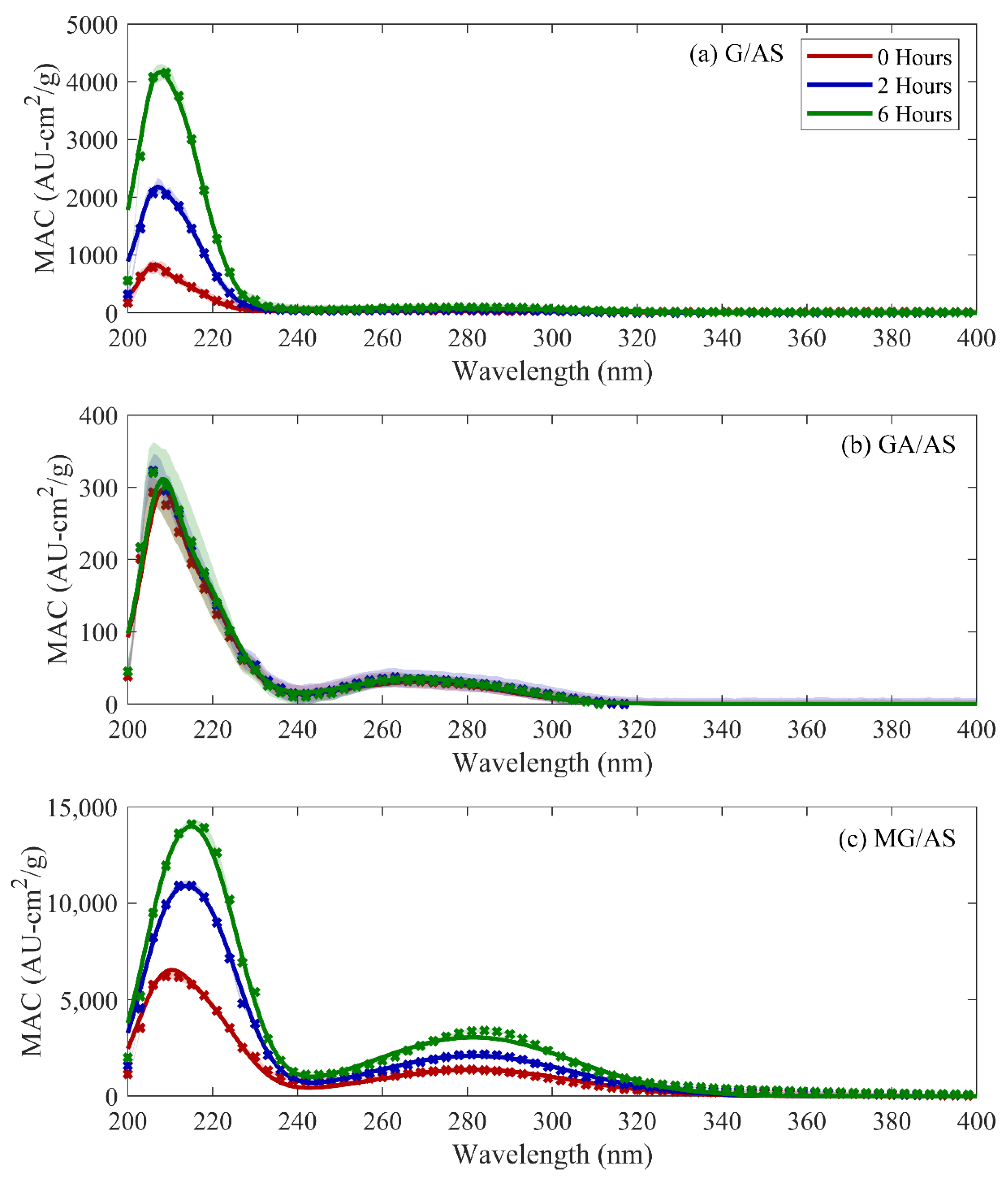
3.1. UV-Visible Absorbance of Aqueous Mimic Solutions
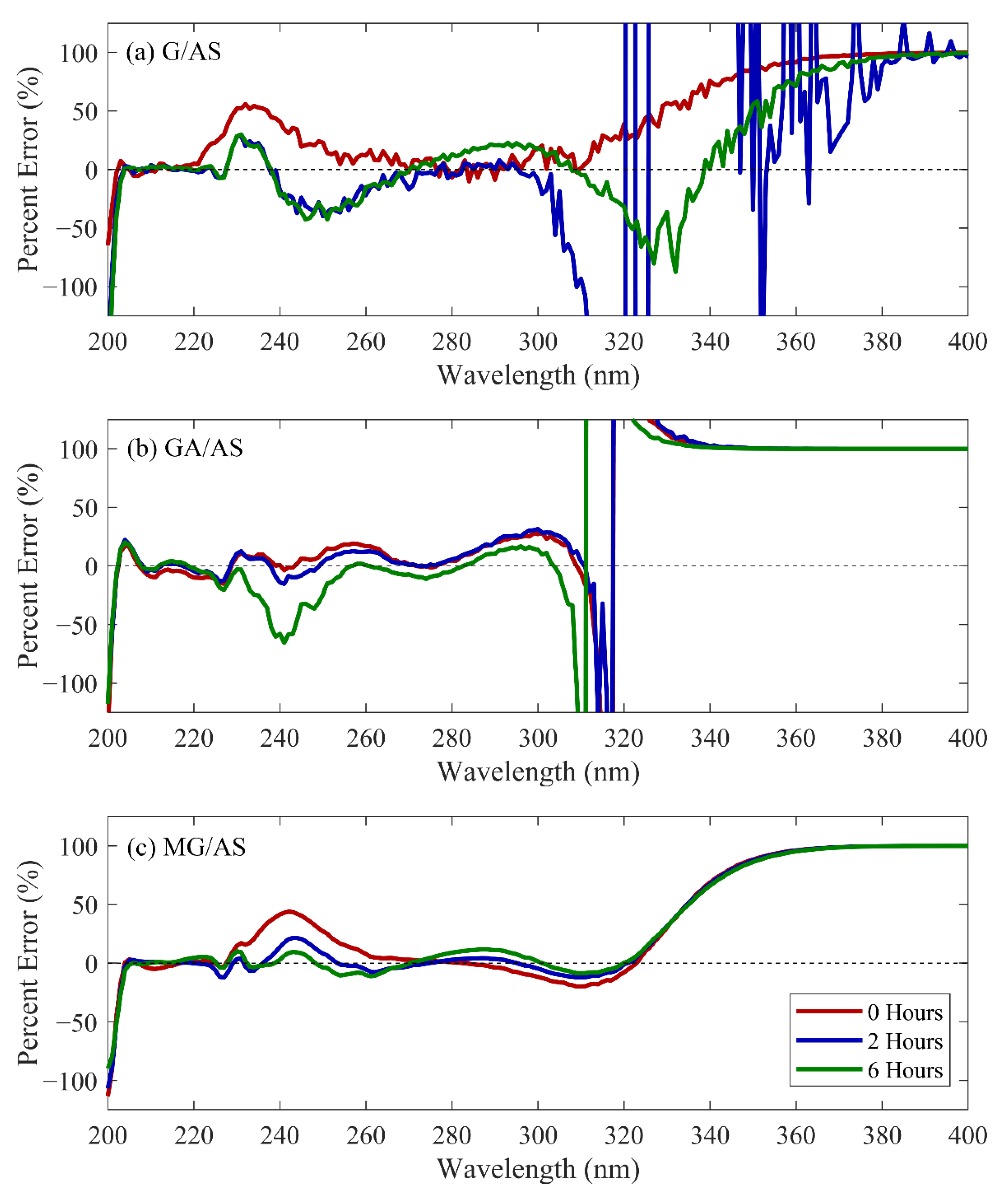
3.2. Model Comparisons
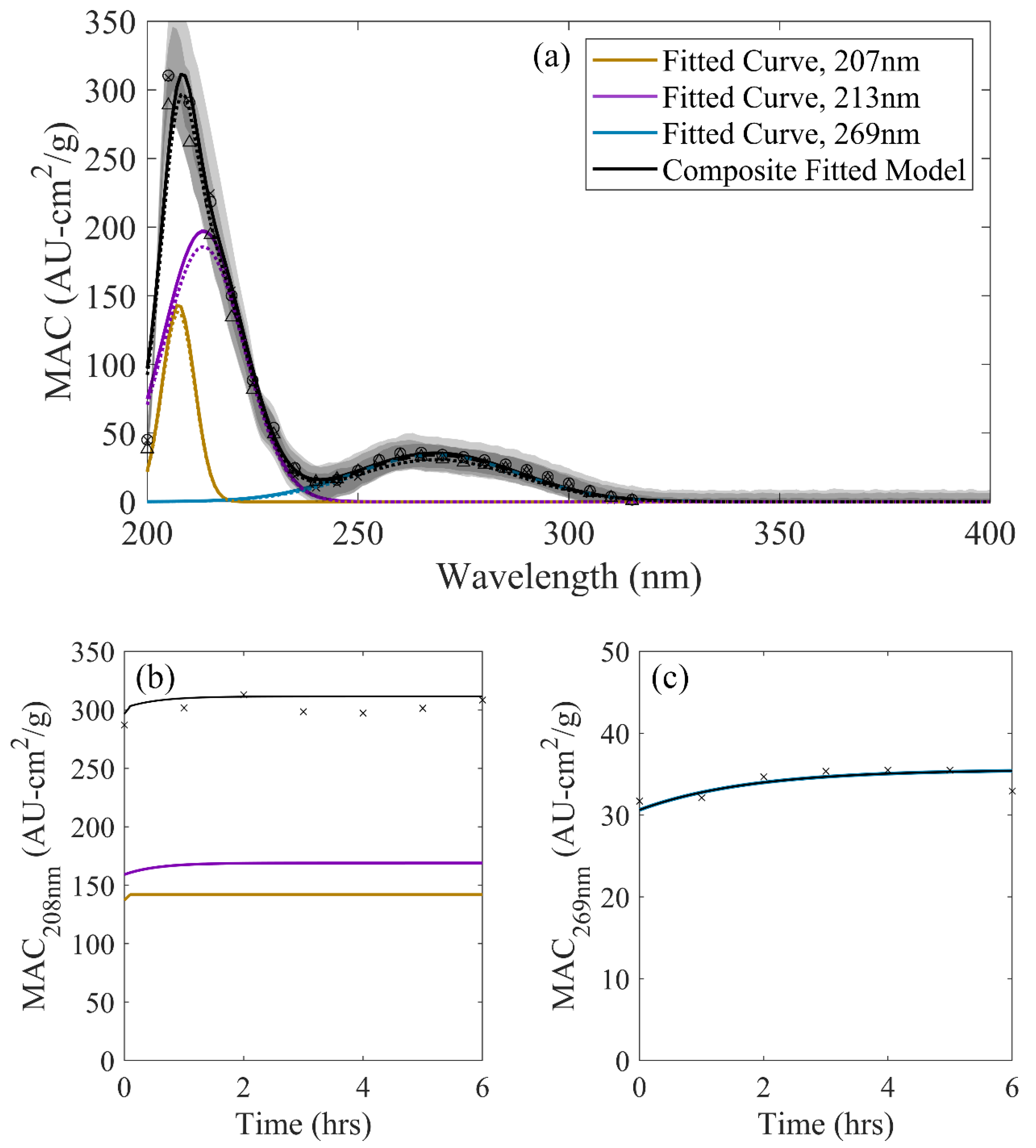
4. Inference of Kinetics from Fitted Time Constants
4.1. Glyoxal + AS
4.2. Glycolaldehyde + AS
4.3. Methylglyoxal + AS
5. Atmospheric Implications
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- McNeill, V.F. Aqueous organic chemistry in the atmosphere: Sources and chemical processing of organic aerosols. Environ. Sci. Technol. 2015, 49, 1237–1244. [Google Scholar] [CrossRef] [PubMed]
- Herrmann, H.; Schaefer, T.; Tilgner, A.; Styler, S.A.; Weller, C.; Teich, M.; Otto, T. Tropospheric Aqueous-Phase Chemistry: Kinetics, Mechanisms, and Its Coupling to a Changing Gas Phase. Chem. Rev. 2015, 115, 4259–4334. [Google Scholar] [CrossRef] [PubMed]
- Ervens, B.; Turpin, B.J.; Weber, R.J. Secondary organic aerosol formation in cloud droplets and aqueous particles (aqSOA): A review of laboratory, field and model studies. Atmos. Chem. Phys. 2011, 11, 11069–11102. [Google Scholar] [CrossRef]
- Faust, J.A.; Wong, J.P.S.; Lee, A.K.Y.; Abbatt, J.P.D. Role of Aerosol Liquid Water in Secondary Organic Aerosol Formation from Volatile Organic Compounds. Environ. Sci. Technol. 2017, 51, 1405–1413. [Google Scholar] [CrossRef]
- Carlton, A.G.; Turpin, B.J. Particle partitioning potential of organic compounds is highest in the Eastern US and driven by anthropogenic water. Atmos. Chem. Phys. 2013, 13, 10203–10214. [Google Scholar] [CrossRef]
- Volkamer, R.; San Martini, F.; Molina, L.T.; Salcedo, D.; Jimenez, J.L.; Molina, M.J. A missing sink for gas-phase glyoxal in Mexico City: Formation of secondary organic aerosol. Geophys. Res. Lett. 2007, 34, L19807. [Google Scholar] [CrossRef]
- Ervens, B.; Carlton, A.G.; Turpin, B.J.; Altieri, K.E.; Kreidenweis, S.M.; Feingold, G. Secondary organic aerosol yields from cloud-processing of isoprene oxidation products. Geophys. Res. Lett. 2008, 35, L02816. [Google Scholar] [CrossRef]
- Hecobian, A.; Zhang, X.; Zheng, M.; Frank, N.; Edgerton, E.S.; Weber, R.J. Water-Soluble Organic Aerosol material and the light-absorption characteristics of aqueous extracts measured over the Southeastern United States. Atmos. Chem. Phys. 2010, 10, 5965–5977. [Google Scholar] [CrossRef]
- Fu, T.-M.; Jacob, D.J.; Wittrock, F.; Burrows, J.P.; Vrekoussis, M.; Henze, D.K. Global budgets of atmospheric glyoxal and methylglyoxal, and implications for formation of secondary organic aerosols. J. Geophys. Res. 2008, 113, D15303. [Google Scholar] [CrossRef]
- Ervens, B.; Volkamer, R. Glyoxal processing by aerosol multiphase chemistry: Towards a kinetic modeling framework of secondary organic aerosol formation in aqueous particles. Atmos. Chem. Phys. 2010, 10, 8219–8244. [Google Scholar] [CrossRef]
- Liggio, J.; Li, S.; McLaren, R. Reactive uptake of glyoxal by particulate matter. J. Geophys. Res. 2005, 110, D10304. [Google Scholar] [CrossRef]
- Galloway, M.M.; Chhabra, P.S.; Chan, A.W.H.; Surratt, J.D.; Flagan, R.C.; Seinfeld, J.H.; Keutsch, F.N. Glyoxal uptake on ammonium sulphate seed aerosol: Reaction products and reversibility of uptake under dark and irradiated conditions. Atmos. Chem. Phys. 2009, 9, 3331–3345. [Google Scholar] [CrossRef]
- De Haan, D.O.; Corrigan, A.L.; Tolbert, M.A.; Jimenez, J.L.; Wood, S.E.; Turley, J.J. Secondary Organic Aerosol Formation by Self-Reactions of Methylglyoxal and Glyoxal in Evaporating Droplets. Environ. Sci. Technol. 2009, 43, 8184–8190. [Google Scholar] [CrossRef] [PubMed]
- De Haan, D.O.; Hawkins, L.N.; Kononenko, J.A.; Turley, J.J.; Corrigan, A.L.; Tolbert, M.A.; Jimenez, J.L. Formation of Nitrogen-Containing Oligomers by Methylglyoxal and Amines in Simulated Evaporating Cloud Droplets. Environ. Sci. Technol. 2011, 45, 984–991. [Google Scholar] [CrossRef]
- Galloway, M.M.; Loza, C.L.; Chhabra, P.S.; Chan, A.W.H.; Yee, L.D.; Seinfeld, J.H.; Keutsch, F.N. Analysis of photochemical and dark glyoxal uptake: Implications for SOA formation. Geophys. Res. Lett. 2011, 38. [Google Scholar] [CrossRef]
- McNeill, V.F.; Woo, J.L.; Kim, D.D.; Schwier, A.N.; Wannell, N.J.; Sumner, A.J.; Barakat, J.M. Aqueous-Phase Secondary Organic Aerosol and Organosulfate Formation in Atmospheric Aerosols: A Modeling Study. Environ. Sci. Technol. 2012, 46, 8075–8081. [Google Scholar] [CrossRef]
- Powelson, M.H.; Espelien, B.M.; Hawkins, L.N.; Galloway, M.M.; De Haan, D.O. Brown Carbon Formation by Aqueous-Phase Carbonyl Compound Reactions with Amines and Ammonium Sulfate. Environ. Sci. Technol. 2014, 48, 985–993. [Google Scholar] [CrossRef]
- Grace, D.N.; Sharp, J.R.; Holappa, R.E.; Lugos, E.N.; Sebold, M.B.; Griffith, D.R.; Hendrickson, H.P.; Galloway, M.M. Heterocyclic Product Formation in Aqueous Brown Carbon Systems. ACS Earth Space Chem. 2019, 3, 2472–2481. [Google Scholar] [CrossRef]
- Schwier, A.N.; Sareen, N.; Mitroo, D.; Shapiro, E.L.; McNeill, V.F. Glyoxal-Methylglyoxal Cross-Reactions in Secondary Organic Aerosol Formation. Environ. Sci. Technol. 2010, 44, 6174–6182. [Google Scholar] [CrossRef]
- Lin, P.; Laskin, J.; Nizkorodov, S.A.; Laskin, A. Revealing Brown Carbon Chromophores Produced in Reactions of Methylglyoxal with Ammonium Sulfate. Environ. Sci. Technol. 2015, 49, 14257–14266. [Google Scholar] [CrossRef]
- Sareen, N.; Schwier, A.N.; Shapiro, E.L.; Mitroo, D.; McNeill, V.F. Secondary organic material formed by methylglyoxal in aqueous aerosol mimics. Atmos. Chem. Phys. 2010, 10, 997–1016. [Google Scholar] [CrossRef]
- Sareen, N.; Schwier, A.N.; Lathem, T.L.; Nenes, A.; McNeill, V.F. Surfactants from the gas phase may promote cloud droplet formation. Proc. Natl. Acad. Sci. USA 2013, 110, 2723–2728. [Google Scholar] [CrossRef] [PubMed]
- Beier, T.; Cotter, E.R.; Galloway, M.M.; Woo, J.L. In Situ Surface Tension Measurements of Hanging Droplet Methylglyoxal/Ammonium Sulfate Aerosol Mimics under Photooxidative Conditions. ACS Earth Space Chem. 2019, 3, 1208–1215. [Google Scholar] [CrossRef]
- Galloway, M.M.; Powelson, M.H.; Sedehi, N.; Wood, S.E.; Millage, K.D.; Kononenko, J.A.; Rynaski, A.D.; De Haan, D.O. Secondary Organic Aerosol Formation during Evaporation of Droplets Containing Atmospheric Aldehydes, Amines, and Ammonium Sulfate. Environ. Sci. Technol. 2014, 48, 14417–14425. [Google Scholar] [CrossRef]
- Nozière, B.; Dziedzic, P.; Córdova, A. Products and Kinetics of the Liquid-Phase Reaction of Glyoxal Catalyzed by Ammonium Ions (NH4+ ). J. Phys. Chem. A 2009, 113, 231–237. [Google Scholar] [CrossRef] [PubMed]
- Loeffler, K.W.; Koehler, C.A.; Paul, N.M.; De Haan, D.O. Oligomer Formation in Evaporating Aqueous Glyoxal and Methyl Glyoxal Solutions. Environ. Sci. Technol. 2006, 40, 6318–6323. [Google Scholar] [CrossRef]
- Stangl, C.M.; Johnston, M.V. Aqueous Reaction of Dicarbonyls with Ammonia as a Potential Source of Organic Nitrogen in Airborne Nanoparticles. J. Phys. Chem. A 2017, 121, 3720–3727. [Google Scholar] [CrossRef]
- Shapiro, E.L.; Szprengiel, J.; Sareen, N.; Jen, C.N.; Giordano, M.R.; McNeill, V.F. Light-absorbing secondary organic material formed by glyoxal in aqueous aerosol mimics. Atmos. Chem. Phys. Discuss. 2009, 9, 59–80. [Google Scholar] [CrossRef]
- Woo, J.L.; Kim, D.D.; Schwier, A.N.; Li, R.; McNeill, V.F. Aqueous aerosol SOA formation: Impact on aerosol physical properties. Faraday Discuss. 2013, 165, 357–367. [Google Scholar] [CrossRef]
- Hawkins, L.N.; Lemire, A.N.; Galloway, M.M.; Corrigan, A.L.; Turley, J.J.; Espelien, B.M.; De Haan, D.O. Maillard Chemistry in Clouds and Aqueous Aerosol As a Source of Atmospheric Humic-Like Substances. Environ. Sci. Technol. 2016, 50, 7443–7452. [Google Scholar] [CrossRef]
- Yi, Y.; Cao, Z.; Zhou, X.; Xue, L.; Wang, W. Formation of aqueous-phase secondary organic aerosols from glycolaldehyde and ammonium sulfate/amines: A kinetic and mechanistic study. Atmos. Environ. 2018, 181, 117–125. [Google Scholar] [CrossRef]
- Hawkins, L.N.; Welsh, H.G.; Alexander, M.V. Evidence for pyrazine-based chromophores in cloud water mimics containing methylglyoxal and ammonium sulfate. Atmos. Chem. Phys. 2018, 18, 12413–12431. [Google Scholar] [CrossRef]
- Grace, D.N.; Sebold, M.B.; Galloway, M.M. Separation and detection of aqueous atmospheric aerosol mimics using supercritical fluid chromatography-mass spectrometry. Atmos. Meas. Tech 2019, 12, 3841–3851. [Google Scholar] [CrossRef]
- Aiona, P.K.; Lee, H.J.; Leslie, R.; Lin, P.; Laskin, A.; Laskin, J.; Nizkorodov, S.A. Photochemistry of Products of the Aqueous Reaction of Methylglyoxal with Ammonium Sulfate. ACS Earth Space Chem. 2017, 1, 522–532. [Google Scholar] [CrossRef]
- de Levie, R. On deconvolving spectra. Am. J. Phys. 2004, 72, 910–915. [Google Scholar] [CrossRef]
- Barker, B.E.; Fox, M.F. Computer resolution of overlapping electronic absorption bands. Chem. Soc. Rev. 1980, 9, 143–184. [Google Scholar] [CrossRef]
- Tang, M.; Alexander, J.M.; Kwon, D.; Estillore, A.D.; Laskina, O.; Young, M.A.; Kleiber, P.D.; Grassian, V.H. Optical and Physicochemical Properties of Brown Carbon Aerosol: Light Scattering, FTIR Extinction Spectroscopy, and Hygroscopic Growth. J. Phys. Chem. A 2016, 120, 4155–4166. [Google Scholar] [CrossRef]
- Li, Z.; Schwier, A.N.; Sareen, N.; McNeill, V.F. Reactive processing of formaldehyde and acetaldehyde in aqueous aerosol mimics: Surface tension depression and secondary organic products. Atmos. Chem. Phys. 2011, 11, 11617–11629. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16; Gaussian, Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Woodward, R.B. Structure and the Absorption Spectra of α,β-Unsaturated Ketones. J. Am. Chem. Soc. 1941, 63, 1123–1126. [Google Scholar] [CrossRef]
- Liljefors, T.; Allinger, N.L. Conformational analysis. 128. The Woodward-Fieser rules and α,β-unsaturated ketones. J. Am. Chem. Soc. 1978, 100, 1068–1073. [Google Scholar] [CrossRef]
- Fieser, L.F.; Fieser, M.; Rajagopalan, S. Absorption Spectroscopy And The Structures Of The Diosterols. J. Org. Chem. 1948, 13, 800–806. [Google Scholar] [CrossRef]
- Ackendorf, J.M.; Ippolito, M.G.; Galloway, M.M. pH Dependence of the Imidazole-2-carboxaldehyde Hydration Equilibrium: Implications for Atmospheric Light Absorbance. Environ. Sci. Technol. Lett. 2017, 4, 551–555. [Google Scholar] [CrossRef]
- Maxut, A.; Nozière, B.; Fenet, B.; Mechakra, H. Formation mechanisms and yields of small imidazoles from reactions of glyoxal with NH4(+) in water at neutral pH. Phys. Chem. Chem. Phys. 2015, 17, 20416–20424. [Google Scholar] [CrossRef]
- Teich, M.; Van Pinxteren, D.; Kecorius, S.; Wang, Z.; Herrmann, H. First Quantification of Imidazoles in Ambient Aerosol Particles: Potential Photosensitizers, Brown Carbon Constituents, and Hazardous Components. Environ. Sci. Technol. 2016, 50, 1166–1173. [Google Scholar] [CrossRef]
- Sareen, N.; Moussa, S.G.; McNeill, V.F. Photochemical Aging of Light-Absorbing Secondary Organic Aerosol Material. J. Phys. Chem. A 2013, 117, 2987–2996. [Google Scholar] [CrossRef]
- Sedehi, N.; Takano, H.; Blasic, V.A.; Sullivan, K.A.; De Haan, D.O. Temperature- and pH-dependent aqueous-phase kinetics of the reactions of glyoxal and methylglyoxal with atmospheric amines and ammonium sulfate. Atmos. Environ. 2013, 77, 656–663. [Google Scholar] [CrossRef]
- Gao, Y.; Zhang, Y. Optical properties investigation of the reactions between methylglyoxal and glycine/ammonium sulfate. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2019, 215, 112–121. [Google Scholar] [CrossRef]

| Species | 0 h Aging | 2 h Aging | 6 h Aging | |||
|---|---|---|---|---|---|---|
| Max % Error | NRMSD | Max % Error | NRMSD | Max % Error | NRMSD | |
| Glyoxal | 55.9 | 0.0100 | 39.9 | 0.0117 | 42.6 | 0.0135 |
| Glycolaldehyde | 27.8 | 0.0125 | 30.5 | 0.0122 | 65.5 | 0.0121 |
| Methylglyoxal | 43.8 | 0.0146 | 21.6 | 0.0104 | 11.7 | 0.0102 |
| Species | Fitted Peak Location (nm) | Potential Structures or Functional Groups | Inferred Rate Constant, (M−1 h−1) |
|---|---|---|---|
| G (2 curves) | 208 | -carbonyls [25], pyrazine derivatives [18], imidazole derivatives [18,43,44] | 0.018 |
| 271 | Pyrazine derivatives [18], aldol condensation products [28], imidazole products [43,44] | --1 | |
| G (3 curves) | 206 | -carbonyls [25], pyrazine derivatives [18], imidazole derivatives [18,43,44] | 0.038 |
| 209 | -carbonyls [25], pyrazine derivatives, imidazole derivatives [18,43] | 0.016 | |
| 267 | Pyrazine derivatives [18], aldol condensation products [28], imidazole products [43,44] | --1 | |
| GA (3 curves) | 207 | -carbonyls [25], pyrazine derivatives, imidazole derivatives [18,43] | --1 |
| 213 | Imidazole derivatives [18,43] | 0.297 | |
| 269 | Pyrazine derivatives [18] | 0.092 | |
| MG (3 curves) | 207 | -carbonyls [25], methylimidazole derivatives [18] | --1 |
| 217 | Methyl ketones [21], dimethylpyrazine derivatives [18] | 0.067 | |
| 281 | Dimethylpyrazine derivatives [18], aldol condensation products [21], methylimidazole derivatives [34] | 0.022 | |
| MG (4 curves) | 207 | -carbonyls [25], methylimidazole derivatives [18] | --1 |
| 218 | Methyl ketones [21], dimethylpyrazine derivatives [18] | 0.066 | |
| 281 | Dimethylpyrazine derivatives [18], aldol condensation products [21], methylimidazole derivatives [34] | 0.016 | |
| 341 | Aldol condensation products [19,21,30] | 0.056 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, M.; Ma, S.; Ferdousi, N.; Dai, Z.; Woo, J.L. Modeling of Carbonyl/Ammonium Sulfate Aqueous Brown Carbon Chemistry via UV/Vis Spectral Decomposition. Atmosphere 2020, 11, 358. https://doi.org/10.3390/atmos11040358
Fan M, Ma S, Ferdousi N, Dai Z, Woo JL. Modeling of Carbonyl/Ammonium Sulfate Aqueous Brown Carbon Chemistry via UV/Vis Spectral Decomposition. Atmosphere. 2020; 11(4):358. https://doi.org/10.3390/atmos11040358
Chicago/Turabian StyleFan, Mengjie, Shiqing Ma, Nahin Ferdousi, Ziwei Dai, and Joseph L. Woo. 2020. "Modeling of Carbonyl/Ammonium Sulfate Aqueous Brown Carbon Chemistry via UV/Vis Spectral Decomposition" Atmosphere 11, no. 4: 358. https://doi.org/10.3390/atmos11040358
APA StyleFan, M., Ma, S., Ferdousi, N., Dai, Z., & Woo, J. L. (2020). Modeling of Carbonyl/Ammonium Sulfate Aqueous Brown Carbon Chemistry via UV/Vis Spectral Decomposition. Atmosphere, 11(4), 358. https://doi.org/10.3390/atmos11040358