A Rapid-Convergence Precise Point Positioning Approach Using Double Augmentations of Low Earth Orbit Satellite and Atmospheric Information
Abstract
:1. Introduction
2. LEO/Atmosphere Double-Augmentation PPP Approach
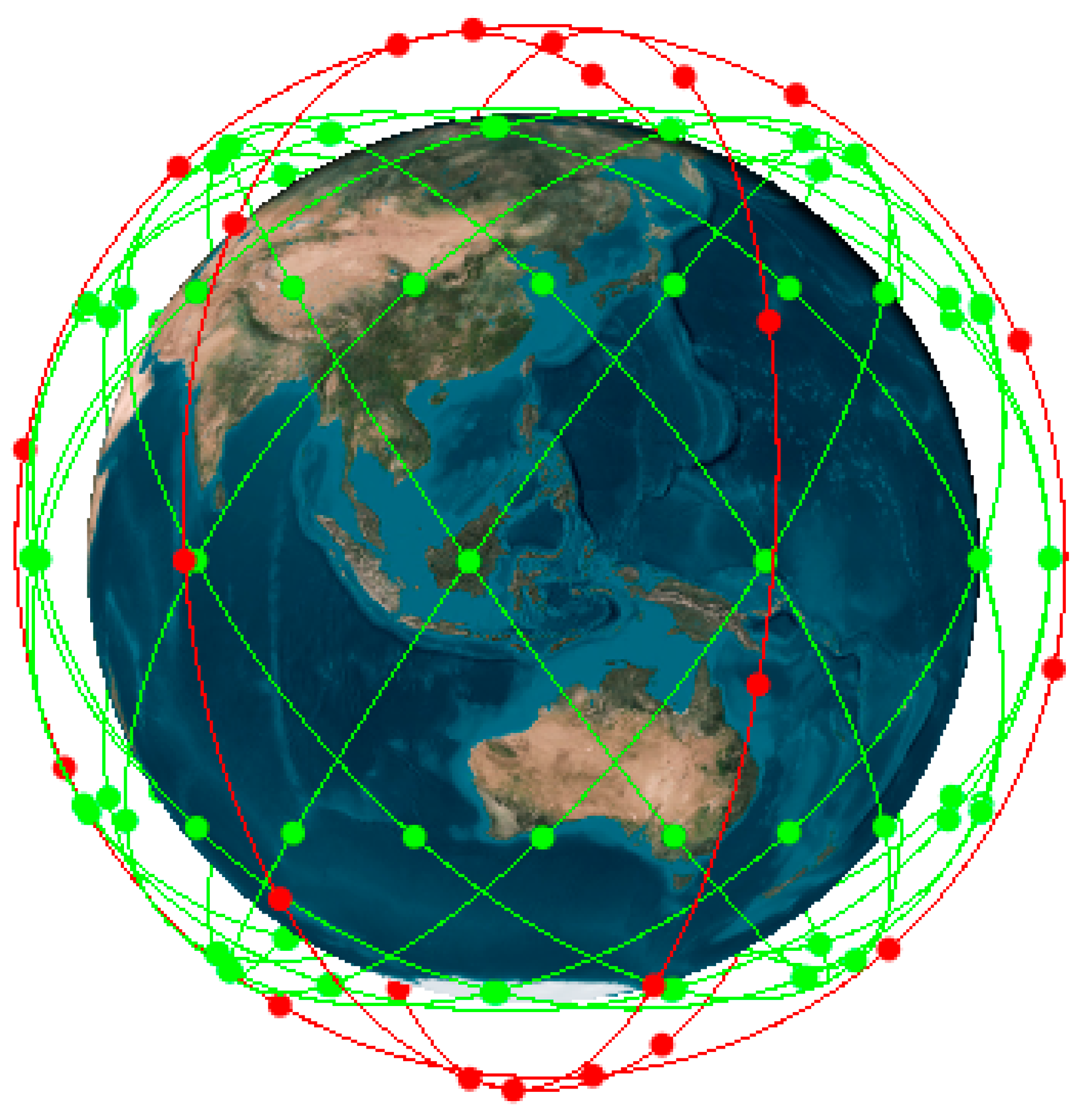
3. LEO Constellation and Data Simulation
4. Experimental Results and Analysis
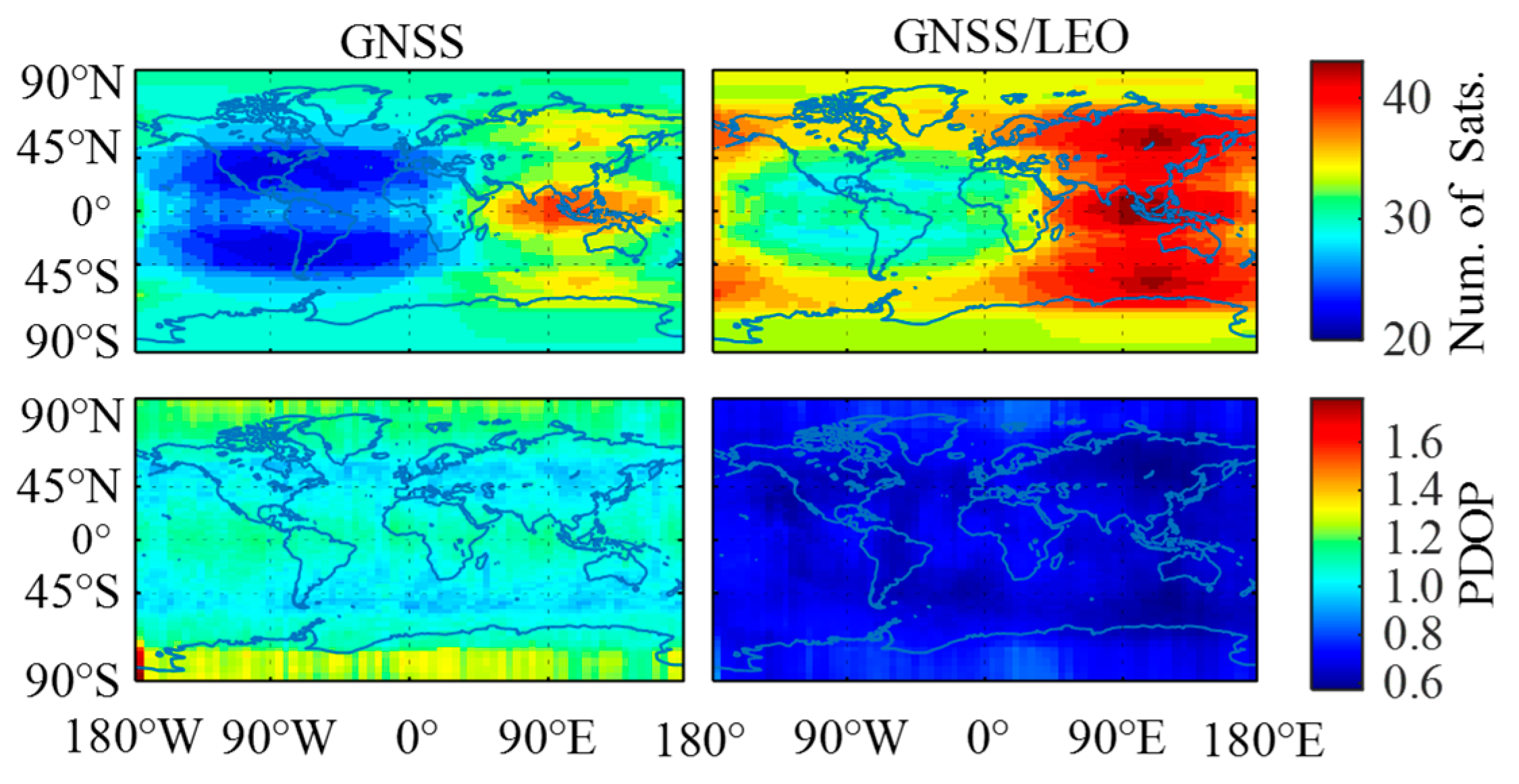
4.1. LEO-Augmented GNSS PPP
4.2. LEO/Atmosphere Double-Augmentation PPP
4.2.1. Data Description
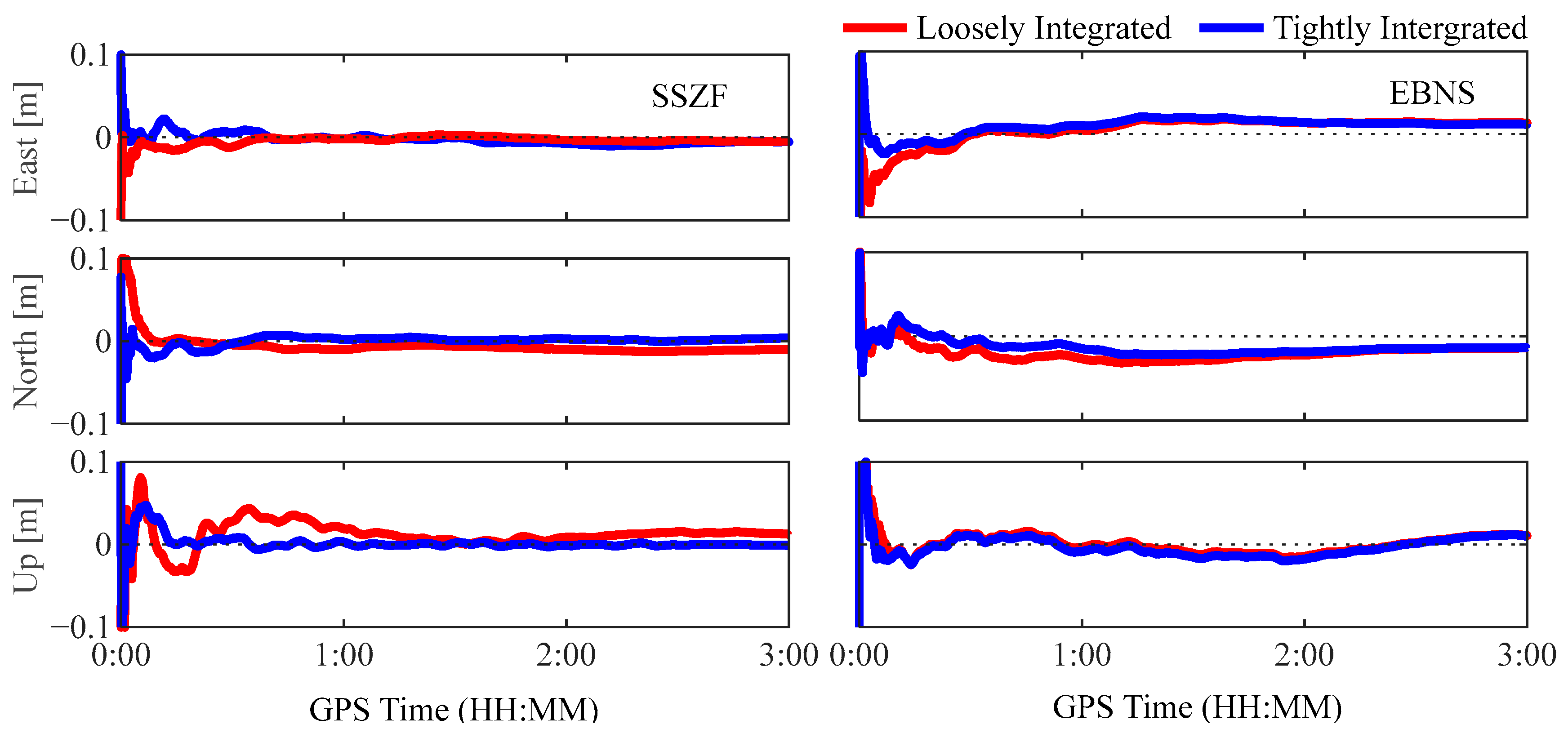
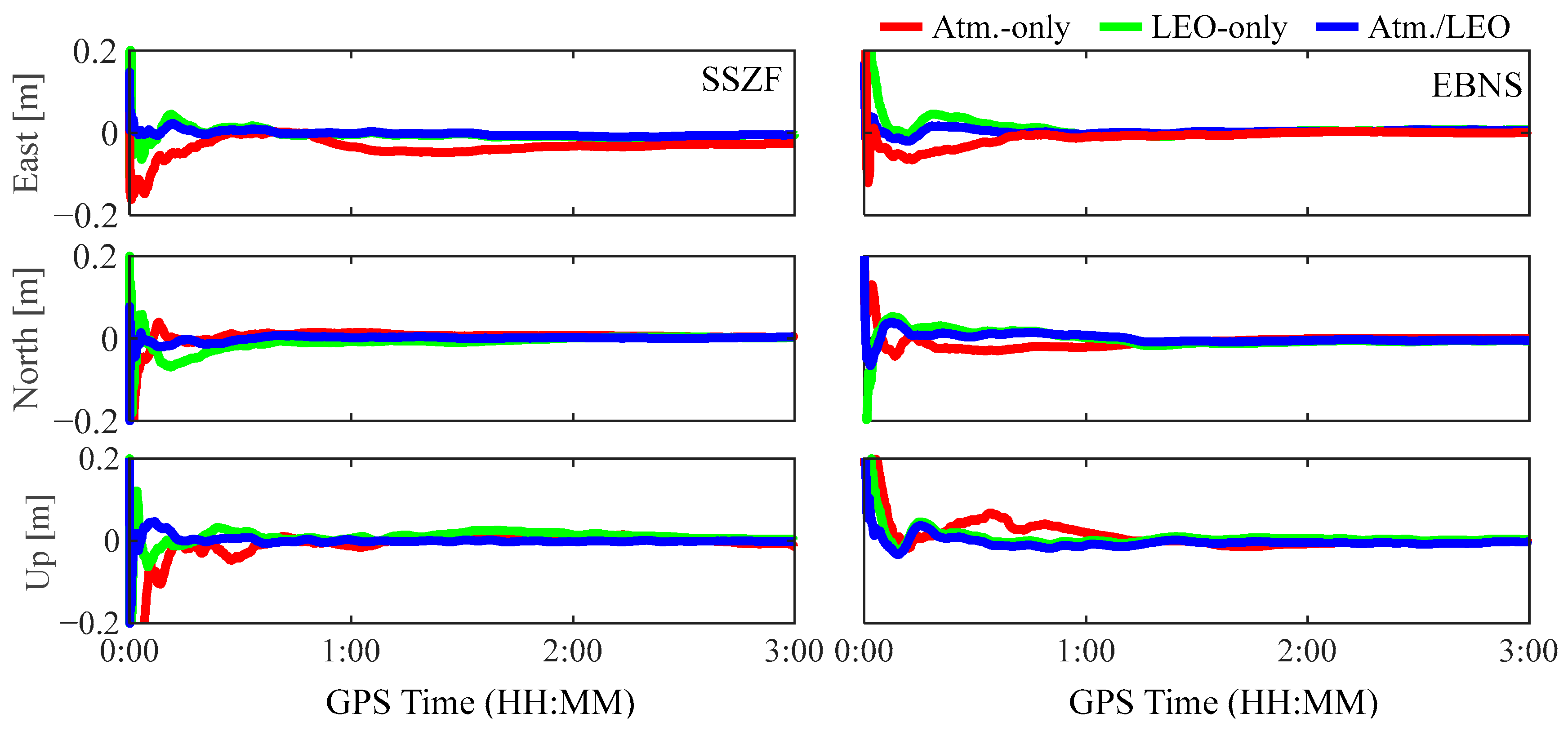
4.2.2. Results and Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zumberge, J.F.; Heflin, M.B.; Jefferson, D.C.; Watkins, M.M.; Webb, F.H. Precise point positioning for the efficient and robust analysis of GPS data from large networks. J. Geophys. Res. Solid Earth 1997, 102, 5005–5017. [Google Scholar] [CrossRef]
- Kouba, J.; Hérous, P. Precise point positioning using IGS orbit and clock products. GPS Solut. 2001, 5, 12–28. [Google Scholar] [CrossRef]
- Bahadur, B.; Nohutcu, M. Comparative analysis of MGEX products for post-processing multi-GNSS PPP. Measurement 2019, 145, 361–369. [Google Scholar] [CrossRef]
- Li, X.; Ma, F.; Li, X.; Lv, H.; Bian, L.; Jiang, Z.; Zhang, X. LEO constellation-augmented multi-GNSS for rapid PPP convergence. J. Geod. 2019, 93, 749–764. [Google Scholar] [CrossRef]
- Wu, G.; Chen, J.; Wu, X.; Hu, J. Modeling and assessment of regional atmospheric corrections based on undifferenced and uncombined PPP-RTK. Acta Geod. Cartogr. Sin. 2020, 49, 1407. [Google Scholar]
- Zhang, X.; Ma, F. Review of the development of LEO navigation-augmented GNSS. Acta Geod. Cartogr. Sin. 2019, 48, 1073. [Google Scholar]
- Zhao, Q.; Pan, S.; Gao, C.; Gao, W.; Xia, Y. BDS/GPS/LEO triple-frequency uncombined precise point positioning and its performance in harsh environments. Measurement 2020, 151, 107216. [Google Scholar] [CrossRef]
- Joerger, M.; Gratton, L.; Pervan, B.; Cohen, C.E. Analysis of Iridium-augmented GPS for floating carrier phase positioning. Navigation 2010, 57, 137–160. [Google Scholar] [CrossRef]
- Su, M.; Su, X.; Zhao, Q.; Liu, J. BeiDou augmented navigation from low earth orbit satellites. Sensors 2019, 19, 198. [Google Scholar] [CrossRef]
- Ke, M.; Lv, J.; Chang, J.; Dai, W.; Tong, K.; Zhu, M. Integrating GPS and LEO to accelerate convergence time of precise point positioning. In Proceedings of the 7th International Conference on Wireless Communications and Signal Processing (WCSP), Nanjing, China, 15–17 October 2015; IEEE: Piscataway, NJ, USA, 2015; pp. 1–5. [Google Scholar]
- Ge, H.; Li, B.; Ge, M.; Zang, N.; Nie, L.; Shen, Y.; Schuh, H. Initial assessment of precise point positioning with LEO enhanced global navigation satellite systems (LeGNSS). Remote Sens. 2018, 10, 984. [Google Scholar] [CrossRef]
- Li, B.; Ge, H.; Ge, M.; Nie, L.; Shen, Y.; Schuh, H. LEO enhanced Global Navigation Satellite System (LeGNSS) for real-time precise positioning services. Adv. Space Res. 2019, 63, 73–93. [Google Scholar] [CrossRef]
- Ge, H.; Li, B.; Nie, L.; Ge, M.; Schuh, H. LEO constellation optimization for LEO enhanced global navigation satellite system (LeGNSS). Adv. Space Res. 2020, 66, 520–532. [Google Scholar] [CrossRef]
- Ma, F.; Zhang, X.; Li, X.; Cheng, J.; Guo, F.; Hu, J.; Pan, L. Hybrid constellation design using a genetic algorithm for a LEO-based navigation augmentation system. GPS Solut. 2020, 24, 62. [Google Scholar] [CrossRef]
- Li, M.; Xu, T.; Guan, M.; Gao, F.; Jiang, N. LEO-constellation-augmented multi-GNSS real-time PPP for rapid re-convergence in harsh environments. GPS Solut. 2022, 26, 29. [Google Scholar]
- Wabbena, G.; Schmitz, M.; Bagge, A. PPP-RTK: Precise point positioning using state-space representation in RTK networks. In Proceedings of the 18th International Technical Meeting of the Satellite Division of the Institute of Navigation (ION GNSS 2005), Long Beach, CA, USA, 13–16 September 2005; pp. 2584–2594. [Google Scholar]
- Li, X.; Zhang, X.; Ge, M. Regional reference network augmented precise point positioning for instantaneous ambiguity resolution. J. Geod. 2011, 85, 151–158. [Google Scholar] [CrossRef]
- Nadarajah, N.; Khodabandeh, A.; Wang, K.; Choudhury, M.; Teunissen, P.J.G. Multi-GNSS PPP-RTK: From large-to small-scale networks. Sensors 2018, 18, 1078. [Google Scholar] [CrossRef] [PubMed]
- Hong, J.; Tu, R.; Zhang, P.; Zhang, R.; Han, J.; Fan, L.; Wang, S.; Lu, X. GNSS rapid precise point positioning enhanced by low Earth orbit satellites. Satell. Navig. 2023, 4, 11. [Google Scholar] [CrossRef]
- Hong, J.; Tu, R.; Zhang, S.; Li, F.; Liu, M.; Lu, X. Inter-Satellite Single-Difference Ionospheric Delay Interpolation Model for PPP-RTK and Its Positioning Performance Verification. Remote Sens. 2022, 14, 4153. [Google Scholar] [CrossRef]
- Li, P.; Cui, B.; Hu, J.; Liu, X.; Zhang, X.; Ge, M.; Schuh, H. PPP-RTK considering the ionosphere uncertainty with cross-validation. Satell. Navig. 2022, 3, 10. [Google Scholar] [CrossRef]
- Cai, C.; Gao, Y. Modeling and assessment of combined GPS/GLONASS precise point positioning. GPS Solut. 2013, 17, 223–236. [Google Scholar]
- Cai, C.; Gao, Y.; Pan, L.; Zhu, J. Precise point positioning with quad-constellations: GPS, BeiDou, GLONASS and Galileo. Adv. Space Res. 2015, 56, 133–143. [Google Scholar]
- Yang, L. The Centispace-1: A LEO Satellite-Based Augmentation System. In Proceedings of the 14th Meeting of the International Committee on Global Navigation Satellite Systems, Bengaluru, India, 8–13 December 2019. [Google Scholar]
- Saastamoinen, J. Atmospheric correction for the troposphere and stratosphere in radio ranging satellites. Use Artif. Satell. Geod. 1972, 15, 247–251. [Google Scholar]







| Items | Configuration |
|---|---|
| Type of observations | Code and carrier phase |
| Sample intervals | 1 s |
| Elevation mask | 7° |
| Signal frequency | GPS L1/L2 |
| Receiver clock offset | GNSS PPP clock offset estimates |
| Satellite clock offset | IGS clock file |
| Tropospheric delay | Saastamonion model for dry delay GNSS PPP estimates for wet delay |
| Ionospheric delay | GIM file |
| Tide | Erp file |
| Hardware delay | DCB file |
| Constellations | Conv. Time/min | East/m | North/m | Up/m |
|---|---|---|---|---|
| GPS | 27.5 | 0.031 | 0.016 | 0.037 |
| GPS/BDS | 20.5 | 0.027 | 0.015 | 0.035 |
| GPS/BDS/GAL | 16.5 | 0.029 | 0.015 | 0.034 |
| GPS/GLO/BDS/GAL | 16.2 | 0.025 | 0.013 | 0.031 |
| GPS/GLO/BDS/GAL/LEO | 3.5 | 0.015 | 0.009 | 0.018 |
| Area | Augmentation | Conv. Time/min | East/m | North/m | Up/m |
|---|---|---|---|---|---|
| Low latitude | Atmosphere-only | 5.1 | 0.019 | 0.011 | 0.025 |
| LEO-only | 3.2 | 0.009 | 0.007 | 0.014 | |
| Atmosphere/LEO | 0.8 | 0.007 | 0.006 | 0.013 | |
| Middle latitude | Atmosphere-only | 6.2 | 0.020 | 0.008 | 0.024 |
| LEO-only | 2.9 | 0.009 | 0.004 | 0.019 | |
| Atmosphere/LEO | 0.8 | 0.007 | 0.004 | 0.012 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, B.; Cai, C.; Pan, L. A Rapid-Convergence Precise Point Positioning Approach Using Double Augmentations of Low Earth Orbit Satellite and Atmospheric Information. Remote Sens. 2023, 15, 5265. https://doi.org/10.3390/rs15225265
He B, Cai C, Pan L. A Rapid-Convergence Precise Point Positioning Approach Using Double Augmentations of Low Earth Orbit Satellite and Atmospheric Information. Remote Sensing. 2023; 15(22):5265. https://doi.org/10.3390/rs15225265
Chicago/Turabian StyleHe, Bei, Changsheng Cai, and Lin Pan. 2023. "A Rapid-Convergence Precise Point Positioning Approach Using Double Augmentations of Low Earth Orbit Satellite and Atmospheric Information" Remote Sensing 15, no. 22: 5265. https://doi.org/10.3390/rs15225265
APA StyleHe, B., Cai, C., & Pan, L. (2023). A Rapid-Convergence Precise Point Positioning Approach Using Double Augmentations of Low Earth Orbit Satellite and Atmospheric Information. Remote Sensing, 15(22), 5265. https://doi.org/10.3390/rs15225265








