Corrections of Mesoscale Eddies and Kuroshio Extension Surface Velocities Derived from Satellite Altimeters
Abstract
1. Introduction
2. Data and Methods
2.1. Satellite Altimeter Data
2.2. Methods
2.2.1. Skewness
2.2.2. Iterative Algorithm
- Determine the initial value;
- Derive the iteration equation;
- Determine the conditions for the termination of the iterative sequence.
2.2.3. Eddy Automated Detection Algorithm
- In the east–west direction along the eddy center, the v’ component of velocity has opposite signs on both sides of the eddy center, which gradually increases away from the center point;
- In the north–south direction along the eddy center, the u’ component of velocity has opposite signs on both sides of the eddy center, which gradually increases away from the center point;
- In the local region of the eddy center, the velocity is the minimum;
- Around the eddy center, the rotation of the eddy velocity vector is consistent. The direction of two neighboring velocity vectors must be in the same or two neighboring quadrants.
2.2.4. Rossby Number
2.2.5. Eddy Kinetic Energy
3. Results
3.1. Cyclogeostrophic Corrected Surface Velocities of Eddy
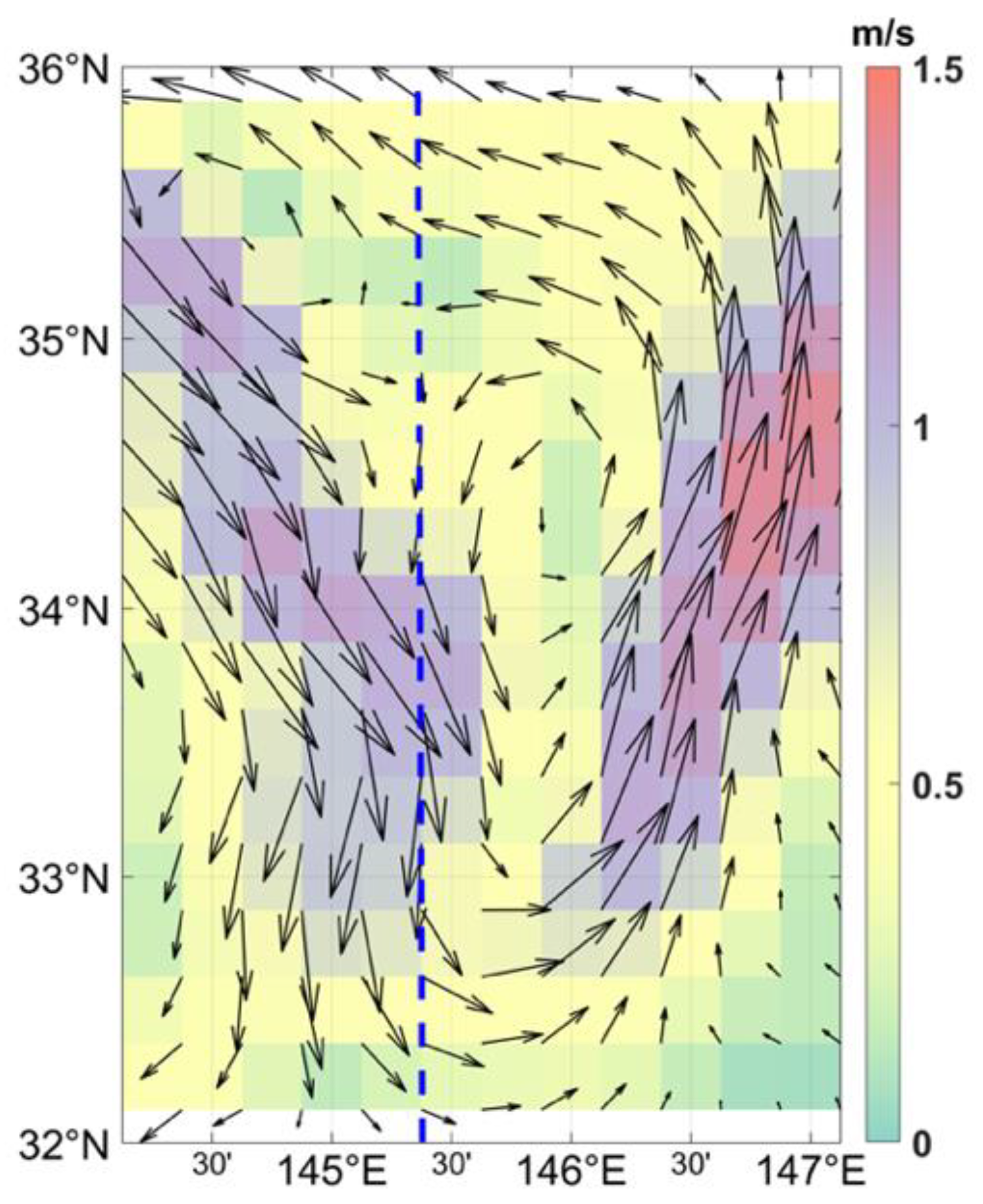
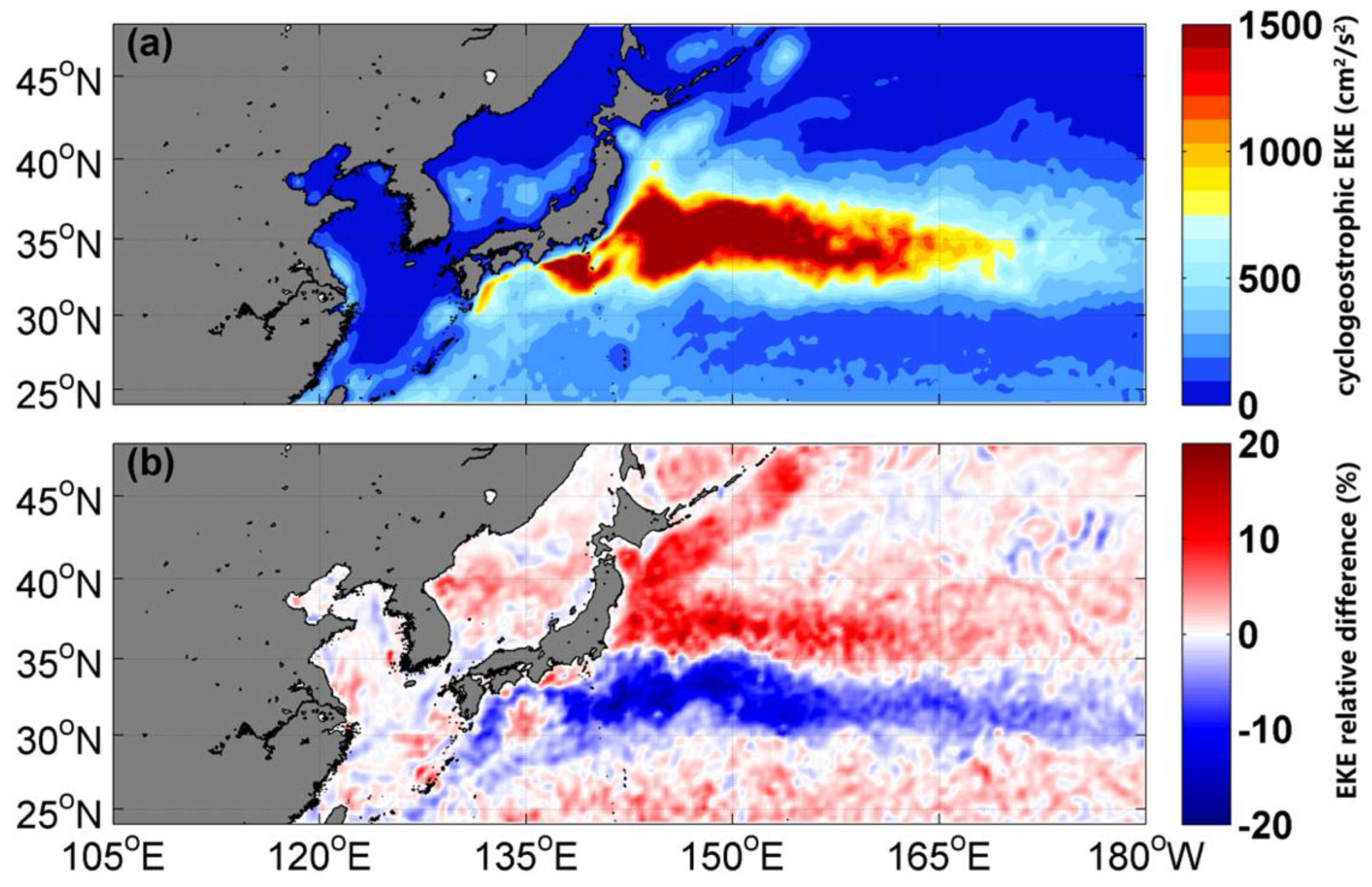
3.2. Cyclogeostrophic Corrected Surface Velocities of Kuroshio Extension
4. Discussion
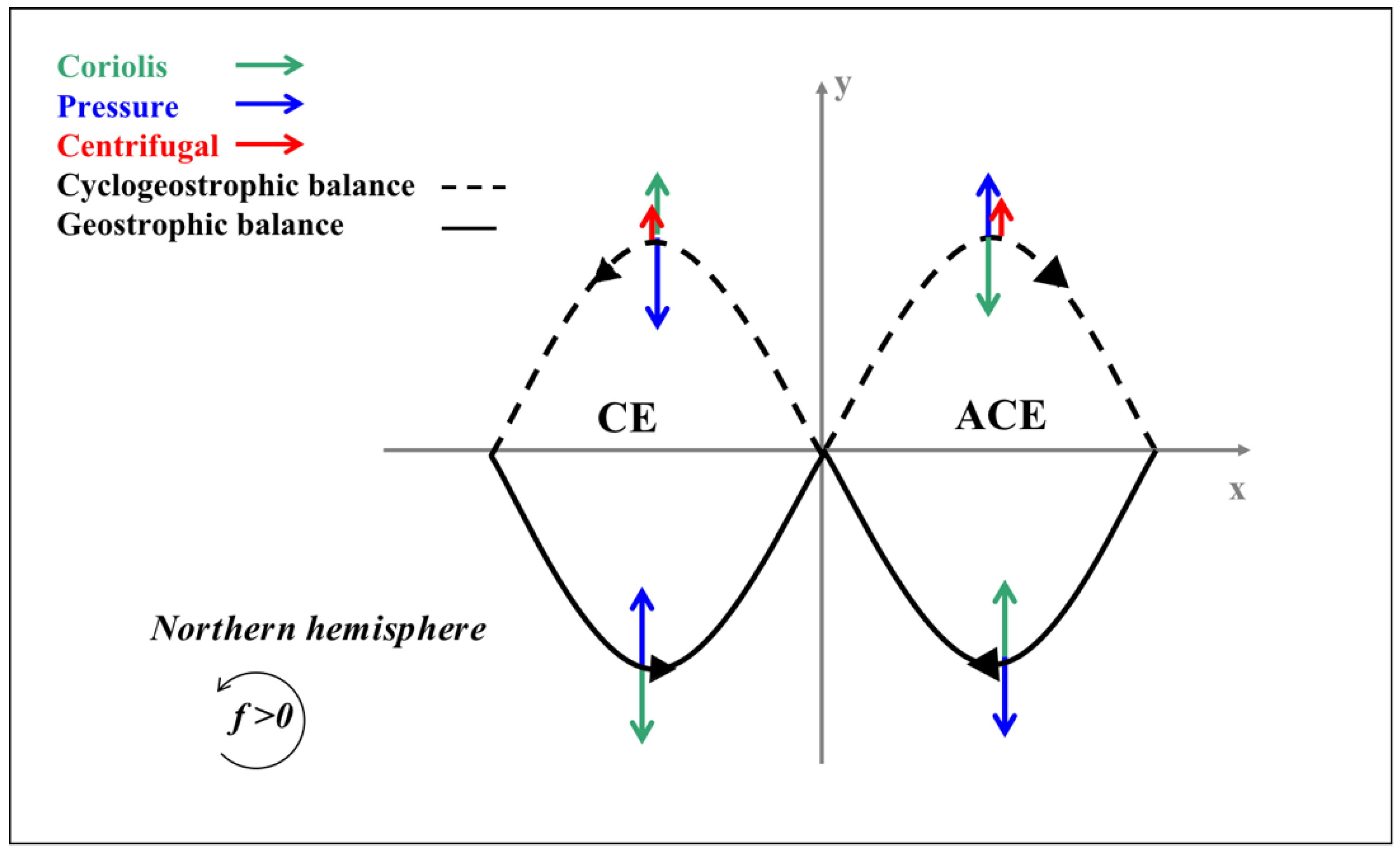
4.1. Interpretation of the Cyclogeostrophic Rossby Number
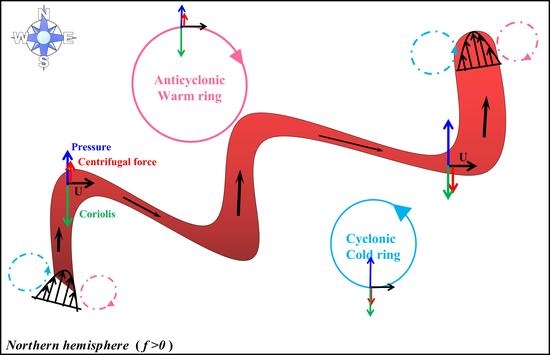
4.2. Vorticity Adjustment of Meander
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dohan, K.; Maximenko, N. Monitoring Ocean Currents with Satellite Sensors. Oceanography 2010, 23, 94–103. [Google Scholar] [CrossRef]
- Chelton, D.B.; Schlax, M.G.; Samelson, R.M.; De Szoeke, R.A. Global observations of large oceanic eddies. Geophys. Res. Lett. 2007, 34, L15606. [Google Scholar] [CrossRef]
- Ji, J.; Dong, C.; Zhang, B.; Liu, Y. An oceanic eddy statistical comparison using multiple observational data in the Kuroshio Extension region. Acta Oceanol. Sin. 2017, 36, 1–7. [Google Scholar] [CrossRef]
- Mendoza, C.; Mancho, A.M.; Rio, M.H. The turnstile mechanism across the Kuroshio current: Analysis of dynamics in altimeter velocity fields. Nonlinear Process. Geophys. 2010, 17, 103–111. [Google Scholar] [CrossRef]
- Leterme, S.C.; Pingree, R.D. The Gulf Stream, rings and North Atlantic eddy structures from remote sensing (Altimeter and SeaWiFS). J. Mar. Syst. 2008, 69, 177–190. [Google Scholar] [CrossRef]
- Ichikawa, K.; Imawaki, S. Fluctuation of the sea surface dynamic topography southeast of Japan as estimated from Seasat altimetry data. J. Oceanogr. 1992, 48, 155–177. [Google Scholar] [CrossRef]
- Woo, H.-J.; Park, K.-A. Estimation of Extreme Significant Wave Height in the Northwest Pacific Using Satellite Altimeter Data Focused on Typhoons (1992–2016). Remote. Sens. 2021, 13, 1063. [Google Scholar] [CrossRef]
- Cao, Y.; Dong, C.; Young, I.R.; Yang, J. Global Wave Height Slowdown Trend during a Recent Global Warming Slowdown. Remote Sens. 2021, 13, 4096. [Google Scholar] [CrossRef]
- Hamlington, B.D.; Leben, R.R.; Kim, K.Y.; Nerem, R.S.; Atkinson, L.P.; Thompson, P.R. The effect of the El Niño-Southern Oscillation on US regional and coastal sea level. J. Geophys. Res. Ocean. 2015, 120, 3970–3986. [Google Scholar] [CrossRef]
- Lukas, R.; Firing, E. The geostrophic balance of the Pacific Equatorial Undercurrent. Deep Sea Research Part A. Oceanogr. Res. Pap. 1984, 31, 61–66. [Google Scholar]
- Cushman-Roisin, B. Frontal geostrophic dynamics. J. Phys. Oceanogr. 1986, 16, 132–143. [Google Scholar] [CrossRef]
- Rikiishi, K.; Sasaki, K. Geostrophic balance of the Kuroshio as inferred from surface current and sea level observations. J. Oceanogr. Soc. Jpn. 1988, 44, 305–314. [Google Scholar] [CrossRef]
- Strub, P.T.; James, C. Altimeter-derived variability of surface velocities in the California Current System: 2. Seasonal circulation and eddy statistics. Deep. Sea Res. Part II Top. Stud. Oceanogr. 2000, 47, 831–870. [Google Scholar] [CrossRef]
- Shankar, D.; Vinayachandran, P.N.; Unnikrishnan A., S. The monsoon currents in the north Indian Ocean. Prog. Oceanogr. 2002, 52, 63–120. [Google Scholar] [CrossRef]
- Armitage, T.W.K.; Bacon, S.; Ridout, A.L.; Petty, A.A.; Wolbach, S.; Tsamados, M. Arctic Ocean surface geostrophic circulation 2003–2014. Cryosphere 2017, 11, 1767–1780. [Google Scholar] [CrossRef]
- Uchida, H.; Imawaki, S.; Hu, J.H. Comparison of Kuroshio surface velocities derived from satellite altimeter and drifting buoy data. J. Oceanogr. 1998, 54, 115–122. [Google Scholar] [CrossRef]
- Fratantoni, D.M. North Atlantic surface circulation during the 1990's observed with satellite-tracked drifters. J. Geophys. Res. Ocean. 2001, 106, 22067–22093. [Google Scholar] [CrossRef]
- Uchida, H.; Imawaki, S. Eulerian mean surface velocity field derived by combining drifter and satellite altimeter data. Geophys. Res. Lett. 2003, 30, 1229. [Google Scholar] [CrossRef]
- Penven, P.; Halo, I.; Pous, S.; Marié, L. Cyclogeostrophic balance in the Mozambique Channel. J. Geophys. Res. Ocean. 2014, 119, 1054–1067. [Google Scholar] [CrossRef]
- Douglass, E.M.; Richman, J.G. Analysis of ageostrophy in strong surface eddies in the Atlantic Ocean. J. Geophys. Res. Ocean. 2015, 120, 1490–1507. [Google Scholar] [CrossRef]
- Shakespeare, C.J. Curved density fronts: Cyclogeostrophic adjustment and frontogenesis. J. Phys. Oceanogr. 2016, 46, 3193–3207. [Google Scholar] [CrossRef]
- Ioannou, A.; Stegner, A.; Tuel, A.; LeVu, B.; Dumas, F.; Speich, S. Cyclostrophic Corrections of AVISO/DUACS Surface Velocities and Its Application to Mesoscale Eddies in the Mediterranean Sea. J. Geophys. Res. Ocean. 2019, 124, 8913–8932. [Google Scholar] [CrossRef]
- Taburet, G.; Sanchez-Roman, A.; Ballarotta, M.; Pujol, M.-I.; Legeais, J.-F.; Fournier, F.; Faugere, Y.; Dibarboure, G. DUACS DT2018: 25 years of reprocessed sea level altimetry products. Ocean Sci. 2019, 15, 1207–1224. [Google Scholar] [CrossRef]
- Von Hippel, P.T. Mean, median, and skew: Correcting a textbook rule. J. Stat. Educ. 2005, 13. [Google Scholar] [CrossRef]
- Ames, W.F. Numerical Methods for Partial Differential Equations; Academic Press: Cambridge, MA, USA, 2014. [Google Scholar]
- Dubey, V.P.; Kumar, R.; Kumar, D. A reliable treatment of residual power series method for time-fractional Black–Scholes European option pricing equations. Phys. A Stat. Mech. Its Appl. 2019, 533, 122040. [Google Scholar] [CrossRef]
- Huang, X.; Zhu, Y.; Vafaei, P.; Moradi, Z.; Davoudi, M. An iterative simulation algorithm for large oscillation of the applicable 2D-electrical system on a complex nonlinear substrate. Eng. Comput. 2021, 38, 3137–3149. [Google Scholar] [CrossRef]
- Bank, R.E.; Rose, D.J. Global approximate Newton methods. Numer. Math. 1981, 37, 279–295. [Google Scholar] [CrossRef]
- Tanakan, S. A new algorithm of modified bisection method for nonlinear equation. Appl. Math. Sci. 2013, 7, 6107–6114. [Google Scholar] [CrossRef]
- Nencioli, F.; Dong, C.; Dickey, T.; Washburn, L.; McWilliams, J.C. A Vector Geometry–Based Eddy Detection Algorithm and Its Application to a High-Resolution Numerical Model Product and High-Frequency Radar Surface Velocities in the Southern California Bight. J. Atmos. Ocean. Technol. 2010, 27, 564–579. [Google Scholar] [CrossRef]
- Dong, C.; Liu, L.; Nencioli, F.; Bethel, B.J.; Liu, Y.; Xu, G.; Ma, J.; Ji, J.; Sun, W.; Shan, H.; et al. The near-global ocean mesoscale eddy atmospheric-oceanic-biological interaction observational dataset. Sci. Data 2022, 9, 1–13. [Google Scholar] [CrossRef]
- Liu, Y.; Dong, C.; Guan, Y.; Chen, D.; McWilliams, J.; Nencioli, F. Eddy analysis in the subtropical zonal band of the North Pacific Ocean. Deep. Sea Res. Part I Oceanogr. Res. Pap. 2012, 68, 54–67. [Google Scholar] [CrossRef]
- Ji, J.; Dong, C.; Zhang, B.; Liu, Y.; Zou, B.; King, G.P.; Xu, G.; Chen, D. Oceanic Eddy Characteristics and Generation Mechanisms in the Kuroshio Extension Region. J. Geophys. Res. Ocean. 2018, 123, 8548–8567. [Google Scholar] [CrossRef]
- Yang, X.; Xu, G.; Liu, Y.; Sun, W.; Xia, C.; Dong, C. Multi-Source Data Analysis of Mesoscale Eddies and Their Effects on Surface Chlorophyll in the Bay of Bengal. Remote Sens. 2020, 12, 3485. [Google Scholar] [CrossRef]
- Maximenko, N.; Niiler, P. Mean surface circulation of the global ocean inferred from satellite altimeter and drifter data. In Proceedings of the Symposium on 15 Years of Progress in Radar Altimetry, Venice, Italy, 13–18 March 2006; European Space Agency Special Publication ESA SP. p. 614. [Google Scholar]
- Qiu, B. Kuroshio and Oyashio currents. In Ocean Currents: A Derivative of the Encyclopedia of Ocean Sciences; Elsevier: New York, NY, USA, 2001; pp. 61–72. [Google Scholar]
- Niiler, P.P.; Maximenko, N.A.; Panteleev, G.G.; Yamagata, T.; Olson, D.B. Near-surface dynamical structure of the Kuroshio Extension. J. Geophys. Res. Ocean. 2003, 108, 3193. [Google Scholar] [CrossRef]
- Ma, C.; Wu, D.; Lin, X. Variability of surface velocity in the Kuroshio Current and adjacent waters derived from Argos drifter buoys and satellite altimeter data. Chin. J. Oceanol. Limnol. 2009, 27, 208–217. [Google Scholar] [CrossRef]
- Tai, C.K.; White, W.B. Eddy variability in the Kuroshio Extension as revealed by Geosat altimetry: Energy propagation away from the jet, Reynolds stress, and seasonal cycle. J. Phys. Oceanogr. 1990, 20, 1761–1777. [Google Scholar] [CrossRef]
- Zhang, D.; Lee, T.N.; Johns, W.E.; Liu, C.-T.; Zantopp, R. The Kuroshio east of Taiwan: Modes of variability and relationship to interior ocean mesoscale eddies. J. Phys. Oceanogr. 2001, 31, 1054–1074. [Google Scholar] [CrossRef]
- Liu, X.; Wang, Q.; Mu, M. Identifying the sensitive areas in targeted observation for predicting the Kuroshio large meander path in a regional ocean model. Acta Oceanol. Sin. 2022, 41, 3–14. [Google Scholar] [CrossRef]
- Sun, W.; An, M.; Liu, J.; Liu, J.; Yang, J.; Tan, W.; Dong, C.; Liu, Y. Comparative analysis of four types of mesoscale eddies in the Kuroshio-Oyashio extension region. Front. Mar. Sci. 2022, 9. [Google Scholar] [CrossRef]
- Cushman-Roisin, B.; Beckers, J.M. Introduction to Geophysical Fluid Dynamics: Physical and Numerical Aspects; Academic Press: Cambridge, MA, USA, 2011. [Google Scholar]



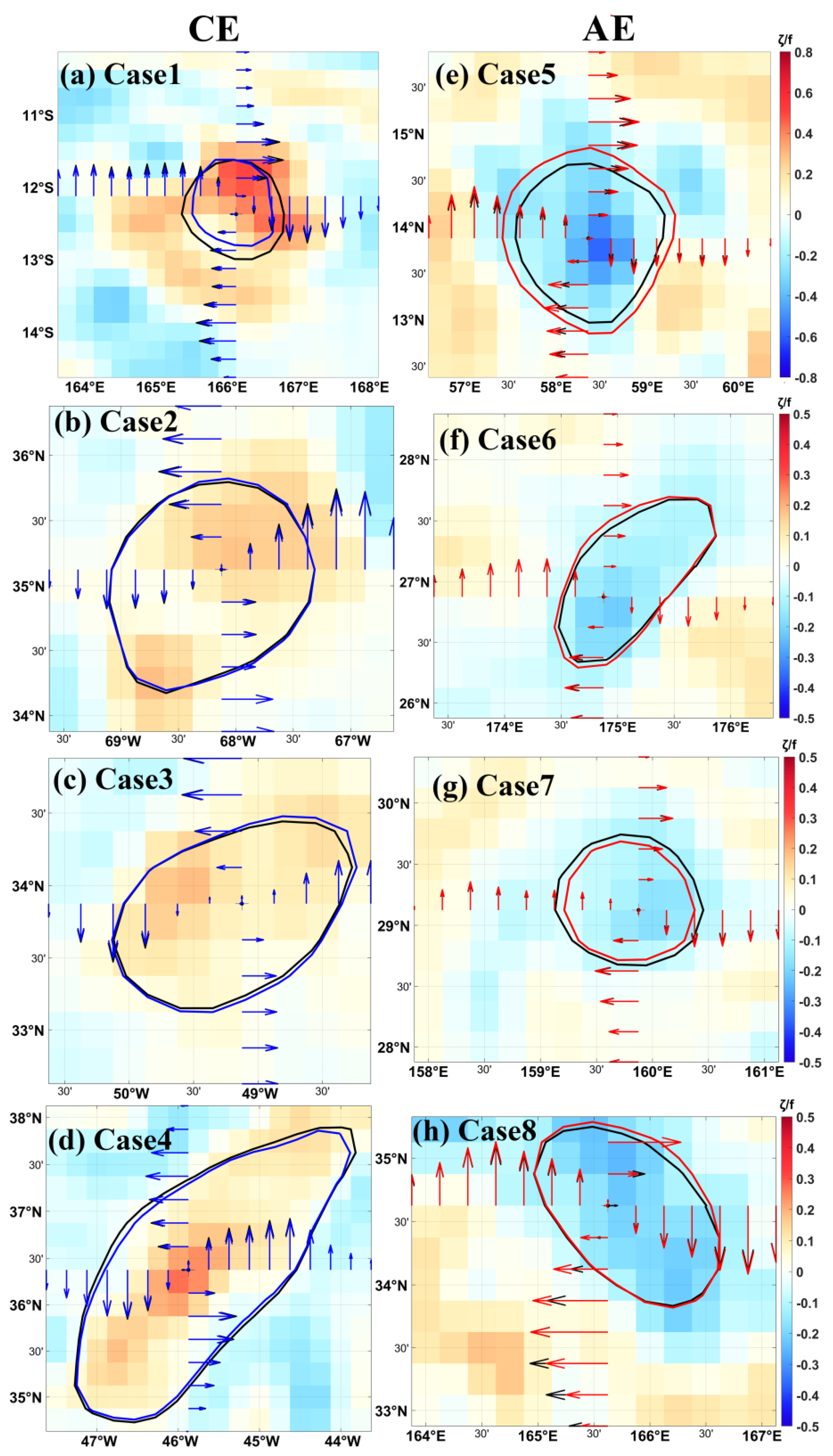


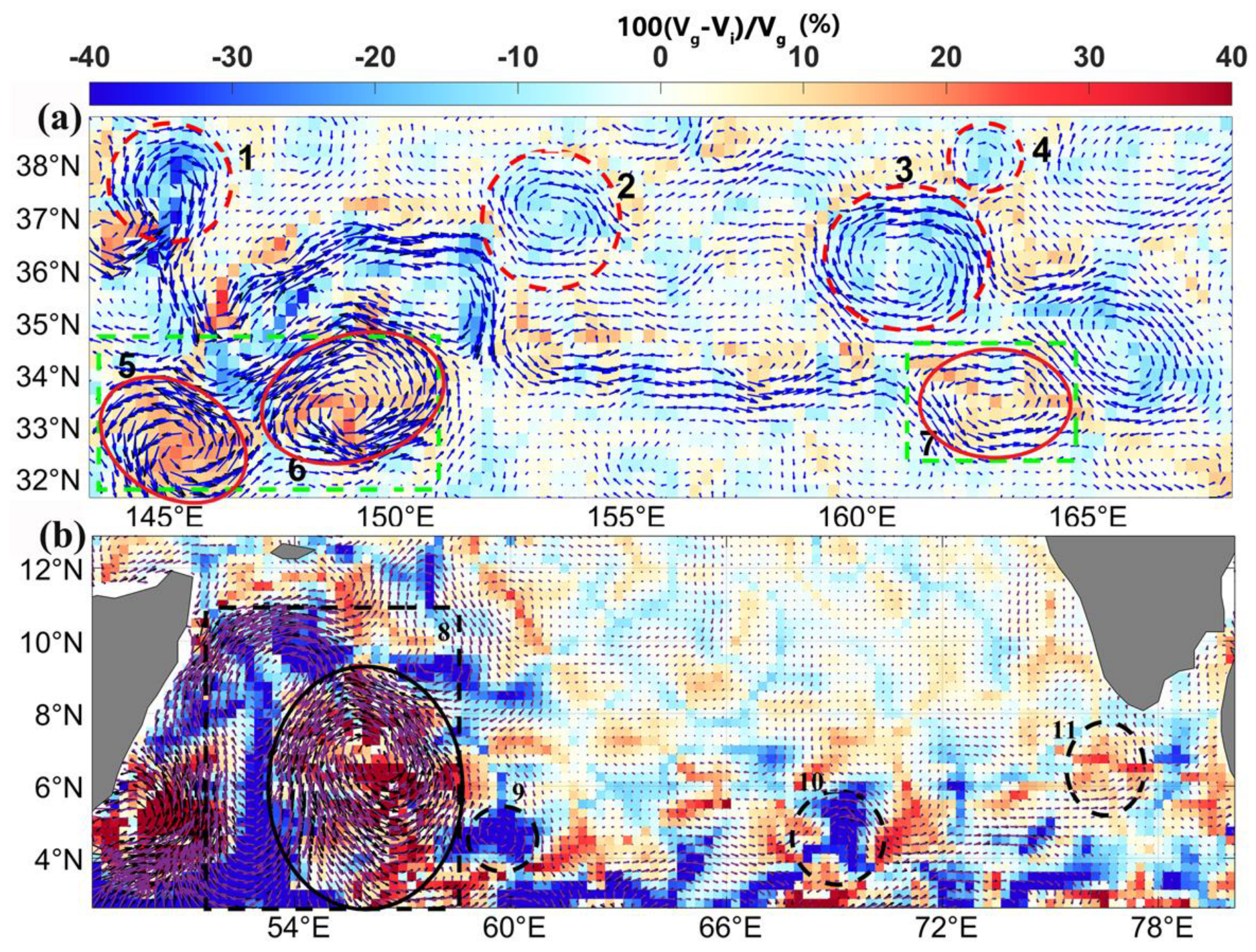

| Case | Type | Vgmax (m/s) | Vimax (m/s) | Lat (°N) | Rog | Roi | av (%) |
|---|---|---|---|---|---|---|---|
| Case 1 | CE 1 | 1.36 | 1.11 | 30–31 | 0.30 | 0.25 | −14.34 |
| Case 2 | CE | 1.13 | 1.08 | 32–34 | 0.08 | 0.07 | −7.90 |
| Case 3 | CE | 1.24 | 1.20 | 35–36 | 0.23 | 0.22 | −11.95 |
| Case 4 | CE | 1.17 | 1.06 | 34–35 | 0.18 | 0.17 | −10.31 |
| Case 5 | CE | 1.33 | 1.19 | 36–37 | 0.21 | 0.19 | −11.09 |
| Case 6 | ACE 2 | 1.05 | 1.14 | 36–38 | 0.12 | 0.13 | 8.69 |
| Case 7 | ACE | 1.25 | 1.36 | 39–41 | 0.07 | 0.08 | 8.81 |
| Case 8 | ACE | 0.85 | 0.86 | 40–42 | 0.08 | 0.09 | 8.92 |
| Case 9 | ACE | 0.14 | 0.16 | 7–8 | 0.11 | 0.12 | 8.94 |
| Case 10 | ACE | 0.41 | 0.52 | 8–9 | 0.23 | 0.29 | 17.22 |
| No. | Vimax (m/s) | f (s−1) | Radius (km) |
|---|---|---|---|
| 1 | 1.2 | 8.9 × 10−5 | 110 |
| 2 | 0.7 | 8.7 × 10−5 | 125 |
| 3 | 1.0 | 8.6 × 10−5 | 150 |
| 4 | 0.4 | 9.0 × 10−5 | 50 |
| 5 | 1.4 | 7.9 × 10−5 | 100 |
| 6 | 1.4 | 8.0 × 10−5 | 150 |
| 7 | 0.8 | 8.0 × 10−5 | 125 |
| 8 | 2.0 | 1.8 × 10−5 | 300 |
| 9 | 0.6 | 1.2 × 10−5 | 50 |
| 10 | 0.8 | 1.2 × 10−5 | 75 |
| 11 | 0.4 | 1.6 × 10−5 | 75 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, Y.; Dong, C.; Qiu, Z.; Bethel, B.J.; Shi, H.; Lü, H.; Cheng, Y. Corrections of Mesoscale Eddies and Kuroshio Extension Surface Velocities Derived from Satellite Altimeters. Remote Sens. 2023, 15, 184. https://doi.org/10.3390/rs15010184
Cao Y, Dong C, Qiu Z, Bethel BJ, Shi H, Lü H, Cheng Y. Corrections of Mesoscale Eddies and Kuroshio Extension Surface Velocities Derived from Satellite Altimeters. Remote Sensing. 2023; 15(1):184. https://doi.org/10.3390/rs15010184
Chicago/Turabian StyleCao, Yuhan, Changming Dong, Zehao Qiu, Brandon J. Bethel, Haiyun Shi, Haibin Lü, and Yinhe Cheng. 2023. "Corrections of Mesoscale Eddies and Kuroshio Extension Surface Velocities Derived from Satellite Altimeters" Remote Sensing 15, no. 1: 184. https://doi.org/10.3390/rs15010184
APA StyleCao, Y., Dong, C., Qiu, Z., Bethel, B. J., Shi, H., Lü, H., & Cheng, Y. (2023). Corrections of Mesoscale Eddies and Kuroshio Extension Surface Velocities Derived from Satellite Altimeters. Remote Sensing, 15(1), 184. https://doi.org/10.3390/rs15010184