Impact of Lock-In Time Constant on Remote Monitoring of Trace Gas in the Atmospheric Column Using Laser Heterodyne Radiometer (LHR)
Abstract
:1. Introduction
2. Materials and Methods
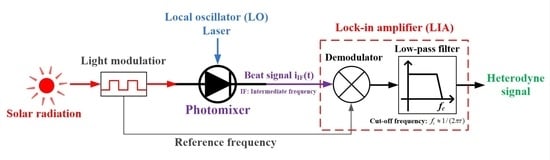
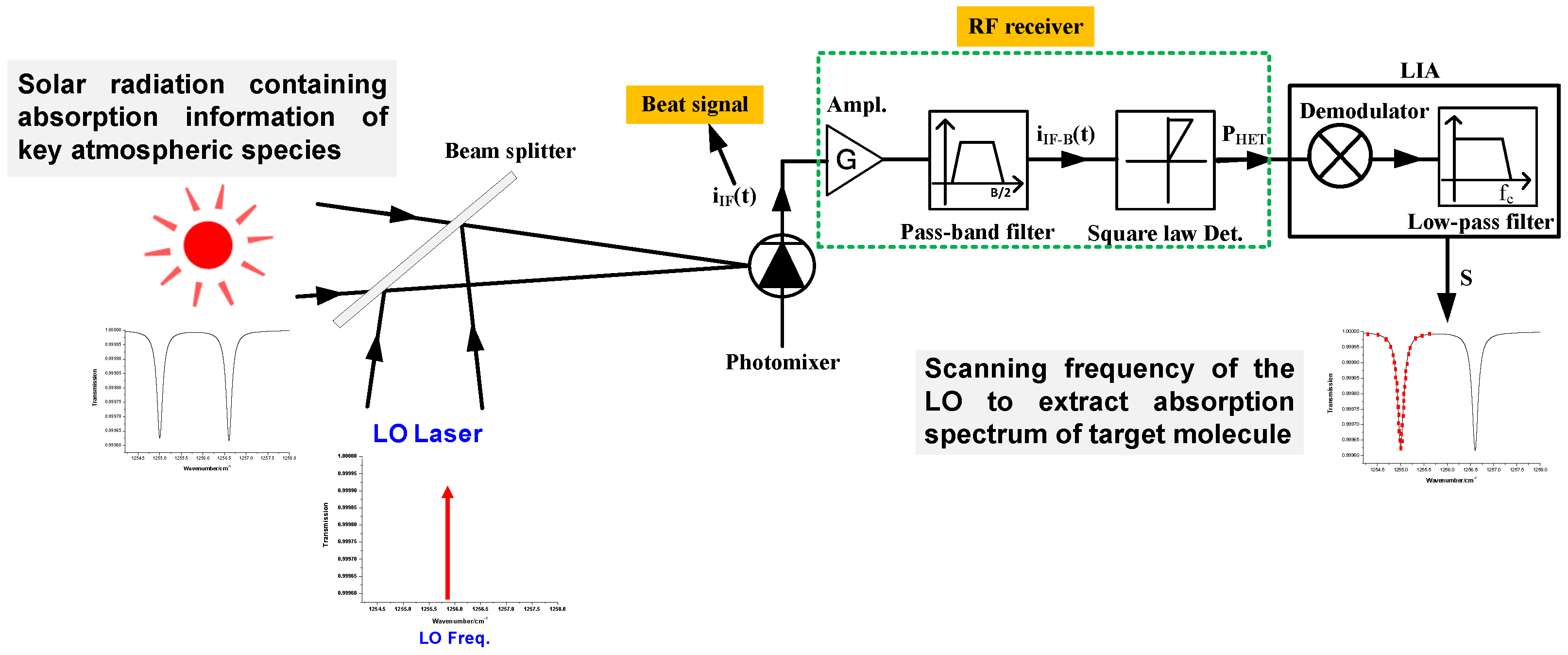
2.1. Principle of Laser Heterodyne Radiometer
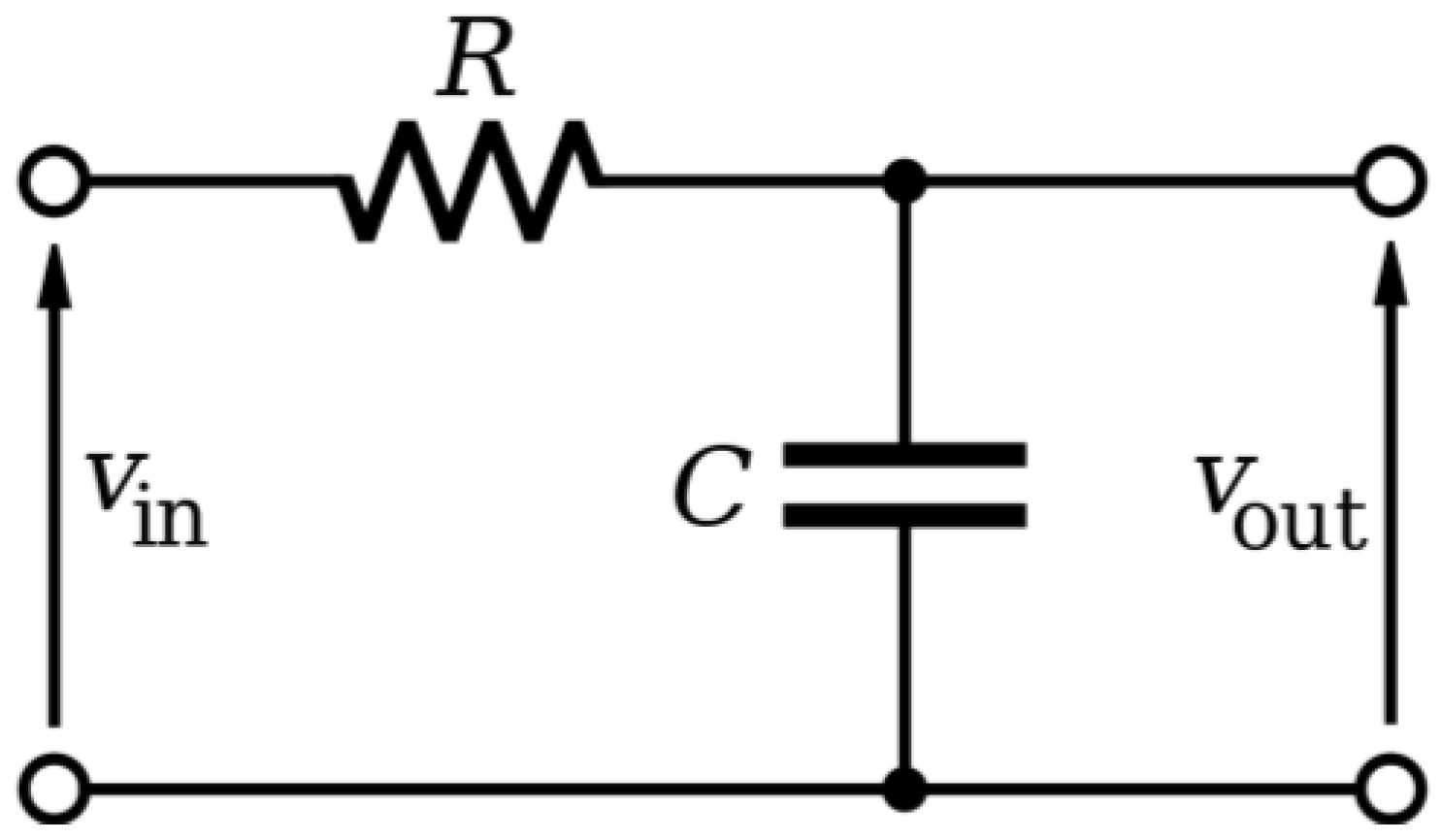
2.2. Analytical Model
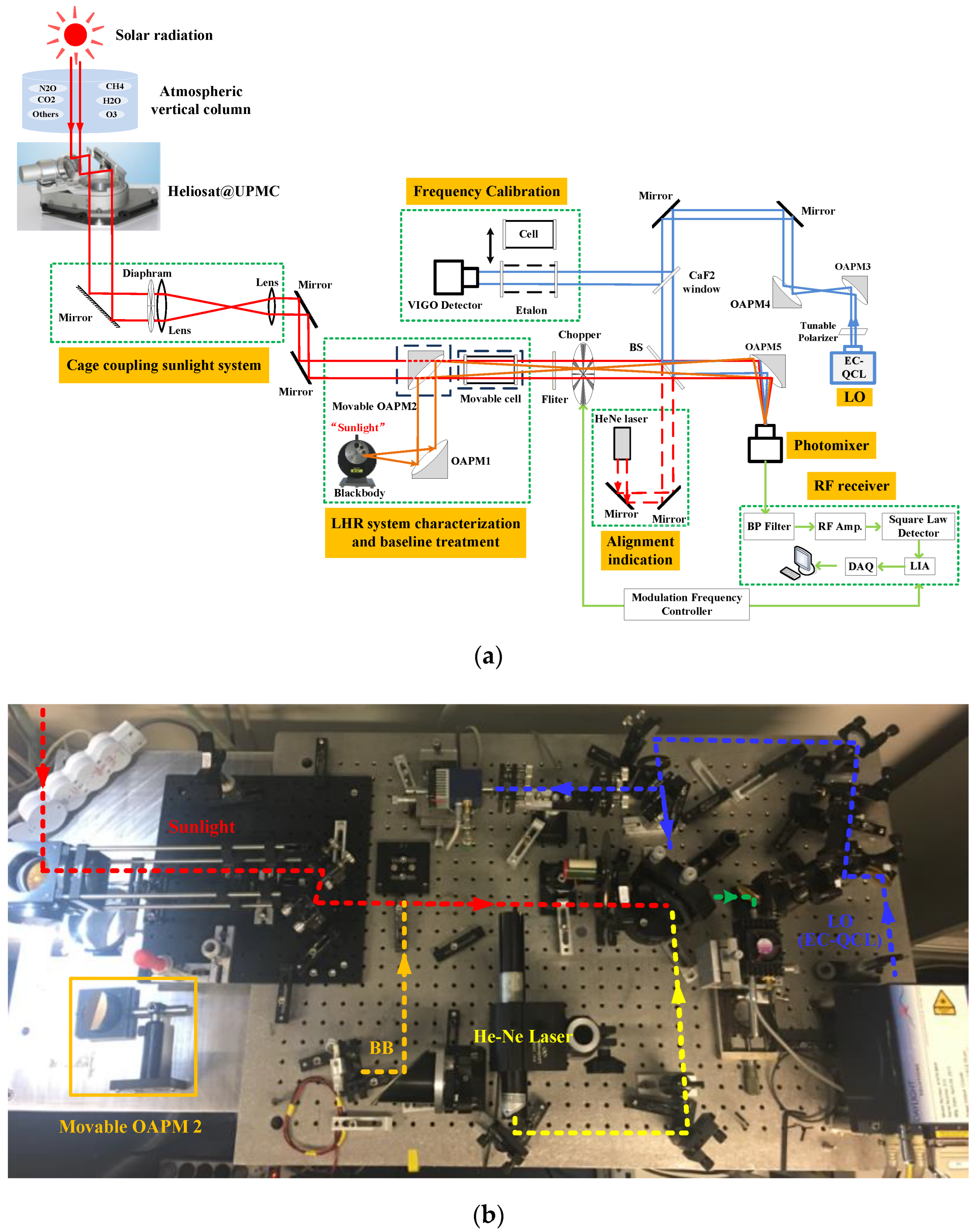
2.3. Experimental System Description
3. Results
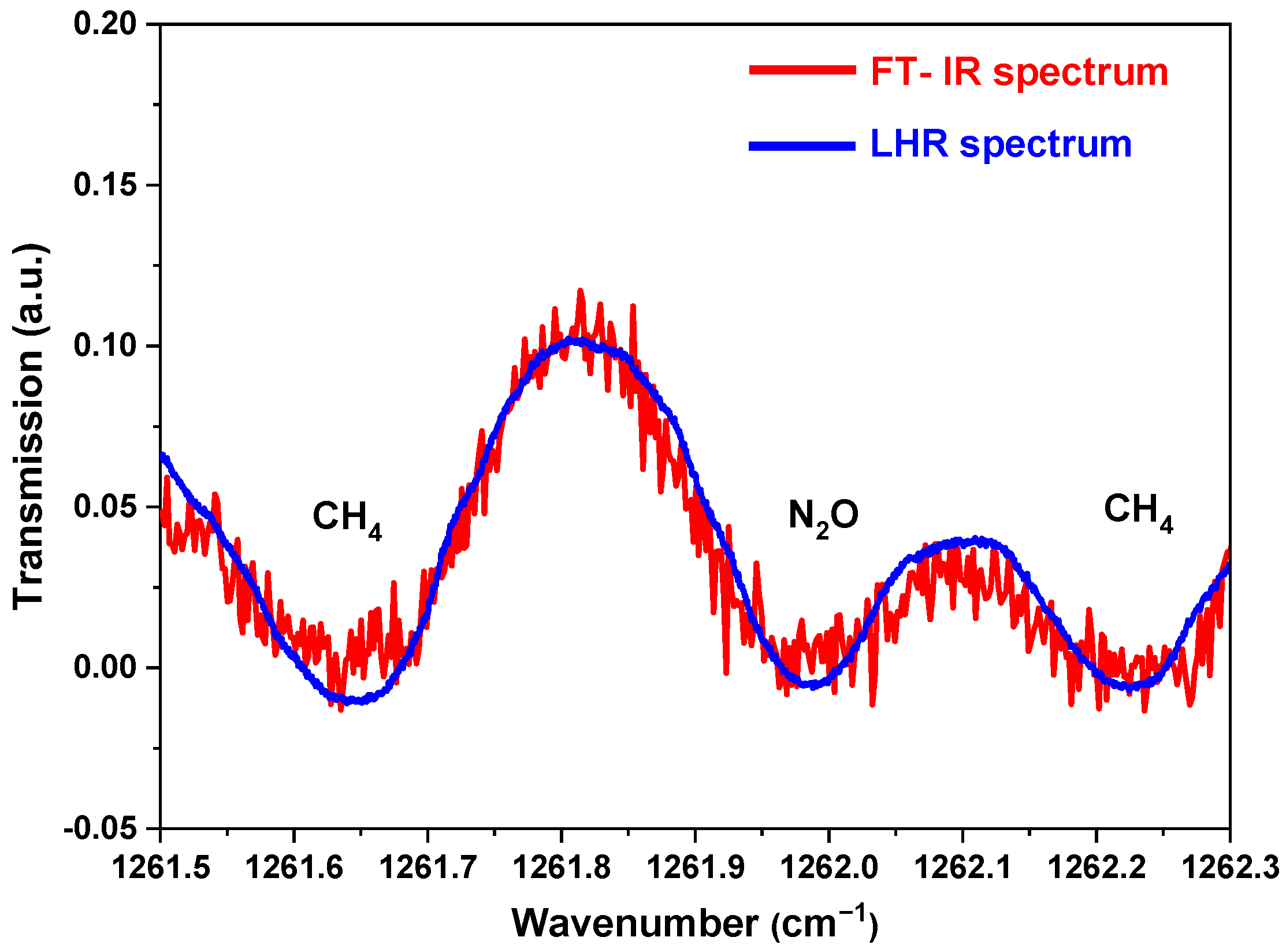
3.1. Experimental Results
3.2. Field Measurement
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lu, X.; Huang, Y.; Wu, P.; Liu, D.; Ma, H.; Wang, G.; Cao, Z. Distributed Feedback Interband Cascade Laser Based Laser Heterodyne Radiometer for Column Density of HDO and CH4 Measurements at Dunhuang, Northwest of China. Remote Sens. 2022, 14, 1489. [Google Scholar] [CrossRef]
- Shen, F.J.; Wang, G.X.; Wang, J.J.; Tan, T.; Wang, G.S.; Jeseck, P.; Té, Y.V.; Gao, X.M.; Chen, W.D. Transportable mid-infrared laser heterodyne radiometer operating in the shot-noise dominated regime. Opt. Lett. 2021, 46, 3171–3174. [Google Scholar] [CrossRef]
- Lu, X.J.; Cao, Z.S.; Tan, T.; Huang, Y.B.; Gao, X.M.; Rao, R.Z. Instrument line shape function of laser heterodyne spectrometer. Acta Phys. Sin. 2019, 68, 064208. [Google Scholar] [CrossRef]
- Tsai, T.; Rose, R.A.; Weidmann, D.; Wysocki, G. Atmospheric vertical profiles of O3, N2O, CH4, CCl2F2, and H2O retrieved from external-cavity quantum-cascade laser heterodyne radiometer measurements. Appl. Opt. 2012, 51, 8779–8792. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, J.J.; Wang, G.S.; Tan, T.; Zhu, G.; Sun, C.Y.; Cao, Z.S.; Chen, W.D.; Gao, X.M. Mid-infrared laser heterodyne radiometer (LHR) based on a 3.53 μm room-temperature interband cascade laser. Opt. Exp. 2019, 27, 9610–9619. [Google Scholar] [CrossRef] [PubMed]
- Brasseur, G.P.; Jacob, D.J. Modeling of Atmospheric Chemistry; Cambridge University Press: Cambridge, UK, 2017; pp. 84–204. [Google Scholar]
- Ehhalt, D.H. Concentrations and distributions of atmospheric trace gases. Ber. Bunsenges. Phys. Chem. 1992, 96, 229–240. [Google Scholar] [CrossRef]
- Tsai, T. External cavity quantum cascade lasers for spectroscopic applications. Doctoral Dissertation, Princeton University, Princeton, NJ, USA, 2012. [Google Scholar]
- Mahieu, E.; Duchatelet, P.; Demoulin, P.; Walker, K.A.; Dupuy, E.; Froidevaux, L.; Randall, C.; Catoire, V.; Strong, K.; Boone, C.D.; et al. Validation of ACE-FTS v2.2 measurements of HCl, HF, CCl3F and CCl2F2 using space-, balloon- and ground-based instrument observations. Atmos. Chem. Phys. 2008, 8, 6199–6221. [Google Scholar] [CrossRef] [Green Version]
- Marquart, S.; Ponater, M.; Mager, F.; Sausen, R. Future development of contrail cover, optical depth, and radiative forcing: Impacts of increasing air traffic and climate change. J. Clim. 2003, 16, 2890–2904. [Google Scholar] [CrossRef]
- Scofield, J.H. Frequency domain description of a lock-in amplifier. Am. J. Phys. 1994, 62, 129–133. [Google Scholar] [CrossRef]
- Wang, G.X.; Rebou, S.; Choque, J.; Fertein, E.; Chen, W.D. Circular regression-based dual-phase lock-in amplifier for coherent recovery of weak signal. Sensors 2017, 17, 2615. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- SRS. About Lock-in Amplifiers. Available online: http://www.thinksrs.com/downloads/PDFs/ApplicationNotes/AboutLIAs.pdf (accessed on 16 May 2015).
- Rohart, F.; Mejri, S.; Sow, P.; Tokunaga, S.K.; Chardonnet, C.; Darquié, B.; Dinesan, H.; Fasci, E.; Castrillo, A.; Gianfrani, L. Absorption line shape recovery beyond the detection bandwidth limit: Application to the precision spectroscopic measurement of the Boltzmann constant. Phys. Rev. A Am. Phys. Soc. 2014, 90, 042506–042630. [Google Scholar] [CrossRef] [Green Version]
- Pelant, I.; Valenta, J. Luminescence Spectroscopy of Semiconductors. Oxford Scholarship Online. 2012. Available online: https://oxford.universitypressscholarship.com/view/10.1093/acprof:oso/9780199588336.001.0001/acprof-9780199588336 (accessed on 1 May 2012).
- Brodersen, S. Noise filter effects in recording spectroscopes. J. Opt. Soc. Am. 1953, 43, 1216–1220. [Google Scholar] [CrossRef]
- Demtröder, W. Laser Spectroscopy: Basic Concepts and Instrumentation; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Kostiuk, T.; Mumma, M.J. Remote sensing by IR heterodyne spectroscopy. Appl. Opt. 1983, 22, 2644–2654. [Google Scholar] [CrossRef] [PubMed]
- Shen, F.J.; Akil, J.; Wang, G.X.; Poupin, C.; Cousin, R.; Siffert, S.; Fertein, E.; Ba, T.; Chen, W.D. Real-time monitoring of N2O production in a catalytic reaction process using mid-infrared quantum cascade laser. J. Quant. Spectrosc. Radiat. Transf. 2018, 221, 1–7. [Google Scholar] [CrossRef]
- Gordon, I.E.; Rothman, L.S.; Hill, C.; Kochanov, R.V.; Tan, Y.; Bernath, P.F.; Birk, M.; Boudon, V.; Campargue, A.; Chance, K.V.; et al. The HITRAN2016 molecular spectroscopic database. J. Quant. Spectrosc. Rad. Transf. 2017, 203, 3–69. [Google Scholar] [CrossRef]
- Té, Y.; Jeseck, P.; Franco, B.; Mahieu, E.; Jones, N.P.; Paton-Walsh, C.; Griffith, D.W.T.; Buchholz, R.R.; Hadji-Lazaro, J.; Hurtmans, D.; et al. Seasonal variability of surface and column carbon monoxide over the megacity Paris, high-altitude Jungfraujoch and Southern Hemispheric Wollongong stations. Atmos. Chem. Phys. 2016, 16, 10911–10925. [Google Scholar] [CrossRef] [Green Version]
- Kämpfer, N. Monitoring Atmospheric Water Vapour: Ground-Based Remote Sensing and In Situ Methods; Springer: New York, NY, USA, 2013; Volume 13, pp. 1–7. [Google Scholar]
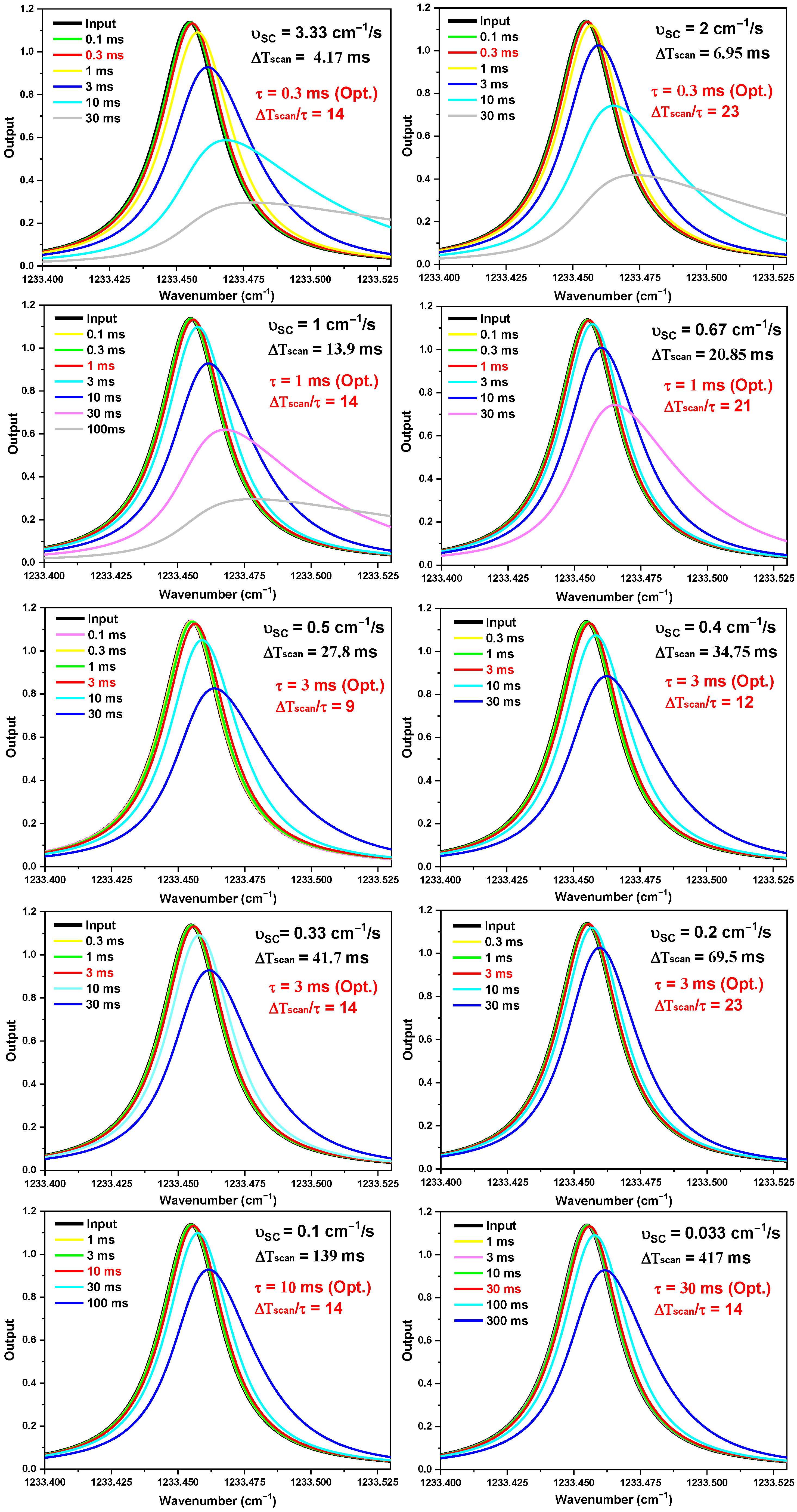


| Halfwidth ΔνHWHM (cm−1) | 0.014 | 0.014 | 0.014 | 0.014 | 0.014 | 0.014 | 0.014 | 0.014 | 0.014 | 0.014 |
| Scanning range Δνscan (cm−1) | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| Scanning period T (s) | 0.3 | 0.5 | 1 | 1.5 | 2 | 2.5 | 3 | 5 | 10 | 30 |
| Scan frequency ν (Hz) | 3.33 | 2 | 1 | 0.67 | 0.5 | 0.4 | 0.33 | 0.2 | 0.1 | 0.033 |
| Scanning speed υsc (cm−1/s) = Δνscan/T | 3.33 | 2 | 1 | 0.67 | 0.5 | 0.4 | 0.33 | 0.2 | 0.1 | 0.033 |
| Scanning time ΔTscan (ms) | 4.17 | 6.95 | 13.9 | 20.85 | 27.8 | 34.75 | 41.7 | 69.5 | 139 | 417 |
| τ (ms) | 0.1 | 0.1 | 0.1 | 0.1 | 0.1 | 0.3 | 0.3 | 0.3 | 1 | 1 |
| 0.3 | 0.3 | 0.3 | 0.3 | 0.3 | 1 | 1 | 1 | 3 | 3 | |
| 1 | 1 | 1 | 1 | 1 | 3 | 3 | 3 | 10 | 10 | |
| 3 | 3 | 3 | 3 | 3 | 10 | 10 | 10 | 30 | 30 | |
| 10 | 10 | 10 | 10 | 10 | 30 | 30 | 30 | 100 | 100 | |
| 30 | 30 | 30 | 30 | 30 | - | - | - | - | 300 | |
| - | - | 100 | - | - | - | - | - | - | - |
| Scanning Frequency (mHz) | 20 | 50 | 100 | 250 |
| Scanning period T (s) | 25 | 10 | 5 | 2 |
| Scanning range Δνscan (cm−1) | 0.787 | 0.787 | 0.787 | 0.787 |
| halfwidth ΔνHWHM (cm−1) | 0.0538 | 0.0538 | 0.0538 | 0.0538 |
| Scanning speed υsc (cm−1/s) = Δνscan/T | 0.03148 | 0.0787 | 0.1574 | 0.3935 |
| Scanning time ΔTscan (ms) = ΔνHWHM /υsc | 1709 | 684 | 342 | 137 |
| τ (ms) | 100 | 30 | 10 | 1 |
| 300 | 100 | 30 | 10 | |
| 1000 | 300 | 100 | 30 | |
| - | 1000 | 300 | - |
| υsc (cm−1/s) | ΔTscan (ms) | τ (ms) | SNR | Δνb (cm−1) | Absorption Depth | δν (cm−1) | Fit Residual (1σ) |
|---|---|---|---|---|---|---|---|
| 0.03148 | 1709 | 100 | 5 | 0.0545 | 0.0126 | 0.0032 | 0.0026 |
| 300 | 8 | 0.0656 | 0.0114 | 0.0095 | 0.0015 | ||
| 1000 | 8 | 0.0711 | 0.0062 | 0.0325 | 0.0008 | ||
| 0.0787 | 684 | 30 | 5 | 0.0548 | 0.01258 | 0.0031 | 0.00230 |
| 100 | 6 | 0.0640 | 0.01244 | 0.0081 | 0.00210 | ||
| 300 | 0.0722 | 0.01154 | 0.0243 | 0.00180 | |||
| 1000 | 6 | 0.1222 | 0.00570 | 0.0821 | 0.00089 | ||
| 0.1574 | 342 | 10 | 5 | 0.0550 | 0.01251 | 0.0016 | 0.00230 |
| 30 | 6 | 0.0627 | 0.01204 | 0.0048 | 0.00209 | ||
| 100 | 7 | 0.0672 | 0.01101 | 0.0181 | 0.00156 | ||
| 300 | 7 | 0.0812 | 0.00824 | 0.0490 | 0.00115 | ||
| 0.3935 | 137 | 1 | 7 | 0.0541 | 0.01261 | 0.0004 | 0.00174 |
| 10 | 9 | 0.0599 | 0.01181 | 0.0041 | 0.00129 | ||
| 30 | 8 | 0.0733 | 0.00736 | 0.0120 | 0.00096 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, F.; Wang, G.; Xue, Z.; Tan, T.; Cao, Z.; Gao, X.; Chen, W. Impact of Lock-In Time Constant on Remote Monitoring of Trace Gas in the Atmospheric Column Using Laser Heterodyne Radiometer (LHR). Remote Sens. 2022, 14, 2923. https://doi.org/10.3390/rs14122923
Shen F, Wang G, Xue Z, Tan T, Cao Z, Gao X, Chen W. Impact of Lock-In Time Constant on Remote Monitoring of Trace Gas in the Atmospheric Column Using Laser Heterodyne Radiometer (LHR). Remote Sensing. 2022; 14(12):2923. https://doi.org/10.3390/rs14122923
Chicago/Turabian StyleShen, Fengjiao, Gaoxuan Wang, Zhengyue Xue, Tu Tan, Zhensong Cao, Xiaoming Gao, and Weidong Chen. 2022. "Impact of Lock-In Time Constant on Remote Monitoring of Trace Gas in the Atmospheric Column Using Laser Heterodyne Radiometer (LHR)" Remote Sensing 14, no. 12: 2923. https://doi.org/10.3390/rs14122923
APA StyleShen, F., Wang, G., Xue, Z., Tan, T., Cao, Z., Gao, X., & Chen, W. (2022). Impact of Lock-In Time Constant on Remote Monitoring of Trace Gas in the Atmospheric Column Using Laser Heterodyne Radiometer (LHR). Remote Sensing, 14(12), 2923. https://doi.org/10.3390/rs14122923