Numerical Simulation of the Simultaneous Development of Multiple Fractures in Horizontal Wells Based on the Extended Finite Element Method
Abstract
1. Introduction
2. Governing Equations
2.1. Model Assumptions
- (1)
- The rock matrix material is considered to be homogeneous and elastic;
- (2)
- The injected fluid is considered incompressible;
- (3)
- The proppant transport within multiple fractures is not taken into account;
- (4)
- The influence of the temperature field is not considered in the simulation.
2.2. The Flow Mode of the Fluid
- (1)
- In the fluid section, we need to describe the fluid flow process and the fluid filtration loss process within the fracture;
- (2)
- In the soil section, we need some initiation and propagation criteria to describe the whole process from the deformation to the destruction of rocks.
2.3. The Formula for Effective Stress
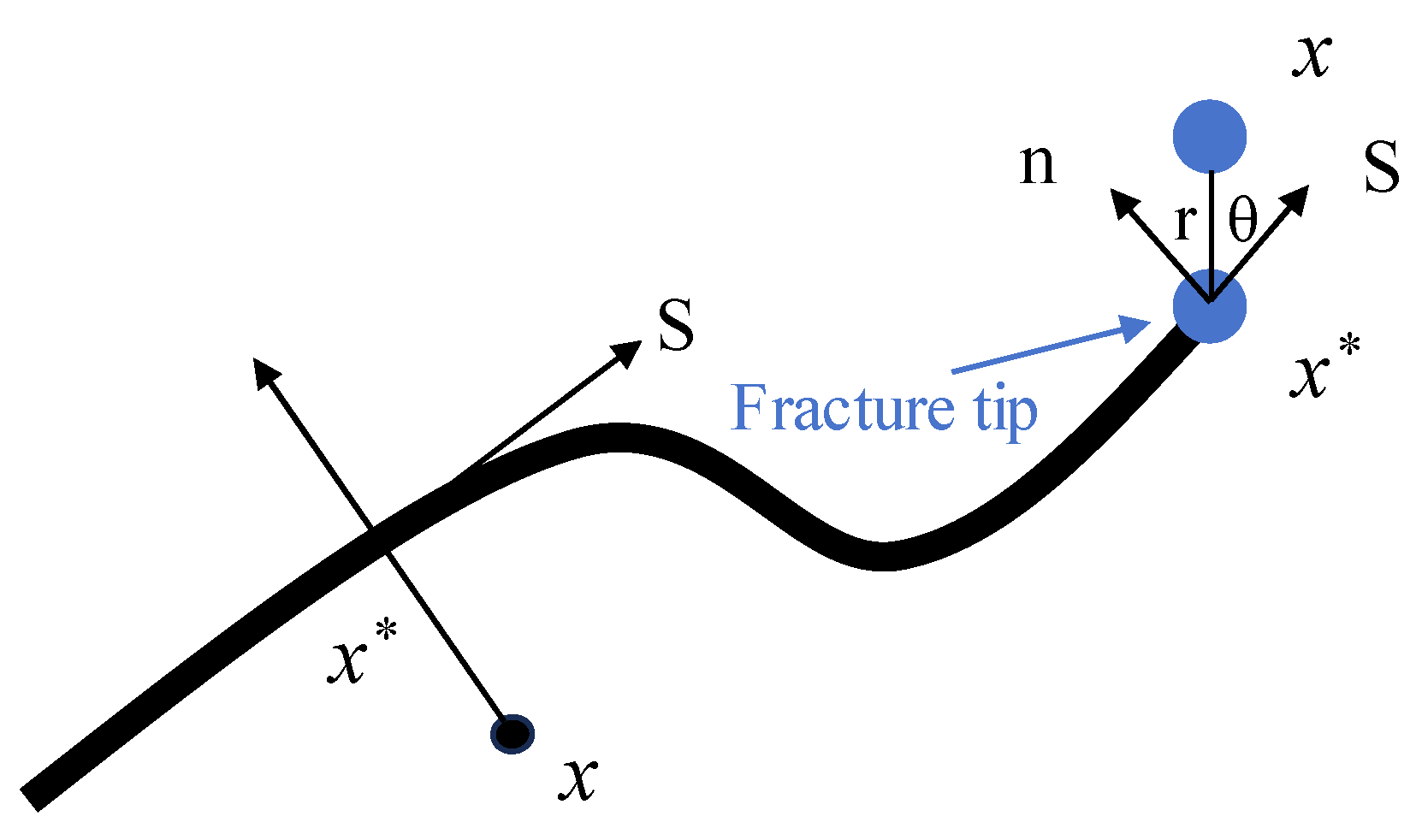
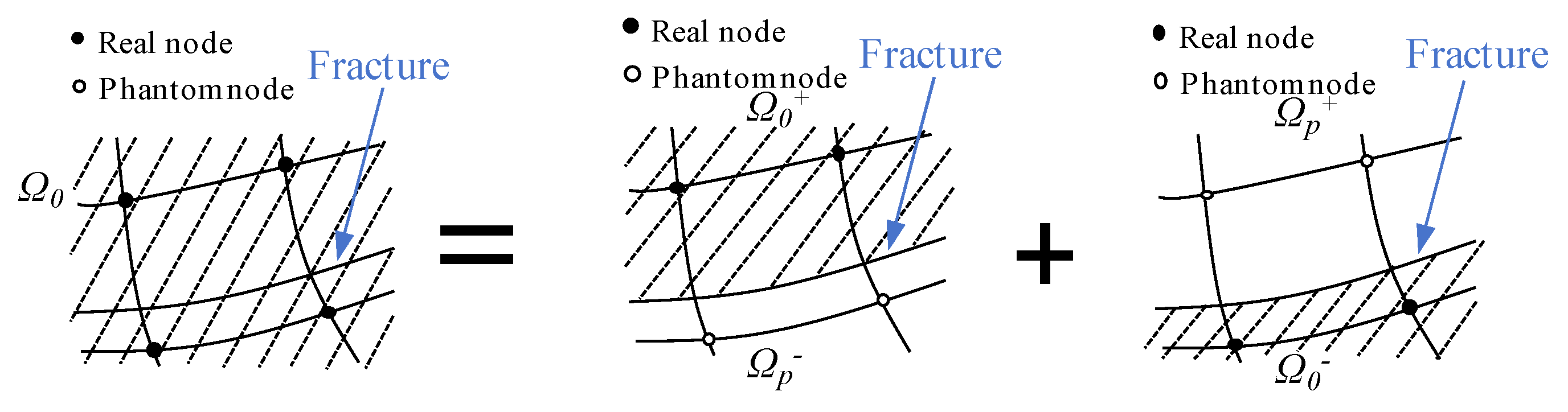
2.4. XFEM Approximation
2.5. The Mechanism of Fracture Failure
3. Model Establishment and Verification
3.1. Establishment of a Multiple-Fracture Development Model and the Parameterization of Different Cases
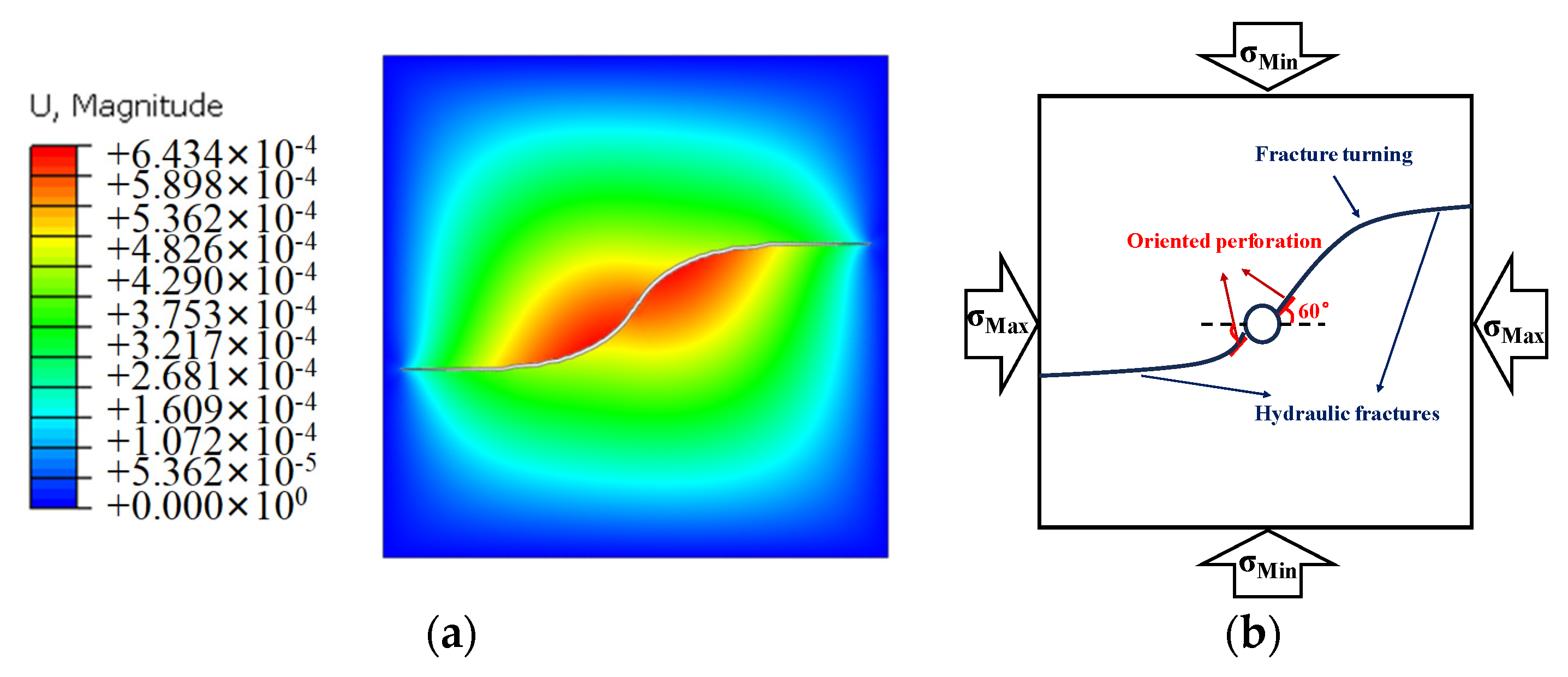
3.2. The Validation of Fracture Propagation
4. Results and Discussion
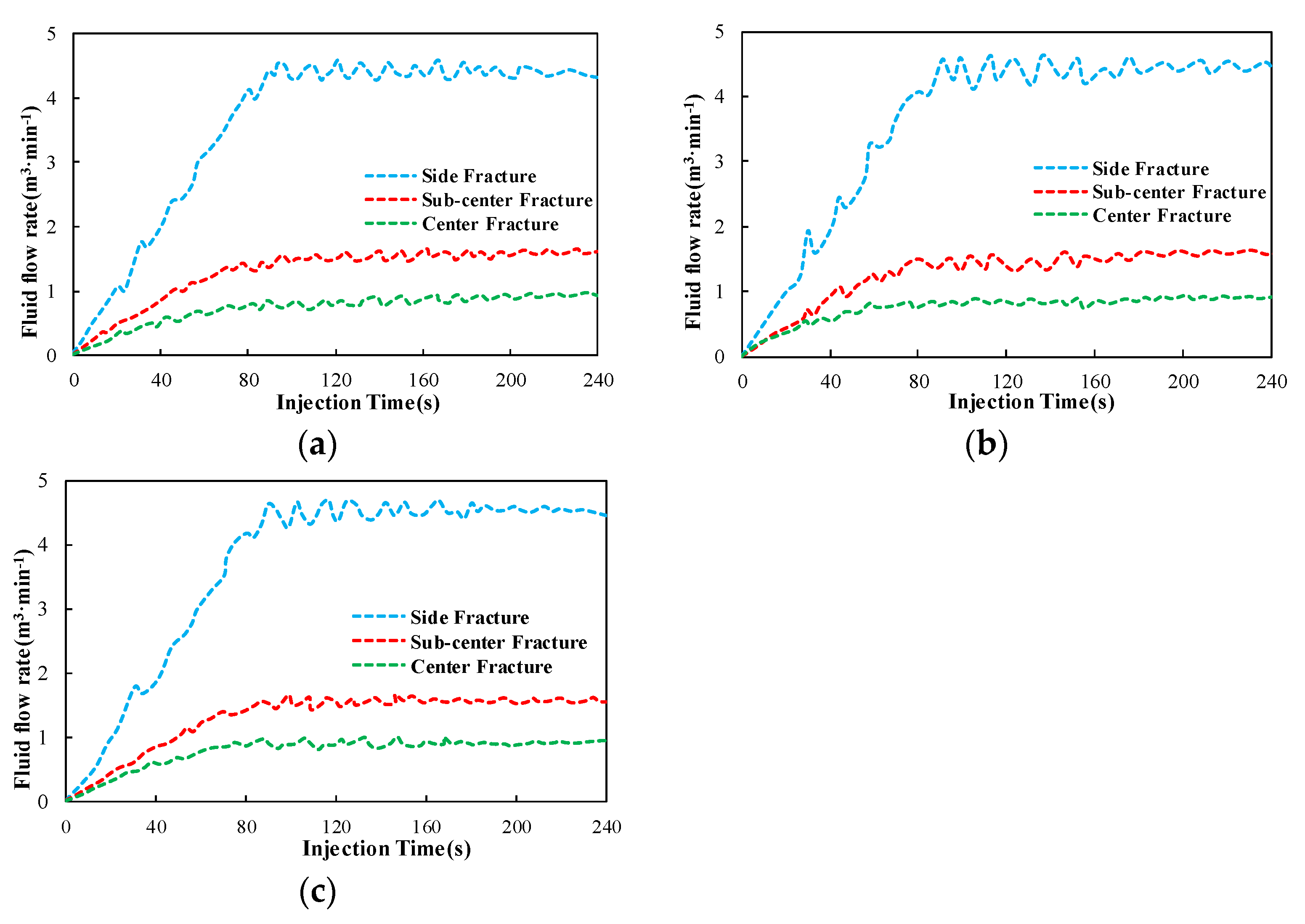
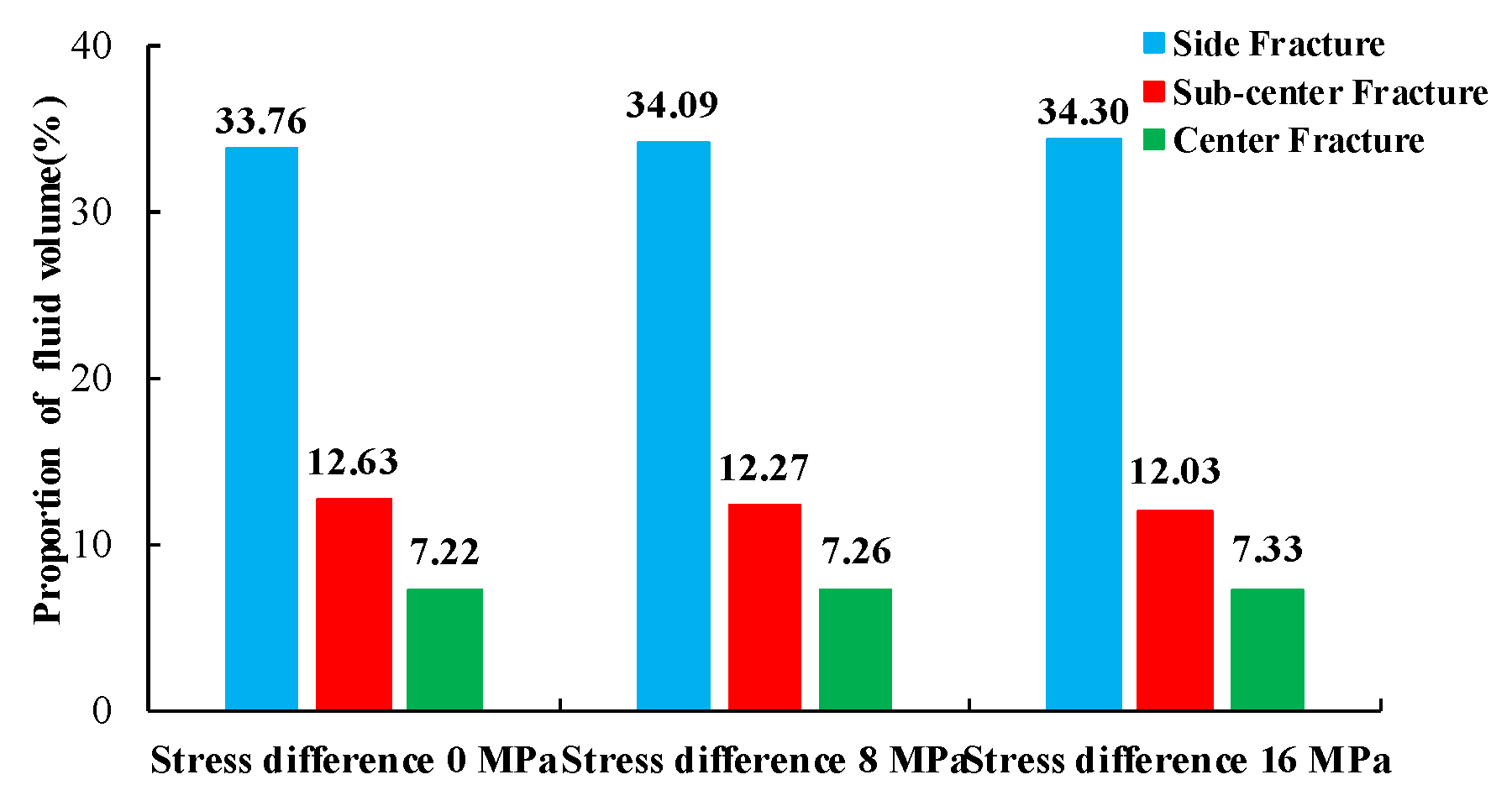
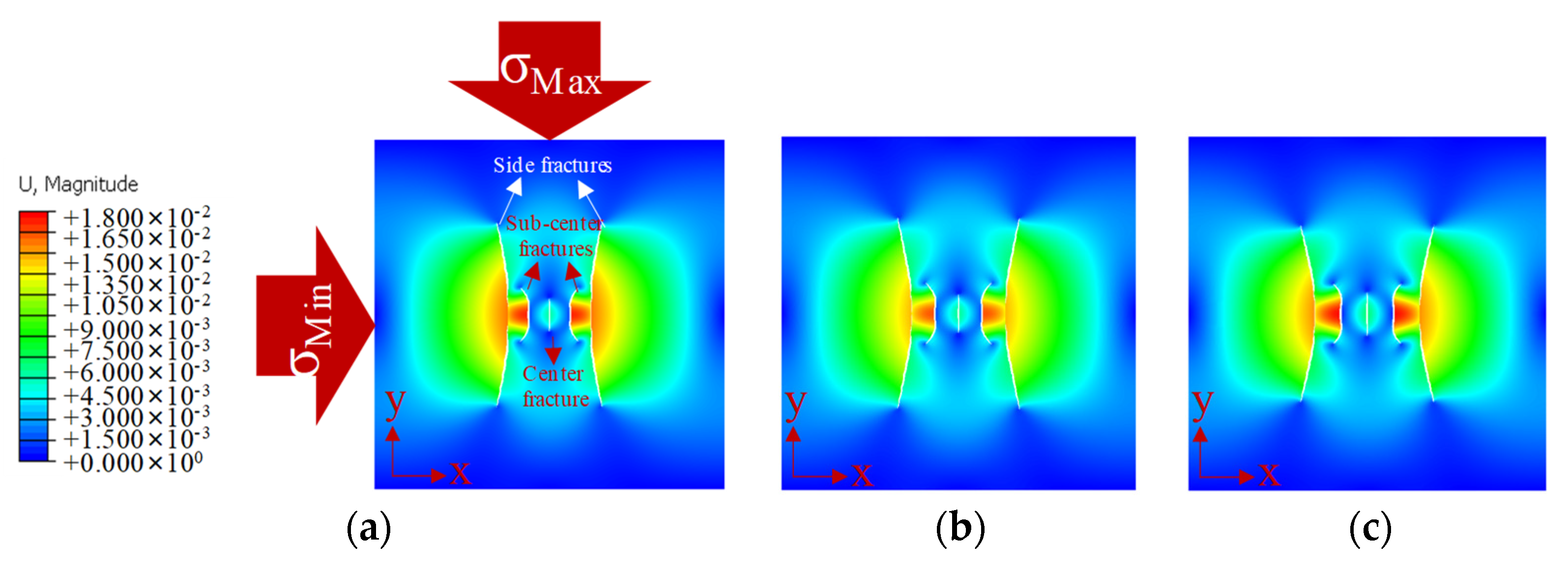
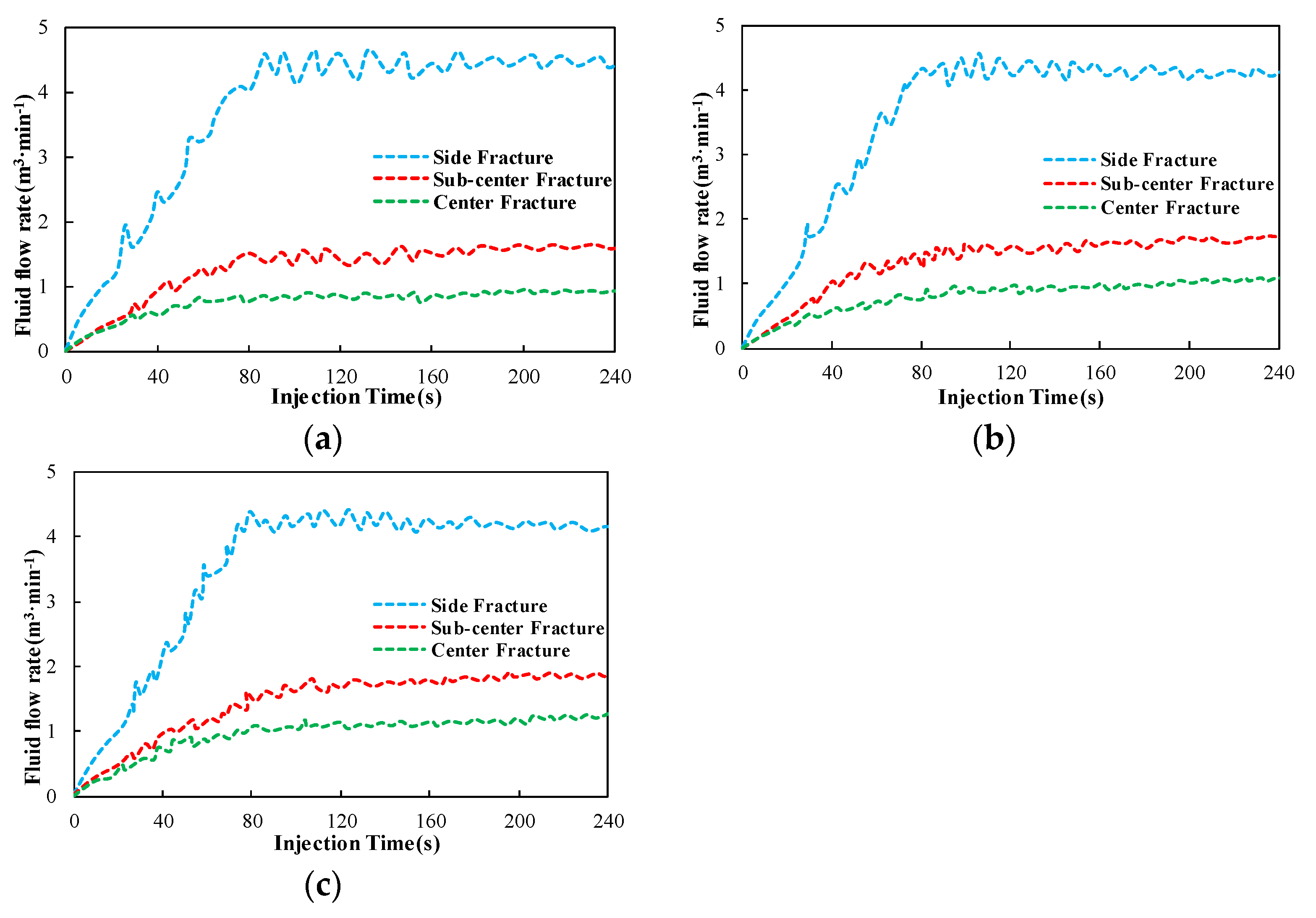
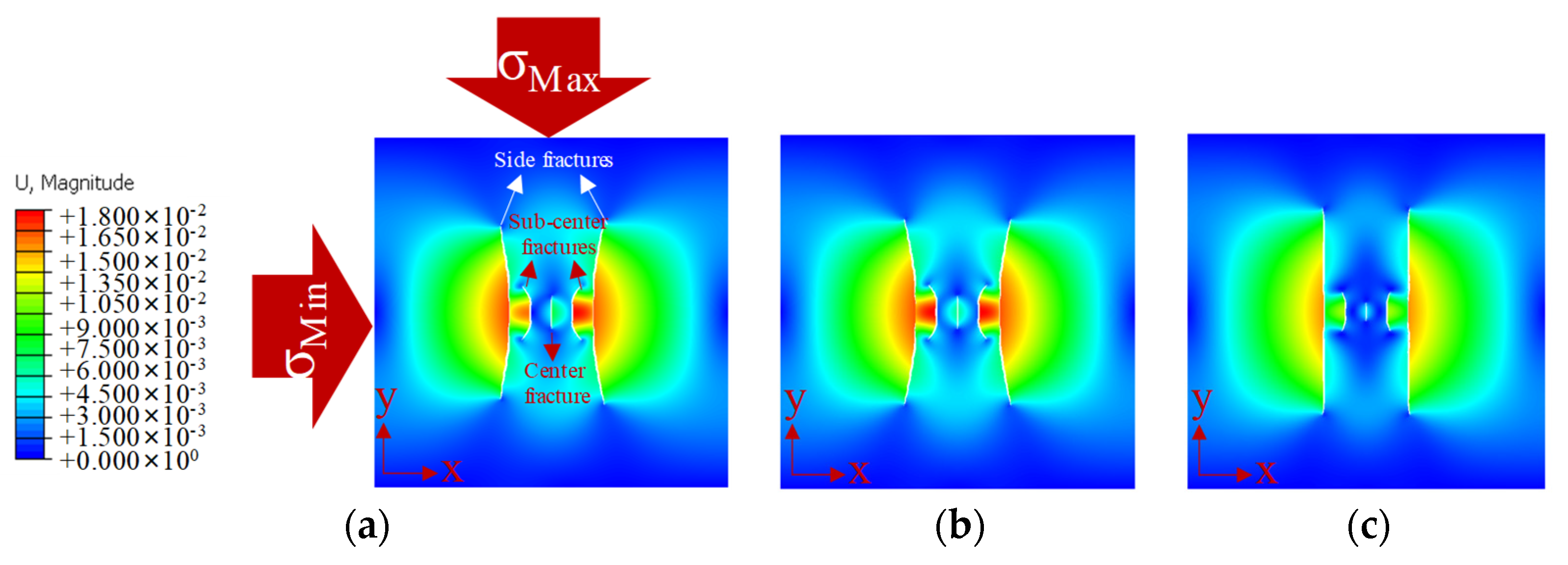
4.1. The Effect of In Situ Stress Difference (Cases 1–3)
4.2. The Effects of Cluster Spacing (Cases 2, 4, and 5)
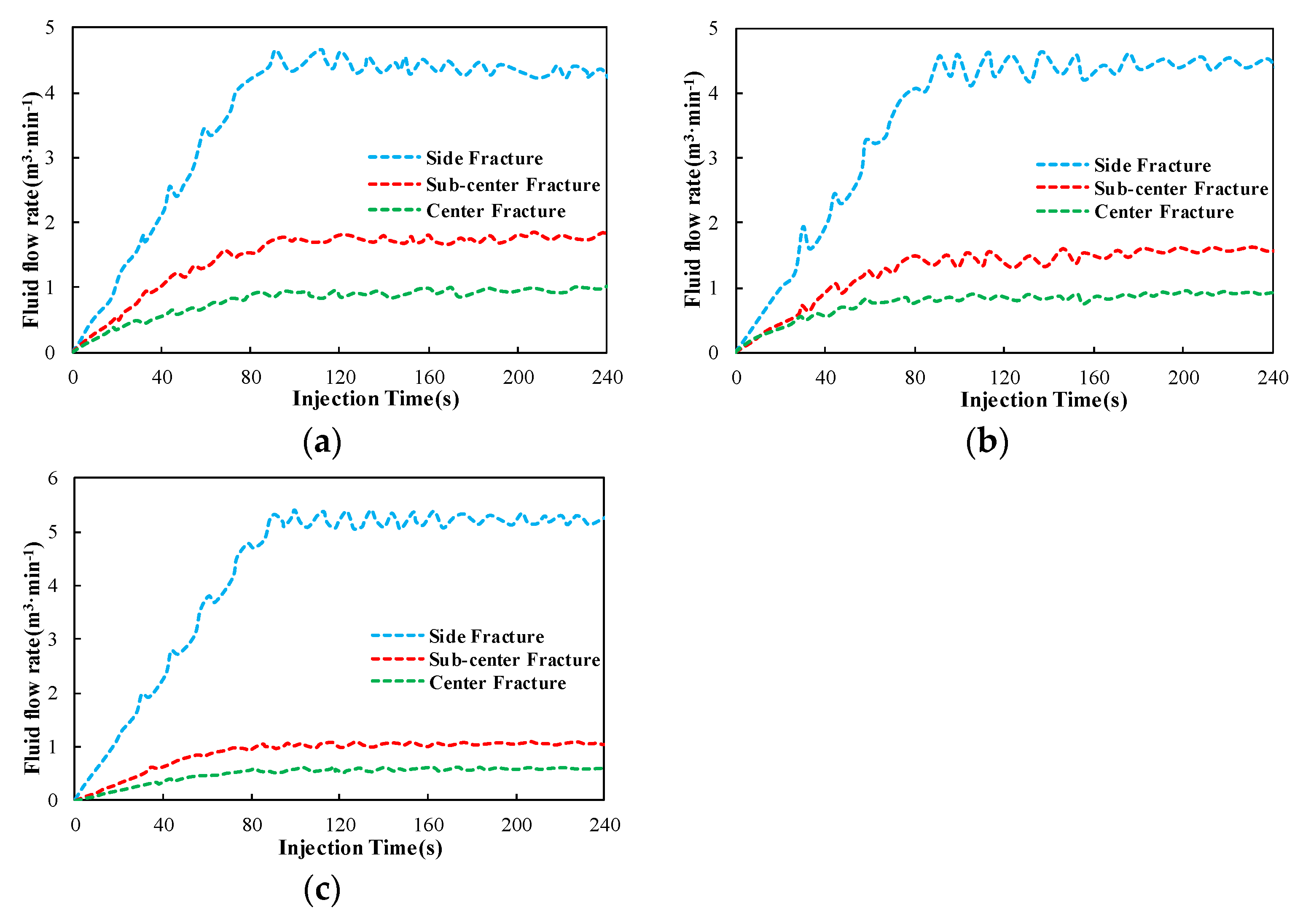
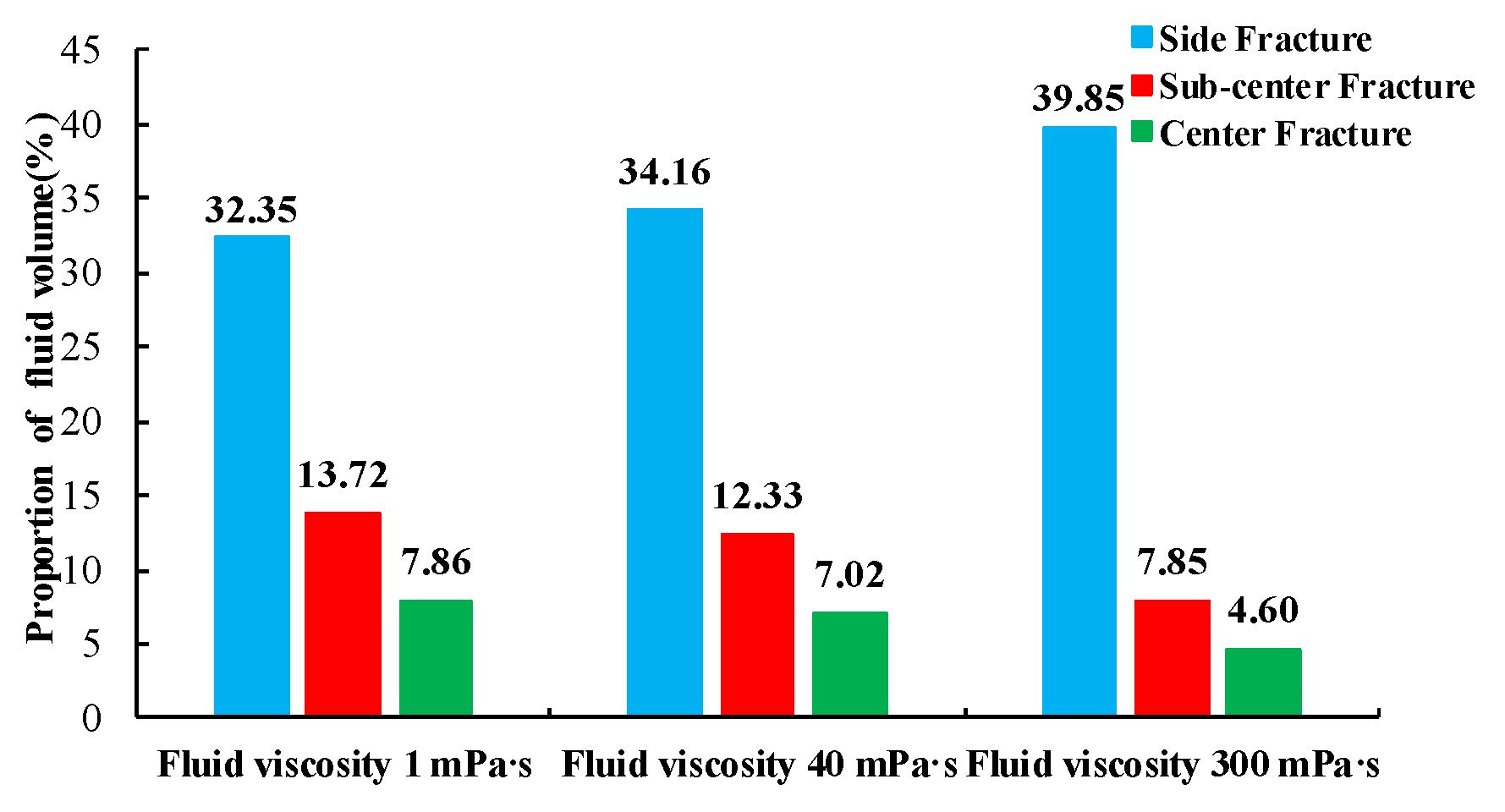
4.3. The Effects of Fracturing Fluid Viscosity (Cases 2, 6, and 7)
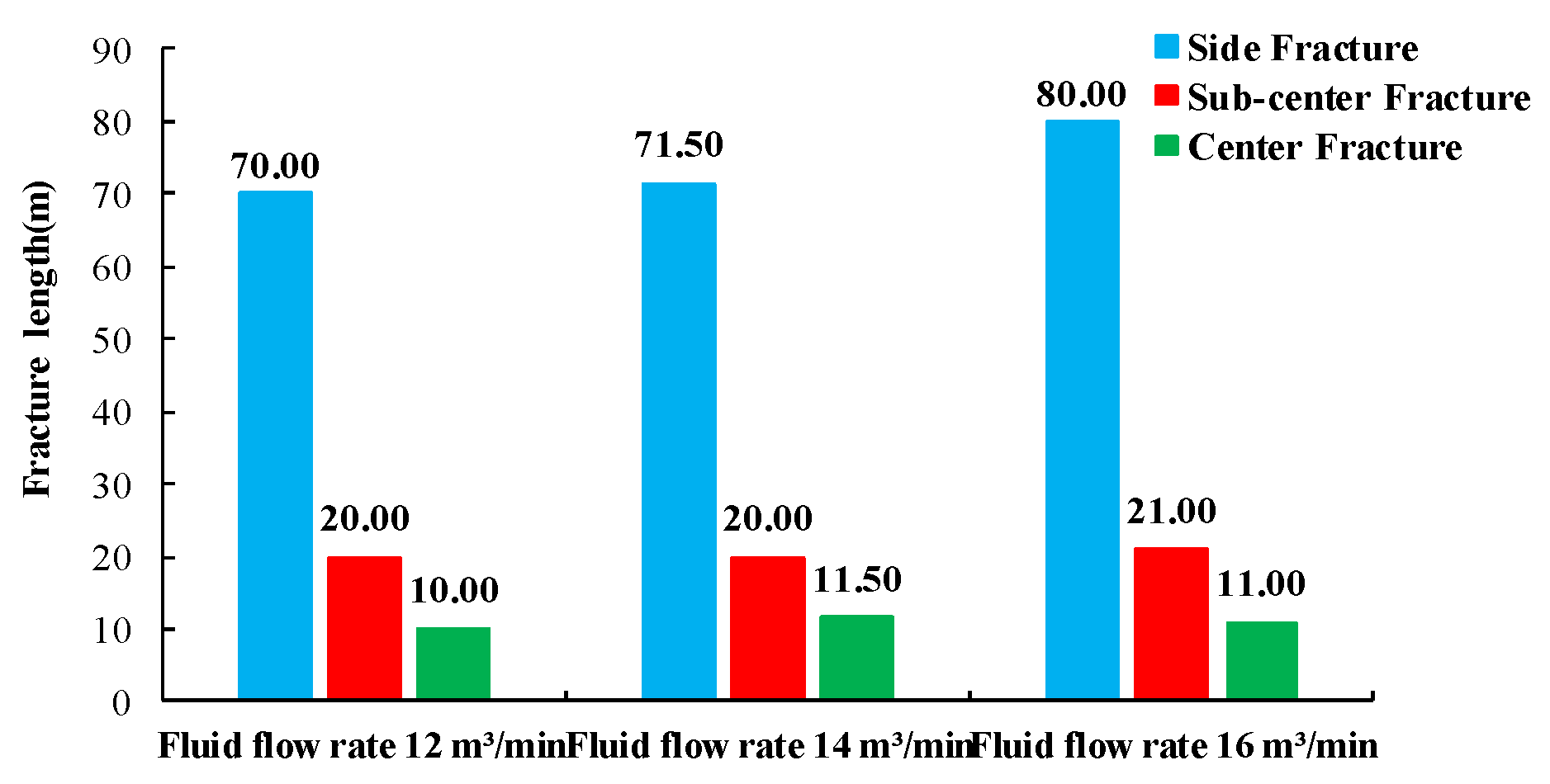
4.4. The Effect of Injection Rate (Cases 2, 8, and 9)
5. Discussion and Conclusions
- The influence of the in situ stress difference on the flow rate distribution among multiple fractures is not obvious. However, with the increase in the in situ stress difference, side fractures with larger deflections gradually propagate along the direction of the horizontal stress with the maximum value. In addition, the fracture length significantly increases.
- The cluster spacing can effectively affect the stress interference between multiple fractures. The uniformity of the fracture morphology and flow rate distribution can be significantly improved by increasing the cluster spacing.
- As the viscosity of the fracturing fluid increases, the stress-shadow effect is further enhanced, and more fluid flows into the dominant fracture. When the viscosity increases from 1 mPa·s to 40 mPa·s, the deflection angle of the side fractures increases, as does the fracture network area. When the viscosity reaches 300 mPa·s, the development of internal fractures is greatly inhibited, and the side fractures are no longer deflected.
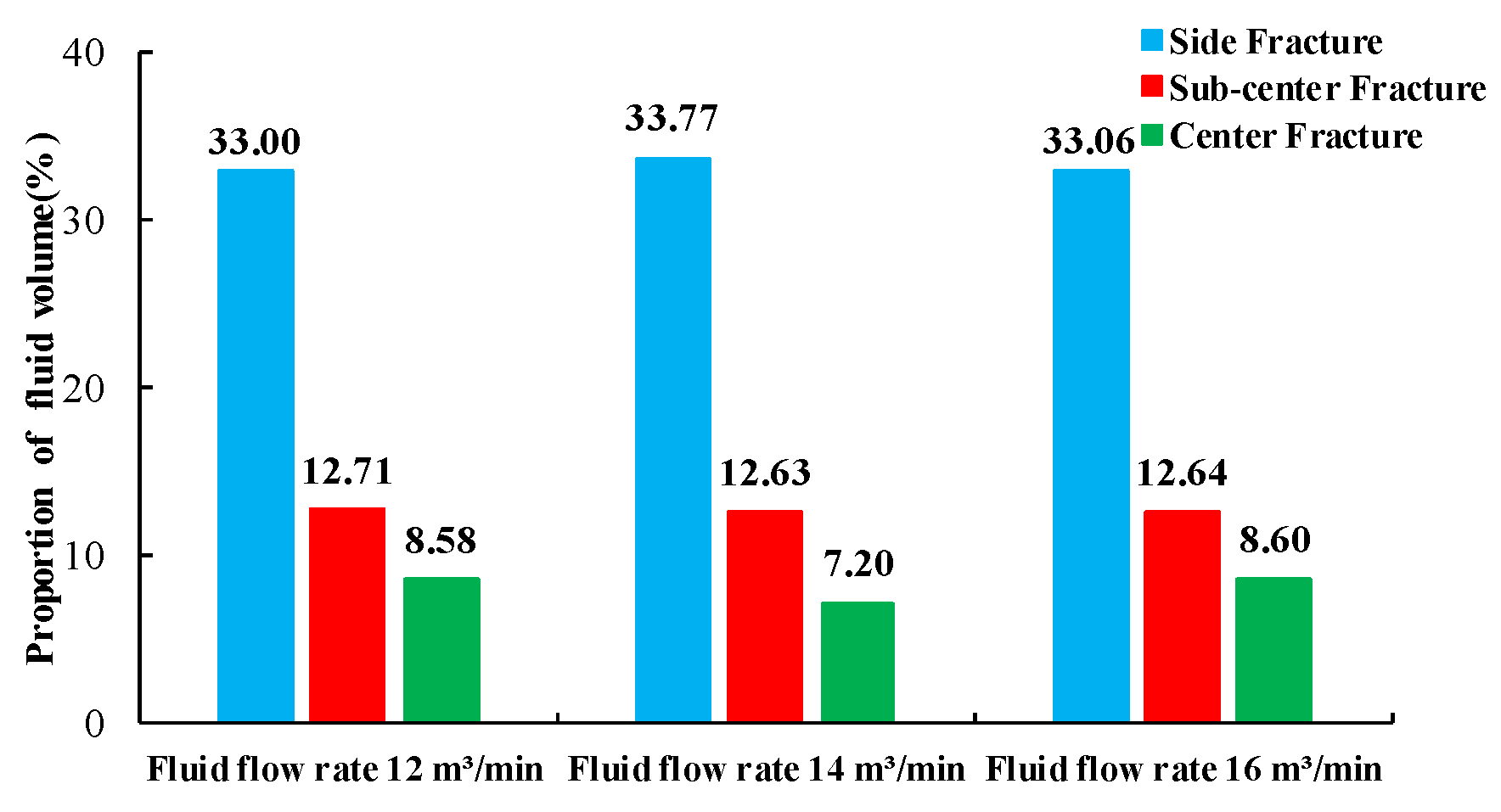
- A higher injection rate has little effect on the inflow of fluid into the internal fractures and on their morphology. On the other hand, with the same proportion of the fluid volume, the length of the side fractures significantly increases, favoring the formation of a larger fracture network area.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, L.; Liu, J.; Wang, W.; Zhao, P.; Lu, Y. Variational Reservoir Characteristics of the Wufeng–Longmaxi Formation from Different Sedimentary Region and Its Implications in Southeastern Sichuan Basin, China. ACS Omega 2023, 8, 20684–20696. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Yao, Y.; Liu, D.; Pan, Z.; Cai, Y. Comparison of Three Key Marine Shale Reservoirs in the Southeastern Margin of the Sichuan Basin, SW China. Minerals 2017, 7, 179. [Google Scholar] [CrossRef]
- Zhao, P.; Liu, J.; Elsworth, D. Numerical study on a multifracture enhanced geothermal system considering matrix permeability enhancement induced by thermal unloading. Renew. Energ. 2023, 2023, 33–44. [Google Scholar] [CrossRef]
- Liu, J.; Bai, X.; Elsworth, D. Evolution of pore systems in low-maturity oil shales during thermal upgrading-Quantified by dynamic SEM and machine learning. Pet. Sci. 2024, in press. [Google Scholar] [CrossRef]
- Liu, J.; Xie, L.; Elsworth, D.; Gan, Q. CO2/CH4 Competitive Adsorption in Shale: Implications for Enhancement in Gas Production and Reduction in Carbon Emissions. Environ. Sci. Technol. 2019, 53, 9328–9336. [Google Scholar] [CrossRef] [PubMed]
- Desroches, J.; Lecampion, B.; Ramakrishnan, H.; Brown, J.E.; Prioul, R. Benefits of Controlled Hydraulic Fracture Placement: Theory and Field Experiment. In Proceedings of the SPE/CSUR Unconventional Resources Conference, Calgary, AB, Canada, 30 September–2 October 2014. [Google Scholar]
- Zhao, J.; Wang, L.; Liu, S.; Kang, Z.; Yang, D.; Zhao, Y. Numerical simulation and thermo-hydro-mechanical coupling model of in situ mining of low-mature organic-rich shale by convection heating. Adv. Geo-Energy Res. 2022, 6, 502–514. [Google Scholar] [CrossRef]
- Wu, K. Simultaneous Multi-Frac Treatments: Fully Coupled Fluid Flow and Fracture Mechanics for Horizontal Wells. In Proceedings of the SPE Annual Technical Conference and Exhibition, New Orleans, LA, USA, 30 September–2 October 2013. [Google Scholar]
- Wu, K.; Olson, J.E.; Balhoff, M.T.; Yu, W. Numerical Analysis for Promoting Uniform Development of Simultaneous Multiple-Fracture Propagation in Horizontal Wells. SPE Prod. Oper. 2016, 32, 41–50. [Google Scholar]
- Warpinski, N.R.; Teufel, L.W. Influence of Geologic Discontinuities on Hydraulic Fracture Propagation. J. Pet. Technol. 1987, 39, 209–220. [Google Scholar] [CrossRef]
- Zeng, F.; Yang, B.; Guo, J.; Chen, Z.; Xiang, J. Experimental and Modeling Investigation of Fracture Initiationfrom Open-Hole Horizontal Wells in Permeable Formations. Rock Mech. 2019, 52, 1133–1148. [Google Scholar] [CrossRef]
- Ha, S.J.; Choo, J.; Yun, T.S. Liquid CO2 Fracturing: Effect of Fluid Permeation on the Breakdown Pressure and Cracking Behavior. Rock Mech. 2018, 51, 3407–3420. [Google Scholar] [CrossRef]
- Zhang, W.; Shi, X.; Jiang, S.; Cao, Q.; Wang, F.; Wang, Z.; Ge, Y.; Du, Y. Experimental study of hydraulic fracture initiation and propagation in highly saturated methane-hydrate-bearing sands. J. Nat. Gas Sci. Eng. 2020, 79, 103338. [Google Scholar] [CrossRef]
- Liu, X.; Qu, Z.; Guo, T.; Sun, Y.; Wang, Z.; Bakhshi, E. Numerical simulation of non-planar fracture propagation in multi-cluster fracturing with natural fractures based on Lattice methods. Eng. Fract. Mech. 2019, 220, 106625. [Google Scholar] [CrossRef]
- Qu, Z.; Tian, Y.; Li, J.; Guo, T. Numerical simulation study on fracture extension and morphology of multi-cluster staged fracturing for horizontal wells. J. China Univ. Pet. Ed. Nat. Sci. 2017, 41, 102–109. [Google Scholar]
- Fallahzadeh, S.H.; Rasouli, V.; Sarmadivaleh, M. An Investigation of Hydraulic Fracturing Initiation and Near-Wellbore Propagation from Perforated Boreholes in Tight Formations. Rock Mech. 2015, 48, 573–584. [Google Scholar] [CrossRef]
- Wu, K.; Olson, J.E. Mechanisms of Simultaneous Hydraulic Fracture Propagation From Multiple Perforation Clusters in Horizontal Wells. SPE J. 2016, 21, 1000–1008. [Google Scholar] [CrossRef]
- Bunger, A.; Jeffrey, R.G.; Zhang, X. Constraints on simultaneous growth of hydraulic fractures from multiple perforation clusters in horizontal wells. SPE J. 2014, 19, 608–620. [Google Scholar] [CrossRef]
- Wang, W.; Da, Z.; Sheng, G.; Qi, Z.; Su, Y. A review of stimulated reservoir volume characterization for multiple fractured horizontal well in unconventional reservoirs. Adv. Geo-Energy Res. 2017, 1, 54–63. [Google Scholar] [CrossRef]
- Miller, C.; Waters, G.; Rylander, E. Evaluation of Production Log Data from Horizontal Wells Drilled in Organic Shales. In Proceedings of the SPE North American Unconventional Gas Conference and Exhibition, Woodlands, TX, USA, 14–16 June 2011. [Google Scholar]
- Van Ketterij, R.B.; De Pater, C.J. Impact of Perforations on Hydraulic Fracture Tortuosity. SPE Prod. Fac. 1999, 14, 117–130. [Google Scholar] [CrossRef]
- Hui, G.; Chen, Z.; Chen, S.; Gu, F. Hydraulic fracturing-induced seismicity characterization through coupled modeling of stress and fracture-fault systems. Adv. Geo-Energy Res. 2022, 6, 269–270. [Google Scholar] [CrossRef]
- Liu, J.; Xie, L.; He, B.; Gan, Q.; Zhao, P. Influence of anisotropic and heterogeneous permeability coupled with in-situ stress on CO2 sequestration with simultaneous enhanced gas recovery in shale: Quantitative modeling and case study. Int. J. Greenhouse Gas Control 2021, 104, 103208. [Google Scholar] [CrossRef]
- Zhao, P.; Liu, J.; Zhang, Y. Experimental and numerical investigations on buffer performance of geofoam subjected by the impact of falling rocks with respect to different shapes. Geotext. Geomembr. 2023, 51, 108–124. [Google Scholar] [CrossRef]
- Abdelaziz, A.; Ha, J.; Li, M.; Magsipoc, E.; Sun, L.; Grasselli, G. Understanding hydraulic fracture mechanisms: From the laboratory to numerical modelling. Adv. Geo-Energy Res. 2023, 7, 66–68. [Google Scholar] [CrossRef]
- Guo, J.; Lu, Q.; Zhu, H.; Wang, Y.; Ma, L. Perforating cluster space optimization method of horizontal well multi-stage fracturing in extremely thick unconventional gas reservoir. J. Nat. Gas Sci. Eng. 2015, 26, 1648–1662. [Google Scholar] [CrossRef]
- Shin, D.H.; Sharma, M.M. Factors Controlling the Simultaneous Propagation of Multiple Competing Fractures in a Horizontal Well. In Proceedings of the SPE Hydraulic Fracturing Technology Conference, Woodlands, TX, USA, 4–6 February 2014. [Google Scholar]
- Wang, B.; Zhou, F.; Wang, D.; Liang, T. Numerical simulation on near-wellbore temporary plugging and diverting during refracturing using XFEM-Based CZM. J. Nat. Gas Sci. Eng. 2018, 55, 368–381. [Google Scholar] [CrossRef]
- Liu, Y.; Tang, D.; Xu, H.; Zhao, T.; Hou, W. Effect of interlayer mechanical properties on initiation and propagation of hydraulic fracturing in laminated coal reservoirs. J. Nat. Gas Sci. Eng. 2022, 208, 109381. [Google Scholar] [CrossRef]
- Huang, C.; Zhu, H.; Wang, J.; Han, J.; Zhou, G.; Tang, X. A FEM-DFN model for the interaction and propagation of multi-cluster fractures during variable fluid-viscosity injection in layered shale oil reservoir. Pet. Sci. 2022, 19, 2796–2809. [Google Scholar] [CrossRef]
- Zhu, H.; Xu, X.; Zhong, A.; Zhang, Q. Numerical simulation of evenly propagating hydraulic fractures with smaller cluster spacing in the horizontal well YYP1 for deep shale oil in the Shengli Oilfield. Oil Gas Geology. 2022, 43, 229–240. [Google Scholar]
- Guo, J.; Zhao, X.; Zhu, H.; Zhang, X.; Pan, R. Numerical simulation of interaction of hydraulic fracture and natural fracture based on the cohesive zone finite element method. J. Nat. Gas Sci. Eng. 2015, 25, 180–188. [Google Scholar] [CrossRef]
- Wang, Y.; Javadi, A.; Fidelibus, C. A hydro-mechanically-coupled XFEM model for the injection-induced evolution of multiple fractures. Int. J. Numer. Anal. Methods Geomech. 2023, 47, 1539–1558. [Google Scholar] [CrossRef]
- Dahi-Taleghani, A.; Olson, J.E. Numerical modeling of multistranded-hydraulic-fracture propagation: Accounting for the interaction between induced and natural fractures. SPE J. 2011, 16, 575–581. [Google Scholar] [CrossRef]
- Gordeliy, E.; Peirce, A. Implicit level set schemes for modeling hydraulic fractures using the XFEM. Comput. Method Appl. Mech. 2013, 266, 125–143. [Google Scholar] [CrossRef]
- Lecampion, B. An extended finite element method for hydraulic fracture problems. Commun. Numer. Methods Eng. 2009, 25, 121–133. [Google Scholar] [CrossRef]
- Leonhart, D.; Meschke, D. Extended finite element method for hydro-mechanical analysis of crack propagation in porous materials. Proc. Appl. Math. Mech. 2011, 11, 161–162. [Google Scholar] [CrossRef]
- Wang, H. Numerical investigation of fracture spacing and sequencing effects on multiple hydraulic fracture interference and coalescence in brittle and ductile reservoir rocks. Eng. Fract. Mech. 2016, 157, 107–124. [Google Scholar] [CrossRef]
- Ma, X.; Zeng, Q. Influence of multi-perforation fracturing on the regularity of hydraulic fractures propagation. Nat. Gas Geosci. 2022, 33, 312–323. [Google Scholar]
- Gordeliy, E.; Peirce, A. Coupling schemes for modeling hydraulic fracture propagation using the XFEM. Comput. Method Appl. Mech. 2013, 253, 305–322. [Google Scholar] [CrossRef]
- Cruz, F.; Rpehl, D.; Vargas, E.D. An XFEM implementation in Abaqus to model intersections between fractures in porous rocks. Comput. Geotech. 2019, 112, 135–146. [Google Scholar] [CrossRef]
- Yang, Q.; Li, B.; Shao, M.; Zhang, H. Production performance of perforation clusters during multistage fracturing in shale gas reservoirs. ACS Omega. 2021, 6, 26231–26238. [Google Scholar] [CrossRef]
- Belytschko, T.; Black, T. Elastic crack growth in finite elements with minimal remeshing. Int. J. Numer. Meth. Eng. 1999, 45, 601–620. [Google Scholar] [CrossRef]
- Jiang, H.; Chen, M.; Zhang, G.; Jin, Y.; Zhang, Z.; Zhu, G. Impact of oriented perforation on hydraulic fracture initiation and propagation. Chin. J. Rock Mech. Eng. 2009, 7, 1321–1326. [Google Scholar]






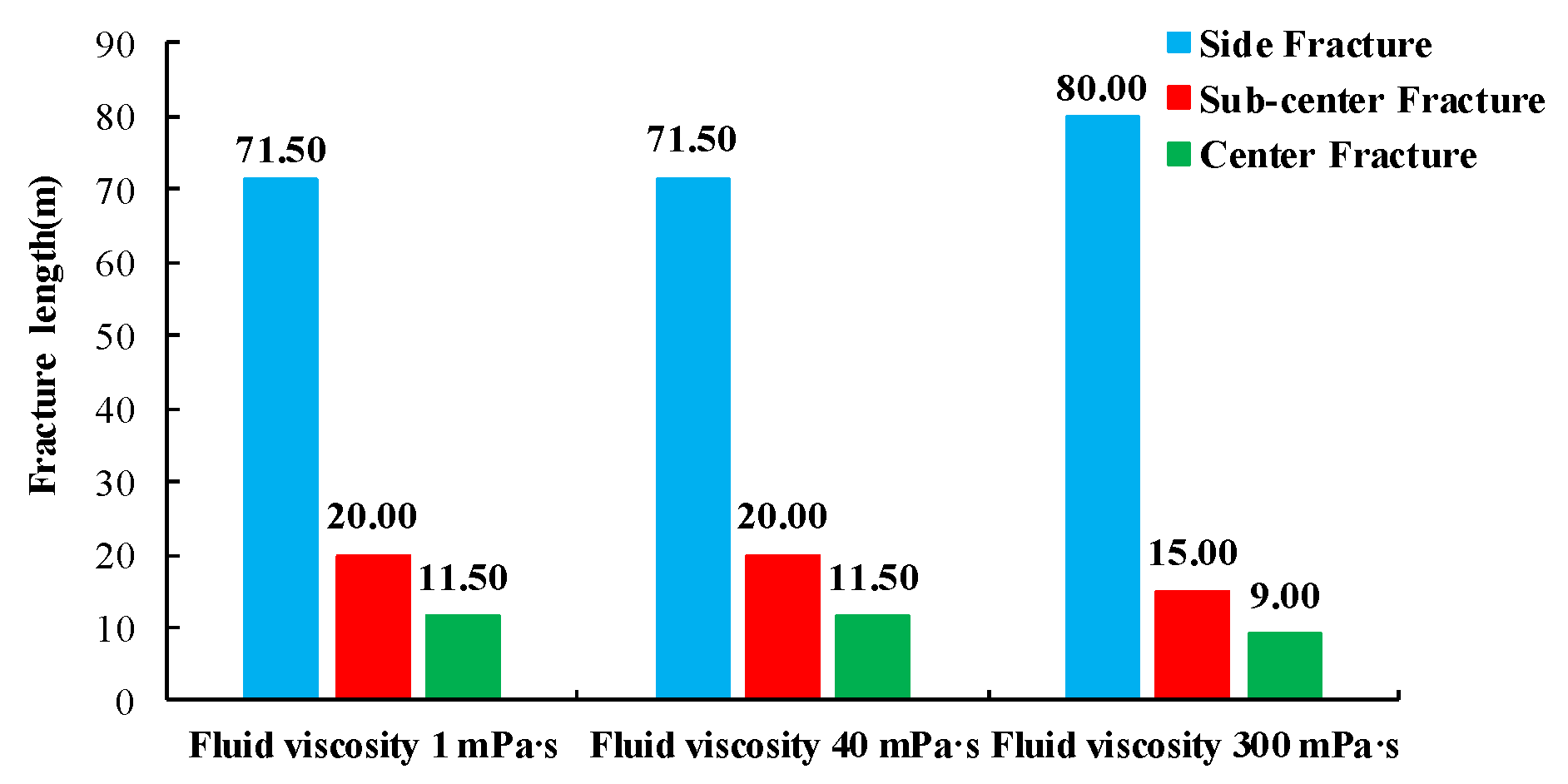


| Unit | Value | |
|---|---|---|
| Maximum horizontal stress | MPa | 98 |
| Minimum horizontal stress | MPa | 90 |
| Poisson’s modulus | - | 0.14 |
| Pore pressure | MPa | 38 |
| Young’s modulus | GPa | 23 |
| Reservoir permeability | mD | 1.4 |
| Reservoir porosity | - | 0.071 |
| Tensile strength | MPa | 12 |
| Case | Cluster Spacing (m) | In Situ Stress Difference (MPa) | Fracture Fluid Viscosity (mPa·s) | Injection Rate (m3/min) |
|---|---|---|---|---|
| Case 1 | 8 | 0 | 40 | 14 |
| Case 2 | 8 | 8 | 40 | 14 |
| Case 3 | 8 | 16 | 40 | 14 |
| Case 4 | 9 | 8 | 40 | 14 |
| Case 5 | 10 | 8 | 40 | 14 |
| Case 6 | 8 | 8 | 1 | 14 |
| Case 7 | 8 | 8 | 300 | 14 |
| Case 8 | 8 | 8 | 40 | 12 |
| Case 9 | 8 | 8 | 40 | 16 |
| Unit | Value | |
|---|---|---|
| Maximum horizontal stress | MPa | 6 |
| Minimum horizontal stress | MPa | 1 |
| Poisson’s modulus | - | 0.23 |
| Young’s modulus | GPa | 8.402 |
| Reservoir permeability | mD | 0.1 |
| Reservoir porosity | % | 1.85 |
| Tensile strength | MPa | 2.59 |
| Injection rate | m3/s | 2.1 × 10−9 |
| Fracturing fluid viscosity | mPa·s | 73 |
| The orientation between perforation and the maximum horizontal principal stress | ° | 60 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ping, E.; Zhao, P.; Zhu, H.; Wang, Y.; Jiao, Z.; Zhao, Q.; Feng, G. Numerical Simulation of the Simultaneous Development of Multiple Fractures in Horizontal Wells Based on the Extended Finite Element Method. Energies 2024, 17, 1057. https://doi.org/10.3390/en17051057
Ping E, Zhao P, Zhu H, Wang Y, Jiao Z, Zhao Q, Feng G. Numerical Simulation of the Simultaneous Development of Multiple Fractures in Horizontal Wells Based on the Extended Finite Element Method. Energies. 2024; 17(5):1057. https://doi.org/10.3390/en17051057
Chicago/Turabian StylePing, Enshun, Peng Zhao, Haiyan Zhu, Yuzhong Wang, Zixi Jiao, Qingjie Zhao, and Gan Feng. 2024. "Numerical Simulation of the Simultaneous Development of Multiple Fractures in Horizontal Wells Based on the Extended Finite Element Method" Energies 17, no. 5: 1057. https://doi.org/10.3390/en17051057
APA StylePing, E., Zhao, P., Zhu, H., Wang, Y., Jiao, Z., Zhao, Q., & Feng, G. (2024). Numerical Simulation of the Simultaneous Development of Multiple Fractures in Horizontal Wells Based on the Extended Finite Element Method. Energies, 17(5), 1057. https://doi.org/10.3390/en17051057








