Small-Signal Stability Analysis and Optimization of Grid-Forming Permanent-Magnet Synchronous-Generator Wind Turbines
Abstract
1. Introduction
2. Impedance Modeling
2.1. System Structure
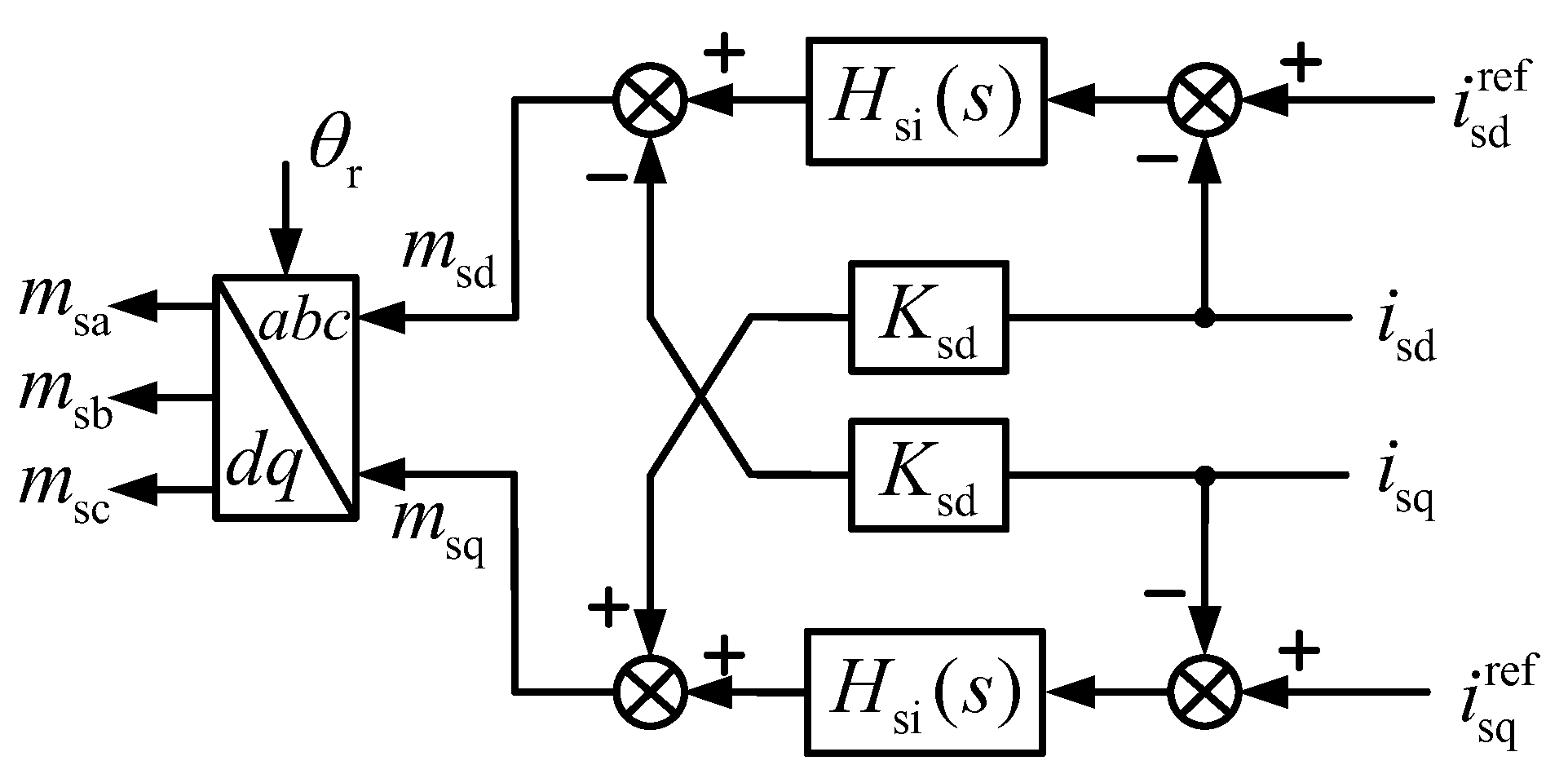
2.2. GSC Modeling
2.3. MSC Modeling
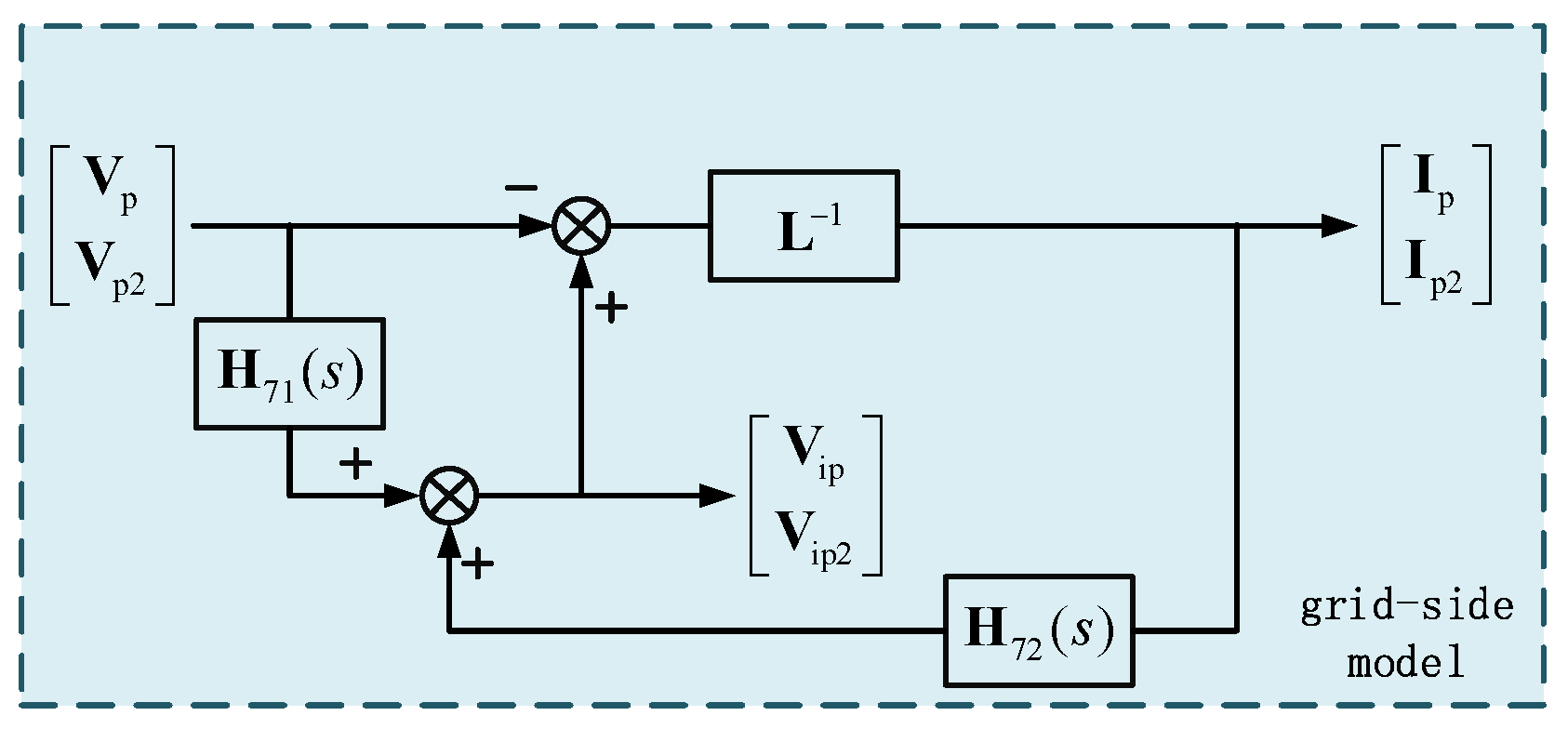
2.4. Overall Model
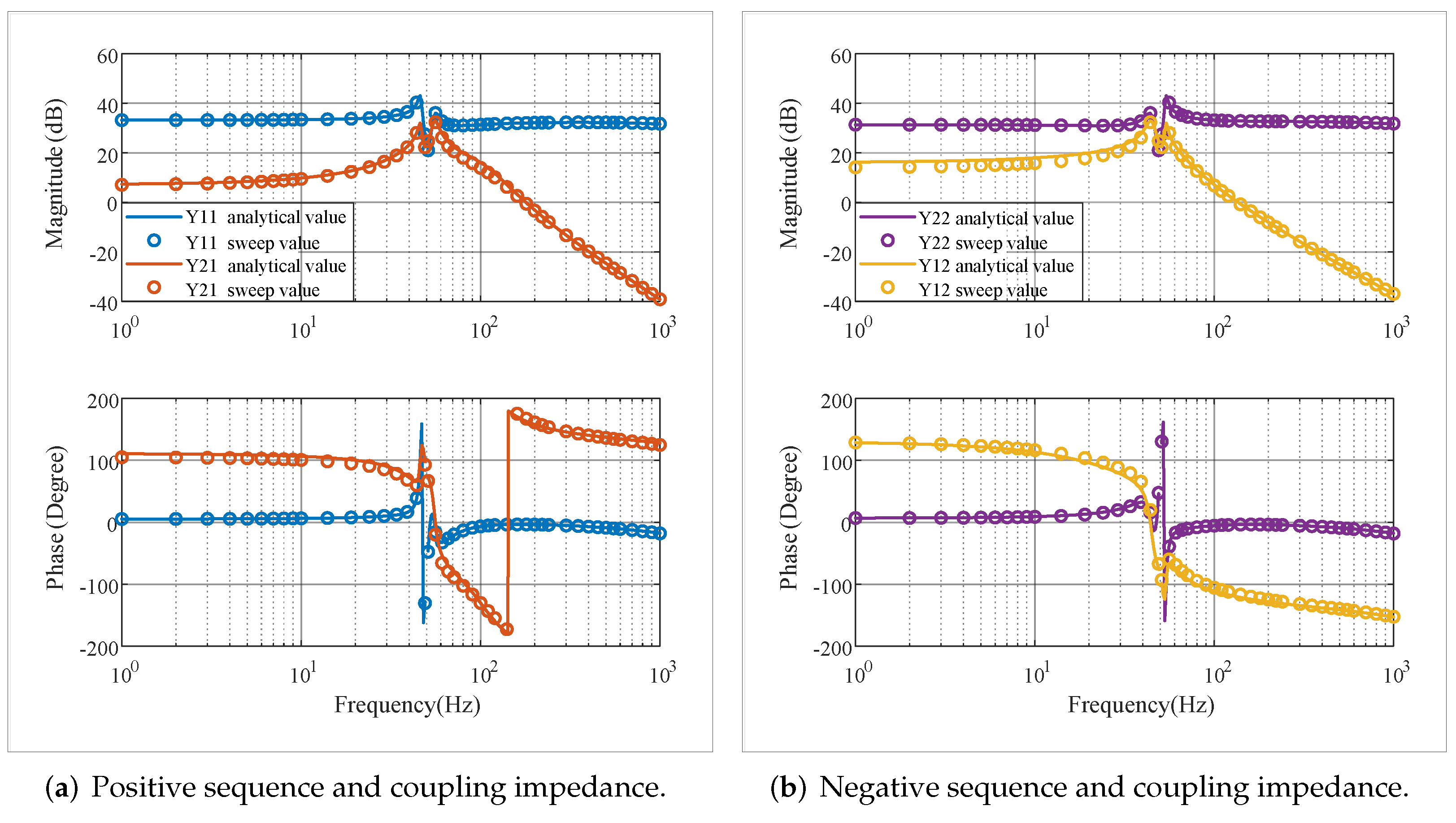
2.5. Model Verification
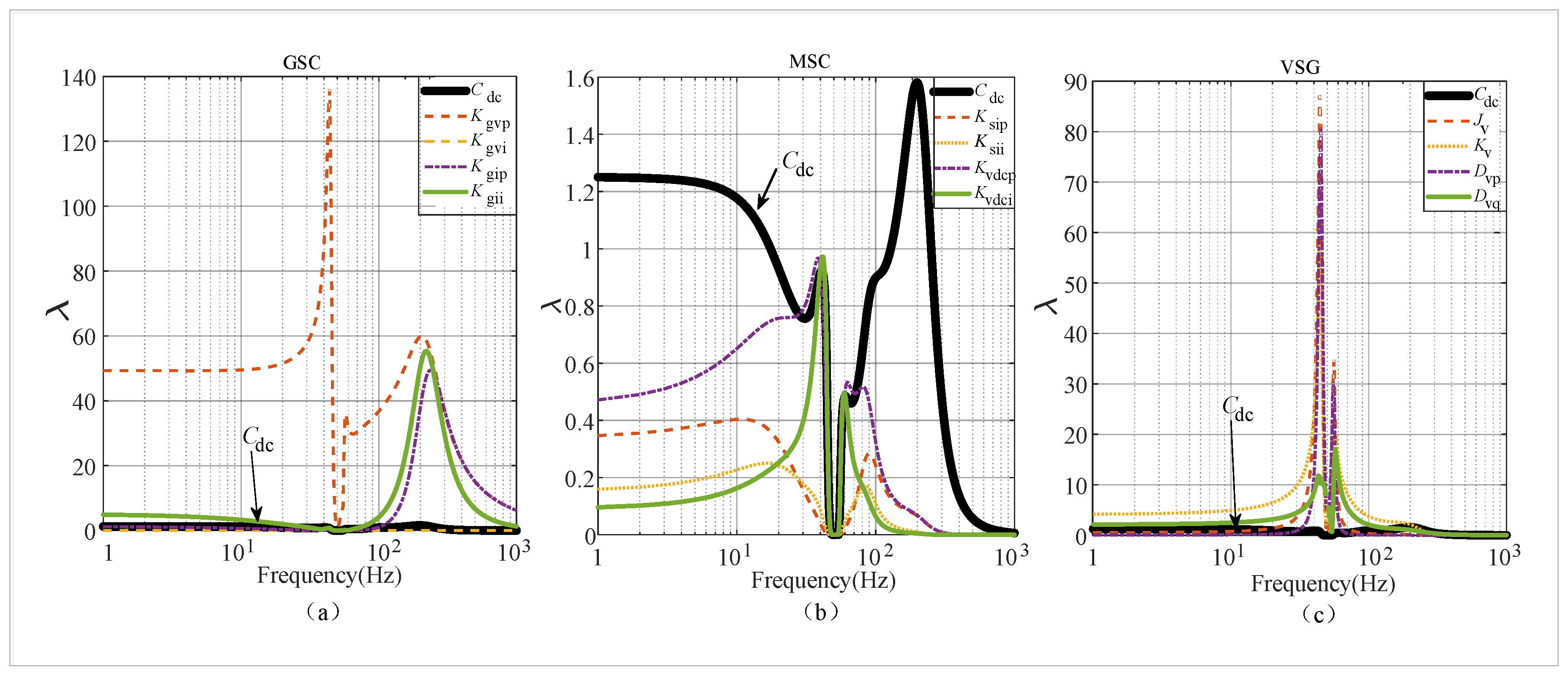
3. Impedance Characteristic Analysis
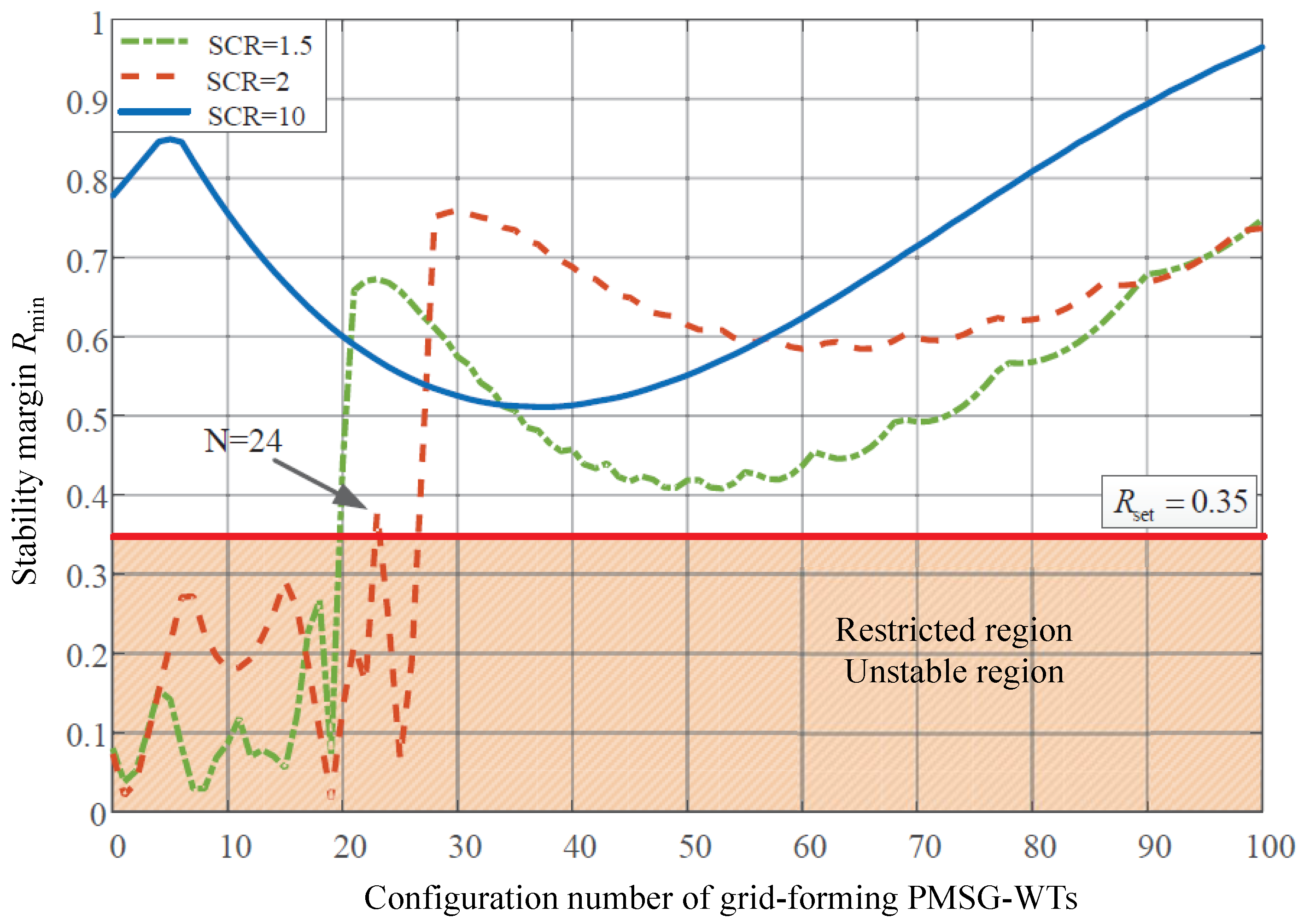
4. Hybrid Wind Farm Oscillation Suppression Strategy
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature:
| virtual inertia | |
| active damping coefficient | |
| reactive inertial coefficient | |
| reactive damping coefficient | |
| output angle of the active power control loop | |
| , | voltage outer loop references |
| AC voltage outer loop | |
| AC current inner loop | |
| current decoupling coefficients conditioner | |
| voltage decoupling coefficients conditioner | |
| rotating angle | |
| rotating angle frequency | |
| PI controller for the MSC’s DC voltage loop | |
| PI controller for the MSC’s current inner loop | |
| current decoupling coefficient for the MSC | |
| stability margin |
Appendix A
References
- Wang, W.; Li, G.; Guo, J. Large-scale renewable energy transmission by HVDC: Challenges and proposals. Engineering 2022, 19, 252–267. [Google Scholar] [CrossRef]
- Cheng, Y.; Fan, L.; Rose, J.; Huang, S.H.; Schmall, J.; Wang, X.; Xie, X.; Shair, J.; Ramamurthy, J.R.; Modi, N.; et al. Real-world subsynchronous oscillation events in power grids with high penetrations of inverter-based resources. IEEE Trans. Power Syst. 2022, 38, 316–330. [Google Scholar] [CrossRef]
- Fan, L.; Miao, Z.; Shah, S.; Cheng, Y.; Rose, J.; Huang, S.H.; Pal, B.; Xie, X.; Modi, N.; Wang, S.; et al. Real-World 20-Hz IBR Subsynchronous Oscillations: Signatures and Mechanism Analysis. IEEE Trans. Energy Convers. 2022, 37, 2863–2873. [Google Scholar] [CrossRef]
- Liu, B.; Zhang, P.; Lu, G.; Chen, X. Influence Analysis of Oscillation Harmonics in LCC-HVDC Delivery System Based on Impedance Modeling. IEEE Trans. Circuits Syst. I-Regul. Pap. 2023, 70, 4194–4203. [Google Scholar] [CrossRef]
- Wang, X.; Taul, M.G.; Wu, H.; Liao, Y.; Blaabjerg, F.; Harnefors, L. Grid-synchronization stability of converter-based resources—An overview. IEEE Open J. Ind. Appl. 2020, 1, 115–134. [Google Scholar] [CrossRef]
- Zhang, H.; Xiang, W.; Lin, W.; Wen, J. Grid forming converters in renewable energy sources dominated power grid: Control strategy, stability, application, and challenges. J. Mod. Power Syst. Clean Energy 2021, 9, 1239–1256. [Google Scholar] [CrossRef]
- Beck, H.P.; Hesse, R. Virtual synchronous machine. In Proceedings of the 2007 9th International Conference on Electrical Power Quality and Utilisation, Barcelona, Spain, 9–11 October 2007; IEEE: Piscataway, NJ, USA, 2007; pp. 1–6. [Google Scholar]
- Zhong, Q.C.; Weiss, G. Synchronverters: Inverters that mimic synchronous generators. IEEE Trans. Ind. Electron. 2010, 58, 1259–1267. [Google Scholar] [CrossRef]
- Rodriguez, P.; Citro, C.; Candela, J.I.; Rocabert, J.; Luna, A. Flexible grid connection and islanding of SPC-based PV power converters. IEEE Trans. Ind. Appl. 2018, 54, 2690–2702. [Google Scholar] [CrossRef]
- Zhang, L.; Harnefors, L.; Nee, H.P. Power-synchronization control of grid-connected voltage-source converters. IEEE Trans. Power Syst. 2009, 25, 809–820. [Google Scholar] [CrossRef]
- Rosso, R.; Wang, X.; Liserre, M.; Lu, X.; Engelken, S. Grid-forming converters: Control approaches, grid-synchronization, and future trends—A review. IEEE Open J. Ind. Appl. 2021, 2, 93–109. [Google Scholar] [CrossRef]
- Guo, J.; Chen, Y.; Wang, L.; Wu, W.; Wang, X.; Shuai, Z.; Guerrero, J.M. Impedance Analysis and Stabilization of Virtual Synchronous Generators with Different DC-Link Voltage Controllers Under Weak Grid. IEEE Trans. Power Electron. 2021, 36, 11397–11408. [Google Scholar] [CrossRef]
- Chen, J.; O’Donnell, T. Parameter Constraints for Virtual Synchronous Generator Considering Stability. IEEE Trans. Power Syst. 2019, 34, 2479–2481. [Google Scholar] [CrossRef]
- Xu, Y.; Nian, H.; Kang, J.; Zhao, J.; Wang, Z.; Zhou, J. Impedance-based Analysis of Potential Stability Risk Between Grid-Forming and Grid-Following Wind Turbine Systems. In Proceedings of the 2021 6th Asia Conference on Power and Electrical Engineering (ACPEE), Chongqing, China, 8–11 April 2021; pp. 858–862. [Google Scholar] [CrossRef]
- Wu, W.; Zhou, L.; Chen, Y.; Luo, A.; Dong, Y.; Zhou, X.; Xu, Q.; Yang, L.; Guerrero, J.M. Sequence-Impedance-Based Stability Comparison Between VSGs and Traditional Grid-Connected Inverters. IEEE Trans. Power Electron. 2019, 34, 46–52. [Google Scholar] [CrossRef]
- Wang, H.; Xie, Z.; Chen, Y.; Wu, W.; Wang, Z.; Lin, G.; Guo, J. Admittance-Based Stability Analysis of Current-Controlled VSG Considering the Frequency Coupling Characteristics. IEEE J. Emerg. Sel. Top. Power Electron. 2023, 11, 1191–1202. [Google Scholar] [CrossRef]
- Li, G.; Chen, Y.; Luo, A.; He, Z.; Wang, H.; Zhu, Z.; Wu, W.; Zhou, L. Analysis and Mitigation of Subsynchronous Resonance in Series-Compensated Grid-Connected System Controlled by a Virtual Synchronous Generator. IEEE Trans. Power Electron. 2020, 35, 11096–11107. [Google Scholar] [CrossRef]
- Wu, W.; Chen, Y.; Zhou, L.; Luo, A.; Zhou, X.; He, Z.; Yang, L.; Xie, Z.; Liu, J.; Zhang, M. Sequence Impedance Modeling and Stability Comparative Analysis of Voltage-Controlled VSGs and Current-Controlled VSGs. IEEE Trans. Ind. Electron. 2019, 66, 6460–6472. [Google Scholar] [CrossRef]
- Wang, G.; Lyu, J.; Rao, Y. Small-Signal Stability Analysis of Hybrid Wind Farm with Grid-Following and Grid-Forming Wind Turbine Generators. In Proceedings of the 2023 IEEE PELS Students and Young Professionals Symposium (SYPS), Shanghai, China, 27–29 August 2023; pp. 1–6. [Google Scholar] [CrossRef]
- Shi, K.; Wang, Y.; Sun, Y.; Xu, P.; Gao, F. Frequency-Coupled Impedance Modeling of Virtual Synchronous Generators. IEEE Trans. Power Syst. 2021, 36, 3692–3700. [Google Scholar] [CrossRef]
- Liu, J.; Xia, Y.; Wei, W.; Feng, Q.; Yang, P. Effect of Control Damping on Small-Signal Stability of Grid-Forming VSCs Considering Interaction Between Inner and Outer Loops. IEEE Trans. Power Electron. 2024, 39, 7685–7695. [Google Scholar] [CrossRef]
- Xu, Y.; Nian, H.; Hu, B.; Sun, D. Impedance Modeling and Stability Analysis of VSG Controlled Type-IV Wind Turbine System. IEEE Trans. Energy Convers. 2021, 36, 3438–3448. [Google Scholar] [CrossRef]
- Shah, C.R.; Molinas, M.; Nilsen, R.; Amin, M. Impedance Reshaping of GFM Converters with Selective Resistive Behaviour for Small-signal Stability Enhancement. In Proceedings of the 2024 IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 25–29 February 2024; pp. 1619–1626. [Google Scholar] [CrossRef]
- Zhou, W.; Mohammed, N.; Bahrani, B. Voltage and Current Dynamics-Canceled Virtual Synchronous Generators for Stability Guarantee. In Proceedings of the 2023 IEEE PES Innovative Smart Grid Technologies—Asia (ISGT Asia), Auckland, New Zealand, 21–24 November 2023; pp. 1–5. [Google Scholar] [CrossRef]
- Yang, Y.; Li, C.; Cheng, L.; Gao, X.; Xu, J.; Blaabjerg, F. A Generic Power Compensation Control for Grid Forming Virtual Synchronous Generator with Damping Correction Loop. IEEE Trans. Ind. Electron. 2023, 71, 10908–10918. [Google Scholar] [CrossRef]
- Shah, C.R.; Molinas, M.; Nilsen, R.; Amin, M. Limitations in Impedance Reshaping of Grid Forming Converters for Instability Prevention. In Proceedings of the 2023 IEEE International Future Energy Electronics Conference (IFEEC), Sydney, Australia, 20–23 November 2023; pp. 526–531. [Google Scholar] [CrossRef]
- Yang, X.; Bao, Y.; Meng, Z.; Xu, C.; Wang, Y.; Xue, H. Restraining Method of Subsynchronous Oscillation in Virtual Synchronous Generator Based on Impedance Reshaping. In Proceedings of the CSEE, Lisbon, Portugal, 29–31 March 2023; pp. 1–13. [Google Scholar]
- Wang, W.; Shi, X.; Wu, G.; Cao, Y. Interaction Between Grid-Forming Converters with AC Grids and Damping Improvement Based on Loop Shaping. IEEE Trans. Power Syst. 2024, 39, 1905–1917. [Google Scholar] [CrossRef]
- Qoria, T.; Gruson, F.; Colas, F.; Kestelyn, X.; Guillaud, X. Analysis of the coupling between the outer and inner control loops of a Grid-forming Voltage Source Converter. In Proceedings of the 2020 22nd European Conference on Power Electronics and Applications (EPE’20 ECCE Europe), Lyon, France, 7–11 September 2020; pp. 1–10. [Google Scholar] [CrossRef]
- Jiang, K.; Liu, D.; Hu, P.; Kan, C. Configuration Analysis of Grid-forming Converter Under 100% Renewable Electricity Systems. In Proceedings of the 2023 26th International Conference on Electrical Machines and Systems (ICEMS), Zhuhai, China, 5–8 November 2023; pp. 1272–1276. [Google Scholar] [CrossRef]
- Liu, X.; Zou, Z.; Tang, J.; Wang, Z.; Cheng, M. Modeling and Stability Analysis of Converter-based Power Systems. In Proceedings of the 2021 IEEE Sustainable Power and Energy Conference (iSPEC), Nanjing, China, 23–25 December 2021; pp. 3134–3142. [Google Scholar] [CrossRef]
- Hu, Y.; Tian, Z.; Zha, X.; Sun, J.; Li, Y.; Wan, Z. Impedance Stability Analysis and Promotion Strategy of Islanded Microgrid Dominated by Grid-connected and Grid-following Converters. Autom. Electr. Power Syst. 2022, 46, 121–131. [Google Scholar]
- Liu, P.; Xie, X.; Shair, J. Adaptive Hybrid Grid-Forming and Grid-Following Control of IBRs with Enhanced Small-Signal Stability Under Varying SCRs. IEEE Trans. Power Electron. 2024, 39, 6603–6607. [Google Scholar] [CrossRef]
- Liu, B.; Li, Z.; Dong, X.; Yu, S.S.; Chen, X.; Oo, A.M.T.; Lian, X.; Shan, Z.; Liu, X. Impedance Modeling and Controllers Shaping Effect Analysis of PMSG Wind Turbines. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 1465–1478. [Google Scholar] [CrossRef]




| Parameters | Symbol | Parameter Name | Value |
|---|---|---|---|
| PMSG | Stator current frequency | 80 Hz | |
| Stator winding resistance | 0.01 W | ||
| Permanent-magnet flux linkage | 1.1 Wb | ||
| Direct axis reactance | H | ||
| Quadrature axis reactance | H | ||
| p | Pairs of poles | 3 | |
| DC bus | DC bus voltage | 1150 V | |
| DC bus capacitor | 3.6 mF | ||
| Filter circuit | Filter inductor | 0.0075 mH | |
| Filter capacitor | 0.6 mF |
| Parameters | Symbol | Parameter Name | Value |
|---|---|---|---|
| VSG | Virtual moment of inertia | 85.5 | |
| Active damping coefficient | 600 | ||
| Reactive inertia coefficient | 2400 | ||
| Reactive damping coefficient | 45,000 | ||
| GSC | Voltage loop PI controller | 40 + 1/s | |
| Voltage decoupling coefficient | 0.1885 | ||
| Current loop PI controller | 0.002 + 1/s | ||
| Current decoupling coefficient | 4.1888 | ||
| Phase voltage amplitude | 563 kV | ||
| Rated power | 1.5 MW | ||
| MSC | DC voltage PI controller | 12 + 240/s | |
| Voltage loop PI controller | 0.002 + 1/s | ||
| Decoupling coefficient | 2.4118 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, G.; Han, R.; Liu, B.; Li, Z. Small-Signal Stability Analysis and Optimization of Grid-Forming Permanent-Magnet Synchronous-Generator Wind Turbines. Energies 2024, 17, 4560. https://doi.org/10.3390/en17184560
Li G, Han R, Liu B, Li Z. Small-Signal Stability Analysis and Optimization of Grid-Forming Permanent-Magnet Synchronous-Generator Wind Turbines. Energies. 2024; 17(18):4560. https://doi.org/10.3390/en17184560
Chicago/Turabian StyleLi, Guanghui, Runqi Han, Bin Liu, and Zhen Li. 2024. "Small-Signal Stability Analysis and Optimization of Grid-Forming Permanent-Magnet Synchronous-Generator Wind Turbines" Energies 17, no. 18: 4560. https://doi.org/10.3390/en17184560
APA StyleLi, G., Han, R., Liu, B., & Li, Z. (2024). Small-Signal Stability Analysis and Optimization of Grid-Forming Permanent-Magnet Synchronous-Generator Wind Turbines. Energies, 17(18), 4560. https://doi.org/10.3390/en17184560






