Variation in Flow Characteristics and Power Performance Due to Axial Distance Optimization in the Design of Counter-Rotating Tidal Turbines
Abstract
1. Introduction
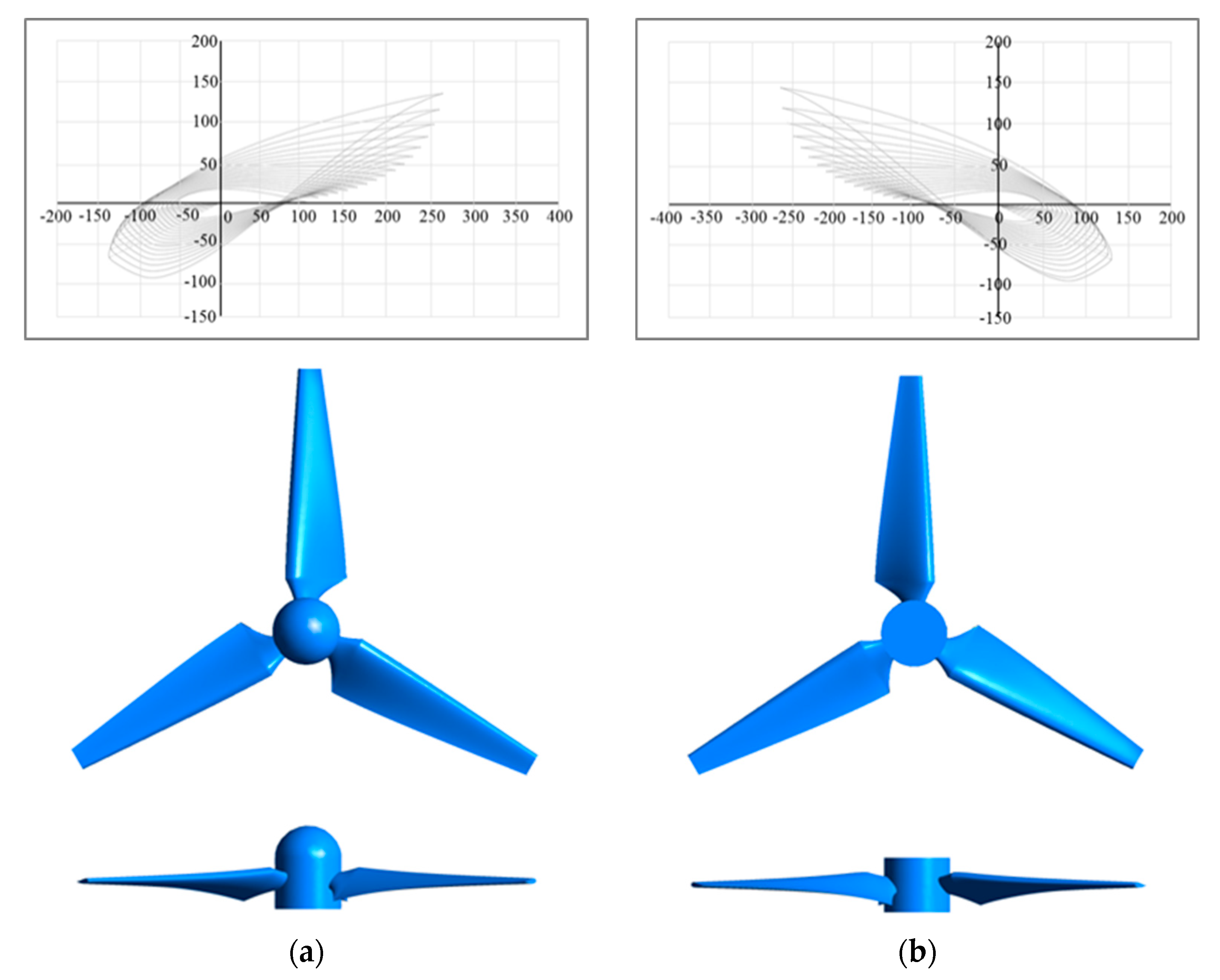
2. Design of Turbine Blade
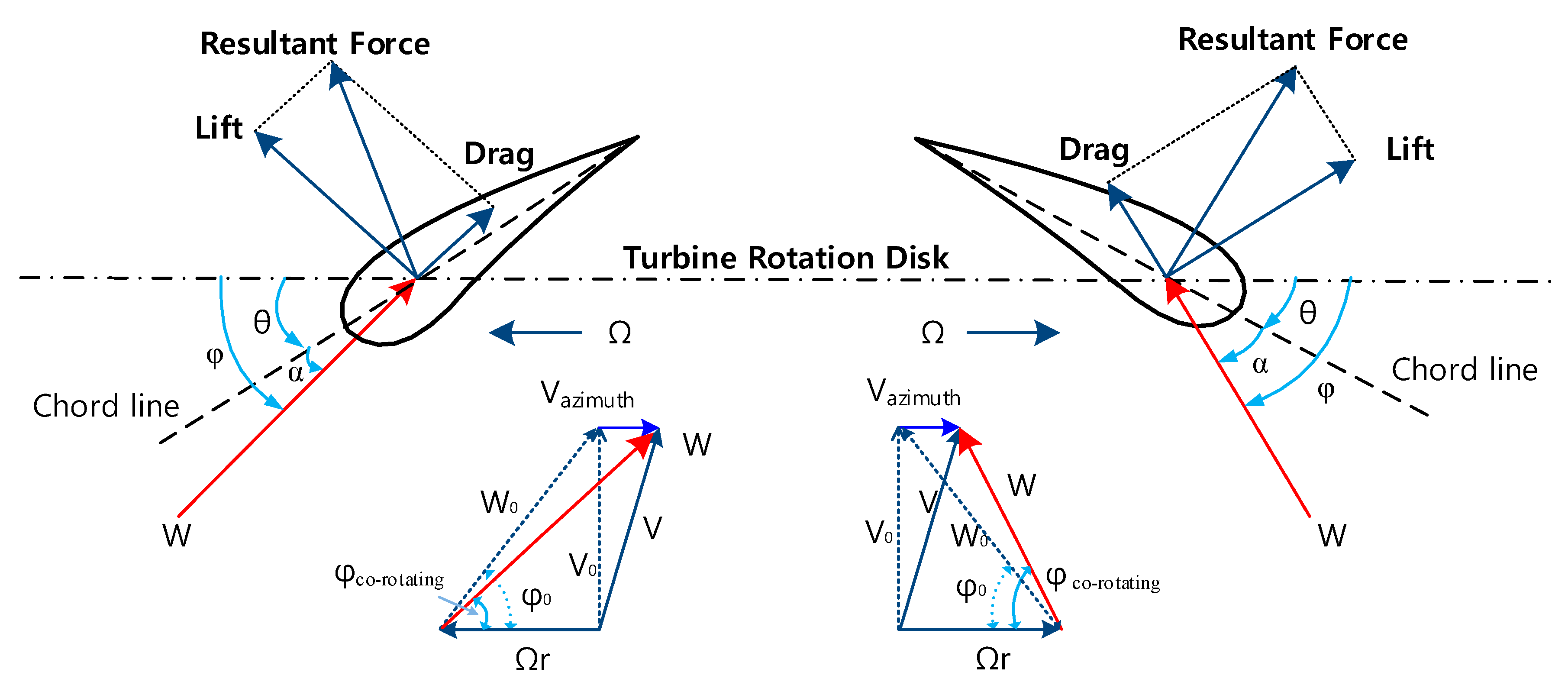
Principle of Design Theory
3. Numerical and Experimental Methods
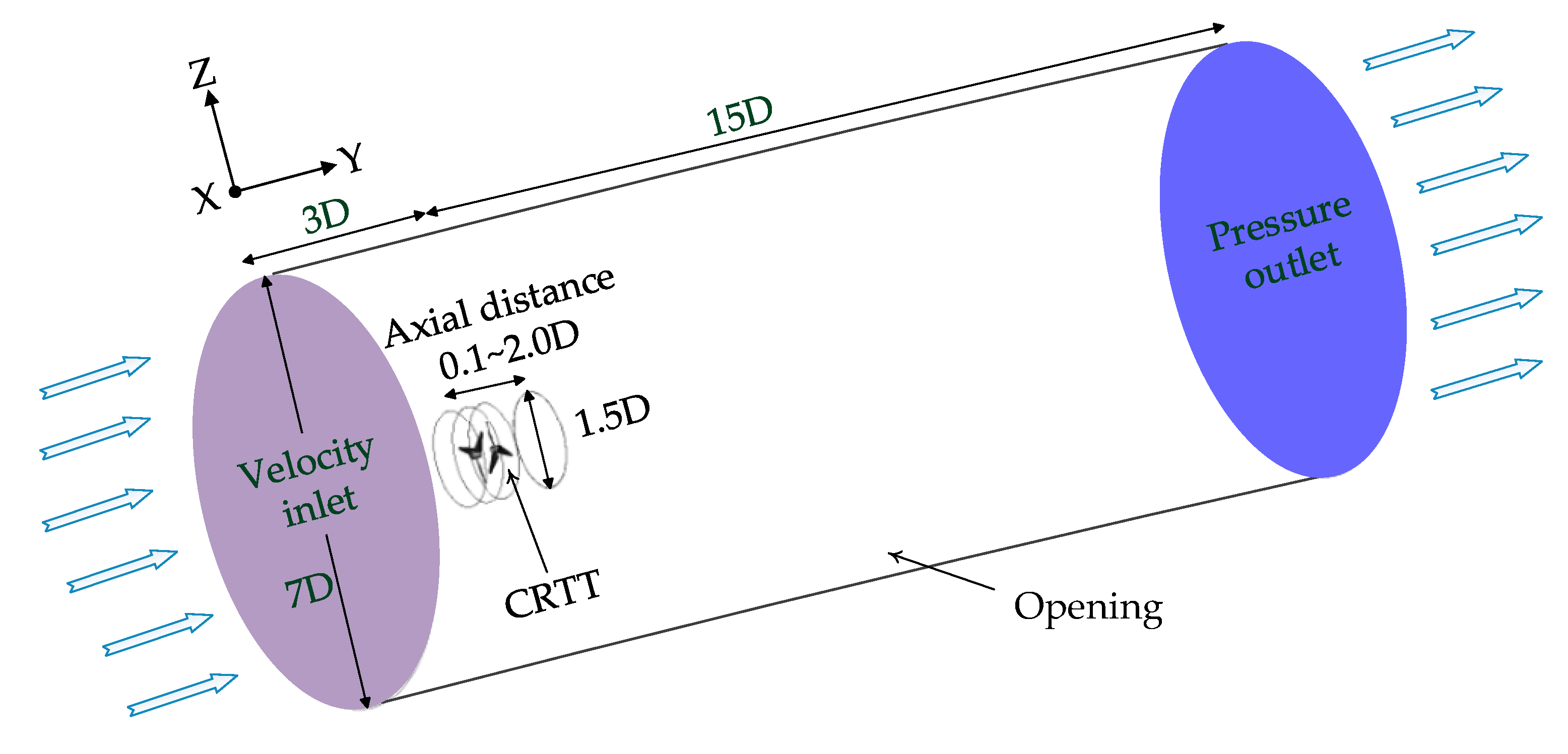
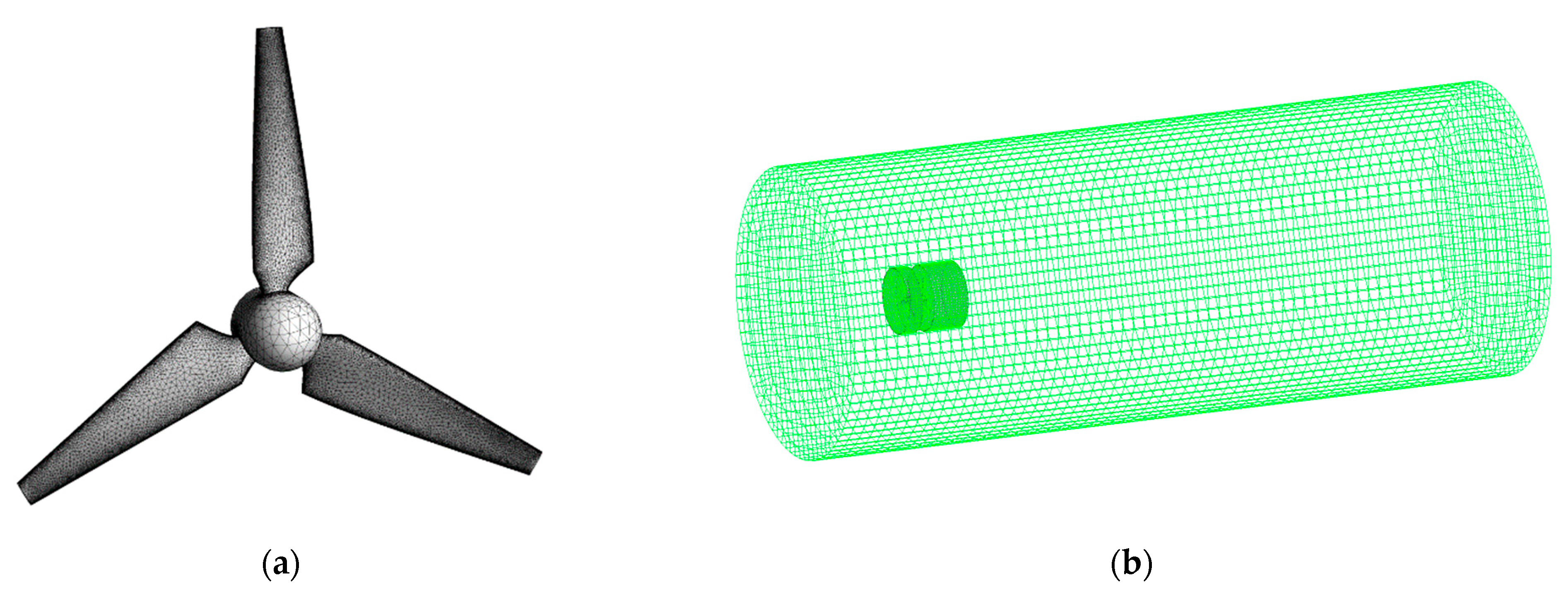
3.1. Numerical Methods
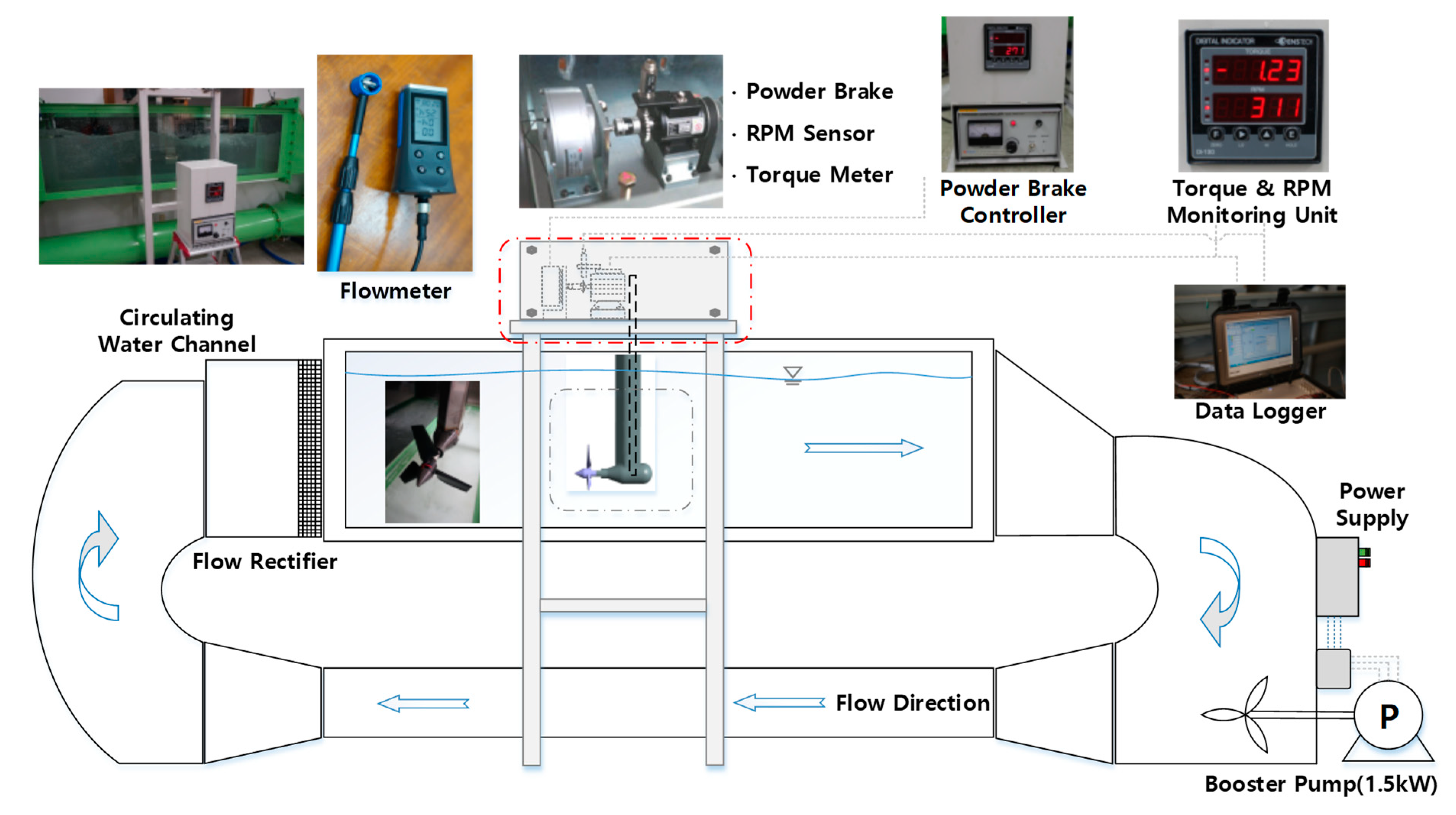
3.2. Experimental Methods
4. Results and Discussion
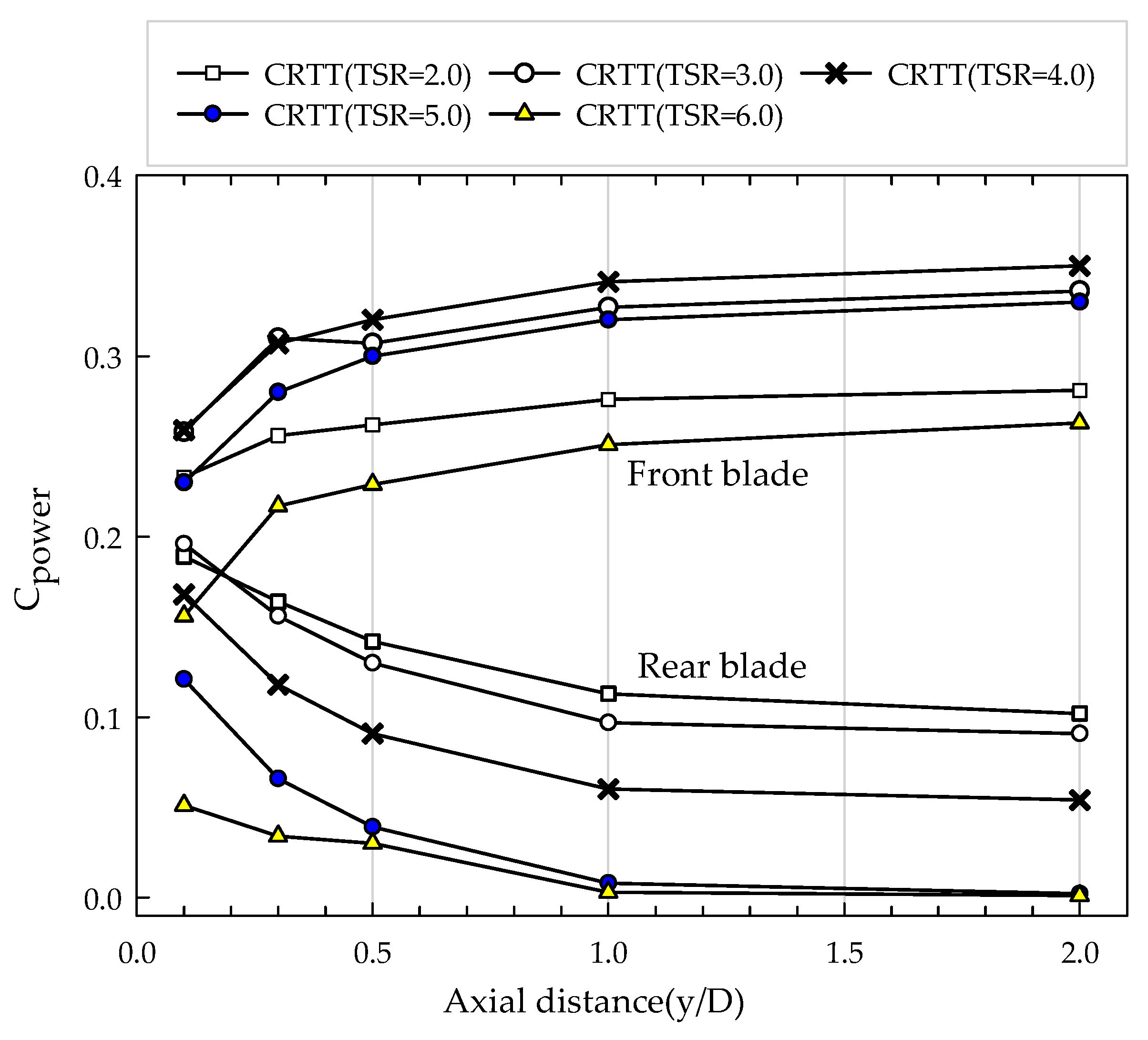
4.1. Evaluation of Power Performance
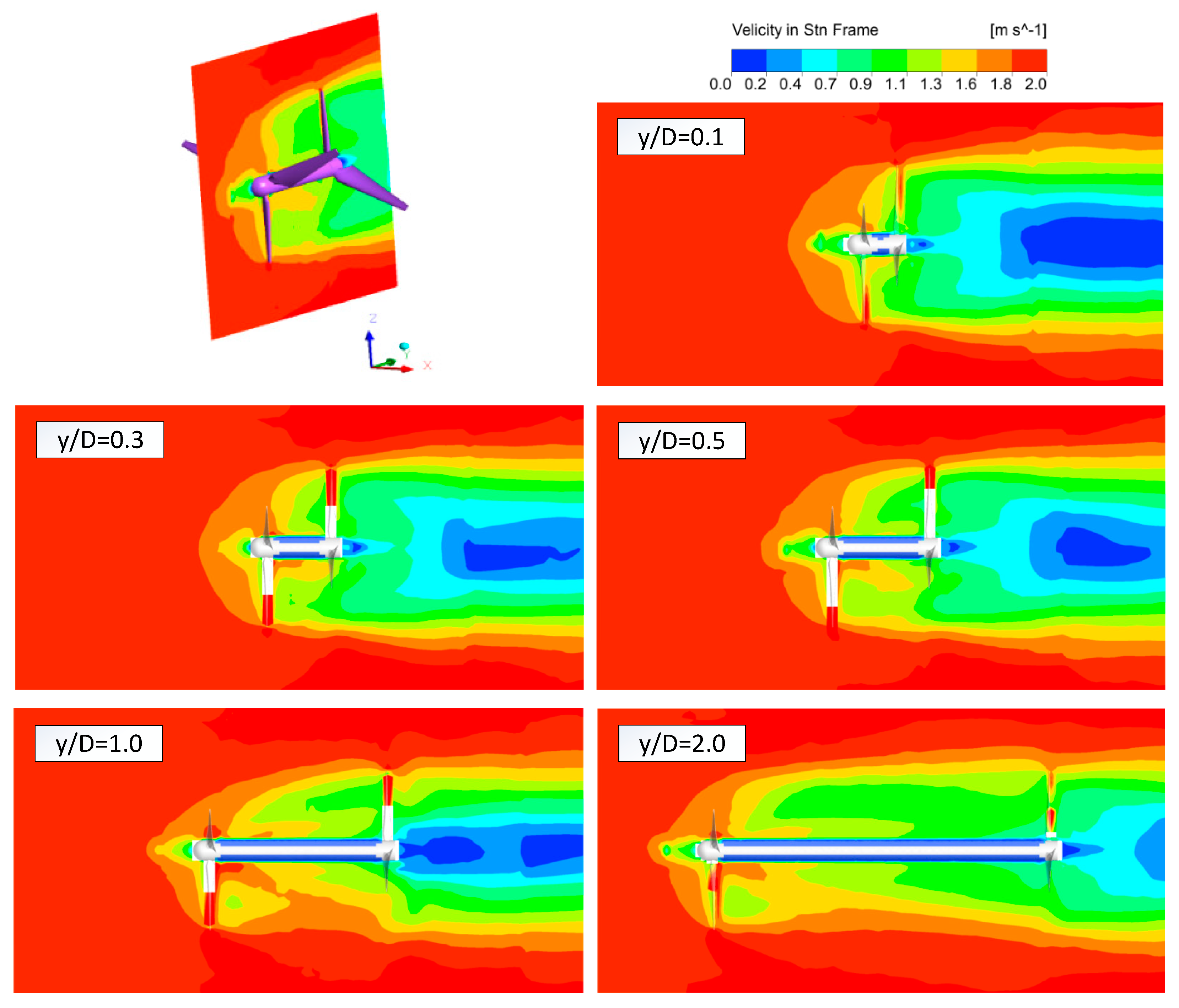
4.2. Velocity Streamline and Flow Separation
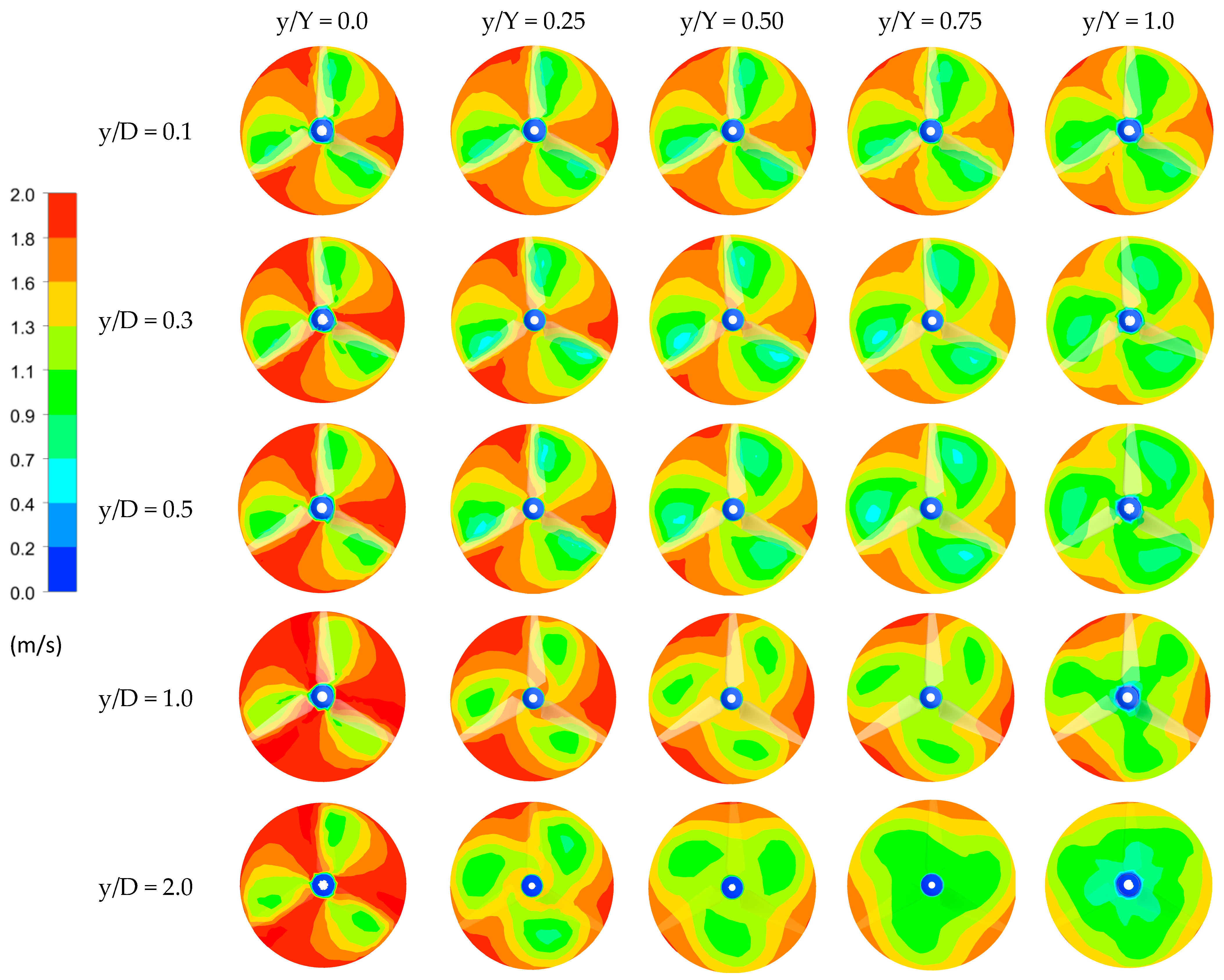
4.3. Flow Velocity Distribution for Counter-Rotating Tidal Turbines
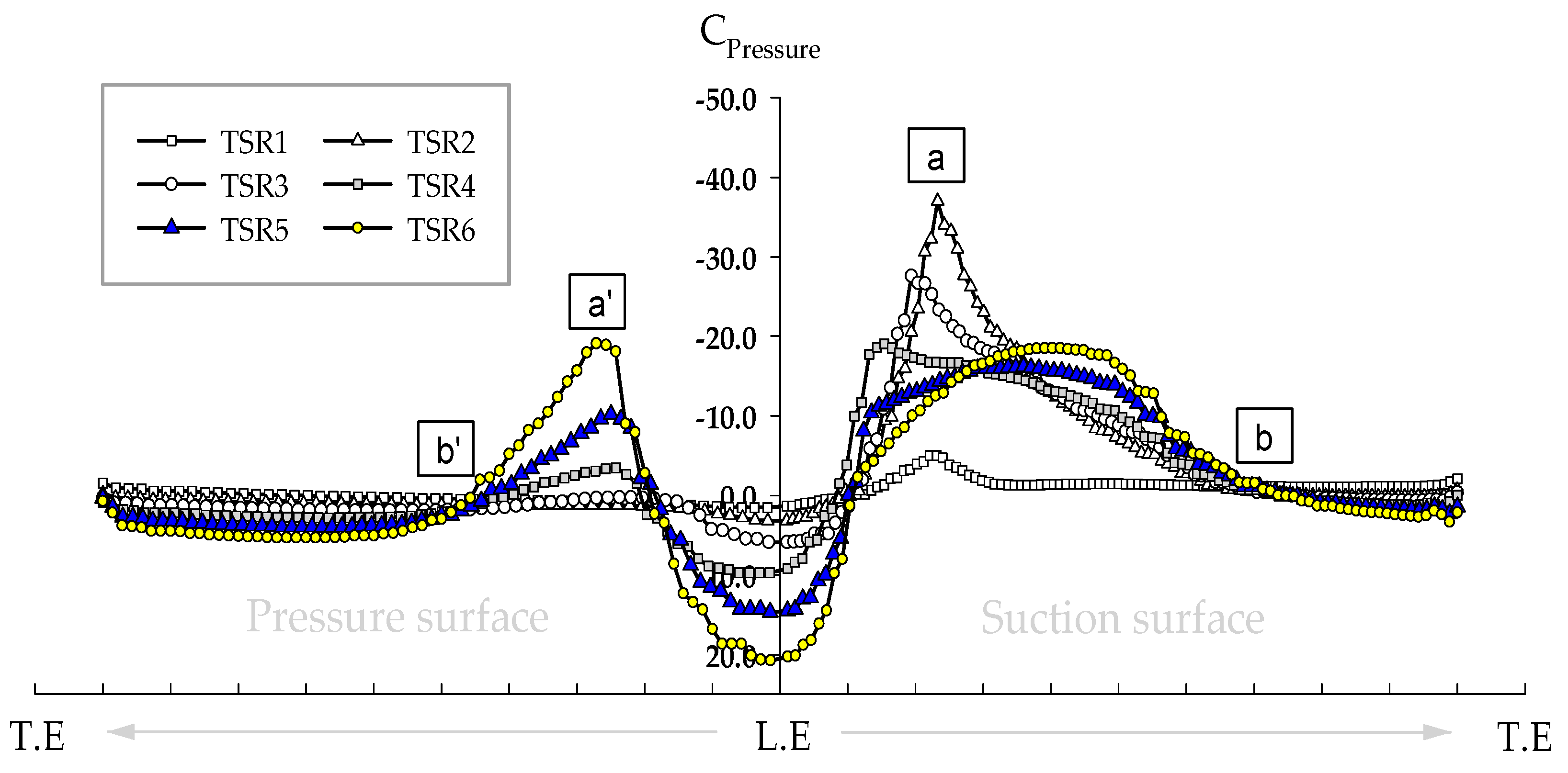
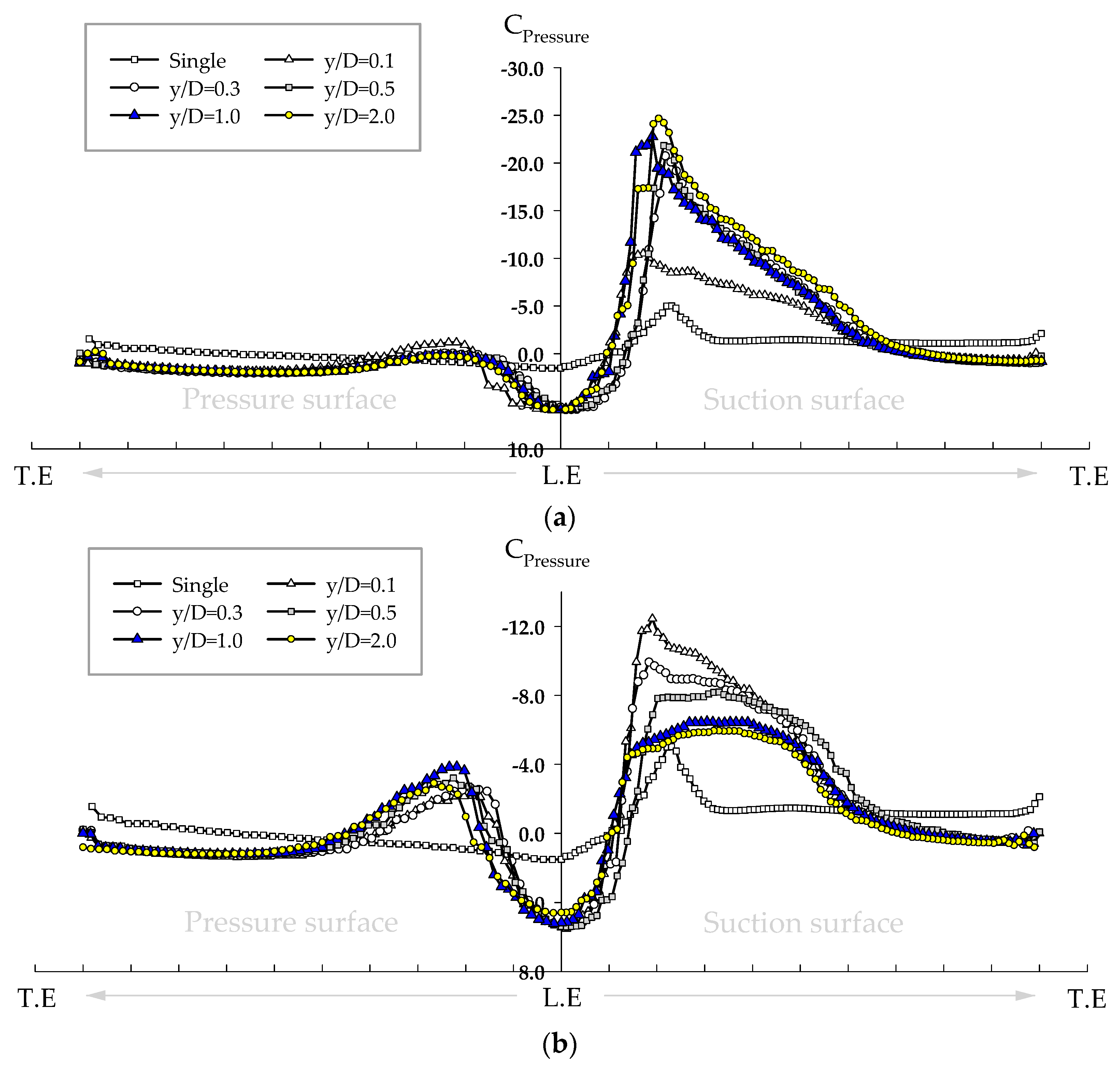
4.4. Pressure Coefficient
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Power coefficient | Maximum efficiency for n actual disks | ||
| Inflow angle | Pitch angle | ||
| Twist angle | BEM | Blade element momentum | |
| TSR | Tip speed ratio | C | Chord length |
| W | Flow velocity | Tip-loss correction factor | |
| r | Radius | D | Diameter |
| Fluid density | Stress tensor | ||
| H | Total enthalpy | Temperature | |
| F | Wall function | GGI | General grid interface |
| SST | Shear stress transport | Radial velocity | |
| T | Torque | A | Turbine area |
| y-plus | CRTT | Counter-rotating tidal turbine | |
| Pressure coefficient | CWC | Circulation water channel | |
| Static pressure | Dynamic pressure |
References
- World Energy Outlook. Overview and Key Findings. Available online: https://www.iea.org/reports/world-energy-outlook-2023/overview-and-key-findings (accessed on 9 April 2024).
- Halawa, E. Sustainable energy: Concept and definition in the context of the energy transition—A critical review. Sustainability 2024, 16, 1523. [Google Scholar] [CrossRef]
- Korea Energy Newspaper. Available online: https://koenergy.co.kr/news/articleView.html?idxno=109209 (accessed on 9 April 2024).
- Korea Environment Corporation. Estimation of National GHG Emission in Waste Sector. Available online: https://www.keco.or.kr/en/lay1/S295T321C331/contents.do (accessed on 9 April 2024).
- 2050 Carbon Neutrality of the Republic of Korea. Available online: https://www.2050cnc.go.kr/base/board/read?boardManagementNo=3&boardNo=347&searchCategory=&page=3&searchType=title&searchWord=%EA%B8%B0%ED%9B%84&menuLevel=3&menuNo=9 (accessed on 9 April 2024).
- Melikoglu, M. Current status and future of ocean energy sources: A global review. Ocean Eng. 2018, 148, 563–573. [Google Scholar] [CrossRef]
- Jo, C.H.; Yim, J.Y.; Lee, K.H.; Rho, Y.H. Performance of horizontal axis tidal current turbine by blade configuration. Renew. Energy 2012, 42, 195–206. [Google Scholar] [CrossRef]
- Jahromi, M.; Maswood, A.; Tseng, K. Long Term Prediction of Tidal Currents. IEEE Syst. J. 2011, 5, 146–155. [Google Scholar] [CrossRef]
- Ko, D.; Chung, J.; Lee, K.; Park, J.; Yi, J. Current policy and technology for tidal current energy in Korea. Energies 2019, 12, 1807. [Google Scholar] [CrossRef]
- Li, Y.; Calışal, S.M. Modeling of twin-turbine systems with vertical axis tidal current turbines: Part I—Power output. Ocean Eng. 2010, 37, 627–637. [Google Scholar] [CrossRef]
- Tatum, S.C.; Frost, C.H.; Allmark, M.; O’Doherty, D.M.; Mason-Jones, A.; Prickett, P.W.; Grosvenor, R.I.; Byrne, C.B.; O’Doherty, T. Wave–current interaction effects on tidal stream turbine performance and loading characteristics. Int. J. Mar. Energy 2016, 14, 161–179. [Google Scholar] [CrossRef]
- Jayaram, V.; Bavanish, B. Design and analysis of gorlov helical hydro turbine on index of revolution. Int. J. Hydrog. Energy 2022, 47, 32804–32821. [Google Scholar] [CrossRef]
- Sitorus, P.E.; Park, J.; Ko, J.H. Hydrodynamic characteristics of cambered NACA0 012 for flexible wing application of a flapping-type tidal stream energy harvesting System. Int. J. Nav. Archit. Ocean Eng. 2019, 14, 100428. [Google Scholar]
- Ocean Tidal and Wave Energy. Available online: https://www.epri.com/research/products/1010489 (accessed on 9 April 2024).
- Jones, I.; Wells, A.K.; Starzmann, R.; Bicshof, S. CFD simulations of the Triton tidal energy platform to analyse the surrounding flow pattern. In Proceedings of the 11th European Wave and Tidal Energy Conference, Nantes, France, 6–11 September 2015. [Google Scholar]
- Clarke, J.A.; Cornor, G.; Grant, A.D.; Johnstone, C.M.; Mackenzie, D. Development of a contra-rotating tidal current turbine and analysis of performance. In Proceedings of the 7th European Wave and Tidal Energy Conference, Porto, Portugal, 11–13 September 2007. [Google Scholar]
- Huang, B.; Shi, J.; Wei, X. Model Testing of a Series of Counter-Rotating Type Horizontal-Axis Tidal Turbines With 500mm Diameter. J. Eng. Gas Turbines Power 2017, 139, 102602. [Google Scholar] [CrossRef]
- Lee, S.; Kim, H.; Son, E.; Lee, S. Effects of design parameters on aerodynamic performance of a counter-rotating wind turbine. Renew. Energy 2012, 42, 140–144. [Google Scholar] [CrossRef]
- Newman, B.G. Multiple actuator-disc theory for wind turbines. J. Wind. Eng. Ind. Aerodyn. 1986, 24, 215–225. [Google Scholar] [CrossRef]
- Lee, N.J.; Kim, I.C.; Kim, C.G.; Hyun, B.S.; Lee, Y.H. Performance study on a counter-rotating tidal current turbine by CFD and model experimentation. Renew. Energy 2015, 79, 122–126. [Google Scholar] [CrossRef]
- Kim, B.S. Multi-MW class wind turbine blade design part I: Aero-structure design and integrated load analysis. Trans. Korean Soc. Mech. Eng. 2014, 38, 289–309. [Google Scholar] [CrossRef]
- Kim, B.S.; Kim, M.E.; Lee, Y.H. Basic configuration design and performance prediction of an 1 MW wind turbine blade. KSFM J. Fluid Mach. 2008, 11, 15–21. [Google Scholar] [CrossRef]
- Hoang, A.D.; Yang, C.J. Design and performance evaluation of a 10kW scale counter-rotating wind turbine rotor. J. Korean Soc. Mar. Environ. Saf. 2014, 20, 104–112. [Google Scholar] [CrossRef]
- NREL Airfoil Families for HAWTs. National Renewable Energy Laboratory (NREL). Available online: https://research-hub.nrel.gov/en/publications/nrel-airfoil-families-for-hawts (accessed on 9 June 2024).
- World Energy Resources Marine Energy 2016. World Energy Council. Available online: https://www.worldenergy.org/assets/images/imported/2016/10/World-Energy-Resources-Full-report-2016.10.03.pdf (accessed on 9 June 2024).
- Alberto, B. State-of-the-art of MW-level capacity oceanic current turbines. J. Nonlinear Eng. 2020, 9, 361–369. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. J. Am. Inst. Aeronaut. Astronaut. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Kolekar, N.; Vinod, A.; Banerjee, A. On blockage Effects for a tidal turbine in free surface proximity. Energies 2019, 12, 3325. [Google Scholar] [CrossRef]
- Bahaj, A.S.; Molland, A.F.; Chaplin, J.R.; Batten, W.M.J. Power and thrust measurements of marine current turbines under various hydrodynamic flow conditions in a cavitation tunnel and a towing tank. Renew. Energy 2007, 32, 407–426. [Google Scholar] [CrossRef]
- Schluntz, J.; Willden, R.H.J. The effect of blockage on tidal turbine rotor design and performance. Renew. Energy 2015, 81, 432–441. [Google Scholar] [CrossRef]
- Chen, T.Y.; Liou, L.R. Blockage corrections in wind tunnel tests of small horizontal-axis wind turbines. Exp. Therm. Fluid Sci. 2011, 35, 565–569. [Google Scholar] [CrossRef]
- Hartwanger, D.; Horvat, A. 3D modelling of a wind turbine using CFD. In Proceedings of the NAFEMS UK Conference 2008, Cheltenham, UK, 10–11 June 2008. [Google Scholar]
- Jo, C.H.; Kim, D.Y.; Hwang, S.J.; Goo, C.H. Shape Design of the Duct for Tidal Converters Using Both Numerical and Experimental Approaches (pre-2015). Energies 2016, 9, 185. [Google Scholar] [CrossRef]
- Yang, C.J. Optimal rotor blade design for tidal in-stream energy. J. Korean Soc. Mar. Environ. Saf. 2011, 17, 75–82. [Google Scholar] [CrossRef][Green Version]
- McCarty, M. The Measurement of the Pressure Distribution over the Wing of an Aircraft in Flight. Master’s Thesis, University of New South Wales, Sydney, Australia, 2008. [Google Scholar]





| Description | Boundary Condition | Value |
|---|---|---|
| Analysis solution | Steady state | - |
| Inlet | Stream velocity | 2.0 m/s |
| Outlet | Static pressure | 1.0 atm |
| Rotor blade | Non-slip wall | - |
| Rotational speed | RPM | 13.8–82.7 |
| Interface area | Frozen rotor | General Grid Interface (GGI) |
| Working fluid | Sea water | 1024 kg/m3, 25 °C |
| Turbulence model | Shear Stress Transport (SST) | - |
| Description | Mesh Type | Elements | Nodes | |
|---|---|---|---|---|
| Rotor blade | Tetrahedral | 176,240 | 99,165 | ~1.3 |
| Rotor field | Tetrahedral | 7,052,100 | 2,084,316 | ~12.9 |
| Domain field | Hexahedral | 1,772,131 | 507,413 | ~717.0 |
| Total | - | 9,000,471 | 2,690,894 | - |
| Number | Elements | Nodes | Power (W) | |
|---|---|---|---|---|
| 1 | 9,000,471 | 2,690,894 | 4.781 | 0.381 |
| 2 | 11,582,225 | 3,679,524 | 5.057 | 0.403 |
| 3 | 15,126,006 | 5,215,864 | 4.996 | 0.398 |
| 4 | 21,341,106 | 7,823,468 | 5.001 | 0.392 |
| 5 | 26,198,652 | 9,711,143 | 5.010 | 0.399 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jeong, H.; Yang, C. Variation in Flow Characteristics and Power Performance Due to Axial Distance Optimization in the Design of Counter-Rotating Tidal Turbines. Energies 2024, 17, 3207. https://doi.org/10.3390/en17133207
Jeong H, Yang C. Variation in Flow Characteristics and Power Performance Due to Axial Distance Optimization in the Design of Counter-Rotating Tidal Turbines. Energies. 2024; 17(13):3207. https://doi.org/10.3390/en17133207
Chicago/Turabian StyleJeong, Haechang, and Changjo Yang. 2024. "Variation in Flow Characteristics and Power Performance Due to Axial Distance Optimization in the Design of Counter-Rotating Tidal Turbines" Energies 17, no. 13: 3207. https://doi.org/10.3390/en17133207
APA StyleJeong, H., & Yang, C. (2024). Variation in Flow Characteristics and Power Performance Due to Axial Distance Optimization in the Design of Counter-Rotating Tidal Turbines. Energies, 17(13), 3207. https://doi.org/10.3390/en17133207





