Cooling Techniques in Direct-Drive Generators for Wind Power Application
Abstract
:1. Introduction
- It can provide a decentralized energy supply to remote places where the electrical grid does not reach. Alternatively, if the grid is present, the wind turbine can pump electrical green generated energy into it;
- Offshore wind turbines can reuse existing technology from oil and gas industries for deployment and maintenance, thus reducing developing costs;
- Up to 80% of the wind turbines’ mass is recyclable since it is made of steel, iron, copper and aluminum;
- Energy payback [4] is the amount of energy generated during the turbine’s lifetime versus the energy spent during its lifecycle (including manufacturing, operation and disposal). In [5], it is shown that the wind power generation energy payback is 5 to 8 months—an improvement over hydro (9 to 12 months) and solar (1 to 2 years). Other energy generation types such as thermoelectric devices have even lower payback since they are not as efficient or deployed on large scale [6]. Oil, coal, gas and nuclear plants consume more energy than they generate, and therefore they never amortize;
2. Direct-Drive Generator Concepts
2.1. Sizing Constraints
2.2. Thermal Constraints
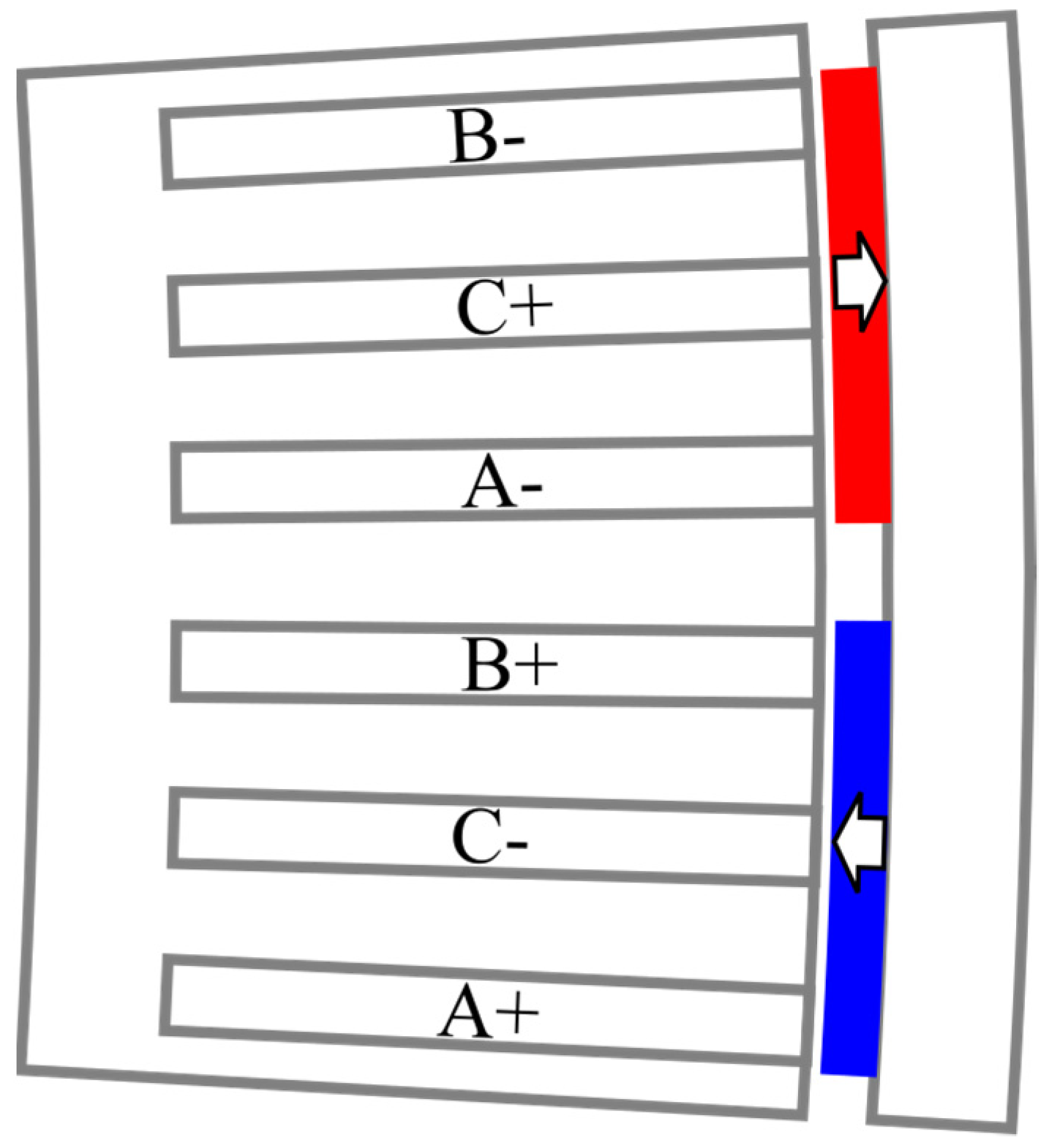
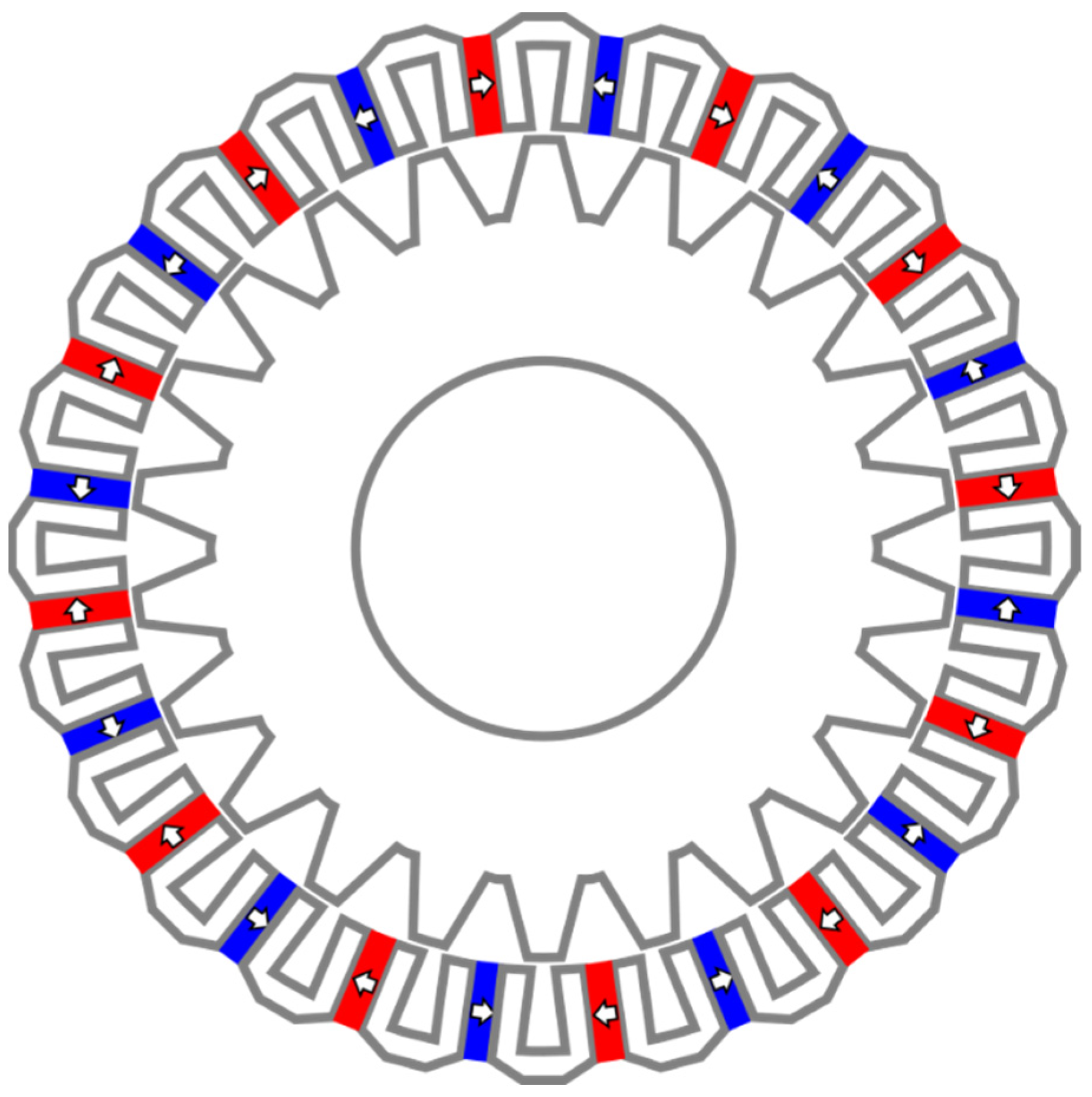
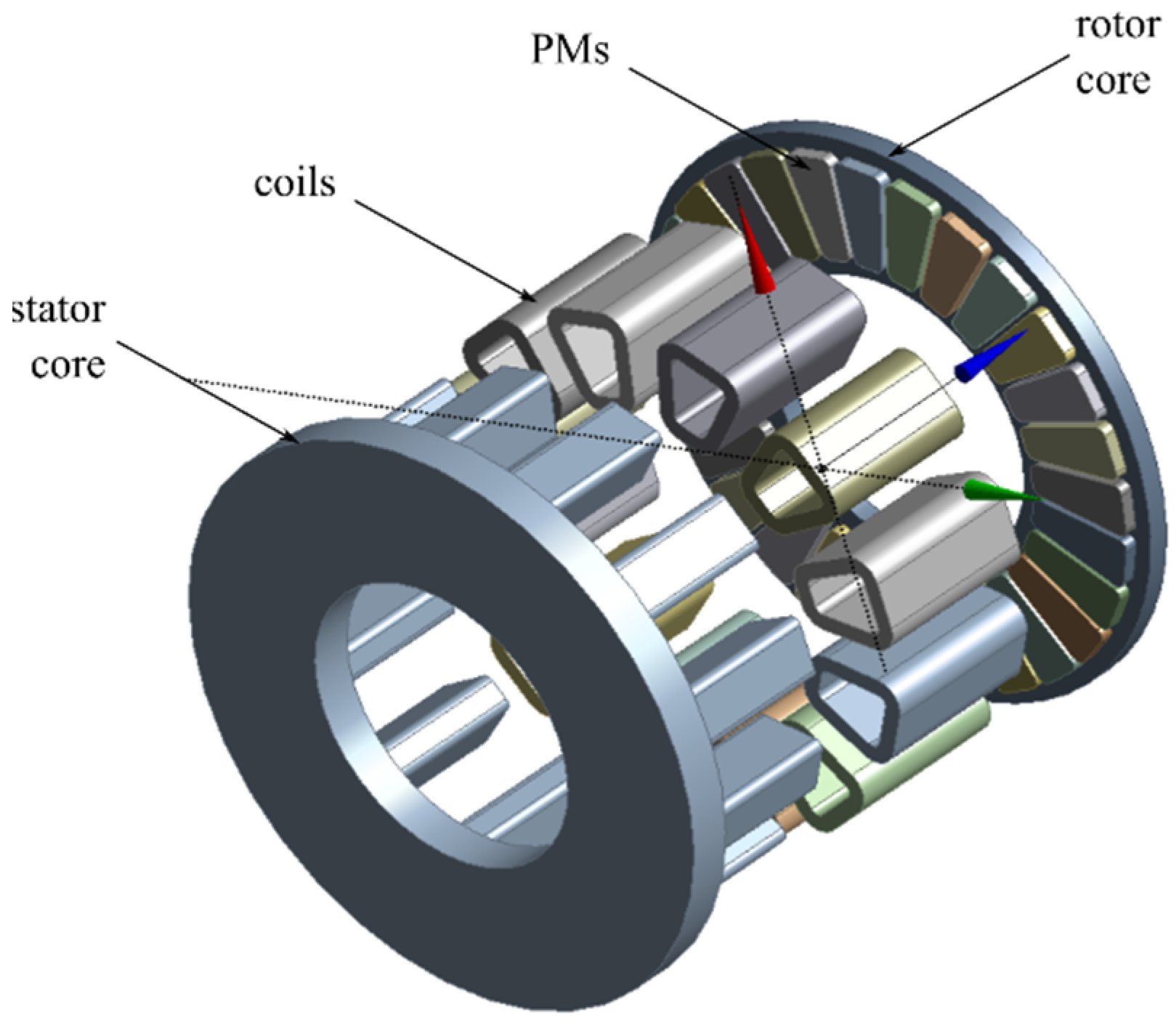
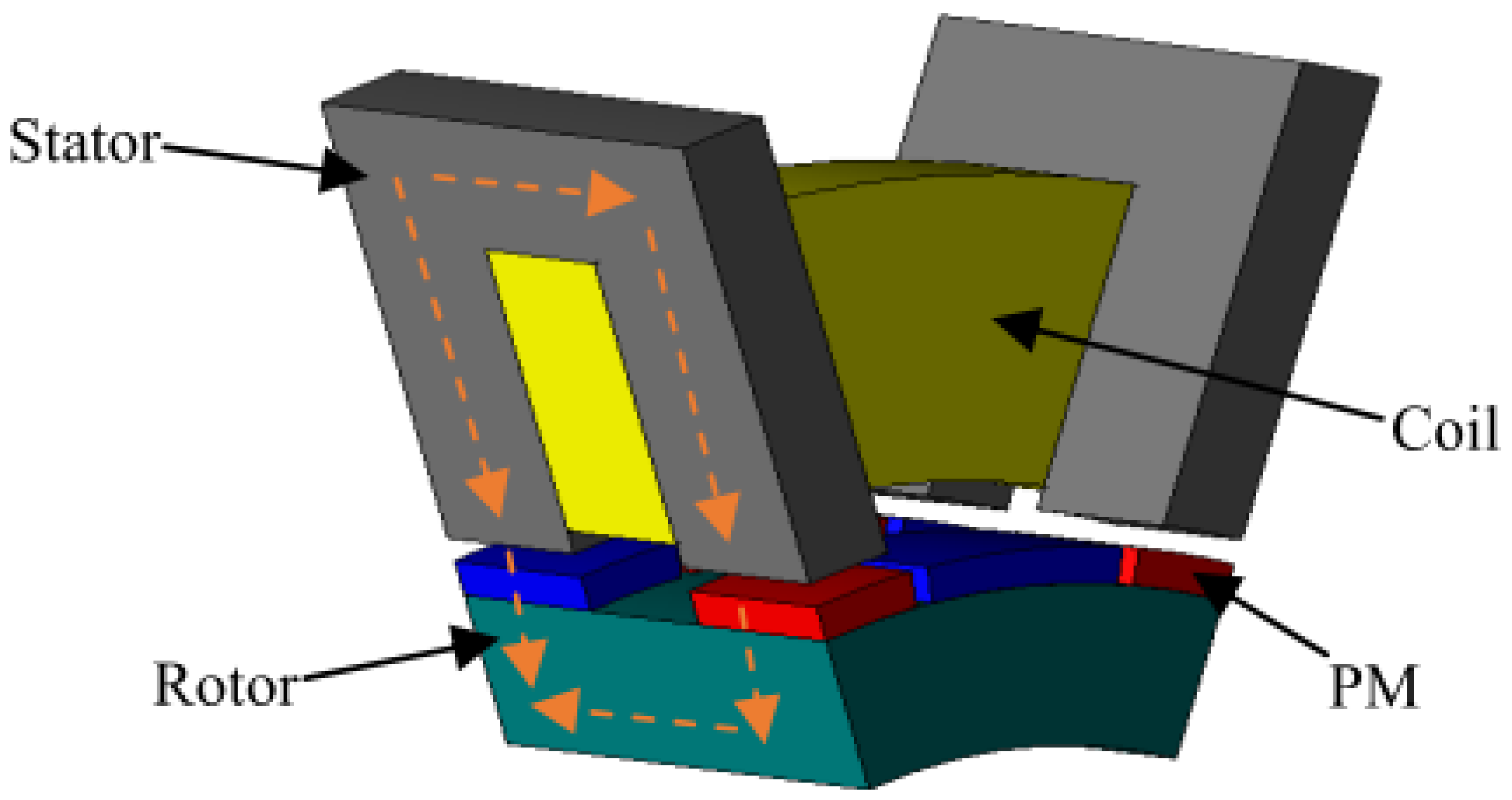
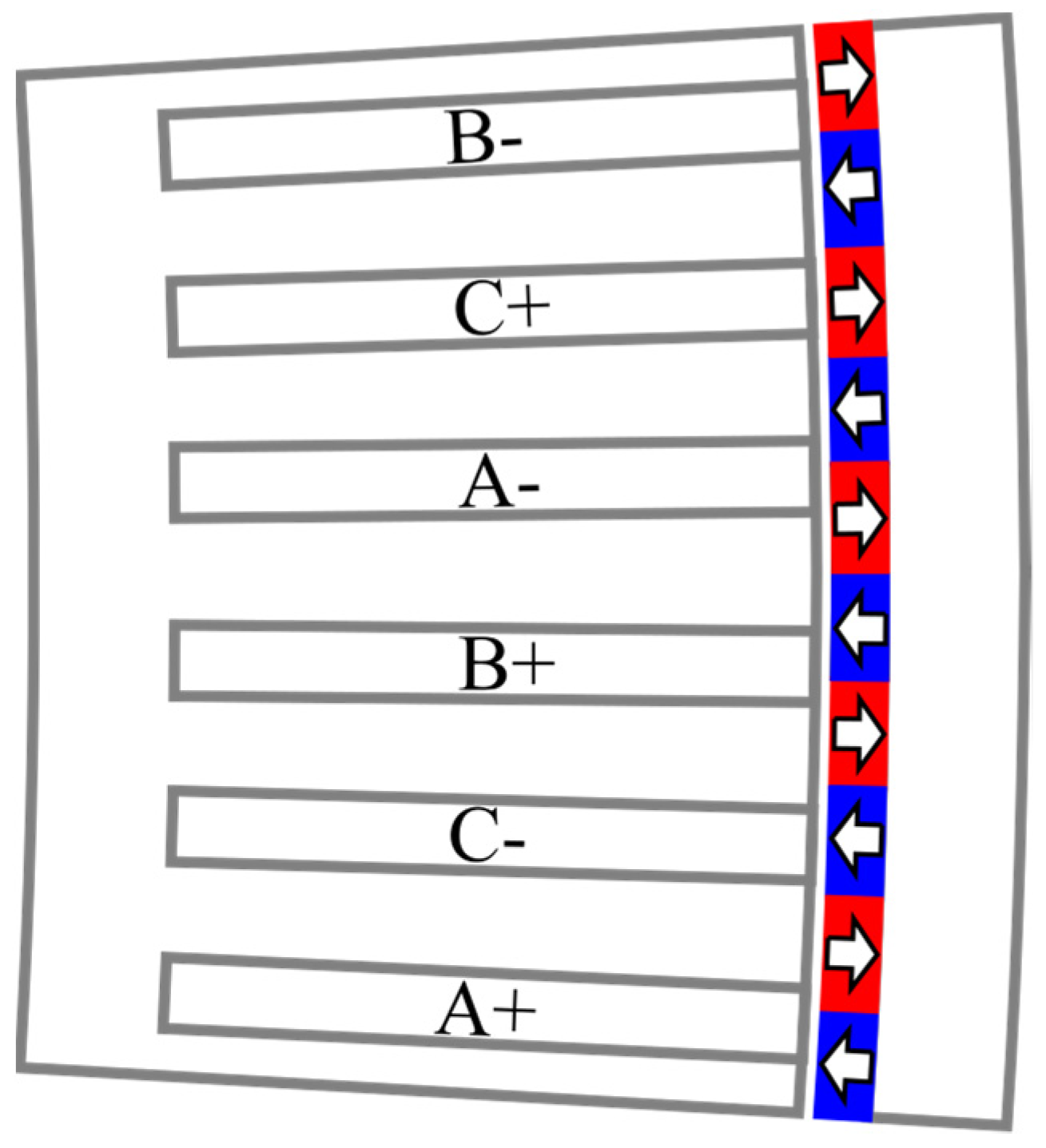
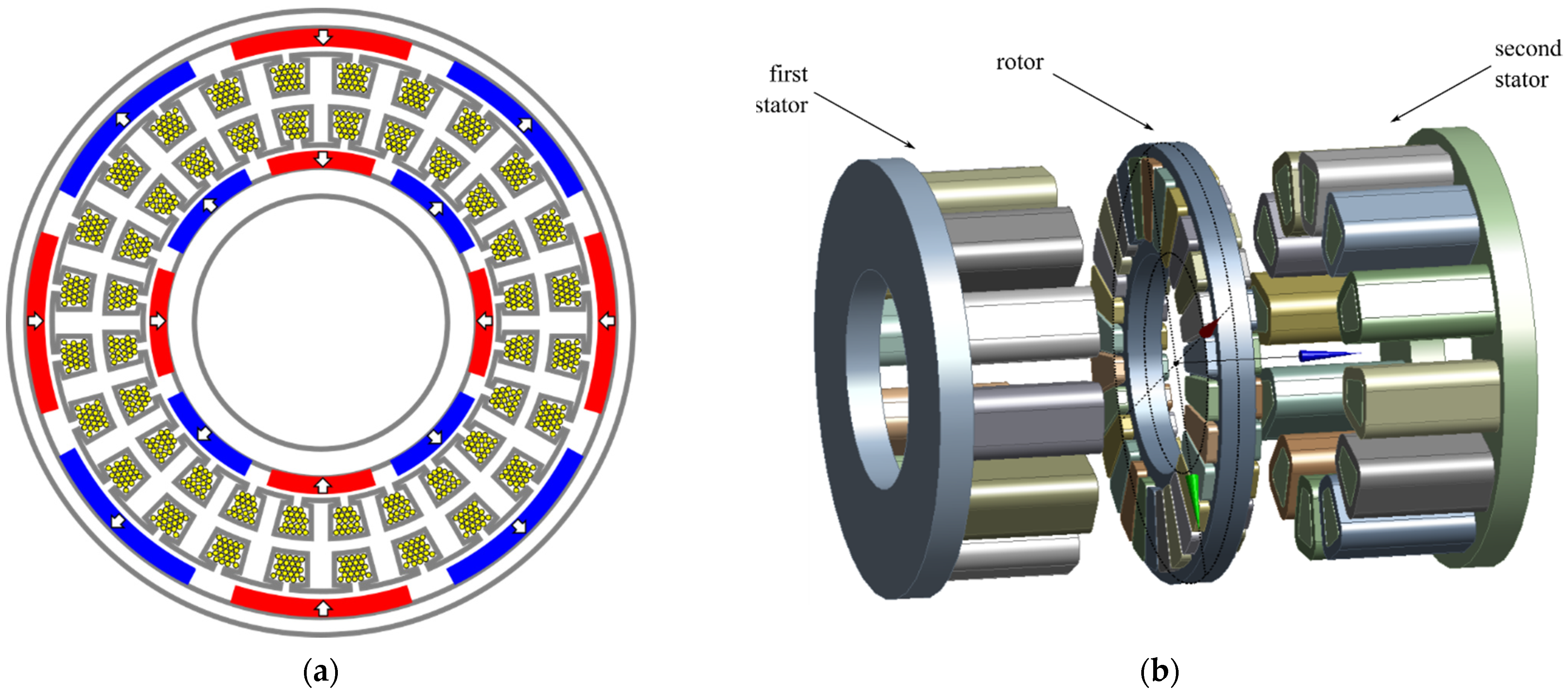
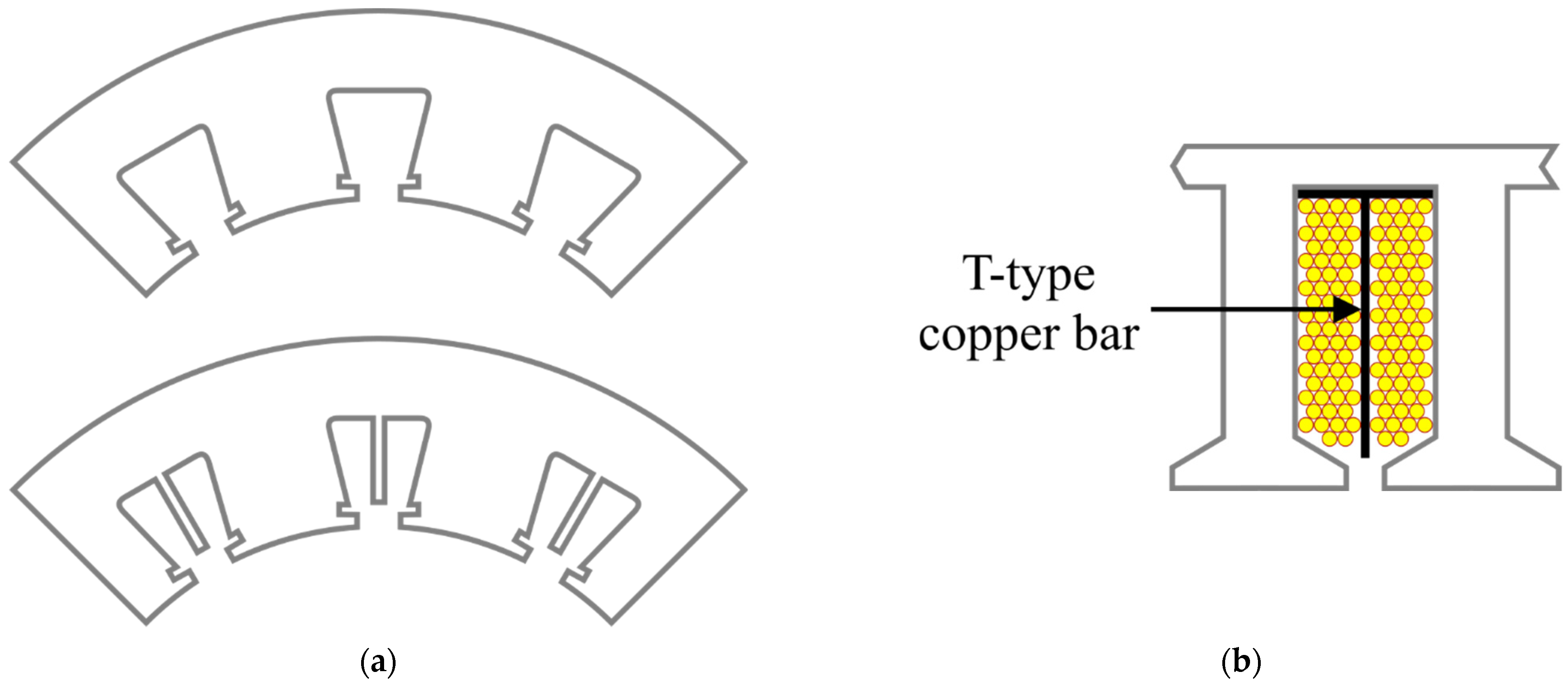
2.3. Topologies
2.4. Superconductor Based Generators
- High power density, even with the associated cryogenic cooling unit. In turn, this technology is directly suitable for direct-drive applications due to high torque density. For the same power ratings as a conventional generator, a superconducting one could achieve 50% size reduction;
- High efficiency due to reduction of Joule losses. Some topologies also avoid iron losses as well due to the removal of the magnetic core as the superconducting topologies can compensate.
3. Cooling Systems for Direct-Drive Generators
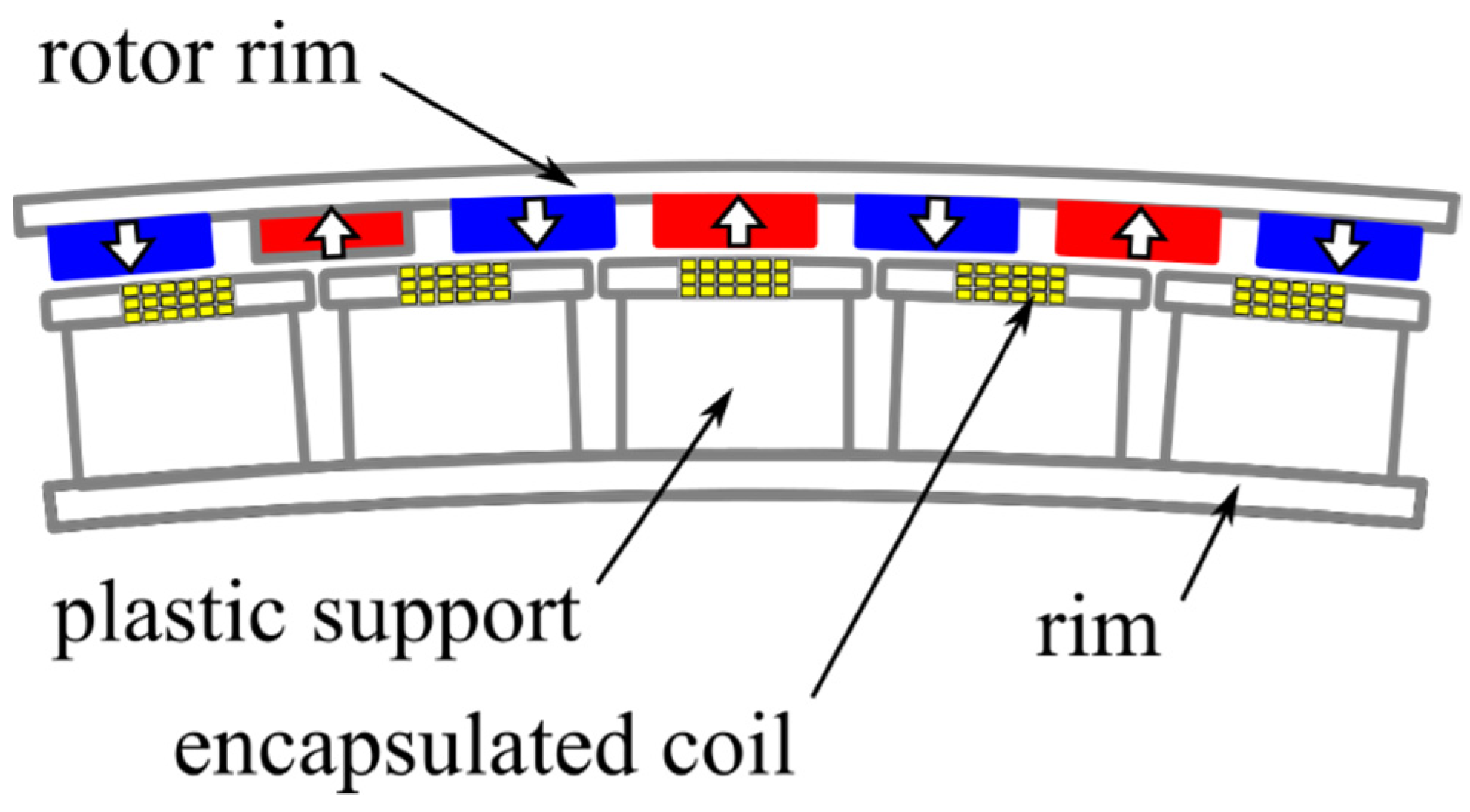
3.1. Passive Cooling Systems
- Designing the machine to reduce the coil cross-section size. Shorter distances from the middle to the side of the coil allow easier heat flow through thermal conduction. Possible options include using multiphase and multipole configurations, which distribute the windings in more slots;
- Increasing the slot filling factor. This reduces the pockets of air or varnish between the wires. The winding’s composite thermal conductivity increases with the slot filling factor [79]. This can be achieved, for example, by using rectangular cross section wires;
- Varnishing the windings. The varnish’s thermal conductivity is about an order of magnitude larger than the air’s, and it displaces the air pockets. Better results can be achieved by using vacuum impregnation as well as varnish with higher thermal conductivity.
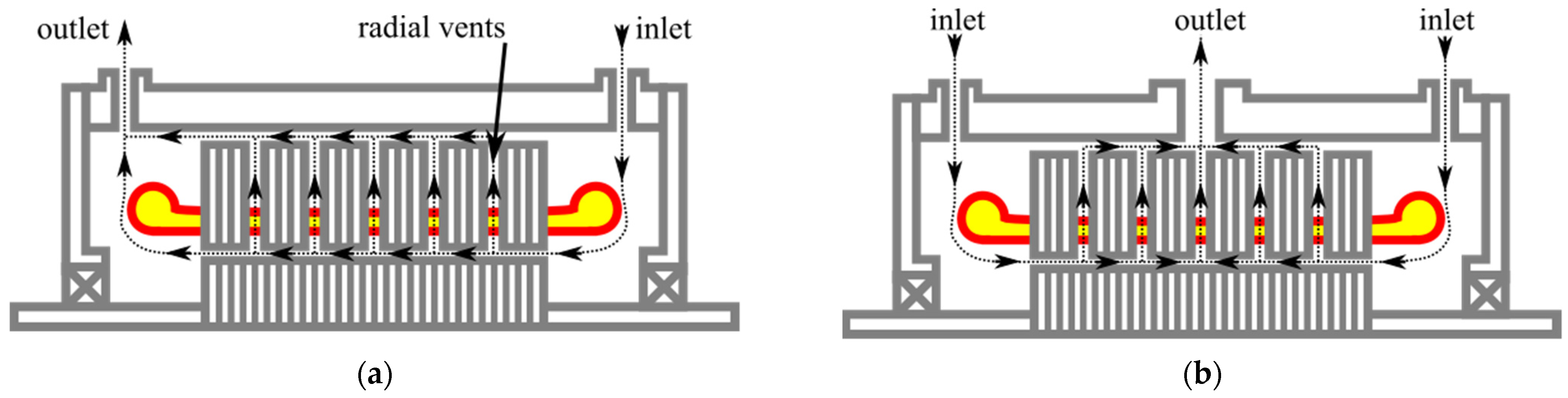
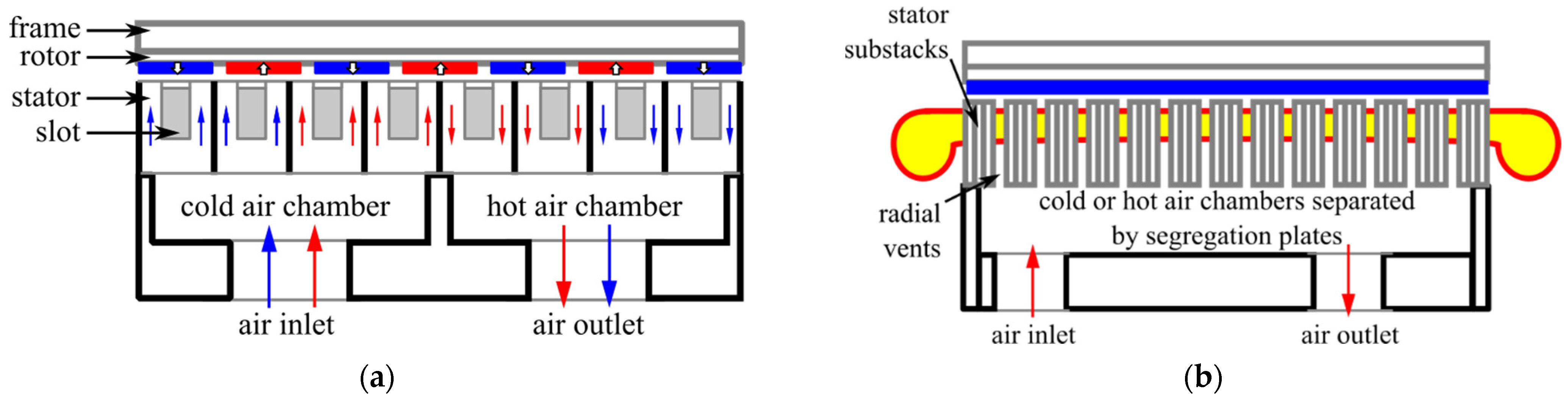
3.2. Forced Air-Cooling Systems
- Open-type housing (open cooling loop) in which the outside air is pushed through the machine. This solution is simpler but requires rugged generator internals in order to withstand contaminants;
- Enclosed-type housing (closed cooling loop). A closed cooling circuit is used which allows purified air. An external heat exchanger is needed to re-cool the air.
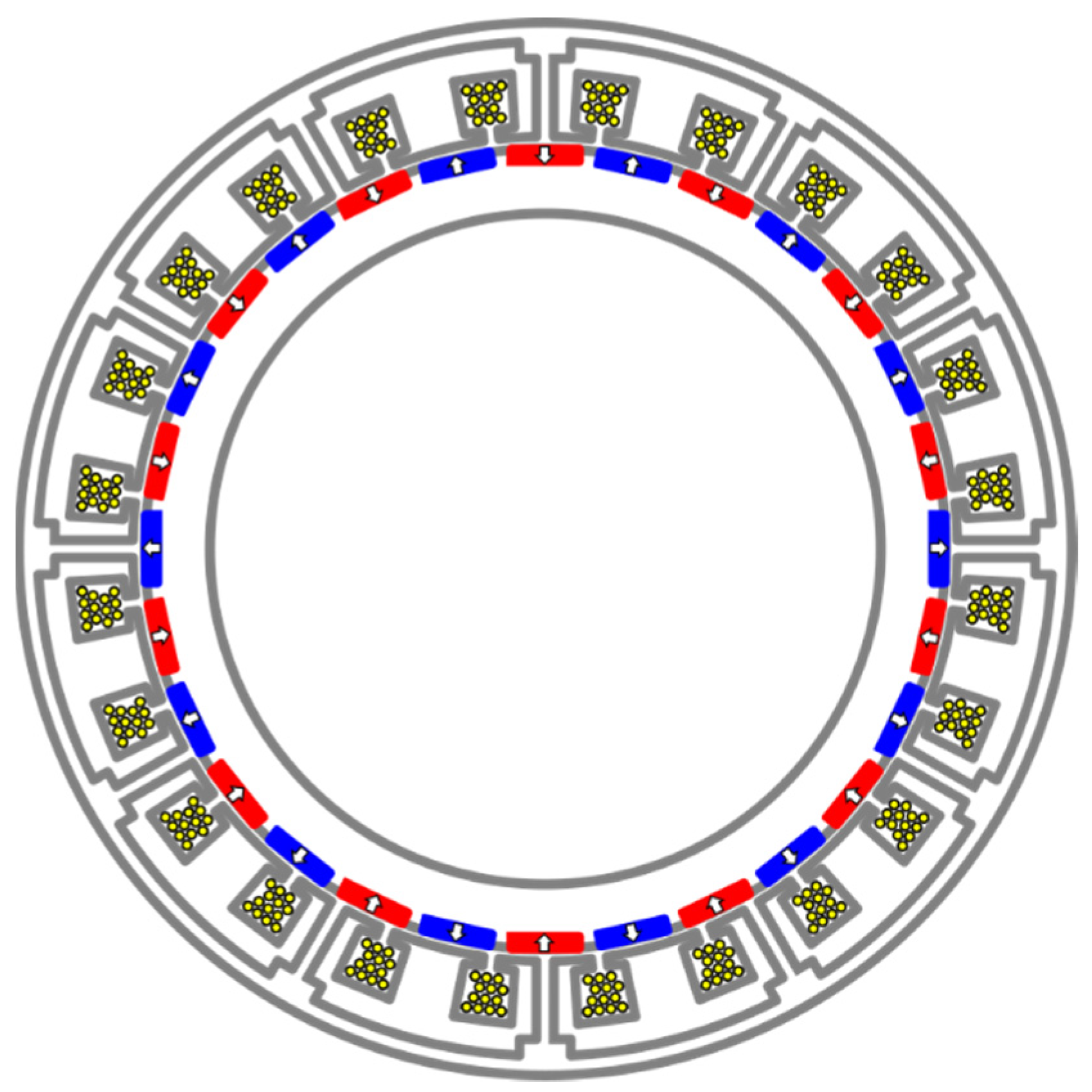
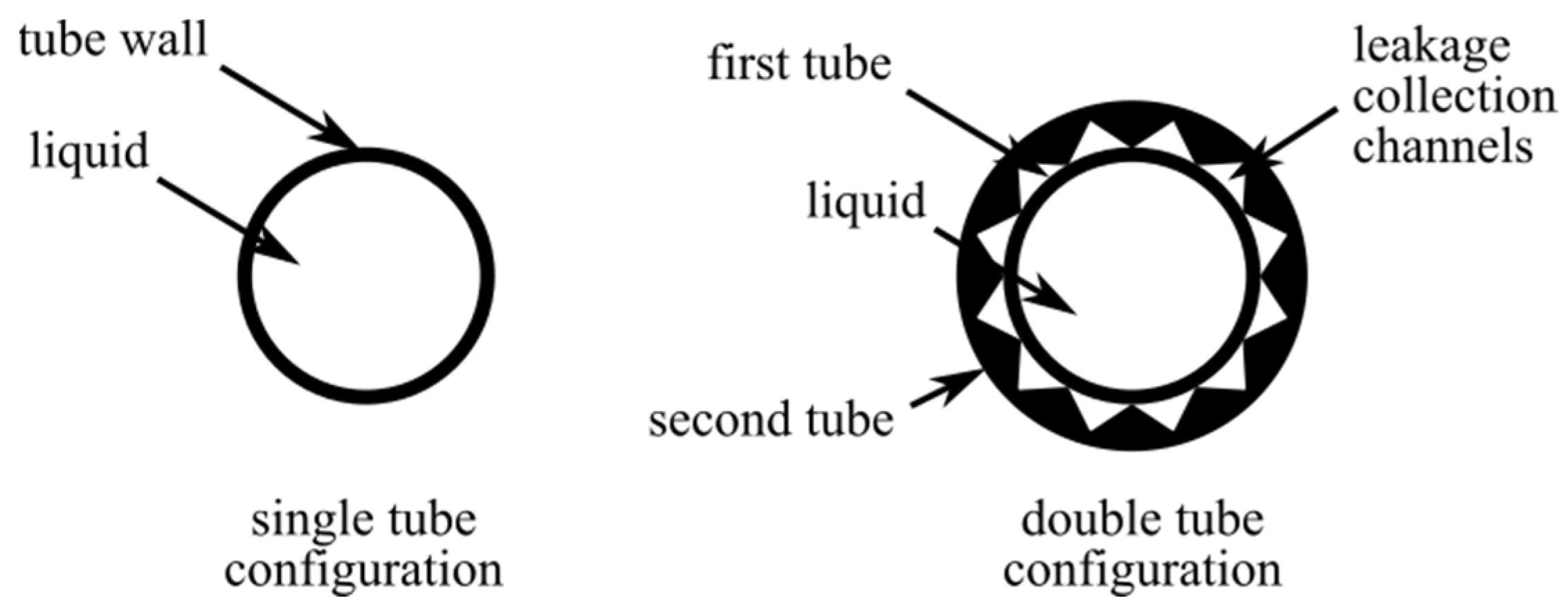
3.3. Liquid Cooling Methods
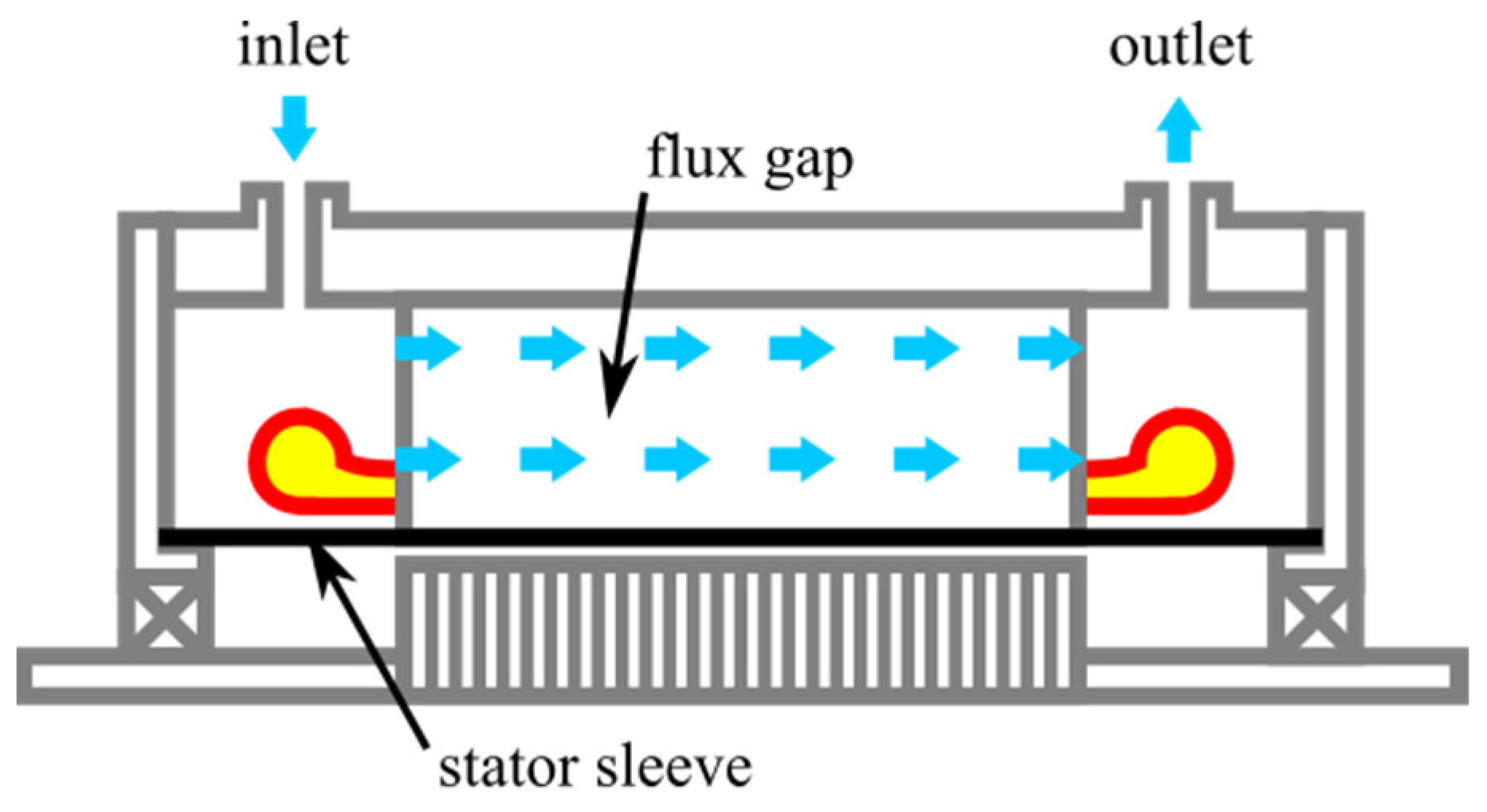
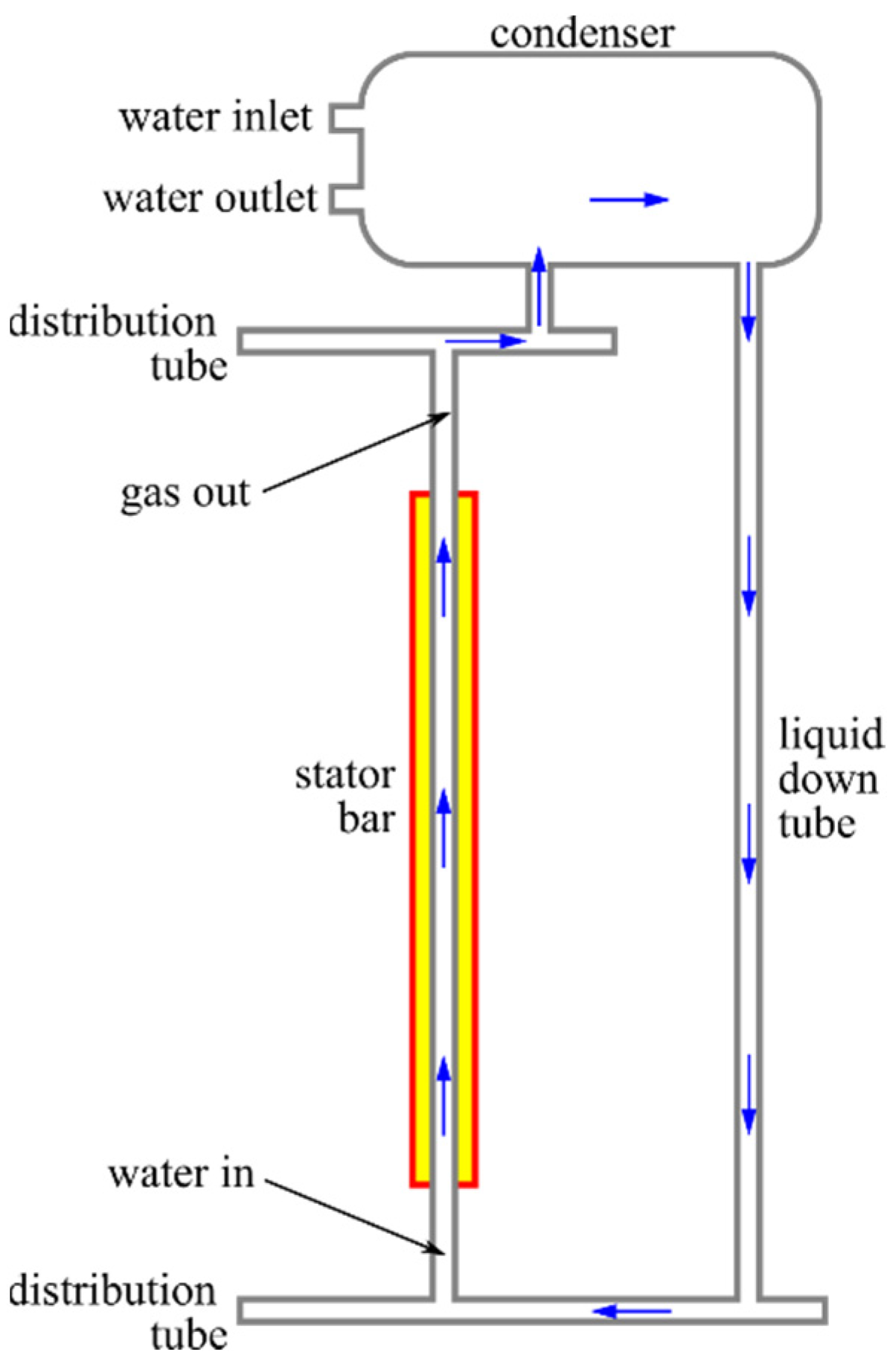
3.4. Multi-Phase Cooling Methods
3.5. Hybrid Cooling Methods
3.6. R&D Cost, Maintenance, Performance and Reliability
- Adopt a safe cooling fluid inside the generator like air or an inert gas. The various sealing systems inside the generator (which prevent leaks) are simpler and require very little maintenance. In the event of a leak, the generator will not be damaged. Air has also the advantage that it is not affected by a freezing point, unlike liquids;
- Adopt a closed loop system. The air is circulated inside the generator and through an external heat exchanger. The generator internals are not exposed to external contaminants like dust, salt, etc., which can further lead to corrosion.
- Air to air (totally enclosed air-to-air cooled—TEAAC or closed air circuit, air cooled—CACA). The primary circuit coolant is air, and the secondary circuit coolant is also air;
- Water to air (totally enclosed water to air—TEWAC or closed air circuit, water cooled—CACW). The primary circuit coolant is air, while the secondary circuit coolant is water.
4. Conclusions
- The highest performing method is the hybrid one, while the lowest performing is the passive cooling (or no cooling). Closed-loop systems incur a penalty as they add extra heat exchangers, which limit heat extraction (i.e., no heat exchanger is perfect). Flooded stator and oil spray can perform better since they are direct cooling types;
- In terms of maintenance, the passive cooling system requires no maintenance (since no active cooling system is present) while the hybrid method needs the most maintenance as it employs two different methods. In addition, the open-loop systems also have increased maintenance since they are exposed to contaminants from the outside environment. For large and offshore generators, expensive filters are required to prevent the contaminants getting inside (the maintenance shift focus on the filter lifetime). Flooded stator and oil spray methods are regarded as higher maintenance than forced-water types (water is contained in the jacket—oil is not);
- It is considered that the total weight of the generator and cooling system combined should be less than a design with no cooling present; otherwise, the cooling system brings no benefits. From this point of view, a generator with passive cooling has the largest weight. Adding any active cooling method will bring an overall weight reduction. Closed loop systems will be heavier than their open-loop counterparts as they need additional heat exchangers. The flooded stator method is also penalized here due to the weight of the liquid inside the stator;
- The cooling capacity (also a measure of scalability) is the lowest for the passive cooling systems. In this case, increasing the rated power of the generator means necessarily increasing the generator size and weight. Adding an active cooling method ensures that the generator size can be kept small, up to a certain point, depending on the coolant used. The hybrid method is the best as it combines advantages from both methods. Closed loops incur a penalty in cooling capacity compared to open-loop methods of the same coolant type;
- In terms of energy, the worst method is the forced-air closed loop method. For this case, fans are required to circulate the coolant in both the primary and secondary cooling circuits. The low effectiveness of air as a coolant fluid is reflected in larger and faster fans needed to increase both the mass flow and air velocity. This is reflected in the cooling hardware as well—a water pump, for example, will be much more compact and less energy intensive;
- The noise is important only for forced air methods due to the large fan size usually employed. For other methods, the pumps are usually smaller and isolated from the outside environment.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- United Nations. Adoptions of the Paris Agreement; United Nations: Paris, France, 2015. [Google Scholar]
- Lee, J.; Zhao, F. Global Wind Report 2021; Global Wind Energy Council: Brussels, Belgium, 2021. [Google Scholar]
- Vestas Wind Systems. Turbines, Life Cycle Assesssment of Offshore and Onshore Sited Wind Power Plants Based on Vestas V90-3.0MW; Vestas Wind Systems: Randers, Denmark, 2006. [Google Scholar]
- Haapala, K.R.; Prempreeda, P. Comparative life cycle assessment of 2.0 MW wind turbines. Int. J. Sustain. Manuf. 2014, 3, 170–185. [Google Scholar] [CrossRef]
- Energy Payback of a Wind Turbine, Vestas Wind Systems. Available online: https://www.vestas.com/en/sustainability/environment/energy-payback (accessed on 1 December 2021).
- Sajid, M.; Hassan, I.; Rahman, M. An overview of cooling of thermoelectric devices. Renew. Sustain. Energy Rev. 2017, 78, 15–22. [Google Scholar] [CrossRef]
- Siemens Gamesa and Siemens Energy to Unlock a New Era of Offshore Green Hydrogen Production, 13 January 2021. Available online: https://press.siemens-energy.com/global/en/pressrelease/siemens-gamesa-and-siemens-energy-unlock-new-era-offshore-green-hydrogen-production (accessed on 1 December 2021).
- Ani, S.O.; Polinder, H.; Ferreira, J.A. Comparison of Energy Yield of Small Wind Turbines in Low Wind Speed Areas. IEEE Trans. Sustain. Energy 2012, 4, 42–49. [Google Scholar] [CrossRef]
- Morandin, M.; Fornasiero, E.; Bolognani, S.; Bianchi, N. Torque and Power Rating of a Wind-Power PM Generator Drive for Maximum Profit-to-Cost Ratio. IEEE Trans. Ind. Appl. 2013, 49, 866–872. [Google Scholar] [CrossRef]
- Polinder, H.; van der Pijl, F.F.A.; de Vilder, G.J.; Tavner, P.J. Comparison of direct-drive and geared generator concepts for wind turbines. IEEE Trans. Energy Convers. 2006, 21, 725–733. [Google Scholar] [CrossRef]
- Camm, E.; Behnke, M.R.; Bolado, O.; Bollen, M.; Bradt, M.; Brooks, C.; Dilling, W.; Edds, M.; Hejdak, W.J.; Houseman, D.; et al. Characteristics of wind turbine generators for wind power plants. In Proceedings of the 2009 IEEE Power & Energy Society General Meeting, Calgary, AB, Canada, 26–30 July 2009; pp. 1–5. [Google Scholar] [CrossRef]
- Jiang, R.; Wang, J.; Guan, Y. Overview of Multi-MW Wind Turbines and Wind Parks. IEEE Trans. Ind. Electron. 2011, 58, 1081–1095. [Google Scholar] [CrossRef]
- Bang, D.; Polinder, H.; Shrestha, G.; Ferreira, J.A. Promising direct-drive generator system for large wind turbines. In Proceedings of the 2008 Wind Power to the Grid—EPE Wind Energy Chapter 1st Seminar, Delft, The Netherlands, 27–28 March 2008. [Google Scholar]
- Semken, R.S.; Polikarpova, M.; Röyttä, P.; Alexandrova, J.; Pyrhönen, J.; Nerg, J.; Mikkola, A.; Backman, J. Direct-drive permanent magnet generators for high-power wind turbines: Benefits and limiting factors. IET Renew. Power Gener. 2012, 6, 1–8. [Google Scholar] [CrossRef]
- Polinder, H.; Ferreira, J.A.; Jensen, B.B.; Abrahamsen, A.B.; Atallah, K.; McMahon, R.A. Trends in Wind Turbine Generator Systems. IEEE J. Emerg. Sel. Top. Power Electron. 2013, 1, 174–185. [Google Scholar] [CrossRef]
- Bang, D.; Polinder, H.; Shrestha, G.; Ferreira, J.A. Review of generator systems for direct-drive wind turbines. In Proceedings of the EWEC 2008, Brussels, Belgium, 31 March–3 April 2008. [Google Scholar]
- Siemens Gamesa Wind Turbines and Services. Available online: https://www.siemensgamesa.com/en-int/products-and-services (accessed on 4 February 2022).
- V236-15.0 MW at a Glance, Vestas. Available online: https://www.vestas.com/en/products/offshore/V236-15MW (accessed on 1 December 2021).
- GE Renewable Energy Products Page. Available online: https://www.ge.com/renewableenergy/wind-energy (accessed on 4 February 2022).
- The Nordex Group Portfolio. Available online: https://www.nordex-online.com/en/product/product-main-page/ (accessed on 4 February 2022).
- Enercon, E-160 EP5. Available online: https://www.enercon.de/en/products/ep-5/e-160-ep5/ (accessed on 12 January 2022).
- Mueller, M.; Polinder, H. Electrical Drives for Direct Drive Renewable Energy Systems; Woodhead Publishing Ltd.: Cambridge, UK, 2013. [Google Scholar] [CrossRef]
- SG 8.0-167 DD Offshore Wind Turbine, Siemens-Gamesa. Available online: https://www.siemensgamesa.com/en-int/products-and-services/offshore/wind-turbine-sg-8-0-167-dd (accessed on 1 December 2021).
- Haliade-X Offshore Wind Turbine, GE Renewable Energy. Available online: https://www.ge.com/renewableenergy/wind-energy/offshore-wind/haliade-x-offshore-turbine (accessed on 1 December 2021).
- SG 11.0-200 DD Offshore Wind Turbine, Siemens-Gamesa. Available online: https://www.siemensgamesa.com/en-int/products-and-services/offshore/wind-turbine-sg-11-0-200-dd (accessed on 1 December 2021).
- SG 14-222 DD Offshore Wind Turbine, Siemens-Gamesa. Available online: https://www.siemensgamesa.com/en-int/products-and-services/offshore/wind-turbine-sg-14-222-dd (accessed on 1 December 2021).
- Popescu, M.; Staton, D.A.; Boglietti, A.; Cavagnino, A.; Hawkins, D.; Goss, J. Modern heat extraction systems for power traction systems. IEEE Trans. Ind. Appl. 2016, 52, 2167–2175. [Google Scholar] [CrossRef]
- Nishanth, F.; Johnson, M.; Severson, E.L. A Review of Thermal Analysis and Management of Power Dense Electric Machines. In Proceedings of the 2021 IEEE International Electric Machines & Drives Conference (IEMDC), Hartford, CT, USA, 17–20 May 2021; pp. 1–8. [Google Scholar] [CrossRef]
- Gai, Y.H.; Kimiabeigi, M.; Yew, C.C.; Deng, X.; Popescu, M.; Goss, J.; Staton, D.A.; Steven, A. Cooling of automotive traction motors: Schemes, examples, and computation methods. IEEE Trans. Ind. Electron. 2019, 68, 1681–1692. [Google Scholar] [CrossRef]
- Polikarpova, M.; Ponomarev, P.; Lindh, P.; Petrov, I.; Jara, W.; Naumanen, V.; Tapia, J.A.; Pyrhonen, J. Hybrid Cooling Method of Axial-Flux Permanent-Magnet Machines for Vehicle Applications. IEEE Trans. Ind. Electron. 2015, 62, 7382–7390. [Google Scholar] [CrossRef]
- Boglietti, A.; Cavagnino, A.; Staton, D.; Shanel, M.; Mueller, M.; Mejuto, C. Evolution and Modern Approaches for Thermal Analysis of Electrical Machines. IEEE Trans. Ind. Electron. 2009, 56, 871–882. [Google Scholar] [CrossRef]
- Growald, P.O.; Kern, T.A. Traction motor cooling systems: A literature review and comparative study. IEEE Trans. Transp. Electrif. 2021, 7, 2892–2913. [Google Scholar] [CrossRef]
- Miller, T.J.E. Brushless Permanent-Magnet and Reluctance Motor Drives; Clarendon Press: Oxford, UK, 1989. [Google Scholar]
- Chen, J.Y.; Nayar, C.V.; Xu, L.Y. Design and finite-element analysis of an outer-rotor permanent-magnet generator for directly coupled wind turbines. IEEE Trans. Ind. Electron. 2000, 36, 3802–3809. [Google Scholar] [CrossRef]
- Choi, J.-Y.; Jang, S.-M.; Song, B.-M. Design of a direct-coupled radial-flux permanent magnet generator for wind turbines. In Proceedings of the 2021 IEEE International Electric Machines & Drives Conference (IEMDC), Hartford, CT, USA, 17–20 May 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Pyrhonen, J.; Alexandrova, Y.; Semken, R.S.; Hamalainen, H. Wind power electrical drives for permanent magnet generators—Development in Finland. In Proceedings of the Elektro 2012, Rajecke Teplice, Slovakia, 21–22 May 2012. [Google Scholar]
- Kowal, D.; Sergeant, P.; Dupre, L.; Vandenbossche, L. The effect of the electrical steel properties on the temperature distribution in direct-drive PM synchronous generators for 5 MW wind turbines. IEEE Trans. Magn. 2013, 49, 5371–5377. [Google Scholar] [CrossRef]
- Xue, S.; Feng, J.H.; Guo, S.Y.; Peng, J.; Chu, W.Q.; Zhu, Z.Q. A new iron loss model for temperature dependencies of hysteresis and eddy current losses in electrical machines. IEEE Trans. Magn. 2018, 54, 8100310. [Google Scholar] [CrossRef]
- Xue, S.; Zhu, Z.Q.; Wang, Y.; Feng, J.G.; Guo, S.Y.; Li, Y.F.; Chen, Z.C.; Peng, J. Thermal-loss coupling analysis of an electrical machine using the improved temperature-dependent iron loss model. IEEE Trans. Magn. 2018, 54, 8105005. [Google Scholar] [CrossRef]
- Ruoho, S.; Kolehmainen, J.; Ikaheimo, J.; Arkkio, A. Interdependence of Demagnetization, Loading, and Temperature Rise in a Permanent-Magnet Synchronous Motor. IEEE Trans. Magn. 2009, 46, 949–953. [Google Scholar] [CrossRef]
- Sebastian, T. Temperature effects on torque production and efficiency of PM motors using NdFeB magnets. IEEE Trans. Ind. Appl. 2002, 31, 353–357. [Google Scholar] [CrossRef]
- Chen, J.Q.; Chen, Z.H.; Wang, D.; Wu, L.T.; Zheng, X.Q.; Birnkammer, F.; Gerling, D. Influence of temperature on mag-netic properties of silicon steel lamination. AIP Adv. 2017, 7, 056113. [Google Scholar]
- Wallscheid, O. Thermal Monitoring of Electric Motors: State-of-the-Art Review and Future Challenges. IEEE Open J. Ind. Appl. 2021, 2, 204–223. [Google Scholar] [CrossRef]
- Ruoho, S.; Dlala, E.; Arkkio, A. Comparison of Demagnetization Models for Finite-Element Analysis of Permanent-Magnet Synchronous Machines. IEEE Trans. Magn. 2007, 43, 3964–3968. [Google Scholar] [CrossRef]
- ARNOLD Magnetic Technologies. RECOMA SmCo Grades. Available online: https://www.arnoldmagnetics.com/wp-content/uploads/2017/10/Recoma-Combined-160301.pdf (accessed on 12 January 2022).
- Pyrhonen, J.; Nerg, J.; Kurronen, P.; Puranen, J.; Haavisto, M. Permanent magnet technology in wind power generators. In Proceedings of the XIX International Conference on Electrical Machines—ICEM 2010, Rome, Italy, 6–8 September 2010; pp. 1–6. [Google Scholar] [CrossRef]
- McDonald, A.; Bhuiyan, N.A. On the Optimization of Generators for Offshore Direct Drive Wind Turbines. IEEE Trans. Energy Convers. 2016, 32, 348–358. [Google Scholar] [CrossRef]
- Arnold Magnetic Technologies, Neodymium Iron Boron Magnet Catalog. Available online: https://www.arnoldmagnetics.com/wp-content/uploads/2019/06/Arnold-Neo-Catalog.pdf (accessed on 28 November 2021).
- IEC. IEC 60085:2007 Electrical Insulation—Thermal Evaluation and Designation. Available online: https://standards.iteh.ai/catalog/standards/iec/2584c3d3-f618-40cc-b0fd-0f9e66f45e84/iec-60085-2007 (accessed on 28 November 2021).
- Grauers, A. Design of Direct-Driven Permanent-Magnet Generators for Wind Turbines. Ph.D. Dissertation, Chalmers University of Technology, Goteborg, Sweden, 1996. [Google Scholar]
- Dubois, M.R.J. Optimized Permanent Magnet Generator Topologies for Direct-Drive Wind Turbines. Ph.D. Dissertation, Technical University of Delft, Delft, The Netherlands, 2004. [Google Scholar]
- Damiano, A.; Marongiu, I.; Monni, A.; Porru, M. Design of a 10 MW multi-phase PM synchronous generator for direct-drive wind turbines. In Proceedings of the IECON 2013—39th Annual Conference of the IEEE Industrial Electronics Society, Vienna, Austria, 10–13 November 2013; pp. 5266–5270. [Google Scholar] [CrossRef]
- Jia, S.; Qu, R.; Li, J.; Fan, X.; Zhang, M. Study of direct-drive permanent-magnet synchronous generators with solid rotor back iron and different windings. IEEE Trans. Ind. Appl. 2016, 52, 1369–1379. [Google Scholar]
- Chen, Y.; Pillay, P.; Khan, A. PM Wind Generator Topologies. IEEE Trans. Ind. Appl. 2005, 41, 1619–1626. [Google Scholar] [CrossRef]
- Meier, F. Permanent-Magnet Synchronous Machines with Non-Overlapping Concentrated Windings for Low-Speed Direct-Drive Applications. Ph.D. Thesis, Royal Institute of Technology School of Electrical Engineering Electrical Machines and Power Electronics, Stockholm, Sweden, 2008. [Google Scholar]
- Padinharu, D.K.K.; Li, G.J.; Zhu, Z.Q.; Foster, M.P.; Stone, D.A.; Griffo, A.; Clark, R.; Thomas, A. Scaling effect on electromagnetic performance of surface-mounted permanent-magnet vernier machine. IEEE Trans. Magn. 2020, 56, 8100715. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Chen, J.T. Advanced Flux-Switching Permanent Magnet Brushless Machines. IEEE Trans. Magn. 2010, 46, 1447–1453. [Google Scholar] [CrossRef]
- Cheng, M.; Hua, W.; Zhang, J.; Zhao, W. Overview of Stator-Permanent Magnet Brushless Machines. IEEE Trans. Ind. Electron. 2011, 58, 5087–5101. [Google Scholar] [CrossRef]
- Li, F.; Hua, W.; Tong, M.; Zhao, G.; Cheng, M. Nine-Phase Flux-Switching Permanent Magnet Brushless Machine for Low-Speed and High-Torque Applications. IEEE Trans. Magn. 2015, 51, 8700204. [Google Scholar] [CrossRef]
- Shao, L.Y.; Hua, W.; Soulard, J.; Zhu, Z.Q.; Wu, Z.Z.; Cheng, M. Electromagnetic performance comparison between 12-phase switched flux and surface-mounted PM machines for direct-drive wind power generation. IEEE Trans. Ind. Appl. 2020, 56, 1408–1422. [Google Scholar] [CrossRef]
- Cheng, M.; Wang, J.; Zhu, S.; Wang, W. Loss Calculation and Thermal Analysis for Nine-Phase Flux Switching Permanent Magnet Machine. IEEE Trans. Energy Convers. 2018, 33, 2133–2142. [Google Scholar] [CrossRef]
- Ojeda, J.; Simoes, M.; Li, G.-J.; Gabsi, M. Design of a Flux-Switching Electrical Generator for Wind Turbine Systems. IEEE Trans. Ind. Appl. 2012, 48, 1808–1816. [Google Scholar] [CrossRef]
- Muljadi, E.; Butterfield, C.; Wan, Y.-H. Axial-flux modular permanent-magnet generator with a toroidal winding for wind-turbine applications. IEEE Trans. Ind. Appl. 1999, 35, 831–836. [Google Scholar] [CrossRef]
- Ani, S.O.; Polinder, H.; Ferreira, J.A. Low cost axial flux permanent magnet generator for small wind turbines, In Proceedings of the ECCE2021, Raleigh, NC, USA, 15–20 September 2012.
- Marignetti, F.; Colli, V.D.; Coia, Y. Design of Axial Flux PM Synchronous Machines Through 3-D Coupled Electromagnetic Thermal and Fluid-Dynamical Finite-Element Analysis. IEEE Trans. Ind. Electron. 2008, 55, 3591–3601. [Google Scholar] [CrossRef]
- Kumar, R.; Zhu, Z.-Q.; Duke, A.; Thomas, A.; Clark, R.; Azar, Z.; Wu, Z.-Y. A Review on Transverse Flux Permanent Magnet Machines for Wind Power Applications. IEEE Access 2020, 8, 216543–216565. [Google Scholar] [CrossRef]
- Husain, T.; Hasan, I.; Sozer, Y.; Husain, I.; Muljadi, E. A comprehensive review of permanent magnet transverse flux ma-chines: Use in direct-drive applications. IEEE Ind. Appl. Mag. 2020, 26, 87–98. [Google Scholar] [CrossRef]
- Ishizaki, A.; Tanaka, T.; Takasaki, K.; Nishikata, S. Theory and optimum design of PM Vernier motor. In Proceedings of the 7th ICEMD, Durham, UK, 11–13 September 1995. [Google Scholar]
- Li, J.; Chau, K.T.; Jiang, J.Z.; Liu, C.; Li, W. A New Efficient Permanent-Magnet Vernier Machine for Wind Power Generation. IEEE Trans. Magn. 2010, 46, 1475–1478. [Google Scholar] [CrossRef]
- Li, D.; Qu, R.; Li, J. Topologies and analysis of flux-modulation machines. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 2153–2160. [Google Scholar] [CrossRef]
- Padinharu, D.K.; Li, G.J.; Zhu, Z.Q.; Azar, Z.; Clark, R.; Thomas, A. Effect of airgap length on electromagnetic performance of permanent magnet Vernier machines with different power ratings. IEEE Trans. Ind. Appl. 2022, 58, 1920–1930. [Google Scholar] [CrossRef]
- Ani, S.; Polinder, H.; Ferreira, J. Energy yield of two generator systems for small wind turbine application. In Proceedings of the 2011 IEEE International Electric Machines & Drives Conference (IEMDC), Niagara Falls, ON, Canada, 15–18 May 2011; pp. 735–740. [Google Scholar] [CrossRef]
- Qu, R.; Lipo, T. Design and Parameter Effect Analysis of Dual-Rotor, Radial-Flux, Toroidally Wound, Permanent-Magnet Machines. IEEE Trans. Ind. Appl. 2004, 40, 771–779. [Google Scholar] [CrossRef]
- Kumar, B.R.; Kumar, K.S. Siva Design of a new dual rotor radial flux BLDC motor with Halbach array magnets for an electric vehicle. In Proceedings of the PEDES2016, Trivandrum, India, 14–17 December 2016. [Google Scholar]
- Bi, Y.; Chai, F.; Chen, L. The Study of Cooling Enhancement in Axial Flux Permanent Magnet Motors for Electric Vehicles. IEEE Trans. Ind. Appl. 2021, 57, 4831–4839. [Google Scholar] [CrossRef]
- Howey, D.A.; Childs, P.R.N.; Holmes, A.S. Air-Gap Convection in Rotating Electrical Machines. IEEE Trans. Ind. Electron. 2010, 59, 1367–1375. [Google Scholar] [CrossRef]
- Bergman, T.L.; Lavine, A.S.; Incropera, F.P.; Dewitt, D.P. Fundamentals of Heat and Mass Transfer, 7th ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2011. [Google Scholar]
- Polikarpova, M. Liquid Cooling Solutions for Rotating Permanent Magnet Synchronous Machines. Ph.D. Dissertation, Lappeenranta University of Technology, Lappeenranta, Finland, 2014. [Google Scholar]
- Staton, D.A.; Cavagnino, A. Convection Heat Transfer and Flow Calculations Suitable for Electric Machines Thermal Models. IEEE Trans. Ind. Electron. 2008, 55, 3509–3516. [Google Scholar] [CrossRef]
- Zhang, F.; Gerada, D.; Xu, Z.; Zhang, X.; Tighe, C.; Zhang, H.; Gerada, C. Back-Iron Extension Thermal Benefits for Electrical Machines With Concentrated Windings. IEEE Trans. Ind. Electron. 2019, 67, 1728–1738. [Google Scholar] [CrossRef]
- Galea, M.; Gerada, C.; Raminosoa, T.; Wheeler, P. A Thermal Improvement Technique for the Phase Windings of Electrical Machines. IEEE Trans. Ind. Appl. 2011, 48, 79–87. [Google Scholar] [CrossRef]
- Zhang, Z.; Matveev, A.; Nilssen, R.; Nysveen, A. Ironless Permanent-Magnet Generators for Offshore Wind Turbines. IEEE Trans. Ind. Appl. 2013, 50, 1835–1846. [Google Scholar] [CrossRef]
- Spooner, E.; Gordon, P.; Bumby, J.; French, C. Lightweight ironless-stator PM generators for direct-drive wind turbines. IEE Proc. Electr. Power Appl. 2005, 152, 17–26. [Google Scholar] [CrossRef]
- Dropkin, D.; Carmi, A. Natural-Convection Heat Transfer From a Horizontal Cylinder Rotating in Air. J. Fluids Eng. 1957, 79, 741–749. [Google Scholar] [CrossRef]
- Nakahama, T.; Biswas, D.; Kawano, K.; Ishibashi, F. Improved Cooling Performance of Large Motors Using Fans. IEEE Trans. Energy Convers. 2006, 21, 324–331. [Google Scholar] [CrossRef]
- Nakahama, T.; Ishibashi, F.; Sato, K.; Kawano, K. Effects of fan blade forward-swept and inclined amounts in electric motors. IEEE Trans. Energy Convers. 2010, 25, 457–464. [Google Scholar] [CrossRef]
- Dabbousi, R.; Balfaqih, H.; Anundsson, Y.; Savinovic, D. A comparison of totally enclosed motor coolers commonly used in the COG industry. In Proceedings of the 2008 5th Petroleum and Chemical Industry Conference Europe—Electrical and Instrumentation Applications, Weimar, Germany, 10–12 June 2008; pp. 1–5. [Google Scholar] [CrossRef]
- Fan, X.G.; Qu, R.H.; Li, J.; Li, D.W.; Zhang, B.; Wang, C. Ventilation and thermal improvement of radial forced air-cooled FSCW permanent magnet synchronous wind generators. IEEE Trans. Ind. Appl. 2017, 53, 3447–3456. [Google Scholar] [CrossRef]
- Nerg, J.; Ruuskanen, V. Lumped-parameter-based thermal analysis of a doubly radial forced-air-cooled direct-driven permanent magnet wind generator, Mathematics and Computers in Simulation (Science Direct). Math. Comput. Simul. 2013, 90, 218–229. [Google Scholar] [CrossRef]
- Pyrhonen, J.; Ruuskanen, V.; Nerg, J.; Puranen, J.; Jussila, H. Permanent-Magnet Length Effects in AC Machines. IEEE Trans. Magn. 2010, 46, 3783–3789. [Google Scholar] [CrossRef]
- Ruuskanen, V.; Nerg, J.; Pyrhonen, J. Effect of lamination stack ends and radial cooling channels on noload voltage and inductances of permanent-magnet synchronous machines. IEEE Trans. Magn. 2011, 47, 4643–4649. [Google Scholar] [CrossRef]
- Zhou, R.; Li, G.J.; Zhu, Z.Q.; Foster, M.P.; Stone, D.A. Improved Cooling in Modular Consequent Pole PM Machine Utilizing Flux Gaps. In Proceedings of the 2020 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 11–15 October 2020; pp. 4253–4260. [Google Scholar] [CrossRef]
- Nollau, A.; Gerling, D. Novel cooling methods using flux-barriers. In Proceedings of the 2014 International Conference on Electrical Machines (ICEM), Berlin, Germany, 2–5 September 2014; pp. 1328–1333. [Google Scholar] [CrossRef]
- Fan, J.X.; Zhang, C.N.; Wang, Z.F.; Strangas, E.G. Thermal analysis of water cooled surface mount permanent magnet electric motor for electric vehicle. In Proceedings of the ICEMS2010, Incheon, Korea, 10–13 October 2010. [Google Scholar]
- Ye, Z.-N.; Luo, W.-D.; Zhang, W.-M.; Feng, Z.-X. Simulative analysis of traction motor cooling system based on CFD. In Proceedings of the 2011 International Conference on Electric Information and Control Engineering, Wuhan, China, 15–17 April 2011; pp. 746–749. [Google Scholar] [CrossRef]
- Pechanek, R.; Bouzek, L. Analyzing of two types water cooling electric motors using computational fluid dynamics. In Proceedings of the 2012 15th International Power Electronics and Motion Control Conference (EPE/PEMC), Novi Sad, Serbia, 4–6 September 2012. [Google Scholar] [CrossRef]
- Di Gerlando, A.; Foglia, G.M.; Iacchetti, M.F.; Perini, R. Thermal modeling for the design and check of an axial flux PM motor. In Proceedings of the ICEM2014, Berlin, Germany, 2–5 September 2014. [Google Scholar]
- Fasquelle, A.; Laloy, D. Water cold plates cooling in a permanent magnet synchronous motor. IEEE Trans. Ind. Appl. 2017, 53, 4406–4413. [Google Scholar] [CrossRef]
- Fan, X.; Li, D.; Qu, R.; Wang, C.; Fang, H. Water Cold Plates for Efficient Cooling: Verified on a Permanent-Magnet Machine With Concentrated Winding. IEEE Trans. Ind. Electron. 2019, 67, 5325–5336. [Google Scholar] [CrossRef]
- Alexandrova, Y. Wind Turbine Direct-Drive Permanent-Magnet Generator with Direct Liquid Cooling for Mass Reduction. Ph.D. Dissertation, Lappeenranta University of Technology, Lappeenranta, Finland, 2014. [Google Scholar]
- Polikarpova, M.; Ponomarev, P.; Röyttä, P.; Semken, S.; Alexandrova, Y.; Pyrhönen, J. Direct liquid cooling for an outer--rotor direct--drive permanent--magnet synchronous generator for wind farm applications. IET Electr. Power Appl. 2015, 9, 523–532. [Google Scholar] [CrossRef]
- Wang, H.Y.; Su, P.S.; Wang, X.H. Calculation on the thermal field of the water cooling stator of Three-Gorge hydro-generator, In Proceedings of the ICEMS2005, Nanjing, China, 26–29 October 2013.
- Venturini, G.; Volpe, G.; Popescu, M. Slot Water Jacket Cooling System for Traction Electrical Machines with Hairpin Windings: Analysis and Comparison. In Proceedings of the 2021 IEEE International Electric Machines & Drives Conference (IEMDC), Hartford, CT, USA, 17–20 May 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Schiefer, M.; Doppelbauer, M. Indirect slot cooling for high-power-density machines with concentrated winding. In Proceedings of the 2015 IEEE International Electric Machines & Drives Conference (IEMDC), Coeur d’Alene, ID, USA, 10–13 May 2015; pp. 1820–1825. [Google Scholar] [CrossRef]
- Gai, Y.; Kimiabeigi, M.; Chong, Y.C.; Widmer, J.D.; Goss, J.; SanAndres, U.; Steven, A.; Staton, D.A. On the Measurement and Modeling of the Heat Transfer Coefficient of a Hollow-Shaft Rotary Cooling System for a Traction Motor. IEEE Trans. Ind. Appl. 2018, 54, 5978–5987. [Google Scholar] [CrossRef]
- Gai, Y.; Chong, Y.C.; Adam, H.; Deng, X.; Popescu, M.; Goss, J. Thermal Analysis of an Oil-Cooled Shaft for a 30,000 r/min Automotive Traction Motor. IEEE Trans. Ind. Appl. 2020, 56, 6053–6061. [Google Scholar] [CrossRef]
- Wang, R.; Fan, X.G.; Li, D.; Qu, R. Comparison of two hollow-shaft liquid cooling methods for high speed permanent magnet synchronous machines. In Proceedings of the ECCE2020, Detroit, MI, USA, 11–15 October 2020. [Google Scholar]
- Ismagilov, F.R.; Vavilov, V.E.; Gusakov, D.V. Design Features of Liquid-Cooled Aviation Starter Generators. In Proceedings of the 2018 IEEE International Conference on Electrical Systems for Aircraft, Railway, Ship Propulsion and Road Vehicles & International Transportation Electrification Conference (ESARS-ITEC), Nottingham, UK, 7–9 November 2018; pp. 1–5. [Google Scholar] [CrossRef]
- Zhou, R.; Li, G.J.; Zhu, Z.Q.; Foster, M.P.; Stone, D.A.; Jia, C.J.; McKeever, P. Novel Liquid Cooling Technology for Modular Consequent-Pole PM Machines. In Proceedings of the 2021 IEEE International Electric Machines & Drives Conference (IEMDC), Hartford, CT, USA, 17–20 May 2021; pp. 1–7. [Google Scholar] [CrossRef]
- La Rocca, A.; Fregni, A.; La Rocca, S.; Gerada, C. Numerical Thermal Modelling of Multiphase Spray Cooling of Hairpin Windings. In Proceedings of the 2020 International Conference on Electrotechnical Complexes and Systems (ICOECS), Ufa, Russia, 27–30 October 2020; pp. 1–5. [Google Scholar] [CrossRef]
- Wang, H.; Li, W.; Guo, H.; Yang, J.; Gu, G. Study of large wind power generator with evaporative cooling system. In Proceedings of the ICEMS2013, Busan, Korea, 26–29 October 2013. [Google Scholar]
- Jiang, W.Y.; Jahns, T.M. Coupled electromagnetic–thermal analysis of electric machines including transient operation based on finite-element techniques. IEEE Trans. Ind. Appl. 2015, 51, 1880–1889. [Google Scholar] [CrossRef]
- Zheng, P.; Liu, R.; Thelin, P.; Nordlund, E.; Sadarangani, C. Research on the Cooling System of a 4QT Prototype Machine Used for HEV. IEEE Trans. Energy Convers. 2008, 23, 61–67. [Google Scholar] [CrossRef]
- Gray, R.F.; Montgomery, L.; Nelson, R.; Pipkin, J.; Joki-Korpela, S.; Caguiat, F. Designing the cooling systems for the world’s most powerful turbogenerator—Olkiluoto unit 3. In Proceedings of the 2006 IEEE Power Engineering Society General Meeting, Montreal, QC, Canada, 18–22 June 2006. [Google Scholar]
- Schrittwieser, M.; Biro, O.; Farnleitner, E.; Kastner, G. Analysis of Temperature Distribution in the Stator of Large Synchronous Machines Considering Heat Conduction and Heat Convection. IEEE Trans. Magn. 2015, 51, 1–4. [Google Scholar] [CrossRef]
- SanAndreas, U.; Gaizka, A.; Poza, J.; Gaizka, U. Design of cooling systems using computational fluid dynamics and analytical thermal models. IEEE Trans. Ind. Electron. 2014, 61, 4383–4391. [Google Scholar] [CrossRef]
- Jokinen, T.; Saari, J. Modelling of the coolant flow with heat flow controlled temperature sources in thermal networks. IEE Proc. Electr. Power Appl. 1997, 144, 338–342. [Google Scholar] [CrossRef]
- Staton, D.; Boglietti, A.; Cavagnino, A. Solving the more difficult aspects of electric motor thermal analysis in small and medium size industrial induction motors. IEEE Trans. Energy Convers. 2005, 20, 620–628. [Google Scholar] [CrossRef]
- Dong, J.; Huang, Y.; Jin, L.; Guo, B.; Lin, H.; Dong, J.; Cheng, M.; Yang, H. Electromagnetic and Thermal Analysis of Open-Circuit Air Cooled High-Speed Permanent Magnet Machines With Gramme Ring Windings. IEEE Trans. Magn. 2014, 50, 8104004. [Google Scholar] [CrossRef]
- Nerg, J.; Rilla, M.; Pyrhonen, J. Thermal Analysis of Radial-Flux Electrical Machines With a High Power Density. IEEE Trans. Ind. Electron. 2008, 55, 3543–3554. [Google Scholar] [CrossRef]
- La Rocca, S.; Pickering, S.J.; Eastwick, C.N.; Gerada, C.; Ronnberg, K. Fluid flow and heat transfer analysis of TEFC machine end regions using more realistic end-winding geometry. J. Eng. 2019, 2019, 3831–3835. [Google Scholar] [CrossRef]
- Mayle, R.; Hess, S.; Hirsch, C.; Van Wolfersdorf, J. Rotor-stator gap flow analysis and experiments. IEEE Trans. Energy Convers. 1998, 13, 101–110. [Google Scholar] [CrossRef]
- Sterling Thermal Technology, An Air-to-Air Cooler for Motors and Generators. Available online: https://www.sterlingtt.com/products-services/single-tube-heat-exchangers/rotating-machine-coolers-caca/ (accessed on 28 November 2021).
- Sterling Thermal Technology, Closed Air Circuit Water Cooled Cooler. Available online: https://www.sterlingtt.com/products-services/single-tube-heat-exchangers/rotating-machine-coolers-cacw/ (accessed on 28 November 2021).
- ABB, ABB’s CAWA Cooler. Available online: https://library.e.abb.com/public/ (accessed on 28 November 2021).
- Nailen, R.L. Understanding the TEWAC Motor. IEEE Trans. Ind. Appl. 1975, IA-11, 350–355. [Google Scholar] [CrossRef]





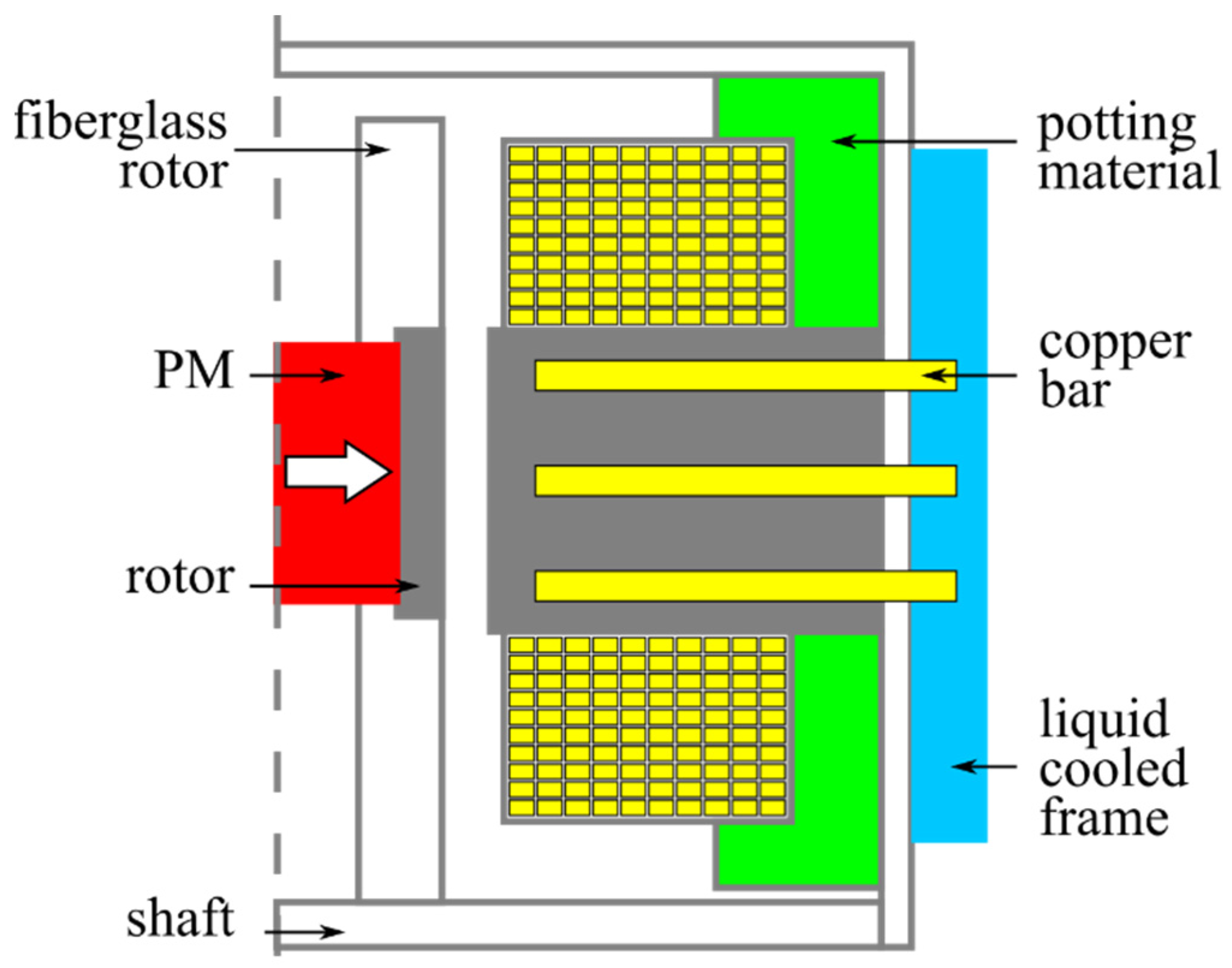

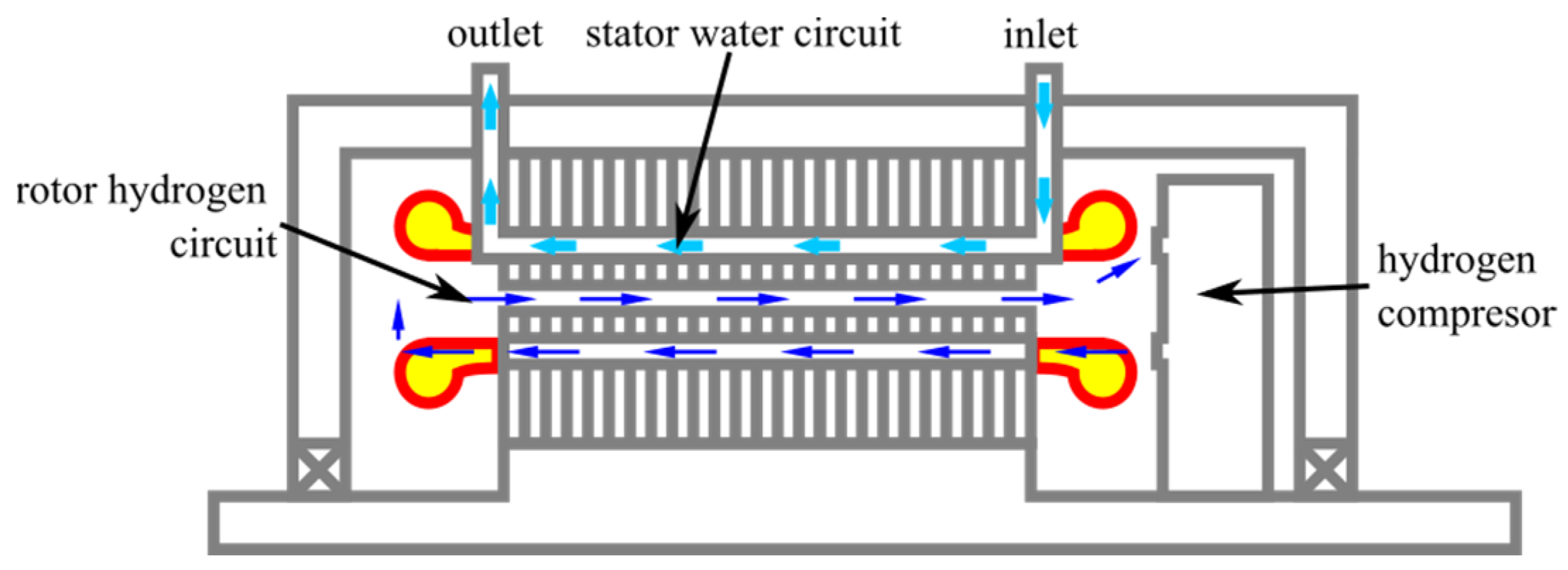
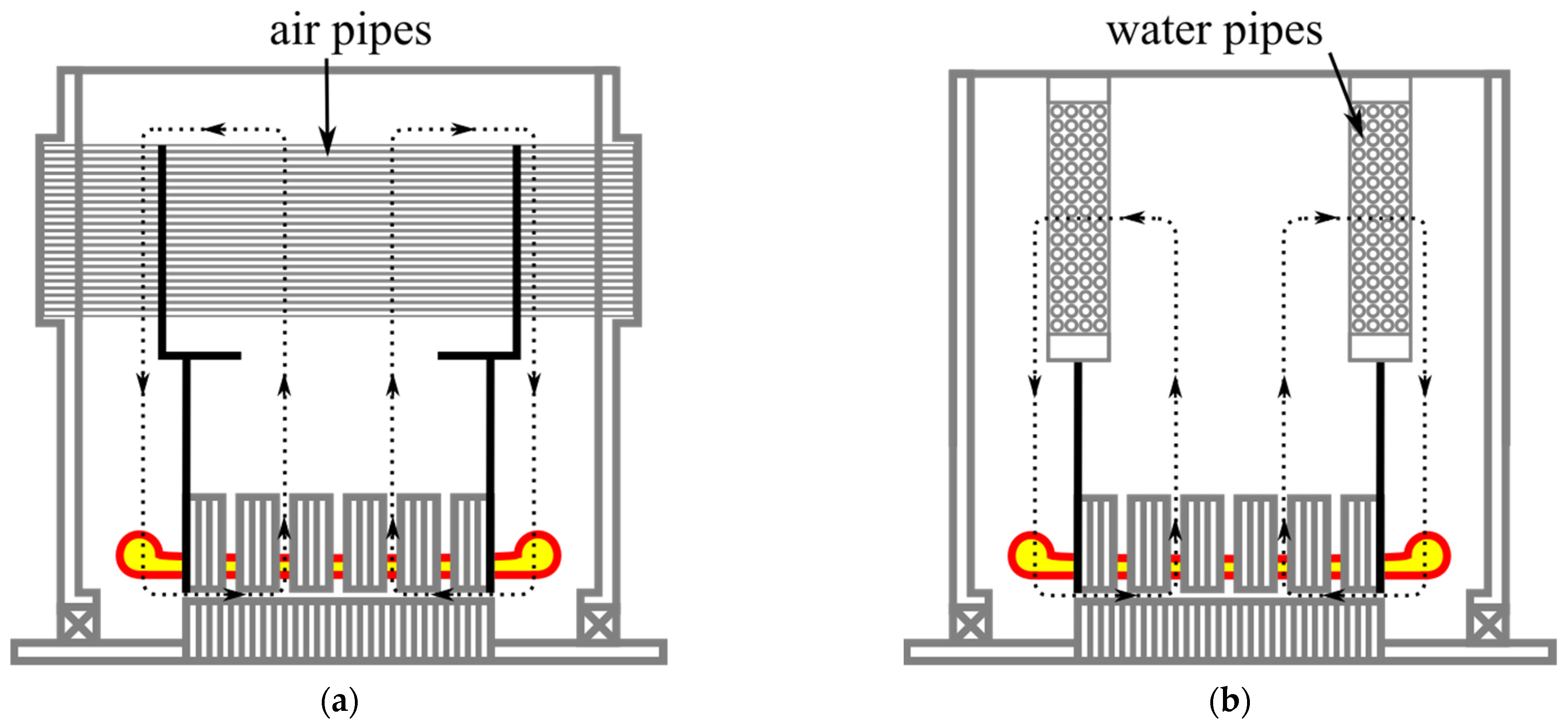
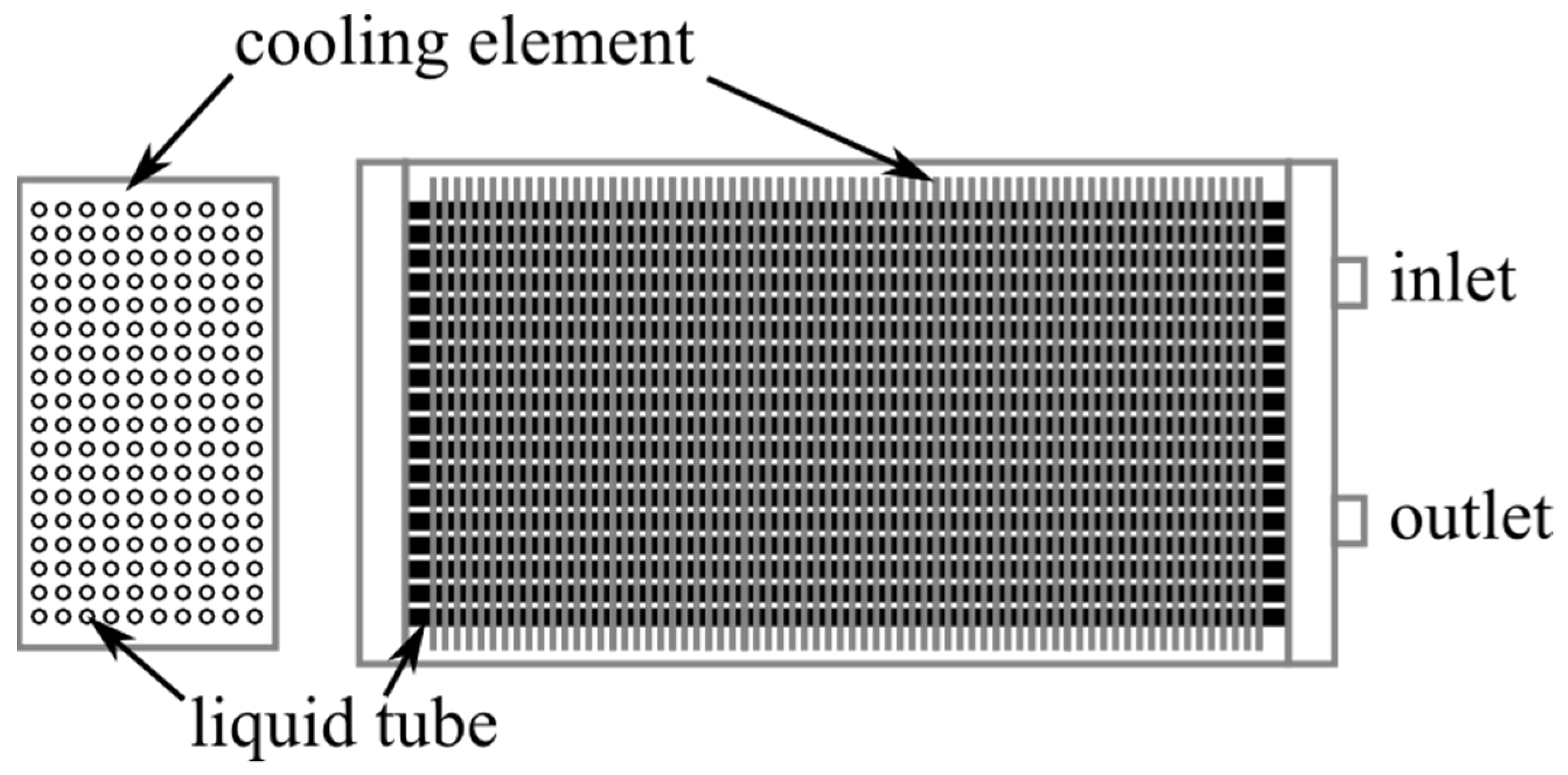
| Reference | Cooling Methods | Applications | Notes |
|---|---|---|---|
| Mueller and Polinder [22] | Passive, forced air and liquid | Wind and marine power generation | Cooling methods are briefly mentioned and not discussed in depth |
| Popescu et al. [27] | Various | Electric traction | |
| Nishanth et al. [28] | Various | Various | |
| Gai et al. [29] | Various | Electric traction | |
| Polikarpova et al. [30] | Liquid through hollow conductors | Direct drive PM generators | Multiple coolant types are compared |
| Boglietti et al. [31] | Various | General | Theory/concepts are provided for thermal design |
| Gronwald and Kern [32] | Various | Electric traction |
| Material | AlNiCo | Ferrite | SmCo | NdFeB |
|---|---|---|---|---|
| Br (T) | 1.35 | 0.4 | 0.8 | 1.2 |
| Hc (kA/m) | 59 | 295 | 2100 | 900 |
| BHmax (kJ/m3) | 60 | 31 | 204 | 325 |
| Tdemagn. (°C) | 525 | 100 | 300 | 150 |
| α (%/°C) | −0.02 | −0.2 | −0.043 | −0.12 |
| β (%/°C) | +0.01 | +0.4 | −0.19 | −0.59 |
| Liquid | ρ (kg/m3) | k (W/m/K) | cp (J/kg/K) |
|---|---|---|---|
| Water | 997 | 0.598 | 4186 |
| Water/Glycol | 1044 | 0.394 | 3620 |
| Oil (PAO) | 1108 | 0.270 | 2633 |
| Method | Performance | Maintenance | Weight | Capacity | Energy | Noise |
|---|---|---|---|---|---|---|
| Passive cooling | ○○○○○ | ○○○○○ | ●●●●● | ○○○○○ | ○○○○○ | ○○○○○ |
| Forced air open loop | ●●○○○ | ●●○○○ | ●●●○○ | ●●○○○ | ●●●●○ | ●●●○○ |
| Forced air closed loop | ●●○○○ | ●●○○○ | ●●●●○ | ●○○○○ | ●●●●● | ●●●●○ |
| Forced water open loop | ●●●○○ | ●●●○○ | ●●○○○ | ●●●○○ | ●●○○○ | ●○○○○ |
| Forced water closed loop | ●●●○○ | ●●○○○ | ●●●○○ | ●●○○○ | ●●●○○ | ●○○○○ |
| Flooded stator | ●●●●○ | ●●●●○ | ●●●○○ | ●●●○○ | ●●○○○ | ●○○○○ |
| Oil spray | ●●●●○ | ●●●●○ | ●●○○○ | ●●●○○ | ●●○○○ | ●○○○○ |
| Multiphase water | ●●●●○ | ●●●○○ | ●●○○○ | ●●●●○ | ●○○○○ | ●○○○○ |
| Hybrid (air, water) | ●●●●● | ●●●●● | ●●○○○ | ●●●●● | ●●●○○ | ●●○○○ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taras, P.; Nilifard, R.; Zhu, Z.-Q.; Azar, Z. Cooling Techniques in Direct-Drive Generators for Wind Power Application. Energies 2022, 15, 5986. https://doi.org/10.3390/en15165986
Taras P, Nilifard R, Zhu Z-Q, Azar Z. Cooling Techniques in Direct-Drive Generators for Wind Power Application. Energies. 2022; 15(16):5986. https://doi.org/10.3390/en15165986
Chicago/Turabian StyleTaras, Petrica, Reza Nilifard, Zi-Qiang Zhu, and Ziad Azar. 2022. "Cooling Techniques in Direct-Drive Generators for Wind Power Application" Energies 15, no. 16: 5986. https://doi.org/10.3390/en15165986
APA StyleTaras, P., Nilifard, R., Zhu, Z.-Q., & Azar, Z. (2022). Cooling Techniques in Direct-Drive Generators for Wind Power Application. Energies, 15(16), 5986. https://doi.org/10.3390/en15165986








