Integration of Photovoltaic Electricity with Shallow Geothermal Systems for Residential Microgrids: Proof of Concept and Techno-Economic Analysis with RES2GEO Model
Abstract
1. Introduction
- Depth of the geothermal reservoir: shallow (<300–500 m), intermediate (<3–5 km), deep (further depth levels);
- Generation type: power generation, direct heat use, geothermal heat pumps.
- Evaluation of the soil properties for aiding the SG system design
- Regional mapping—by geographical coordinates and depth
- Evaluation of the regional potential to cover local heating needs
- Evaluation of the legislation and regulations across the EU
- Operational issues—evaluation of thermal SG capacity replenishment and deterioration of underground sites, dynamic operation planning using SG sites as heat storage
- Intensification of the SG heat extraction via innovative heat exchanger design and borehole design adjustment
- Maintenance and reliability issues related to scaling
- Techno-economic-environmental feasibility evaluation of SG for district heating with heat pumps.
2. Materials and Methods
2.1. Modelling of a Shallow Geothermal Reservoir and a Borehole Heat Exchanger
2.2. Techno-Economic Analysis and Levelised Cost of Electricity and Heating Energy
3. Setup of the Hypothetical Case Study
4. Results and Discussion
4.1. Preliminary Testing
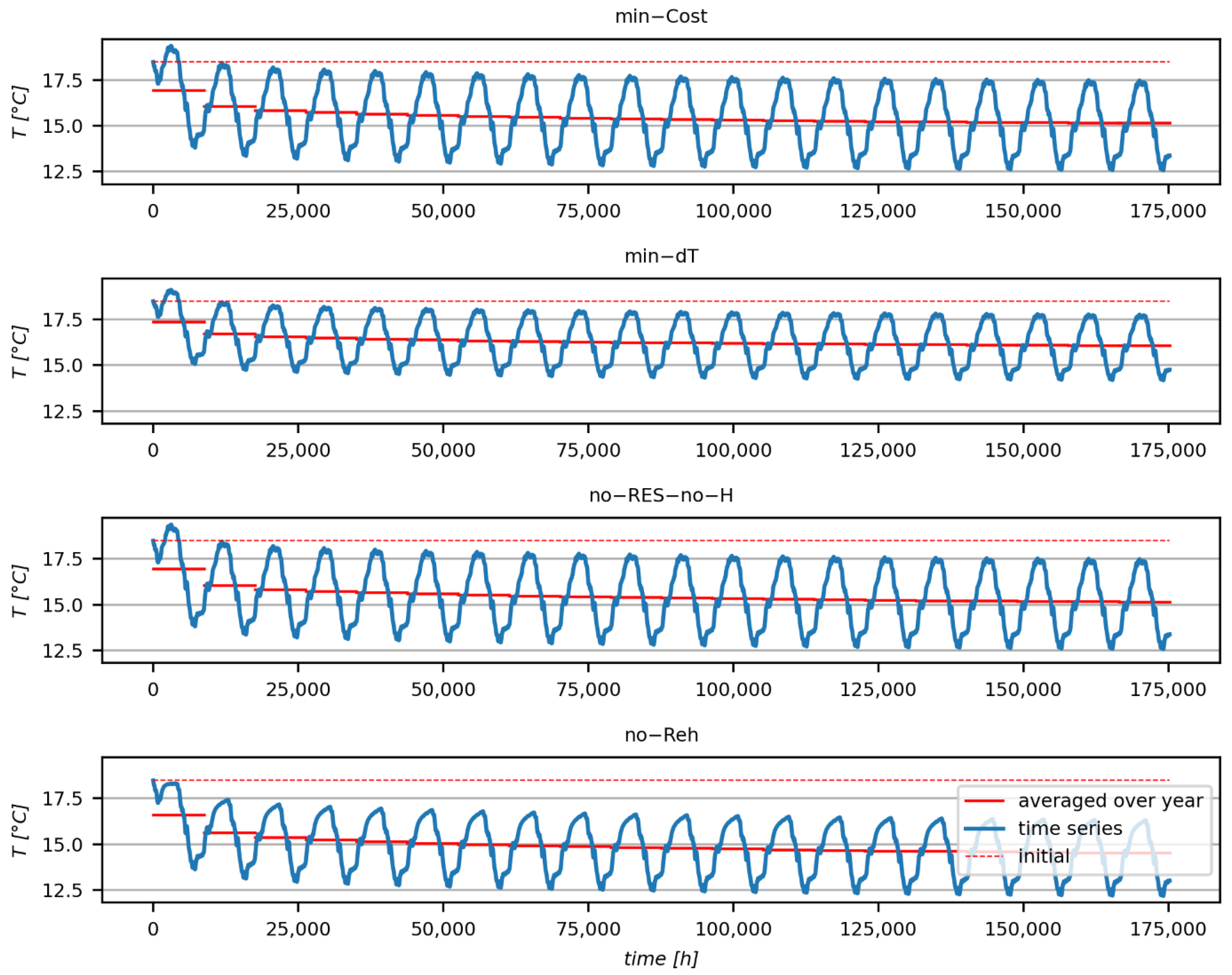
4.2. Time Series Analysis for the Selected Cases
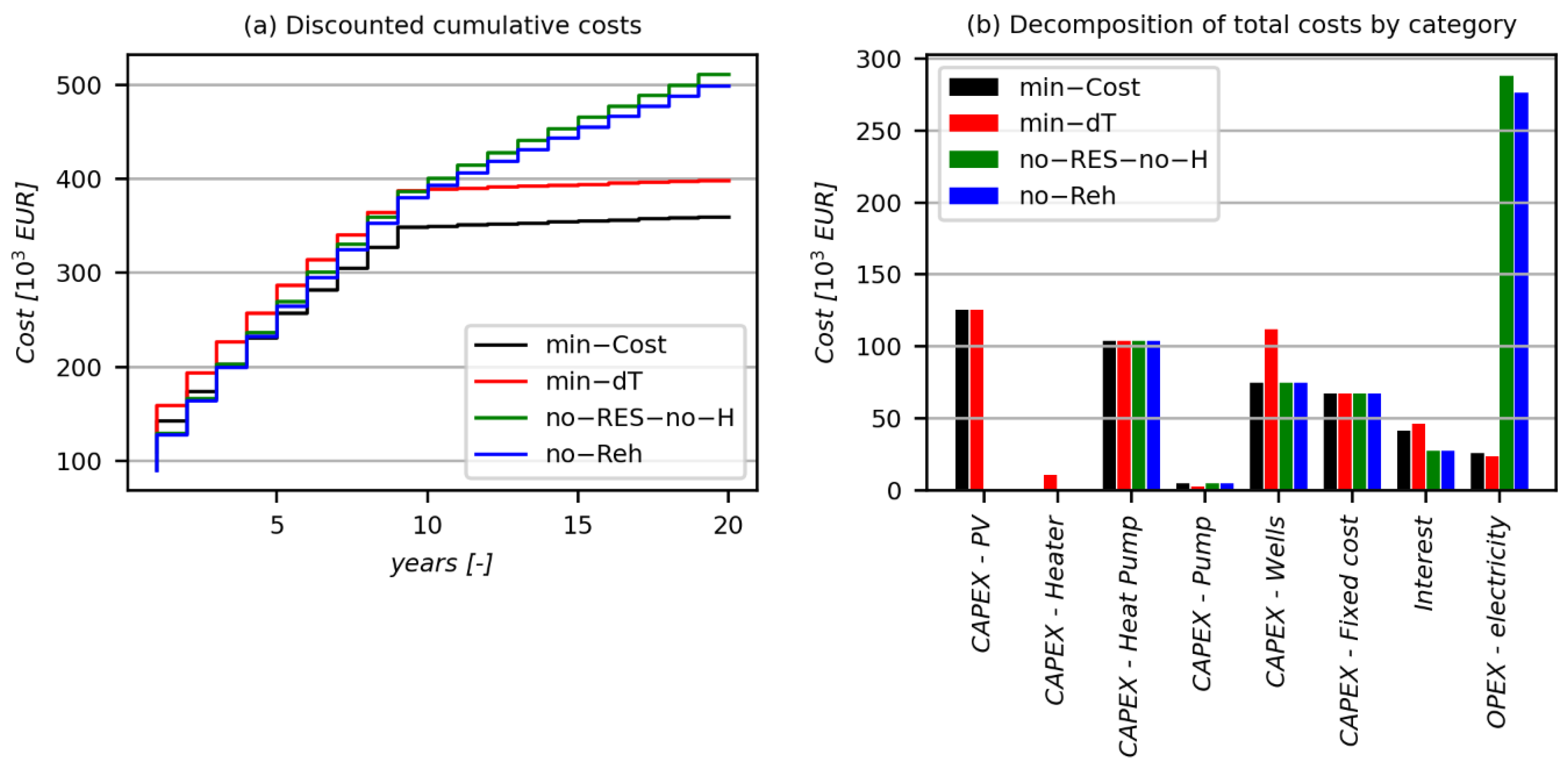
4.3. Techno-Economic Analysis Based on Long-Term Simulation Results
4.4. Mitigation of CO2 Emissions
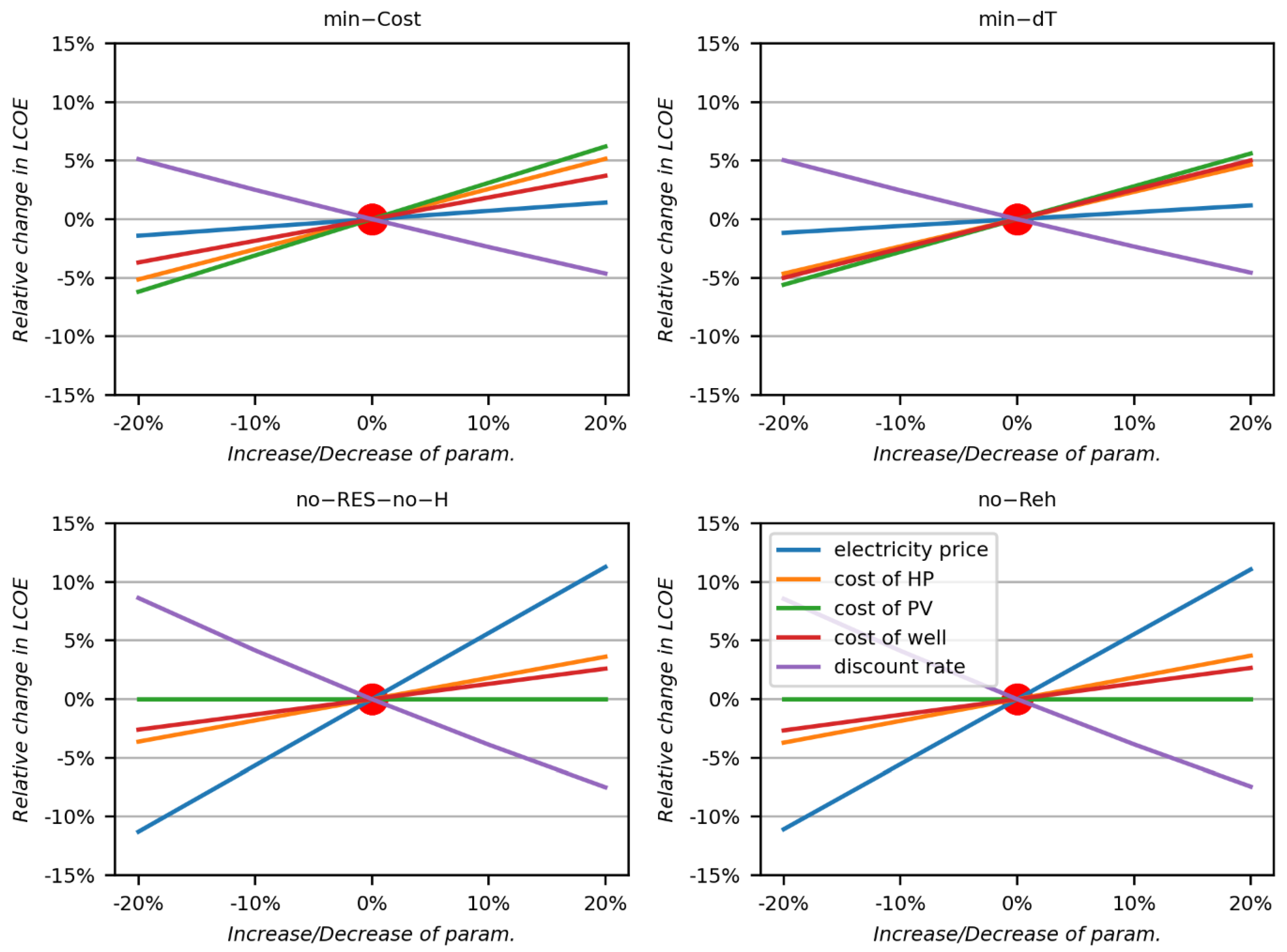
4.5. Sensitivity Analysis Based on the Uncertainty of Input Costs
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| A | area, m2 |
| C | total cost, EUR |
| c | specific heat capacity, J/kgK |
| d | discount rate, % |
| e | electric energy, kWh |
| H | height of calculation domain (reservoir), m |
| Inv | total investment, EUR |
| k | overall heat transfer coefficient, W/m2K |
| L | length, m |
| m | mass |
| N | number of years in analysis, - |
| p, P | price, EUR/kWh |
| q | thermal energy, kWh |
| r | radius, m |
| T | temperature, K or °C |
| t | time, h |
| V | volume, m3 |
| x | ratio, - |
| Subscripts | |
| B | boundary |
| cool | cooling |
| d | discounted |
| E, N, S, W | east, north, south and west orientation |
| el | electricity |
| Eq | equity |
| dem | demand |
| exp | exported |
| fix | fixed part of cost (not elsewhere specified) |
| H | heater |
| HP | heat pump |
| i | specific year |
| imp | imported |
| m2 | per sq. meter |
| net | netto |
| P | pump |
| R | reservoir |
| th | thermal |
| tot | total |
| w | water |
| Greek letters | |
| α | heat transfer coefficient, W/m2K |
| δ | distance, m |
| λ | thermal conductivity, W/mK |
| ρ | density, kg/m3 |
| ϕ | heat flux, W |
Abbreviations
| BHE | Borehole Heat Exchanger |
| CAPEX | Capital Expenditures |
| CHP | Combined Heat and Power |
| COP | Coefficient Of Performance (heat pump) |
| DSO | Distribution System Operator |
| EU | European Union |
| EUR | € (currency) |
| ELT | Entering Load Temperature (heat pump) |
| EST | Entering Source Temperature (heat pump) |
| GHG | Greenhouse Gas |
| GIS | Geographical Information Systems |
| GSHP | Ground Source Heat Pump |
| IPMT | Interest Payment |
| LCOE | Levelized Cost of Energy |
| LLT | Leaving Load Temperature (heat pump) |
| LST | Leaving Source Temperature (heat pump) |
| OPEX | Operating Expenses |
| PPMT | Principal Payment |
| PV | Photovoltaic |
| RES2GEO | Renewable Energy Sources to Geothermal |
| SG | Shallow Geothermal |
| TRT | Thermal Response Test |
References
- Klemeš, J.J. Assessing and Measuring Environmental Impact and Sustainability; Butterworth-Heinemann/Elsevier: Oxford, UK; Waltham, MA, USA, 2015; ISBN 978-0-12-799968-5. [Google Scholar]
- Perry, S.; Klemeš, J.; Bulatov, I. Integrating Waste and Renewable Energy to Reduce the Carbon Footprint of Locally Integrated Energy Sectors. Energy 2008, 33, 1489–1497. [Google Scholar] [CrossRef]
- Mohammad Rozali, N.E.; Wan Alwi, S.R.; Abdul Manan, Z.; Klemeš, J.J.; Hassan, M.Y. Optimal Sizing of Hybrid Power Systems Using Power Pinch Analysis. J. Clean. Prod. 2014, 71, 158–167. [Google Scholar] [CrossRef]
- EGEC Geothermal. Available online: https://www.egec.org/the-geothermal-energy-market-grows-exponentially-but-needs-the-right-market-conditions-to-thrive/ (accessed on 2 December 2020).
- Pérez, R.E. European Federation of Geologists. Available online: https://eurogeologists.eu/esteban-shallow-geothermal-energy-geological-energy-for-the-ecological-transition-and-its-inclusion-in-european-and-national-energy-policies/ (accessed on 1 December 2020).
- Dalla Longa, F.; Nogueira, L.P.; Limberger, J.; van Wees, J.-D.; van der Zwaan, B. Scenarios for geothermal energy deployment in Europe. Energy 2020, 206, 118060. [Google Scholar] [CrossRef]
- Kurevija, T. Analysis of potentials of shallow geothermal resources in heat pump systems in the city of Zagreb. Goriva Maz. 2008, 47, 373–390. [Google Scholar]
- Macenić, M.; Kurevija, T.; Strpić, K. Systematic review of research and utilisation of shallow geothermal energy in Croatia. Min. Geol. Pet. Eng. Bull. 2018, 33, 37–46. [Google Scholar] [CrossRef]
- Hajovsky, R.; Vojcinak, P.; Pies, M.; Koziorek, J. Thermal Response Test (TRT)—System for Measurement of Thermal Response of Rock Massif. IFAC Proc. Vol. 2013, 46, 126–131. [Google Scholar] [CrossRef]
- Bu, X.; Ran, Y.; Zhang, D. Experimental and Simulation Studies of Geothermal Single Well for Building Heating. Renew. Energy 2019, 143, 1902–1909. [Google Scholar] [CrossRef]
- He, Y.; Bu, X. A Novel Enhanced Deep Borehole Heat Exchanger for Building Heating. Appl. Therm. Eng. 2020, 178, 115643. [Google Scholar] [CrossRef]
- Rybach, L.; Mégel, T.; Eugster, W. At what time scale are geothermal resources renewable? In Proceedings of the World Geothermal Congress, Kyushu, Tohoku, Japan, 28 May–10 June 2000; Available online: https://www.geothermal-energy.org/pdf/IGAstandard/WGC/2000/R0900.PDF (accessed on 19 March 2021).
- Seyam, S.; Dincer, I.; Agelin-Chaab, M. Thermodynamic analysis of a hybrid energy system using geothermal and solar energy sources with thermal storage in a residential building. Energy Storage 2019. [Google Scholar] [CrossRef]
- The World Bank. Available online: https://data.worldbank.org/topic/urban-development (accessed on 1 December 2020).
- European Commission. 2030 Climate & Energy Framework. Available online: https://ec.europa.eu/clima/policies/strategies/2030_en (accessed on 1 December 2020).
- Kerry, S.; Baume, O.; Caruso, G.; Ulrich, L. GIS-based modelling of shallow geothermal energy potential for CO2. Renew. Energy 2016, 86, 1023–1036. [Google Scholar] [CrossRef]
- Walch, A.; Mohajeri, N.; Gudmundsson, A.; Scartezzini, J.-L. Quantifying the Technical Geothermal Potential from Shallow Borehole Heat Exchangers at Regional Scale. Renew. Energy 2021, 165, 369–380. [Google Scholar] [CrossRef]
- Ramos-Escudero, A.; García-Cascales, M.S.; Cuevas, J.M.; Sanner, B.; Urchueguía, J.F. Spatial Analysis of Indicators Affecting the Exploitation of Shallow Geothermal Energy at European Scale. Renew. Energy 2021, 167, 266–281. [Google Scholar] [CrossRef]
- Perković, L.; Mikulčić, H.; Pavlinek, L.; Wang, X.; Vujanović, M.; Tan, H.; Baleta, J.; Duić, N. Coupling of cleaner production with a day-ahead electricity market: A hypothetical case study. J. Clean. Prod. 2017, 143, 1011–1020. [Google Scholar] [CrossRef]
- Urbaniec, K.; Mikulčić, H.; Duić, N. SDEWES 2014—Sustainable Development of Energy, Water and Environment Systems. J. Clean. Prod. 2016, 130, 1–11. [Google Scholar] [CrossRef]
- Perković, L.; Mikulčić, H.; Duić, N. Multi-objective optimisation of a simplified factory model acting as a prosumer on the electricity market. J. Clean. Prod. 2018, 167, 1438–1449. [Google Scholar] [CrossRef]
- Mikulčić, H.; Ridjan Skov, I.; Dominković, D.F.; Wan Alwi, S.R.; Manan, Z.A.; Tan, R.; Duić, N.; Hidayah Mohamad, S.N.; Wang, X. Flexible Carbon Capture and Utilisation technologies in future energy systems and the utilisation pathways of captured CO2. Renew. Sustain. Energy Rev. 2019, 114, 109338. [Google Scholar] [CrossRef]
- Wood, E. Microgrid Knowledge. Available online: https://microgridknowledge.com/microgrid-defined/ (accessed on 1 December 2020).
- Ali, A.; Li, W.; Hussain, R.; He, X.; Williams, B.W.; Memon, A.H. Overview of Current Microgrid Policies, Incentives and Barriers in the European Union, United States and China. Sustainability 2017, 9, 1146. [Google Scholar] [CrossRef]
- Al-Khoury, R. Computational Modeling of Shallow Geothermal Systems; CRC Press: Boca Raton, FL, USA, 2012; ISBN 978-0-429-16576-4. [Google Scholar]
- Vieira, A.; Alberdi-Pagola, M.; Christodoulides, P.; Javed, S.; Loveridge, F.; Nguyen, F.; Cecinato, F.; Maranha, J.; Florides, G.; Prodan, I.; et al. Characterisation of Ground Thermal and Thermo-Mechanical Behaviour for Shallow Geothermal Energy Applications. Energies 2017, 10, 2044. [Google Scholar] [CrossRef]
- Casasso, A.; Sethi, R.G. POT: A Quantitative Method for the Assessment and Mapping of the Shallow Geothermal Potential. Energy 2016, 106, 765–773. [Google Scholar] [CrossRef]
- Ondreka, J.; Rüsgen, M.I.; Stober, I.; Czurda, K. GIS-Supported Mapping of Shallow Geothermal Potential of Representative Areas in South-Western Germany—Possibilities and Limitations. Renew. Energy 2007, 32, 2186–2200. [Google Scholar] [CrossRef]
- Tissen, C.; Menberg, K.; Benz, S.A.; Bayer, P.; Steiner, C.; Götzl, G.; Blum, P. Identifying Key Locations for Shallow Geothermal Use in Vienna. Renew. Energy 2020, 167, 1–19. [Google Scholar] [CrossRef]
- Dalla Santa, G.; Galgaro, A.; Sassi, R.; Cultrera, M.; Scotton, P.; Mueller, J.; Bertermann, D.; Mendrinos, D.; Pasquali, R.; Perego, R.; et al. An Updated Ground Thermal Properties Database for GSHP Applications. Geothermics 2020, 85, 101758. [Google Scholar] [CrossRef]
- Somogyi, V.; Sebestyén, V.; Nagy, G. Scientific Achievements and Regulation of Shallow Geothermal Systems in Six European Countries—A Review. Renew. Sustain. Energy Rev. 2017, 68, 934–952. [Google Scholar] [CrossRef]
- Cai, S.; Li, X.; Zhang, M.; Fallon, J.; Li, K.; Cui, T. An Analytical Full-Scale Model to Predict Thermal Response in Boreholes with Groundwater Advection. Appl. Therm. Eng. 2020, 168, 114828. [Google Scholar] [CrossRef]
- Meng, B.; Vienken, T.; Kolditz, O.; Shao, H. Evaluating the Thermal Impacts and Sustainability of Intensive Shallow Geothermal Utilisation on a Neighborhood Scale: Lessons Learned from a Case Study. Energy Convers. Manag. 2019, 199, 111913. [Google Scholar] [CrossRef]
- Vienken, T.; Kreck, M.; Dietrich, P. Monitoring the Impact of Intensive Shallow Geothermal Energy Use on Groundwater Temperatures in a Residential Neighborhood. Geotherm. Energy 2019, 7, 8. [Google Scholar] [CrossRef]
- Lyu, W.; Li, X.; Yan, S.; Jiang, S. Utilizing Shallow Geothermal Energy to Develop an Energy Efficient HVAC System. Renew. Energy 2020, 147, 672–682. [Google Scholar] [CrossRef]
- Sofyan, S.E.; Hu, E.; Kotousov, A.; Riayatsyah, T.M.I.; Thaib, R. Mathematical Modelling and Operational Analysis of Combined Vertical–Horizontal Heat Exchanger for Shallow Geothermal Energy Application in Cooling Mode. Energies 2020, 13, 6598. [Google Scholar] [CrossRef]
- Kurevija, T.; Macenić, M.; Borović, S. Impact of Grout Thermal Conductivity on the Long-Term Efficiency of the Ground-Source Heat Pump System. Sustain. Cities Soc. 2017, 31, 1–11. [Google Scholar] [CrossRef]
- Sikos, L.; Klemeš, J. Reliability, Availability and Maintenance Optimisation of Heat Exchanger Networks. Appl. Therm. Eng. 2010, 30, 63–69. [Google Scholar] [CrossRef]
- Choi, H.; Kim, J.; Shim, B.O.; Kim, D. Characterization of Aquifer Hydrochemistry from the Operation of a Shallow Geothermal System. Water 2020, 12, 1377. [Google Scholar] [CrossRef]
- Kljajić, M.V.; Anđelković, A.S.; Hasik, V.; Munćan, V.M.; Bilec, M. Shallow Geothermal Energy Integration in District Heating System: An Example from Serbia. Renew. Energy 2020, 147, 2791–2800. [Google Scholar] [CrossRef]
- Fan, Y.V.; Varbanov, P.S.; Klemeš, J.J.; Romanenko, S.V. Urban and Industrial Symbiosis for Circular Economy: Total EcoSite Integration. J. Environ. Manag. 2021, 279, 111829. [Google Scholar] [CrossRef]
- Python Software Foundation. Python Language Reference, Version 3.8. Available online: www.python.org (accessed on 10 February 2021).
- ECOFOREST Heat Pumps Technical Data. Available online: www.ecoforest.es (accessed on 1 December 2020).
- Connolly, D.; Drysdale, D.; Hansen, K.; Novosel, T. Creating Hourly Profiles to Model both Demand and Supply. Background Report 2, Stratego Project. Available online: https://heatroadmap.eu/wp-content/uploads/2018/09/STRATEGO-WP2-Background-Report-2-Hourly-Distributions-1.pdf (accessed on 19 March 2021).
- Photovoltaic Geographical Information System. Available online: Ec.europa.eu/jrc/en/pvgis (accessed on 10 March 2021).
- Eurostat. Available online: https://ec.europa.eu/eurostat. (accessed on 1 December 2020).
- Chew, K.H.; Klemeš, J.J.; Wan Alwi, S.R.; Manan, Z.A. Process Modifications to Maximise Energy Savings in Total Site Heat Integration. Appl. Therm. Eng. 2015, 78, 731–739. [Google Scholar] [CrossRef]
- Klemeš, J.J.; Varbanov, P.S. Process Intensification and Integration: An Assessment. Clean Technol. Environ. Policy 2013, 15, 417–422. [Google Scholar] [CrossRef]
- Baleta, J.; Mikulčić, H.; Klemeš, J.J.; Urbaniec, K.; Duić, N. Integration of Energy, Water and Environmental Systems for a Sustainable Development. J. Clean. Prod. 2019, 215, 1424–1436. [Google Scholar] [CrossRef]
- Ministry of Environment and Energy of the Republic of Croatia, Annual Energy Report—Energy in Croatia. 2018. Available online: www.eihp.hr/wp-content/uploads/2020/04/Energija2018.pdf (accessed on 11 March 2021).
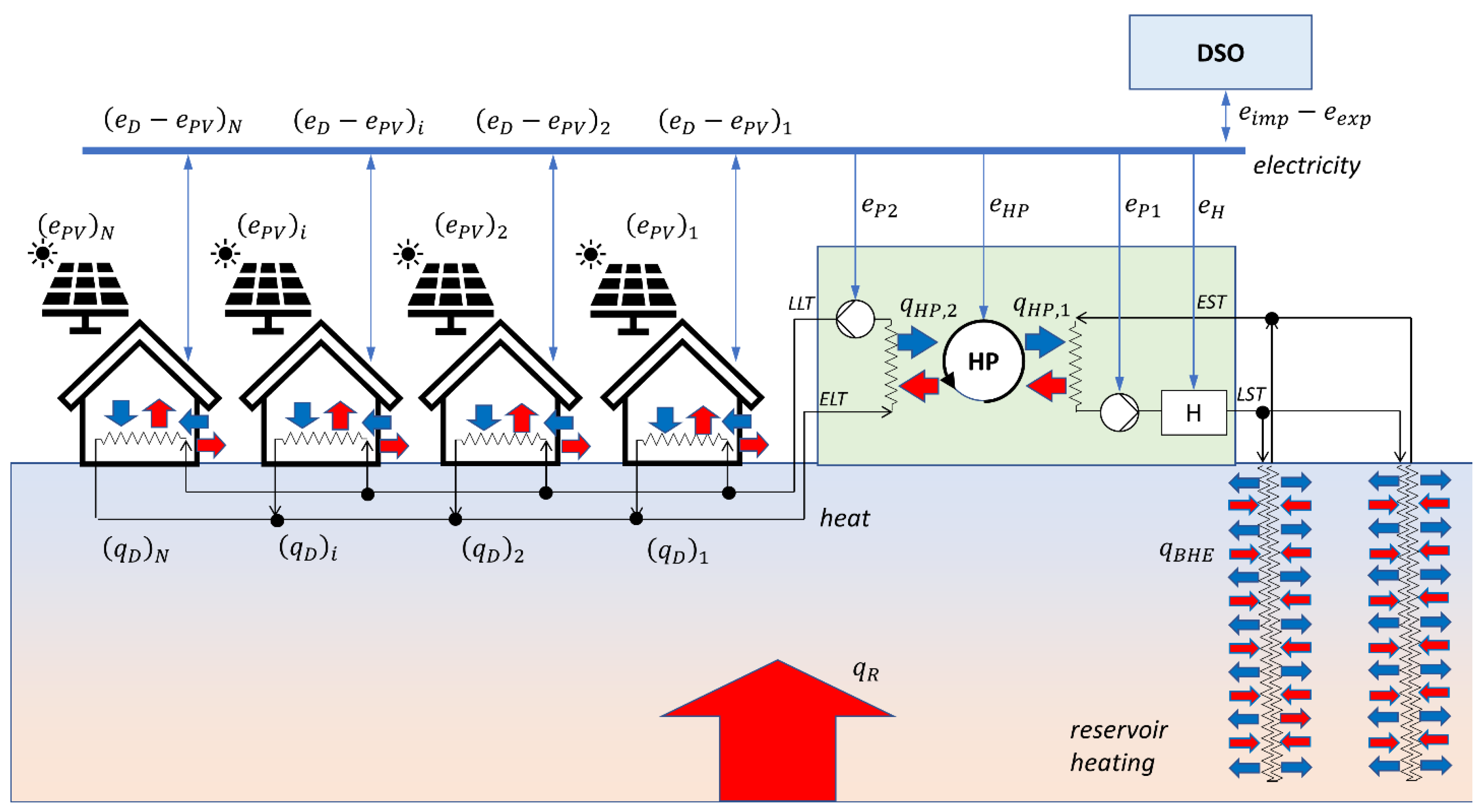

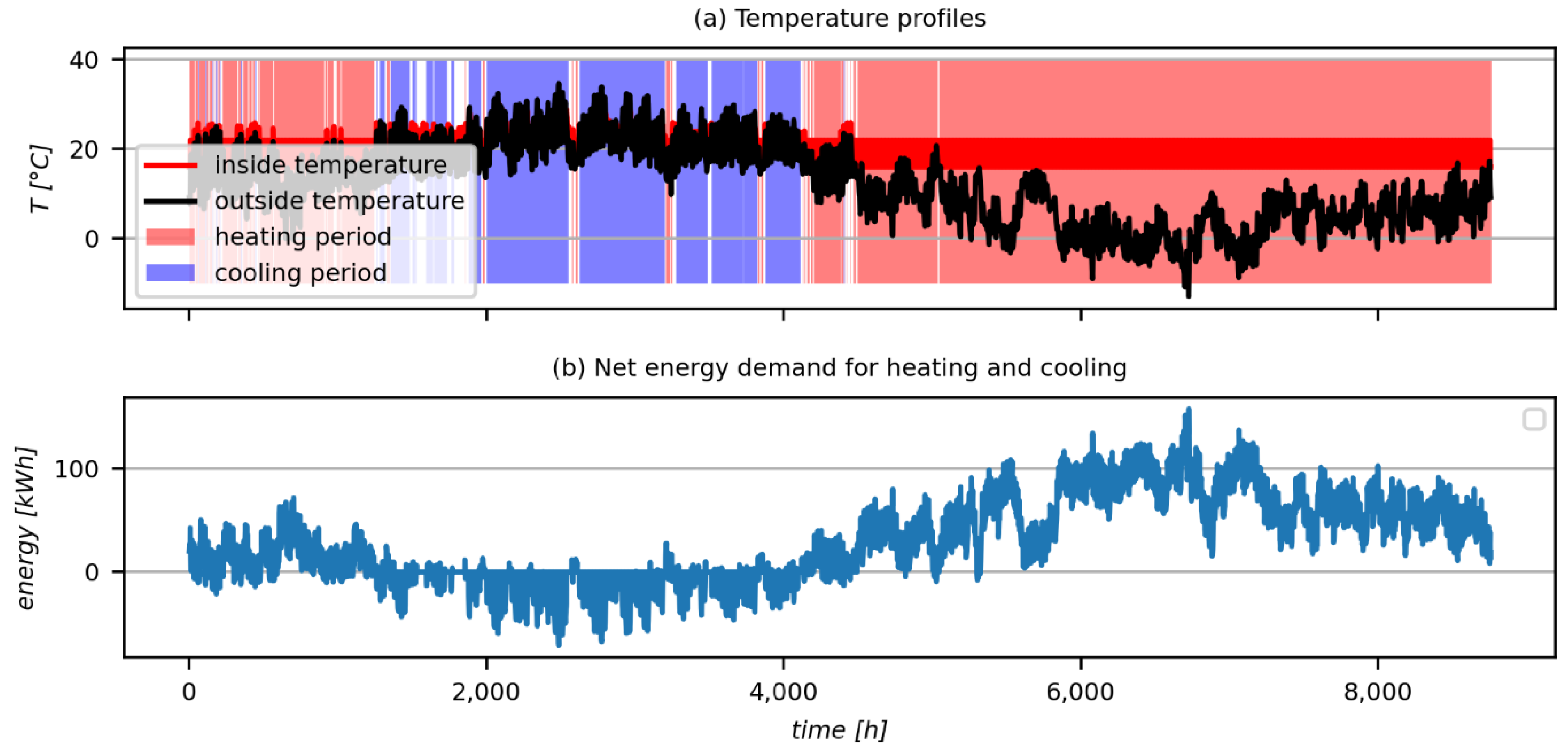
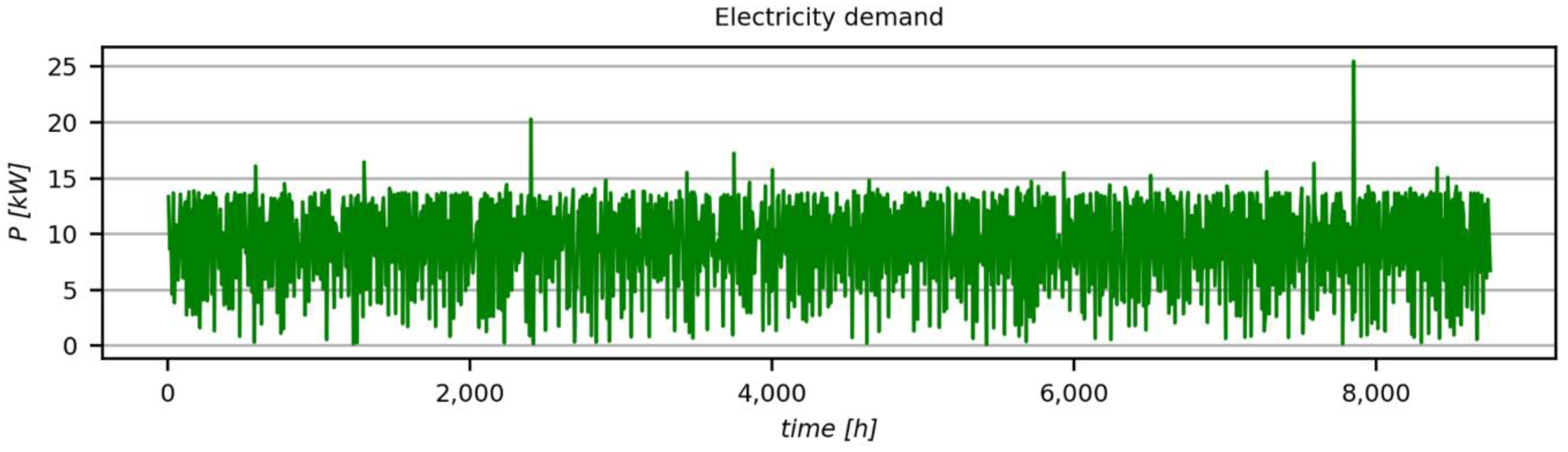
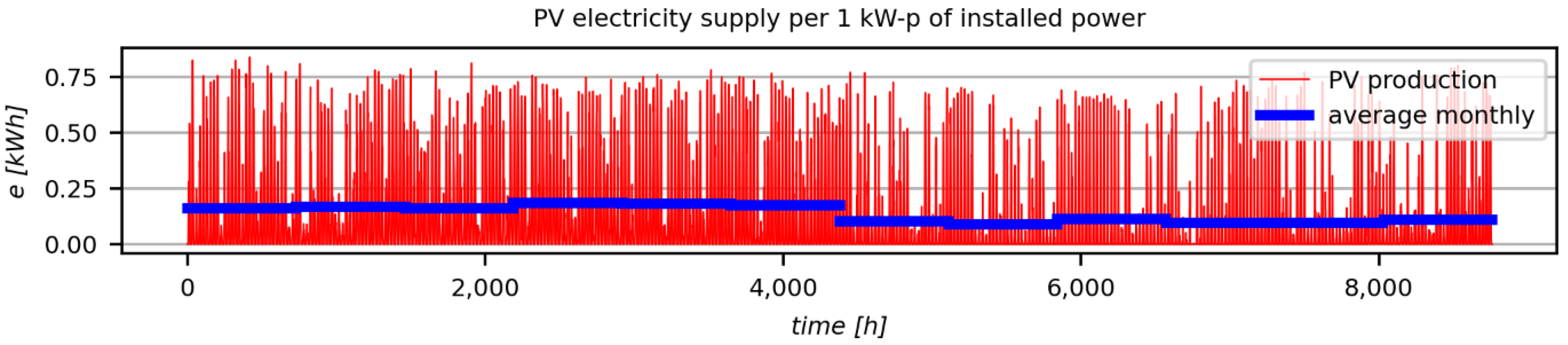
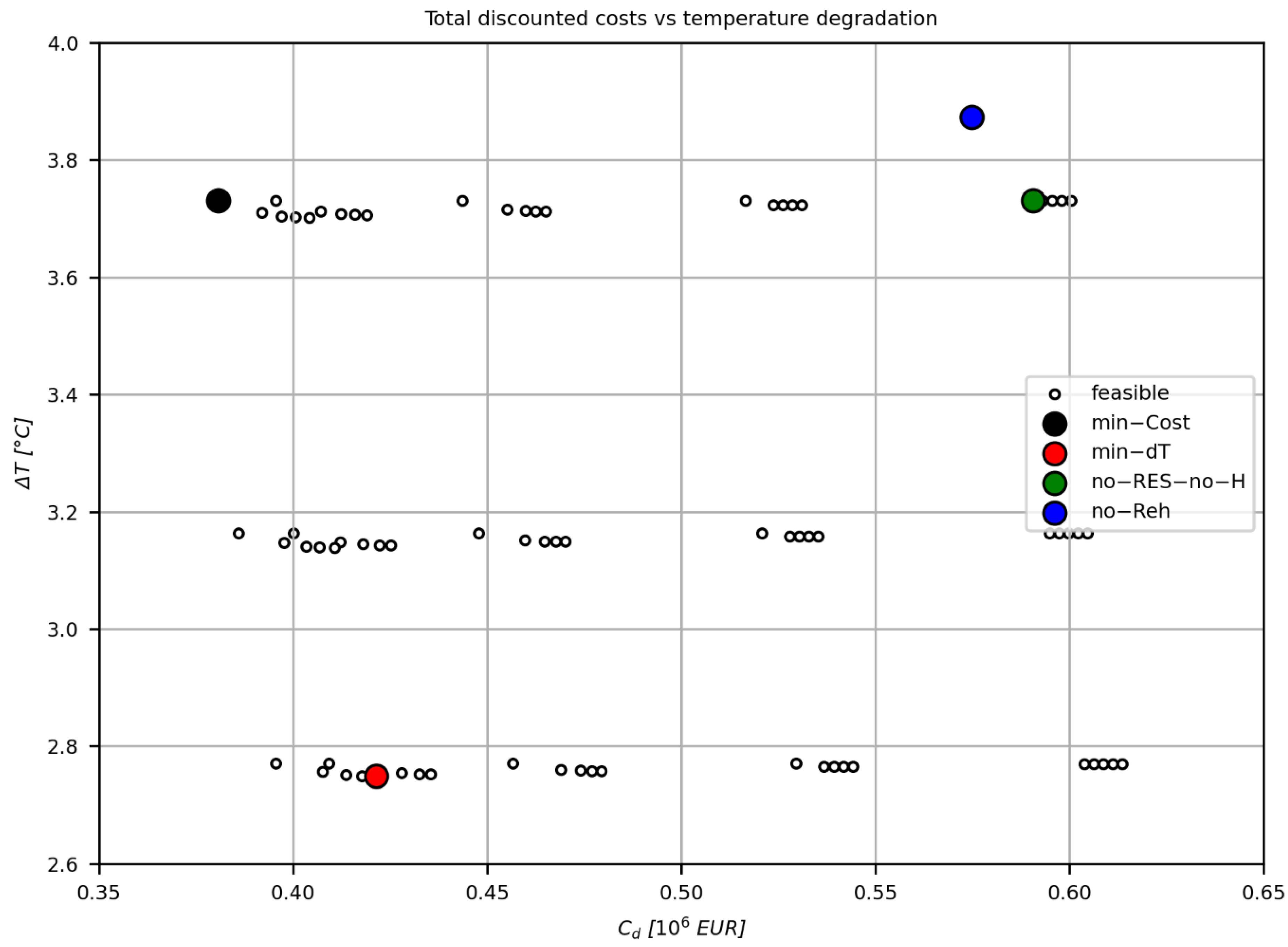
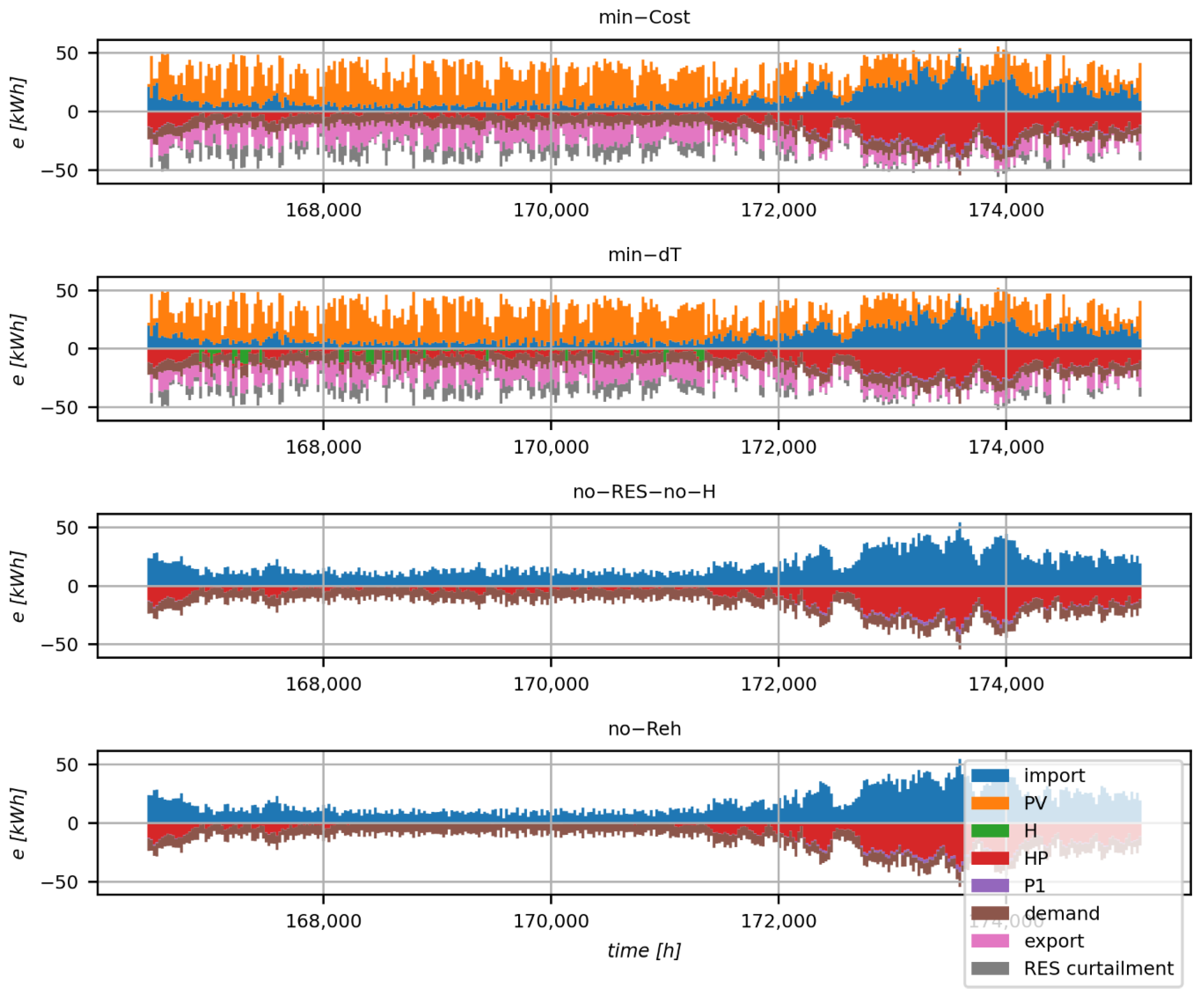
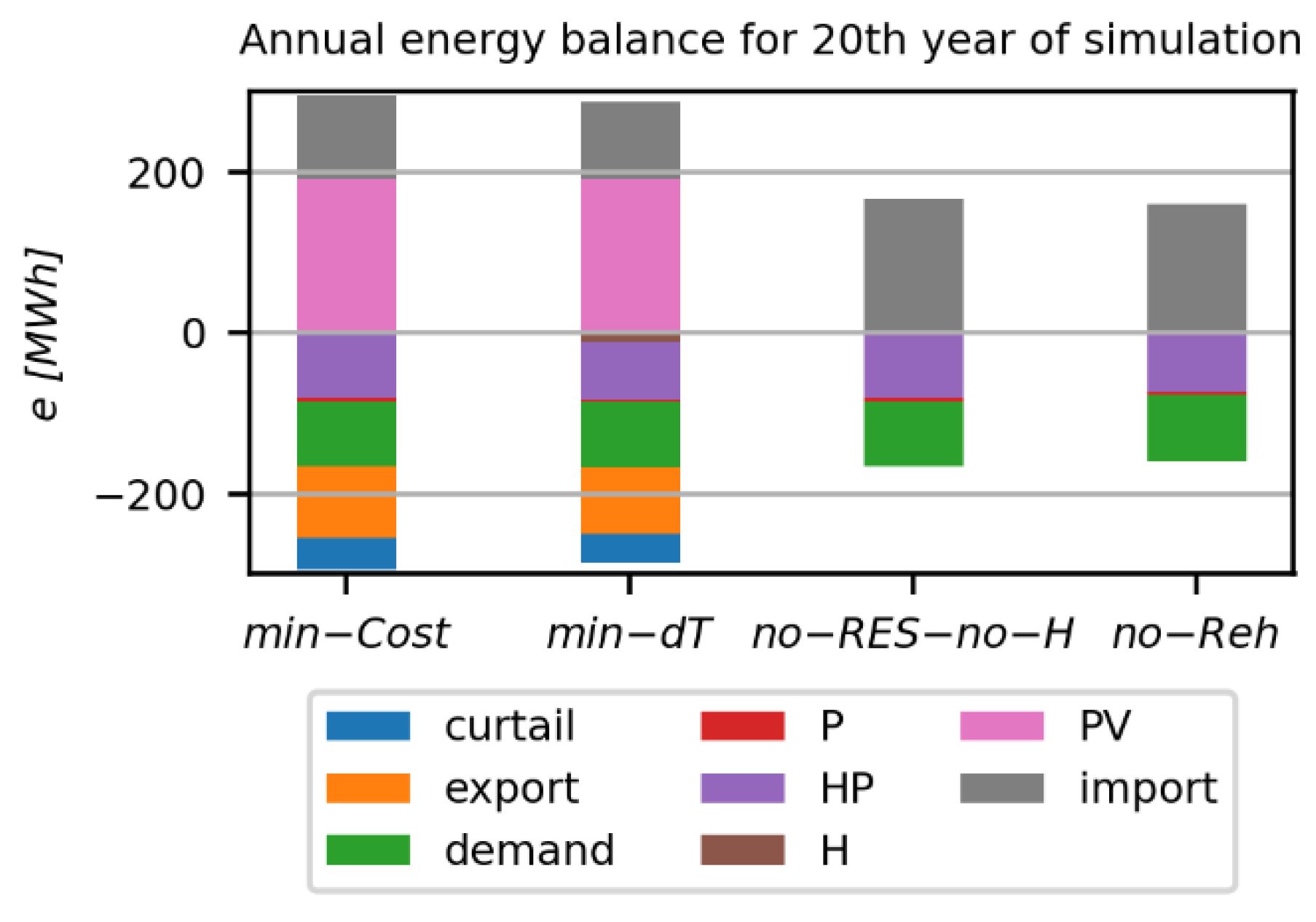
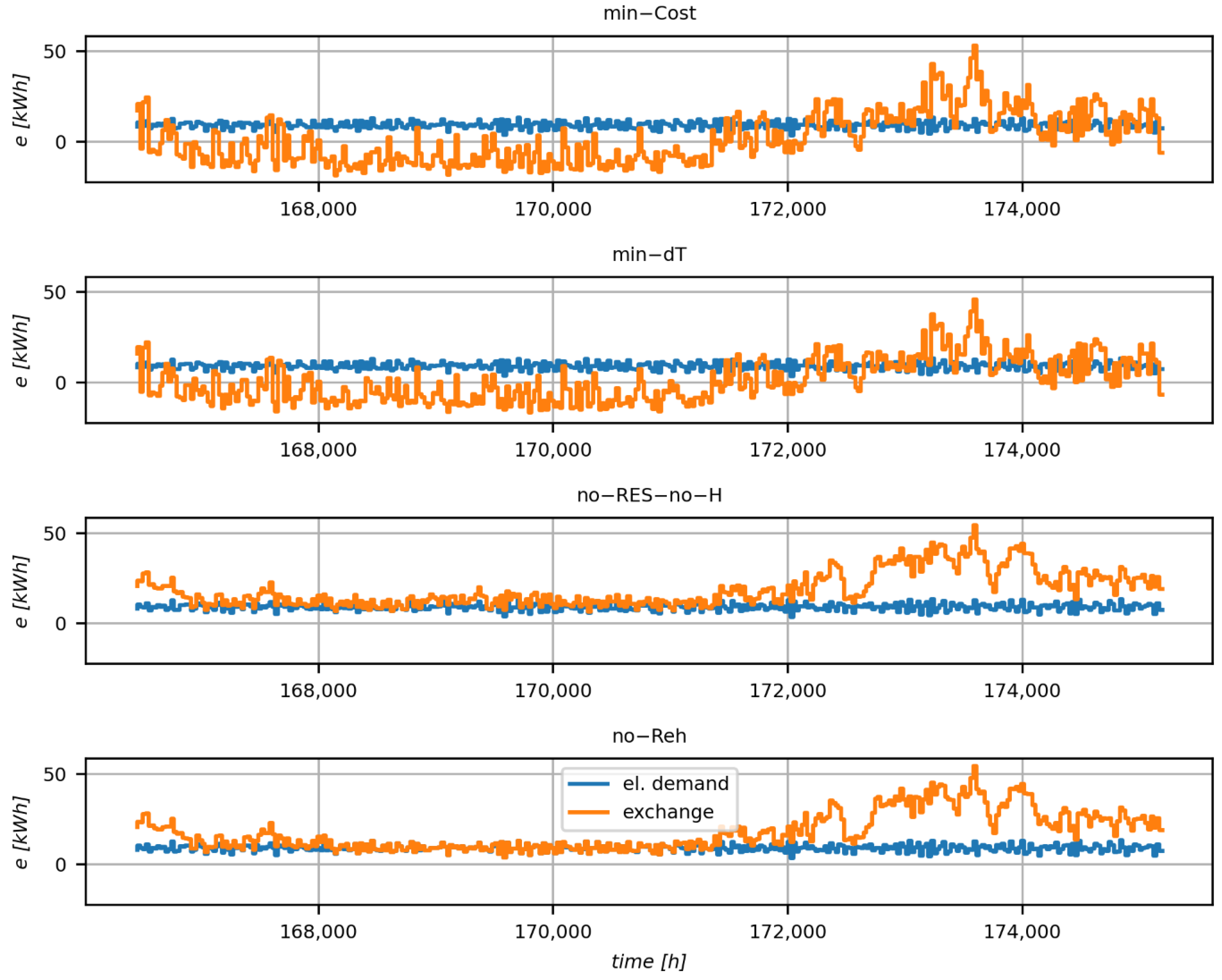
| Parameters | Heating Demand (Thermal) | Cooling Demand (Thermal) | Electricity Demand |
|---|---|---|---|
| Total annual energy demand, MWh/y | 281 | 30 | 81 |
| Annual energy demand per area, kWh/y/m2 | 56.2 | 6.0 | 16.2 |
| Maximum power demand, kW | 158 | 71 | 31 |
| Design Case | HP Reheat | PPV, kW | PH, kW | Number of Wells- |
|---|---|---|---|---|
| min-Cost | Yes | 160 | 0 | 16 |
| min-dT | Yes | 160 | 80 | 24 |
| no-RES-no-H | Yes | 0 | 0 | 16 |
| no-Reh | No | 0 | 0 | 16 |
| Design Case | Net Electricity Exchange, MWh | Curtailment of the PV, MWh | An Overall Temperature Drop of the Reservoir, °C | LCOE, EUR/MWh |
|---|---|---|---|---|
| min-Cost | 296 | 796 | 3.3 | 45 |
| min-dT | 268 | 748 | 2.4 | 50 |
| no-RES-no-H | 3320 | 0 | 3.3 | 65 |
| no-Reh | 3184 | 0 | 4.0 | 64 |
| Design Case | Total Imported Electricity, MWh | Total Emissions from the Grid, tCO2 | Mitigation of Emissions Compared to the Alternative, % |
|---|---|---|---|
| min-Cost | 2080 | 220 | 83 |
| min-dT | 1921 | 203 | 84 |
| no-RES-no-H | 3320 | 351 | 72 |
| no-Reh | 3184 | 337 | 73 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Perković, L.; Leko, D.; Brettschneider, A.L.; Mikulčić, H.; Varbanov, P.S. Integration of Photovoltaic Electricity with Shallow Geothermal Systems for Residential Microgrids: Proof of Concept and Techno-Economic Analysis with RES2GEO Model. Energies 2021, 14, 1923. https://doi.org/10.3390/en14071923
Perković L, Leko D, Brettschneider AL, Mikulčić H, Varbanov PS. Integration of Photovoltaic Electricity with Shallow Geothermal Systems for Residential Microgrids: Proof of Concept and Techno-Economic Analysis with RES2GEO Model. Energies. 2021; 14(7):1923. https://doi.org/10.3390/en14071923
Chicago/Turabian StylePerković, Luka, Domagoj Leko, Amalia Lekić Brettschneider, Hrvoje Mikulčić, and Petar S. Varbanov. 2021. "Integration of Photovoltaic Electricity with Shallow Geothermal Systems for Residential Microgrids: Proof of Concept and Techno-Economic Analysis with RES2GEO Model" Energies 14, no. 7: 1923. https://doi.org/10.3390/en14071923
APA StylePerković, L., Leko, D., Brettschneider, A. L., Mikulčić, H., & Varbanov, P. S. (2021). Integration of Photovoltaic Electricity with Shallow Geothermal Systems for Residential Microgrids: Proof of Concept and Techno-Economic Analysis with RES2GEO Model. Energies, 14(7), 1923. https://doi.org/10.3390/en14071923









