Study of Intrinsic Dissipation Due to Thermoelastic Coupling in Gyroscope Resonators
Abstract
:1. Introduction
2. Theoretical Description of Thermoelastic Coupling Effects in Micro-Resonators
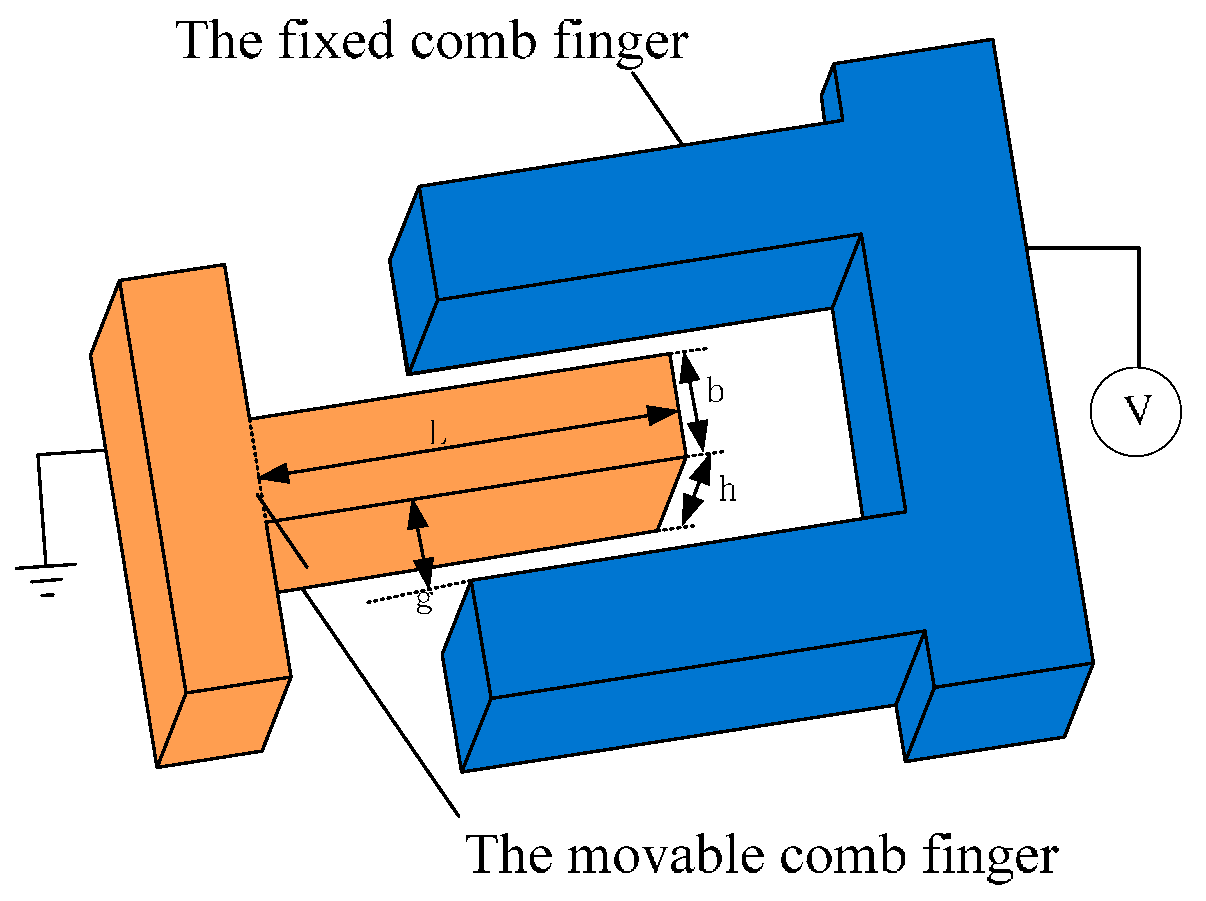
2.1. The Micro-Resonator Model Design
2.2. Governing Equations of Resonator under Electrostatic-Forced Vibration and Free Vibration
3. Analysis Methods of the Coupling Equations
3.1. Direct Coupling Method in Resonator with Electrostatic Load
3.2. Direct Coupling Method in Resonator without Electrostatic Load
3.3. Analytical Approaches to Resonator in Transverse Vibration
4. Numerical Results
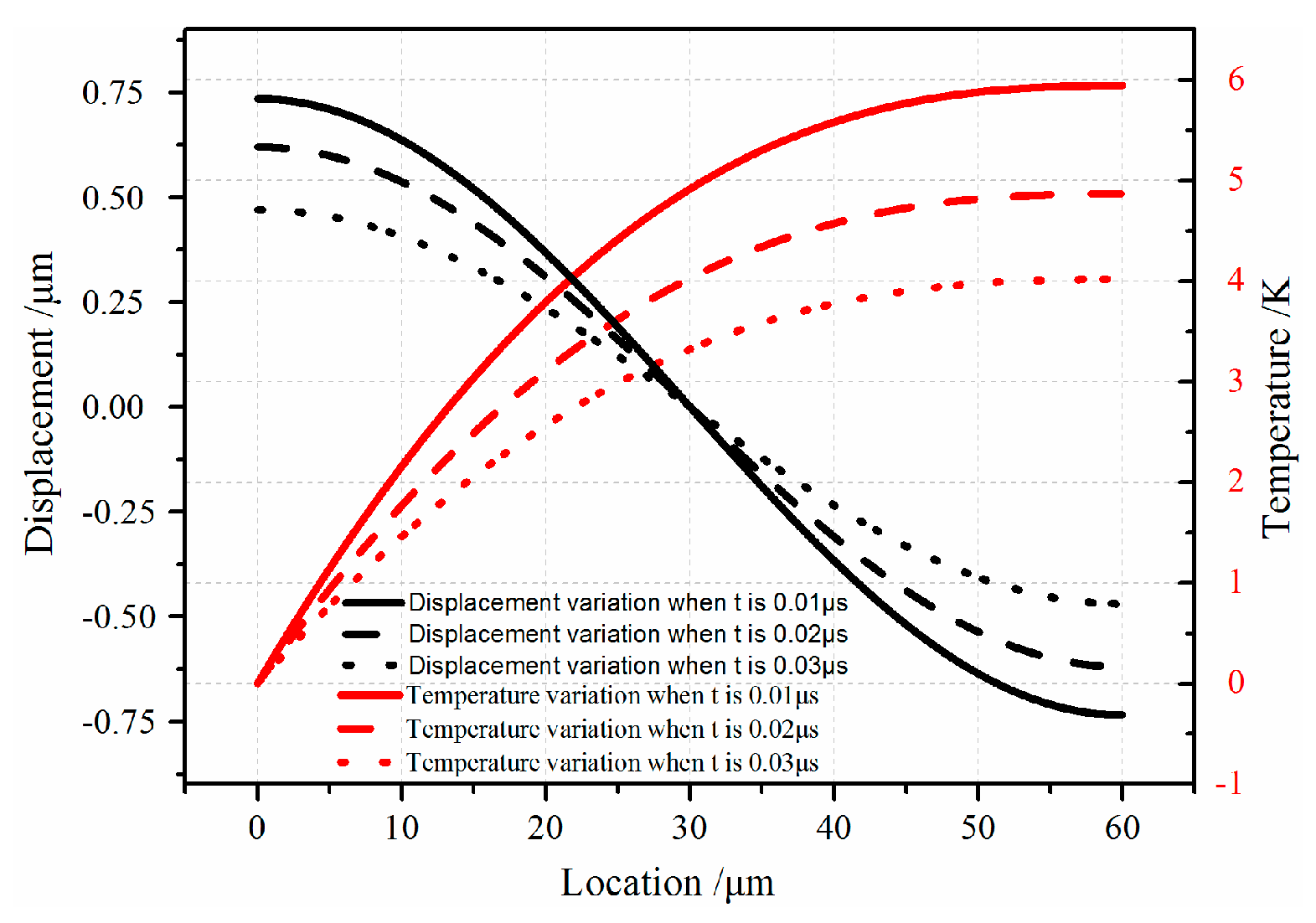
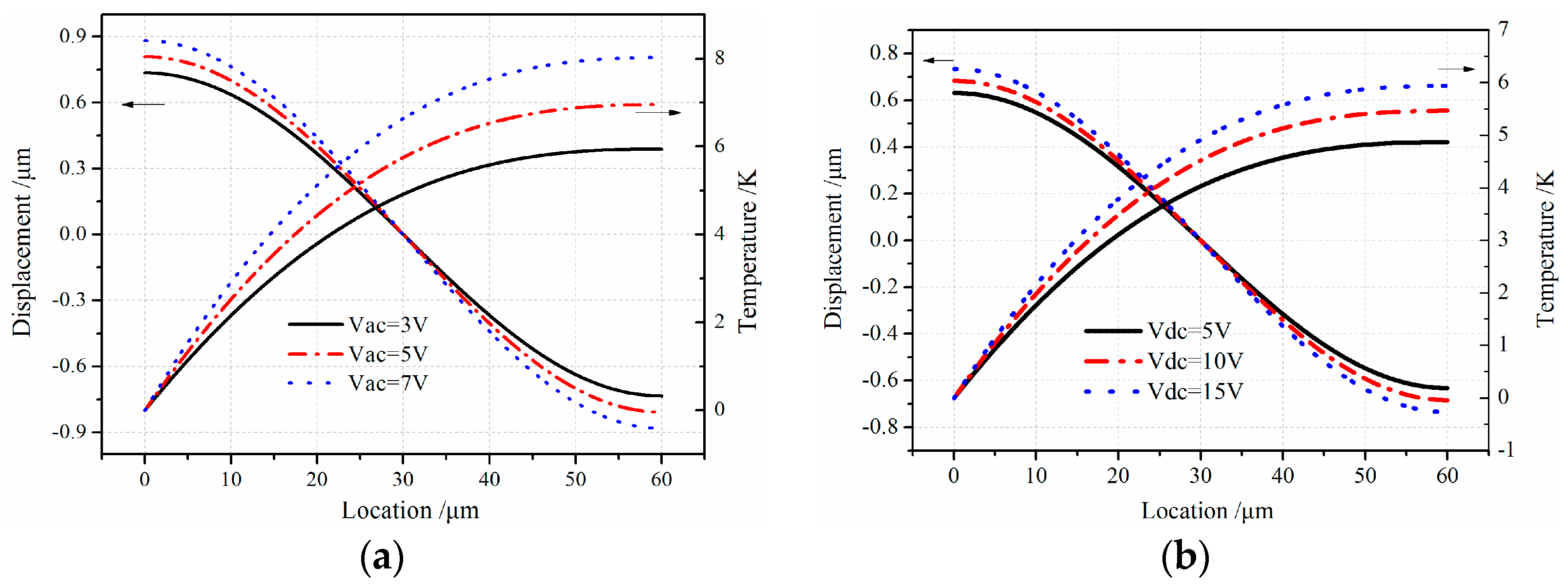
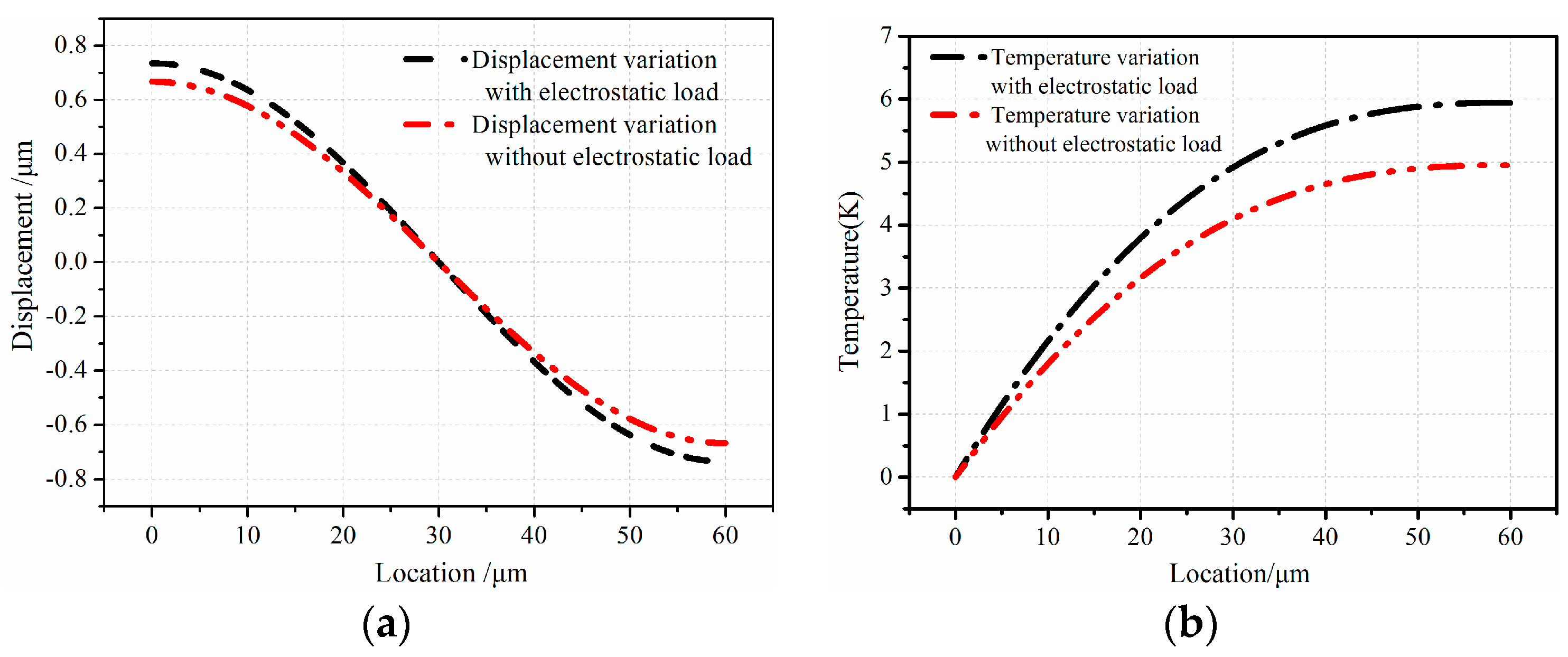
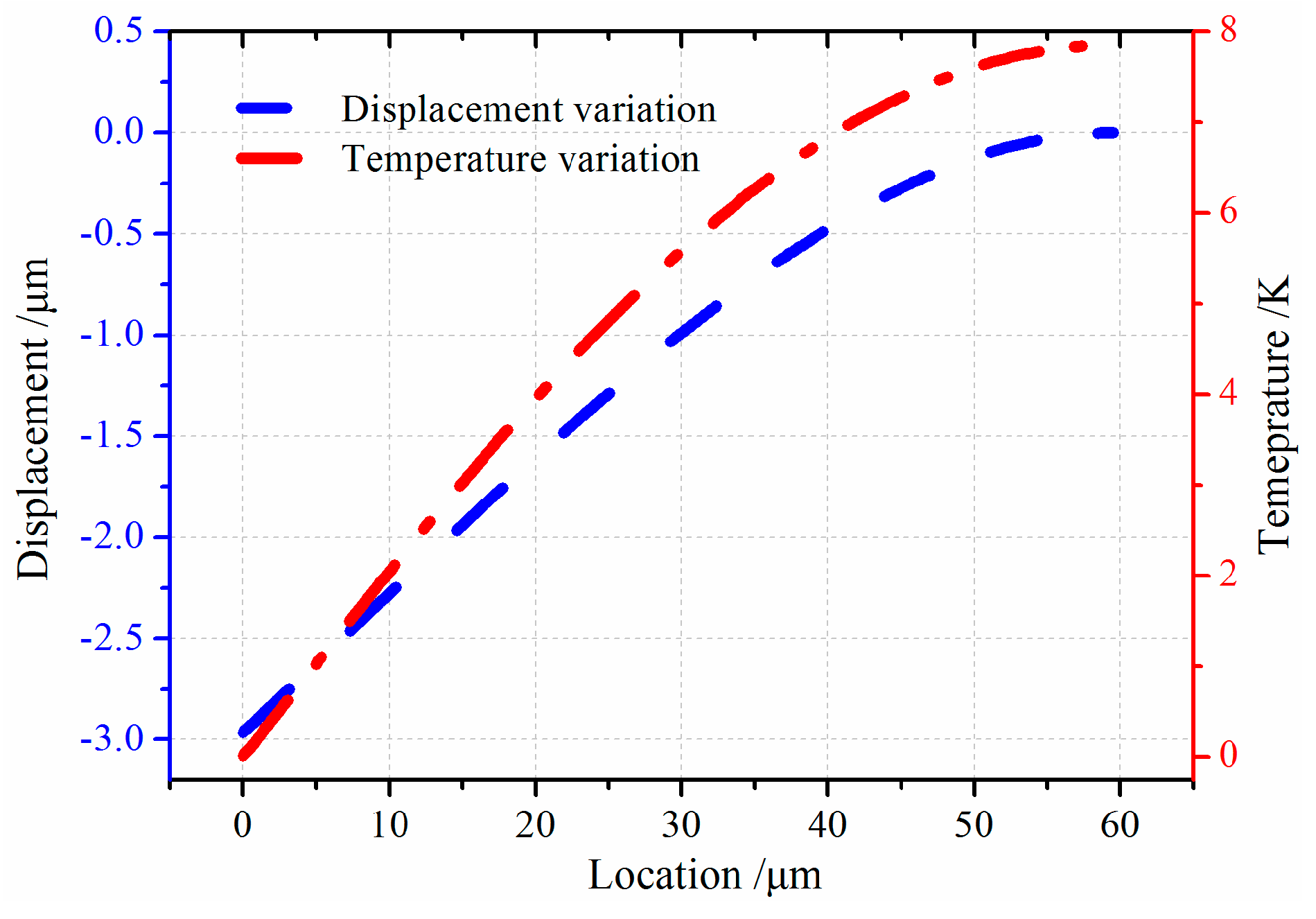
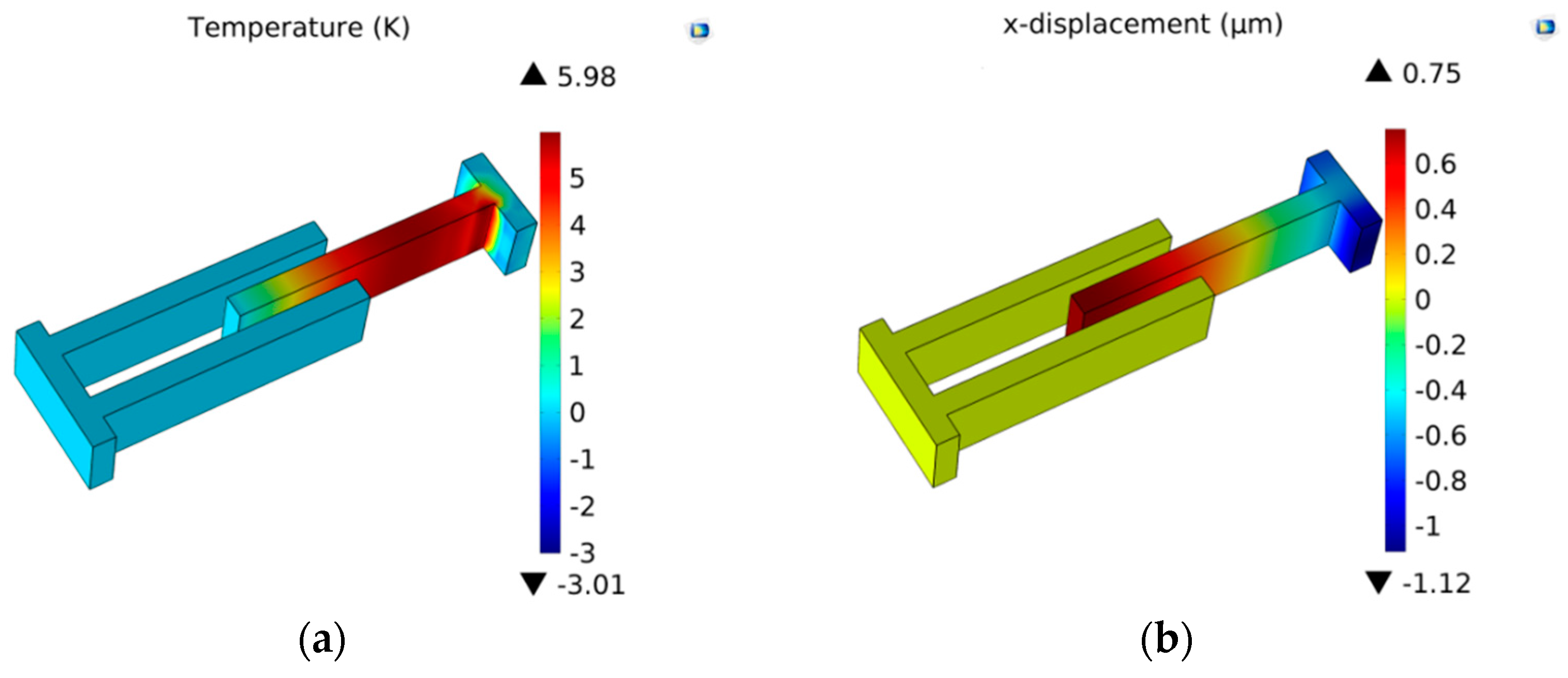
4.1. Thermoelastic Behaviors of Micro-Comb Finger Vibration with Electrostatic Load
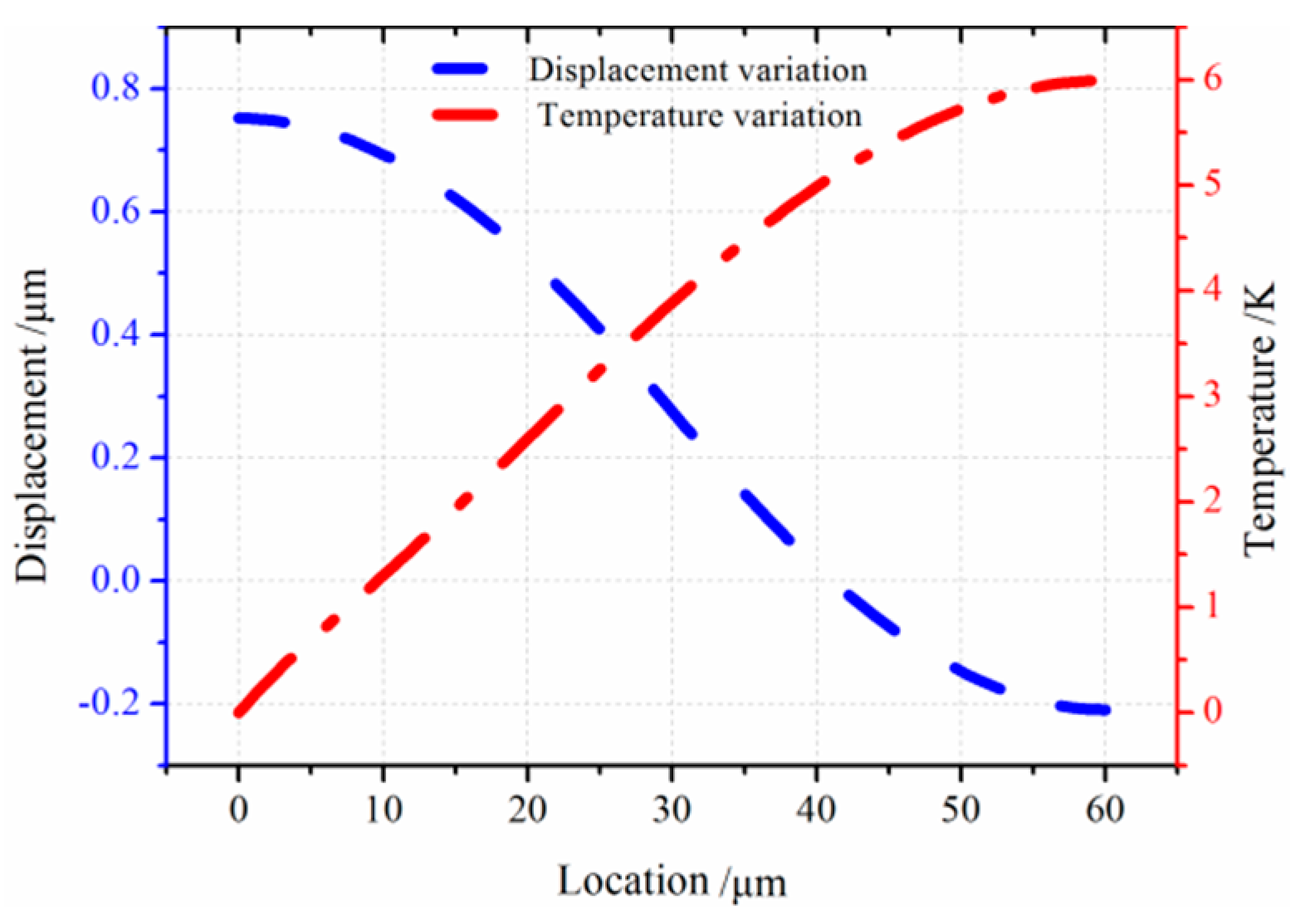
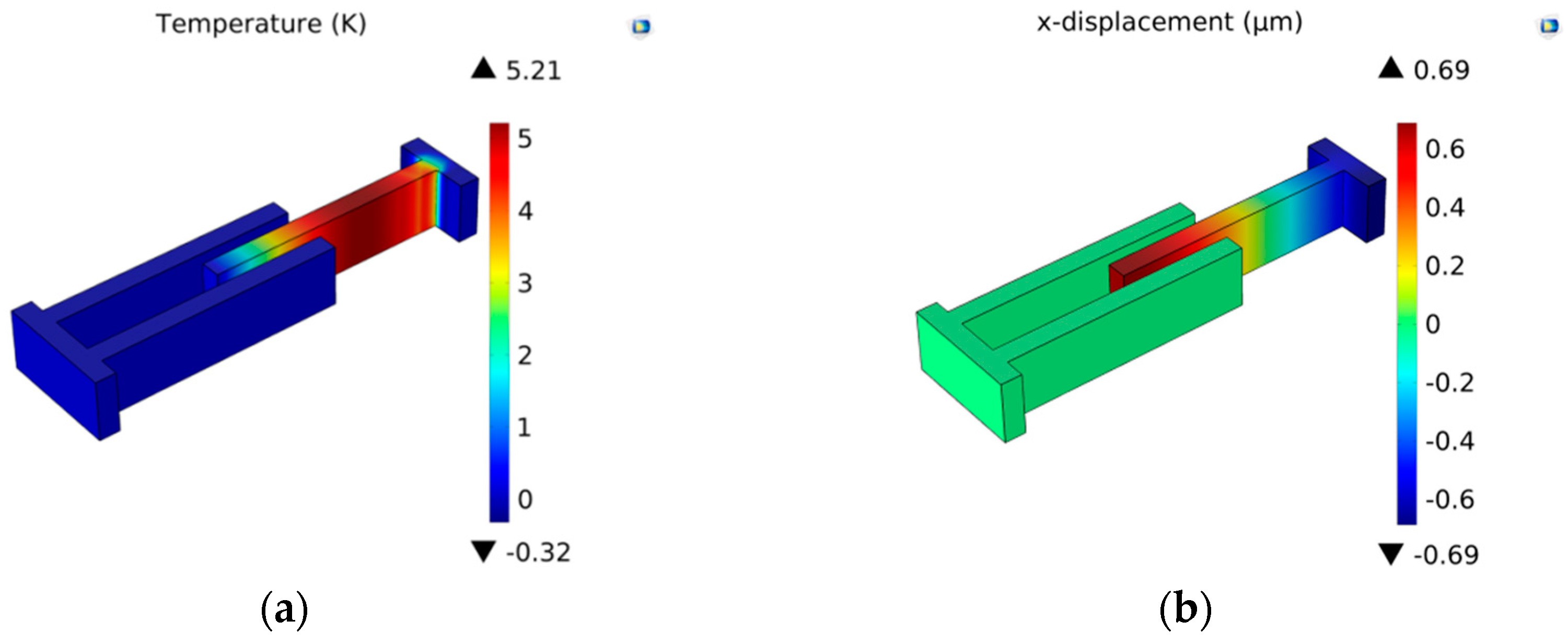
4.2. Thermoelastic Behaviors of Micro-Comb Finger Vibration without Electrostatic Load
4.3. Micro-Comb Finger Thermoelastic Behaviors for Bending Vibration in Sense-Mode
4.4. Simulation Results
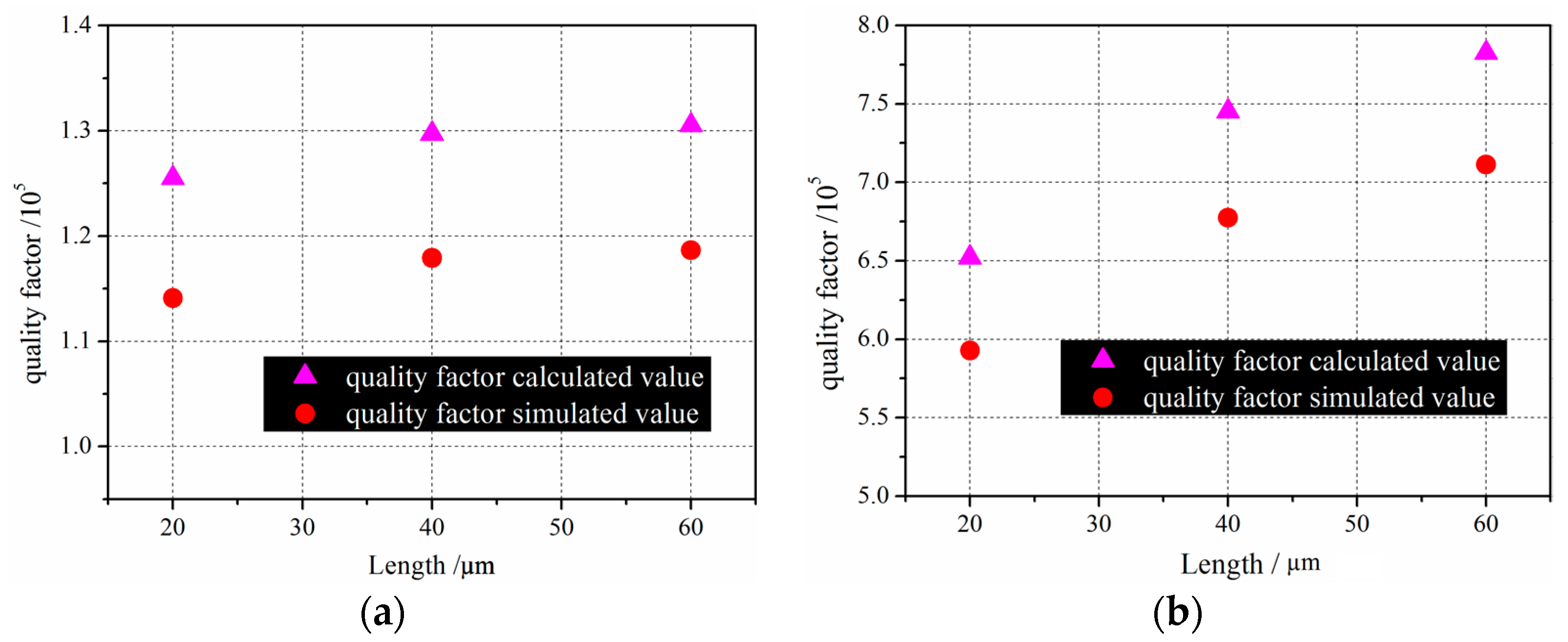
5. The Thermoelastic Damping and Quality Factor
6. Experimental Verification
7. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Kuells, R.; Nau, S.; Salk, M.; Thoma, K. Novel piezoresistive high-g accelerometer geometry with very high sensitivity-bandwidth product. Sens. Actuators A Phys. 2012, 182, 41–48. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, W.; Zhang, F.; Wang, B. A new MEMS gyroscope used for single-channel damping. Sensors 2015, 15, 10146–10165. [Google Scholar] [CrossRef] [PubMed]
- Siao, A.S.; Chao, C.K.; Hsiao, C.C. Study on Pyroelectric Harvesters with Various Geometry. Sensors 2015, 15, 19633–19648. [Google Scholar] [CrossRef] [PubMed]
- Hao, Z.; Xu, Y.; Durgam, S.K. A thermal-energy method for calculating thermoelastic damping in micromechanical resonators. J. Sound Vib. 2009, 322, 870–882. [Google Scholar] [CrossRef]
- Zener, C. Internal friction in solids. I. Theory of internal friction in reeds. Phys. Rev. 1937, 52, 230. [Google Scholar] [CrossRef]
- Zener, C. Internal friction in solids II. General theory of thermoelastic internal friction. Phys. Rev. 1938, 53, 90. [Google Scholar] [CrossRef]
- Lifshitz, R.; Roukes, M.L. Thermoelastic damping in micro-and nanomechanicalsystems. Phys. Rev. B 2000, 61, 5600. [Google Scholar] [CrossRef]
- Rezazadeh, G.; Saeedivahdat, A.; Pesteii, S.M.; Farzi, B. Study of thermoelastic damping in capacitive micro-beam resonators using hyperbolic heat conduction model. Sens. Transducers J. 2009, 108, 54–72. [Google Scholar]
- Jiao, W.; Song, J.; Guo, F. Thermoelastic damping of micro resonators operating in the longitudinal vibration mode: In comparison with the case of flexural vibration. Mech. Res. Commun. 2014, 62, 31–36. [Google Scholar] [CrossRef]
- Guo, F.L.; Rogerson, G.A. Thermoelastic coupling effect on a micro-machined beam resonator. Mech. Res. Commun. 2003, 30, 513–518. [Google Scholar] [CrossRef]
- Kausinis, S.; Yee, K.; Barauskas, R. Estimation of thermo-elastic damping of vibrations in micro-electro-mechanical systems resonators: Finite element modeling. J. Micro/Nanolith. MEMS MOEMS 2012, 11. [Google Scholar] [CrossRef]
- Guo, X.; Yi, Y.B.; Pourkamali, S. A finite element analysis of thermoelastic damping in vented MEMS beam resonators. Int. J. Mech. Sci. 2013, 74, 73–82. [Google Scholar] [CrossRef]
- Yi, Y.B. Finite Element Analysis of Thermoelastic Damping in Contour-Mode Vibrations of Micro and Nanoscale Ring, Disk, and Elliptical Plate Resonators. J. Vib. Acoust. 2010, 132, 1154–1174. [Google Scholar] [CrossRef]
- Duwel, A.; Candler, R.N.; Kenny, T.W.; Varghese, M. Engineering MEMS resonators with low thermoelastic damping. Microelectromech. Syst. J. 2006, 15, 1437–1445. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Younis, M.I. Modeling and simulations of thermoelastic damping in microplates. J. Micromech. Microeng. 2004, 14. [Google Scholar] [CrossRef]
- De, S.K.; Aluru, N.R. Theory of thermoelastic damping in electrostatically actuated microstructures. Phys. Rev. B 2006, 74. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Zenkour, A.M. Thermoelastic problem of an axially moving microbeam subjected to an external transverse excitation. J. Theor. Appl. Mech. 2015, 53, 167–178. [Google Scholar] [CrossRef]
- Bassiouny, E. Thermo-elastic behavior of thin sandwich panel made of piezoelectric layers. Appl. Math. Comput. 2012, 218, 10009–10021. [Google Scholar] [CrossRef]
- Sharma, J.N.; Kaur, R. On the flexural vibrations of generalized thermo-elasto-diffusive thin cantilever beam. J. Vib. Control 2016, 22, 1618–1632. [Google Scholar] [CrossRef]
- Tzou, D.Y. Macro-to Microscale Heat Transfer: The Lagging Behavior; John Wiley & Sons: Hoboken, NJ, USA, 2014. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Course of Theoretical Physics Vol. 7: Theory and Elasticity; Pergamon Press: Oxford, UK, 1959. [Google Scholar]
- Xu, Y.; Wang, R.; Durgam, S.K.; Hao, Z.; Vahala, L. Numerical models and experimental investigation of energy loss mechanisms in SOI-based tuning-fork gyroscopes. Sens. Actuators A Phys. 2009, 152, 63–74. [Google Scholar] [CrossRef]

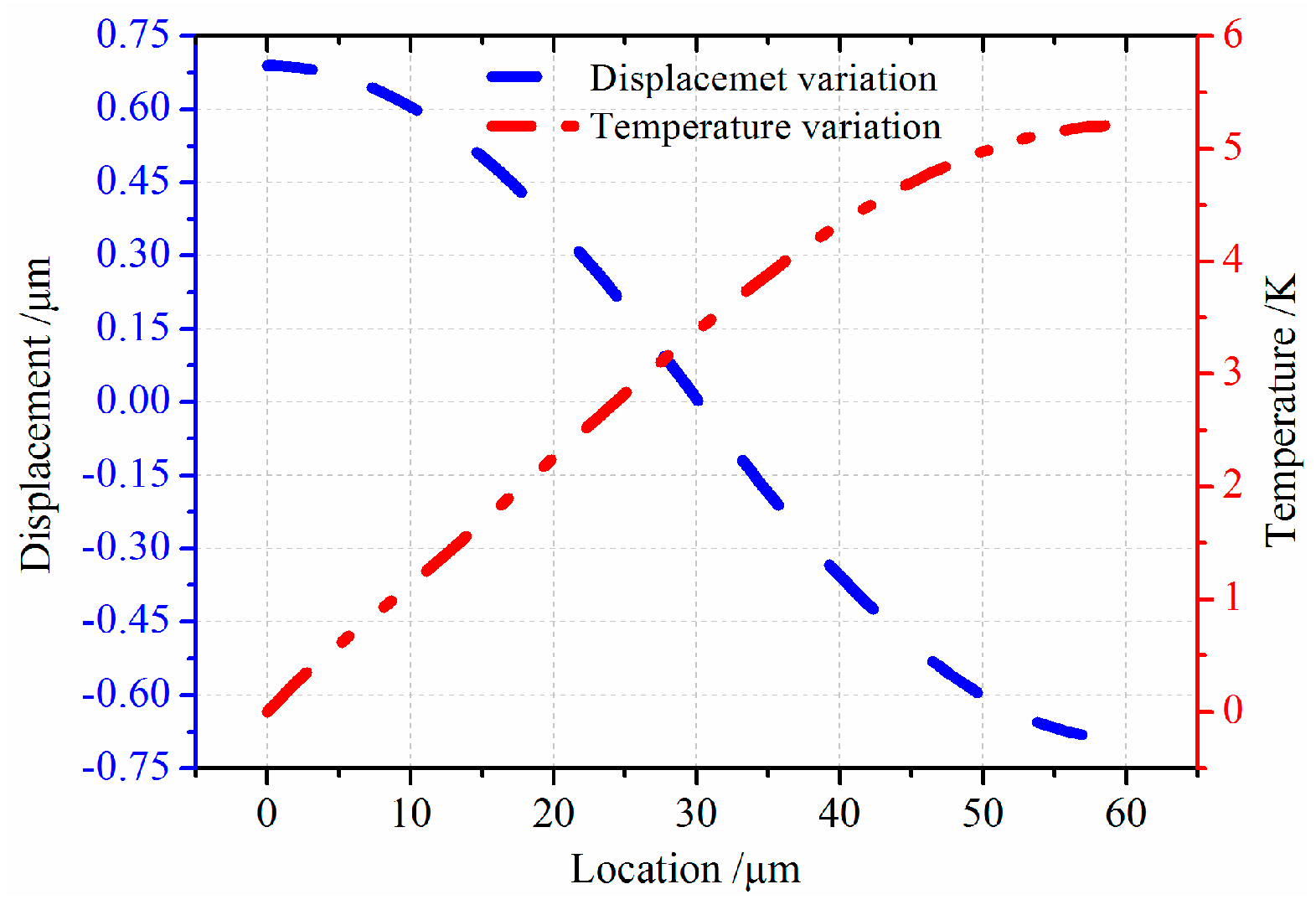
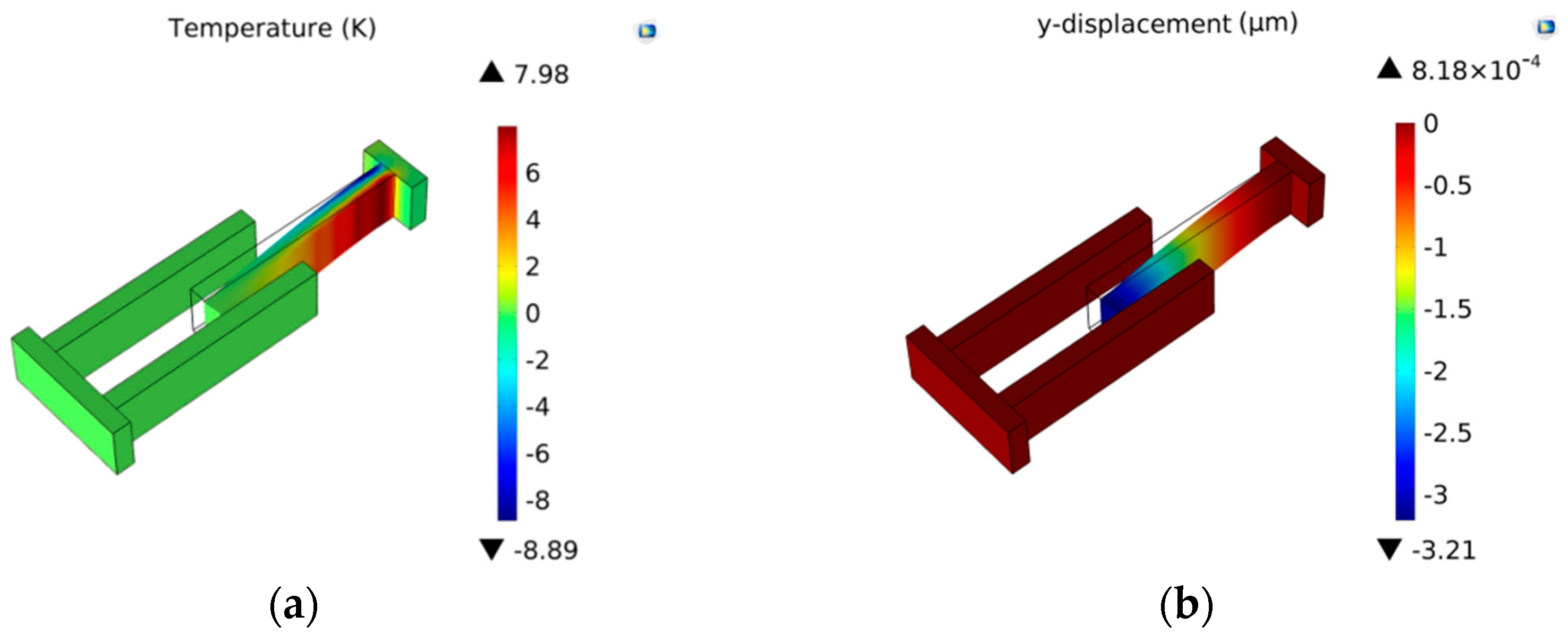

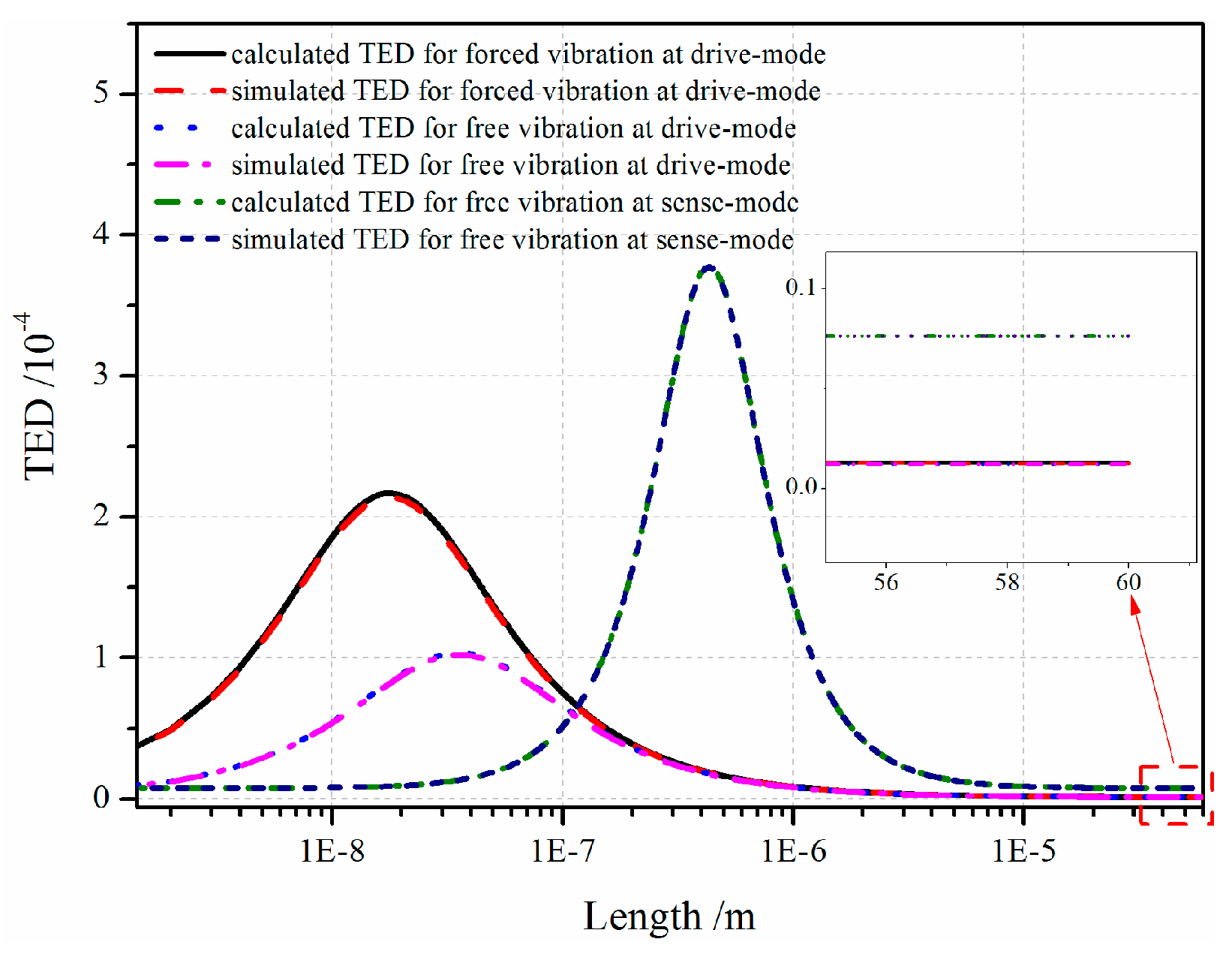
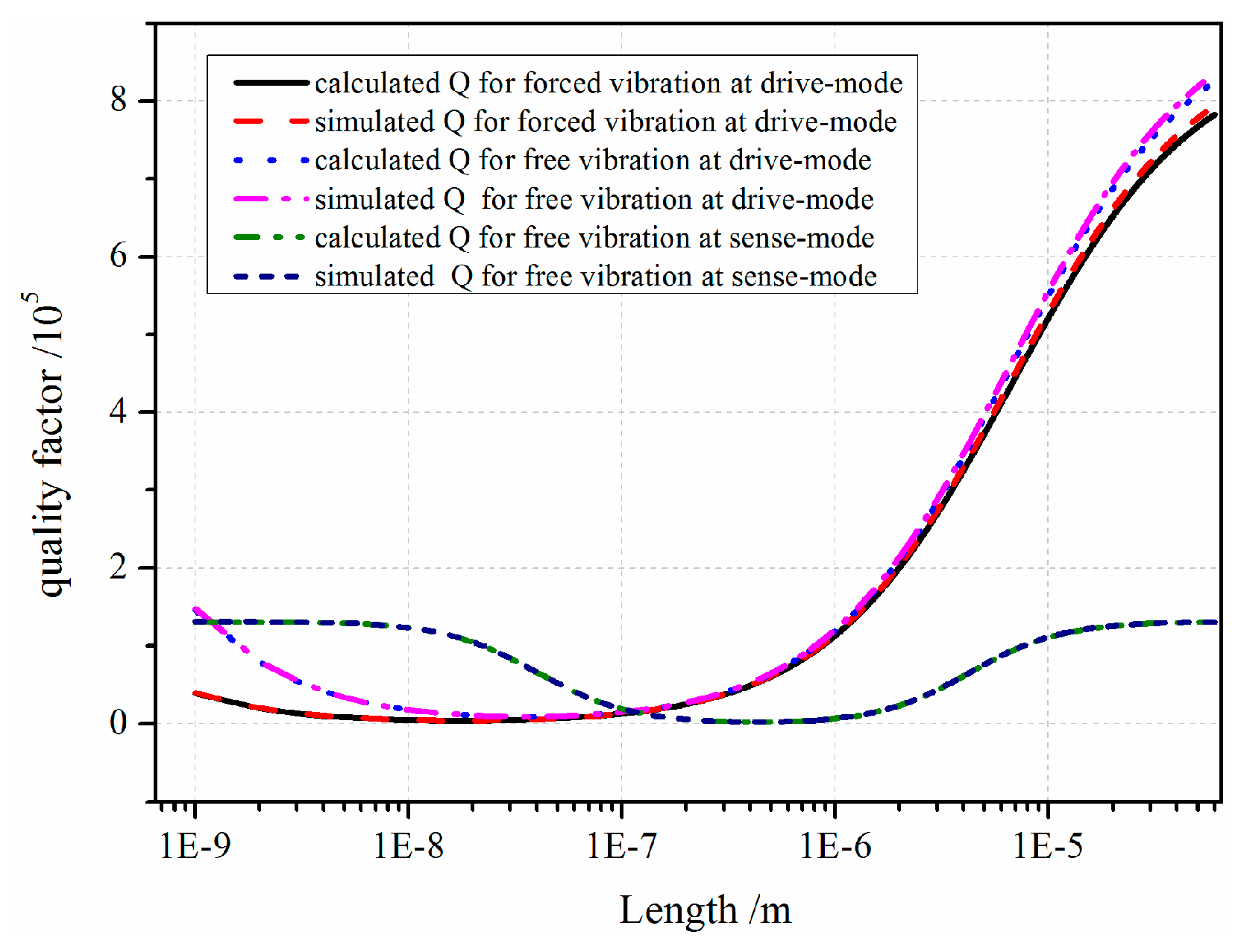
| Mechanical | Thermal | ||||||||
| Values | 165 | 0.22 | 2330 | values | 2.6 × 10−6 | 159 | 713 | 300 | |
| Physical | Electrical | ||||||||
| Values | 60 × 10−6 | 15 × 10−6 | 5 × 10−6 | 5 × 10−6 | Values | 3 | 15 | 8.854 × 10−12 | 0.01 |
| Vibration Mode | Displacement/µm | Temperature/K | ||||
|---|---|---|---|---|---|---|
| Drive-mode | Forced vibration | Theoretical value | 0.735 | −0.735 | 5.943 | 0 |
| Simulation value | 0.752 | −0.752 | 5.977 | 0 | ||
| Error rate (%) | 2.312 | 2.312 | 0.572 | / | ||
| Free vibration | Theoretical value | 0.667 | −0.698 | 4.950 | 0 | |
| Simulation value | 0.69 | −0.750 | 5.210 | 0 | ||
| Error rate (%) | 3.448 | 3.448 | 5.252 | / | ||
| Sense-mode | Theoretical value | −2.966 | 0 | 0 | 7.85 | |
| Simulation value | −3.17 | 0 | 0 | 7.98 | ||
| Error rate (%) | 6.878 | / | / | 1.656 | ||
| Vibration Mode | Drive-Mode | Sense-Mode | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Forced Vibration | Free Vibration | ||||||||
| Theoretical Value | Simulation Value | Error Rate % | Theoretical Value | Simulation Value | Error Rate % | Theoretical Value | Simulation Value | Error Rate % | |
| Frequency/HZ | 4.406 × 108 | 4.409 × 108 | 0.068 | 4.403 × 108 | 4.406 × 108 | 0.068 | 2.264 × 108 | 2.261 × 108 | 0.133 |
| TED | 1.278 × 10−6 | 1.260 × 10−6 | 1.41 | 1.212 × 10−6 | 1.198 × 10−6 | 1.259 | 7.662 × 10−6 | 7.746 × 10−6 | 1.10 |
| Q | 7.825 × 105 | 7.937 × 105 | 1.47 | 8.251 × 105 | 8.347 × 105 | 1.163 | 1.305 × 105 | 1.291 × 105 | 1.07 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Gao, S.; Niu, S.; Liu, H. Study of Intrinsic Dissipation Due to Thermoelastic Coupling in Gyroscope Resonators. Sensors 2016, 16, 1445. https://doi.org/10.3390/s16091445
Li C, Gao S, Niu S, Liu H. Study of Intrinsic Dissipation Due to Thermoelastic Coupling in Gyroscope Resonators. Sensors. 2016; 16(9):1445. https://doi.org/10.3390/s16091445
Chicago/Turabian StyleLi, Changlong, Shiqiao Gao, Shaohua Niu, and Haipeng Liu. 2016. "Study of Intrinsic Dissipation Due to Thermoelastic Coupling in Gyroscope Resonators" Sensors 16, no. 9: 1445. https://doi.org/10.3390/s16091445
APA StyleLi, C., Gao, S., Niu, S., & Liu, H. (2016). Study of Intrinsic Dissipation Due to Thermoelastic Coupling in Gyroscope Resonators. Sensors, 16(9), 1445. https://doi.org/10.3390/s16091445






