Thermochemical Characterization of Sulfur-Containing Furan Derivatives: Experimental and Theoretical Study
Abstract
1. Introduction
2. Materials and Methods
2.1. Compounds and Purity Control
2.2. Combustion Calorimetry Measurements
2.3. Calvet Microcalorimetry Measurements
2.4. Computational Details
3. Results
3.1. Combustion Calorimetry—Standard Molar Enthalpies of Formation in the Condensed Phase
3.2. Vacuum Drop–Microcalorimetric Technique—Enthalpy of Vaporization
3.3. Computational Results
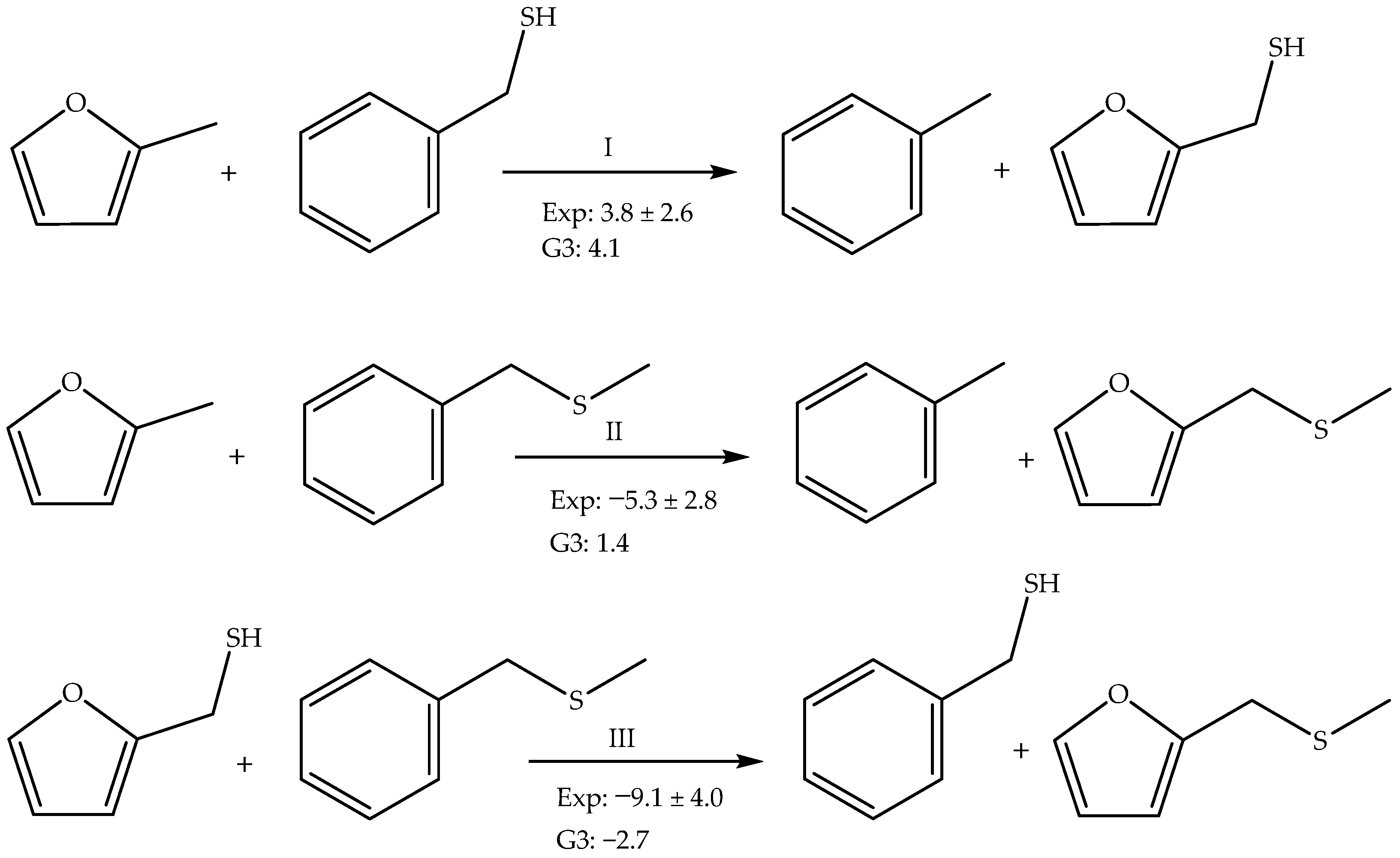
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tominaga, T.; Blanchard, L.; Darriet, P.; Dubourdieu, D. A powerful aromatic volatile thiol, 2-furanmethanethiol, exhibiting roast coffee aroma in wines made from several Vitis vinifera grape varieties. J. Agric. Food Chem. 2000, 48, 1799–1802. [Google Scholar] [CrossRef] [PubMed]
- Grosch, W.; Czerny, M.; Mayer, F.; Moors, A. Sensory studies on the key odorants of roasted coffee. In Caffeinated Beverages: Health Benefits, Physiological Effects, and Chemistry; Parliment, T.H., Ho, C.-T., Schieberle, P., Eds.; ACS Symposium Series; American Chemical Society: Washington, DC, USA, 2000; Chapter 21. [Google Scholar]
- Meng, Q.; Hatakeyama, M.; Sugawara, E. Formation by yeast of 2-furanmethanethiol and ethyl 2-mercaptopropionate aroma compounds in Japanese soy sauce. Biosci. Biotechnol. Biochem. 2014, 78, 109–114. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Wang, B.; Cao, Y. Characterization of potent odorants causing meaty odor reduction in thermal process flavorings with beef-like odor by the sensomics approach. Food Chem. 2023, 426, 136649. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, C.; Sun, L.; Li, M.; Zhu, Y.; Deng, W.; Yu, J.; Zhang, W.; Song, Z. Investigating flavor and quality characteristics in Chinese bacon from different regions using integrated GC-IMS, electronic sensory assessment, and sensory analysis. Meat Sci. 2025, 220, 109709. [Google Scholar] [CrossRef]
- Li, L.; Belloch, C.; Flores, M. A comparative study of savory and toasted aromas in dry cured loins versus dry fermented sausages. LWT 2023, 173, 114305. [Google Scholar] [CrossRef]
- Shen, Y.; Hu, L.-T.; Xia, B.; Ni, Z.-J.; Elam, E.; Thakur, K.; Zhang, J.-G.; Wei, Z.-J. Effects of different sulfur-containing substances on the structural and flavor properties of defatted sesame seed meal derived Maillard reaction products. Food Chem. 2021, 365, 130463. [Google Scholar] [CrossRef]
- Ribeiro da Silva, M.A.V.; Amaral, L.M.P.F. Standard molar enthalpies of formation of 2-furancarbonitrile, 2-acetylfuran, and 3-furaldehyde. J. Chem. Thermodyn. 2009, 41, 26–29. [Google Scholar] [CrossRef]
- Ribeiro da Silva, M.A.V.; Amaral, L.M.P.F. Standard molar enthalpies of formation of some vinylfuran derivatives. J. Chem. Thermodyn. 2009, 41, 349–354. [Google Scholar] [CrossRef]
- Ribeiro da Silva, M.A.V.; Amaral, L.M.P.F. Standard molar enthalpies of formation of some methylfuran derivatives. J. Therm. Anal. Calorim. 2010, 100, 375–380. [Google Scholar] [CrossRef]
- Ribeiro da Silva, M.A.V.; Amaral, L.M.P.F. Thermochemical study of 2,5-dimethyl-3-furancarboxilic acid, 4,5-dimethyl-2-furaldehyde and 3-acetyl-2,5-dimethylfuran. J. Chem. Thermodyn. 2011, 43, 1–8. [Google Scholar] [CrossRef]
- Amaral, L.M.P.F.; Almeida, A.R.R.P.; Ribeiro da Silva, M.A.V. Thermochemical research on furfurylamine and 5-methylfurfurylamine: Experimental and computational insights. Molecules 2024, 29, 2729. [Google Scholar] [CrossRef]
- Sunner, S. Basic principles of combustion calorimetry. In Experimental Chemical Thermodynamics; Sunner, S., Månsson, M., Eds.; Pergamon Press: Oxford, UK, 1979; Chapter 2; Volume 1. [Google Scholar]
- Ribeiro da Silva, M.A.V.; Ferrão, M.L.C.C.H.; Jiye, F. Standard enthalpies of combustion of the six dichlorophenols by rotating-bomb calorimetry. J. Chem. Thermodyn. 1994, 26, 839–846. [Google Scholar] [CrossRef]
- Good, W.D.; Scott, D.W.; Waddington, G. Combustion Calorimetry of Organic Fluorine Compounds by a Rotating-Bomb Method. J. Phys. Chem. 1956, 60, 1080–1089. [Google Scholar] [CrossRef]
- Coops, J.; Jessup, R.S.; Van Nes, K. Calibration of Calorimeters for Reactions in a Bomb at Constant Volume. In Experimental Thermochemistry; Rossini, F.D., Ed.; Interscience: New York, NY, USA, 1956; Chapter 3; Volume 1. [Google Scholar]
- Standard Reference Material® 39j; Certificate of Analysis, Benzoic Acid, Calorimetric Standard. National Institute of Standards & Technology: Gaithersburg, MD, USA, 2007.
- Skinner, H.A.; Snelson, A. The heats of combustion of the four isomeric butyl alcohols. Trans. Faraday Soc. 1960, 6, 1776–1783. [Google Scholar] [CrossRef]
- Waddington, G.; Sunner, S.; Hubbard, W.N. Combustion in a bomb of organic sulfur compounds. In Experimental Thermochemistry; Rossini, F.D., Ed.; Interscience: New York, NY, USA, 1956; Chapter 7; Volume 1. [Google Scholar]
- Santos, L.M.N.B.F.; Silva, M.T.; Schröder, B.; Gomes, L. Labtermo: Methodologies for the calculation of the corrected temperature rise in isoperibol calorimetry. J. Therm. Anal. Calorim. 2007, 89, 175–180. [Google Scholar] [CrossRef]
- Vogel, A.I. Quantitative Inorganic Analysis; Longmans: London, UK, 1978. [Google Scholar]
- Aldrich. Chemical Handbook of Fine Chemicals and Laboratory Equipment; Sigma-Aldrich Chemical Co.: Gillingham, UK, 2011. [Google Scholar]
- Prohaska, T.; Irrgeher, J.; Benefield, J.; Böhlke, J.; Chesson, L.; Coplen, T.; Ding, T.; Dunn, P.; Gröning, M.; Holden, N.; et al. Standard atomic weights of the elements 2021 (IUPAC Technical Report). Pure Appl. Chem. 2022, 94, 573–600. [Google Scholar] [CrossRef]
- Adedeji, F.A.; Larange, D.; Brown, S.; Connor, J.A.; Leung, M.L.; Paz-Andrade, I.M.; Skinner, H.A. Thermochemistry of arene chromium tricarbonyls and the strenghts of arene-chromium bonds. J. Organomet. Chem. 1975, 97, 221–228. [Google Scholar] [CrossRef]
- Ribeiro da Silva, M.A.V.; Matos, M.A.R.; Amaral, L.M.P.F. Thermochemical study of 2-, 4-, 6-, and 8-methylquinoline. J. Chem. Thermodyn. 1995, 27, 565–574. [Google Scholar] [CrossRef]
- Santos, L.M.N.B.; Schröder, B.; Fernandes, O.O.P.; Ribeiro da Silva, M.A.V. Measurement of enthalpies of sublimation by drop method in a Calvet type calorimeter: Design and test of a new system. Thermochim. Acta 2004, 415, 15–20. [Google Scholar] [CrossRef]
- Sabbah, R.; Xu-Wu, A.; Chickos, J.S.; Leitão, M.L.P.; Roux, M.V.; Torres, L.A. Reference materials for calorimetry and differential thermal analysis. Thermochim. Acta 1999, 331, 93–204. [Google Scholar] [CrossRef]
- Curtiss, L.A.; Raghavachari, K.; Redfern, P.C.; Rassolov, V.; Pople, J.A. Gaussian-3 (G3) theory for molecules containing first and second-row atoms. J. Chem. Phys. 1998, 109, 7764–7776. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A. Gaussian 09, Revision A.02; Gaussian, Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
- Cox, J.D.; Wagman, D.D.; Medvedev, V.A. CODATA Key Values for Thermodynamics; Hemisphere: New York, NY, USA, 1989. [Google Scholar]
- Wagman, D.D.; Evans, W.H.; Parker, V.B.; Schumm, R.H.; Halow, I.; Bailey, S.M.; Churney, K.L.; Nuttall, R.L. The NBS tables of chemical thermodynamic properties. Selected values for inorganic and C1 and C2 organic substances in SI units. J. Phys. Chem. Ref. Data 1982, 11, 2–392. Available online: https://srd.nist.gov/JPCRD/jpcrdS2Vol11.pdf (accessed on 20 October 2019).
- Rossini, F.D. Assignment of uncertainties to thermochemical data. In Experimental Thermochemistry; Rossini, F.D., Ed.; Interscience: New York, NY, USA, 1956; Chapter 14; Volume 1. [Google Scholar]
- Olofsson, G. Assignment of uncertainties. In Combustion Calorimetry; Sunner, S., Månsson, M., Eds.; Pergamon: Oxford, UK, 1979; Chapter 6. [Google Scholar]
- Merrick, J.P.; Moran, D.; Radom, L. An evaluation of harmonic vibrational frequency scale factors. J. Phys. Chem. A 2007, 111, 11683–11700. [Google Scholar] [CrossRef]
- Roux, M.V.; Temprado, M.; Chickos, J.S.; Nagano, Y. Critically Evaluated Thermochemical Properties of Polycyclic Aromatic Hydrocarbons. J. Phys. Chem. Ref. Data 2008, 37, 1855–1996. [Google Scholar] [CrossRef]
- Pedley, J.B.; Naylor, R.D.; Kirby, S.P. Thermochemical Data of Organic Compounds; Chapman and Hall: New York, NY, USA, 1986. [Google Scholar]
- Manion, J.A. Evaluated enthalpies of formation of the stable closed shell C1 and C2 chlorinated hydrocarbons. J. Phys. Chem. Ref. Data 2002, 31, 123–172. [Google Scholar] [CrossRef]
- Hawari, J.A.; Griller, D.; Lossing, F.P. Thermochemistry of perthiyl radicals. J. Am. Chem. Soc. 1986, 108, 3273–3275. [Google Scholar] [CrossRef]
- Cohen, N.; Benson, S.W. Estimation of heats of formation of organic compounds by additivity methods. Chem. Rev. 1993, 93, 2419–2438. [Google Scholar] [CrossRef]
- Benson, S.W.; Cruickshank, F.R.; Golden, D.M.; Haugen, G.R.; O’Neal, H.E.; Rodgers, A.S.; Shaw, R.; Walsh, R. Additivity rules for the estimation of thermochemical properties. Chem. Rev. 1969, 69, 279–324. [Google Scholar] [CrossRef]
- Joback, K.G.; Reid, R.C. Estimation of pure-component properties from group-contributions. Chem. Eng. Commun. 1987, 57, 233–243. [Google Scholar] [CrossRef]
- Cnstantinou, L.; Gani, R. New group contribution method for estimating properties of pure compounds. AIChE J. 1994, 40, 1697–1710. [Google Scholar] [CrossRef]
- Marrero, J.; Gani, R. Group-contribution based estimation of pure component properties. Fluid Phase Equilib. 2001, 183–184, 183–208. [Google Scholar] [CrossRef]
- Eugene, S.; Domalski, E.S.; Hearing, E.D. Estimation of the Thermodynamic Properties of C-H-N-O-S-Halogen Compounds at 298.15 K. J. Phys. Chem. Ref. Data 1993, 22, 805–1159. [Google Scholar] [CrossRef]
- Cox, J.D. A Method for Estimating the Enthalpies of Formation of Benzene Derivatives in the Gas State; NPL Report CHEM 83; National Physical Laboratory: Teddington, UK, 1978. [Google Scholar]
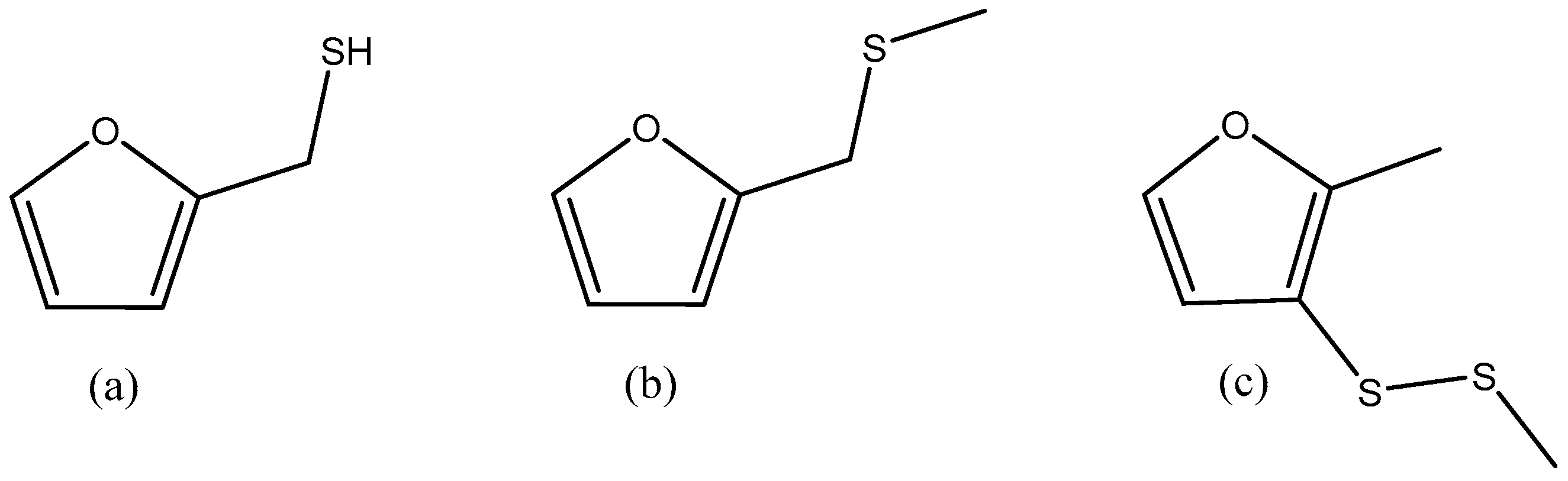

| 2-Furanmethanethiol | Furfuryl Methyl Sulfide | Methyl 2-methyl-3-furyl Disulfide |
|---|---|---|
| −29,284.68 | −31,152.30 | −28,662.57 |
| −29,287.28 | −31,152.78 | −28,661.47 |
| −29,275.86 | −31,151.91 | −28,642.81 |
| −29,266.72 | −31,152.02 | −28,656.62 |
| −29,270.64 | −31,149.41 | −28,651.97 |
| −29,268.02 | −31,149.29 | −28,649.01 |
| −28,648.10 | ||
| a | ||
| −29,275.5 ± 3.6 | −31,151.3 ± 0.6 | −28,653.2 ± 2.8 |
| Compound | − | − | |
|---|---|---|---|
| 2-Furanmethanethiol | 3342.3 ± 1.3 | 3348.5 ± 1.3 | −78.5 ± 1.4 |
| Furfuryl methyl sulfide | 3393.4 ± 1.2 | 4000.8 ± 1.2 | −105.6 ± 1.4 |
| Methyl 2-methyl-3-furyl disulfide | 4591.9 ± 1.6 | 4603.1 ± 1.6 | −105.2 ± 1.8 |
| Compound | ||||
|---|---|---|---|---|
| 2-Furanmethanethiol | 329.0 | 52.2 ± 0.1 | 3.6 | 48.6 ± 1.0 |
| Furfuryl methyl sulfide | 334.3 | 58.3 ± 0.3 | 5.1 | 53.2 ± 1.2 |
| Methyl 2-methyl-3-furyl disulfide | 334.3 | 62.6 ± 0.5 | 6.1 | 56.5 ± 1.5 |
| Compound | ||||
|---|---|---|---|---|
| Exp a | G3 b | |||
| 2-Furanmethanethiol | −78.5 ± 1.4 | 48.6 ± 1.0 | −29.9 ± 1.7 | −30.3 ± 4.5 |
| Furfuryl methyl sulfide | −105.6 ± 1.4 | 53.2 ± 1.2 | −52.4 ± 1.8 | −50.8 ± 4.1 |
| Methyl 2-methyl-3-furyl disulfide | −105.2 ± 1.8 | 56.5 ± 1.5 | −48.7 ± 2.3 | −57.5 ± 2.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amaral, L.M.P.F.; Ribeiro da Silva, M.A.V. Thermochemical Characterization of Sulfur-Containing Furan Derivatives: Experimental and Theoretical Study. Thermo 2025, 5, 11. https://doi.org/10.3390/thermo5010011
Amaral LMPF, Ribeiro da Silva MAV. Thermochemical Characterization of Sulfur-Containing Furan Derivatives: Experimental and Theoretical Study. Thermo. 2025; 5(1):11. https://doi.org/10.3390/thermo5010011
Chicago/Turabian StyleAmaral, Luísa M. P. F., and Manuel A. V. Ribeiro da Silva. 2025. "Thermochemical Characterization of Sulfur-Containing Furan Derivatives: Experimental and Theoretical Study" Thermo 5, no. 1: 11. https://doi.org/10.3390/thermo5010011
APA StyleAmaral, L. M. P. F., & Ribeiro da Silva, M. A. V. (2025). Thermochemical Characterization of Sulfur-Containing Furan Derivatives: Experimental and Theoretical Study. Thermo, 5(1), 11. https://doi.org/10.3390/thermo5010011









