An Electron Waveguide Model for FDSOI Transistors
Abstract
:1. Introduction
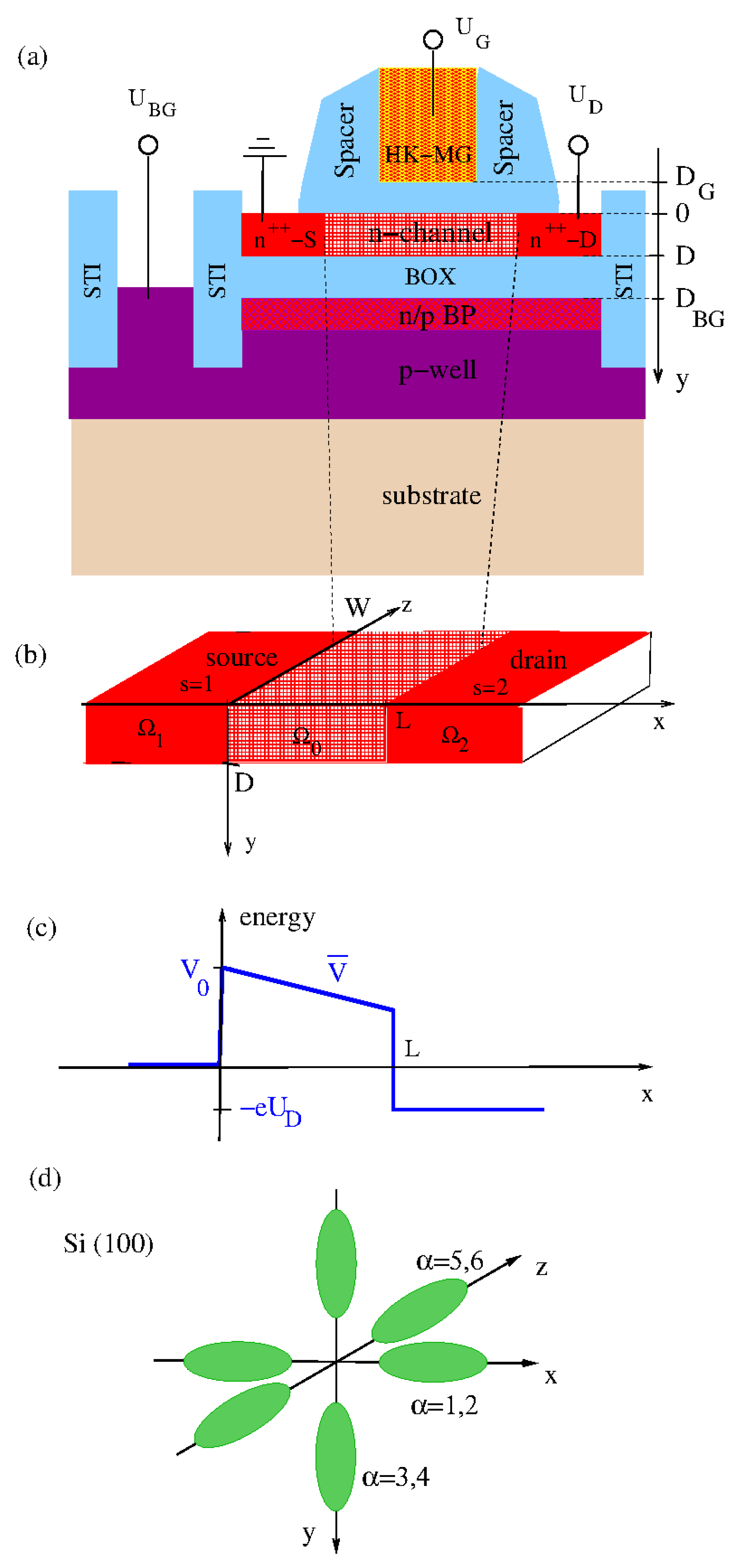
2. Materials and Methods
2.1. Quantum Transport Model
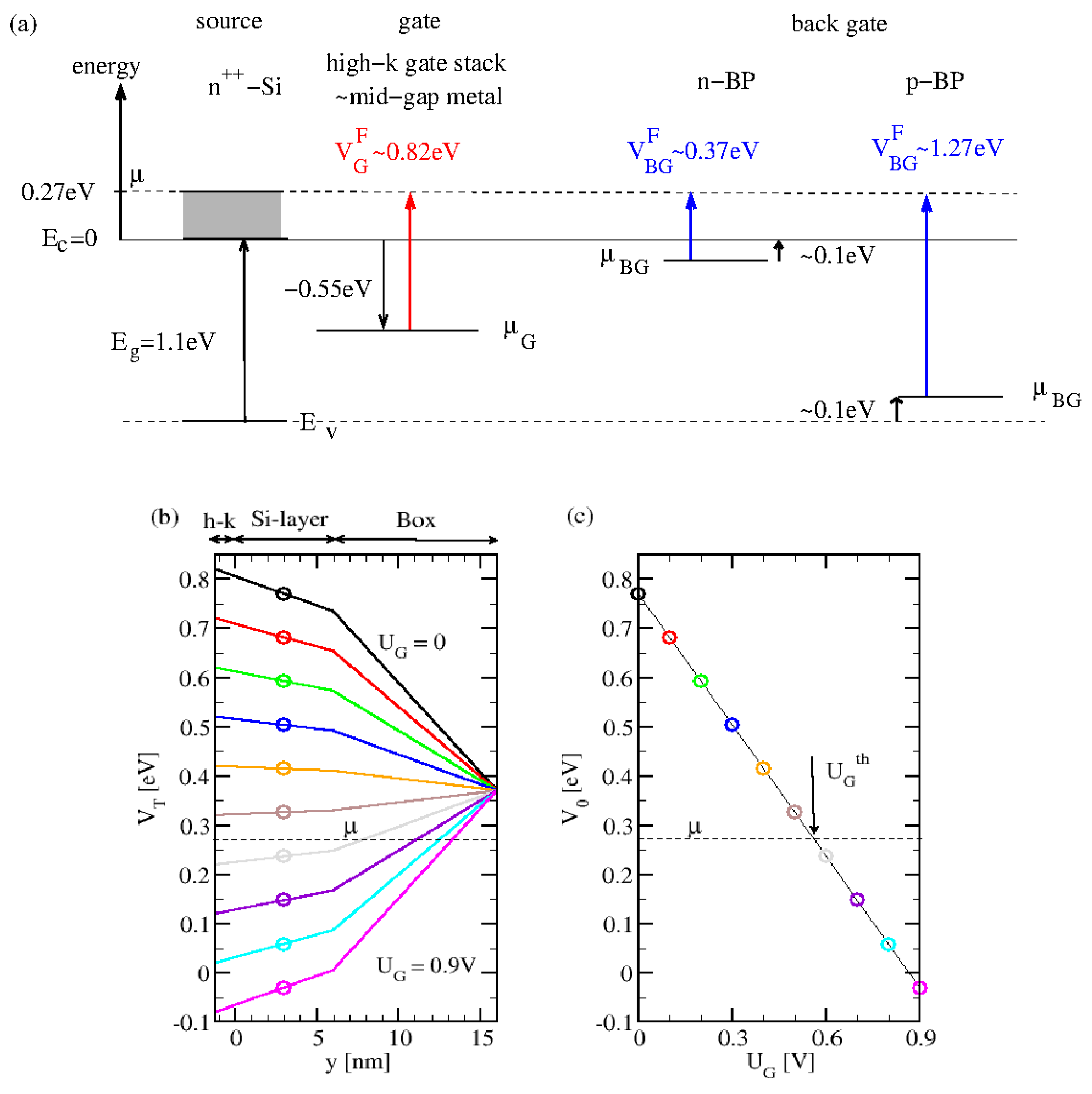
2.2. Potential in Abrupt Transition Approximation
3. Results
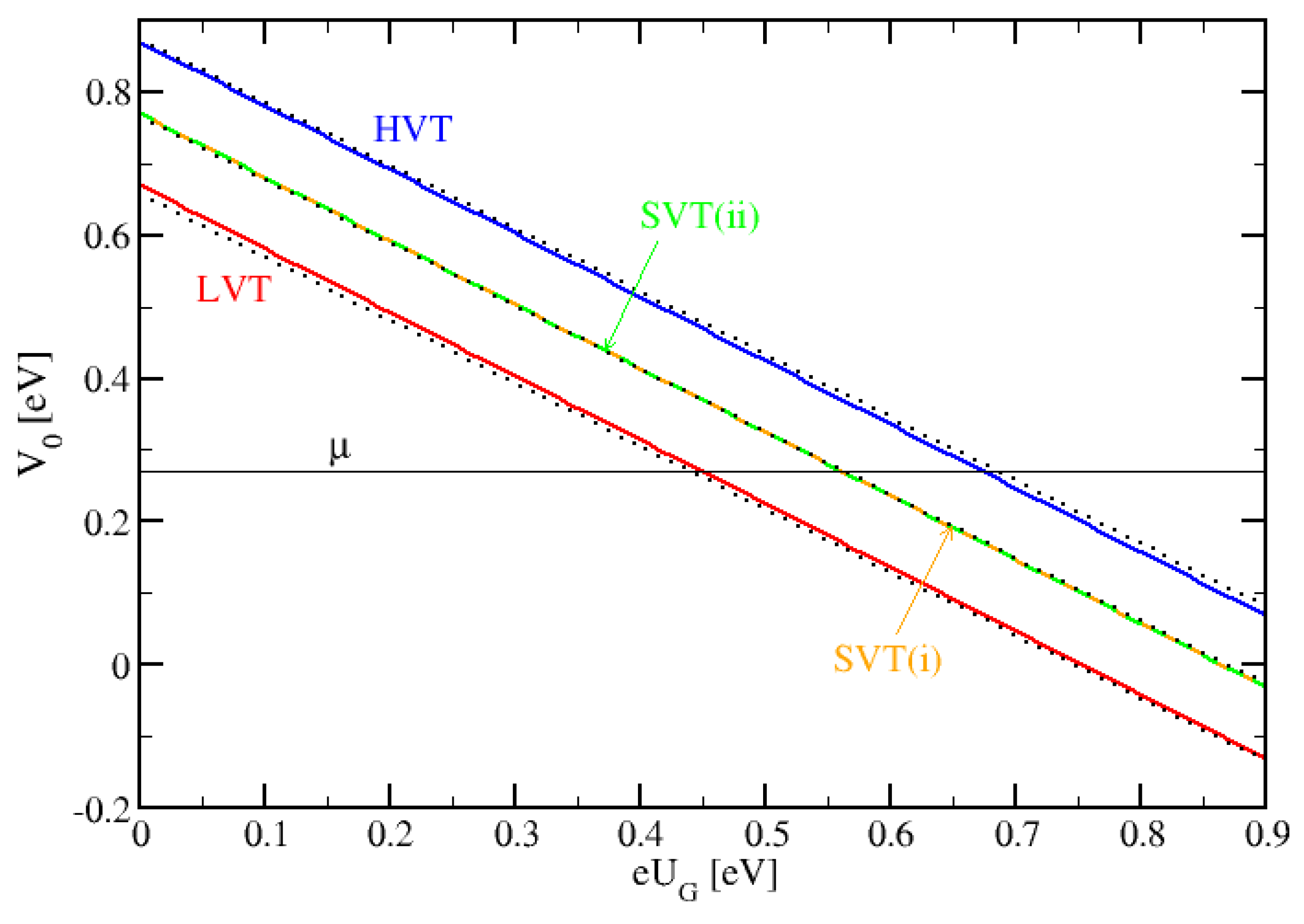
- n-BP with : standard-VT (SVT (i)), identical with Figure 2b;
- n-BP with : low-VT (LVT);
- p-BP with : high-VT (HVT);
- p-BP with standard-VT (SVT (ii)).
4. Discussion
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| MDPI | Multidisciplinary Digital Publishing Institute |
| DOAJ | Directory of open access journals |
| TLA | Three letter acronym |
| LD | Linear dichroism |
Appendix A. Adoption of a Previous Transistor model for the Calculation of the Drain Current
Appendix B. The Chemical Potential in Source and Drain
Appendix C. Solution of the Poisson Equation
- The matching condition leads to
- The matching condition leads to
- The matching condition leads to
- The matching condition leads to
Appendix D. Analytical Approximations for the Barrier Haight and the Threshold Voltage
References
- Eugster, C.C.; del Alamo, J. Tunneling spectroscopy of an electron waveguide. Phys. Rev. Lett. 1991, 67, 3586. [Google Scholar] [CrossRef] [PubMed]
- Eugster, C.C.; del Alamo, J. Electron waveguide devices. Superlattices Microstruct. 1998, 23, 121–137. [Google Scholar]
- van Wees, B.J.; van Houten, H.; Beenakker, C.W.J.; Williamson, J.G.; Kouwenhoven, L.P.; van der Marel C., T. Foxon, D. Quantized conductance of point contacts in a two-dimensional. Phys. Rev. Lett. 1988, 60, 848. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wharam, D.A.; Thornton, T.H.; Newbury, R.; Pepper, M.; Ahmed, H.; Frost, J.E.F.; Hasko, D.G.; Peacock, D.C.; Ritchie, D.A.; Jones, G.A.C. One-dimensional transport and the quantisation of the ballistic resistance. J. Phys. C 1988, 21, L209. [Google Scholar] [CrossRef]
- Cheng, K.; Khakifirooz, A. Fully depleted SOI (FDSOI) technology. Sci. China Inf. Sci. 2016, 59, 61402. [Google Scholar] [CrossRef] [Green Version]
- Pandey, A. Recent Trends in Novel Semiconductor Devices. Silicon 2022. [Google Scholar] [CrossRef]
- Nemnes, G.A.; Wulf, U.; Racec, P.N. Nano-transistors in the Landauer-Büttiker formalism. J. Appl. Phys. 2004, 96, 596–604. [Google Scholar] [CrossRef]
- Nemnes, G.A.; Wulf, U.; Racec, P.N. Nonlinear I-V characteristics of nanotransistors in the Landauer-Büttiker formalism. J. Appl. Phys. 2005, 98, 084308. [Google Scholar] [CrossRef]
- Wulf, U.; Kučera, J.; Richter, H.; Horstmann, M.; Wiatr, M.; Höntschel, J. Channel engineering for nanotransistors in a semiempirical quantum transport model. Mathematics 2017, 5, 68. [Google Scholar] [CrossRef] [Green Version]
- Wulf, U. A One-Dimensional Effective Model for Nanotransistors in Landauer-Büttiker Formalism. Micromachines 2020, 11, 359. [Google Scholar] [CrossRef] [Green Version]
- Horstmann, M.; Greenlaw, D.; Feudel, T.; Wei, A.; Frohberg, K.; Burbach, G.; Gerhardt, M.; Lenski, M.; Stephan, R.; Wieczorek, K.; et al. Sub-50 nm gate length SOI transistor development for high performance microprocessors. Mater. Sci. Eng. B 2004, 114, 3–8. [Google Scholar] [CrossRef]
- Numata, T.; Irisawa, T.; Tezuka, T.; Koga, J.; Hirashita, N.; Usuda, K.; Toyoda, E.; Miyamura, Y.; Tanabe, A.; Sugiyama, N.; et al. Performance enhancement of partially and fully depleted strained-SOI MOSFETs. IEEE Trans. Electron Devices 2006, 53, 1030–1038. [Google Scholar] [CrossRef]
- Fenouillet-Beranger, C.; Denormel, S.; Icard, B.; Boeuf, F.; Coignus, J.; Faynot, L.; Brevard, L.; Buj, C.; Soonekindt, C.; Todeschini, J.; et al. Fully-Depleted SOI Technology using High-K and Single-Metal Gate for 32 nm Node LSTP Applications featuring 0.179 μm2 6T-SRAM bitcell. In Proceedings of the 2007 IEEE International Electron Devices Meeting (IEDM), Washington, DC, USA, 10–12 December 2007. [Google Scholar]
- Fenouillet-Beranger, C.; Thomas, O.; Perreau, P.; Noel, J.P.; Bajolet, A.; Haendler, S.; Tosti, L.; Barnola, S.; Beneyton, R.; Perrot, C.; et al. Efficient Multi-VT FDSOI technology with UTBOX for low power circuit design. In Proceedings of the 2010 Symposium on VLSI Technology (VLSIT), Honolulu, HI, USA, 15–17 June 2010. [Google Scholar]
- Cheng, K.; Khakifirooz, A.; Kulkarni, P.; Edge, L.F.; Reznicek, A.; Adam, T.; He, H.; Seo, S.C.; Kanakasabapathy, S.; Schmitz, S.; et al. Challenges and Solutions of Extremely Thin SOI (ETSOI) for CMOS Scaling to 22 nm Node and Beyond. ECS Trans. 2010, 27, 951. [Google Scholar] [CrossRef]
- Liu, Q.; Monsieur, F.; Kumar, A.; Yamamoto, T.; Yagishita, A.; Kulkarnic, P.; Ponoth, S.; Loubet, N.; Cheng, K.; Khakifirooz, A.; et al. Impact of back bias on ultra-thin body and BOX (UTBB) devices. In Proceedings of the 2011 Symposium on VLSI Technology—Digest of Technical Papers, Kyoto, Japan, 14–16 June 2011. [Google Scholar]
- Skotnicki, T. Competitive SOC with UTBB SOI. In Proceedings of the 2011 IEEE International SOI Conference (SOI), Tempe, AZ, USA, 3–6 October 2011. [Google Scholar]
- Noel, J.; Thomas, O.; Jaud, M.A.; Weber, O.; Poiroux, T.; Fenouillet-Beranger, C.; Rivallin, P.; Scheiblin, P.; Andrieu, F.; Vinet, M.; et al. Multi-VT UTBB FDSOI Device Architectures for Low-Power CMOS Circuit. IEEE Trans. Electron Divices 2011, 58, 2473–2482. [Google Scholar] [CrossRef]
- Doris, B.; DeSalvo, B.; Cheng, K.; Morin, P.; Vinet, M. Planar Fully-Depleted-Silicon-On-Insulator technologies: Toward the 28 nm node and beyond. Solid-State Electron. 2016, 117, 37–59. [Google Scholar] [CrossRef]
- Venugopal, R.; Ren, Z.; Datta, S.; Lundstrom, M.S.; Jovanovic, D. Simulating quantum transport in nanoscale transistors: Real versus mode-space approaches. J. Appl. Phys. 2002, 92, 3730–3739. [Google Scholar] [CrossRef]
- Venugopal, R.; Goasguen, S.; Datta, S.; Lundstrom, M.S. Quantum mechanical analysis of channel access geometry and series resistance in nanoscale transistors. J. Appl. Phys. 2004, 95, 292–305. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.; Polizzi, E.; Lundstrom, M. A three-dimensional quantum simulation of silicon nanowire transistors with the effective-mass approximation. J. Appl. Phys. 2004, 96, 2192–2203. [Google Scholar] [CrossRef] [Green Version]
- Polizzi, E.; Abdallah, N.B. Subband decomposition approach for the simulation of quantum electron transport in nanostructures. J. Comput. Phys. 2005, 202, 150–180. [Google Scholar] [CrossRef]
- Luisier, M.; Schenk, A.; Fichtner, W. Quantum transport in two- and three-dimensional nanoscale transistors: Coupled mode effects in the nonequilibrium Green’s function formalism. J. Appl. Phys. 2006, 100, 043713. [Google Scholar] [CrossRef] [Green Version]
- Vyurkov, V.; Semenikhin, I.; Filippov, S.; Orlikovsky, A. Quantum simulation of an ultrathin body field-effect transistor with channel imperfections. Solid State Electron. 2012, 70, 106–113. [Google Scholar] [CrossRef]
- Chu, Y.; Sarangapani, P.; Charles, J.; Klimeck, G.; Kubis, T. Explicit screening full band quantum transport model for semiconductor nanodevices. J. Appl. Phys. 2018, 123, 244501. [Google Scholar] [CrossRef]
- Chu, Y.; Lu, S.-C.; Chowdhury, N.; Povolotskyi, M.; Klimeck, G.; Mohamed, M.; Palacios, T. Superior Performance of 5-nm Gate Length GaN Nanowire nFET for Digital Logic Applications. IEEE Electron. Device Lett. 2019, 40, 874–877. [Google Scholar] [CrossRef]
- Martinez, A.; Barker, J.R. Quantum Transport in a Silicon Nanowire FET Transistor: Hot Electrons and Local Power Dissipation. Materials 2020, 13, 3326. [Google Scholar] [CrossRef]
- Sun, B.; Richstein, B.; Liebisch, P.; Frahm, T.; Scholz, S.; Trommer, J.; Mikolajick, T.; Knoch, J. On the Operation Modes of Dual-Gate Reconfigurable Nanowire Transistors. IEEE Trans. Electron. Devices 2021, 68, 3684–3689. [Google Scholar] [CrossRef]
- Prakash, O.; Dabhi, C.K.; Chauhan, Y.S.; Amrouch, H. Transistor Self-Heating: The Rising Challenge for Semiconductor Testing. In Proceedings of the 2021 IEEE 39th VLSI Test Symposium (VTS), San Diego, CA, USA, 25–28 April 2021. [Google Scholar]
- Liu, R.; Li, X.; Sun, Y.; Li, F.; Shi, Y. Thermal Coupling Among Channels and Its DC Modeling in Sub-7-nm Vertically Stacked Nanosheet Gate-All-Around Transistor. IEEE Trans. Electron. Devices 2021, 68, 6563–6570. [Google Scholar] [CrossRef]
- Young, K.K. Short-channel effect in fully depleted SOI MOSFETs. IEEE Trans. Electron. Devices 1989, 36, 399–402. [Google Scholar] [CrossRef]
- Yan, R.H.; Ourmazd, A.; Lee, K.F. Scaling the Si MOSFET: From bulk to SOI to bulk. IEEE Trans. Electron. Devices 1992, 39, 1704–1710. [Google Scholar] [CrossRef] [Green Version]
- Tsormpatzoglou, A.; Dimitriadis, C.A.; Clerc, R.; Rafhay, Q.; Panannakakis, G.; Ghibaudo, G. Semi-Analytical Modeling of Short-Channel Effects in Si and Ge Symmetrical Double-Gate MOSFETs. IEEE Trans. Electron. Devices 2007, 54, 1943–1952. [Google Scholar] [CrossRef]
- Mohammadi, H.; Abdullah, H.; Dee, V.F. A review on modeling the channel potential in multi-gate MOSFETs. Sains Malays. 2014, 43, 861–866. [Google Scholar]
- Li, X.; Ma, L.; Ai, Y.; Han, W. From parabolic approximation to evanescent mode analysis on SOI MOSFET. J. Semicond. 2017, 38, 024005. [Google Scholar] [CrossRef]

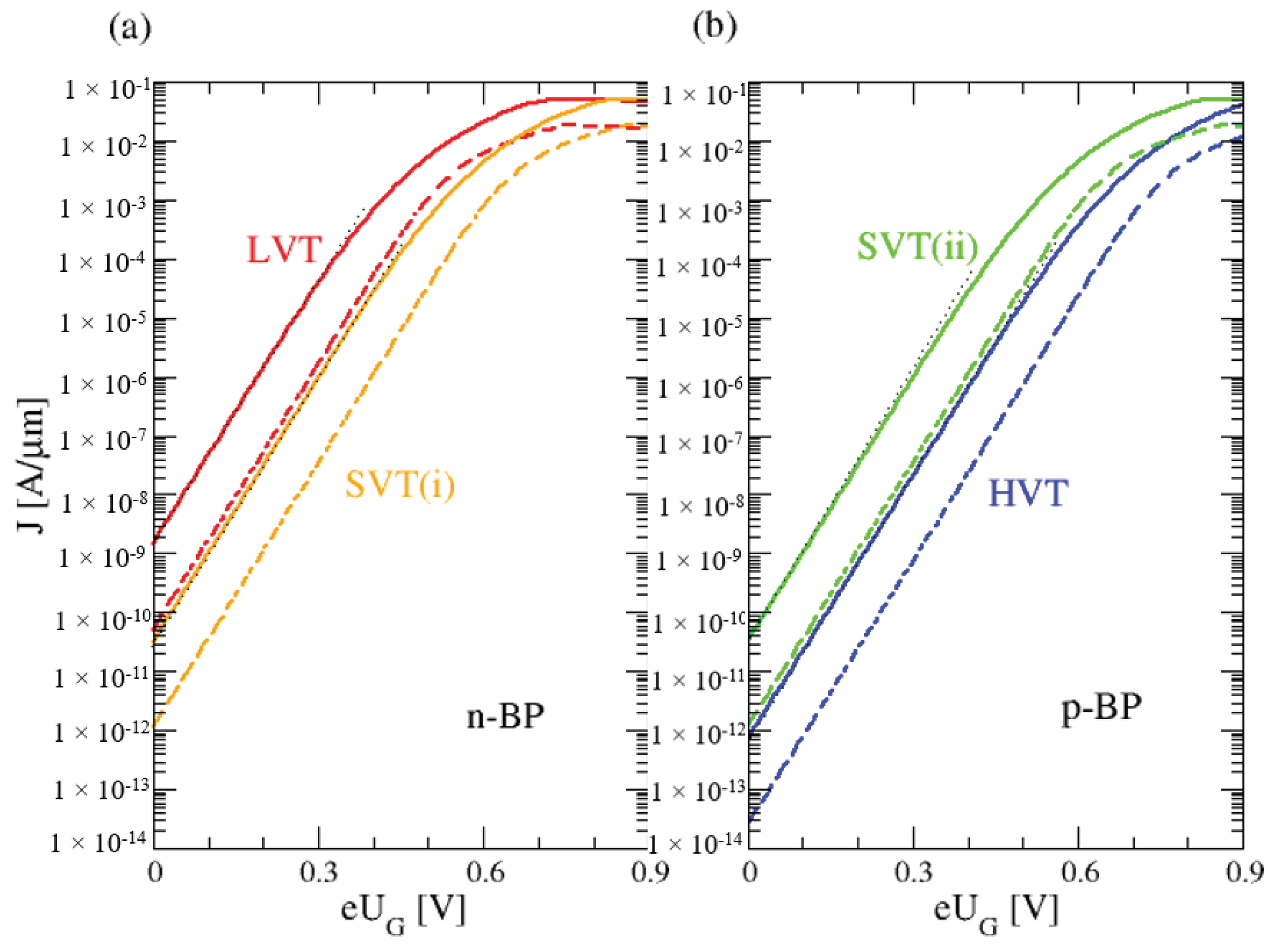
| Type | [mV] | [mV/dec] | ||
|---|---|---|---|---|
| LVT | 440 | 45,900 | 1474 | 70 |
| SVT(i) | 563 | 49,452 | 32 | 70 |
| SVT(ii) | 563 | 44,952 | 32 | 70 |
| HVT(i) | 686 | 39,385 | 0.7 | 70 |
| LVT | 278 | 480 | 2139 | 77 |
| SVT(i) | 429 | 332 | 16 | 76 |
| SVT(ii) | 430 | 333 | 17 | 77 |
| HVT(i) | 567 | 208 | 0.1 | 73 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wulf, U. An Electron Waveguide Model for FDSOI Transistors. Solids 2022, 3, 203-218. https://doi.org/10.3390/solids3020014
Wulf U. An Electron Waveguide Model for FDSOI Transistors. Solids. 2022; 3(2):203-218. https://doi.org/10.3390/solids3020014
Chicago/Turabian StyleWulf, Ulrich. 2022. "An Electron Waveguide Model for FDSOI Transistors" Solids 3, no. 2: 203-218. https://doi.org/10.3390/solids3020014
APA StyleWulf, U. (2022). An Electron Waveguide Model for FDSOI Transistors. Solids, 3(2), 203-218. https://doi.org/10.3390/solids3020014





