Similarity of Heterogeneous Kinetics to Delay of Double-Layer Capacitance Using Chronoamperometry
Abstract
1. Introduction
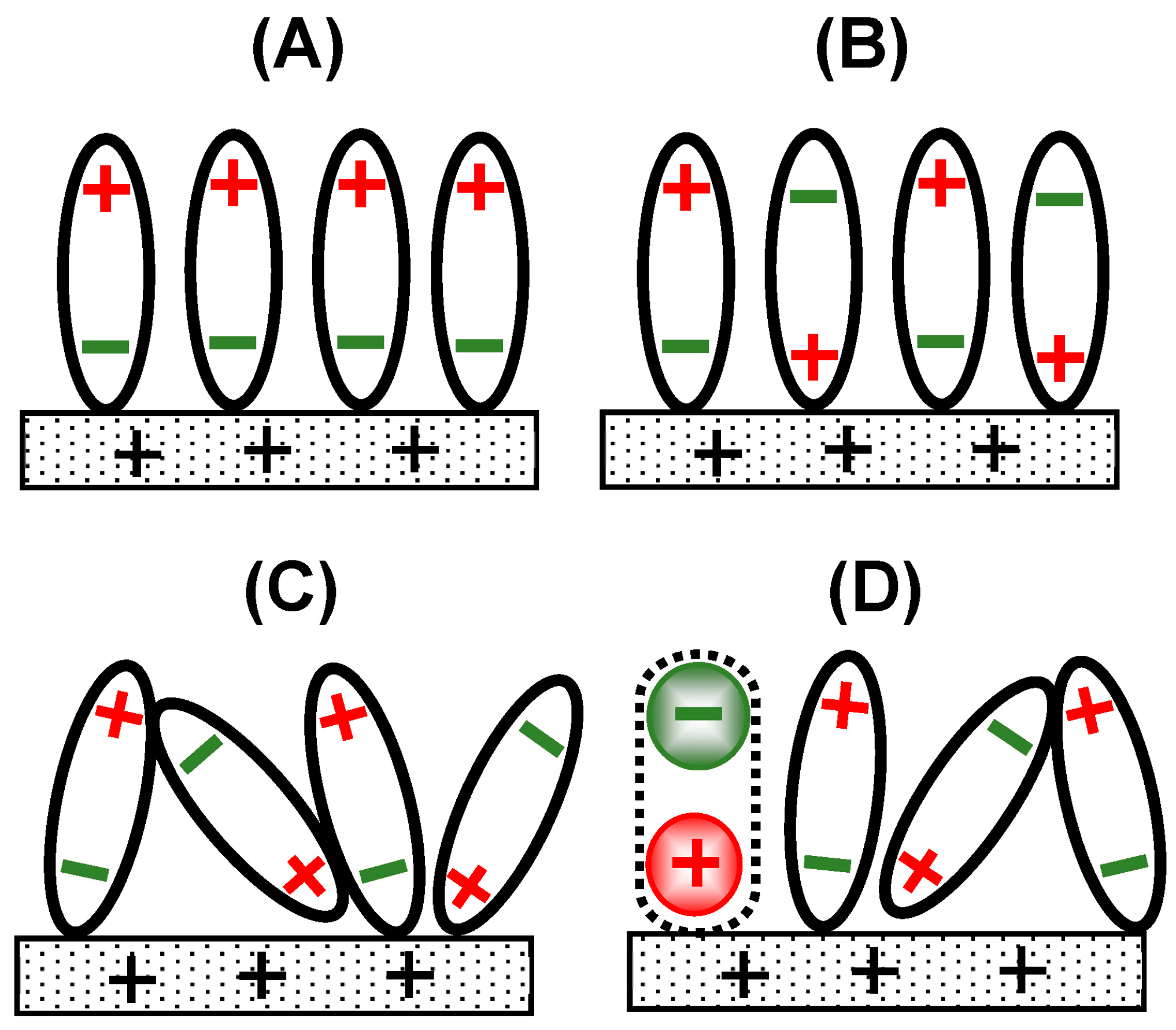
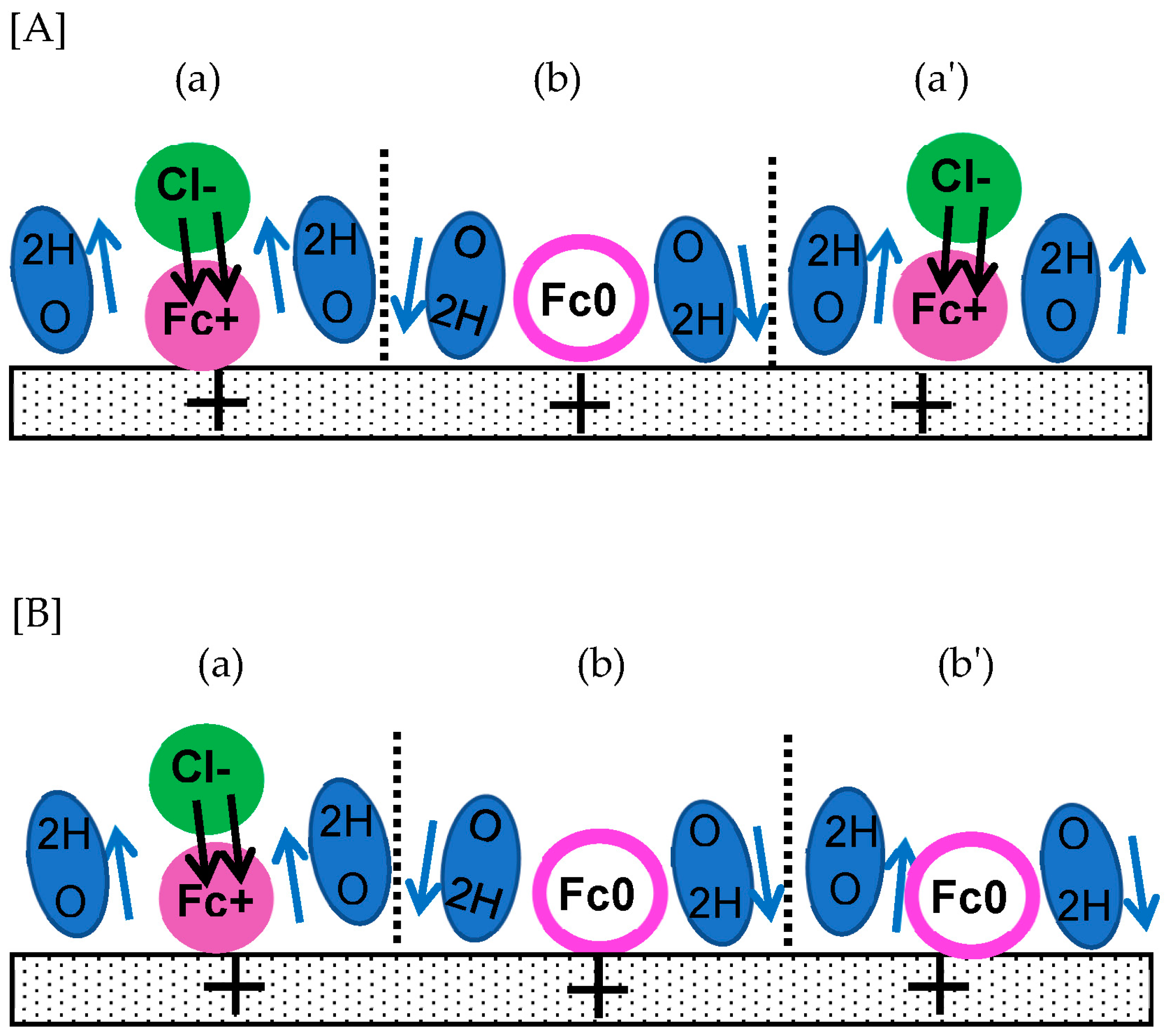
2. Theory
3. Experimental Section
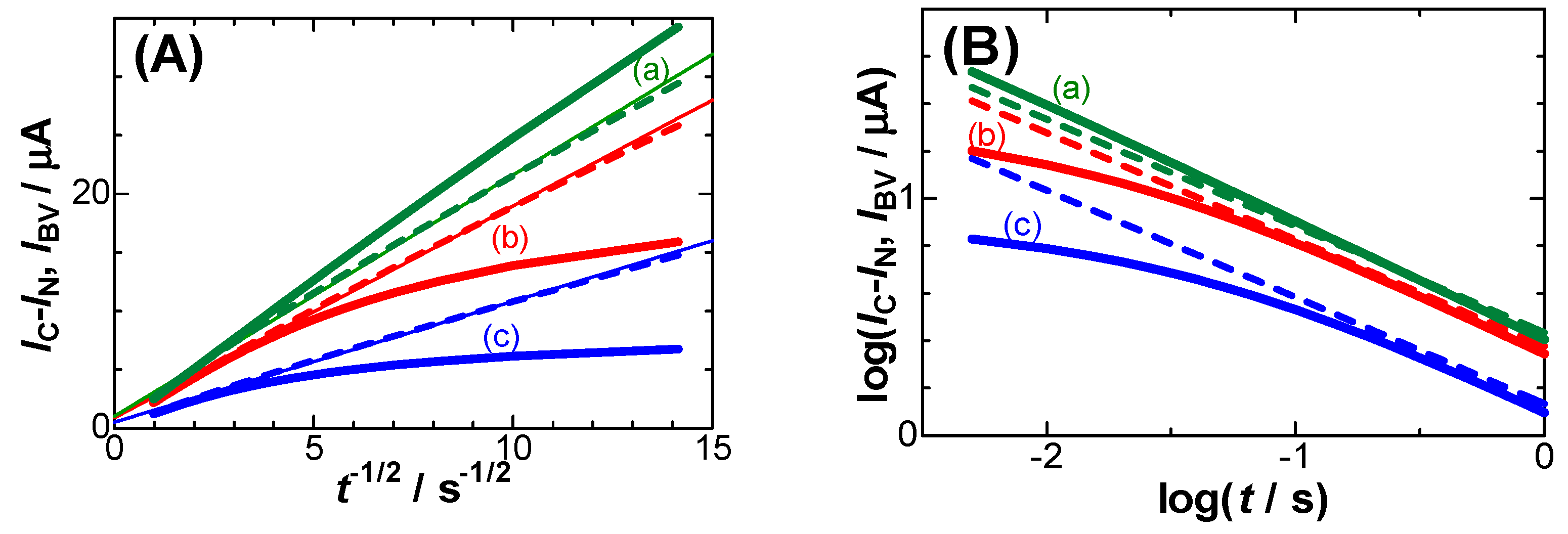
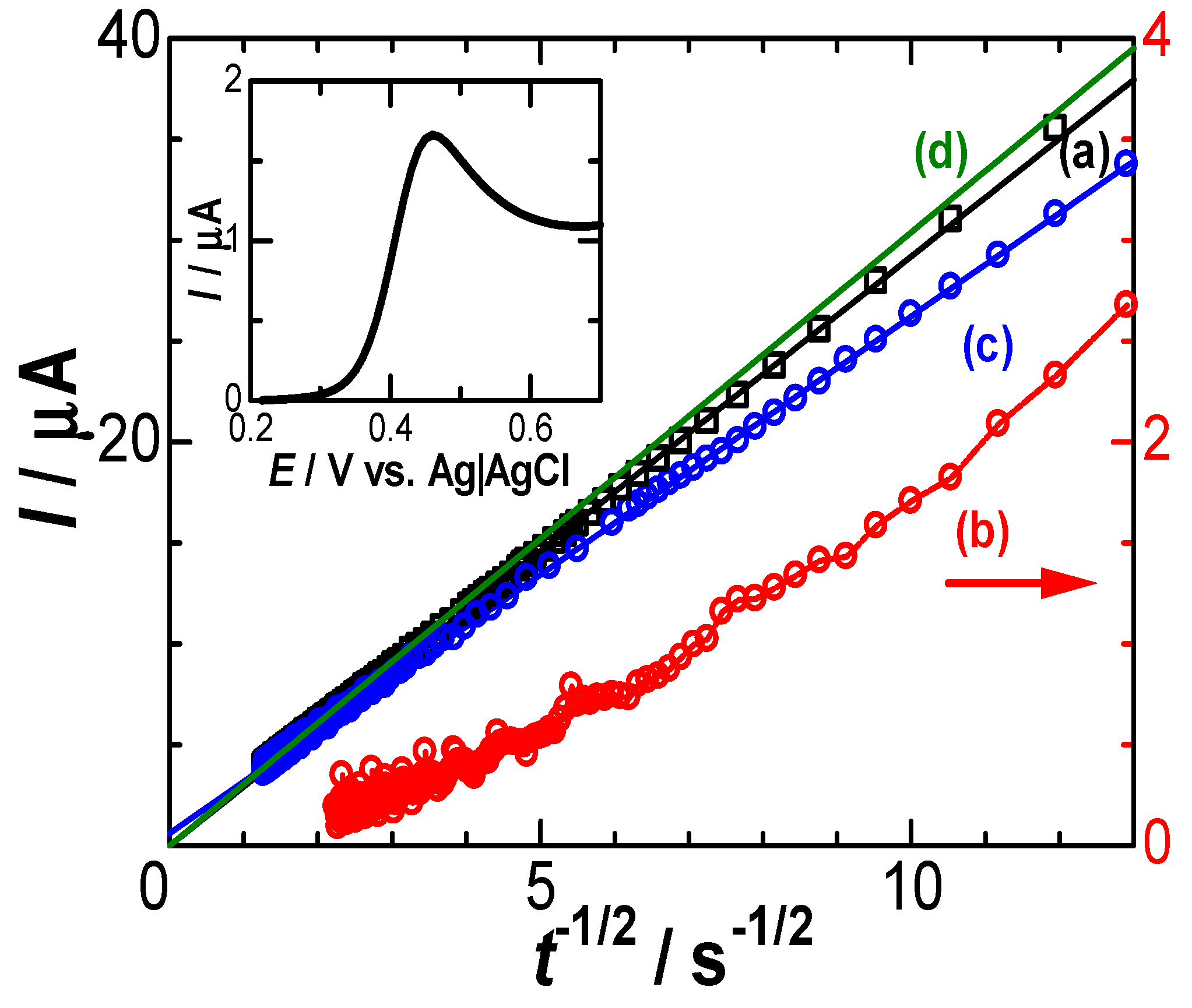
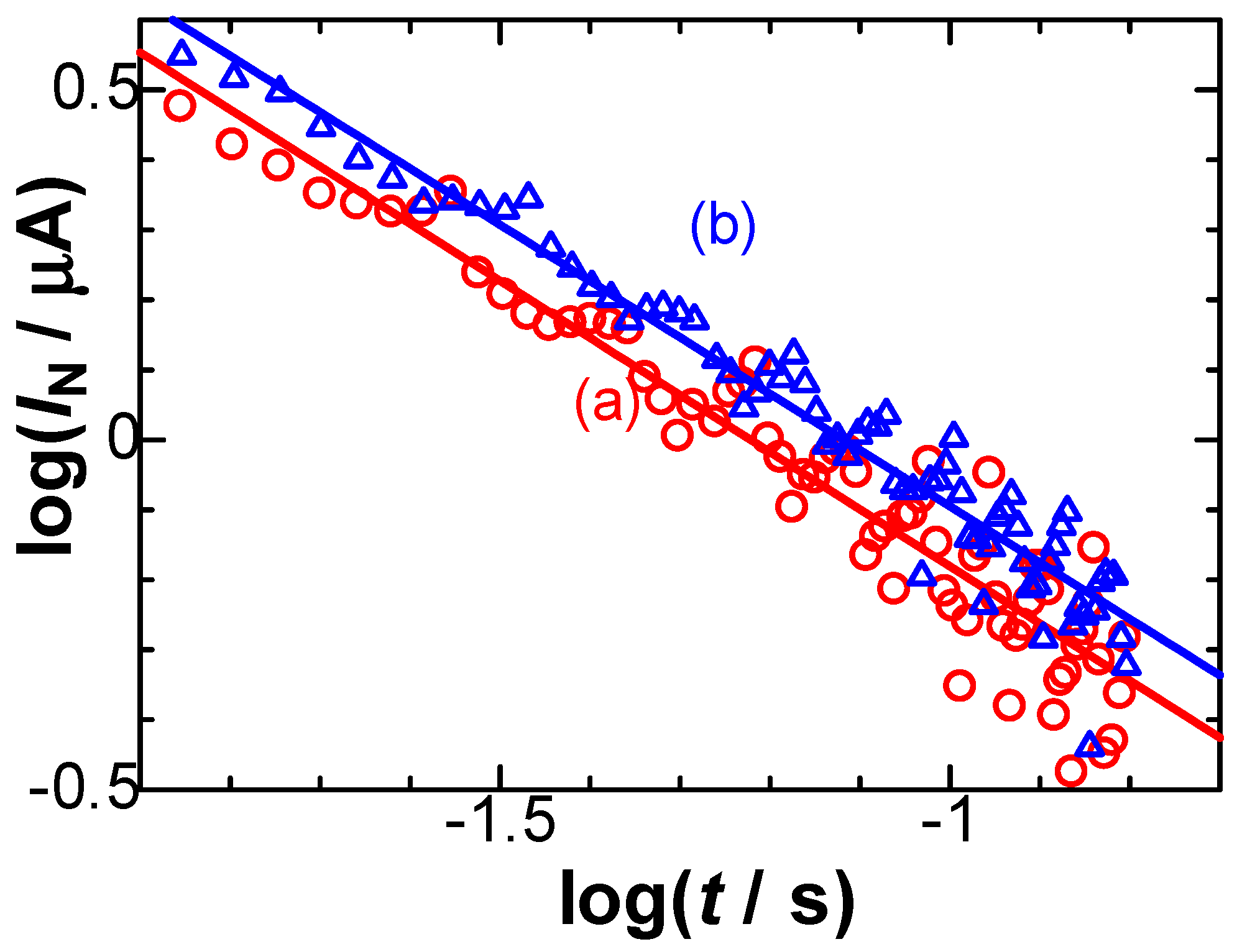
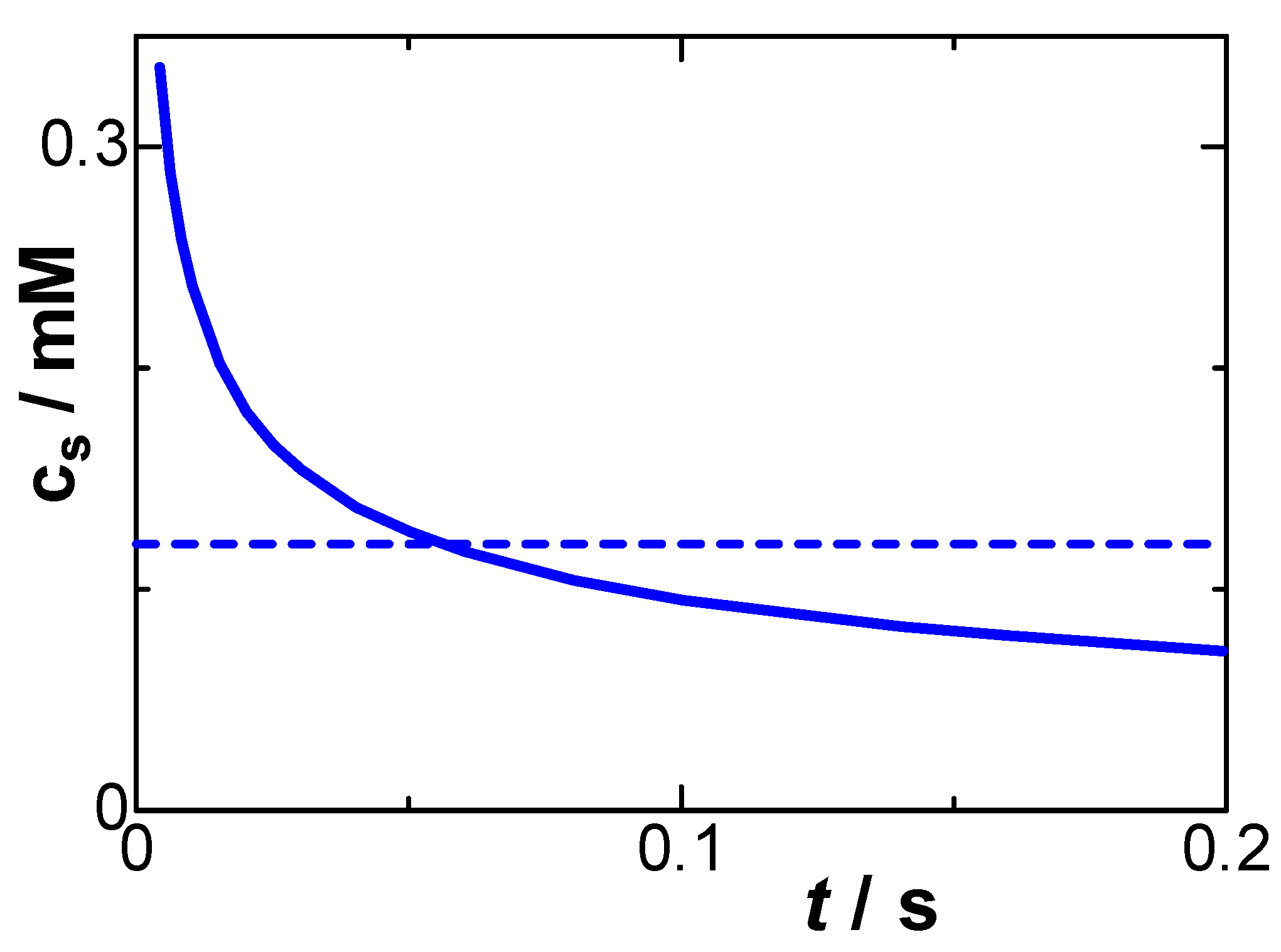
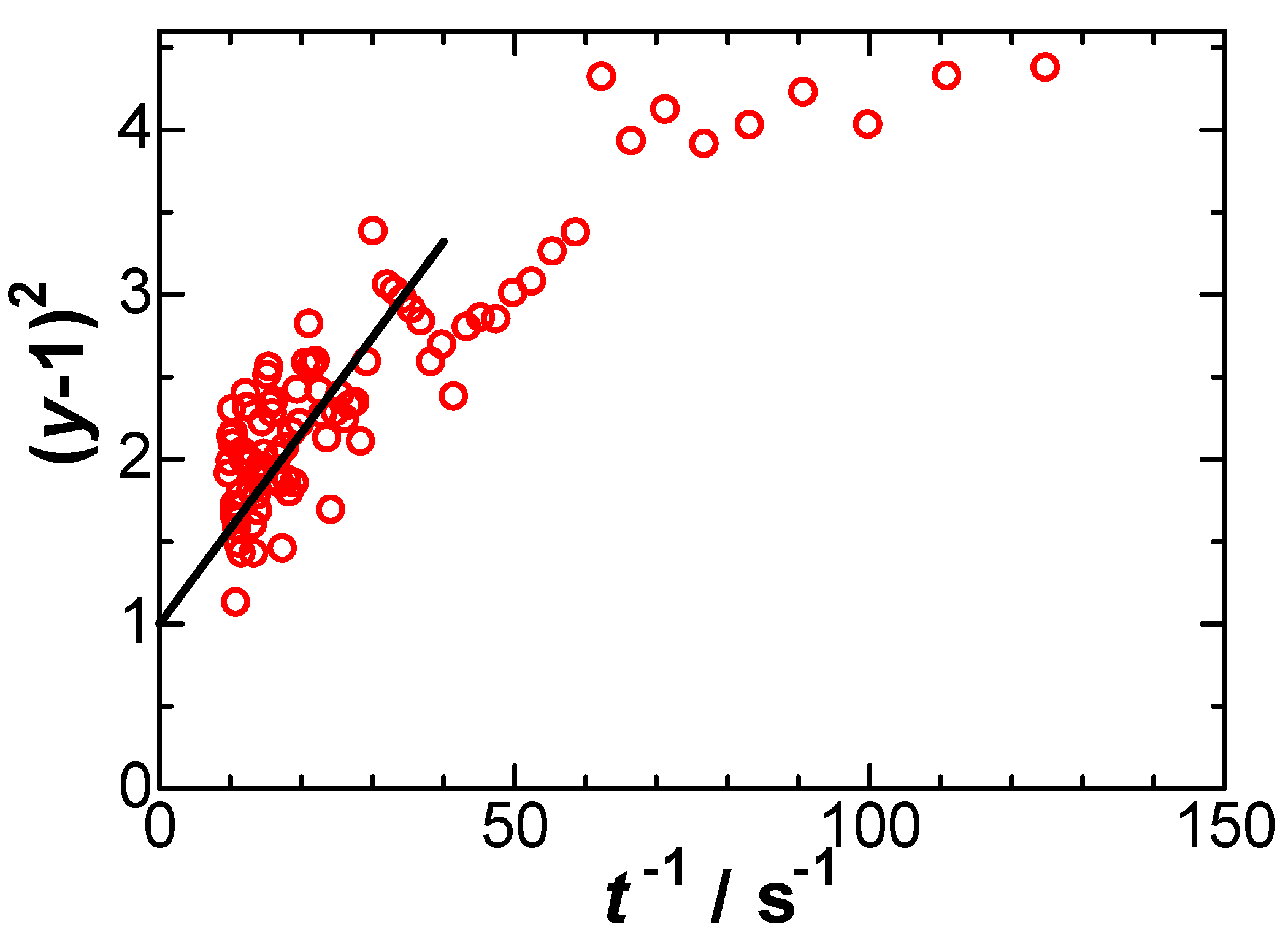
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Conflicts of Interest
References
- Gerhardt, R. Impedance Spectroscopy and Mobility Spectra. In Encyclopedia of Condensed Matter Physics; Elsevier: Amsterdam, The Netherlands, 2005; pp. 350–363. [Google Scholar] [CrossRef]
- Frolov, D.S.; Zubkov, V.I. Frequency dispersion of capacitance–voltage characteristics in wide bandgap semiconductor-electrolyte junctions. Semicond. Sci. Technol. 2016, 31, 125013. [Google Scholar] [CrossRef]
- Lasia, A. Modern Aspects of Electrochemistry, 32; White, R.E., Conway, B.E., Bockris, J.O.’M., Eds.; Kluwer Academic/Plenum Publishers: New York, NY, USA, 1999; p. 143. [Google Scholar]
- Brug, G.J.; Van Den Eeden, A.L.G.; Sluyters-Rehbach, M.; Sluyters, J.H. The analysis of electrode impedances complicated by the presence of a constant phase element. J. Electroanal. Chem. Interfacial. Electrochem. 1984, 176, 275–295. [Google Scholar] [CrossRef]
- Nyikos, L.; Pajkossy, T. Fractal dimension and fractional power frequency-dependent impedance of blocking electrodes. Electrochim. Acta 1985, 30, 1533–1540. [Google Scholar] [CrossRef]
- Zoltowski, P. On the electrical capacitance of interfaces exhibiting constant phase element behaviour. J. Electroanal. Chem. 1998, 443, 149–154. [Google Scholar] [CrossRef]
- Schalenbach, M.; Durmus, Y.E.; Robinson, S.A.; Tempel, H.; Kungl, H.; Eichel, R.-A. Physicochemical Mechanisms of the Double-Layer Capacitance Dispersion and Dynamics: An Impedance Analysis. J. Phys. Chem. C 2021, 125, 5870–5879. [Google Scholar] [CrossRef]
- Hou, Y.; Aoki, K.J.; Chen, J.; Nishiumi, T. Solvent Variables Controlling Electric Double Layer Capacitance at the Metal–Solution Interface. J. Phys. Chem. C 2014, 118, 10153–10158. [Google Scholar] [CrossRef]
- Aoki, K.; Hou, Y.; Chen, J.; Nishiumi, T. Resistance associated with measurements of capacitance in electric double layers. J. Electroanal. Chem. 2012, 689, 124–129. [Google Scholar] [CrossRef]
- Aoki, K.J.; Chen, J. Effects of the dipolar double layer on elemental electrode processes at micro- and macro-interfaces. Faraday Discuss. 2018, 210, 219–234. [Google Scholar] [CrossRef]
- Schalenbach, M.; Durmus, Y.E.; Tempel, H.; Kungl, H.; Eichel, R.-A. Double layer capacitances analysed with impedance spectroscopy and cyclic voltammetry: Validity and limits of the constant phase element parameterization. Phys. Chem. Chem. Phys. 2021, 23, 21097–21105. [Google Scholar] [CrossRef]
- Atkins, P.; de Paula, J. Physical Chemistry, 10th ed.; Oxford University Press: Oxford, UK, 2014; pp. 664–665. [Google Scholar]
- Chen, Z.; Wasson, M.C.; Drout, R.J.; Robison, L.; Idrees, K.B.; Knapp, J.G.; Son, F.A.; Zhang, X.; Hierse, W.; Kühn, C.; et al. The state of the field: From inception to commercialization of metal–organic frameworks. Faraday Discuss. 2020, 225, 9–69. [Google Scholar] [CrossRef]
- Kikuchi, R. A Theory of Cooperative Phenomena. Phys. Rev. 1951, 81, 988–1003. [Google Scholar] [CrossRef]
- Aoki, K.J. Molecular interaction model for frequency-dependence of double layer capacitors. Electrochim. Acta 2016, 188, 545–550. [Google Scholar] [CrossRef]
- Griffiths, D.J. Introduction to Electrodynamics, 2nd ed.; Prentice Hall: Hoboken, NJ, USA, 1989; pp. 158–164. [Google Scholar]
- Aoki, K.J.; Chen, J.; Zeng, X.; Wang, Z. Decrease in the double layer capacitance by faradaic current. RSC Adv. 2017, 7, 22501–22509. [Google Scholar] [CrossRef]
- Aoki, K.J.; Chen, J.; Tang, P. Capacitive Currents Flowing in the Direction Opposite to Redox Currents. J. Phys. Chem. C 2018, 122, 16727–16732. [Google Scholar] [CrossRef]
- Aoki, K.J.; Chen, J.; Wang, R. Stripped Charge of Ag Less than Deposited one Owing to Negative Capacitance Caused by Redox Reactions. Electroanalysis 2019, 31, 2303–2310. [Google Scholar] [CrossRef]
- Tang, P.; Aoki, K.J.; Chen, J. Reduction Charge Smaller than the Deposited One in Cathodic Stripping Voltammograms of AgCl. Am. J. Anal. Chem. 2019, 10, 286–295. [Google Scholar] [CrossRef]
- Aoki, K.J.; Chen, J.; Liu, Y.; Jia, B. Peak potential shift of fast cyclic voltammograms owing to capacitance of redox reactions. J. Electroanal. Chem. 2020, 856, 113609. [Google Scholar] [CrossRef]
- Aoki, K.J.; Taniguchi, S.; Chen, J. Participation in Negative Capacitance of Diffusion-Controlled Voltammograms of Hemin. ACS Omega 2020, 5, 29447–29452. [Google Scholar] [CrossRef]
- Liu, Y.; Aoki, K.J.; Chen, J. A Loss of Charge at Reduction of Hydrogen Ion by Fast Scan Voltammetry. J. Electrochem. Soc. 2022, 169, 036510. [Google Scholar] [CrossRef]
- Atkins, P.; de Paula, J. Atkins’ Physical Chemistry, 10th ed.; Oxford University Press: Oxford, UK, 2014; pp. 894–901. [Google Scholar]
- Compton, R.G.; Banks, C.E. Understanding Voltammetry, 2nd ed.; Imperial College Press: London, UK, 2010. [Google Scholar]
- Weaver, M.J. Redox reactions at metal–solution interfaces. Weaver, M.J. In Electrode Kinetics: Reactions; Compton, R.G., Ed.; Elsevier Science: Amsterdam, The Netherlands, 1988; Chapter 1. [Google Scholar]
- Marcus, R.; Sutin, N. Electron transfers in chemistry and biology. Biochim. Biophys. Acta 1985, 811, 265–322. [Google Scholar] [CrossRef]
- Hush, N. Electron transfer in retrospect and prospect 1: Adiabatic electrode processes. J. Electroanal. Chem. 1999, 470, 170–195. [Google Scholar] [CrossRef]
- Fawcett, W.R.; Opallo, M. The Kinetics of Heterogeneous Electron Transfer Reaction in Polar Solvents. Chem. Int. Ed. Engl. 1994, 33, 2131–2143. [Google Scholar] [CrossRef]
- Henstridge, M.C.; Laborda, E.; Wang, Y.; Suwatchara, D.; Rees, N.; Molina, A.; Martínez-Ortiz, F.; Compton, R.G. Giving physical insight into the Butler–Volmer model of electrode kinetics: Application of asymmetric Marcus–Hush theory to the study of the electroreductions of 2-methyl-2-nitropropane, cyclooctatetraene and europium(III) on mercury microelectrodes. J. Electroanal. Chem. 2012, 672, 45–52. [Google Scholar] [CrossRef]
- Clegg, A.D.; Rees, N.; Klymenko, O.; Coles, B.A.; Compton, R.G. Marcus theory of outer-sphere heterogeneous electron transfer reactions: High precision steady-state measurements of the standard electrochemical rate constant for ferrocene derivatives in alkyl cyanide solvents. J. Electroanal. Chem. 2005, 580, 78–86. [Google Scholar] [CrossRef]
- Aoki, K.J.; Zhang, C.; Chen, J.; Nishiumi, T. Heterogeneous reaction rate constants by steady-state microelectrode techniques and fast scan voltammetry. J. Electroanal. Chem. 2013, 706, 40–47. [Google Scholar] [CrossRef]
- Henstridge, M.C.; Laborda, E.; Rees, N.V.; Compton, R.G. Marcus–Hush–Chidsey theory of electron transfer applied to voltammetry: A review. Electrochim. Acta 2012, 84, 12–20. [Google Scholar] [CrossRef]
- Opałło, M. The solvent effect on the electro-oxidation of 1,4-phenylenediamine. The influence of the solvent reorientation dynamics on the one-electron transfer rate. Chem. Soc. Faraday Trans. I 1986, 82, 339–347. [Google Scholar] [CrossRef]
- Alexandrov, I. Physical aspects of charge transfer theory. Chem. Phys. 1980, 51, 449–457. [Google Scholar] [CrossRef]
- Calef, D.F.; Wolynes, P.G. Classical solvent dynamics and electron transfer. 1. Continuum theory. J. Phys. Chem. 1983, 87, 3387–3400. [Google Scholar] [CrossRef]
- Weaver, M.J. Dynamical solvent effects on activated electron-transfer reactions: Principles, pitfalls, and progress. Chem. Rev. 1992, 92, 463–480. [Google Scholar] [CrossRef]
- Clegg, A.D.; Rees, N.V.; Klymenko, O.V.; Coles, B.A.; Compton, R.G. Marcus Theory of Outer-Sphere Heterogeneous Electron Transfer Reactions: Dependence of the Standard Electrochemical Rate Constant on the Hydrodynamic Radius from High Precision Measurements of the Oxidation of Anthracene and Its Derivatives in Nonaqueous Solvents Using the High-Speed Channel Electrode. J. Am. Chem. Soc. 2004, 126, 6185–6192. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Yang, H.; Bard, A.J. Variation of the heterogeneous electron-transfer rate constant with solution viscosity: Reduction of aqueous solutions of [(EDTA)chromium(III)]- at a mercury electrode. J. Am. Chem. Soc. 1987, 109, 1916–1920. [Google Scholar] [CrossRef]
- Aoki, K.J.; Chen, J.; He, R. Potential Step for Double-Layer Capacitances Obeying the Power Law. ACS Omega 2020, 5, 7497–7502. [Google Scholar] [CrossRef] [PubMed]
- Hou, Y.; Aoki, K.J.; Chen, T.N.J. Invariance of Double Layer Capacitance to Polarized Potential in Halide Solutions. Univers. J. Chem. 2013, 1, 162–169. [Google Scholar] [CrossRef]
- Matsuda, H.; Ayabe, Y.; Elekt, Z. The theory of the cathode-ray polarography of Randles-Sevcik. Phys. Chem. 1955, 59, 494–503. [Google Scholar]
- Abramowitz, M.; Stegun, I.A. Handbook of mathematical functions, National Bureau of Standards. In Applied Mathematics Series—55; Tenth Printing, Eq. (6.2.1), Eq. (6.1.35), Eq. (7.1.13); National Institute of Informatics: Tokyo, Japan, 1972; pp. 257–289. [Google Scholar]
- Osteryoung, R.A.; Osteryoung, J.; Albery, W.J.; Rogers, G.T. Pulse voltammetric methods of analysis. Phisl. Trans. R. Lond. A 1981, 302, 315–326. [Google Scholar] [CrossRef]
- Lovrić, M.; Osteryoung, J. Theory of differential normal pulse voltammetry. Electrochim. Acta 1982, 27, 963–968. [Google Scholar] [CrossRef]
- Stojek, Z. Pulse Voltammetry. In Electroanalytical Methods; Springer: Berlin/Heidelberg, Germany, 2005; pp. 99–110. [Google Scholar] [CrossRef]
- Bard, A.J.; Faulkner, L.R. Electrochemical Methods, 2nd ed.; Kohn Wiley & Sons: New York, NY, USA, 2001; pp. 191–192. [Google Scholar]
- Aoki, K.J.; Chen, J. Tips of Voltammetry. In Voltammetry; IntechOpen: London, UK, 2018; pp. 1–19. [Google Scholar] [CrossRef]
- Aoki, K.; Honda, K.; Tokuda, K.; Matsuda, H. Voltammetry at microcylinder electrodes. Part II. Chronoamperometry. J. Electroanal. Chem. Interfacial Electrochem. 1985, 186, 79–86. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.; Aoki, K.J.; Chen, J. Similarity of Heterogeneous Kinetics to Delay of Double-Layer Capacitance Using Chronoamperometry. Electrochem 2023, 4, 301-312. https://doi.org/10.3390/electrochem4020021
Liu Y, Aoki KJ, Chen J. Similarity of Heterogeneous Kinetics to Delay of Double-Layer Capacitance Using Chronoamperometry. Electrochem. 2023; 4(2):301-312. https://doi.org/10.3390/electrochem4020021
Chicago/Turabian StyleLiu, Yuanyuan, Koichi Jeremiah Aoki, and Jingyuan Chen. 2023. "Similarity of Heterogeneous Kinetics to Delay of Double-Layer Capacitance Using Chronoamperometry" Electrochem 4, no. 2: 301-312. https://doi.org/10.3390/electrochem4020021
APA StyleLiu, Y., Aoki, K. J., & Chen, J. (2023). Similarity of Heterogeneous Kinetics to Delay of Double-Layer Capacitance Using Chronoamperometry. Electrochem, 4(2), 301-312. https://doi.org/10.3390/electrochem4020021







