Stiction and Friction of Nano- and Microtextured Liquid Silicon Rubber Surface Formed by Injection Molding
Abstract
1. Introduction
- (A)
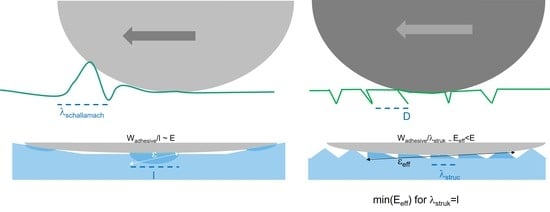
- Is the elastic length l of the interface crack suitable to separate the friction behaviors of nano- and microstructured surfaces?
- (B)
- Can these friction modulations be generated in dry or lubricated conditions?
- (C)
- Can anisotropic friction be generated at 90° or 180°?
- (D)
- What is the effect of structuring the mean distance lstruc with respect to lschallamach on the magnitude of the coefficient of friction?
- (E)
- Can the friction coefficient modulations be brought into interference with the hypothetical tendency to form Schallamach waves, and what is the effect of this as a function of the sliding speed?
- Isotropic and anisotropic statistical nanostructures via pyramids and rodlike structures;
- Isotropic and anisotropic microstructures produced via polishing with pyramid nanostructures;
- Linear groove structures for defined geometric structures with pyramid nanostructures;
- A molded skin of the California king snake as a biologically motivated structure.
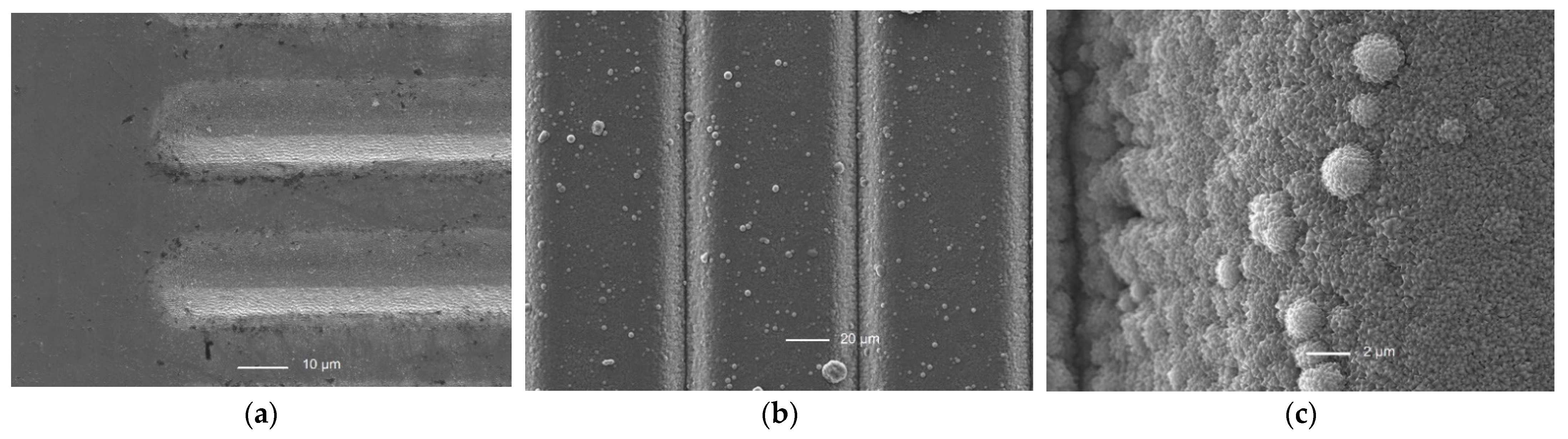
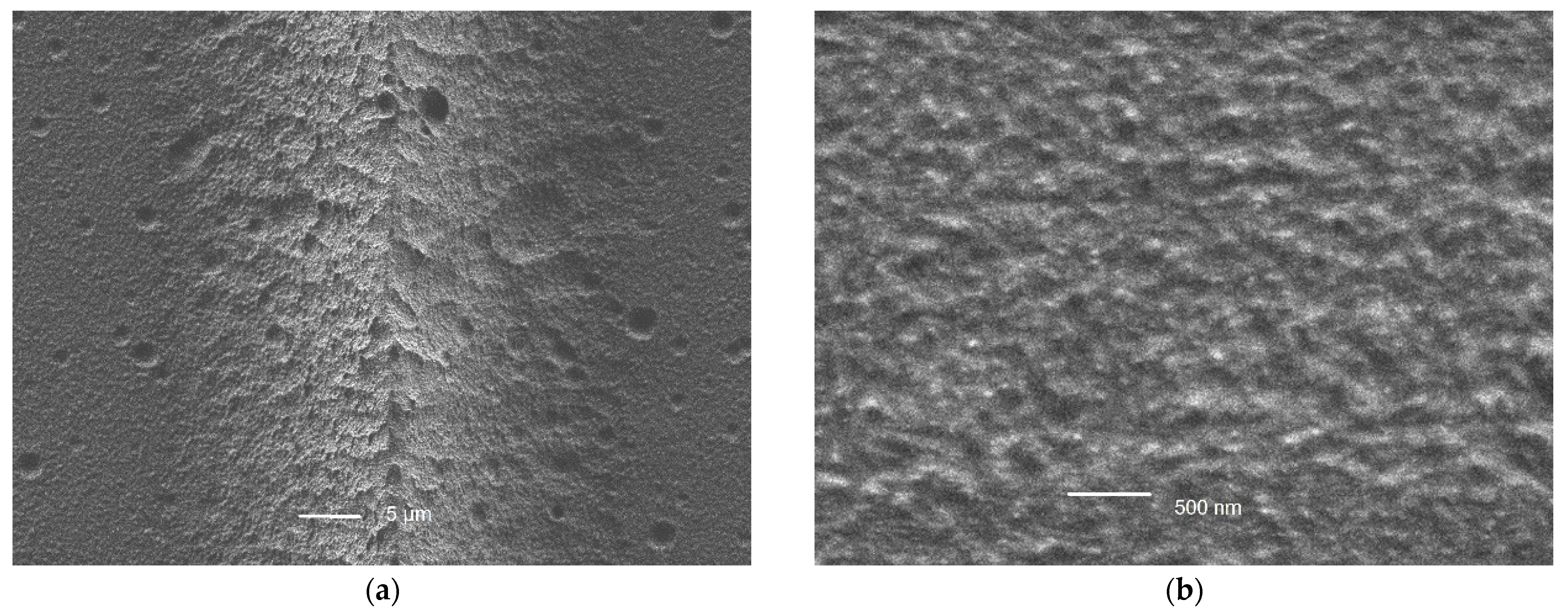
2. Materials and Methods
2.1. Materials
2.2. Tribological Experiments
2.3. Contact Angle Measurements
3. Results
3.1. Energies
3.2. Friction Measurements
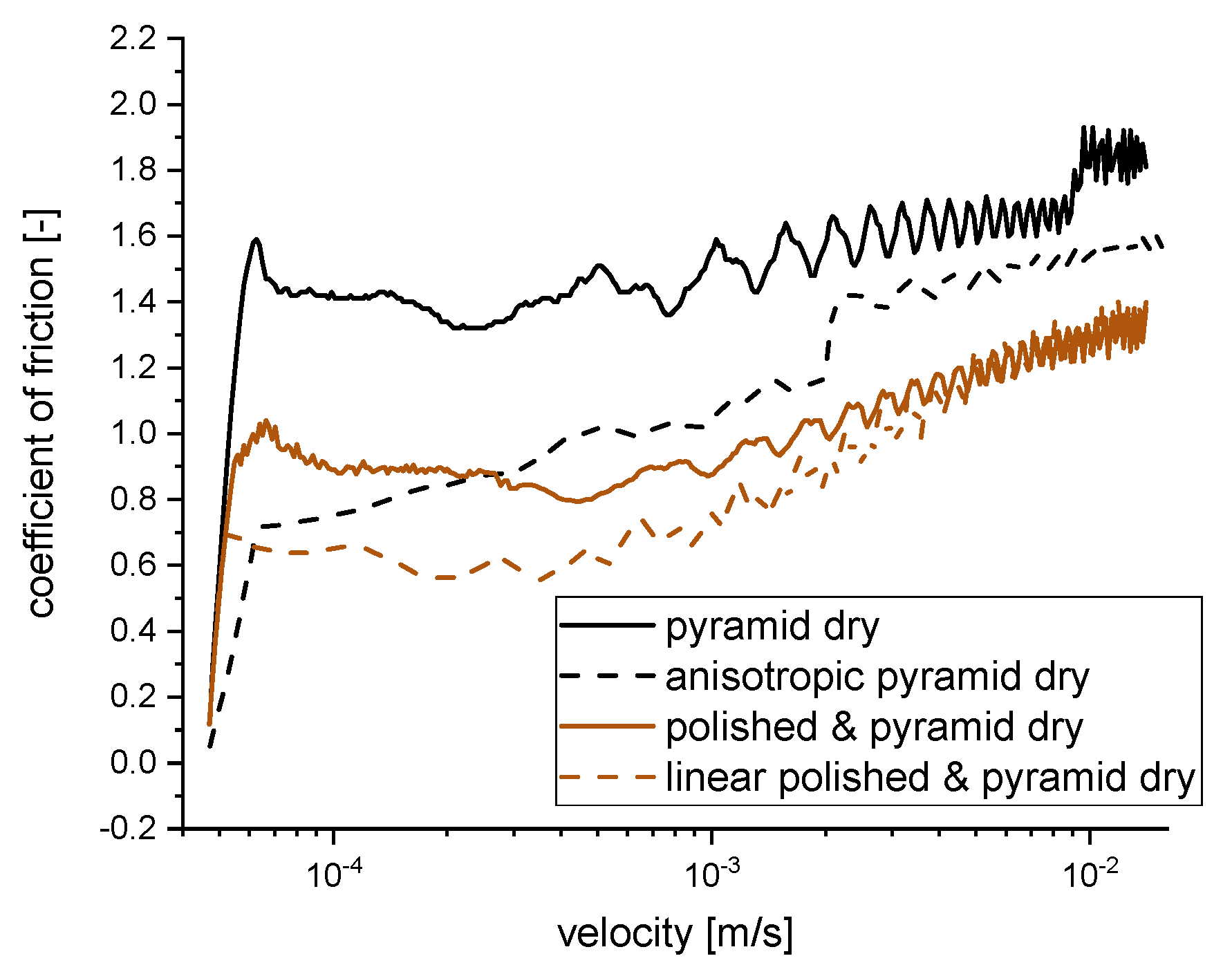
3.2.1. General Friction Data
3.2.2. Is the Elastic Length l of the Interface Crack Suitable to Separate the Friction Behavior of Nano- and Microstructured Surfaces?
- Isotropic and anisotropic pyramid nanostructures;
- A pyramid nanostructure on a mirrorlike surface and on a ground surface;
- An isotropic ground surface and a linear and thus anisotropic ground surface.
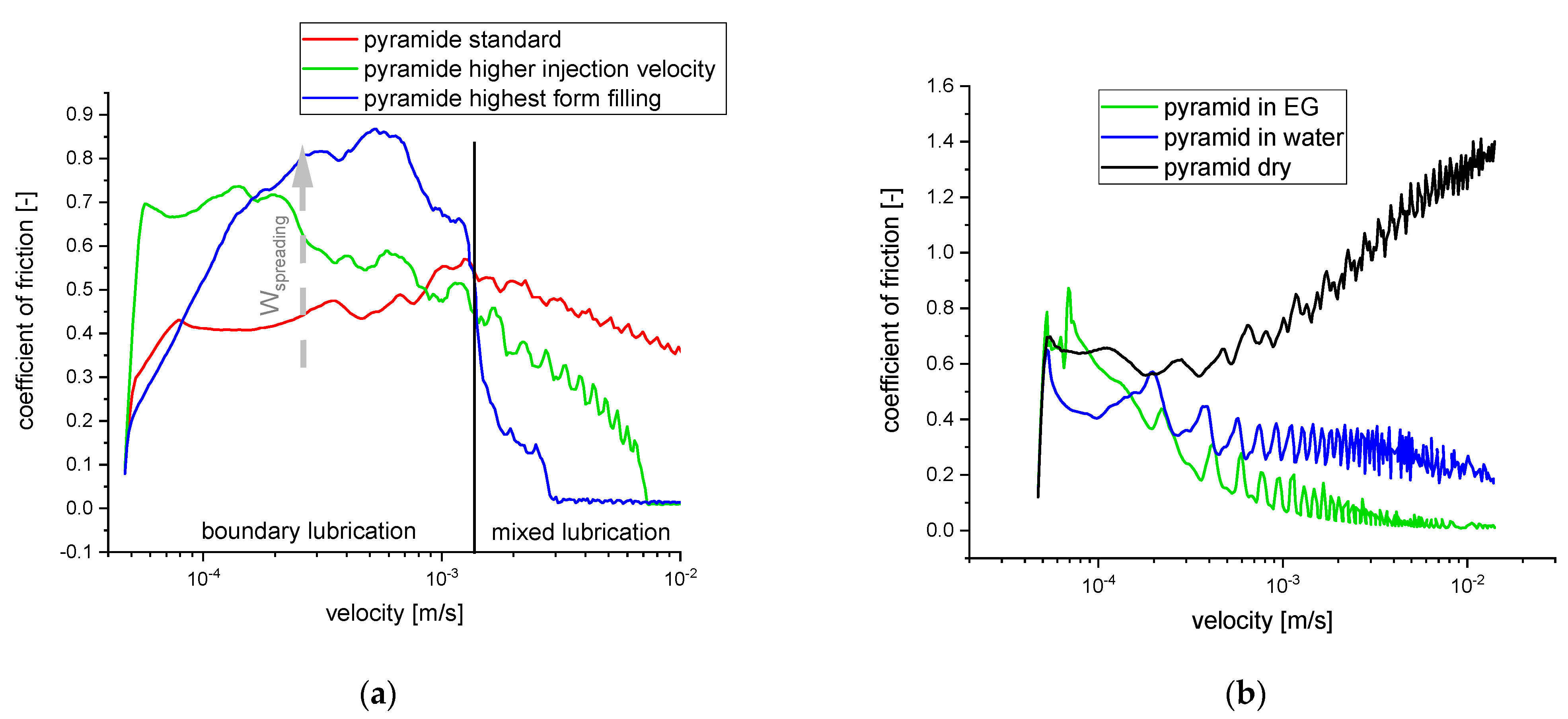
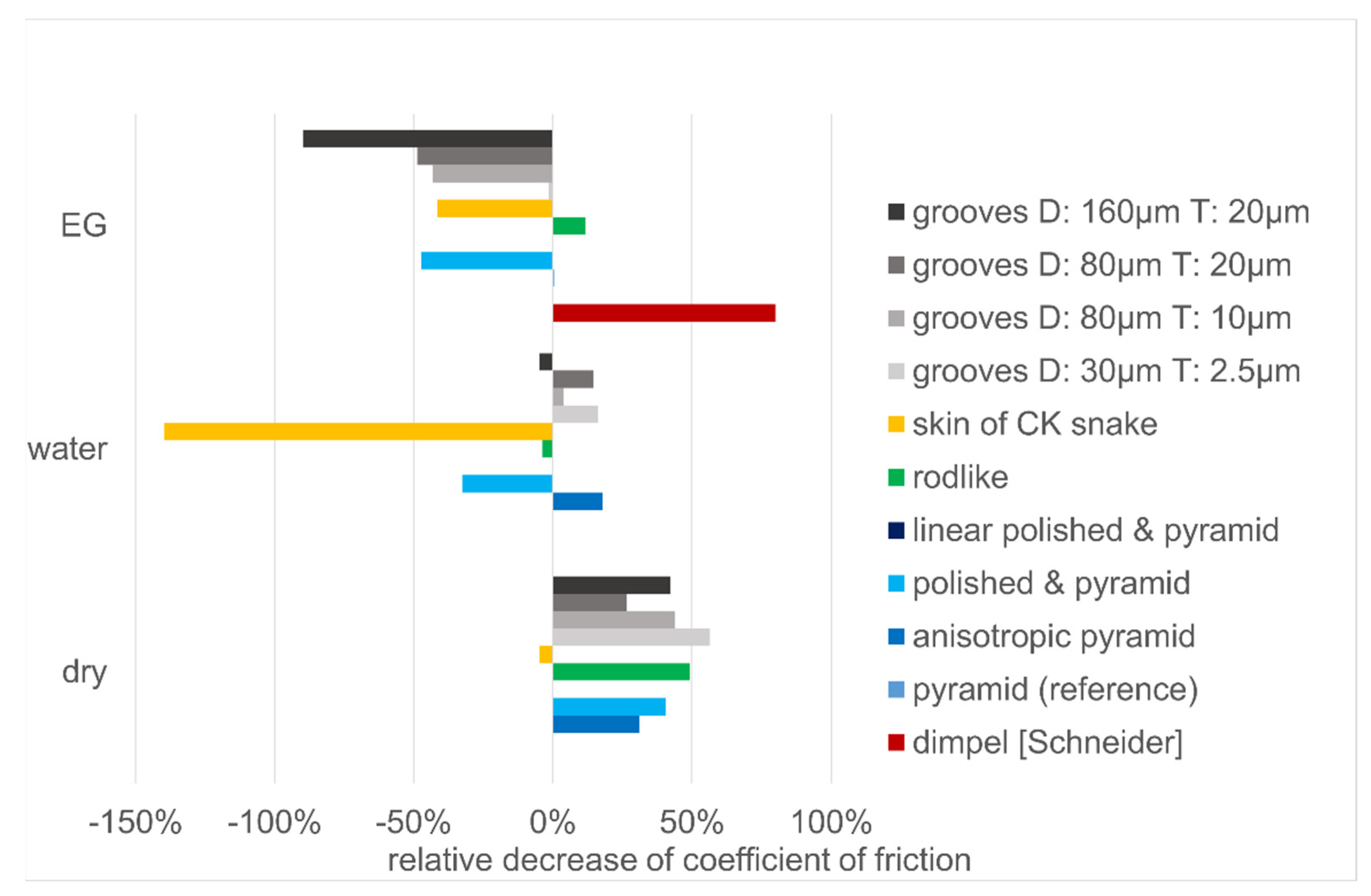
3.2.3. Can These Friction Modulations Be Generated in Dry or Lubricated Conditions?
3.2.4. What Effect on Friction Can Be Expected for Different Structures?
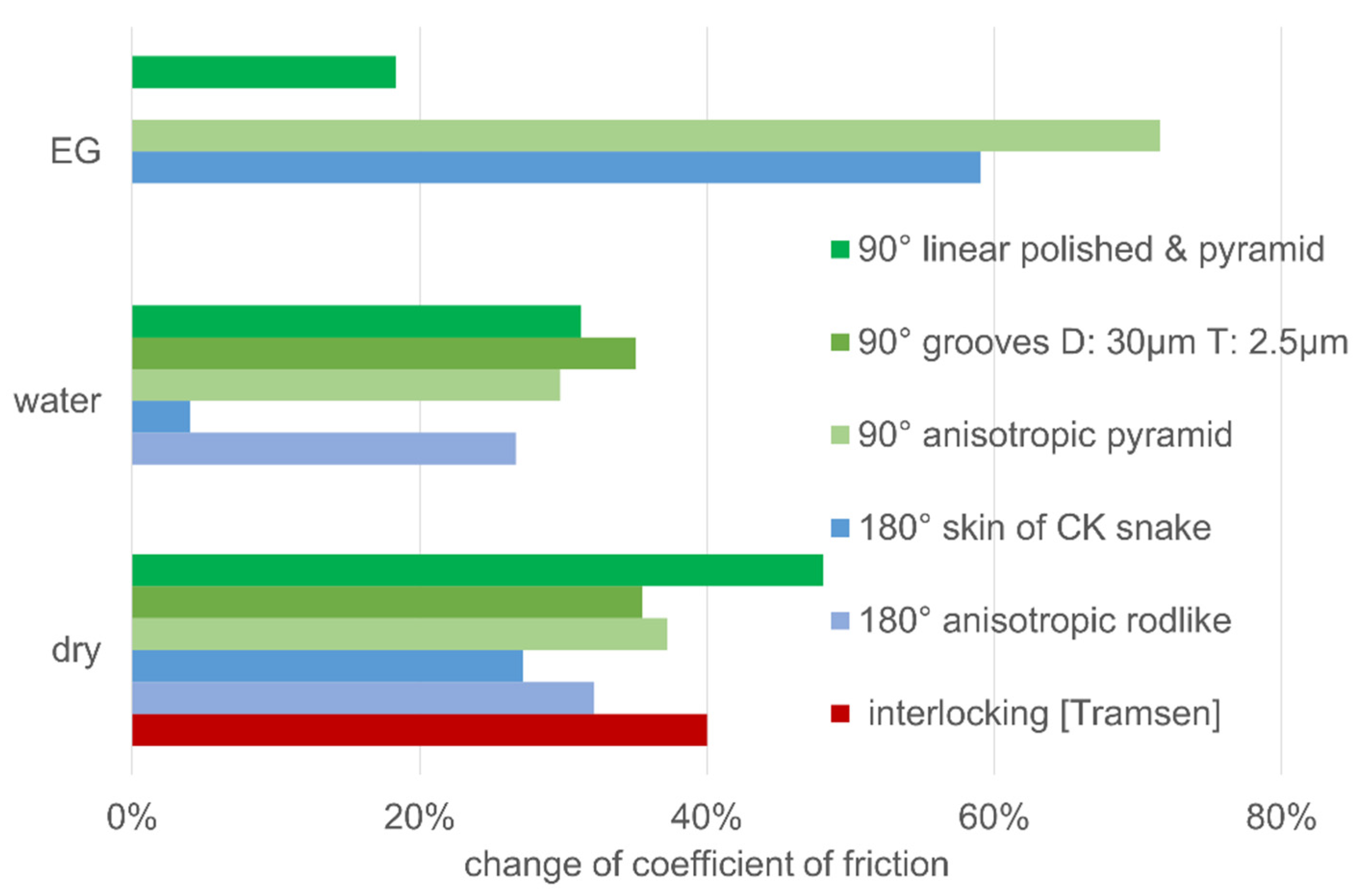
3.2.5. Can Anisotropic Friction Be Generated at 90° or 180°?
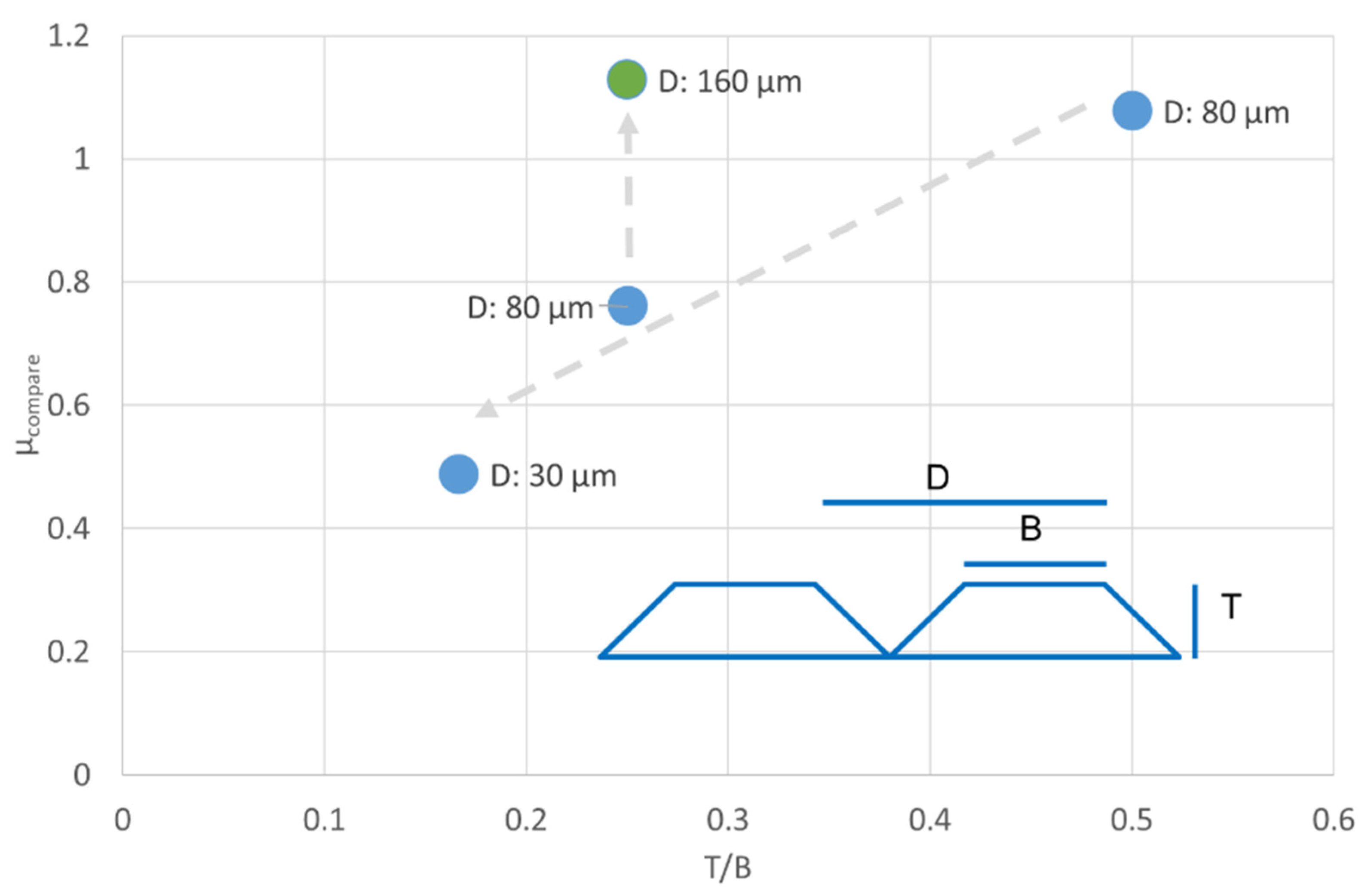
3.2.6. Friction of Periodic Microstructure Grooves
- (A)
- What is the effect of structuring the mean distance lstruc with respect to lschallmach on the magnitude of the coefficient of friction?
- (B)
- Can the friction coefficient modulations be brought into interference with the hypothetical tendency to form Schallamach waves, and what is the effect of this as a function of the sliding velocity?
4. Discussion
5. Conclusions
- “Disturbing” the isotropic growth process by skewing the substrate in the process;
- Choosing a layer material with, e.g., an anisotropic layer structure;
- Pre-structuring the substrate (e.g., by grinding and polishing).
- Can increase the adhesive friction of surfaces during sliding;
- Increase the wetting tendency of liquids and decrease adhesive friction.
- Increase the movement amplitude of stick-slip and decrease dry friction;
- Absorb shear deformation at low velocities;
- Reduce film formation during lubrication and increase friction.
- Enable frictional anisotropy.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Schallamach, A. How does rubber slide? Wear 1971, 17, 301–312. [Google Scholar] [CrossRef]
- Von Goeldel, S.; Reichenbach, T.; König, F.; Mayrhofer, L.; Moras, G.; Jacobs, G.; Moseler, M. A Combined Experimental and Atomistic Investigation of PTFE Double Transfer Film Formation and Lubrication in Rolling Point Contacts. Tribol. Lett. 2021, 69, 1–16. [Google Scholar] [CrossRef]
- Burris, D.L.; Sawyer, W.G. Improved wear resistance in alumina-PTFE nanocomposites with irregular shaped nanoparticles. Wear 2006, 260, 915–918. [Google Scholar] [CrossRef]
- Vogel, S.; Brenner, A.; Schlüter, B.; Blug, B.; Kirsch, F.; van Roo, T. Laser Structuring and DLC Coating of Elastomers for High Performance Applications; Universitätsbibliothek der RWTH Aachen: Aachen, Germany, 2022. [Google Scholar]
- Grützmacher, P.G.; Profito, F.J.; Rosenkranz, A. Multi-Scale Surface Texturing in Tribology—Current Knowledge and Future Perspectives. Lubricants 2019, 7, 95. [Google Scholar] [CrossRef]
- Ripoll, M.R.; Podgornik, B.; Vižintin, J. Finite element analysis of textured surfaces under reciprocating sliding. Wear 2011, 271, 952–959. [Google Scholar] [CrossRef]
- Liu, Y.; Li, J.; Yi, S.; Ge, X.; Chen, X.; Luo, J. Enhancement of friction performance of fluorinated graphene and molybdenum disulfide coating by microdimple arrays. Carbon 2020, 167, 122–131. [Google Scholar] [CrossRef]
- Tramsen, H.T.; Gorb, S.N.; Zhang, H.; Manoonpong, P.; Dai, Z.; Heepe, L. Inversion of friction anisotropy in a bio-inspired asymmetrically structured surface. J. R. Soc. Interface 2018, 15, 20170629. [Google Scholar] [CrossRef]
- Tramsen, H.T.; Heepe, L.; Homchanthanakul, J.; Wörgötter, F.; Gorb, S.N.; Manoonpong, P. Getting grip in changing environments: The effect of friction anisotropy inversion on robot locomotion. Appl. Phys. A 2021, 127, 1–9. [Google Scholar] [CrossRef]
- Scherge, M.; Gorb, S. Biological Micro-and Nanotribology: Nature’s Solutions; Springer: Berlin, Germany; London, UK, 2011. [Google Scholar]
- Wu, W.; Lutz, C.; Mersch, S.; Thelen, R.; Greiner, C.; Gomard, G.; Hölscher, H. Characterization of the microscopic tribological properties of sandfish (Scincus scincus) scales by atomic force microscopy. Beilstein J. Nanotechnol. 2018, 9, 2618–2627. [Google Scholar] [CrossRef]
- Schneider, J.; Djamiykov, V.; Greiner, C. Friction reduction through biologically inspired scale-like laser surface textures. Beilstein J. Nanotechnol. 2018, 9, 2561–2572. [Google Scholar] [CrossRef]
- Fukahori, Y.; Sakulkaew, K.; Busfield, J. Elastic–viscous transition in tear fracture of rubbers. Polymer 2013, 54, 1905–1915. [Google Scholar] [CrossRef]
- Wu-Bavouzet, F.; Clain-Burckbuchler, J.; Buguin, A.; De Gennes, P.-G.; Brochard-Wyart, F. Stick-Slip: Wet Versus Dry. J. Adhes. 2007, 83, 761–784. [Google Scholar] [CrossRef]
- Fukahori, Y.; Gabriel, P.; Busfield, J. How does rubber truly slide between Schallamach waves and stick–slip motion? Wear 2010, 269, 854–866. [Google Scholar] [CrossRef]
- Rand, C.J.; Crosby, A.J. Insight into the periodicity of Schallamach waves in soft material friction. Appl. Phys. Lett. 2006, 89, 261907. [Google Scholar] [CrossRef]
- Rand, C.J.; Crosby, A.J. Friction of soft elastomeric wrinkled surfaces. J. Appl. Phys. 2009, 106, 064913. [Google Scholar] [CrossRef]
- Schapery, R. A model for the prediction of rubber friction with Schallamach waves. Tribol. Int. 2019, 143, 106018. [Google Scholar] [CrossRef]
- Israelachvili, J.N. Intermolecular and Surface Forces; Elsevier: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Martin, P.; Brochard-Wyart, F. Dewetting at Soft Interfaces. Phys. Rev. Lett. 1998, 80, 3296–3299. [Google Scholar] [CrossRef]
- Ucar, H.; Basdogan, I. Dynamic characterization and modeling of rubber shock absorbers: A comprehensive case study. J. Low Freq. Noise, Vib. Act. Control 2017, 37, 509–518. [Google Scholar] [CrossRef]
- Battermann, W.; Köhler, R. Elastomere Federung, Elastische Lagerungen: Grundlagen Ingenieurmässiger Berechnungund Konstruktion; W. Ernst: Berlin, Germany, 1982. [Google Scholar]
- Zhibo, C.; Zhaoqian, S.; Dandan, H.; Genzong, L.; Jian, W.; Benlong, S.; Yuyan, L.; Youshan, W. From small wrinkles to Schallamach waves during rubber friction: In situ experiment and 3D simulation. Polym. Test. 2021, 96, 107084. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Dowson, D. Isothermal Elastohydrodynamic Lubrication of Point Contacts: Part 1—Theoretical Formulation. J. Lubr. Technol. 1976, 98, 223–228. [Google Scholar] [CrossRef]
- Anders, A. A structure zone diagram including plasma-based deposition and ion etching. Thin Solid Film. 2009, 518, 4087–4090. [Google Scholar] [CrossRef]
- Thornton, J.A. The microstructure of sputter-deposited coatings. J. Vac. Sci. Technol. A Vac. Surf. Film. 1986, 4, 3059–3065. [Google Scholar] [CrossRef]
- Burmeister, F.; Hagen, J.; Denter, J.; Wirth, M.; Fromm, A.; Kleer, G. Hybrid Inorganic-Organic Functional Coatings for Injection Molding Applications. Plasma Process. Polym. 2009, 6, S1–S5. [Google Scholar] [CrossRef]
- Weißer, D.F.; Walz, D.; Schmid, J.; Mayer, D.; Deckert, M.H. Calculating the temperature and degree of cross-linking for liquid silicone rubber processing in injection molding. J. Adv. Manuf. Process. 2020, 3, 13. [Google Scholar] [CrossRef]
- Abdel-Wahed, S.A.; Koplin, C.; Jaeger, R.; Scherge, M. On the Transition from Static to Dynamic Boundary Friction of Lubricated PEEK for a Spreading Adhesive Contact by Macroscopic Oscillatory Tribometry. Lubricants 2017, 5, 21. [Google Scholar] [CrossRef]
- Koplin, C.; Abdel-Wahed, S.A.; Jaeger, R.; Scherge, M. The Transition from Static to Dynamic Boundary Friction of a Lubricated Spreading and a Non-Spreading Adhesive Contact by Macroscopic Oscillatory Tribometry. Lubricants 2019, 7, 6. [Google Scholar] [CrossRef]
- Baum, M.J.; Heepe, L.; Fadeeva, E.; Gorb, S.N. Dry Friction of Microstructured Polymer Surfaces Inspired by Snake Skin; Gottfried Wilhelm Leibniz Universität Hannover; Technische Informationsbibliothek (TIB): Hannover, Germany, 2014. [Google Scholar]
- Putelat, T.; Dawes, J.H.; Willis, J.R. On the microphysical foundations of rate-and-state friction. J. Mech. Phys. Solids 2011, 59, 1062–1075. [Google Scholar] [CrossRef]








Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koplin, C.; Weißer, D.F.; Fromm, A.; Deckert, M.H. Stiction and Friction of Nano- and Microtextured Liquid Silicon Rubber Surface Formed by Injection Molding. Appl. Mech. 2022, 3, 1270-1287. https://doi.org/10.3390/applmech3040073
Koplin C, Weißer DF, Fromm A, Deckert MH. Stiction and Friction of Nano- and Microtextured Liquid Silicon Rubber Surface Formed by Injection Molding. Applied Mechanics. 2022; 3(4):1270-1287. https://doi.org/10.3390/applmech3040073
Chicago/Turabian StyleKoplin, Christof, Dennis F. Weißer, Alexander Fromm, and Matthias H. Deckert. 2022. "Stiction and Friction of Nano- and Microtextured Liquid Silicon Rubber Surface Formed by Injection Molding" Applied Mechanics 3, no. 4: 1270-1287. https://doi.org/10.3390/applmech3040073
APA StyleKoplin, C., Weißer, D. F., Fromm, A., & Deckert, M. H. (2022). Stiction and Friction of Nano- and Microtextured Liquid Silicon Rubber Surface Formed by Injection Molding. Applied Mechanics, 3(4), 1270-1287. https://doi.org/10.3390/applmech3040073