Passive-Tuned Mass Dampers for the Pointing Accuracy Mitigation of VLBI Earth-Based Antennae Subject to Aerodynamic Gust
Abstract
1. Introduction
2. Methodology
2.1. Optimization Framework
2.2. Structural Reduced Order Model
3. Application
3.1. Reduced Order Model and Normal Modes Analysis
3.2. Uncontrolled Dynamic Aeroelastic Response of the VLBI Antenna Structure
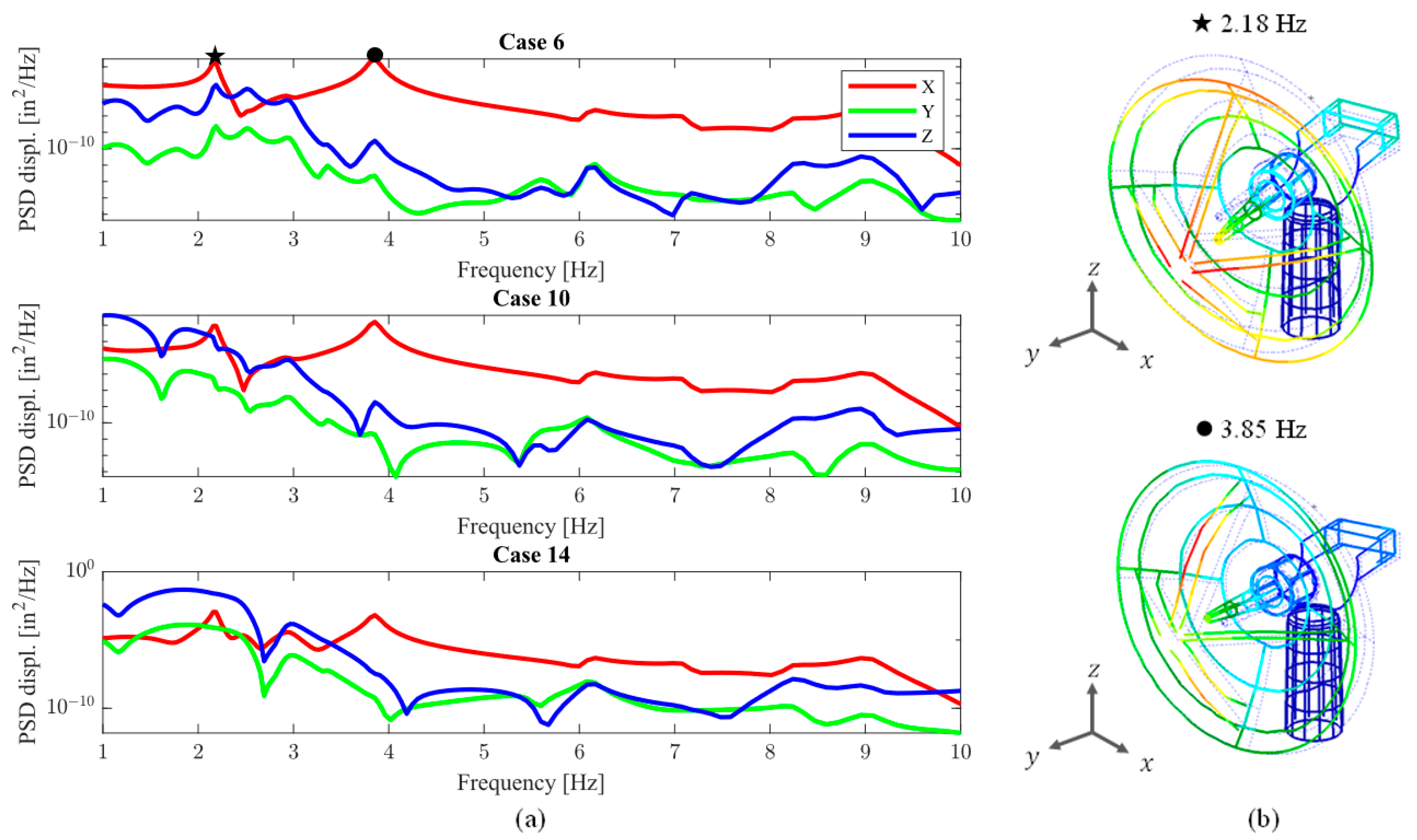
3.2.1. Dynamic Aeroelastic Response Analysis
3.2.2. Aeroelastic Response Fitness Function Definition
4. Results and Discussion
- Crossover fraction: 80%;
- Elitism probability: 2%;
- Migration factor: 20%;
- Migration interval: 20;
- Pareto fraction: 35%.
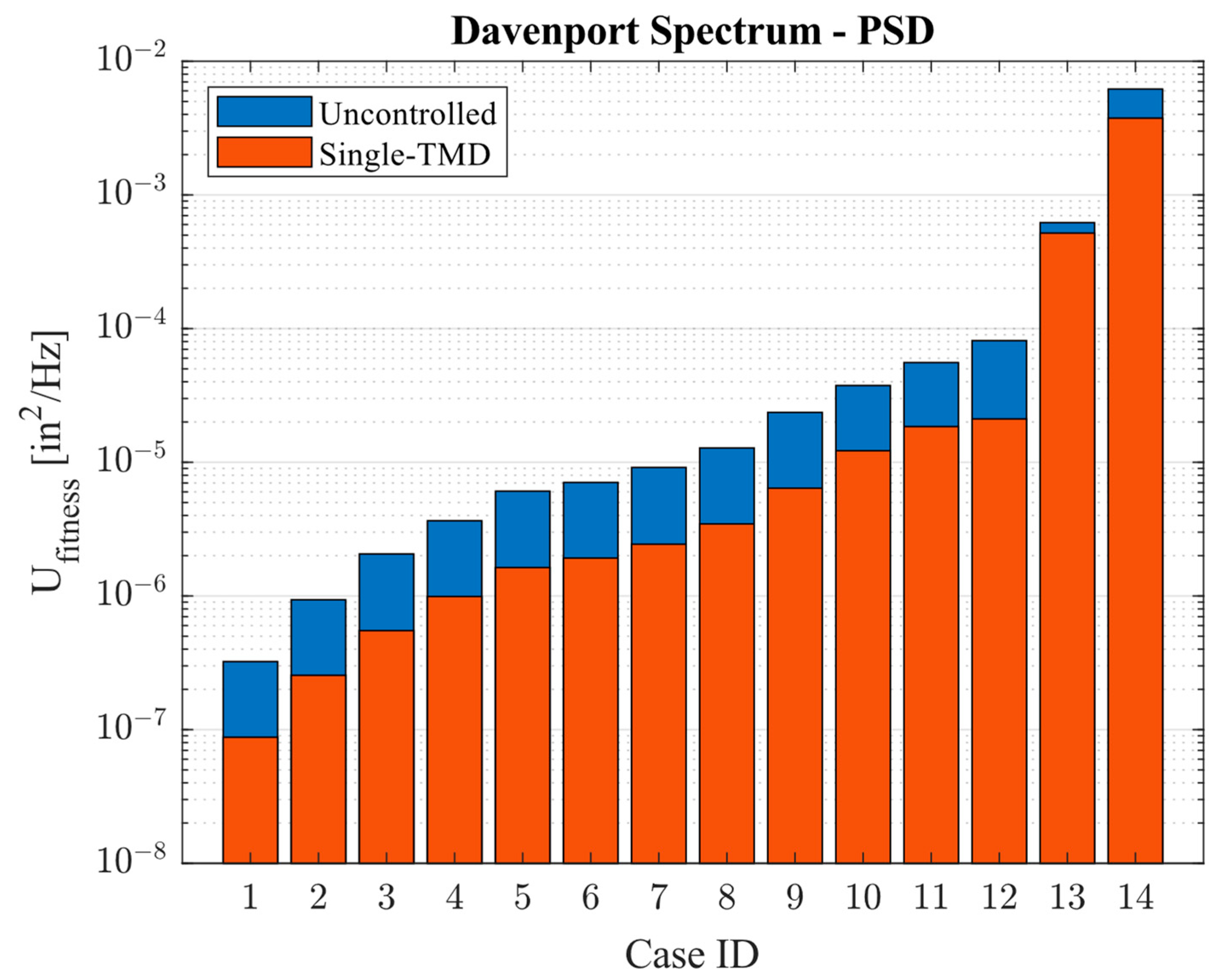
4.1. Frequency Domain Analysis with the PSD Modeled with the Davenport Spectrum
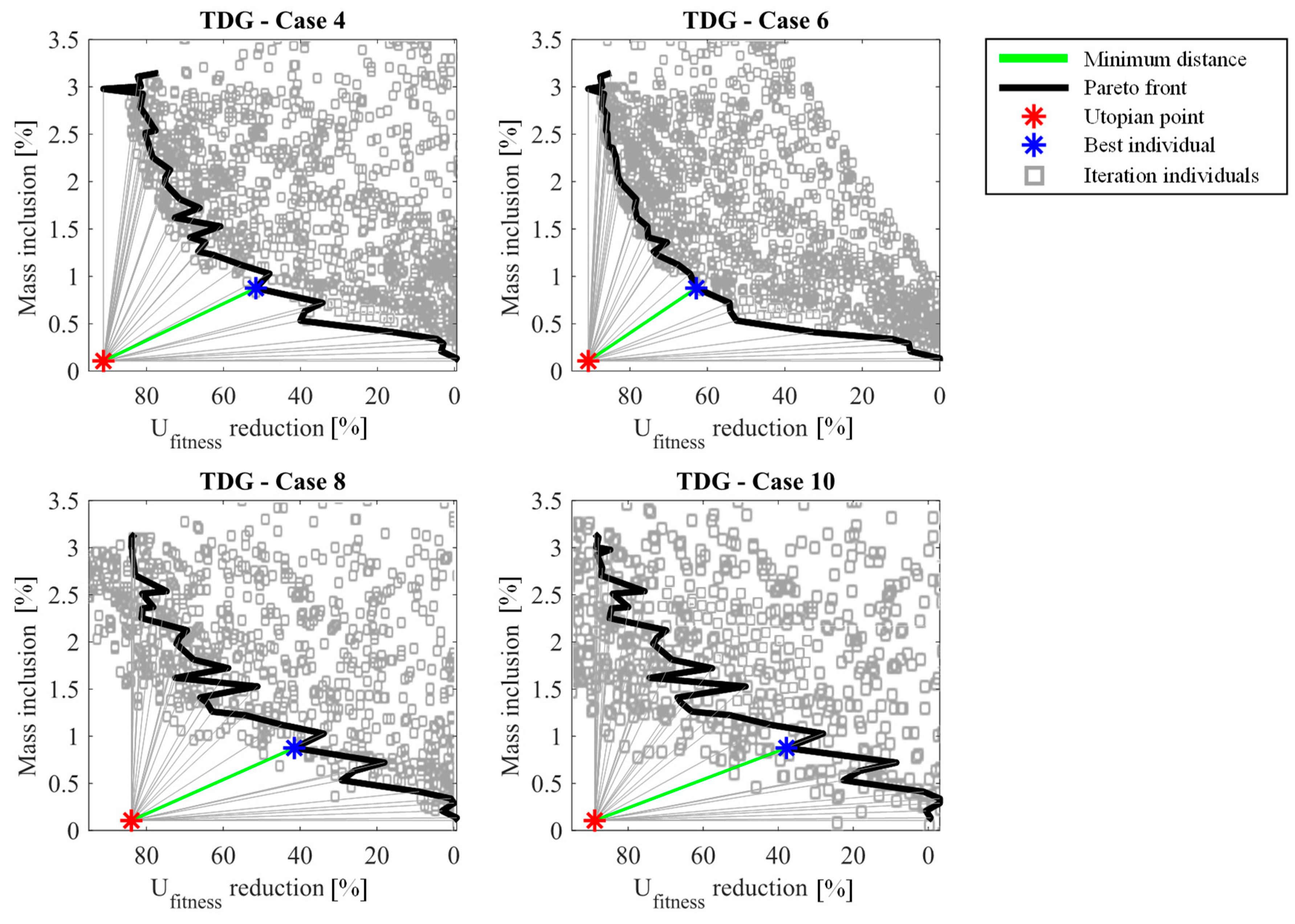
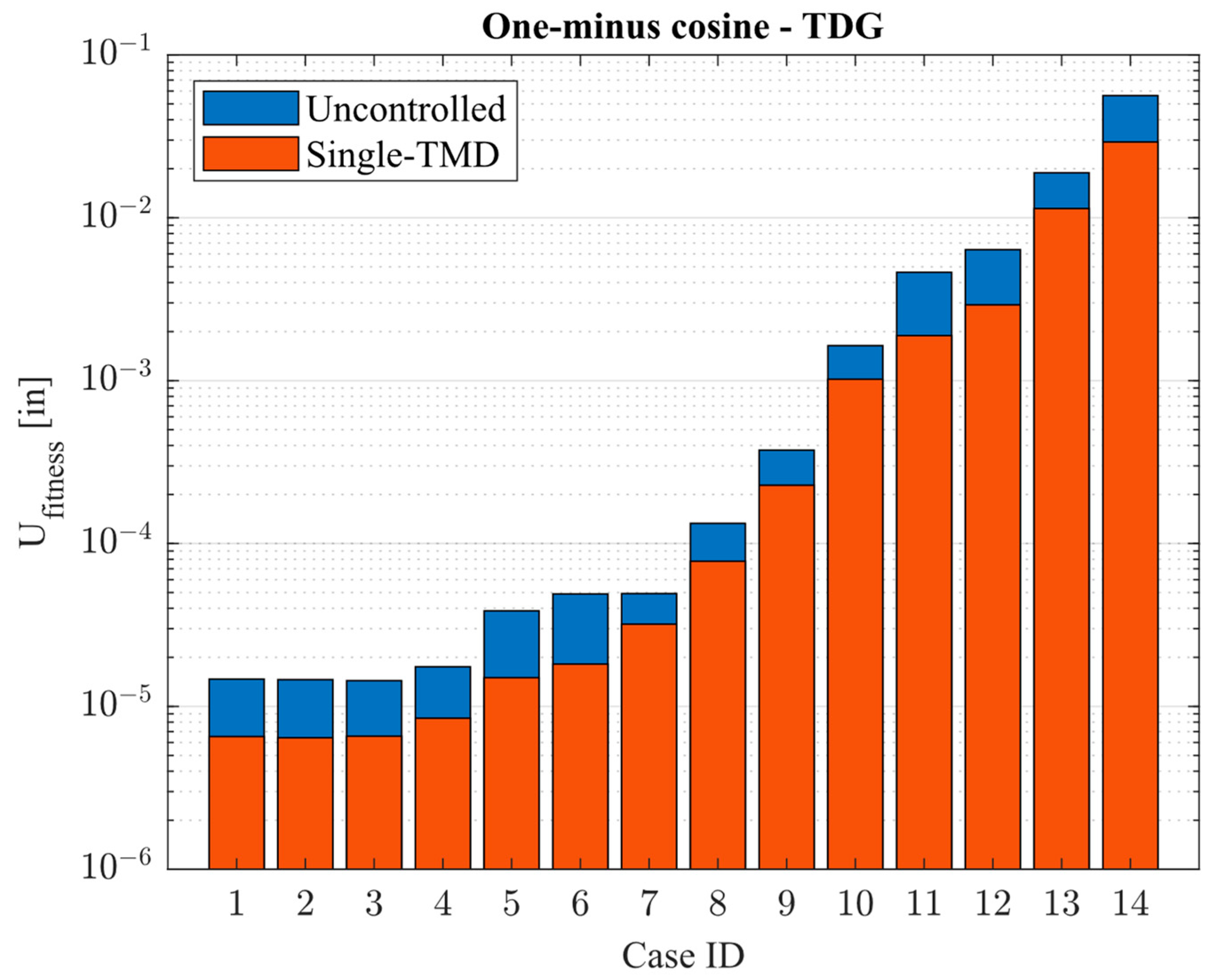
4.2. Time Domain Analysis with the One-Minus Cosine Gust Model
4.3. Comparison of the Aeroelastic Response Fitness for the Gust Models
- The first is the most formal and accurate, which would be to concurrently run the optimization framework considering all 14 operational cases for both gust models. This way, it would be possible to determine a set of design variables that globally minimizes the aeroelastic response of the VLBI antenna. The drawback of this method lies on the computational capacity limitation to run the framework, which would exponentially increase the computation time to evaluate the fitness functions, making it nearly impossible to obtain results within a reasonable time.
- The second is an approximation approach, and it is related to testing the permutation of best individuals previously found using the optimization framework independently for each gust excitation profile. An example would be taking the optimal set of design variables from the frequency-domain analysis and testing it within the time-domain analysis, quantifying the performance, and vice versa. This way, the best individual can be selected based on this proposed criterion. The drawback of this approach lies in the fact that not necessarily the optimal solution for one model will attenuate the other one, thus existing the possibility of the response being magnified by the inclusion of the STMD.
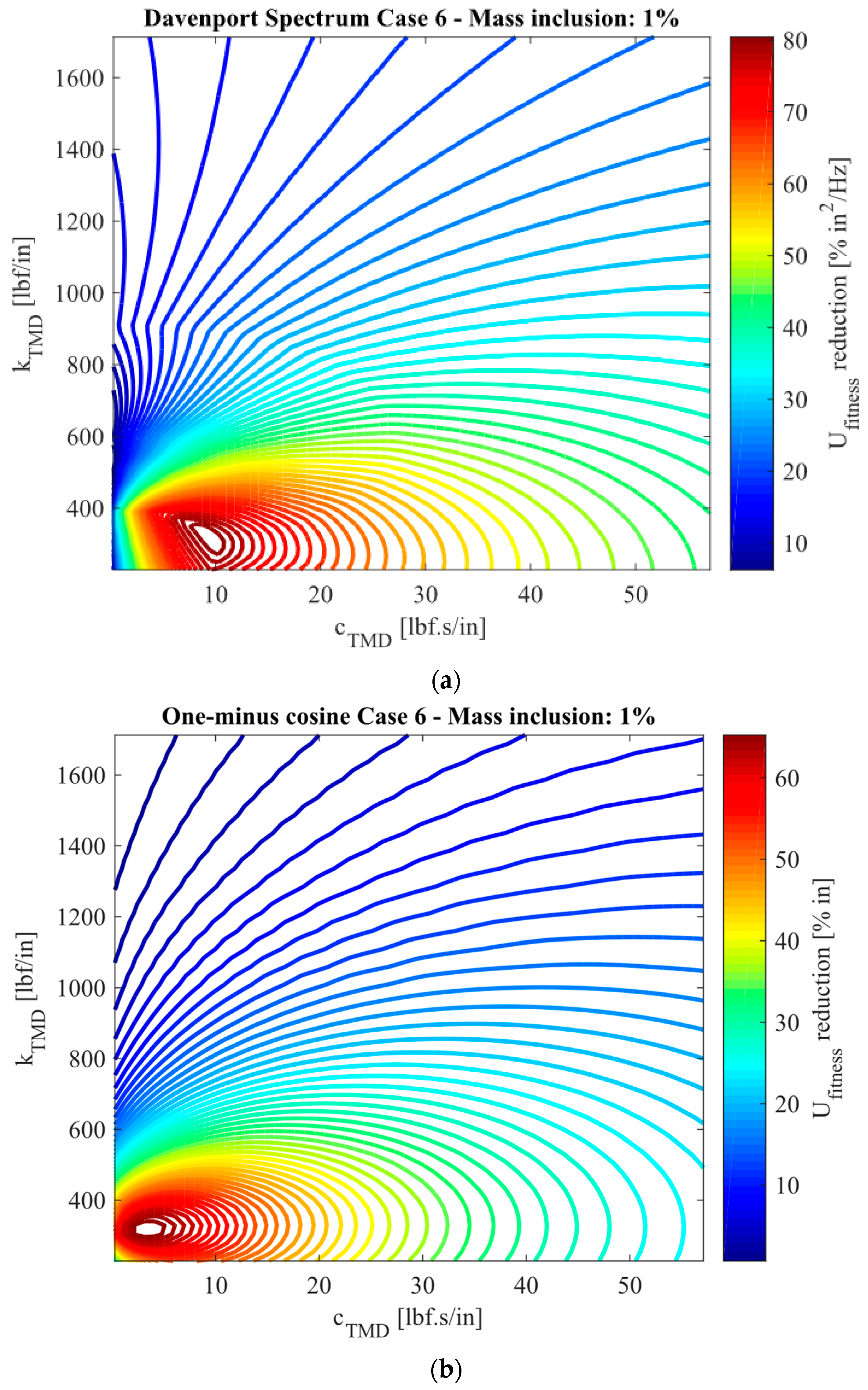
4.4. Factorial Analysis of TMD Design Variables with a Defined Mass Inclusion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Acronyms
| Acronym | Definition |
| CBRM | Craig-Bampton Reduction Method |
| DFEM | Detailed Finite Element Model |
| DLM | Doublet-Lattice Method |
| DS | Davenport Spectrum |
| FE | Finite Element |
| MAC | Modal Assurance Criteria |
| MTMD | Multiple Tuned Mass Dampers |
| NASA | National Aeronautics and Space Administration |
| PSD | Power Spectral Density |
| RIDTMD | Rotational Inertia Double Tuned Mass Dampers |
| ROM | Reduced Order Model |
| RSSQ | Root Sum of Squares |
| STMD | Single Tuned Mass Damper |
| TDG | Tuned Discrete Gust |
| TMD | Tuned Mass Dampers |
| VLBI | Very-Long-Baseline Interferometry |
References
- Inoue, M.; Algaba-Marcos, J.C.; Asada, K.; Blundell, R.; Brisken, W.; Burgos, R.; Chang, C.; Chen, M.; Doeleman, S.S.; Fish, V.; et al. Greenland telescope project: Direct confirmation of black hole with sub-millimeter VLBI. Radio Sci. 2014, 49, 564–571. [Google Scholar] [CrossRef]
- Hobiger, T.; Kondo, T.; Schuh, H. Very long baseline interferometry as a tool to probe the ionosphere. Radio Sci. 2006, 41, 1–10. [Google Scholar] [CrossRef]
- Brunthaler, A.; Reid, M.; Menten, K.; Zheng, X.-W.; Bartkiewicz, A.; Choi, Y.; Dame, T.; Hachisuka, K.; Immer, K.; Moellenbrock, G.; et al. The Bar and Spiral Structure Legacy (BeSSeL) survey: Mapping the Milky Way with VLBI astrometry. Astron. Nachrichten 2011, 332, 461–466. [Google Scholar] [CrossRef]
- Cotton, W.D. Very Long Baseline Interferometry. In High Angular Resolution in Astrophysics; Springer: Dordrecht, The Netherlands, 1997; pp. 165–191. [Google Scholar]
- Meeks, M.; Ball, J.; Hull, A. The pointing calibration of the Haystack antenna. IEEE Trans. Antennas Propag. 1968, 16, 746–751. [Google Scholar] [CrossRef]
- Guiar, C.; Lansing, F.; Riggs, R. Antenna Pointing Systematic Error Model Derivations; NASA Technical Reports Server: Washington, DC, USA, 1987. [Google Scholar]
- Kong, D.-Q.; Wang, S.-G.; Wang, J.-Q.; Wang, M.; Zhang, H.-B. A new calibration model for pointing a radio telescope that considers nonlinear errors in the azimuth axis. Res. Astron. Astrophys. 2014, 14, 733–740. [Google Scholar] [CrossRef]
- Gawronski, W.; Baher, F.; Gama, E. Track level compensation look-up table improves antenna pointing precision. Interplanet. Netw. Prog. Rep. 2006, 6273, 627316. [Google Scholar] [CrossRef]
- Titov, O.; Tregoning, P. Effect of post-seismic deformation on earth orientation parameter estimates from VLBI observations: A case study at Gilcreek, Alaska. J. Geodesy 2005, 79, 196–202. [Google Scholar] [CrossRef]
- Eisentraeger, P.; Suess, M. Verification of the active deformation compensation system of the LMT/GTM by end-to-end simulations. In Radio Telescopes; SPIE: Bellingham, DC, USA, 2000; Volume 4015, pp. 488–497. [Google Scholar] [CrossRef]
- Cullum, M.; Spyromilio, J. Thermal and wind control of the VLT. In Proceedings of the Telescope Structures, Enclosures, Controls, Assembly/Integration/Validation, and Commissioning, Munich, Germany, 27 March–1 April 2000; pp. 194–201. [Google Scholar] [CrossRef]
- Schüler, T.; Kronschnabl, G.; Plötz, C.; Neidhardt, A.; Bertarini, A.; Bernhart, S.; La Porta, L.; Halsig, S.; Nothnagel, A. Initial Results Obtained with the First TWIN VLBI Radio Telescope at the Geodetic Observatory Wettzell. Sensors 2015, 15, 18767–18800. [Google Scholar] [CrossRef]
- Gawronski, W.; Souccar, K. Control systems of the Large Millimeter Telescope. In Astronomical Structures and Mechanisms Technology; IEEE: Piscataway, NJ, USA, 2004; Volume 5495, pp. 104–115. [Google Scholar] [CrossRef]
- Gawronski, W. Modeling wind-gust disturbances for the analysis of antenna pointing accuracy. IEEE Antennas Propag. Mag. 2004, 46, 50–58. [Google Scholar] [CrossRef]
- Gawronski, W.; Bienkiewicz, B.; Hill, R.E. Pointing-error simulations of the DSS-13 antenna due to wind disturbances. TDA Prog. Rep. 1992, 7477, 109–134. [Google Scholar]
- Gawronski, W. Control and pointing challenges of antennas and telescopes. In Proceedings of the 2005, American Control Conference 2005, Portland, OR, USA, 8–10 June 2005; Volume 6, pp. 3758–3769. [Google Scholar] [CrossRef]
- Kareem, A.; Kijewski, T.; Tamura, Y. Mitigation of motions of tall buildings with specific examples of recent applications. Wind. Struct. An Int. J. 1999, 2, 201–251. [Google Scholar] [CrossRef]
- Tsioustas, C.; Loupis, M. Design, simulation and experimental verification of an active inductance for a low frequency tuned mass damper in an aircraft fuselage active vibration cancellation system. In Proceedings of the 2018 International Symposium on Industrial Electronics INDEL 2018, Banja Luka, Bosnia and Herzegovina, 1–3 November 2018; pp. 4–8. [Google Scholar] [CrossRef]
- Connor, J.J. Introduction to Structural Motion Control; Prentice Hall Pearson Education, Incorporated: Upper Saddle River, NJ, USA, 2003. [Google Scholar]
- Zhang, Z.; Høeg, C. Inerter-enhanced tuned mass damper for vibration damping of floating offshore wind turbines. Ocean Eng. 2021, 223, 108663. [Google Scholar] [CrossRef]
- Chen, D.; Huang, S.; Huang, C.; Liu, R.; Ouyang, F. Passive control of jacket–type offshore wind turbine vibrations by single and multiple tuned mass dampers. Mar. Struct. 2020, 77, 102938. [Google Scholar] [CrossRef]
- del Campo, J.O.M.; Pozos-Estrada, A.; Pozos-Estrada, O. Development of fragility curves of land-based wind turbines with tuned mass dampers under cyclone and seismic loading. Wind. Energy 2021, 24, 737–753. [Google Scholar] [CrossRef]
- Boonyapinyo, V.; Aksorn, A.; Lukkunaprasit, P. Suppression of aerodynamic response of suspension bridges during erection and after completion by using tuned mass dampers. Wind. Struct. 2007, 10, 1–22. [Google Scholar] [CrossRef]
- Lin, Y.-Y.; Cheng, C.-M.; Lee, C.-H. A tuned mass damper for suppressing the coupled flexural and torsional buffeting response of long-span bridges. Eng. Struct. 2000, 22, 1195–1204. [Google Scholar] [CrossRef]
- Xu, Y.; Kwok, K.; Samali, B. Control of wind-induced tall building vibration by tuned mass dampers. J. Wind. Eng. Ind. Aerodyn. 1992, 40, 1–32. [Google Scholar] [CrossRef]
- Xu, K.; Bi, K.; Han, Q.; Li, X.; Du, X. Using tuned mass damper inerter to mitigate vortex-induced vibration of long-span bridges: Analytical study. Eng. Struct. 2019, 182, 101–111. [Google Scholar] [CrossRef]
- Jiang, Z. The impact of a passive tuned mass damper on offshore single-blade installation. J. Wind. Eng. Ind. Aerodyn. 2018, 176, 65–77. [Google Scholar] [CrossRef]
- Ubertini, F.; Comanducci, G.; Laflamme, S. A parametric study on reliability-based tuned-mass damper design against bridge flutter. J. Vib. Control. 2017, 23, 1518–1534. [Google Scholar] [CrossRef]
- Kwok, K.; Samali, B. Performance of tuned mass dampers under wind loads. Eng. Struct. 1995, 17, 655–667. [Google Scholar] [CrossRef]
- MathWorks. MATLAB Software. Available online: https://www.mathworks.com/products/matlab.html (accessed on 20 July 2022).
- MacNeal, R.H. The NASTRAN Theoretical Manual; Scientific and Technical Information Office, National Aeronautics and Space Administration: Washington, DC, USA, 1970.
- Albano, E.; Rodden, W.P. A doublet-lattice method for calculating lift distributions on oscillating surfaces in subsonic flows. AIAA J. 1969, 7, 279–285. [Google Scholar] [CrossRef]
- Craig, R.R.; Bampton, M.C.C. Coupling of substructures for dynamic analyses. AIAA J. 1968, 6, 1313–1319. [Google Scholar] [CrossRef]
- Libelli, S.M.; Alba, P. Adaptive Mutation in Genetic Algorithms. Soft Comput. 2000, 4, 76–80. [Google Scholar] [CrossRef]
- Lu, L.; Anderson-Cook, C.M.; Robinson, T.J. Optimization of Designed Experiments Based on Multiple Criteria Utilizing a Pareto Frontier. Technometrics 2011, 53, 353–365. [Google Scholar] [CrossRef]
- Kasprzak, E.; Lewis, K. Pareto analysis in multiobjective optimization using the collinearity theorem and scaling method. Struct. Multidiscip. Optim. 2001, 22, 208–218. [Google Scholar] [CrossRef]
- Szparaga, A.; Stachnik, M.; Czerwińska, E.; Kocira, S.; Dymkowska-Malesa, M.; Jakubowski, M. Multi-objective optimization based on the utopian point method applied to a case study of osmotic dehydration of plums and its storage. J. Food Eng. 2018, 245, 104–111. [Google Scholar] [CrossRef]
- Gasparetto, V.E.L.; Reid, J.; Parsons, W.P.; ElSayed, M.S.A.; Saad, M.; Shieldand, S.; Brown, G.L.; Hilliard, L.M. Multi-Objective Design Optimization of Multiple Tuned Mass Dampers for Attenuation of Dynamic Aeroelastic Response of Aerospace Structures. Aerospace 2023, 10, 235. [Google Scholar] [CrossRef]
- Thomas, P.V.; ElSayed, M.S.A.; Walch, D. Review of Model Order Reduction Methods and Their Applications in Aeroelasticity Loads Analysis for Design Optimization of Complex Airframes. J. Aerosp. Eng. 2019, 32, 04018156. [Google Scholar] [CrossRef]
- Allemang, R.J. The modal assurance criterion–Twenty years of use and abuse. Sound Vib. 2003, 37, 14–21. [Google Scholar]
- Boivin, A.; Boilard, F.; Messier, P.L.; Tremblay, P. MAYA HTT. In 12 m VLBI Antenna Structural Simulation; Internal Report; Intertronics Solutions Inc.: Quebec, QC, Canada, 2014. [Google Scholar]
- Nieto, M.G.; Thomas, P.V.; ElSayed, M.S.A.; Saad, M.; Brown, G.L.; Hilliard, L.M. Development of Efficient Dynamic Aeroelasticity Model for High Fidelity Pointing Accuracy Assessment of VLBI Earth-Based Radio Antennas. Int. J. Aeronaut. Space Sci. 2020, 21, 693–706. [Google Scholar] [CrossRef]
- Davenport, A.G. The spectrum of horizontal gustiness near the ground in high winds. Q. J. R. Meteorol. Soc. 1962, 88, 197–198. [Google Scholar] [CrossRef]
- Orban, F. Damping of materials and members in structures. J. Physics Conf. Ser. 2011, 268, 012022. [Google Scholar] [CrossRef]
- Roughen, K.M.; Baker, M.L.; Fogarty, T. Computational Fluid Dynamics and Doublet-Lattice Calculation of Unsteady Control Surface Aerodynamics. J. Guid. Control. Dyn. 2001, 24, 160–166. [Google Scholar] [CrossRef]
- Warburton, G.B. Optimum absorber parameters for various combinations of response and excitation parameters. Earthq. Eng. Struct. Dyn. 1982, 10, 381–401. [Google Scholar] [CrossRef]
- Thomas, P.V.; ElSayed, M.S.; Walch, D. Development of high fidelity reduced order hybrid stick model for aircraft dynamic aeroelasticity analysis. Aerosp. Sci. Technol. 2019, 87, 404–416. [Google Scholar] [CrossRef]
- Abd-Elwahab, M.; Sherif, H.A. Pre-tensioned Layer Damping as a New Approach for Vibration Control of Elastic Beams. J. Vib. Acoust. 2006, 128, 338–346. [Google Scholar] [CrossRef]




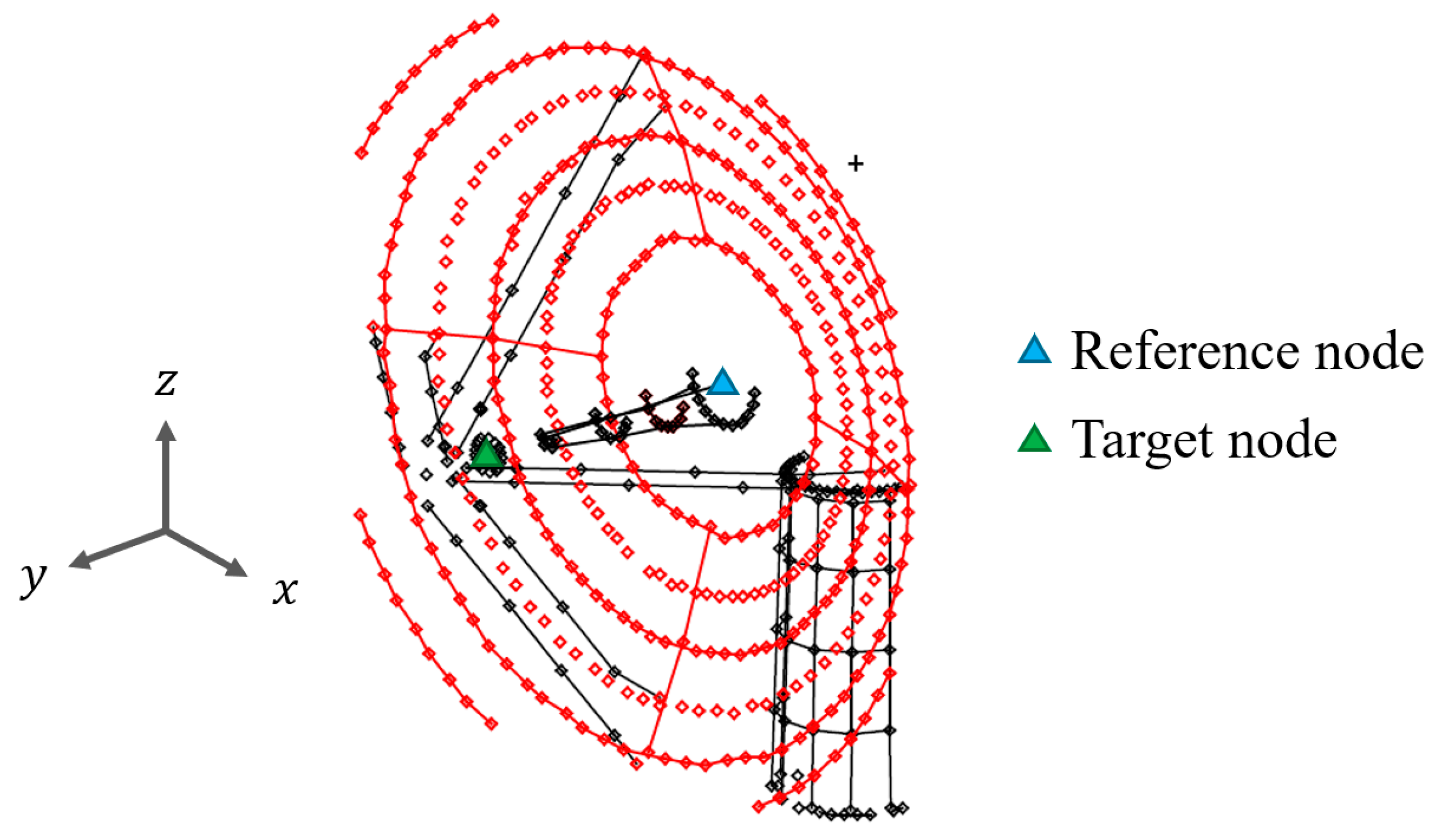






| Model | Computational Time Analysis—First 30 Flexible Modes (s) | |
|---|---|---|
| Normal Modes (SOL103) | Dynamic Aeroelasticity (SOL146) | |
| DFEM [41] | 1638.0 | 2352.6 |
| ROM—Nieto et al. [42] | 65.4 | 186.4 |
| ROM—Gasparetto et al. [38] | 17.7 | 61.0 |
| Case ID | Disturbance Speed Δv [in/s] | Mean Wind Speed vm [in/s] | Mach Number | Dynamic Pressure [lb/in3] |
|---|---|---|---|---|
| 1 | 34.15 | 109.36 | 0.008173 | 0.000555 |
| 2 | 51.20 | 164.04 | 0.012259 | 0.001249 |
| 3 | 68.20 | 218.72 | 0.016346 | 0.002221 |
| 4 | 85.26 | 273.40 | 0.020432 | 0.003470 |
| 5 | 102.30 | 328.08 | 0.024519 | 0.004997 |
| 6 * | 109.10 | 349.95 | 0.026153 | 0.005686 |
| 7 | 119.30 | 382.76 | 0.028605 | 0.006802 |
| 8 | 136.20 | 437.44 | 0.032692 | 0.008884 |
| 9 | 170.20 | 546.80 | 0.040865 | 0.013881 |
| 10 | 204.00 | 656.16 | 0.049038 | 0.019989 |
| 11 | 237.90 | 765.52 | 0.057211 | 0.027207 |
| 12 | 271.70 | 874.89 | 0.065384 | 0.035535 |
| 13 | 305.50 | 984.25 | 0.073557 | 0.044974 |
| 14 † | 339.20 | 1093.61 | 0.081730 | 0.055524 |
| Modal Frequency DFEM SOL103 [Hz] | Frequency ROM SOL146 [Hz] | Error [%] |
|---|---|---|
| 2.214 | 2.188 | 1.2 |
| 3.943 | 3.855 | 2.2 |
| 6.491 | 6.166 | 5.0 |
| 8.843 | 8.241 | 6.8 |
| 9.109 | 8.432 | 7.4 |
| Bounds | mTMD [lb] | cTMD [lbf.s/in] | kTMD [lbf/in] |
|---|---|---|---|
| Lower (min.) | 77.2 | 0.30 | 228.40 |
| Upper (max.) | 3861.0 | 571.0 | 17,130.0 |
| Configuration ID | Gust Model | m (lb) | c (lbf.s/in) | k (lbf/in) |
|---|---|---|---|---|
| A | PSD | 8.48 × 102 | 1.67 × 101 | 5.04 × 102 |
| B | TDG | 6.76 × 102 | 6.07 × 100 | 2.93 × 102 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gasparetto, V.E.L.; Reid, J.; ElSayed, M.S.A. Passive-Tuned Mass Dampers for the Pointing Accuracy Mitigation of VLBI Earth-Based Antennae Subject to Aerodynamic Gust. Appl. Mech. 2023, 4, 816-840. https://doi.org/10.3390/applmech4030042
Gasparetto VEL, Reid J, ElSayed MSA. Passive-Tuned Mass Dampers for the Pointing Accuracy Mitigation of VLBI Earth-Based Antennae Subject to Aerodynamic Gust. Applied Mechanics. 2023; 4(3):816-840. https://doi.org/10.3390/applmech4030042
Chicago/Turabian StyleGasparetto, Victor E. L., Jackson Reid, and Mostafa S. A. ElSayed. 2023. "Passive-Tuned Mass Dampers for the Pointing Accuracy Mitigation of VLBI Earth-Based Antennae Subject to Aerodynamic Gust" Applied Mechanics 4, no. 3: 816-840. https://doi.org/10.3390/applmech4030042
APA StyleGasparetto, V. E. L., Reid, J., & ElSayed, M. S. A. (2023). Passive-Tuned Mass Dampers for the Pointing Accuracy Mitigation of VLBI Earth-Based Antennae Subject to Aerodynamic Gust. Applied Mechanics, 4(3), 816-840. https://doi.org/10.3390/applmech4030042





