Al-RHEA Particulates MMCs by PM Route: Mechanical Properties and Sliding Wear Response
Abstract
:1. Introduction
2. Materials and Methods
2.1. Nanoindentation Testing
2.2. Wear Testing
3. Results and Discussion
3.1. Nanoindentation and Creep
Basic Mechanical Property Assessment
- Nanoindentation hardness, HV: The hardness values seem to follow an ordinary trend. More specifically, the reinforcing phase shows the higher hardness values, significantly higher than pure Al and the composite’s matrix phase, which is expected since it is a hard refractory high entropy alloy. The composite’s matrix phase appears to be slightly higher than pure Al. This difference arises from the fact that in the case of the matrix phase, it was observed form the composite microstructure as it was seen in previous work [26] a significant amount of fine-sized reinforcing particles is dispersed within the matrix region. The presence of such fine particles inhibits the matrix plastic deformation by restricting the mobility of dislocations. This phenomenon is typical in the mechanical response of metal matrix composites [28]. Since the ease of plastic deformation is retarded, and by recalling the hardness it is practically the resistance to the later (plastic deformation), this increase in the matrix hardness compared with that of pure Al is easily explained. The different loading speeds do not seem to have any significant effect of the hardness of the different phases.
- Modulus of elasticity in nanoindentation, Eit: As in the case of hardness, it can be observed that the Eit values increase form the pure Al, to the composite matrix and the reinforcing phase. This trend is also expected. The reinforcing phase, firstly, is a BCC solid solution of refractory elements that establishes strong interatomic bonds and intense elastic stress fields within the lattice, due to the intensive lattice distortion effect caused by the presence of the multiple, different in size elements [11,29]. These factors explain the high modulus of elasticity values for the reinforcing phase. The Eit values of the composite’s matrix phase, on the other hand, seems to be significantly higher than that of pure Al. This fact is also associated with presence of fine reinforcing phase particles homogeneously distributed within the matrix. If we recall the microstructure of the composites [26], it can be seen that the very fine size of the reinforcing particles leads to a particle distribution where the inter-particle spacing is low. This practically means that during the indentation testing, the indenter is facing and measuring the combined effect of both Al and reinforcing particles. It measures, hence, a mixed Eit which resembles the modulus of elasticity measured in a particulate reinforced metal matrix composite, where the values obtained are a combination of both phases and usually estimated by a rule of mixture approach [30]. It is, therefore, expected that the contribution of the hard and of high Eit fine reinforcing particles increase the Eit values of the matrix phase. Another issue to be addressed is the effect of the different loading speed on the Eit values.
- Ratio of the energy absorbed in the elastic region over the total absorbed energy, nit: The nit ratio, usually follows the same trend with hardness. If we take into consideration that hardness is the resistance to plastic deformation, an increased hardness results in an increase in the energy absorbed in the elastic region, i.e., an increase in the nit ratio.
3.2. Creep Assessment
3.2.1. Calculations
3.2.2. Assessment
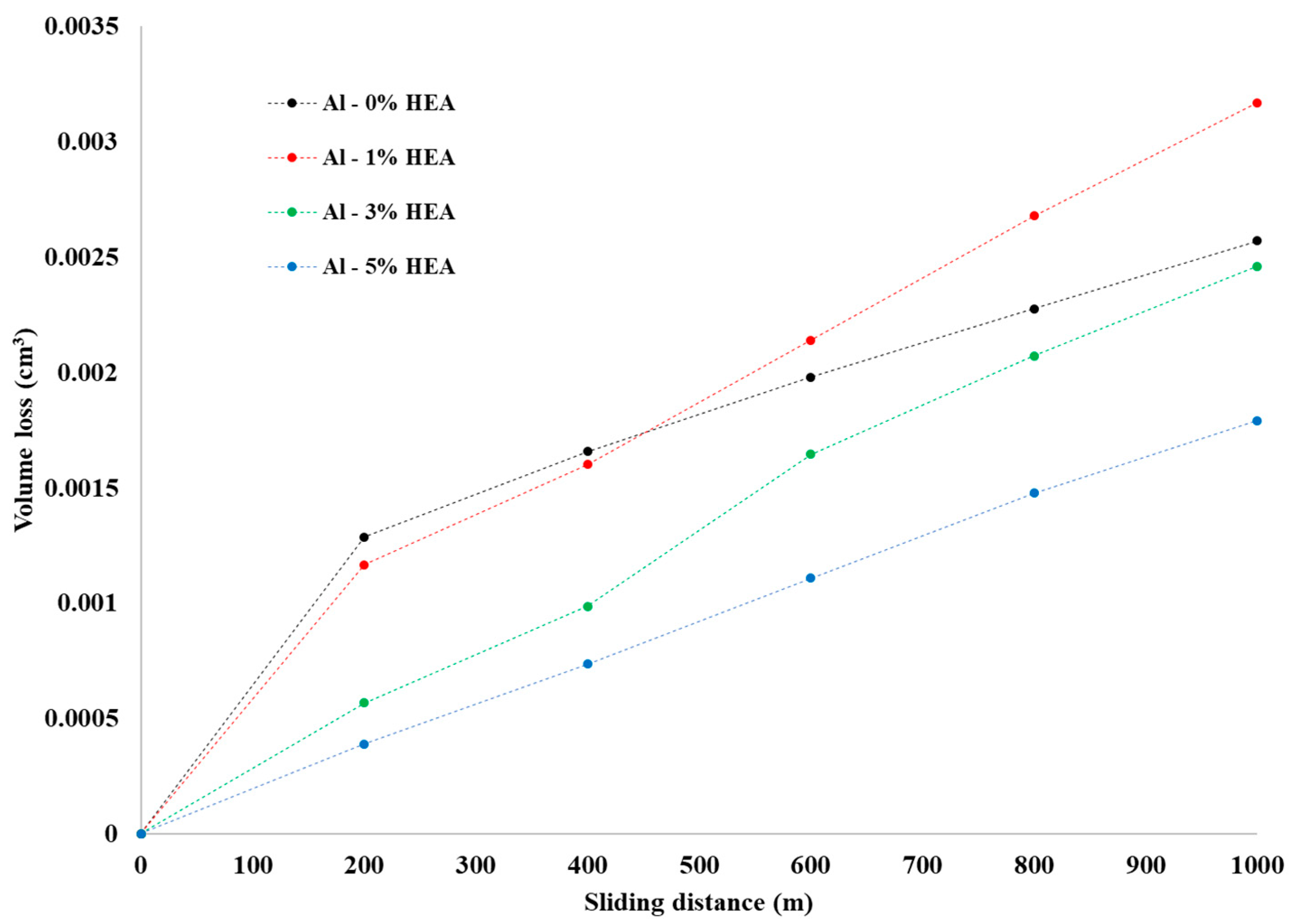
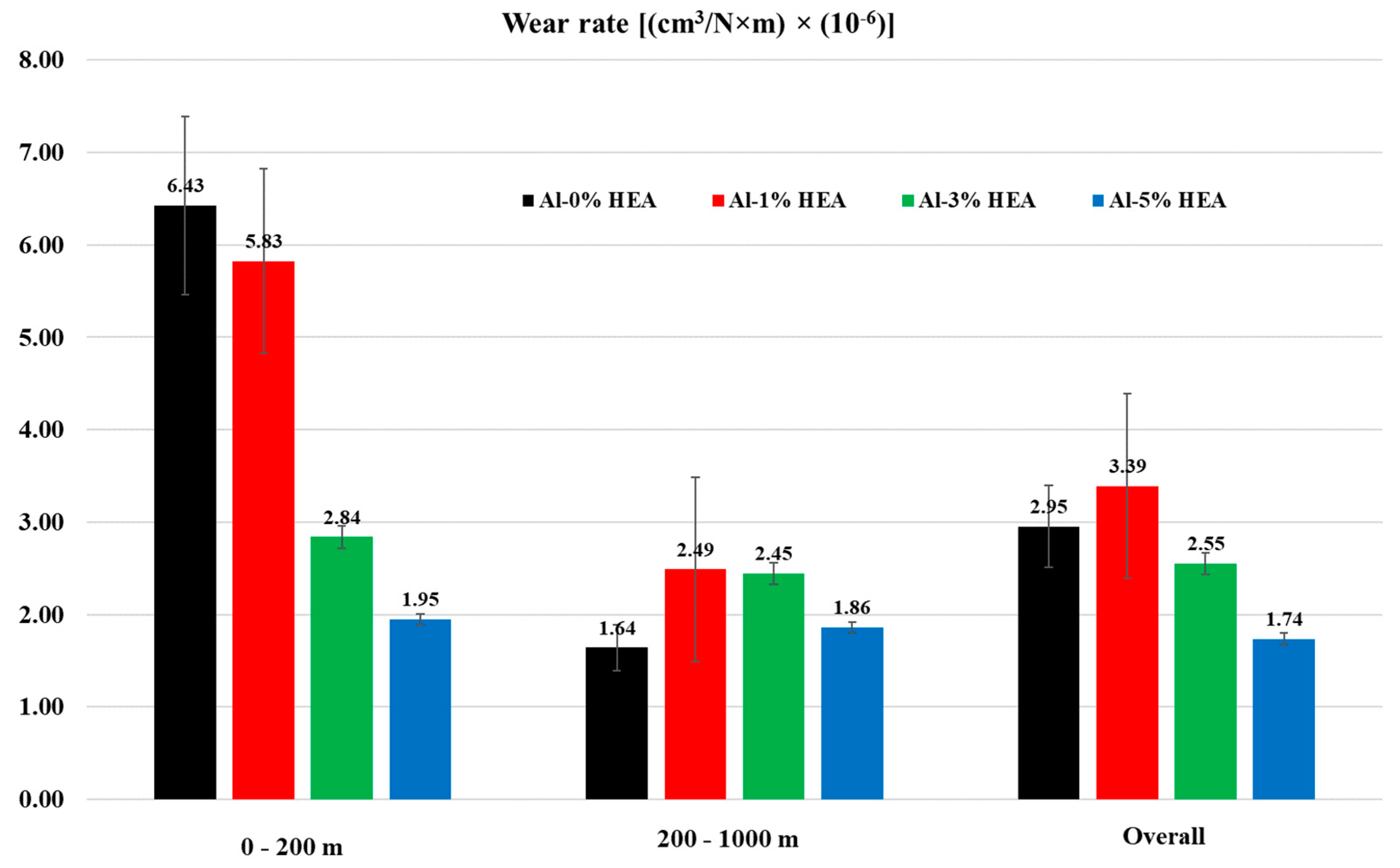
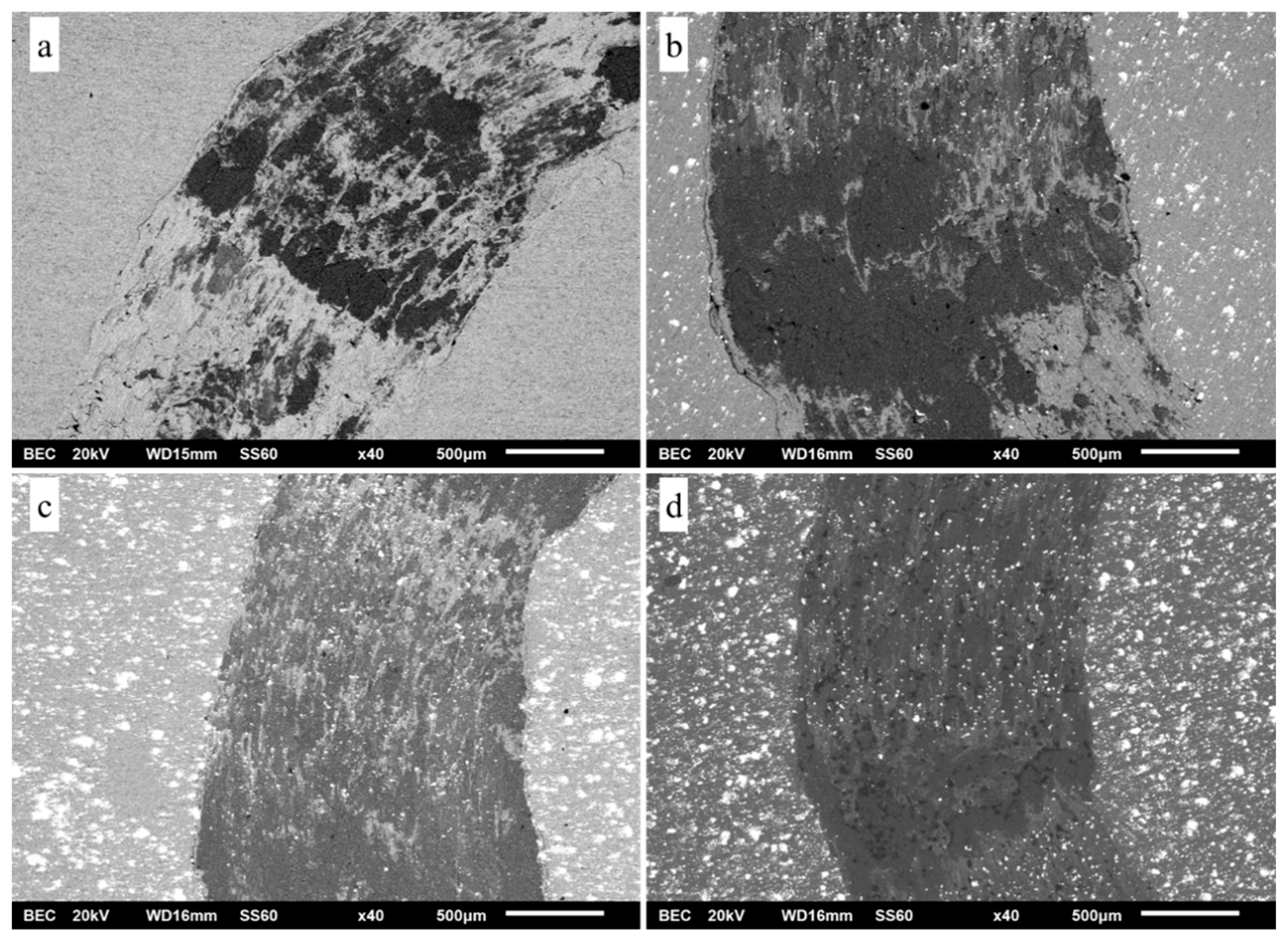
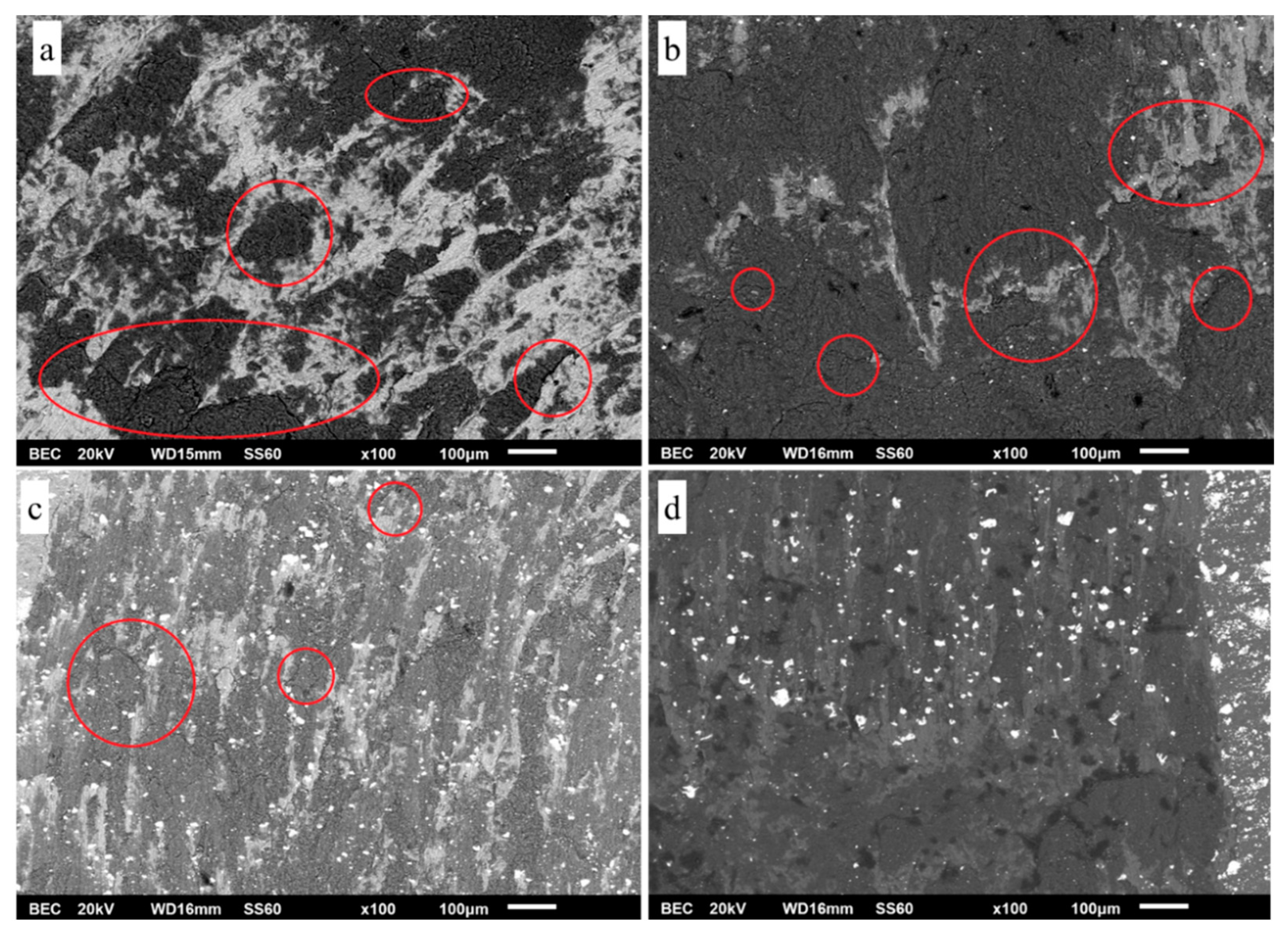
3.3. Sliding Wear Response
4. Conclusions
- Al matrix composites reinforced by RHEA particulates were assessed as far as their mechanical properties and creep response are concerned based on nanoindentation testing. Their sliding wear response was also evaluated;
- The addition of RHEA particulates enhanced hardness, elastic moduli, and ratio of energy absorbed in elastic region of the matrix phase in the 5 vol.% reinforced system compared with the pure aluminum specimen;
- The three areas of interest showed no serrations during the loading stage prior to creep for the two different loading speeds, i.e., no creep phenomena were expressed during the loading stage;
- The creep response of the three different constitutes (pure Al, matrix of the 5 vol.% system and coarse RHEA particulates of the 5 vol.% composite) seem to be highly affected by the maximum shear stress upon which creep is initiated (τmax) which is directly mirrored in the values of the critical for nucleation volume (Vcr) which, in turn, directly affects the necessary dislocation density and mobility for creep;
- As far as the wear response is concerned, the increase in the reinforcement percentage lowers the wear rates;
- Intense wear with hill—valley morphology was evident in all specimens;
- Oxidation—delamination was identified as the main wear mechanism accompanied by the contribution of an abrasive wear mode;
- Conclusively, it is evident that the presence of the fine size particulates enhanced the creep response of the produced composites, where the coarse particles were mainly contributed to the enhancement of their sliding wear resistance.
Author Contributions
Funding
Conflicts of Interest
References
- Verma, V.; Khvan, A. A short review on Al MMC with reinforcement addition effect on their mechanical and wear behaviour. In Advances in Composite Materials Development; IntechOpen: London, UK, 2019. [Google Scholar]
- Hu, Q.; Zhao, H.; Li, F. Microstructures and properties of SiC particles reinforced aluminum-matrix composites fabricated by vacuum-assisted high pressure die casting. Mater. Sci. Eng. A 2017, 680, 270–277. [Google Scholar] [CrossRef]
- Yuan, Z.; Tian, W.; Li, F.; Fu, Q.; Hu, Y.; Wang, X. Microstructure and properties of high-entropy alloy reinforced aluminum matrix composites by spark plasma sintering. J. Alloys Compd. 2019, 806, 901–908. [Google Scholar] [CrossRef]
- Chen, J.; Niu, P.; Wei, T.; Hao, L.; Liu, Y.; Wang, X.; Peng, Y. Fabrication and mechanical properties of AlCoNiCrFe high-entropy alloy particle reinforced Cu matrix composites. J. Alloys Compd. 2015, 649, 630–634. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, J.; Li, Z.; Wang, X.; Fan, X.; Liu, J. Formation of transition layer and its effect on mechanical properties of AlCoCrFeNi high-entropy alloy/Al composites. J. Alloys Compd. 2019, 780, 558–564. [Google Scholar] [CrossRef]
- Soorya Prakash, K.; Gopal, P.M.; Purusothaman, M.; Sasikumar, M. Fabrication and characterization of metal-high entropy alloy composites. Int. J. Met. 2020, 14, 547–555. [Google Scholar] [CrossRef]
- Vijayakumar, S.; Karunamoorthy, L. Wear characterization of aluminium metal matrix composites. Adv. Compos. Lett. 2013, 22, 096369351302200401. [Google Scholar] [CrossRef]
- Gousia, V.; Tsioukis, A.; Lekatou, A.; Karantzalis, A.E. Al-MoSi2 Composite Materials: Analysis of Microstructure, Sliding Wear, Solid Particle Erosion, and Aqueous Corrosion. J. Mater. Eng. Perform. 2016, 25, 3107–3120. [Google Scholar] [CrossRef]
- Clyne, T.; Withers, P. An Introduction to Metal Matrix Composites; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Brown, K.R.; Venie, M.S.; Woods, R.A. The Increasing Use of Aluminum in Automotive Applications; Springer: Berlin/Heidelberg, Germany, 1995. [Google Scholar]
- Yeh, J.W.; Chen, S.K.; Lin, S.J.; Gan, J.Y.; Chin, T.S.; Shun, T.T.; Tsau, C.H.; Chang, S.Y. Nanostructured high-entropy alloys with multiple principal elements: Novel alloy design concepts and outcomes. Adv. Eng. Mater. 2004, 6, 299–303. [Google Scholar] [CrossRef]
- Jien-Wei, Y. Recent progress in high entropy alloys. Ann. Chim. Sci. Mat. 2006, 31, 633–648. [Google Scholar]
- Prabakaran, R.; Sait, A.N.; Sentilkumar, V. Synthesis and characteristization of high entropy alloy (CrMnFeNiCu) reinforced AA6061 aluminium matrix composite. J. Mech. Mech. Eng. 2017, 21, 415–424. [Google Scholar]
- Chinababu, M.; Krishna, N.N.; Sivaprasad, K.; Prashanth, K.G.; Rao, E.B. Evolution of Microstructure and Mechanical Properties of LM25–HEA Composite Processed through Stir Casting with a Bottom Pouring System. Materials 2022, 15, 230. [Google Scholar] [CrossRef] [PubMed]
- Luo, K.; Xiong, H.; Zhang, Y.; Gu, H.; Li, Z.; Kong, C.; Yu, H. AA1050 metal matrix composites reinforced by high-entropy alloy particles via stir casting and subsequent rolling. J. Alloys Compd. 2022, 893, 162370. [Google Scholar] [CrossRef]
- Meng, G.; Yue, T.M.; Lin, X.; Yang, H.; Xie, H.; Ding, X. Laser surface forming of AlCoCrCuFeNi particle reinforced AZ91D matrix composites. Opt. Laser Technol. 2015, 70, 119–127. [Google Scholar] [CrossRef]
- Meng, G.; Lin, X.; Xie, H.; Wang, C.; Wang, S.; Ding, X. Reinforcement and substrate interaction in laser surface forming of AlCoCrCuFeNi particle reinforced AZ91D matrix composites. J. Alloys Compd. 2016, 672, 660–667. [Google Scholar] [CrossRef]
- Mussert, K.M.; Vellinga, W.P.; Bakker, A.; Van Der Zwaag, S. A nano-indentation study on the mechanical behaviour of the matrix material in an AA6061-Al2O3 MMC. J. Mater. Sci. 2002, 37, 789–794. [Google Scholar] [CrossRef]
- Li, W.B.; Henshall, J.L.; Hooper, R.M.; Easterling, K.E. The mechanisms of indentation creep. Acta Metall. Et Mater. 1991, 39, 3099–3110. [Google Scholar] [CrossRef]
- Sannino, A.; Rack, H. Dry sliding wear of discontinuously reinforced aluminum composites: Review and discussion. Wear 1995, 189, 1–19. [Google Scholar] [CrossRef]
- Deuis, R.; Subramanian, C.; Yellup, J. Dry sliding wear of aluminium composites—A review. Compos. Sci. Technol. 1997, 57, 415–435. [Google Scholar] [CrossRef]
- Kumar, G.V.; Rao, C.; Selvaraj, N. Mechanical and tribological behavior of particulate reinforced aluminum metal matrix composites–a review. J. Miner. Mater. Charact. Eng. 2011, 10, 59. [Google Scholar] [CrossRef]
- Suh, N.P. The delamination theory of wear. Wear 1973, 25, 111–124. [Google Scholar] [CrossRef]
- Jiang, J.; Stott, F.; Stack, M. A mathematical model for sliding wear of metals at elevated temperatures. Wear 1995, 181, 20–31. [Google Scholar] [CrossRef]
- Heilmann, P.; Don, J.; Sun, T.C.; Rigney, D.A.; Glaeser, W.A. Sliding wear and transfer. Wear 1983, 91, 171–190. [Google Scholar] [CrossRef]
- Ananiadis, E.; Argyris, K.T.; Matikas, T.E.; Sfikas, A.K.; Karantzalis, A.E. Microstructure and Corrosion Performance of Aluminium Matrix Composites Reinforced with Refractory High-Entropy Alloy Particulates. Appl. Sci. 2021, 11, 1300. [Google Scholar] [CrossRef]
- Oliver, W.C.; Pharr, G.M. Measurement of hardness and elastic modulus by instrumented indentation: Advances in understanding and refinements to methodology. J. Mater. Res. 2004, 19, 3–20. [Google Scholar] [CrossRef]
- Rohatgi, P.; Asthana, R.; Das, S. Solidification, structures, and properties of cast metal-ceramic particle composites. Int. Met. Rev. 1986, 31, 115–139. [Google Scholar] [CrossRef]
- Cantor, B.; Chang, I.T.; Knight, P.; Vincent, A.J. Microstructural development in equiatomic multicomponent alloys. Mater. Sci. Eng. A 2004, 375, 213–218. [Google Scholar] [CrossRef]
- Mortensen, A.; Jin, I. Solidification processing of metal matrix composites. Int. Mater. Rev. 1992, 37, 101–128. [Google Scholar] [CrossRef]
- Zhang, L.; Yu, P.; Cheng, H.; Zhang, H.; Diao, H.; Shi, Y.; Chen, B.; Chen, P.; Feng, R.; Bai, J.; et al. Nanoindentation creep behavior of an Al 0.3 CoCrFeNi high-entropy alloy. Metall. Mater. Trans. A 2016, 47, 5871–5875. [Google Scholar] [CrossRef]
- Karantzalis, A.E.; Sioulas, D.; Poulia, A.; Mathiou, C.; Georgatis, E. A first approach on the assessment of the creep behavior of MoTaNbVxTi high entropy alloys by indentation testing. SN Appl. Sci. 2020, 2, 950. [Google Scholar] [CrossRef]
- Wang, Z.; Guo, S.; Wang, Q.; Liu, Z.; Wang, J.; Yang, Y.; Liu, C.T. Nanoindentation characterized initial creep behavior of a high-entropy-based alloy CoFeNi. Intermetallics 2014, 53, 183–186. [Google Scholar] [CrossRef]
- Yu, P.F.; Feng, S.D.; Xu, G.S.; Guo, X.L.; Wang, Y.Y.; Zhao, W.; Qi, L.; Li, G.; Liaw, P.K.; Liu, R.P. Room-temperature creep resistance of Co-based metallic glasses. Scr. Mater. 2014, 90, 45–48. [Google Scholar] [CrossRef]
- Wang, D.; Tan, J.; Li, C.J.; Qin, X.M.; Guo, S.F. Enhanced creep resistance of Ti30Al25Zr25Nb20 high-entropy alloy at room temperature. J. Alloys Compd. 2021, 885, 161038. [Google Scholar] [CrossRef]
- Ma, Y.; Feng, Y.H.; Debela, T.T.; Peng, G.J.; Zhang, T.H. Nanoindentation study on the creep characteristics of high-entropy alloy films: Fcc versus bcc structures. Int. J. Refract. Met. Hard Mater. 2016, 54, 395–400. [Google Scholar] [CrossRef]
- Ma, Z.S.; Long, S.G.; Zhou, Y.C.; Pan, Y. Indentation scale dependence of tip-in creep behavior in Ni thin films. Scr. Mater. 2008, 59, 195–198. [Google Scholar] [CrossRef]
- Choi, I.C.; Yoo, B.G.; Kim, Y.J.; Jang, J.I. Indentation creep revisited. J. Mater. Res. 2012, 27, 3–11. [Google Scholar] [CrossRef]
- Karantzalis, A.E.; Poulia, A.; Kamnis, S.; Sfikas, A.; Fotsis, A.; Georgatis, E. Modification of Cantor High Entropy Alloy by the Addition of Mo and Nb: Microstructure Evaluation, Nanoindentation-Based Mechanical Properties, and Sliding Wear Response Assessment. Alloys 2022, 1, 70–92. [Google Scholar] [CrossRef]
- Mandal, A.; Murty, B.; Chakraborty, M. Sliding wear behaviour of T6 treated A356–TiB2 in-situ composites. Wear 2009, 266, 865–872. [Google Scholar] [CrossRef]
- Hamid, A.A.; Ghosh, P.K.; Jain, S.; Ray, S. The influence of porosity and particles content on dry sliding wear of cast in situ Al (Ti)–Al2O3 (TiO2) composite. Wear 2008, 265, 14–26. [Google Scholar] [CrossRef]
- Shipway, P.; Kennedy, A.; Wilkes, A. Sliding wear behaviour of aluminium-based metal matrix composites produced by a novel liquid route. Wear 1998, 216, 160–171. [Google Scholar] [CrossRef]
- Basavarajappa, S.; Chandramohan, G.; Mahadevan, A.; Thangavelu, M.; Subramanian, R.; Gopalakrishnan, P. Influence of sliding speed on the dry sliding wear behaviour and the subsurface deformation on hybrid metal matrix composite. Wear 2007, 262, 1007–1012. [Google Scholar] [CrossRef]
- Yang, L. The effect of nominal specimen contact area on the wear coefficient of A6061 aluminium matrix composite reinforced with alumina particles. Wear 2007, 263, 939–948. [Google Scholar] [CrossRef]
- Chung, S.; Hwang, B.H. A microstructural study of the wear behaviour of SiCp/Al composites. Tribol. Int. 1994, 27, 307–314. [Google Scholar] [CrossRef]
- Tesfay, A.W.; Nath, S.; Ray, S. Effect of transfer layer on dry sliding wear behaviour of cast Al-based composites synthesized by addition of TiO2 and MoO3. Wear 2009, 266, 1082–1090. [Google Scholar] [CrossRef]
- Hutchings, I.; Shipway, P. Tribology: Friction and Wear of Engineering Materials; Butterworth-Heinemann: Oxford, UK, 2017. [Google Scholar]
- Mandal, A.; Chakraborty, M.; Murty, B. Effect of TiB2 particles on sliding wear behaviour of Al–4Cu alloy. Wear 2007, 262, 160–166. [Google Scholar] [CrossRef]
- Gómez-del Río, T.; Rico, A.; Garrido, M.A.; Poza, P.; Rodríguez, J. Temperature and velocity transitions in dry sliding wear of Al–Li/SiC composites. Wear 2010, 268, 700–707. [Google Scholar]
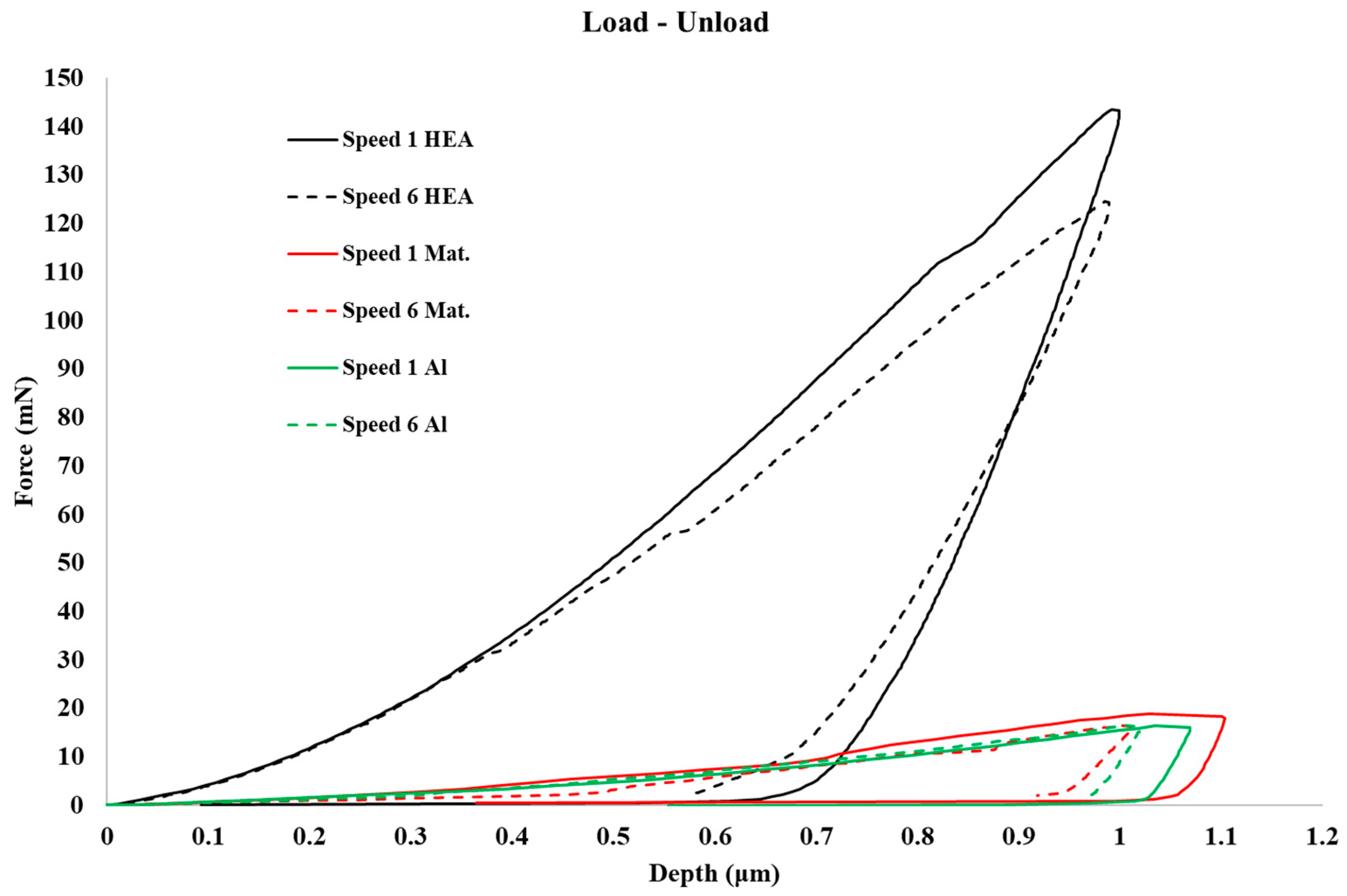
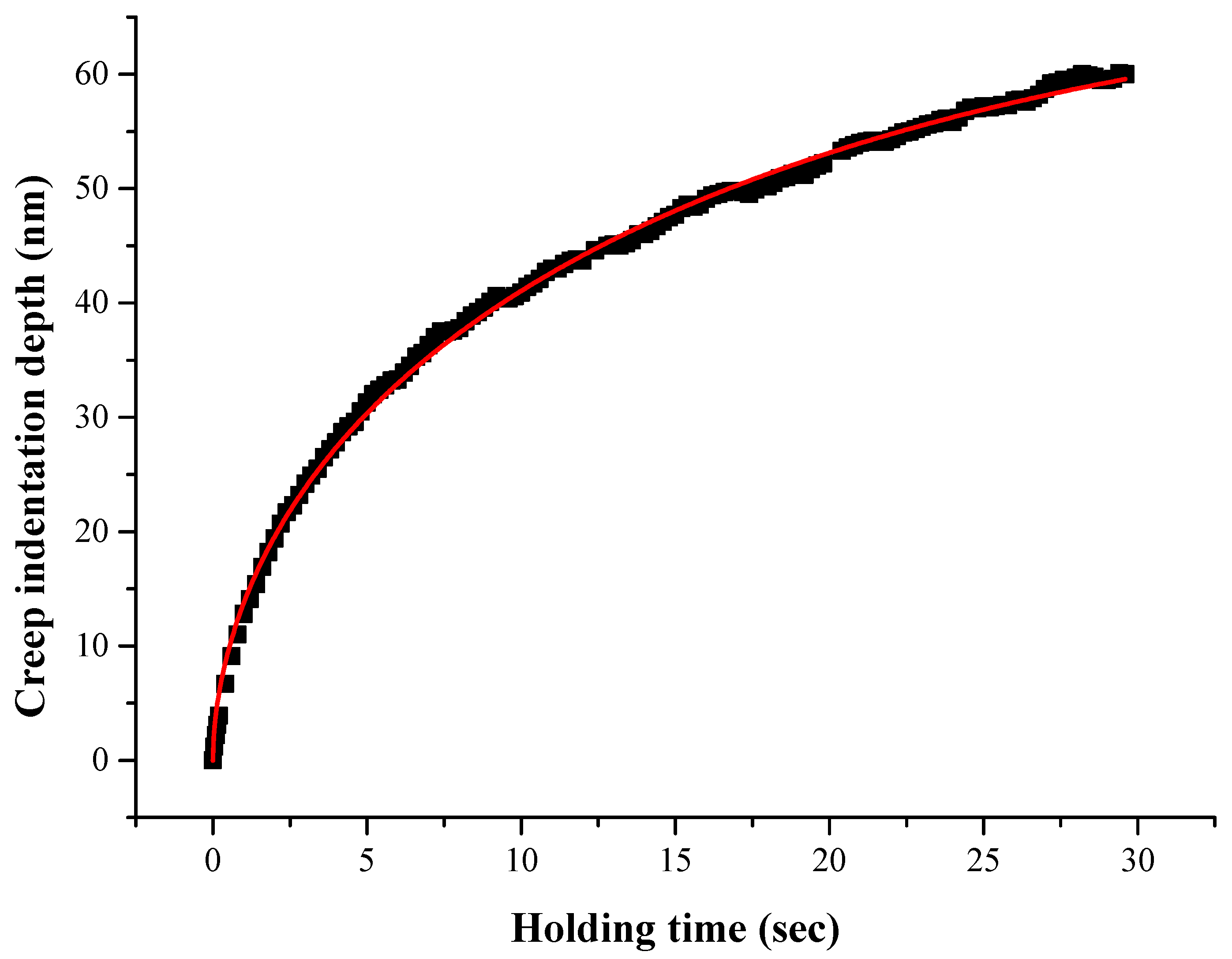
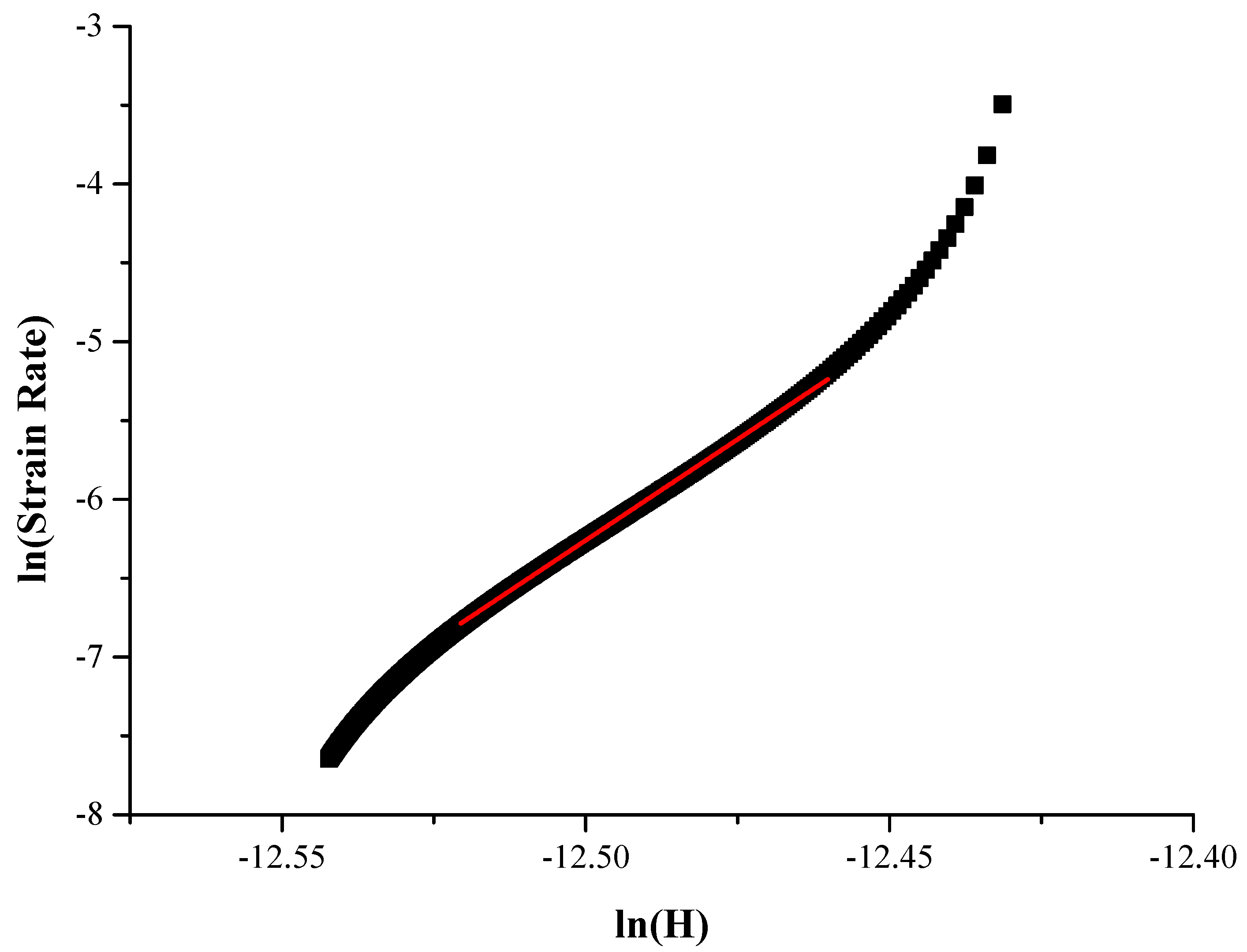

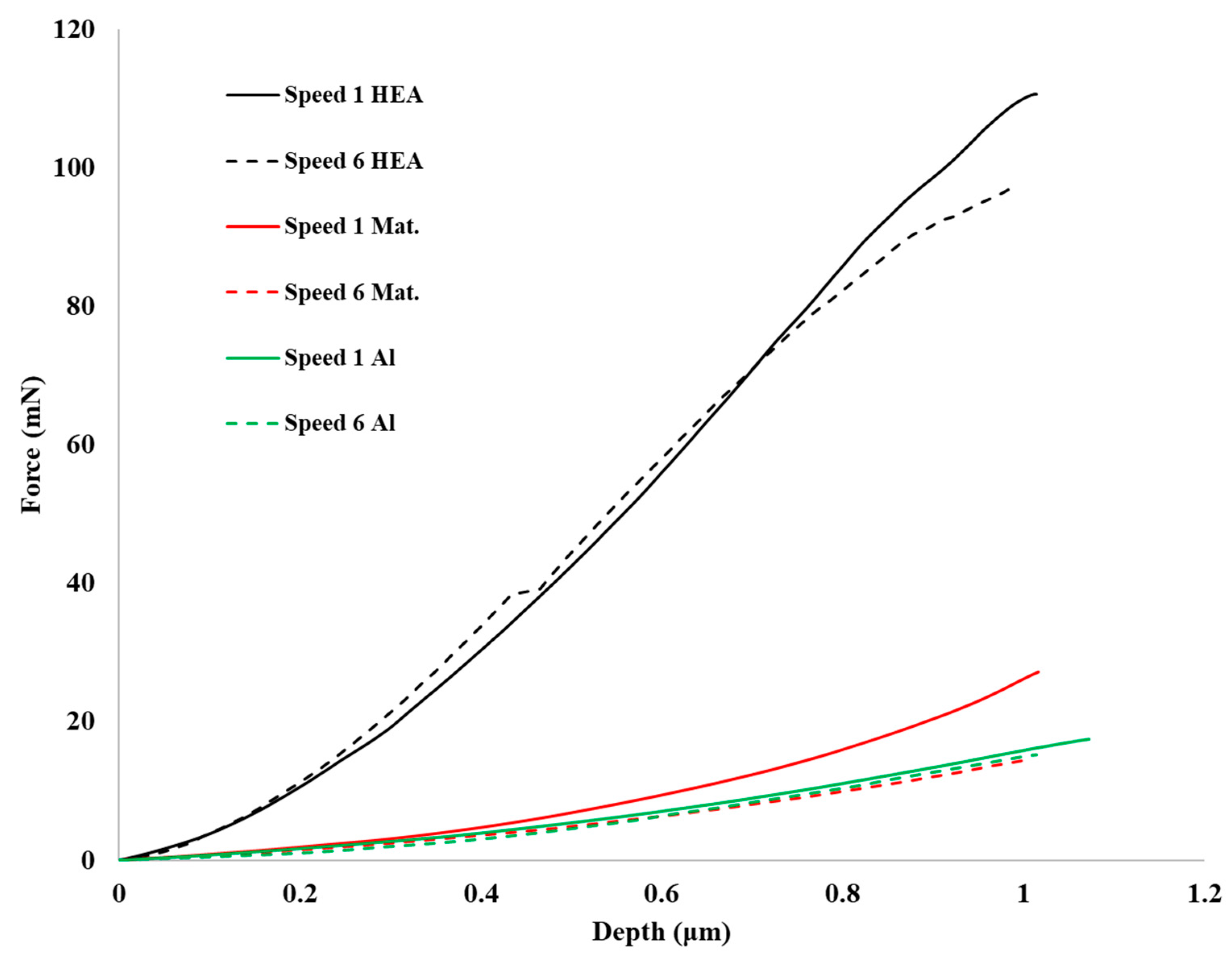



| Speed 1 (13.3240 mN/s) | |||
|---|---|---|---|
| Hardness (HV) | Eit (GPa) | nit (%) | |
| Pure Al | 50.88 ± 1.73 | 68.95 ± 1.17 | 6.58 ± 0.66 |
| Matrix | 59.14 ± 10.33 | 93.22 ± 5.4 | 13.51 ± 1.76 |
| HEA | 653.24 ± 113.63 | 129.04 ± 19.22 | 31.16 ± 3.6 |
| Speed 6 (2.2207 mN/s) | |||
|---|---|---|---|
| Hardness (HV) | Eit (GPa) | nit (%) | |
| Pure Al | 56.29 ± 2.11 | 62.69 ± 6 | 6.03 ± 0.59 |
| Matrix | 60.26 ± 5.87 | 80.83 ± 21 | 7.57 ± 3.96 |
| HEA | 685.95 ± 137.14 | 112.13 ± 23.48 | 33.12 ± 4.53 |
| Phase | Loading Speed (mN/s) | hcreep (nm) | n actual | n Extrapolated | m Actual | m Extrapolated | Hmax (GPa) | τmax (GPa) | Vcr Actual (nm3) | Vcr Extrapolated (nm3) |
|---|---|---|---|---|---|---|---|---|---|---|
| RHEA | 13.3240 | 50.4 | 44.7 | 44.9 | 0.026 | 0.027 | 5.18 | 1.00 | 0.181 | 0.175 |
| 2.2207 | 43.7 | 41.7 | 41.5 | 0.031 | 0.031 | 4.80 | 0.92 | 0.182 | 0.182 | |
| Matrix | 13.3240 | 43.8 | 51.6 | 49.7 | 0.028 | 0.027 | 0.70 | 0.133 | 1.821 | 1.744 |
| 2.2207 | 27.8 | 86.0 | 101.4 | 0.012 | 0.0098 | 0.57 | 0.109 | 3.247 | 3.778 | |
| Pure Al | 13.3240 | 49.4 | 38.1 | 35.3 | 0.033 | 0.041 | 0.62 | 0.119 | 1.311 | 1.218 |
| 2.2207 | 46.7 | 18.1 | 17.7 | 0.065 | 0.067 | 0.32 | 0.121 | 0.606 | 0.591 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ananiadis, E.A.; Karantzalis, A.E.; Exarchos, D.A.; Matikas, T.E. Al-RHEA Particulates MMCs by PM Route: Mechanical Properties and Sliding Wear Response. Appl. Mech. 2022, 3, 1145-1162. https://doi.org/10.3390/applmech3030065
Ananiadis EA, Karantzalis AE, Exarchos DA, Matikas TE. Al-RHEA Particulates MMCs by PM Route: Mechanical Properties and Sliding Wear Response. Applied Mechanics. 2022; 3(3):1145-1162. https://doi.org/10.3390/applmech3030065
Chicago/Turabian StyleAnaniadis, Elias Anastasios, Alexander Efstathios Karantzalis, Dimitrios A. Exarchos, and Theodore E. Matikas. 2022. "Al-RHEA Particulates MMCs by PM Route: Mechanical Properties and Sliding Wear Response" Applied Mechanics 3, no. 3: 1145-1162. https://doi.org/10.3390/applmech3030065
APA StyleAnaniadis, E. A., Karantzalis, A. E., Exarchos, D. A., & Matikas, T. E. (2022). Al-RHEA Particulates MMCs by PM Route: Mechanical Properties and Sliding Wear Response. Applied Mechanics, 3(3), 1145-1162. https://doi.org/10.3390/applmech3030065






