Is the H Atom Surrounded by A Cloud of Virtual Quanta Due to the Lamb Shift?

Abstract

The Lamb shift, one of the most fundamental interactions in atomic physics, arises from the interaction of H atoms with the electromagnetic fluctuations of the quantum vacuum. The energy shift has been computed in a variety of ways. The energy shift, as Feynman, Power, and Milonni demonstrated, equals the change in the vacuum energy in the volume containing the H atoms due to the change in the index of refraction arising from the presence of the H atoms. Using this result and a group theoretical calculation of the contribution to the Lamb shift from each frequency of the vacuum fluctuations, in this paper we obtain an expression for the region of the vacuum energy for each frequency $\omega$ around the H atom due to the Lamb shift. This same field plays an essential role in the van der Waals force. We show the ground state atom is surrounded by a region of positive vacuum energy that extends well beyond the atom for low frequencies. This region can be described as a steady state cloud of vacuum fluctuations. For energies $E=\hslash \omega$ less than 1 eV, where ℏ is the reduced Planck constant and $\omega$ is frequency, the radius of the positive energy region is shown to be approximately 14.4/E Å. For a vacuum fluctuation of wavelength, $\lambda$, the radius is $(\alpha /2\pi )\lambda$, where $\alpha$ is the fine-structure constant. Thus, for long wavelengths, the region has macroscopic dimensions. The energy–time uncertainty relation predicts a maximum possible radius that is larger than the radius based on the radiative shift calculations by a factor of $1/4\alpha$.

1. Introduction

Feynman called the three-page 1947 non-relativistic Lamb shift calculation by Hans Bethe the most important calculation in quantum electrodynamics because it tamed the infinities plaguing earlier attempts [1]. The seminal process in the calculation was to subtract the divergent energy shift for free electrons from the expression for the total energy shift, which reduced the divergence to a manageable logarithmic divergence. Bethe believed the radiative shift was primarily a non-relativistic phenomena and used a frequency cut-off corresponding to the the mass of the electron. When the sum over all states was evaluated numerically, his renormalized result provided a finite prediction of the energy difference between the $2S_{1/2}$ and $2P_{1/2}$ levels of the hydrogen atom that was close to the experimental values [2,3]. There are numerous higher-order contributions to the Lamb shift [4,5,6] that have been computed precisely. For example, to secure the highest accuracy possible, the upper limit of integration for the Bethe log, which Bethe took as the mass of the electron, was increased to about ${10}^{42}$ times the mass of the electron, securing a precision of 23 digits [7].

This paper, however, is not focused on high accuracy but on the spectral interpretation of the dominant lowest-order non-relativistic radiative shift, which is what Bethe calculated, and which accounts for about 97% of the total shift. This shift can be interpreted as arising from virtual transitions of the H atom induced by the quantum fluctuations of the electromagnetic field. Since the vacuum field contains all frequencies, virtual transitions to all states, bound and scattering, are possible. These short lived virtual transitions result in a slight shift in the average energy of the atom, the radiative Lamb shift [8]. The radiative shift for the ground state is positive because all virtual transitions are to higher energy levels. This same mechanism shifts every atomic energy level. For most conditions H atoms are primarily in the ground state, which is why the focus in this paper is on the ground state radiative shift and its interpretation in terms of the frequencies of the virtual transitions. The Lamb shift can also be described as an interaction of the electron with its own radiation field, yielding the exact same results as if calculated with the vacuum field [8,9]. Thus the results described in this paper do not depend on the presence of vacuum fluctuations.

Both the Lamb shift and van der Waals forces can be interpreted in terms of the interactions of atoms with the quantum fluctuations of the electromagnetic fields. When only one atom is present, the interaction results in the field around the atom corresponding to the Lamb shift. If multiple atoms are present, these clouds affect neighboring atoms; along with the zero-point field, this interaction leads to the van der Waals force.

Feynman provided an alternative non-relativistic description of the origin of the Lamb shift which is the conceptual basis for our calculation of the vacuum energy cloud. He expressed the Lamb shift in terms of the change in energy of vacuum fluctuations due to the presence of H atoms altering the index of refraction in the region around the atom [10]. The change in the refractive index alters the frequencies present, altering the energy. The actual calculation was done by Power, who showed that the shift in the energy in the vacuum field around an H atom in a large box exactly equals the non-relativistic radiative shift predicted by Bethe [9,11,12]. In this calculation, Power had to subtract the divergent effect of the free electrons to secure the same result as Bethe. This paper does not explore the details of Power’s calculation, but just uses the conclusion that the shift in the energy in the vacuum field around the atom equals the Lamb shift.

In a similar spirit, for a single H atom, Milonni computed the shift in the atomic energy level due to the Stark effect arising from the atom’s exposure to the vacuum field, finding that the shift in the atom’s energy level exactly equals the change in the energy of the vacuum fluctuations and that both equal the Lamb shift as computed by Bethe [13] (p. 438).

We have previously calculated the non-relativistic Lamb shift using SO(4,2) group theory to transform Bethe’s renormalized expression for the shift before any approximations to simplify its evaluation. The level shift is expressed as an integral of a shift spectral density over the frequency of the vacuum fluctuations [12,14] (In ref [14], there are two typographical errors: The right side of Equation (297) should have a plus sign not a minus sign; on the right side of Equation (299) the integral should have a minus sign and the ln-term should be $+{\delta }_{\mathrm{LO}}ln\frac{2}{{\left(Z\alpha \right)}^2}$.). There is no sum over states as in Bethe’s evaluation of the shift. This approach provides an analytical expression for the contribution of each frequency of the vacuum fluctuations to the radiative Lamb shift. This expression allows us to compute the volume corresponding to the spectral components present in the Lamb shift.

The calculations by Power and Milonni show that for the ground state 1S Lamb shift, which is positive, the energy density of the fluctuating zero-point field around the atom must increase such that the integral of the energy over the volume surrounding the atom gives the 1S Lamb shift. The increased energy is supplied by the quantum fluctuations of the electromagnetic field. By comparing the needed vacuum energy obtained from our calculation of the the spectral shift density [12,14] with the known energy density of the free vacuum fluctuations, it is possible to compute the volume of vacuum energy needed for each spectral component of the shift. For energies above about 100 eV, the spectral volume is much smaller than the region occupied by the ground state wavefunction; for energies below about 1 eV, the spectral volume is significantly larger than the ground state wavefunction. Consequently, the focus of this paper is on the low energy regime. For this regime, it is shown that the radius of the spherical spectral volume for a vacuum fluctuation of wavelength $\lambda$ is approximately $(\alpha /2\pi )\lambda$, where $\alpha$ is the fine structure constant. A simple estimate of the size of the virtual photon cloud based on the uncertainty relation for energy and time predicts a maximum radius of the spectral volume which is larger than that predicted by the Lamb shift model by a factor of $1/4\alpha$ [8,15].

2. Radiative Shift and Spectral Density Calculations

The group theoretical approach is based solely on the Schrodinger and Klein–Gordon equations of motion in the non-relativistic dipole approximation, with minimal coupling to the electrons field. We obtain the result [14]:

$$\Delta E_{NL}=\frac{2\alpha }{3\pi {\left(mc\right)}^2}{\int }_0^{\hslash {\omega }_c}dE\langle NL|p_i\frac{H-E_N}{H-(E_N-E)-iϵ}{p}_i|NL\rangle ,$$

(1)

where $E=\hslash \omega$ is the energy of the vacuum field, ℏ is the reduced Planck constant, $\omega$ the radial frequency, c is the speed of light, m is the mass of the electron, $p_i$ denotes the momentum operator for direction i. The Hamiltonian is $H=\frac{p^2}{2m}-\frac{Z\alpha \hslash c}{r}$, where $p^2$ is the momentum squared operator, r is the position operator for the electron, Z is the atomic number for the nucleus, and the states $|NL\rangle$ are the H atom energy eigenstates with energy $E_N$. Here, ${\omega }_c$ is a cutoff frequency for the integration such that $\hslash {\omega }_c=m{c}^2=511$ keV. Equation (1) represents the renormalized shift; the divergent shift due to the free electron has been subtracted. Inserting a complete set of states, $1=\sum |n\rangle \langle n|$, where $H|n\rangle =E_n|n\rangle$, gives Bethe’s expression for the finite observable shift $\Delta E_{NL}$ for the state $|NL\rangle$:

$$\Delta E_{NL}={\int }_0^{\hslash {\omega }_c}dE\frac{2\alpha }{3\pi {\left(mc\right)}^2}\sum _n{\left|{\mathbf{p}}_{nN}\right|}^2\frac{(E_n-E_N)}{E_n-E_N+E-iϵ}.$$

(2)

Bethe performed the integration over E, assuming that $m{c}^2\gg |E_n-E_N|$, to obtain an expression for the Lamb shift for an S state with principle quantum number N [3]:

$$\Delta E_N^{\mathrm{Bethe}}=\frac{4\alpha }{3\pi }{\left(\frac{1}{mc}\right)}^2\sum _n{\left|{\mathbf{p}}_{nN}\right|}^2(E_n-E_N)ln\left(\frac{m{c}^2}{E_n-E_N}\right).$$

(3)

To simplify the evaluation of the sum, Bethe took the ln term out of the summation and replaced $E_n$ with an average energy. An alternative approach is taken in this paper.

One can express the radiative shift $\Delta E_1$ for the 1S ground state as an integral over the renormalized spectral shift density $d\Delta E_1/dE$ [12]:

$$\Delta E_1={\int }_0^{m{c}^2}\frac{d\Delta E_1}{dE}dE.$$

(4)

This equation is a definition of the spectral shift density: if a level shift is expressed as an integral over energy, then the integrand is the spectral shift density. Since the shift $\Delta E_1$ is in units of eV, the spectral shift density $\frac{d\Delta E_1}{dE}$ has the units of eV/eV, which is dimensionless. The spectral shift density from Bethe’s formulation equals the integrand in Equation (2), which requires the evaluation of a sum over all states, including scattering states. A more convenient expression for the spectral shift density for the renormalized ground state can be obtained by transforming Equation (1) using group theory [14]:

$$\frac{d\Delta E_1}{dE}=\frac{4{\alpha }^3}{3\pi }{e}^{-2\varphi }sinh\varphi {\int }_0^{\infty }ds{e}^{s{e}^{-\varphi }}\frac{1}{{sinh}^2(s/2)}\frac{1}{{\left(coth(s/2)+cosh\varphi \right)}^3}.$$

(5)

The integral over s can be evaluated exactly by Mathematica for specific values of the dimensionless normalized frequency variable, $\varphi$:

$$\varphi =\frac{1}{2}ln\left(1+\frac{E}{|E_1|}\right),$$

(6)

where $E_1$ is the ground state energy of −13.6 eV.

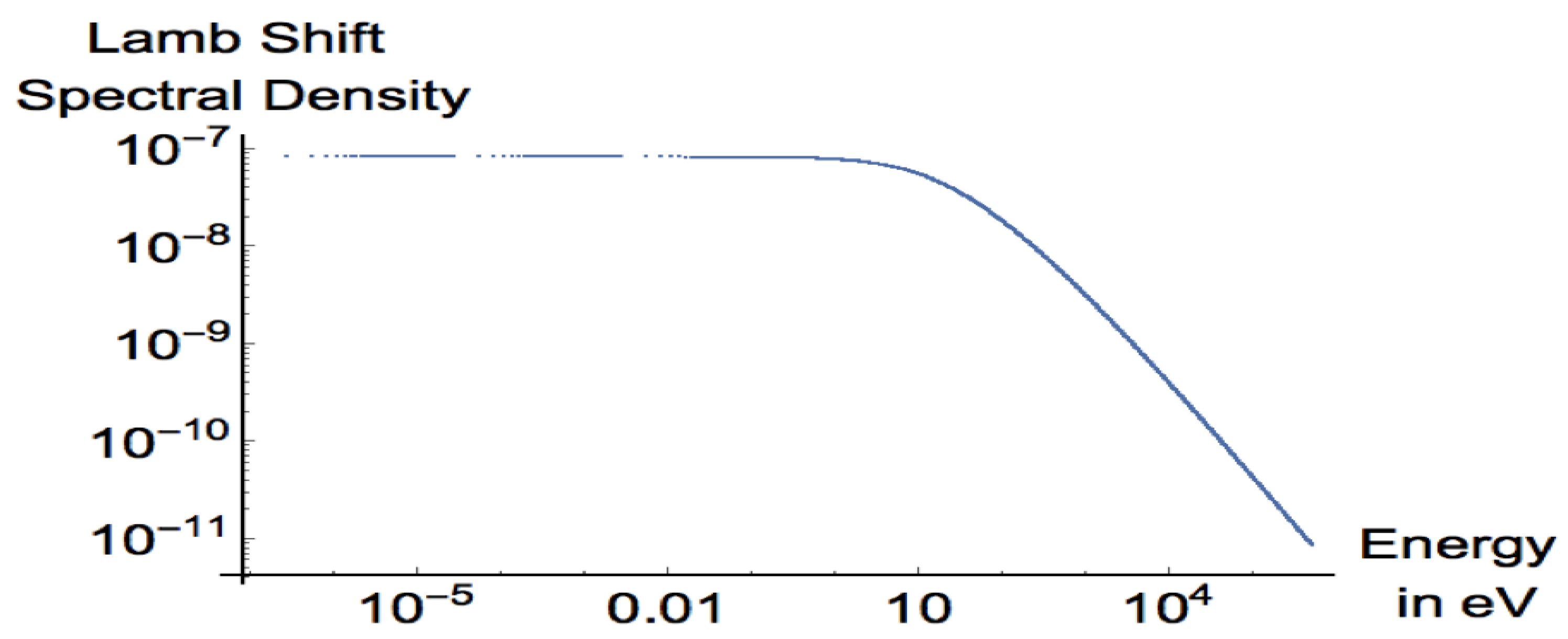
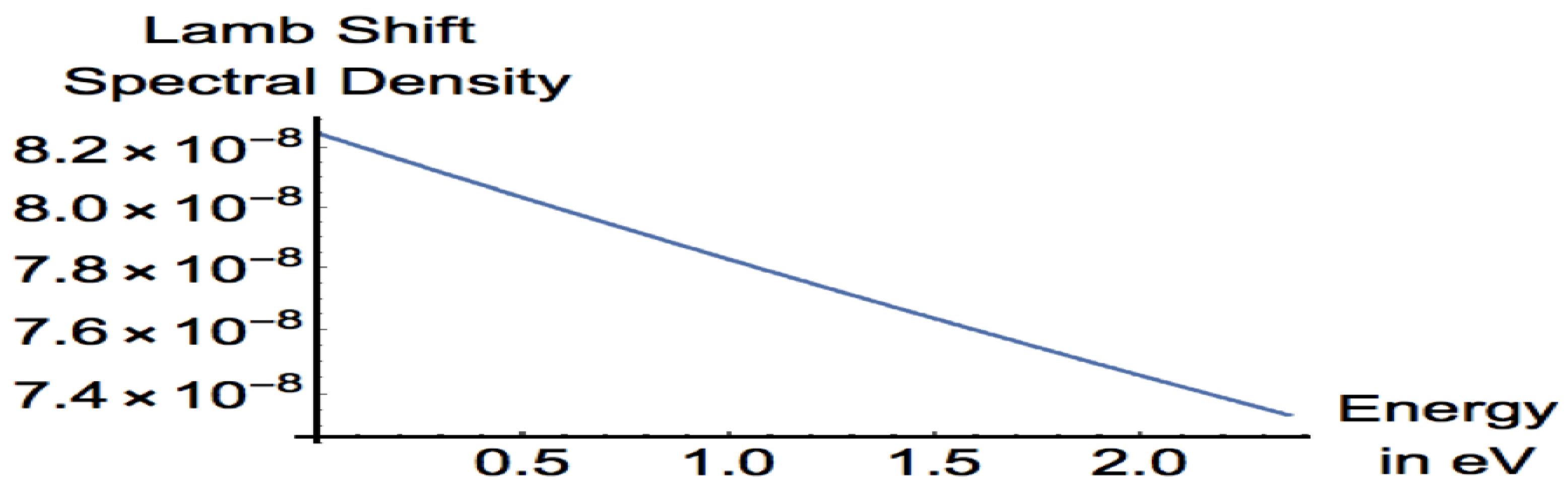
Figure 1 shows a log–log plot of the spectral density $\frac{d\Delta E_1}{dE}$ of the ground state Lamb shift (with Z = 1) for the entire range of energy, E, computed from Equation (5). For energies above about 100 eV the spectral density is approximately proportional to 1/E, whereas below about 10 eV the spectral density increases slowly to a maximum at $E=0$, as shown in Figure 2.

The low-energy limit of the group theoretical result from Equation (5) for the S state shift density can be taken analytically, giving [12]

$$\frac{d\Delta E_n}{dE}{|}_{E\to 0}=\frac{2\alpha }{3\pi }\frac{{\left(Z\alpha \right)}^2}{n^2}-\frac{\alpha }{\pi m{c}^2}E,$$

(7)

where n is the principle quantum number. The corresponding spectral density for the ground state with $n=1\mathrm{and}Z=1$ is

$$\frac{d\Delta E_1}{dE}{|}_{E\to 0}=\frac{4\alpha \times 13.6}{3\pi m{c}^2}\left(1-\frac{3E}{4\times 13.6}\right)=8.253\times {10}^{-8}(1-0.0551E),$$

(8)

which is in agreement with Figure 2. As E decreases to zero, the spectral density increases linearly to a constant value, $\frac{4\alpha }{3\pi }\frac{|E_n|}{m{c}^2}=2{\alpha }^3{Z}^2/3\pi n^2=8.253\times {10}^{-8}/n^2$. The intercept follows $1/n^2$ behaviour and the slope equals $\alpha /\pi m{c}^2$. For $E<1$ eV, the ground state spectral density equals 8.25 × ${10}^{-8}$ to within about $5\%$.

3. Computing the Size of the Vacuum Energy Field

Consider a large box containing H atoms in the ground state. The spectral density $\frac{d\Delta E_1}{dE}$ of the ground state shift and the energy density of the quantum vacuum with no H atoms present are both known. In the box containing the H atoms, the vacuum field density must increase such that the integral of the energy density over the volume provides the 1S Lamb shift. This increase in vacuum energy results from the vacuum fluctuations, which have a free-field spectral energy density (energy/volume-frequency) equal to [9]

$${\rho }_0\left(\omega \right)=\frac{\hslash {\omega }^3}{2{\pi }^2{c}^3}$$

(9)

where c is in cm/s, $\omega$ is the radial frequency in ${\mathrm{s}}^{-1}$ and $\rho$ has units of $\mathrm{erg}/\mathrm{c}{\mathrm{m}}^3-{\mathrm{s}}^{-1}$. If the frequency is measured in eV then it is essentially the energy $E=\hslash \omega$ and the vacuum spectral energy density has units of (eV/cm${}^3-$ eV) = 1/cm${}^3$ and is

$${\rho }_0\left(E\right)=\frac{E^3}{2{\pi }^2{\hslash }^3{c}^3},$$

(10)

so that the integral ${\int }_{E_1}^{E_2}{\rho }_0\left(E\right)dE$ represents the energy density eV/cm${}^3$ in the energy interval $E_1$ to $E_2$. The question being addressed here is: what volume of vacuum energy of density ${\rho }_0\left(E\right)$ is required to supply the amount of energy corresponding to the radiative shift? The total renormalized radiative shift $\Delta E_1$ can be expressed as the integral of the vacuum energy density ${\rho }_0\left(E\right)$ over an effective volume $V_1\left(E\right)$:

$$\Delta E_1={\int }_0^{m{c}^2}dE{\rho }_0\left(E\right)V_1\left(E\right),$$

(11)

with the same upper limit for E as used previously [9]. Recall the definition of the spectral shift density Equation (4):

$$\Delta E_1={\int }_0^{m{c}^2}dE\frac{\Delta E_1}{dE}.$$

(12)

Comparing Equation (11) with Equation (12) shows that the effective spectral volume $V_1\left(E\right)$ needed to insure energy balance at each energy E is [12]

$$V_1\left(E\right)=\frac{d\Delta E_1}{dE}\frac{1}{{\rho }_0\left(E\right)}.$$

(13)

The spectral volume $V_1\left(E\right)$ has dimensions of cm${}^3$ and contains the amount of vacuum energy at energy value E that corresponds to the ground state spectral density at the same energy E.

Equations (11)–(13) are general equations and apply to any calculation of the radiative Lamb shift that can be expressed as an integral over the vacuum energy, as in Equation (4). The utility of Equations (11)–(13) lies in our ability to provide an explicit analytical expression for the spectral shift using our group theoretical results [14].

An example of Equation (11) is in the calculation of the Lamb shift as a Stark shift by Milonni [9]. Consider the energy $W=-\frac{1}{2}\mathbf{d}·\mathbf{E}\left(\omega \right)$ for a dipole $\mathbf{d}$ in an isotropic field, $\mathbf{E}\left(\omega \right)$. Assuming that the dipole is induced by the field, then $\mathbf{d}\left(\omega \right)=\alpha \left(\omega \right)\mathbf{E}\left(\omega \right)$. The energy for an atom A at ${\mathbf{x}}_a$ with polarizability ${\alpha }_A\left(\omega \right)$ can be expressed as [9]

$$W_A=-\frac{1}{2}{\int }_0^{\infty }d\omega {\alpha }_A\left(\omega \right)\langle {\mathbf{E}}^2\left(\omega \right)\rangle .$$

(14)

For the Lamb shift, $\langle \mathbf{E}{\left(\omega \right)}^2\rangle =4\pi {\rho }_0\left(\omega \right)$, where ${\rho }_0$ is the zero-point vacuum spectral energy density, one obtains

$$W_A=-2\pi {\int }_0^{\infty }d\omega {\alpha }_A\left(\omega \right){\rho }_0\left(\omega \right).$$

(15)

The polarizability is provided by the Kramers–Heisenberg formula, and has units of volume. This expression for the Lamb shift has the same form as Equation (11). To complete the Stark shift calculation, the contribution from free electrons needs to be subtracted, after which the final result is identical to that of Bethe [9].

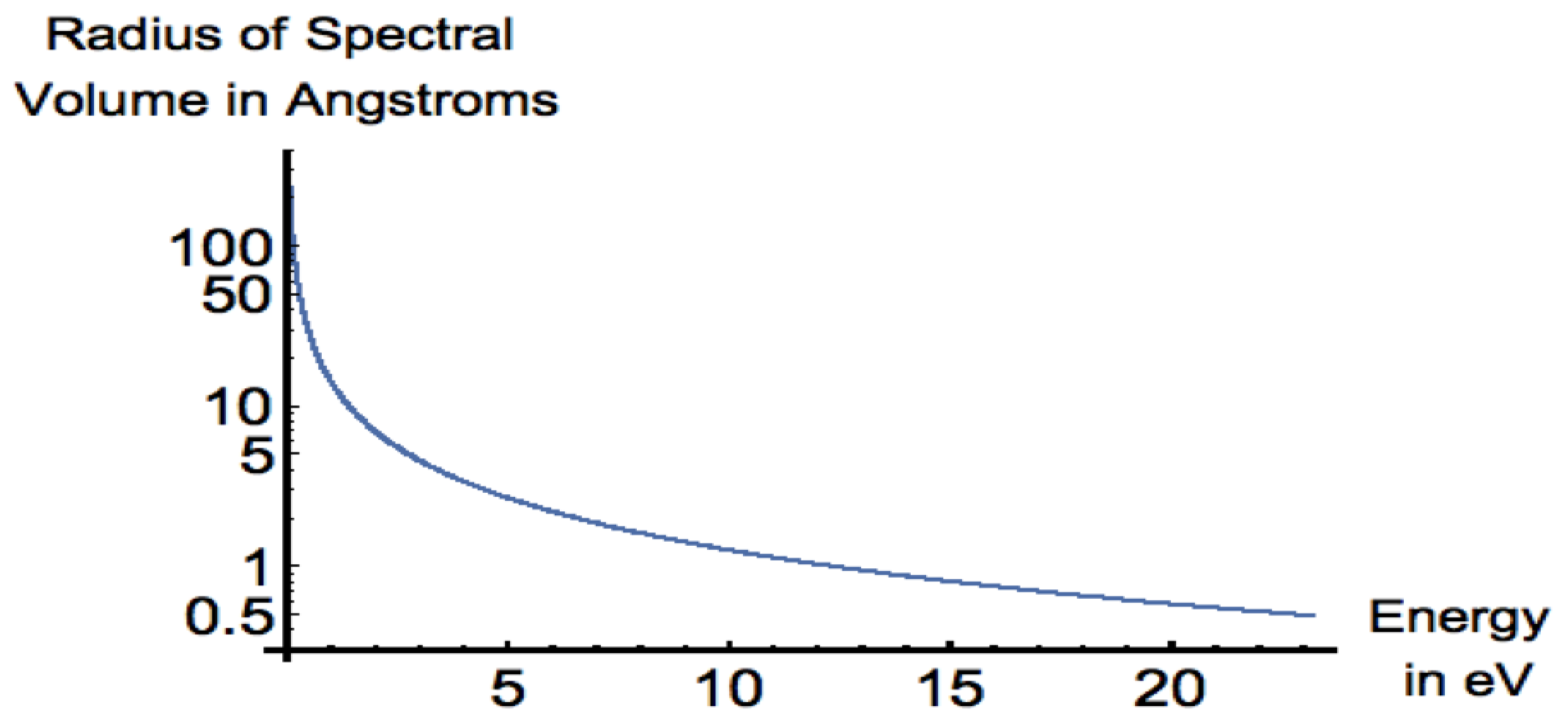
The spectral volume, $V_1\left(E\right)$, in Equation (13) is assumed to be spherical since the ground state is an S state, so the radius can be calculated from the known spectral volume. In Section 4 this assumption is discussed in more detail. Figure 3 shows a log–log plot of the radius in Å of the spectral volume, $V_1\left(E\right)$, for the ground state as a function of E. Figure 4 shows the radius of the spherical spectral volume for energies below 23 eV. For an energy of 21.7 eV, the spectral radius equals the mean radius of the ground state wavefunction of 0.53 Å. For energies less than 21.7 eV, the radius will be greater than the ground state radius. For an energy of 1 eV, the radius is about 14 Å. This calculation predicts that there is a sphere of positive vacuum energy of radius 14 A around the atom, corresponding to the 1 eV shift in the spectral density.

For low-energy vacuum fluctuations, the spectral density from Equation (7) can be approximated for an S state with principle quantum number n as a constant:

$$\frac{d\Delta E_n}{dE}{|}_{E\to 0}=\frac{2\alpha }{3\pi }\frac{{\left(Z\alpha \right)}^2}{n^2}.$$

(16)

Equation (16) is accurate to about 5% at 1 eV, and the accuracy increases as the energy decreases. This approximation corresponds to the end point $E=0$ of the nearly horizontal portion of the spectral density in Figure 1. For these low energies, the spectral volume, $V_n\left(E\right)$, from Equation (13) is

$$V_n\left(E\right)=\frac{4\pi }{3}\frac{\alpha {\left(Z\alpha \right)}^2}{n^2}\frac{{(\hslash c)}^3}{E^3}.$$

(17)

Assuming a spherical spectral volume of radius $R_V\left(E\right)$ for a state n, one obtains:

$$R_V\left(E\right)={\left[\frac{\alpha {\left(Z\alpha \right)}^2}{n^2}\right]}^{1/3}\frac{\hslash c}{E},$$

(18)

which for low E for the 1S state of hydrogen gives

$$R_V\left(E\right)=\alpha \frac{\hslash c}{E}=\frac{14.4\AA }{E\mathrm{eV}}.$$

(19)

The H atom is surrounded by a steady-state cloud of virtual quanta; this cloud has a radius $R_V\left(E\right)$ and is continuously emitted and reabsorbed by the field. The virtual quanta cannot leave the vicinity of the atom, as they are reabsorbed. This vacuum energy density of the cloud is positive in the sense that it is above the free-field vacuum energy density.

It is remarkable that the asymptotic low energy spectral radius, $R_V\left(E\right)$, in Equation (19) has such a simple form. This result can be rewritten using the definition, $\alpha =e^2/\hslash c$, with e the elementary charge, as

$$E=\frac{e^2}{R_V\left(E\right)}.$$

(20)

Thus the Coulomb energy for two electrons separated by a distance $R_V\left(E\right)$ equals the energy $E=\hslash \omega$ of the corresponding vacuum virtual photon.

It is interesting to compare the radius $R_V\left(E\right)$ of the spectral volume with the wavelength, $\lambda$, of the vacuum fluctuation corresponding to $E=\hslash \omega =2\pi \hslash c/\lambda$. For the ground state, this gives

$$R_V\left(E\right)=\frac{\alpha }{2\pi }\lambda =\frac{\lambda }{861}.$$

(21)

The radius of the spectral volume equals $\alpha /2\pi$ times the wavelength of the corresponding vacuum fluctuation. Thus long-wavelength vacuum fluctuations produce macroscopic regions of positive vacuum energy for the hydrogen ground state.

Comparison to Predictions from the Uncertainty Relation

A simple analysis using the uncertainty relation can provide an order of magnitude estimate of the largest extent of the positive energy vacuum field. The hydrogen atom is a quantum system, and its ground state energy can consequently vary for a time interval $\tau$ by an amount $\Delta E_u$ which is constrained by the uncertainty relation [13] (p. 201)

$$\Delta E_u\tau <\hslash /2.$$

(22)

The variation in energy is modeled by the emission and absorption of virtual photons of energy $\Delta E_u=\hslash {\omega }_u$ and frequency ${\omega }_u$. Since the velocity of the photon is c, in the time $\tau$ it can travel a distance $2R_u$ where [15]

$$R_u<\frac{\hslash c}{4\Delta E_u}=\frac{c}{4{\omega }_u}=\frac{\lambda }{8\pi },$$

(23)

where $\lambda$ is the wavelength of the virtual photon. Comparing Equation (23) to Equation (21) shows that for the same energy virtual photon,

$$R_V=4\alpha R_u.$$

(24)

For vacuum fluctuations of energy E below about 1 eV, the dimension $R_V$ of the virtual cloud predicted by an analysis of the ground state Lamb shift is $4\alpha$ times smaller than the maximum extent, $R_u$, allowed by the uncertainty relation. Ref. [15] has suggested that $(4\pi /3)\alpha$ can be considered the mean density of virtual photons in the region around the atom, which may explain the difference between $R_u$ and $R_V$.

4. Significance of the Zero-Point Field around the Atom

The cloud of quantum fluctuations surrounding the H atom can be interpreted as resulting from the scattering of the free-field vacuum fluctuations by the atom. The zero-point field activates the atom in a continuous process, creating the steady state cloud of quantum fluctuations that is described in this paper. As the derivation of the Lamb shift in terms of the Stark effect suggests, the zero-point field induces an instantaneous dipole moment in the atom that leads to a dipole field. The continuous stochastic excitation from the zero-point field leads to a sum of incoherent contributions that average to a spherically symmetric cloud [13] (p. 201).

One can imagine the atom undergoing virtual transitions from the ground state to all higher energy states and then returning to the ground state in accordance with the time–energy uncertainty relation. For a zero-point fluctuation of wavelength $\lambda$, our calculations show that the cloud extends about $\alpha \lambda /2\pi$ from the nucleus, which can be a macroscopic distance. Thus far, direct measurement of such vacuum fluctuations has eluded experimentalists. There are two ways of exploring the significance of this cloud of vacuum energy: first, by computing estimates of the mean energy density; and second, by explaining its role in the creation of van der Waals forces under the assumption that another H atom is nearby.

4.1. Energy Density of the Zero-Point Field around the Atom

Using the results of Section 3, it is possible to compute the energy density of this field as a function of distance for different wavelength or energy intervals of the zero-point field. Consider a spherical shell: the inner radius corresponds to one energy and is given by Equation (19). The outer radius corresponds to a slightly smaller energy. For this energy interval, one can estimate the contribution to the total Lamb shift by integration of the curve in Figure 1 [12]. For energies below 1 eV the contribution to the ground state Lamb shift is about 0.24% of the total shift. In this low energy range, the contribution to the shift scales linearly with the energy, as shown in Figure 2. This allows us to compute a mean energy density, ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}$, of the quantum fluctuations in a spherical shell.

The density of the Lamb shift energy in the spherically symmetric region of vacuum energy surrounding the H atom can be analyzed in terms of shells with an outer radius of $R=\alpha \hslash c/E$ and inner radius of $R_1=\alpha \hslash c/E_1$. It is convenient to let $E_1=\beta E$, where $\beta >1$. Assuming that both energies are less than 1 eV, one can integrate the Lamb shift (LS) spectral density from Equation (16) for the ground state and Z = 1 to obtain

$$\Delta E_{\mathrm{LS}}^{\mathrm{shell}}\left(E\right)={\int }_E^{\beta E}dE\frac{2{\alpha }^3}{3\pi }=\frac{2{\alpha }^3}{3\pi }E(\beta -1)$$

(25)

to an accuracy of about 5%. The volume of the shell is

$$V^{\mathrm{shell}}\left(E\right)=\frac{4\pi }{3}{(\alpha \hslash c)}^3\left(1-\frac{1}{{\beta }^3}\right)\frac{1}{E^3}.$$

(26)

Therefore the Lamb shift energy density (in erg/cm${}^3$) in the shell is

$${\rho }_{\mathrm{LS}}^{\mathrm{shell}}\left(E\right)=\frac{\Delta E_{\mathrm{LS}}^{\mathrm{shell}}\left(E\right)}{V^{\mathrm{shell}}\left(E\right)}=\frac{1}{2{\pi }^2}\frac{1}{{(\hslash c)}^3}\frac{\beta -1}{1-1/{\beta }^3}{E}^4.$$

(27)

The Lamb shift energy density for a shell with outer radius $R=\alpha \hslash c/E$, and inner radius $R_1=\alpha \hslash c/\left(\beta E\right)$, is proportional to $E^4$ or $1/R_V^4\left(E\right)$. Figure 5 shows the value of ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}$ as a function of the inner radius (in Å), where the outer radius is 1.03 times the inner radius ($\beta =1.03$).

One can compare the energy density ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}\left(E\right)$ from Equation (27) (in erg/cm${}^3$) to the energy density ${\rho }_0^{\mathrm{shell}}\left(E\right)$ (in erg/cm${}^3$) of the free zero-point vacuum field for the same spectral interval, i.e., from E to $\beta E$:

$${\rho }_0^{\mathrm{shell}}\left(E\right)={\int }_E^{\beta E}dE{\rho }_0\left(E\right)=\frac{1}{8{\pi }^2{\hslash }^3{c}^3}{E}^4({\beta }^4-1).$$

(28)

One finds that the ratio

$$\frac{{\rho }_{\mathrm{LS}}^{\mathrm{shell}}\left(E\right)}{{\rho }_0^{\mathrm{shell}}\left(E\right)}=4\frac{\beta -1}{1-1/{\beta }^3}\frac{1}{{\beta }^4-1}=g\left(\beta \right),$$

(29)

is a constant that depends on $\beta$. The Lamb shift energy density for the shell is directly proportional to the free vacuum energy density for the same energy interval. This result follows for low E since the spectral density, $\frac{d\Delta E_1}{dE}$ from Equation (16), is a constant. Comparison with Equation (13) shows that ${\rho }_0\left(E\right)V_1\left(E\right)$ is therefore constant and independent of E for low E values.

The function $g\left(\beta \right)$ is singular at $\beta =1$ and decreases rapidly as $\beta$ increases. For $1<\beta <1.35$, $g\left(\beta \right)$ is greater than 1. For $\beta$ of (1.01, 1.02, 1.03, 1.05, 1.1), the corresponding values of $g\left(\beta \right)$ are $(33.5,16.8,11.3,6.8,3.5)$. For these shells, ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}\left(E\right)$ is always larger than ${\rho }_0^{\mathrm{shell}}\left(E\right)$. Just as the free-field vacuum fluctuations are important in many physical systems, the field of fluctuations due to the Lamb shift must be equally important.

Table 1. The inner and outer radii for a spherical shell around the atom, the corresponding fluctuation energies ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}$ and ${\rho }_0^{\mathrm{shell}}$, and the ratio of ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}$ to ${\rho }_0^{\mathrm{shell}}$.

Inner and Outer Radii of Spherical Shell (in Å)	Quantum Fluctuation Energy Range (in eV)	Mean Shell Lamb Shift Energy Density, ${\mathit{\rho }}_{\mathbf{LS}}^{\mathbf{shell}}$ (in erg/cm${}^3$)	Mean Shell Free Field Energy Density, ${\mathit{\rho }}_0^{\mathbf{shell}}$ (in erg/cm${}^3$)	${\mathit{\rho }}_{\mathbf{LS}}^{\mathbf{shell}}/{\mathit{\rho }}_0^{\mathbf{shell}}$
4.64 to 8.13	Visible 3.10 to 1.77	98.7	218	0.45
10 to 20	0.72 to 1.44	3.34	10.65	0.314
20 to 30	0.48 to 0.72	0.399	0.570	0.700
30 to 40	0.36 to 0.48	0.1024	0.0959	1.068
40 to 50	0.288 to 0.36	0.0373	0.0262	1.16
50 to 60	0.240 to 0.288	0.0167	0.00941	1.77
60 to 70	0.2057 to 0.240	0.00852	0.00403	2.12
70 to 80	0.180 to 0.2057	0.00480	0.00196	2.45
80 to 90	0.160 to 0.180	0.00291	0.00104	2.79
90 to 100	0.144 to 0.16	0.00186	0.000595	3.13
140 to 150	0.096 to 0.1029	0.000344	0.0000718	$4.78$
200 to 210	0.0686 to 0.072	8.59 × ${10}^{-5}$	1.25 × ${10}^{-5}$	6.87
300 to 310	0.0465 to 0.048	1.76 × ${10}^{-5}$	1.67 × ${10}^{-6}$	$10.49$
400 to 410	0.0351 to 0.036	5.63 × ${10}^{-6}$	4.27 × ${10}^{-7}$	13.16
1000 to 1020	0.01412 to 0.0144	1.46 × ${10}^{-7}$	8.58 × ${10}^{-9}$	16.97

shows the results of computing the energy densities for different spherical shells. The first row corresponds to a shell with the frequency range of the visible spectrum (400 nm to 700 nm), for which the energy density in the shell, ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}$, is 98.7 erg/cm${}^3$, which is 45% of the corresponding ${\rho }_0^{\mathrm{shell}}$ (the free-field energy density for the shell) of 218 erg/cm${}^3$. For cases with $\beta <1.35$, the ratio ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}/{\rho }_0^{\mathrm{shell}}$ in the fifth column is greater than one.

The energy densities ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}$ for the shells are significant, for example, compared to the energy densities, ${\rho }_{\mathrm{bb}}$, for black body radiation over the same spectral intervals. For a temperature of 600 K (for which the peak intensity is at about 5 micrometers or 0.25 eV) the ratio of ${\rho }_{\mathrm{LS}}^{\mathrm{shell}}/{\rho }_{\mathrm{bb}}$ is 2.8 ×${10}^4,148$, and $10.1$, respectively, for the shells with radii 20–30 Å, 50–60 Å, and 200–210 Å. Of course black body radiation is ordinary electromagnetic radiation, while the Lamb shift energy consists of vacuum fluctuations of the electromagnetic field.

4.2. Relationship between the Zero-Point Field around the Atom and van der Waals Forces

Zero-temperature Lamb shifts and van der Waals interactions have straightforward physical interpretations in terms of fluctuating zero-point fields [16]. Here, we consider an isolated atom A and describe the fluctuating field around this atom that arises from its interaction with the free field vacuum fluctuations. The field around atom A corresponds to the non-relativistic Lamb shift for atom A. If another atom is present, the field around A plays an essential role in the van der Waals forces between the atoms.

To illustrate this, generalize Equation (14) for the energy of an induced dipole at A to include a second atom B. The total field is ${\mathbf{E}}_{\mathbf{k},\omega }$ and the combined energy is [9] (Section 3.11)

$$W_{AB}=-\frac{1}{2}\sum _{\mathbf{k}\omega }{\alpha }_A\left({\omega }_k\right)\langle {\mathbf{E}}_{\mathbf{k}\omega }^2({\mathbf{x}}_A,t)\rangle ,$$

(30)

where ${\alpha }_A\left({\omega }_k\right)$ is the polarizability of atom A, $\mathbf{k}$ is the wave vector, and t denotes the time. The presence of the second atom breaks the spherical symmetry so a summation over $\mathbf{k}$ for the non-isotropic field is included. The total field acting on A is assumed to be the sum of the zero-point field ${\mathbf{E}}_{0,\mathbf{k}\omega }({\mathbf{x}}_A,t)$ acting on A and the field produced at A by atom B:

$${\mathbf{E}}_{\mathbf{k}\omega }({\mathbf{x}}_A,t)={\mathbf{E}}_{0,\mathbf{k}\omega }({\mathbf{x}}_A,t)+{\mathbf{E}}_{B,\mathbf{k}\omega }({\mathbf{x}}_A,t).$$

(31)

Each atom is “driven” by the zero-point field at its location, creating a fluctuating dipole field about the atom. The field about atom B affects atom A and vice versa. The portion of the energy $W_{AB}$ that depends on the distance between the atoms corresponds to the van der Waals force, and is

$$W_{AB}^{\mathrm{vdW}}=-\frac{1}{2}\sum _{\mathbf{k}\omega }{\alpha }_A\left({\omega }_k\right)\langle {\mathbf{E}}_{0,\mathbf{k}\omega }({\mathbf{x}}_A,t){\mathbf{E}}_{B,\mathbf{k}\omega }({\mathbf{x}}_A,t)+{\mathbf{E}}_{B,\mathbf{k}\omega }({\mathbf{x}}_A,t){\mathbf{E}}_{0,\mathbf{k}\omega }({\mathbf{x}}_A,t)\rangle .$$

(32)

The term ${\alpha }_A\left({\omega }_k\right){\langle {\mathbf{E}}_{0,\mathbf{k}\omega }({\mathbf{x}}_A,t)\rangle }^2$ in the summation in Equation (30) does not depend on the separation between the atoms, and corresponds to the Lamb shift for atom A. This is the field of vacuum fluctuations about the atom which represents the atom’s response to the vacuum field described in this paper. From Equation (32), one can immediately see that this field plays an essential role in the van der Waals force. Similarly, this field would be essential for the Casimir–Polder force between an atom and a surface [9].

The term ${\mathbf{E}}_{B,\mathbf{k}\omega }({\mathbf{x}}_A,t)$ represents the field from the induced dipole at atom B, and is proportional to the polarizability ${\alpha }_B\left({\omega }_k\right)$ of atom B. After computation, the final expression for the van der Waals force is shown to be a symmetric integral over $\omega$ of the product ${\alpha }_A\left(\omega \right){\alpha }_B\left(\omega \right)$ times a function of $\omega$ and $r=|{\mathbf{x}}_A-{\mathbf{x}}_B|$.

Along with the free field, the field about the atom corresponding to the Lamb shift affects the nearby atom and results in a fluctuating induced dipole moment. The correlation between the fluctuating dipole moments at the two locations gives rise to the van der Waals forces. The correlation falls off rapidly with frequency and with the distance r between the two locations, giving the $r^{-6}$-dependence of the non-retarded van der Waals interaction [9]. The cloud of zero-point fluctuations about the H atom described in this paper is fundamental to the van der Waals forces as well as to the Lamb shift. These phenomena are linked in that both arise from the interaction between atoms and the fluctuating zero-point field.

Van der Waals forces tend to become retarded for distances greater than about $a_0/\alpha$ ($a_0$ being the Bohr radius of the ground state wavefunction), or about 70 Å. From the calculations in Table 1, one can see that lower energy fluctuations are responsible for these dispersion forces.

5. Conclusions

The nonrelativistic Lamb shift can be interpreted as being due to the interaction between atoms and the fluctuating zero-point electromagnetic field of the quantum vacuum. The renormalized radiative Lamb shift can be expressed in terms of a spectral shift density, which is a function of the frequency $\omega$ or energy $E=\hslash \omega$ of the vacuum field. The integral of the spectral density from $E=0$ to the rest mass energy of an electron, 511 keV, gives the nonrelativistic radiative shift for that state of the atom. Feynman, Power, and Milonni showed that the radiative shift equals the change in the energy of the vacuum fluctuations in the region containing the H atom. Using this result with a group-theoretical calculation of the contribution to the Lamb shift from each frequency of the vacuum fluctuations, one can obtain an expression for the size of the region of vacuum energy corresponding to each value of the vacuum energy E around the H atom. The spectral volume for the energy E around an H atom contains vacuum fluctuations of energy E; the total energy of these fluctuations equals the radiative shift corresponding to that energy E. For the ground state, the energy density in the spectral volume is positive, meaning that it is above the free-field energy density. For $E>23$ eV, the radius of the region of positive energy vacuum fluctuations is less than the atomic radius; on the other hand, for energies less than 1 eV the radius is shown to be approximately $\alpha \hslash c/E=14.4/E$ Å, and can be much larger than the ground state wavefunction. The radius of the spectral volume can also be expressed in terms of the wavelength of the corresponding vacuum fluctuations as $\alpha \lambda /2\pi =\lambda /861$. An estimate of the extent of photons from virtual transitions based on the uncertainty relation for time and energy predicts a maximum radius that is about $1/4\alpha$ larger that the radius based on the radiative shift calculations.

The vacuum energy field around the H atom described in this paper plays an essential role in the van der Waals forces as well as in the Lamb shift. These phenomena are linked since both arise from the interaction between atoms and the fluctuating zero-point field.

The calculations in this paper were performed for the ground state of H, which has a positive radiative shift. States with a negative radiative shift, such as 2P, would have a spectral volume as well; however, the energy would be negative, i.e., below the free-field vacuum energy. Notably, this analysis is complicated by the fact that the 2P state decays to the 1S state.