Probing Different Characteristics of Shell Evolution Driven by Central, Spin-Orbit, and Tensor Forces
Abstract
:1. Introduction
2. Shell Evolution Caused by the SDPF-MU Interaction
2.1. Monopole Matrix Elements
- the central force includes density dependence;
- the two-body spin–orbit force is included in addition.
- Similar to the central force, the strengths for the orbitals are weaker than those of .
- Although the absolute values of the tensor matrix elements are much smaller than those of the central force, the difference between the largest matrix element and the smallest one reaches MeV, equivalent to that of the central force.
- The label consists of two characters: the first and the second ones are intended to grade the central and tensor monopole matrix elements, respectively. The net effect of these two characters stands for a rough estimate of the total monopole matrix element.
- Each part is evaluated on a scale of five levels defined by −, , 0, , and +, to indicate relative attraction within each type of force. The ”−” character is given to the most attractive (i.e., largest negative) pairs, and the ”+” character is given to the least attractive (or most repulsive) pairs among the whole monopole matrix elements of the central or tensor force.
- The first character gets ”−” for , or ”+” for .
- When the first character is ”−”, the second character gets either ”−”, 0 or ”+” depending on the relative spin direction mentioned above. When the first character is ”+”, the second character is replaced by , 0, or .
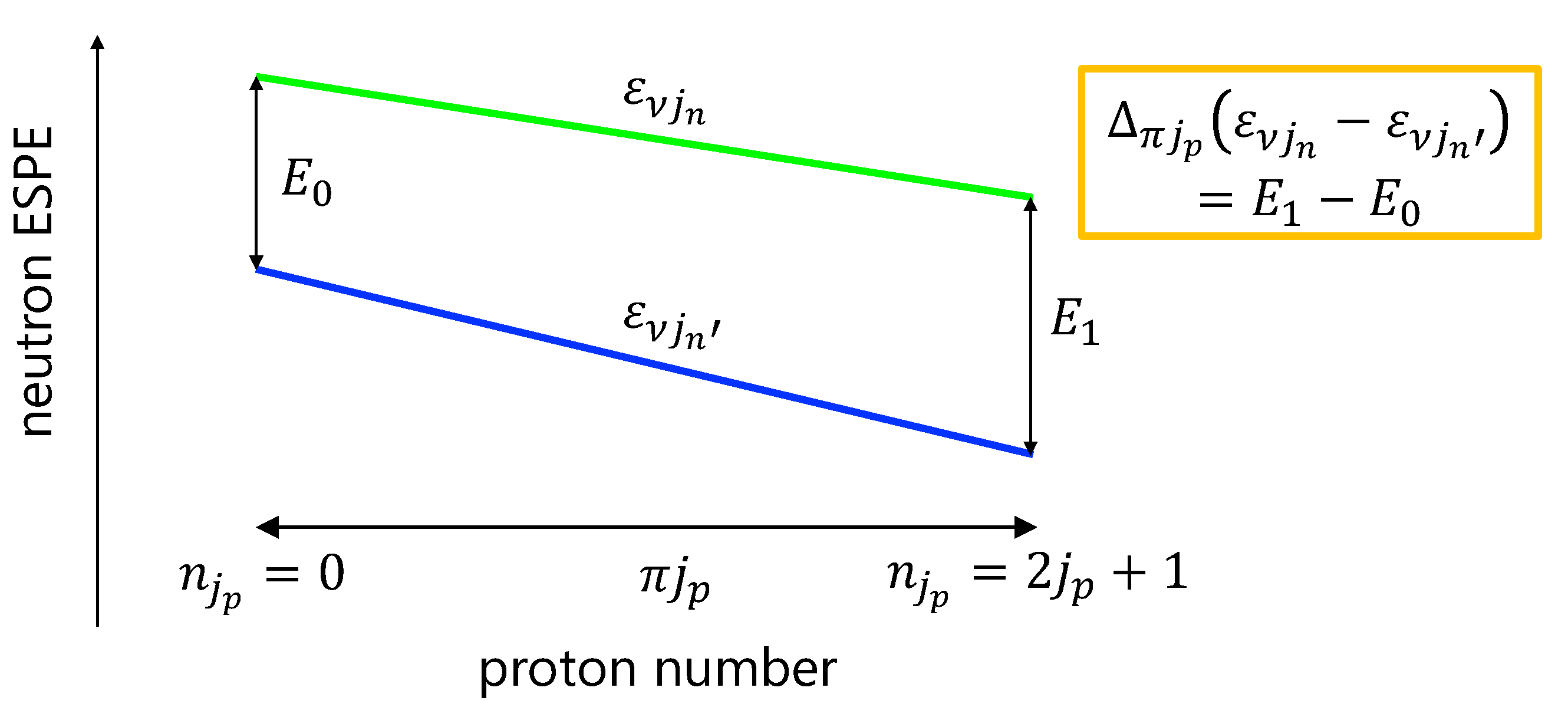
2.2. Effective Single-Particle Energies
3. Comparison to Experimental Data
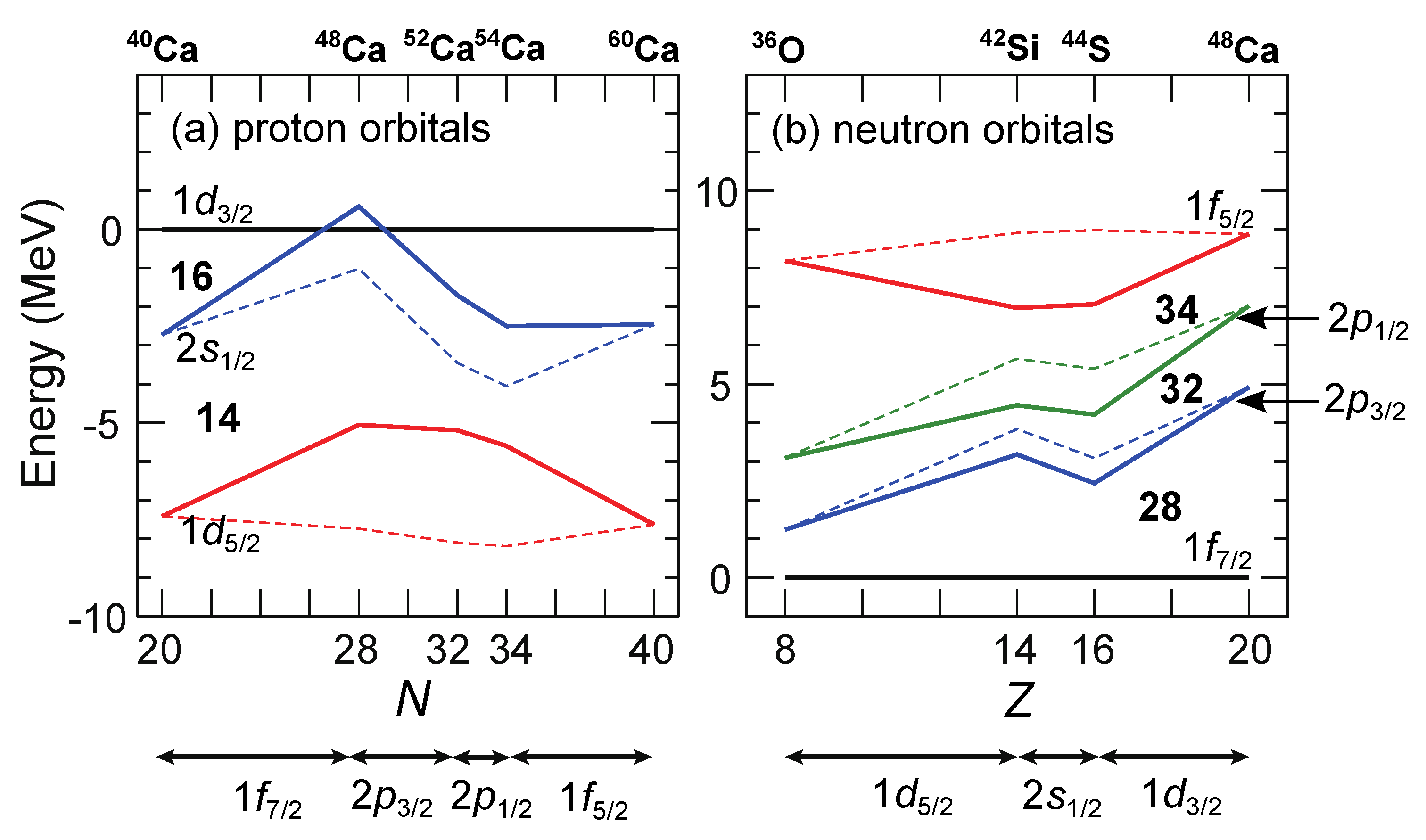
3.1. Proton Shell Evolution
3.1.1. From to
3.1.2. From to and Beyond
3.2. Neutron Shell Evolution
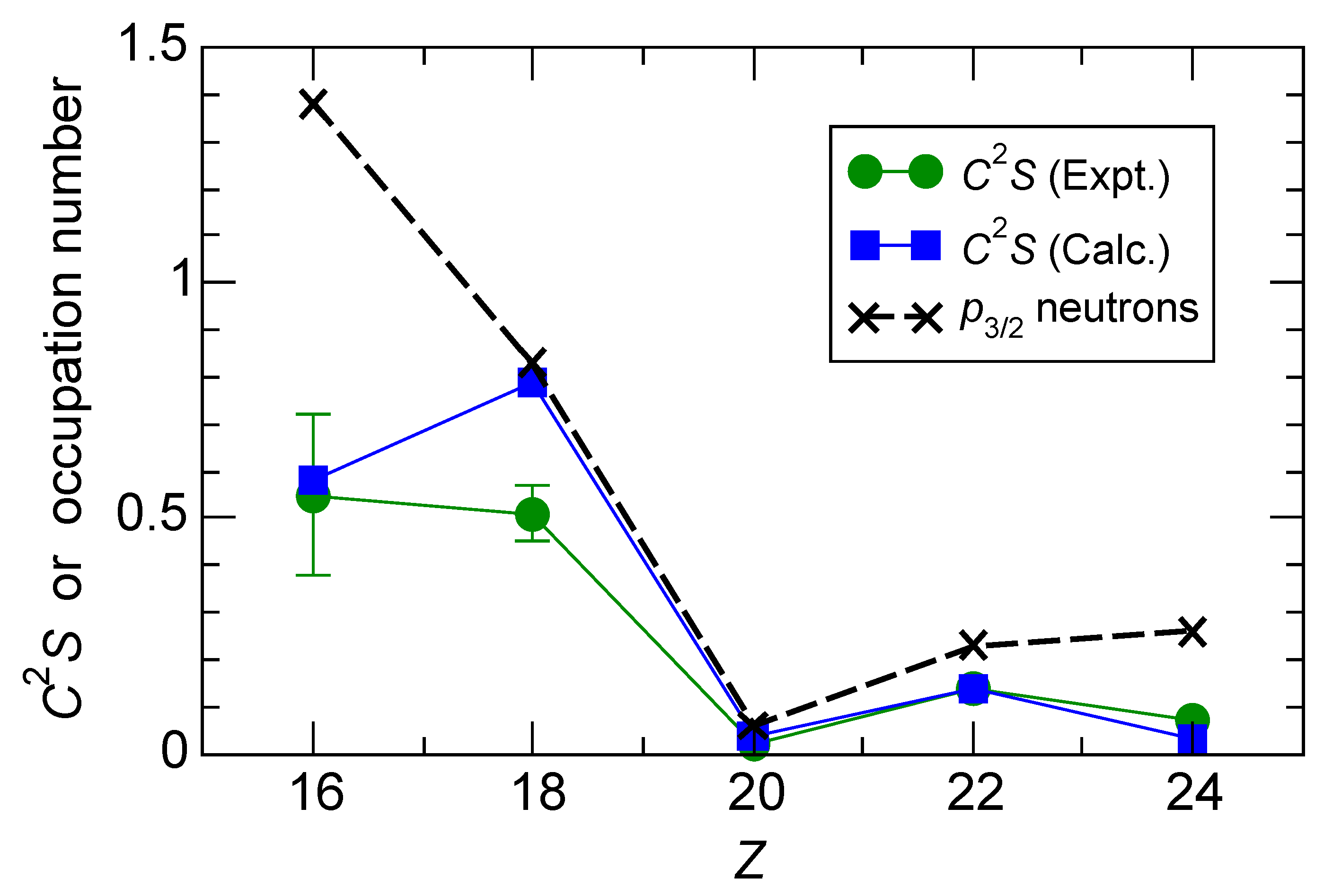
3.2.1. From to
3.2.2. From to
3.2.3. From to
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Otsuka, T.; Gade, A.; Sorlin, O.; Suzuki, T.; Utsuno, Y. Evolution of shell structure in exotic nuclei. Rev. Mod. Phys. 2020, 92, 015002. [Google Scholar] [CrossRef] [Green Version]
- Talmi, I.; Unna, I. Order of Levels in the Shell Model and Spin of Be11. Phys. Rev. Lett. 1960, 4, 469–470. [Google Scholar] [CrossRef]
- Bansal, R.; French, J. Even-parity-hole states in f7/2-shell nuclei. Phys. Lett. 1964, 11, 145–148. [Google Scholar] [CrossRef]
- Poves, A.; Zuker, A. Theoretical spectroscopy and the fp shell. Phys. Rep. 1981, 70, 235–314. [Google Scholar] [CrossRef]
- McGrory, J.B.; Wildenthal, B.H.; Halbert, E.C. Shell-Model Structure of 42−50Ca. Phys. Rev. C 1970, 2, 186–212. [Google Scholar] [CrossRef]
- Caurier, E.; Martínez-Pinedo, G.; Nowacki, F.; Poves, A.; Zuker, A.P. The shell model as a unified view of nuclear structure. Rev. Mod. Phys. 2005, 77, 427–488. [Google Scholar] [CrossRef] [Green Version]
- Storm, M.H.; Watt, A.; Whitehead, R.R. Crossing of single-particle energy levels resulting from neutron excess in the sd shell. J. Phys. Nucl. Phys. 1983, 9, L165–L168. [Google Scholar] [CrossRef]
- Warburton, E.K.; Becker, J.A.; Brown, B.A. Mass systematics for A = 29–44 nuclei: The deformed A ~32 region. Phys. Rev. C 1990, 41, 1147–1166. [Google Scholar] [CrossRef] [Green Version]
- Federman, P.; Pittel, S. Towards a unified microscopic description of nuclear deformation. Phys. Lett. B 1977, 69, 385–388. [Google Scholar] [CrossRef]
- Otsuka, T.; Fujimoto, R.; Utsuno, Y.; Brown, B.A.; Honma, M.; Mizusaki, T. Magic numbers in exotic nuclei and spin-isospin properties of the NN interaction. Phys. Rev. Lett. 2001, 87, 082502. [Google Scholar] [CrossRef] [Green Version]
- Utsuno, Y.; Otsuka, T.; Mizusaki, T.; Honma, M. Varying shell gap and deformation in N ∼ 20 unstable nuclei studied by the Monte Carlo shell model. Phys. Rev. C 1999, 60, 054315. [Google Scholar] [CrossRef]
- Otsuka, T.; Suzuki, T.; Fujimoto, R.; Grawe, H.; Akaishi, Y. Evolution of nuclear shells due to the tensor force. Phys. Rev. Lett. 2005, 95, 232502. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Otsuka, T.; Suzuki, T.; Honma, M.; Utsuno, Y.; Tsunoda, N.; Tsukiyama, K.; Hjorth-Jensen, M. Novel features of nuclear forces and shell evolution in exotic nuclei. Phys. Rev. Lett. 2010, 104, 012501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Burgunder, G.; Sorlin, O.; Nowacki, F.; Giron, S.; Hammache, F.; Moukaddam, M.; de Séréville, N.; Beaumel, D.; Càceres, L.; Clément, E.; et al. Experimental Study of the two-body spin-orbit force in nuclei. Phys. Rev. Lett. 2014, 112, 042502. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Smirnova, N.; Bally, B.; Heyde, K.; Nowacki, F.; Sieja, K. Shell evolution and nuclear forces. Phys. Lett. B 2010, 686, 109–113. [Google Scholar] [CrossRef] [Green Version]
- Tsunoda, N.; Otsuka, T.; Tsukiyama, K.; Hjorth-Jensen, M. Renormalization persistency of the tensor force in nuclei. Phys. Rev. C 2011, 84, 044322. [Google Scholar] [CrossRef]
- Yuan, C.; Suzuki, T.; Otsuka, T.; Xu, F.; Tsunoda, N. Shell-model study of boron, carbon, nitrogen, and oxygen isotopes with a monopole-based universal interaction. Phys. Rev. C 2012, 85, 064324. [Google Scholar] [CrossRef] [Green Version]
- Utsuno, Y.; Otsuka, T.; Brown, B.A.; Honma, M.; Mizusaki, T.; Shimizu, N. Shape transitions in exotic Si and S isotopes and tensor-force-driven Jahn-Teller effect. Phys. Rev. C 2012, 86, 051301. [Google Scholar] [CrossRef] [Green Version]
- Honma, M.; Otsuka, T.; Brown, B.A.; Mizusaki, T. Shell-model description of neutron-rich pf-shell nuclei with a new effective interaction GXPF1. Eur. Phys. J. Hadron. Nucl. 2005, 25, 499–502. [Google Scholar] [CrossRef]
- Richter, W.; Van Der Merwe, M.; Julies, R.; Brown, B. New effective interactions for the 0f1p shell. Nucl. Phys. A 1991, 523, 325–353. [Google Scholar] [CrossRef]
- Utsuno, Y.; Otsuka, T.; Shimizu, N.; Honma, M.; Mizusaki, T.; Tsunoda, Y.; Abe, T. Recent shell-model results for exotic nuclei. Epj Web Conf. 2014, 66, 02106. [Google Scholar] [CrossRef] [Green Version]
- Bertsch, G.; Borysowicz, J.; McManus, H.; Love, W. Interactions for inelastic scattering derived from realistic potentials. Nucl. Phys. A 1977, 284, 399–419. [Google Scholar] [CrossRef]
- Brown, B.A.; Wildenthal, B.H. Status of the nuclear shell model. Annu. Rev. Nucl. Part. Sci. 1988, 38, 29–66. [Google Scholar] [CrossRef]
- Honma, M.; Otsuka, T.; Brown, B.A.; Mizusaki, T. Effective interaction for pf-shell nuclei. Phys. Rev. C 2002, 65, 061301. [Google Scholar] [CrossRef] [Green Version]
- Utsuno, Y.; Shimizu, N.; Otsuka, T.; Yoshida, T.; Tsunoda, Y. Nature of Isomerism in Exotic Sulfur Isotopes. Phys. Rev. Lett. 2015, 114, 032501. [Google Scholar] [CrossRef] [Green Version]
- Shimizu, N.; Mizusaki, T.; Utsuno, Y.; Tsunoda, Y. Thick-restart block Lanczos method for large-scale shell-model calculations. Comput. Phys. Commun. 2019, 244, 372–384. [Google Scholar] [CrossRef] [Green Version]
- Cottle, P.D.; Kemper, K.W. Persistence of the N = 28 shell closure in neutron-rich nuclei. Phys. Rev. C 1998, 58, 3761–3762. [Google Scholar] [CrossRef]
- Sorlin, O.; Porquet, M.G. Nuclear magic numbers: New features far from stability. Prog. Part. Nucl. Phys. 2008, 61, 602–673. [Google Scholar] [CrossRef] [Green Version]
- Banks, S.; Spicer, B.; Shute, G.; Officer, V.; Wagner, G.; Dollhopf, W.; Qingli, L.; Glover, C.; Devins, D.; Friesel, D. The 48Ca(, 3He)47K reaction at 80 MeV. Nucl. Phys. A 1985, 437, 381–396. [Google Scholar] [CrossRef]
- Doll, P.; Wagner, G.; Knöpfle, K.; Mairle, G. The quasihole aspect of hole strength distributions in odd potassium and calcium isotopes. Nucl. Phys. A 1976, 263, 210–236. [Google Scholar] [CrossRef]
- Go, S.; Ideguchi, E.; Yokoyama, R.; Aoi, N.; Azaiez, F.; Furutaka, K.; Hatsukawa, Y.; Kimura, A.; Kisamori, K.; Kobayashi, M.; et al. High-spin states in 35S. Phys. Rev. C 2021, 103, 034327. [Google Scholar] [CrossRef]
- Papuga, J.; Bissell, M.L.; Kreim, K.; Blaum, K.; Brown, B.A.; De Rydt, M.; Garcia Ruiz, R.F.; Heylen, H.; Kowalska, M.; Neugart, R.; et al. Spins and Magnetic Moments of 49K and 51K: Establishing the 1/2+ and 3/2+ Level Ordering Beyond N = 28. Phys. Rev. Lett. 2013, 110, 172503. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sun, Y.; Obertelli, A.; Doornenbal, P.; Barbieri, C.; Chazono, Y.; Duguet, T.; Liu, H.; Navrátil, P.; Nowacki, F.; Ogata, K.; et al. Restoration of the natural E(1/) − E(3/) energy splitting in odd-K isotopes towards N = 40. Phys. Lett. B 2020, 802, 135215. [Google Scholar] [CrossRef]
- Grasso, M.; Ma, Z.Y.; Khan, E.; Margueron, J.; Giai, N.V. Evolution of the proton sd states in neutron-rich Ca isotopes. Phys. Rev. C 2007, 76, 044319. [Google Scholar] [CrossRef] [Green Version]
- Nakada, H.; Sugiura, K.; Margueron, J. Tensor-force effects on single-particle levels and proton bubble structure around the Z or N = 20 magic number. Phys. Rev. C 2013, 87, 067305. [Google Scholar] [CrossRef] [Green Version]
- Kirson, M. Spin-tensor decomposition of nuclear effective interactions. Phys. Lett. B 1973, 47, 110–114. [Google Scholar] [CrossRef]
- Piskoř, Š.; Franc, P.; Křemének, J.; Schäferlingová, W. Spectroscopic information on 35S and 37S from the (d, p) reaction. Nucl. Phys. A 1984, 414, 219–239. [Google Scholar] [CrossRef]
- Uozumi, Y.; Kikuzawa, N.; Sakae, T.; Matoba, M.; Kinoshita, K.; Sajima, S.; Ijiri, H.; Koori, N.; Nakano, M.; Maki, T. Shell-model study of 40Ca with the 56-MeV (, p) reaction. Phys. Rev. C 1994, 50, 263–274. [Google Scholar] [CrossRef]
- Evaluated Nuclear Structure Data File (ENSDF). Available online: http://www.nndc.bnl.gov/ensdf/ (accessed on 1 January 2022).
- Lu, F.; Lee, J.; Tsang, M.B.; Bazin, D.; Coupland, D.; Henzl, V.; Henzlova, D.; Kilburn, M.; Lynch, W.G.; Rogers, A.M.; et al. Neutron-hole states in 45Ar from 1H(46Ar, d) 45Ar reactions. Phys. Rev. C 2013, 88, 017604. [Google Scholar] [CrossRef] [Green Version]
- Momiyama, S.; Wimmer, K.; Bazin, D.; Belarge, J.; Bender, P.; Elman, B.; Gade, A.; Kemper, K.W.; Kitamura, N.; Longfellow, B.; et al. Shell structure of 43S and collapse of the N = 28 shell closure. Phys. Rev. C 2020, 102, 034325. [Google Scholar] [CrossRef]
- Chen, S.; Lee, J.; Doornenbal, P.; Obertelli, A.; Barbieri, C.; Chazono, Y.; Navrátil, P.; Ogata, K.; Otsuka, T.; Raimondi, F.; et al. Quasifree neutron knockout from 54Ca corroborates arising N = 34 neutron magic number. Phys. Rev. Lett. 2019, 123, 142501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Steppenbeck, D.; Takeuchi, S.; Aoi, N.; Doornenbal, P.; Matsushita, M.; Wang, H.; Baba, H.; Fukuda, N.; Go, S.; Honma, M.; et al. Evidence for a new nuclear ‘magic number’ from the level structure of 54Ca. Nature 2013, 502, 207–210. [Google Scholar] [CrossRef] [PubMed]
- Utsuno, Y.; Otsuka, T.; Tsunoda, Y.; Shimizu, N.; Honma, M.; Togashi, T.; Mizusaki, T. Recent advances in shell evolution with shell-model calculations. JPS Conf. Proc. 2015, 6, 010007. [Google Scholar] [CrossRef] [Green Version]
- Steppenbeck, D.; Takeuchi, S.; Aoi, N.; Doornenbal, P.; Matsushita, M.; Wang, H.; Utsuno, Y.; Baba, H.; Go, S.; Lee, J.; et al. Low-Lying Structure of 50Ar and the N = 32 Subshell Closure. Phys. Rev. Lett. 2015, 114, 252501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Liu, H.N.; Obertelli, A.; Doornenbal, P.; Bertulani, C.A.; Hagen, G.; Holt, J.D.; Jansen, G.R.; Morris, T.D.; Schwenk, A.; Stroberg, R.; et al. How robust is the N = 34 subshell closure? First spectroscopy of 52Ar. Phys. Rev. Lett. 2019, 122, 072502. [Google Scholar] [CrossRef] [Green Version]
- Rotaru, F.; Negoita, F.; Grévy, S.; Mrazek, J.; Lukyanov, S.; Nowacki, F.; Poves, A.; Sorlin, O.; Borcea, C.; Borcea, R.; et al. Unveiling the Intruder deformed state in 34Si. Phys. Rev. Lett. 2012, 109, 092503. [Google Scholar] [CrossRef] [Green Version]
- Mutschler, A.; Lemasson, A.; Sorlin, O.; Bazin, D.; Borcea, C.; Borcea, R.; Dombrádi, Z.; Ebran, J.P.; Gade, A.; Iwasaki, H.; et al. A proton density bubble in the doubly magic 34Si nucleus. Nat. Phys. 2017, 13, 152–156. [Google Scholar] [CrossRef] [Green Version]
- Kay, B.P.; Hoffman, C.R.; Macchiavelli, A.O. Effect of weak binding on the apparent spin-orbit splitting in nuclei. Phys. Rev. Lett. 2017, 119, 182502. [Google Scholar] [CrossRef] [Green Version]
- Brown, S.M.; Catford, W.N.; Thomas, J.S.; Fernández-Domínguez, B.; Orr, N.A.; Labiche, M.; Rejmund, M.; Achouri, N.L.; Al Falou, H.; Ashwood, N.I.; et al. Low-lying neutron f p-shell intruder states in 27Ne. Phys. Rev. C 2012, 85, 011302. [Google Scholar] [CrossRef] [Green Version]
- Matta, A.; Catford, W.N.; Orr, N.A.; Henderson, J.; Ruotsalainen, P.; Hackman, G.; Garnsworthy, A.B.; Delaunay, F.; Wilkinson, R.; Lotay, G.; et al. Shell evolution approaching the N = 20 island of inversion: Structure of 29Mg. Phys. Rev. C 2019, 99, 044320. [Google Scholar] [CrossRef]
- Piskoř, Š.; Novák, J.; Šimečková, E.; Cejpek, J.; Kroha, V.; Dobeš, J.; Navrátil, P. A study of the 30Si(d, p)31Si reaction. Nucl. Phys. A 2000, 662, 112–124. [Google Scholar] [CrossRef]



| C | T | LS | Total | Spin Direction | Label | C + T | ||
|---|---|---|---|---|---|---|---|---|
| - | 0 | antiparallel | − − | |||||
| - | 0 | antiparallel | − − | |||||
| - | 0 | 0 | no direction | −0 | ||||
| - | 0 | 0 | no direction | −0 | ||||
| - | 0 | parallel | − + | |||||
| - | 0 | parallel | − + | |||||
| - | 1 | antiparallel | +(−) | |||||
| - | 1 | antiparallel | +(−) | |||||
| - | 0 | 1 | no direction | |||||
| - | 0 | 1 | no direction | |||||
| - | 1 | parallel | ||||||
| - | 1 | parallel |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Utsuno, Y. Probing Different Characteristics of Shell Evolution Driven by Central, Spin-Orbit, and Tensor Forces. Physics 2022, 4, 185-201. https://doi.org/10.3390/physics4010014
Utsuno Y. Probing Different Characteristics of Shell Evolution Driven by Central, Spin-Orbit, and Tensor Forces. Physics. 2022; 4(1):185-201. https://doi.org/10.3390/physics4010014
Chicago/Turabian StyleUtsuno, Yutaka. 2022. "Probing Different Characteristics of Shell Evolution Driven by Central, Spin-Orbit, and Tensor Forces" Physics 4, no. 1: 185-201. https://doi.org/10.3390/physics4010014
APA StyleUtsuno, Y. (2022). Probing Different Characteristics of Shell Evolution Driven by Central, Spin-Orbit, and Tensor Forces. Physics, 4(1), 185-201. https://doi.org/10.3390/physics4010014





