1. Introduction
The generation and propagation of action potentials in neurons are generally described by an electric model proposed by Hodgkin and Huxley [
1] that has been very useful for explaining the generation of electric polarization confined close to the lipid membrane of a neuron via gated injection of excess charges through ion channels. To explain the slowdown of the polarization pulse signals along the neuron from close to the velocity of light of c = 3 × 10
8 m/s in usual electromagnetic wave guides to measured propagation velocities of action potentials, ranging between 1 and 100 m/s, it employs a rather dissipative RC-network of resistors R and capacitors C with RC time constants in the range of milliseconds. It ignores the mechanical motion of the confining elastic membrane of a neuron and the presence of dipolar screening of ionic charges through the omnipresent dipolar H
2O molecules that tend to form a hydration shell around any local ionic charge. In contrast, there have been several mechanical models proposed starting with work by Helmholtz in 1850 [
2], followed by Wilke (1912) [
3], Tasaki et al. (1949) [
4], Heimburg and Jackson (2005) [
5], and Engelbrecht et al. [
6]. All of these are discussed in conjunction with the Hodgkin–Huxley model in extensive recent reviews by Peyrard [
7] and Holland et al. [
8].
I became aware of the different mechanics-based approaches in 2018 through a talk by Matthias Schneider presented at the NIM conference “The Future of Nanoscience”, organized by the excellence initiative “Nanosystems Initiative Munich (NIM)” in September 2018 based on previous publications, e.g., [
9,
10] and an article by Douglas Fox on “The Brain Reimagined” published in April 2018 [
11]. Both raised my curiosity and I started studying the relevant literature to better understand these mechanical concepts without getting fully convinced by the proposed models. With my own participation in collaborative experiments on ballistic transport of excitonic dipoles via surface acoustic waves (SAW) [
12] and studies of acoustoelectric generated sound pulses propagating in high-quality nanostring resonators [
13,
14,
15] I started to develop my own approach to a soliton-like propagation [
16] of dielectric polarization [
17]. Then, already retired for several years and without any student support and suitable laboratory, I got engaged in conceptional work combined with simple experimental studies to develop the model based on acoustoelectric generation and propagation of action potentials. I learned that individual soliton-like acoustic pulses can transport highly complex information content about their generating source and their propagation path and carry nearly undamped and phase-coherent energetic acoustoelectric wave packets that can be transformed into presumably phase-coherent electrochemical processes at synaptic transfer positions between neurons. Here, the term acoustoelectric should be understood rather generally, namely that propagating anisotropic deformations can cause propagating electric potentials with both longitudinal and transverse components of essentially a piezoelectric nature as in semiconducting [
12] or insulating solid materials, e.g., [
13,
14,
15] and, relying on ionic charge-density-dependent interactions in incompressible liquids, also mechanically caused transient changes of conductance involving partially diffusive charge propagation. Electrolytes near mechanically deformable interfaces can support both but, as discussed in detail below, the coherent propagation of interfacial polarization via rotation of water dipoles in neurons is likely to dominate the incoherent transport of ionic charges. Potentially, it, thus, can exchange information between our feet and our brain to perform analog computation and data storage with phase-coherent soliton-like hydro-acoustoelectric wave packets, somewhat similar to holography with light waves. At least, the concept of coherent information exchange via acoustoelectric waves that act as a conveyor belt for charge-neutral polarization signals, e.g., [
12], is similar to the speech exchange in a tin-can telephone and is able to propagate complex information through a tensional prestressed, quasi-one-dimensional tubular system, filled with an incompressible water-based electrolyte.
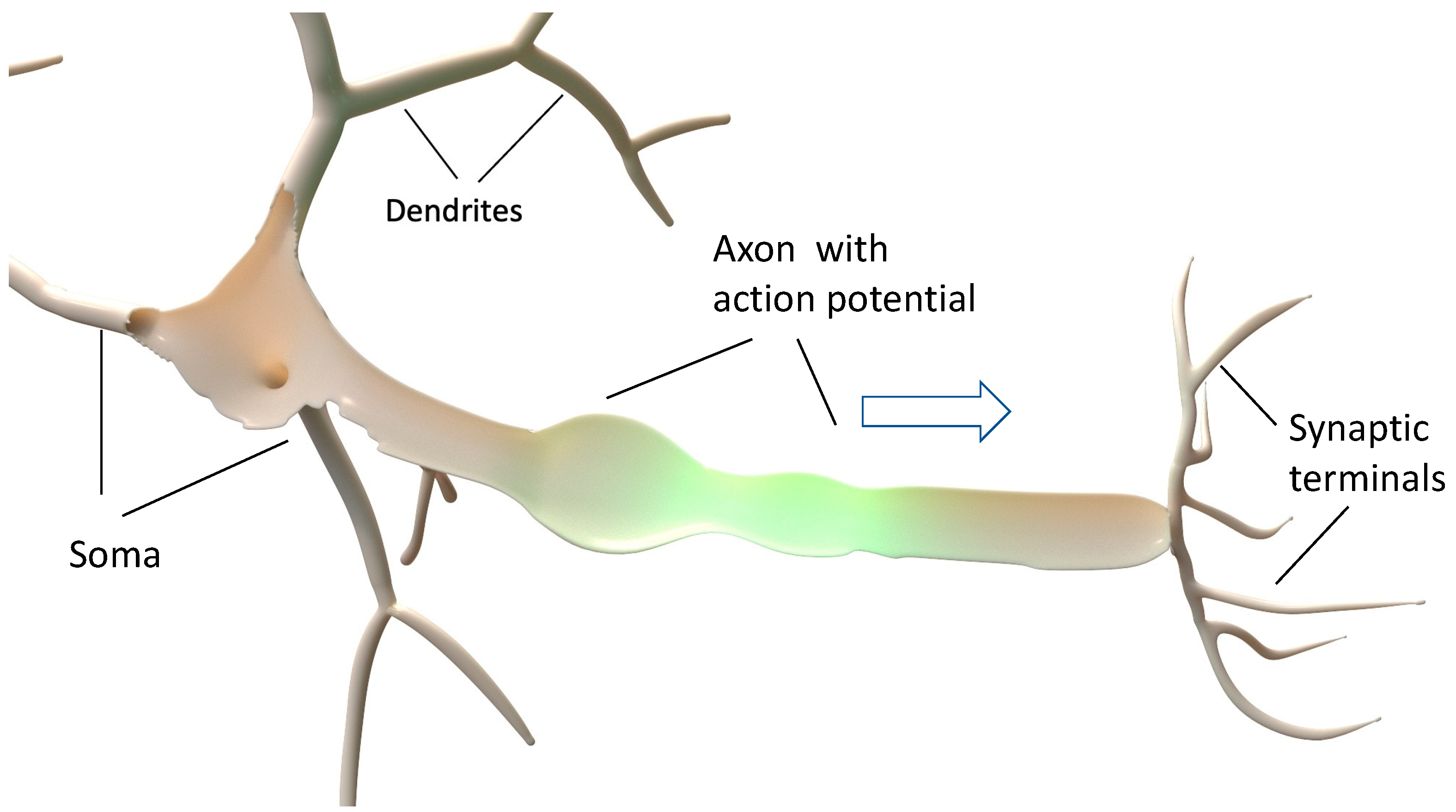
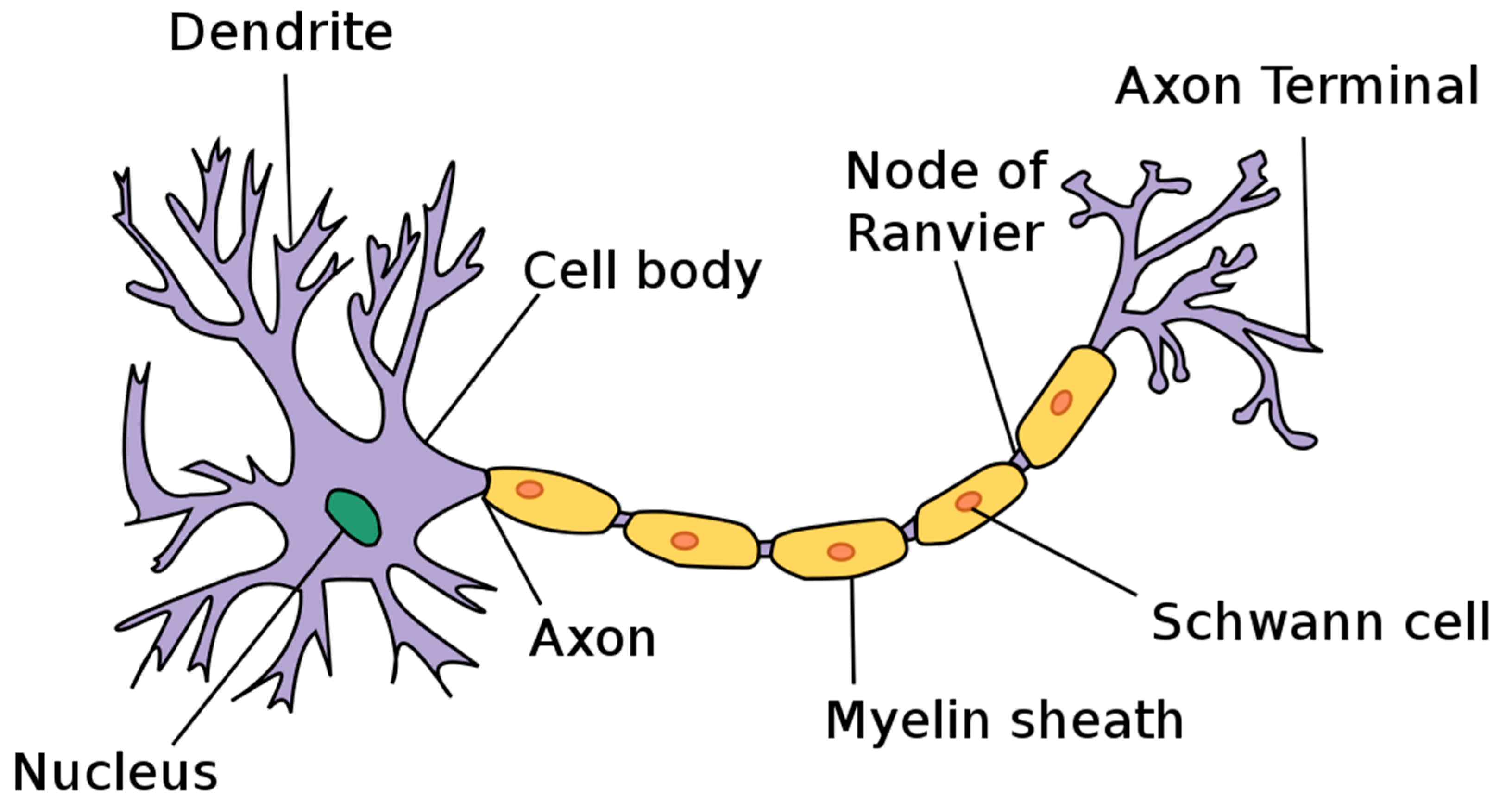
Figure 1 displays schematically the structure of a neuron, consisting of the soma as the generator of acoustoelectric action potentials, the axon as a waveguide for the propagation of soliton-like action potentials as indicated by the yellow center and the synaptic terminals as transducers which coherently transduce action potentials via chemical and/or electromechanical processes to other neurons or cells acting as sensors. The acoustoelectric approach based on capillary interface waves directly explains the measured propagation velocities of action potentials and also gives reasonable estimates of the signal strength it generates and transports. It can explain the design of the soma and its dendritic arms filled with individual ion channels to generate specific pulse shapes that carry information about their sender. It can also explain the action of myelination [
18] to modulate propagation velocities of action potentials and act as coupled acoustoelectric resonators that can focus energy onto the nodes of Ranvier to activate ion channels through force gradients. Possibly, it can explain why nature has chosen spiral molecules in the myelinated walls to control wave chirality and to act as acoustoelectric resonators filtering the wavelength and chirality of overtones. It also provides nonlinear enhancements of the tangential strain of the axon membrane and electric fields near the nodes of Ranvier to enable the electric-field-controlled opening of nearby ion channels. Possibly, the underlying physics can also explain long-established concepts in medical treatment such as acupuncture and osteopathic treatment since its main ingredients are based on water-based oscillating electrolytes in flexible tubing combined with electromechanical or chemical actuation.
Of course, further experimental and theoretical work is needed to quantitatively confirm the concept introduced here. Experimentally, one needs to verify the phase-coherent and chiral propagation of action potentials as discussed below by optical monitoring the spatial and temporal behavior of propagating action potentials with high resolution, e.g., by employing fluorescent local optical emitters [
18], interferometric detection [
19,
20], magnetooptic sensors [
21], and electric-field-dependent optical phenomena such as the Franz–Keldysh effect [
22]. Spectral analysis using optical, mechanical, and electromagnetic methods can also enable a wide-band Fourier-transform analysis of the frequency components of the shapes of individual action potentials without smoothing the pulses by external RC-time constants [
23]. Optics can confirm the mechanical and electrical signatures of interfacial waves and detect the one-dimensional phase-coherent acoustoelectric propagation of confined electrical polarization by interface acoustic waves [
12]. Theoretical work with finite-element calculations should help to quantitatively model the here-discussed propagation of a rather long wavelength and low amplitude interfacial waves with reasonable boundary conditions. A Monte Carlo-type calculation could improve insight into the dynamics of propagating polarization waves at the molecular scale.
5. Approaching the Mechanism of the Propagation of Action Potentials in Neurons
In principle, I want to demonstrate that a neuron can be considered as a complex acoustoelectric resonator system in which the local injection of ions triggers a quasi-ballistically propagating KdV-soliton-like action potential pulse that is phase-coherently transported by an axially propagating acoustoelectric interface excitation with an essentially constant group velocity [
16]. Since a neuron, as displaced in
Figure 1, consists of mostly tubular components, let us consider an axon-like tube, as displayed in
Figure 3, of length L and diameter D with L >> D = 2R and
λc >> D confined by a thin elastic bilipid membrane of thickness d ≈ 7 nm and closed at both ends with the soma and the synaptic terminal, respectively. The axon is filled with a water-based incompressible electrolyte and experiences a tensile prestress by an internal hydrostatic overpressure of typically 10
4 Pa, predominantly caused by hypertonic osmotic forces. Note that the length scales in
Figure 3 are drastically distorted as for the example axon used here; we have L~1 m,
λc = 3 mm, D = 10 μm, and d ≈ 7 nm, t ≈ 1 nm.
Without electric bias across the neuron membrane, one would expect the polarization of water molecules near the lipid membrane to arrange antiferroelectrically in a predominantly laminar fashion to minimize their orientational polarization P
⊥ oriented perpendicular to the membrane at any given temperature. Uniformly charging the membrane capacitor by providing excess ionic charges at the membrane interior through a bias voltage will induce a finite polarization of the membrane as well of the adjacent water molecules that form a hydration shell around the ions, at least partially screening the Coulomb potential of the locally bound ionic excess charges. With the outside potential of the neuron at potential
V = 0, neurons at rest exhibit a typical inside bias voltage of V
0 ≈ −70 mV. That generates a bare electric field of strength E
0 ≈ 10
7 V/m. The resulting excess ionic charges attracted to the inside of the membrane form a dipolar polarization oriented perpendicular to the membrane–electrolyte interface opposing the bare electric field and are screened by the dipolar hydration shell toward the charge-neutral cylindrical bulk electrolyte within a Debye length of typically
t ≈ 1 nm. The resulting voltage-dependent force acts perpendicular to the membrane and increases the tube radius at the rest voltage V
0 by Δ
R as shown by the colored sections on the right-hand side of
Figure 3. If one would now apply in a quasi-stationary picture an inside bias oscillating in proportion to V
0 cosz one would get a spatially oscillating membrane with amplitude Δ
R~Δ
F⊥, as shown in
Figure 3.
From derivative δ
W/δ
R of the energy
W of the cylindrical capacitor radius
R and length L with
W= ½
CV2 = (
πRLε0/d)
V2 and the fact that the membrane thickness d does not noticeably change with voltage
V, the dipolar force per unit length that increases the radius of the tube is:
With the above values of
V0,
d, and
one obtains with
F⊥/
L =
κΔ
R/
R an increase in tube radius of Δ
R ≈ 2 nm as roughly indicated in
Figure 3. This value compares reasonably well with typically observed radial deformations of axons caused by a transient action potential [
5,
19]. Correspondingly, one can estimate the shear angle
Θ ≈ RΦ/(
λc/4) with
Φ = 90° by which the surface normal of the capillary wave is maximally tilted with respect to the normal of the membrane–electrolyte interface at rest. With the numbers above, one estimates
Θ ≈ 1 degree. This seems too small to expect a sufficiently large axial polarization but note that the shear angle determines only the axis around which the polarization vector rotates. This will be discussed in detail below.
The left portion of
Figure 3 tries to illustrate what happens when we use the injection of positive ions through the ion channel indicated in the center of the tube. Here, the dynamic deformation of the capacitor membrane is no longer the result of an imaginary quasistatic voltage but is induced as a traveling wave propagating from the stationary position of each ion channel toward both the left-hand side and the right-hand side with a wavelength
λc. The total length of this wave packet is determined by the rate of injection of the positively charged ions, which defines the number of injected charges per unit length with respect to the number of polarizing charges per
λc that are defined by the membrane voltage V
0 at rest.
In the following, it is assumed that the charge density along the perimeter of any perpendicular cut through the axon tube is uniform whereas it changes axially according to the local radial and tangential electric-field components. This seems appropriate since the electric field changes on a length scale
λc/D and, thus, is essentially independent in strength for a radial cut through the tubular axon. In addition, for a radially uniform membrane, the internal overpressure of the membrane assures that the cross section remains circular since the contained liquid is essentially incompressible. Of course, the lipid membrane will be axially stretched or compressed with respect to the rest position according to the local changes in axial and radial forces. Since the linear momentum of the injected ions along the radial direction is much smaller than the local radial and axial momentum created by the relocation of water dipoles between the axial minima and maxima driven by the axial and radial electrical field gradients, one can assume that the relatively slowly propagating potential spiral will be filled with ions with the goal to depolarize the membrane rather uniformly in both the radial and axial directions. Therefore, one can expect that the axial distribution of the injected charge will reflect the joint forces of the local electric field and the axial polarization gradients and will concentrate depolarizing charges more around the region of the highest absolute value of the electric field. It, thus, seems appropriate to approximate the axial variation of the perpendicularly acting force Δ
F⊥ generated by the local electric field E
⊥ acting on the membrane and propagating with constant velocity
cc and a wavelength
λE =
2λC corresponding wave vector
kE =
½kc into one axial direction of an axon by:
The capacitance per length
λC/2 is
≈ 1.2 × 10
−10 As/V and the corresponding total charge needed to depolarize this capacitance is Q
T = C(
when the effective bias voltage oscillates sinusoidally between
V =
V0 and
V = 0 V. Providing this charge by singly charged ions requires N = C
V0/(2e) ≈ 2 × 10
7 ions to achieve complete depolarization at the minimum of Δ
R. The corresponding areal ion density N/πR
λC yields an average ionic distance before depolarization of Δi ≈ 50 nm. Since action potentials measured on single ion channels exhibit depolarizing currents pulses of typically 3 pA in 0.5 ms [
30] and thus deliver roughly N = 2 × 10
4 singly charged ions per depolarizing pulse, one needs, depending on axon radius, the depolarizing charge from up to 1000 simultaneously activated ion channels to generate an individual action potential pulse that fully depolarizes a
λC/2-section of an axon while propagating with the constant group velocity. The position-dependent propagation delay between the charge packets injected at each participating ion channel thus determines the shape of the resulting action potential and provides a status report of the neuron and its environment typically lasting several milliseconds. Changes in the status are defined and transmitted by the generation of sequential action potential pulses with delay intervals of typically over 100 milliseconds. With 1/8
CV02, one obtains a local energy reduction of such a depolarizing dipolar packet of Δ
W~10
−13 J. Since this is a collectively propagating mode similar to the acoustoelectric pulses discussed in
Section 3, disorder and damping are most likely very small. All of this seems to be quite similar to what was observed in the propagation of transverse mode pulses as studied in suspended nanostrings, discussed in
Section 2 and [
15].
So far, we have not considered that an unpolarized breathing-mode wave propagating axially with a constant velocity can be described by two circular polarized waves with opposing chirality. If generated with a current pulse through an ion channel, one can expect to generate two polarization pulses of finite length propagating along the axis in opposite directions. It is illuminating to recognize that an individual right-hand circular polarized (RHCP-) wave packet confined to a tubular skin of water propagates from the ion channel to the left as indicated on the upper channel and in the section cut on the left of
Figure 3a and an (LHCP-) wave propagates to the right. The superposition of both chiral modes in one propagation direction reflects a charge-neutral donut-like polarization ring surfing on the axially propagating potential gradient of the capillary wave. Basically, the motion of such a chiral wave packet is in analogy to a surfer surfing on the gradient potential of a spiral wave with wavelength
λC, as indicated by the axial RHCP-spiral on the left section of
Figure 3a. However, in the absence of gravity, the surfer would be pressed to the propagating spiral wave by centrifugal forces only. Considering the pulsed dipolar polarization excitation as charge-neutral quasiparticles surfing on a capillary breathing mode consisting of two oppositely rotating dipolar chiral modes, I named them dipolitons.
Avoiding mode mixing, such propagating polarization rings are likely to be topologically protected, thus propagating quasi-ballistically with little damping. This is indicated for the RHCP-mode propagation on the left of
Figure 3. Note that for each chiral component, the total perpendicular polarization of the ring propagates on a spiral around the radius R, as indicated in the 3D RHCP spiral inserted on the left into
Figure 3. Note that the inside of the membrane appears to be twisted by rotation with period
λC for a given chirality. The superposition of two opposing chiral modes propagating in the same axial direction results in a propagating breathing mode and by locally opposing radial motion removes the twist of the membrane and from a stationary observer yields an oscillating polarization vector that rotates around the tilted axis normal to the membrane interface, as described above. The periodic stretching of the membrane with period T =
λC/c
c increases the amplitude of the axial and radial oscillations of the screening water dipoles, describing their motion around the axis tilted by Θ with respect to the normal of the axial propagation direction. Once such a propagating pulse is formed by the reorientation of water molecules by tangential and rotational movements; one can assume that it propagates with its impressed electrical polarization rather undamped as long as the flow within a wavelength
section of the capillary wave is laminar. Typical values of the geometric dimensions of an axon and the propagation velocities of one have Reynolds numbers of order 100 and can assume laminar flow.
Since the water in the electrolyte is incompressible, the screening dipoles in the high-field Debye skin will be driven by an angular momentum initiated by the depolarizing pulse of ions. For symmetry reasons, the linear momentum
p, characterizing the axial propagation of the capillary wave pulse, is accompanied by two angular momenta
L = ½
Rxp of opposing chirality. Confining both the RHCP-wave packet and LHCP-wave packet to a narrow ring in the radial plane will generate a torque of T = 1/2 d
L/dt along the +z and −z direction since motion along z is essentially confined to axial transport. Within the wavelength of
λE = 2
λc, the polarization will be opposing to the local electric field so that for a given chirality the polarization vector will move on a spiral of spatial period
λE as indicated for the RHCP in the 3D picture inserted into
Figure 3 carried by the cylindrical potential gradients at the same propagation velocity as the capillary wave. In that sense, the tilt of the polarization vector with respect to the axis of the axon will depend on the initiating impulse by the injected ions and, if that pulse transports a charge which corresponds to the initial depolarizing charge of a single ion channel, it will generate a single force impulse of length
λc/4. From this point of view, the polarization pulse will propagate similarly to the pulse initiated by rotating a single pendulum by 180 degrees to initiate a pendulum soliton, as illustrated in Video 3 of
Appendix A. Alternatively, one can describe the rotation of the polarization wave as propagating axially along the neuron with velocity c
c and oscillating axially around the tilt axis in a coordinate system rotating with angular frequency Ω as an H
2O-based dipolar polarization P. Note that the membrane polarization vector will oscillate with a smaller amplitude opposing the shear force of the locally stretched membrane whereas the H
2O- based polarization oscillation moving with respect to the inner interface of the membrane will experience an additional Coriolis-like shear force which increases the forward amplitude and contributes to the solitonic character of the wave pulse.
The coupling between the electric polarization and the tilt vector is determined in part by the anisotropic dielectric susceptibility
χ of confined water. As studied in nanometer narrow channels fabricated out of graphene-based heterostructures, the anisotropy of
χ has been studied by the Manchester group around Andre Geim by spatially resolved capacitive studies with a scanning dielectric microscope [
31]. They revealed the anisotropy of the dielectric tensor yielding
χ// ≈ 70. This triggered the theoretically confirming work in the group around Roland Netz in Berlin that extended the studies also to bilayer interfaces confirming the experimental results [
32]. With these, it is justified to use here values for the appropriate value for
χ//~70 and increase the in-plane axial polarization P
‖ of the screening water dipoles in the Debye layer to a value comparable to P
⊥, thus, achieving a dynamic angle in the range around 45 degrees. Note this polarization results predominantly from the orientation of water dipoles which are able to follow the driving electric field up to radio frequencies without appreciable dissipation. Subsequent studies of the Manchester group also showed that sodium ions in a narrow channel adhere to the wall whereas protons continue to be mobile down to atomic-length scales [
33], thus, justifying the here assumed little damping of oscillating water dipoles in their motion within the narrow high field region near the membrane–water interface.
Whereas the field-effect confined water is essentially incompressible, the membrane also carrying the excess ionic charge is highly compressible, thus, again justifying the relative motion of the water polarization with respect to the one of the membrane. For a more quantitative description of the relation between the amplitude and shape of polarization components parallel and perpendicular to the propagation direction, self-consistent numerical finite-element calculations of the charge-neutral polarization current are desirable. However, it should be clear that the polarization current is not carried by long-range mass and charge transport but propagates essentially charge neutral similar to waves through a chain of coupled pendula, as shown in Video 3 of
Appendix A or La Ola waves in a fully packed soccer stadium.
So far, we have discussed the effect of a single charge package injected through an ion channel into any tubular part of a neuron, resulting in the generation of two oppositely propagating de- and repolarizing pulses as part of a hydrodynamic breathing-mode capillary wave propagating quasi-one-dimensionally in a tube filled with an incompressible electrolyte and confined by a thin flexible membrane. Now we focus on the soma and its dendrites as a generator of an action potential. As sketched in
Figure 1, the soma is a compact resonator system reminiscent of bagpipes with several dendrites and the central soma containing on the order of 30–1000 simultaneously activated ion channels and opening to a single axon waveguide. Since the soma usually has dimensions that are smaller than a typical wavelength
λc of a capillary wave in the axon, we can consider the individual polarization pulses generated within less than 1 ms to superimpose into a collective pulse that is broadened and distorted by phase delays related to the time delay of the propagation of pulse waves from the individual ion channels in the dendrites and the central Helmholtz-like resonator of the soma. In addition, polarization pulses generated in the direction opposite to the transition from the soma into the axon will be at least partially reflected and enter the axon with an additional delay [
34]. Note that the elastic reflection of a chiral wave reverses its chirality and, thus, constructively interferes with the chiral wave component directly propagating toward the axon. Assuming that all generated pulses propagate phase-preserving with the same group velocity, one obtains at the entrance of the axon a pulses wave with a shape as illustrated in
Figure 4 that is characteristic of most action potentials. As discussed above, the depolarization of an axon requires typically 10
7 ions/ms and, with 1000 ion channels contributing with 10.000 Na
+ ions, each one can expect the rising flank of the action potential to last about 1 ms. During this time, the capillary wave propagates for typically 3 mm, significantly more than the dimensions of the soma and its dendrites. Once neutralized and repolarized by an exchange of positive- and negative-charged excess ions in the electrolyte it returns with some overshoot to the rest state and, thus, propagates with the originally imposed axial polarization through the axon.
All ion channels contained in the dendrite and the soma cause wave packets in opposing directions, as discussed in
Figure 3. The fact that the soma opens toward the axon breaks this axial symmetry and will force wave packets that originally propagate from the injecting ion channel in the direction away from the transition between the soma and the axon to be reflected at the closed end of each dendrite. This will change their chirality and direction to increase the net momentum towards the axon. These cause propagation delays that extend the pulse shape toward its end. Such reflections are illustrated in
Figure A1 and the associated Video 4 of
Appendix A where the soliton-like propagation through a mechanical spiral with reflecting ends is experimentally visualized. Shorter delay are caused by the fact that ion channels in the soma are placed at different positions along a length ≪
λc..
Hence, simultaneously started pulses moving towards the axon will experience a shorter distance-related delay without drastically changing the shape of the action potential. The steep rise at the beginning of the action potential and the extended back end can explain the shape change of the observed action potential [
35] but its causes are rather likely propagation delays of phase-preserving charge-neutral polarization pulses and probably are not broadened significantly by damping through diffusive behavior of excess charges or viscous forces. Since the action potential contains strong axial potential gradients, it can synchronously activate ion channels on its path if an excess charge deficit occurs and fills up to the original potential level.
In my understanding, the pulse shape of action potentials should not change significantly with the distance from the soma because it contains the status message that the soma wants to distribute. Here, spatially and temporally resolved techniques with optical [
18,
19,
20,
21,
22], magnetic [
23], or capacitive probes [
31] will help in studying the dynamics of propagating action potentials. Suitably designed to prevent the distortion of pulse shapes by externally imposed instrumental time constants, they could correlate the signal shape with the positions of the emitting ion channels and will be very helpful to obtain a more quantitative understanding. Correlation studies between locally measured gradient fields and the start of ion injection could profit from Franz–Keldysh effect tuning [
22] of fluorescent emitters inserted in the membrane under triggered excitation.
The model presented so far also helps to understand why nature has chosen myelination to improve the axial transport of action potentials, as illustrated in
Figure 5. Myelination has a twofold effect [
17,
36,
37,
38]. One is that the myelinated sections have stiffer walls, increasing the propagation velocity, and possess no ion channels in the center region. As acoustic resonators, the myelinated section can increase local fields and concentrate axial field gradients near their end close to the nodes of Ranvier. Ion channels located there can utilize the increased gradient fields to lower the activation threshold for ion injection and coherently preserve the ionic density by synchronously filling up the excess ion reservoir. In addition, the chiral network of the myelinated membranes may support filtering by preferring one chirality for sending signals in a preferred direction. This would allow the use of chirality as a tool for separating forward transport from the soma along the axon from backward transport towards the soma. Allowing efficient two-way communication opposing solitonic signals will not destroy each other by destructive interference, as illustrated in Video 2 of
Appendix A. Myelination may also be utilized for frequency-component filtering and for the reduction of noise caused by the irregular motion of the axon in its environment.
Finally, myelination, combined with chiral propagation, has the potential to avoid reflection from the synaptic terminations since, similar to phase matching between electromagnetic waveguides and cavities, nature could use it to concentrate the energy of action potentials into the synaptic terminations to activate other coherently transducing processes such as chemical transduction or acoustoelectric isolators or signal splitters. In a more general context, the importance of propagation of information in biological systems via coherent waves beyond quasistatic reaction–diffusion concepts have been already addressed by Robert Laughlin nearly a decade ago [
39] but seems to be still neglected in conventional approaches to biological functions. Here, further experimental studies with an improved temporal and spatial resolution of propagating action potentials based on local electric, magnetic, and optical probes and correlated detection methods would, in conjunction with accompanying theoretical investigations, certainly improve our understanding of the character and sound of action potentials and more generally of information processing in living systems.
6. Some Conclusions
The proposed model rather simply explains the signal generation of electric polarization components through local charge injection through individual ion channels that, in turn, causes rotation of water molecules in the hydration shell around the injected excess ions. This local motion of water molecules initiates capillary wave packets at each participating ion channel that superimpose, coming from different parts of the soma with its dendrites, with different small time delays of order or smaller than milliseconds to generate an individual characteristic action potential at the entrance to the axon with temporal shapes that function analogously to the click sounds in marine animal communication and contain a characteristic spectrum of higher frequency components that all propagate with the same group velocity, essentially defined by geometric and elastic parameters, as defined by Equation (1). The electromechanical coupling defining the propagating axial polarization is dominated by the strong anisotropy of the dielectric function of the nanometer-thin water skin in the high-electric-field Debye regime adjacent to the interior of the confining lipid membrane which is responsible for the relatively strong axial polarization caused by a relatively small tilt angle of the membrane interface. Both the effect of the elasticity of the lipid membrane and the anisotropic response of the water dipoles surrounding the excess ion charges are not addressed by the Hodgkin–Huxley model and are not known to be reported elsewhere by the author.
Another important feature is the fact that capillary waves in a tensionally prestressed tube are likely to propagate soliton-like with little dissipation in analogy to acoustoelectric sound waves in prestressed insulating nanostrings, as discussed above. This reflects the concept of tensional integrity, abbreviated to tensegrity and introduced in the 1960s by architect Buckminster Fuller and now, under the name of biotensegrity, getting increasingly popular in the biophysics of cells and bodies. By tensional prestress and imprinting information into resilient temporal shapes, action potentials can be preserved in low-loss propagation thus efficiently transporting analog sound-like information by individual shape-preserving acoustoelectric capillary wave pulses. Frequency [
23] and shape [
35] analysis in the recent literature support this concept but do not provide the acoustoelectric description discussed here. This concept differs substantially from other Morse-code-based and essentially digital models that encode information in zero-signal temporal intervals of typically 100 ms between individual action potentials. This needs more time to transfer more specific information via on–off signals, making it hard to explain the short reaction times to nervous signals observed in nature. The model proposed here should not only help to increase our understanding of neural communication but hopefully should also contribute to increasing conservative methods of healing neural ailments.
In any case, a deeper understanding of the function of neurons beyond the Hodgkin–Huxley model based on a detailed understanding of the coupling of electrodynamic and hydrodynamic interactions is certainly highly desirable and needed for a better understanding of our nervous systems. I personally find the route via coherent interaction of propagating acoustoelectric wave pulses containing characteristic high-frequency components a more promising approach than thinking of digital communications as a Morse code. However, a combination of both using phase-preserving pulse shapes in combination with discrete time intervals may also be an option. In any case, I consider it also as another example that nature has utilized rather intricate physical concepts long before physicists became aware of them.
As an individual approaching the age of eighty, I happened to be able to use my own body as a case study for parts of the proposed model of neuron function. When I pulled too heavy a load nearly three years ago, I injured my lumbar spine. Apparently, it also squeezed a neuron on its route through my hip to my left leg which got numb and partially paralyzed. I tried to fight this conservatively with medical gymnastics and acupuncture which both helped. Being introduced to massage of my fasciae, I discovered that by using this on my left leg along the routing of the affected nerve, I was able to remove the neural blockade and interpreted it as removing an acoustoelectric impedance mismatch of the nerve. During the same period, I also experienced numbness in my left arm and hand, diagnosed as polyneuropathy, and, again, by massage of the relevant fasciae, I was able to remove this defect so far permanently. The combination of fasciae massage and Pilates training, both techniques that may be associated with the restoration of biotensegrity, made me convinced that the above-proposed model helped me substantially to get rid of some age-related defects so that I can now again walk, bicycle, row, and, occasionally, lift a heavy beer mug again without pain. Of course, this individual case study is not the basis for this manuscript but perhaps it will help others to treat nerve-related ailments conservatively.