Plasma-Assisted Abatement of Per- and Polyfluoroalkyl Substances (PFAS): Thermodynamic Analysis and Validation in Gliding Arc Discharge
Abstract
1. Introduction
1.1. Per- and Polyfluoroalkyl Substances’ (PFAS) Contamination in the Environment
1.2. The Promising Potential of Non-Equilibrium Plasma for the Treatment of PFAS-Contaminated Water
2. Materials and Methods
2.1. Thermodynamic Modeling
2.1.1. Enthalpy of PFOA Mineralization in Water
2.1.2. Enthalpy of Formation of PFOA
2.1.3. Thermodynamic Model of PFOA Mineralization in Water
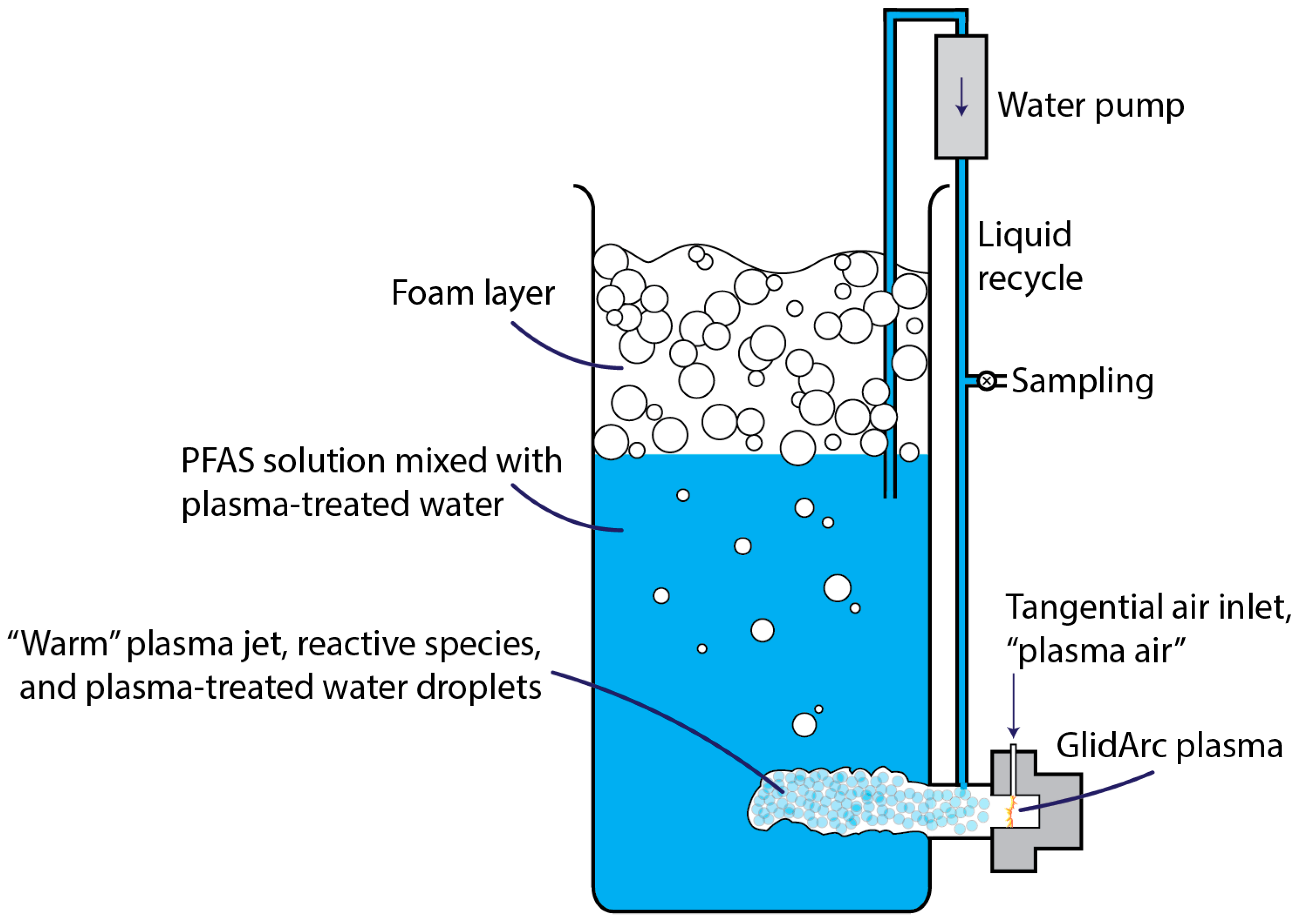
2.2. Experimental Validation of Thermodynamic Model Using GAP Liquid Treatment System
2.2.1. GAP Liquid Treatment System
- Liquid was now continuously recycled from the top of the reactor (near the air–water interface) to just above the plasmatron;
- Samples were taken from the recycle line;
- Atomization air was removed;
- Recycle through the plasmatron was removed.
2.2.2. PFOA and PFOS GAP Treatment Experiments
2.2.3. GAP Energy Input Calculations
2.2.4. Fluoride Analysis via Ion-Selective Electrode
2.3. Data Analysis and Graphing
2.4. Preliminary Kinetic Analysis
2.4.1. Hydroxyl Radical Activation Energy
2.4.2. Hydrated Electron Activation Energy
| (a) | ||
| Type | Number of Bonds | BDE (kJ/mol) |
| O–H | 1 | 425.76 [68] |
| C()–F | 1 | 449.07 [46] |
| (b) | ||
| Type | Number of Bonds | BDE (kJ/mol) |
| H–F | 1 | 565 [56] |
| C–O | 1 | 358 [56] |
3. Results and Discussion
3.1. Enthalpy Calculations
3.2. Thermodynamic Model of PFOA Mineralization in Water and Experimental Validation Using GAP Liquid Treatment System
3.3. Preliminary Kinetic Analysis
4. Conclusions
- The estimated enthalpy of PFOA mineralization in water (Equation (1)) is 480 kJ/mol, making the reaction endothermic. Thus, plasma reactive species alone cannot accelerate the reaction, and energy input (e.g., heat) is required for PFOA mineralization in plasma treatment systems;
- The estimated enthalpy of the formation of PFOA is −4640 kJ/mol;
- Though energy is required, PFOA mineralization is possible with a lower energy barrier than that seen in thermal treatment, likely in part due to catalysis by plasma reactive species such as •OH and e−(aq). This is validated by the GAP experiments presented here as well as other cited publications;
- The activation energies for •OH and e−(aq), separately, to react with the alpha C–F bond in PFOA are estimated to be around 1 eV and 2 eV, respectively.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lindstrom, A.B.; Strynar, M.J.; Libelo, E.L. Polyfluorinated Compounds: Past, Present, and Future. Environ. Sci. Technol. 2011, 45, 7954–7961. [Google Scholar] [CrossRef] [PubMed]
- Lau, C.; Anitole, K.; Hodes, C.; Lai, D.; Pfahles-Hutchens, A.; Seed, J. Perfluoroalkyl Acids: A Review of Monitoring and Toxicological Findings. Toxicol. Sci. 2007, 99, 366–394. [Google Scholar] [CrossRef]
- Xiao, F.; Sasi, P.C.; Yao, B.; Kubátová, A.; Golovko, S.A.; Golovko, M.; Soli, D. Thermal Stability and Decomposition of Perfluoroalkyl Substances on Spent Granular Activated Carbon. Environ. Sci. Technol. Lett. 2020, 7, 343–350. [Google Scholar] [CrossRef]
- Watanabe, N.; Takata, M.; Takemine, S.; Yamamoto, K. Thermal mineralization behavior of PFOA, PFHxA, and PFOS during reactivation of granular activated carbon (GAC) in nitrogen atmosphere. Environ. Sci. Pollut. Res. 2018, 25, 7200–7205. [Google Scholar] [CrossRef] [PubMed]
- Lind, A.-S. An Assessment of Thermal Desorption as a Remediation Technique for Per- and Polyfluoroalkyl Substances (PFASs) in Contaminated Soil; Uppsala University: Uppsala, Sweden, 2018. [Google Scholar]
- Crownover, E.; Oberle, D.; Kluger, M.; Heron, G. Perfluoroalkyl and polyfluoroalkyl substances thermal desorption evaluation. Remediat. J. 2019, 29, 77–81. [Google Scholar] [CrossRef]
- Lu, D.; Sha, S.; Luo, J.; Huang, Z.; Jackie, X.Z. Treatment train approaches for the remediation of per- and polyfluoroalkyl substances (PFAS): A critical review. J. Hazard. Mater. 2020, 386, 121963. [Google Scholar] [CrossRef]
- LaZerte, J.D.; Hals, L.J.; Reid, T.S.; Smith, G.H. Pyrolyses of the Salts of the Perfluoro Carboxylic Acids. J. Am. Chem. Soc. 1953, 75, 4525–4528. [Google Scholar] [CrossRef]
- Krusic, P.J.; Marchione, A.A.; Roe, D.C. Gas-phase NMR studies of the thermolysis of perfluorooctanoic acid. J. Fluor. Chem. 2005, 126, 1510–1516. [Google Scholar] [CrossRef]
- Horst, J.; McDonough, J.; Ross, I.; Houtz, E. Understanding and Managing the Potential By-Products of PFAS Destruction. Groundw. Monit. Remediat. 2020, 40, 17–27. [Google Scholar] [CrossRef]
- Duchesne, A.L.; Brown, J.K.; Patch, D.J.; Major, D.W.; Weber, K.P.; Gerhard, J.I. Remediation of PFAS-Contaminated Soil and Granular Activated Carbon by Smoldering Combustion. Environ. Sci. Technol. 2020, 54, 12631–12640. [Google Scholar] [CrossRef]
- Zhang, W.; Cao, H.; Subramanya, S.M.; Savage, P.; Liang, Y. Destruction of Perfluoroalkyl Acids Accumulated in Typha latifolia through Hydrothermal Liquefaction. ACS Sustain. Chem. Eng. 2020, 8, 9257–9262. [Google Scholar] [CrossRef]
- Yu, J.; Nickerson, A.; Li, Y.; Fang, Y.; Strathmann, T.J. Fate of per- and polyfluoroalkyl substances (PFAS) during hydrothermal liquefaction of municipal wastewater treatment sludge. Environ. Sci. Water Res. Technol. 2020, 6, 1388–1399. [Google Scholar] [CrossRef]
- Longendyke, G.K.; Katel, S.; Wang, Y. PFAS fate and destruction mechanisms during thermal treatment: A comprehensive review. Environ. Sci. Process. Impacts 2022, 24, 196–208. [Google Scholar] [CrossRef] [PubMed]
- Hogue, C. US military sued to stop PFAS incineration. CEN Glob. Enterp. 2020, 98, 27. [Google Scholar] [CrossRef]
- Crossen, B. What the DoD PFAS Incineration Halt Means for Water Professionals. Water and Wastes Digest (WWD). 2022. Available online: https://www.wwdmag.com/wastewater-treatment/article/11004156/what-the-dod-pfas-incineration-halt-means-for-water-professionals (accessed on 20 January 2023).
- Groele, J.R.; Sculley, N.; Olson, T.M.; Foster, J.E. An investigation of plasma-driven decomposition of per- and polyfluoroalkyl substances (PFAS) in raw contaminated ground water. J. Appl. Phys. 2021, 130, 053304. [Google Scholar] [CrossRef]
- Zhang, H. Enhancing Interface Reactions by Introducing Microbubbles into a Plasma Treatment Process for Efficient Decomposition of PFOA. Environ. Sci. Technol. 2021, 55, 16067–16077. [Google Scholar] [CrossRef] [PubMed]
- Zhan, J.; Zhang, A.; Héroux, P.; Guo, Y.; Sun, Z.; Li, Z.; Zhao, J.; Liu, Y. Remediation of perfluorooctanoic acid (PFOA) polluted soil using pulsed corona discharge plasma. J. Hazard. Mater. 2020, 387, 121688. [Google Scholar] [CrossRef]
- Tachibana, K.; Takeuchi, N.; Yasuoka, K. Reaction Process of Perfluorooctanesulfonic Acid (PFOS) Decomposed by DC Plasma Generated in Argon Gas Bubbles. IEEE Trans. Plasma Sci. 2014, 42, 786–793. [Google Scholar] [CrossRef]
- Stratton, G.R.; Dai, F.; Bellona, C.L.; Holsen, T.M.; Dickenson, E.R.V.; Thagard, S.M. Plasma-Based Water Treatment: Efficient Transformation of Perfluoroalkyl Substances in Prepared Solutions and Contaminated Groundwater. Environ. Sci. Technol. 2017, 51, 1643–1648. [Google Scholar] [CrossRef]
- Singh, R.K.; Multari, N.; Nau-Hix, C.; Woodard, S.; Nickelsen, M.; Thagard, S.M.; Holsen, T.M. Removal of Poly- and Per-Fluorinated Compounds from Ion Exchange Regenerant Still Bottom Samples in a Plasma Reactor. Environ. Sci. Technol. 2020, 54, 13973–13980. [Google Scholar] [CrossRef]
- Singh, R.K.; Multari, N.; Nau-Hix, C.; Anderson, R.H.; Richardson, S.D.; Holsen, T.M.; Thagard, S.M. Rapid Removal of Poly- and Perfluorinated Compounds from Investigation-Derived Waste (IDW) in a Pilot-Scale Plasma Reactor. Environ. Sci. Technol. 2019, 53, 11375–11382. [Google Scholar] [CrossRef] [PubMed]
- Singh, R.K.; Brown, E.; Thagard, S.M.; Holsen, T.M. Treatment of PFAS-containing landfill leachate using an enhanced contact plasma reactor. J. Hazard. Mater. 2020, 408, 124452. [Google Scholar] [CrossRef] [PubMed]
- Saleem, M.; Tomei, G.; Beria, M.; Marotta, E.; Paradisi, C. Highly efficient degradation of PFAS and other surfactants in water with atmospheric RAdial plasma (RAP) dis-charge. Chemosphere 2022, 307, 135800. [Google Scholar] [CrossRef] [PubMed]
- Saleem, M.; Biondo, O.; Sretenović, G.; Tomei, G.; Magarotto, M.; Pavarin, D.; Marotta, E.; Paradisi, C. Comparative performance assessment of plasma reactors for the treatment of PFOA; reactor design, kinetics, mineralization and energy yield. Chem. Eng. J. 2020, 382, 123031. [Google Scholar] [CrossRef]
- Palma, D.; Papagiannaki, D.; Lai, M.; Binetti, R.; Sleiman, M.; Minella, M.; Richard, C. PFAS Degradation in Ultrapure and Groundwater Using Non-Thermal Plasma. Molecules 2021, 26, 924. [Google Scholar] [CrossRef]
- Nau-Hix, C.; Multari, N.; Singh, R.K.; Richardson, S.; Kulkarni, P.; Anderson, R.H.; Holsen, T.M.; Thagard, S.M. Field Demonstration of a Pilot-Scale Plasma Reactor for the Rapid Removal of Poly- and Perfluoroalkyl Substances in Groundwater. ACS EST Water 2021, 1, 680–687. [Google Scholar] [CrossRef]
- Mahyar, A.; Miessner, H.; Mueller, S.; Aziz, K.H.H.; Kalass, D.; Moeller, D.; Kretschmer, K.; Manuel, S.R.; Noack, J. Development and Application of Different Non-thermal Plasma Reactors for the Removal of Perfluorosurfactants in Water: A Comparative Study. Plasma Chem. Plasma Process. 2019, 39, 531–544. [Google Scholar] [CrossRef]
- Lewis, A.J.; Joyce, T.; Hadaya, M.; Ebrahimi, F.; Dragiev, I.; Giardetti, N.; Yang, J.; Fridman, G.; Rabinovich, A.; Fridman, A.A.; et al. Rapid degradation of PFAS in aqueous solutions by reverse vortex flow gliding arc plasma. Environ. Sci. Water Res. Technol. 2020, 6, 1044–1057. [Google Scholar] [CrossRef]
- Jovicic, V.; Khan, M.J.; Zbogar-Rasic, A.; Fedorova, N.; Poser, A.; Swoboda, P.; Delgado, A. Degradation of Low Concentrated Perfluorinated Compounds (PFCs) from Water Samples Using Non-Thermal Atmospheric Plasma (NTAP). Energies 2018, 11, 1290. [Google Scholar] [CrossRef]
- Hayashi, R.; Obo, H.; Takeuchi, N.; Yasuoka, K. Decomposition of Perfluorinated Compounds in Water by DC Plasma within Oxygen Bubbles. Electr. Eng. Jpn. 2015, 190, 9–16. [Google Scholar] [CrossRef]
- Guo, J. Theoretical and experimental insights into electron-induced efficient defluorination of perfluorooctanoic acid and per-fluorooctane sulfonate by mesoporous plasma. Chem. Eng. J. 2022, 430, 132922. [Google Scholar] [CrossRef]
- Gao, Z. Theoretical and experimental insights into the mechanisms of C6/C6 PFPiA degradation by dielectric barrier discharge plasma. J. Hazard. Mater. 2022, 424, 127522. [Google Scholar] [CrossRef] [PubMed]
- Aziz, K.H.H.; Miessner, H.; Mahyar, A.; Mueller, S.; Moeller, D.; Mustafa, F.; Omer, K.M. Degradation of perfluorosurfactant in aqueous solution using non-thermal plasma generated by nano-second pulse corona discharge reactor. Arab. J. Chem. 2021, 14, 103366. [Google Scholar] [CrossRef]
- Pinkard, B.R.; Shetty, S.; Stritzinger, D.; Bellona, C.; Novosselov, I.V. Destruction of perfluorooctanesulfonate (PFOS) in a batch supercritical water oxidation reactor. Chemosphere 2021, 279, 130834. [Google Scholar] [CrossRef] [PubMed]
- Krause, M.J.; Thoma, E.; Sahle-Damesessie, E.; Crone, B.; Whitehill, A.; Shields, E.; Gullett, B. Supercritical Water Oxidation as an Innovative Technology for PFAS Destruction. J. Environ. Eng. 2022, 148, 05021006. [Google Scholar] [CrossRef]
- Gole, V.L.; Fishgold, A.; Sierra-Alvarez, R.; Deymier, P.; Keswani, M. Treatment of perfluorooctane sulfonic acid (PFOS) using a large-scale sonochemical reactor. Sep. Purif. Technol. 2018, 194, 104–110. [Google Scholar] [CrossRef]
- Moriwaki, H.; Takagi, Y.; Tanaka, M.; Tsuruho, K.; Okitsu, K.; Maeda, Y. Sonochemical Decomposition of Perfluorooctane Sulfonate and Perfluorooctanoic Acid. Environ. Sci. Technol. 2005, 39, 3388–3392. [Google Scholar] [CrossRef]
- Campbell, T.; Hoffmann, M.R. Sonochemical degradation of perfluorinated surfactants: Power and multiple frequency effects. Sep. Purif. Technol. 2015, 156, 1019–1027. [Google Scholar] [CrossRef]
- Schaefer, C.E.; Andaya, C.; Urtiaga, A.; McKenzie, E.R.; Higgins, C.P. Electrochemical treatment of perfluorooctanoic acid (PFOA) and perfluorooctane sulfonic acid (PFOS) in groundwater impacted by aqueous film forming foams (AFFFs). J. Hazard. Mater. 2015, 295, 170–175. [Google Scholar] [CrossRef]
- Zhuo, Q.; Deng, S.; Yang, B.; Huang, J.; Yu, G. Efficient Electrochemical Oxidation of Perfluorooctanoate Using a Ti/SnO2-Sb-Bi Anode. Environ. Sci. Technol. 2011, 45, 2973–2979. [Google Scholar] [CrossRef]
- Veciana, M.; Bräunig, J.; Farhat, A.; Pype, M.-L.; Freguia, S.; Carvalho, G.; Keller, J.; Ledezma, P. Electrochemical oxidation processes for PFAS removal from contaminated water and wastewater: Fundamentals, gaps and opportunities towards practical implementation. J. Hazard. Mater. 2022, 434, 128886. [Google Scholar] [CrossRef]
- Wang, Y. Electrochemical degradation of perfluoroalkyl acids by titanium suboxide anodes. Environ. Sci. Water Res. Technol. 2020, 6, 144–152. [Google Scholar] [CrossRef]
- Liu, Z.; Bentel, M.J.; Yu, Y.; Ren, C.; Gao, J.; Pulikkal, V.F.; Sun, M.; Men, Y.; Liu, J. Near-Quantitative Defluorination of Perfluorinated and Fluorotelomer Carboxylates and Sulfonates with Integrated Oxidation and Reduction. Environ. Sci. Technol. 2021, 55, 7052–7062. [Google Scholar] [CrossRef]
- Bentel, M.J.; Yu, Y.; Xu, L.; Li, Z.; Wong, B.M.; Men, Y.; Liu, J. Defluorination of Per- and Polyfluoroalkyl Substances (PFASs) with Hydrated Electrons: Structural Dependence and Implications to PFAS Remediation and Management. Environ. Sci. Technol. 2019, 53, 3718–3728. [Google Scholar] [CrossRef]
- Scholtz, V.; Pazlarova, J.; Souskova, H.; Khun, J.; Julak, J. Nonthermal plasma—A tool for decontamination and disinfection. Biotechnol. Adv. 2015, 33, 1108–1119. [Google Scholar] [CrossRef] [PubMed]
- Lau, S.F.; Suzuki, H.; Wunderlich, B. The thermodynamic properties of polytetrafluoroethylene. J. Polym. Sci. Polym. Phys. Ed. 1984, 22, 379–405. [Google Scholar] [CrossRef]
- Snitsiriwat, S.; Hudzik, J.M.; Chaisaward, K.; Stoler, L.R.; Bozzelli, J.W. Thermodynamic Properties: Enthalpy, Entropy, Heat Capacity, and Bond Energies of Fluorinated Carboxylic Acids. J. Phys. Chem. A 2022, 126, 3–15. [Google Scholar] [CrossRef] [PubMed]
- Rayne, S.; Forest, K. Comparative semiempirical, ab initio, and density functional theory study on the thermodynamic properties of linear and branched perfluoroalkyl sulfonic acids/sulfonyl fluorides, perfluoroalkyl carboxylic acid/acyl fluorides, and perhydroalkyl sulfonic acids, alkanes, and alcohols. J. Mol. Struct. THEOCHEM 2010, 941, 107–118. [Google Scholar] [CrossRef]
- Hidalgo, A.; Giroday, T.; Mora-Diez, N. Thermodynamic stability of neutral and anionic PFOAs. Theor. Chem. Accounts 2015, 134, 124. [Google Scholar] [CrossRef]
- Blotevogel, J.; Giraud, R.J.; Rappé, A.K. Incinerability of PFOA and HFPO-DA: Mechanisms, kinetics, and thermal stability ranking. Chem. Eng. J. 2023, 457, 141235. [Google Scholar] [CrossRef]
- Baggioli, A.; Sansotera, M.; Navarrini, W. Thermodynamics of aqueous perfluorooctanoic acid (PFOA) and 4,8-dioxa-3H-perfluorononanoic acid (DONA) from DFT calculations: Insights into degradation initiation. Chemosphere 2018, 193, 1063–1070. [Google Scholar] [CrossRef] [PubMed]
- Rabinovich, A.; Nirenberg, G.; Kocagoz, S.; Surace, M.; Sales, C.; Fridman, A. Scaling Up of Non-Thermal Gliding Arc Plasma Systems for Industrial Applications. Plasma Chem. Plasma Process. 2021, 42, 35–50. [Google Scholar] [CrossRef]
- ALFABET: A Machine-Learning Derived, Fast, Accurate Bond Dissociation Enthalpy Tool. 2022. Available online: https://bde.ml.nrel.gov/ (accessed on 16 August 2022).
- Kildahl, N.K. Bond Energy Data Summarized. J. Chem. Educ. 1995, 72, 423. [Google Scholar] [CrossRef]
- Rumble, J.R. Bond Dissociation Energies in Polyatomic Molecules. In CRC Handbook of Chemistry and Physics, 104th ed.; CRC: Boca Raton, FL, USA, 2010. [Google Scholar]
- Dolder, S. MoleculeSketch [Mobile Application Software]. 2018. App Store. Available online: https://www.apple.com/app-store/ (accessed on 12 May 2023).
- HSC Chemistry Version 6.1; Outotec: Pori, Finland, 1974–2007.
- Quiñones, O.; Snyder, S.A. Occurrence of Perfluoroalkyl Carboxylates and Sulfonates in Drinking Water Utilities and Related Waters from the United States. Environ. Sci. Technol. 2009, 43, 9089–9095. [Google Scholar] [CrossRef] [PubMed]
- U.S. Environmental Protection Agency. Method 9214: Potentiometric Determination of Fluoride in Aqueous Samples with Ion-Selective Electrode; U.S. Environmental Protection Agency: Washington, DC, USA, 1996.
- MATLAB Version 9.13.0.2126072; The MathWorks Inc.: Natick, MA, USA, 2022.
- Fridman, A. Plasma Chemistry; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Van Hoomissen, D.J.; Vyas, S. Early Events in the Reductive Dehalogenation of Linear Perfluoroalkyl Substances. Environ. Sci. Technol. Lett. 2019, 6, 365–371. [Google Scholar] [CrossRef]
- Qu, Y.; Zhang, C.; Li, F.; Chen, J.; Zhou, Q. Photo-reductive defluorination of perfluorooctanoic acid in water. Water Res. 2010, 44, 2939–2947. [Google Scholar] [CrossRef]
- Ando, K.; Hynes, J.T. Molecular Mechanism of HF Acid Ionization in Water: An Electronic Structure—Monte Carlo Study. J. Phys. Chem. A 1999, 103, 10398–10408. [Google Scholar] [CrossRef]
- Myers, R.T. The periodicity of electron affinity. J. Chem. Educ. 1990, 67, 307. [Google Scholar] [CrossRef]
- Ruscic, B.; Wagner, A.F.; Harding, L.B.; Asher, R.L.; Feller, D.; Dixon, D.A.; Peterson, K.A.; Song, Y.; Qian, X.; Ng, C.-Y.; et al. On the Enthalpy of Formation of Hydroxyl Radical and Gas-Phase Bond Dissociation Energies of Water and Hydroxyl. J. Phys. Chem. A 2002, 106, 2727–2747. [Google Scholar] [CrossRef]
- Oliver, G.D.; Eaton, M.; Huffman, H.M. The Heat Capacity, Heat of Fusion and Entropy of Benzene. J. Am. Chem. Soc. 1948, 70, 1502–1505. [Google Scholar] [CrossRef]
- Messerly, J.; Finke, H. Hexafluorobenzene and 1,3-difluorobenzene low-temperature calorimetric studies and chemical thermodynamic properties. J. Chem. Thermodyn. 1970, 2, 867–880. [Google Scholar] [CrossRef]
- Kulkarni, P.R.; Richardson, S.D.; Nzeribe, B.N.; Adamson, D.T.; Kalra, S.S.; Mahendra, S.; Blotevogel, J.; Hanson, A.; Dooley, G.; Maraviov, S.; et al. Field Demonstration of a Sonolysis Reactor for Treatment of PFAS-Contaminated Groundwater. J. Environ. Eng. 2022, 148, 06022005. [Google Scholar] [CrossRef]
- Du, C.M.; Sun, Y.W.; Zhuang, X.F. The Effects of Gas Composition on Active Species and Byproducts Formation in Gas—Water Gliding Arc Discharge. Plasma Chem. Plasma Process. 2008, 28, 523–533. [Google Scholar] [CrossRef]
- Roy, N.C.; Talukder, M.R. Effect of pressure on the properties and species production in gliding arc Ar, O2, and air discharge plasmas. Phys. Plasmas 2018, 25, 093502. [Google Scholar] [CrossRef]
- Vecitis, C.D.; Park, H.; Cheng, J.; Mader, B.T.; Hoffmann, M.R. Treatment technologies for aqueous perfluorooctanesulfonate (PFOS) and perfluorooctanoate (PFOA). Front. Environ. Sci. Eng. China 2009, 3, 129–151. [Google Scholar] [CrossRef]
- Javed, H.; Lyu, C.; Sun, R.; Zhang, D.; Alvarez, P.J. Discerning the inefficacy of hydroxyl radicals during perfluorooctanoic acid degradation. Chemosphere 2020, 247, 125883. [Google Scholar] [CrossRef]
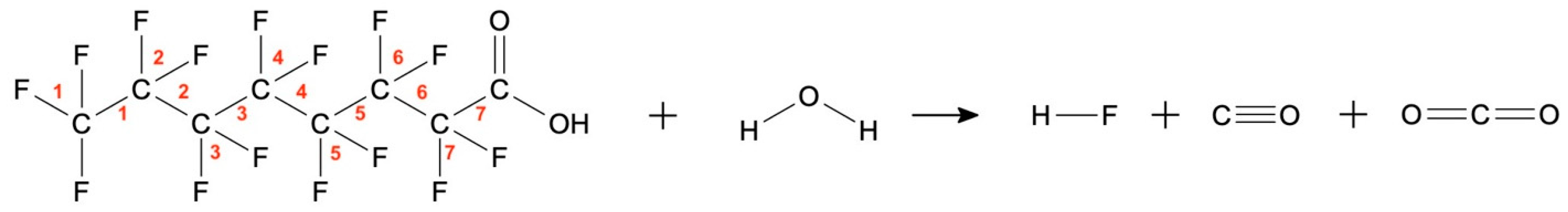


| (a) | ||
| Type | Number of Bonds | BDE (kJ/mol) |
| O–H (in H2O) | 14 | 497.32 [57] |
| C=O | 1 | 799 [56] |
| C–O | 1 | 464.0056 [55] |
| O–H (in PFOA) | 1 | 475.79 [53] |
| C–F (1) | 3 | 492.50 [46] |
| C–F (2) | 2 | 453.13 [46] |
| C–F (3) | 2 | 446.77 [46] |
| C–F (4) | 2 | 447.73 [46] |
| C–F (5) | 2 | 448.78 [46] |
| C–F (6) | 2 | 452.12 [46] |
| C–F (7) | 2 | 449.07 [46] |
| C–C (1) | 1 | 383.6728 [55] |
| C–C (2) | 1 | 371.1208 [55] |
| C–C (3) | 1 | 371.9576 [55] |
| C–C (4) | 1 | 372.3760 [55] |
| C–C (5) | 1 | 376.9784 [55] |
| C–C (6) | 1 | 349.7824 [55] |
| C–C (7) | 1 | 371.5392 [55] |
| = 18,171 kJ/mol | ||
| (b) | ||
| Type | Number of Bonds | BDE (kJ/mol) |
| C≡O | 7 | 1076.63 [57] |
| C=O (in CO2) | 2 | 804.42 [57] |
| H–F | 15 | 569.68 [57] |
| = 17,690.44 kJ/mol | ||
| Compound | Phase | , kJ/mol) |
|---|---|---|
| H2O | l | −285.8 [59] |
| HF | aq | −332.6 [59] |
| CO | g | −110.5 [59] |
| CO2 | g | −393.5 [59] |
| Parent Compound | Plasma Gas | Plasma Power (W) |
|---|---|---|
| PFOA | Argon | 180 |
| PFOS | Argon | 180 |
| PFOA | Air | 350 |
| PFOS | Air | 375 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Surace, M.J.; Murillo-Gelvez, J.; Shaji, M.A.; Fridman, A.A.; Rabinovich, A.; McKenzie, E.R.; Fridman, G.; Sales, C.M. Plasma-Assisted Abatement of Per- and Polyfluoroalkyl Substances (PFAS): Thermodynamic Analysis and Validation in Gliding Arc Discharge. Plasma 2023, 6, 419-434. https://doi.org/10.3390/plasma6030029
Surace MJ, Murillo-Gelvez J, Shaji MA, Fridman AA, Rabinovich A, McKenzie ER, Fridman G, Sales CM. Plasma-Assisted Abatement of Per- and Polyfluoroalkyl Substances (PFAS): Thermodynamic Analysis and Validation in Gliding Arc Discharge. Plasma. 2023; 6(3):419-434. https://doi.org/10.3390/plasma6030029
Chicago/Turabian StyleSurace, Mikaela J., Jimmy Murillo-Gelvez, Mobish A. Shaji, Alexander A. Fridman, Alexander Rabinovich, Erica R. McKenzie, Gregory Fridman, and Christopher M. Sales. 2023. "Plasma-Assisted Abatement of Per- and Polyfluoroalkyl Substances (PFAS): Thermodynamic Analysis and Validation in Gliding Arc Discharge" Plasma 6, no. 3: 419-434. https://doi.org/10.3390/plasma6030029
APA StyleSurace, M. J., Murillo-Gelvez, J., Shaji, M. A., Fridman, A. A., Rabinovich, A., McKenzie, E. R., Fridman, G., & Sales, C. M. (2023). Plasma-Assisted Abatement of Per- and Polyfluoroalkyl Substances (PFAS): Thermodynamic Analysis and Validation in Gliding Arc Discharge. Plasma, 6(3), 419-434. https://doi.org/10.3390/plasma6030029






