Free Vibration Characteristics of Functionally Graded Material (FGM) Beams on Three-Parameter Viscoelastic Foundation

Abstract

This study numerically investigated free vibration characteristics of functionally graded material (FGM) beams on Winkler–Pasternak three-parameter elastic foundations using the modified generalized differential quadrature (MGDQ) method. To compare the effects of different beam theories on the predicted frequency responses, an nth order generalized beam theory was employed to establish the governing equations of the system’s dynamic model within the Hamilton framework. As a pioneering effort, a MATLAB (version 2021a) computational program implementing the MGDQ method was developed to obtain the free vibration responses of foundation-supported FGM beams. Parametric analyses were conducted through numerical simulations to systematically examine the influences of various factors, including beam theories, damping coefficients, foundation stiffness parameters, boundary conditions, gradient indices, and span-to-thickness ratios, on the natural frequencies and damping ratios of FGM beams. The findings provide an essential theoretical foundation for dynamic characteristic analysis and functional design of foundation-supported FGM beam structures.

1. Introduction

Dynamic characteristics analysis of beams on elastic foundations has garnered significant attention owing to their broad range of applications in civil engineering, rail transit, mechanical systems, and biomedical engineering [1,2,3,4]. Current elastic foundation models primarily include the Winkler–Pasternak [1], Kelvin [2], Vlasov [3], and Kerr [4] models. Among these, the Winkler–Pasternak two-parameter model is widely adopted by researchers [5,6], as it effectively characterizes both the compressive and shear interactions between the foundation and beam, thus offering a realistic representation of elastic foundation behavior. Notably, Thambiratnam & Zhuge [7] pioneered dynamic analysis of beams under moving loads on elastic foundations; they established a theoretical framework for time-domain response prediction. Kim & Roesset [8] further quantified the influence of frequency-independent damping in foundation models on dynamic responses, highlighting the necessity of viscosity characterization. Furthermore, the damping effects of foundation viscosity are unavoidable in practical engineering. For instance, studies [9,10,11] employed Euler beam theory (also known as classical beam theory (CBT)) to numerically investigate the vibration characteristics of homogeneous beams on viscoelastic foundations using diverse analytical approaches. Recent advances in viscoelastic modeling emphasize the importance of fractional calculus, as demonstrated by Sorrentino & Fasana [12], who integrated fractional derivative models into finite element analysis of vibrating systems, capturing frequency-dependent damping effects more accurately than classical integer-order approaches.

Functionally graded material (FGM) beams, featuring spatially continuous gradation of multiphase materials, enable tailored material parameter distributions. This customizable feature has led to innovative applications in cutting-edge fields, such as aerospace thermal protection systems and heterogeneous interfaces in energy equipment. Extending this concept to plate structures, a recent work [13] investigated free vibration of FGM plates on elastic foundations, revealing micromechanical model sensitivity in predicting mode shapes—a finding that underscores the need for refined modeling in FGM beam studies. Precise analysis of the dynamic behavior of FGM beams holds critical theoretical value for overcoming multi-objective optimization challenges under extreme conditions and ensuring operational safety, thus making it a frontier topic in smart material mechanics.

Existing research predominantly adopts forward analysis frameworks, combining analytical and numerical methods to explore the dynamic mechanisms of FGM beams [14,15,16,17,18,19,20,21,22,23,24,25,26]. In theoretical modeling, Simsek [14] pioneered a unified Lagrange frequency equation system encompassing seven shear deformation theories. Kahya et al. [15] developed finite element models for free vibration and buckling analysis based on Timoshenko’s first-order shear beam theory (FSBT). The integration of advanced viscoelastic models was facilitated by Lesieutre & Lee [16], who developed a finite element method with frequency-dependent constrained layer damping and demonstrated enhanced energy dissipation modeling capabilities for beam structures. For coupled-field analysis, some studies [17,18,19] systematically investigated vibration responses under thermo-mechanical multiphysical coupling. Pu Yu et al. [20] resolved the dynamic prediction challenges of porous FGM beams under hygro-thermo-mechanical coupling using the n-th order generalized beam theory (GBT) and Navier’s analytical method.

Some notable advancements in elastic foundation effects include the following: Ying et al. [21] established a free vibration model for FGM simply supported beams on elastic foundations using state-space methods based on 2D linear elasticity theory, while Pu Yu et al. [22] revealed the regulatory effects of Winkler–Pasternak foundation parameters on vibration modes via 2D differential quadrature methods. Zahedinejad [23] quantified thermal effects on spectral characteristics of foundation beams by integrating Reddy’s third-order shear theory (TBT) with differential quadrature techniques. Sun et al. [24] provided critical insights into memory-dependent behaviors through comparative analysis of constant-order versus variable-order fractional models, thus establishing theoretical foundations for selecting viscoelastic constitutive models in foundation-beam interactions. Deng et al. [25] pioneered quantitative stability evaluation of dual-FGM beam systems using the FSBT-based dynamic stiffness matrices. Recently, Pu Yu et al. [26] employed GBT and a modified generalized differential quadrature (MGDQ) method to numerically study the vibration characteristics of FGM beams on elastic foundations under initial axial loads; specifically, they unveiled the dual coupling between buckling and vibrational static-dynamic behaviors.

Moreover, Duc et al. [27] developed a nonlinear dynamic model for piezoelectric FGM hyperbolic shells embedded in elastic foundations under damping-thermal-electrical-mechanical loads. Using stress functions, the Galerkin method, and the fourth-order Runge-Kutta method, they solved the nonlinear vibration problem, emphasizing the effects of material properties and coupled loads on nonlinear dynamic responses. Loghman et al. [28] considered the viscoelastic internal damping of materials and, based on modified couple stress theory and Von Kármán nonlinear relations, applied finite difference and finite element methods to analyze the influence of fractional damping coefficients, scale parameters, and material gradient indices on the nonlinear free and forced vibration characteristics of FGM microbeams. Despite these advancements, research on the vibration behavior of FGM beams on viscoelastic foundations remains limited.

This work introduces a novel approach by incorporating the foundation’s damping effect from an energy dissipation perspective. As an exploratory effort, we developed the system’s dynamic model based on n-th order GBT and Hamilton’s principle. Through the introduction of boundary condition control parameters, we achieved unified derivation of modified weighting coefficients for beams with three boundary configurations: clamped-clamped (C-C), clamped-supported (C-S), and supported-supported (S-S). This study pioneers the application of the MGDQ method to numerically solve the frequency response of free vibrations in FGM beams resting on Winkler–Pasternak three-parameter elastic foundations. Finally, comprehensive parametric analyses were conducted to investigate the effects of multiple factors, including beam theories, damping coefficients, foundation stiffness parameters, boundary conditions, gradient indices, and span-to-thickness ratios, on the frequency characteristics of FGM beams.

2. Governing Differential Equations

2.1. Governing Equations of Dynamic System

As illustrated in Figure 1, consider an FGM beam resting on a three-parameter viscoelastic foundation, with length L, width B, and height H. The beam comprises a ceramic-rich upper surface and a metal-rich lower surface. A Cartesian coordinate system is defined such that the x-axis aligns with the beam’s longitudinal direction; the z-axis corresponds to the thickness direction, and the x-y plane coincides with the geometric midplane of the beam. The beam is subjected to a transversely distributed dynamic load q(x,t). The foundation is characterized by three parameters: the Winkler stiffness coefficient kw, Pasternak shear stiffness coefficient kp, and transverse viscous damping coefficient cd.

The material properties (elastic modulus E, Poisson’s ratio ν, and density ρ) vary continuously along the thickness direction (z) following the Voigt-type power-law mixture model. These graded properties are expressed as:

$$P(z)=P_{\mathrm{m}}+(P_{\mathrm{c}}-P_{\mathrm{m}}){({\displaystyle \frac{1}{2}}+{\displaystyle \frac{z}{h}})}^p,$$

(1)

where p denotes the material gradient index, and the subscripts m and c represent the metallic and ceramic constituents, respectively.

To compare the predictive differences among various beam theories regarding free vibration responses, this study employs an n-th order GBT framework, where the displacement field at any point within the beam can be expressed as:

$$\left\{\begin{array}{l}{u}_x(x,z,t)=u(x,t)-z{\displaystyle \frac{\partial w}{\partial x}}+f(z)[\phi (x,t)+{\displaystyle \frac{\partial w}{\partial x}}]\hfill \\ u_y(x,z,t)=0\hfill \\ u_z(x,z,t)=w(x,t)\hfill \end{array}\right.,$$

(2)

$$f(z)=z-{\displaystyle \frac{h}{2n}}{({\displaystyle \frac{2z}{h}})}^n,$$

(3)

where u(x,t) denotes the axial displacement at time t; φ(x,t) represents the cross-sectional rotation; and ω(x,t) corresponds to the generalized transverse displacements associated with the i-th deformation mode. The function f(z) represents the transverse shear stress shape function corresponding to the n-th order GBT [29]. When shear deformation effects are neglected (i.e., n = 1), the GBT reduces to the CBT; when a parabolic distribution of shear strain is assumed through the beam thickness (i.e., n = 3), the GBT degenerates into Timoshenko Beam Theory (TBT). For a constant shear strain assumption across the beam thickness (i.e., n = ∞).

Based on the geometric equations of small deformation, the expressions for non-zero strain components can be derived as:

$$\left\{\begin{array}{l}{\epsilon }_x={\displaystyle \frac{\partial u}{\partial x}}-z{\displaystyle \frac{{\partial }^{2}w}{\partial x^2}}+f(z)({\displaystyle \frac{\partial \phi }{\partial x}}+{\displaystyle \frac{{\partial }^{2}w}{\partial x^2}})\hfill \\ {\gamma }_{xz}=g(z)({\displaystyle \frac{\partial w}{\partial x}}+\phi )\hfill \end{array}\right.,$$

(4)

where g(z) denotes the transverse shear strain shape function.

The constitutive relations are expressed as:

$$\left\{\begin{array}{l}{\sigma }_x=E(z){\epsilon }_x\hfill \\ {\tau }_{xz}=G(z){\gamma }_{xz}\hfill \end{array}\right.,$$

(5)

$$G(z)={\displaystyle \frac{E(z)}{2\left[1+\mu (z)\right]}},$$

(6)

where G(z) represents the shear modulus varying along the thickness direction.

The variation in the system’s kinetic energy (δT), strain energy (δVε), and external work and viscoelastic foundation potential (δV) are expressed as:

$$\delta T={\displaystyle {\int }_0^L{\displaystyle {\int }_A\rho (z)(\frac{\partial u_x}{\partial t}\frac{\partial \delta u_x}{\partial t}+\frac{\partial u_z}{\partial t}\frac{\partial \delta u_z}{\partial t})}}\mathrm{d}A\mathrm{d}x,$$

(7)

$$\delta V_{\mathsf{\epsilon }}={\displaystyle {\int }_0^L{\displaystyle {\int }_A({\sigma }_x\delta {\epsilon }_x+{\tau }_{xz}\delta {\gamma }_{xz})}}\mathrm{d}A\mathrm{d}x,$$

(8)

$$\delta V={\displaystyle {\int }_0^L\left[(k_{\mathrm{w}}w-c_{\mathrm{d}}\frac{\partial w}{\partial t}+q)\delta w+k_{\mathrm{p}}\frac{\partial w}{\partial x}\frac{\partial \delta w}{\partial x}\right]}\mathrm{d}x.$$

(9)

Applying Hamilton’s principle:

$${\displaystyle {\int }_{t_1}^{t_2}(\delta T-\delta V_{\mathsf{\epsilon }}-\delta V)}\mathrm{d}t=0.$$

(10)

Substituting Equations (2)–(9) into Equation (10), followed by simplification and rearrangement, yields the governing dynamic equation of the system:

$$\begin{gathered} S_1{\displaystyle \frac{{\mathrm{d}}^{2}u}{\mathrm{d}{x}^2}}+S_3{\displaystyle \frac{{\mathrm{d}}^2\phi }{\mathrm{d}{x}^2}}-S_7{\displaystyle \frac{{\mathrm{d}}^{3}w}{\mathrm{d}{x}^3}}-I_0\ddot{u}-I_2\ddot{\phi }-I_6{\displaystyle \frac{\mathrm{d}\ddot{w}}{\mathrm{d}x}}=0, \\ \begin{array}{l}{S}_3{\displaystyle \frac{{\mathrm{d}}^{2}u}{\mathrm{d}{x}^2}}+S_6{\displaystyle \frac{{\mathrm{d}}^2\phi }{\mathrm{d}{x}^2}}-S_0(\phi +{\displaystyle \frac{\mathrm{d}w}{\mathrm{d}x}})+S_8{\displaystyle \frac{{\mathrm{d}}^{3}w}{\mathrm{d}{x}^3}}-\\ I_2\ddot{u}-I_5\ddot{\phi }-I_7{\displaystyle \frac{\mathrm{d}\ddot{w}}{\mathrm{d}x}}=0\end{array}, \\ \begin{array}{l}{S}_7{\displaystyle \frac{{\mathrm{d}}^{3}u}{\mathrm{d}{x}^3}}-S_8{\displaystyle \frac{{\mathrm{d}}^3\phi }{\mathrm{d}{x}^3}}+S_9{\displaystyle \frac{{\mathrm{d}}^{4}w}{\mathrm{d}{x}^4}}+S_0({\displaystyle \frac{\mathrm{d}\phi }{\mathrm{d}x}}+{\displaystyle \frac{{\mathrm{d}}^{2}w}{\mathrm{d}{x}^2}})-\\ k_{\mathrm{w}}w+k_{\mathrm{p}}{\displaystyle \frac{{\mathrm{d}}^{2}w}{\mathrm{d}{x}^2}}-c_{\mathrm{d}}\dot{w}-I_0\ddot{w}+I_6{\displaystyle \frac{\mathrm{d}\ddot{u}}{\mathrm{d}x}}+\\ I_7{\displaystyle \frac{\mathrm{d}\ddot{\phi }}{\mathrm{d}x}}-I_8{\displaystyle \frac{{\mathrm{d}}^2\ddot{w}}{\mathrm{d}{x}^2}}=q(x,t)\end{array}, \end{gathered}$$

(11)

The elastic coefficients are defined as:

$$\begin{gathered} \left\{S_i\right\}={\displaystyle {\int }_{A}E(z) \left\{1,z,f,z^2,zf,f^2\right\}}dA,(i=1,2,\dots 6), \\ {S}_0={\displaystyle {\int }_{A}G(z)g^{2}dA}, S_7=S_2-S_3, S_8=S_6-S_5, \\ {S}_9=2S_5-S_4-S_6. \end{gathered}$$

(12)

The inertia coefficient is defined as:

$$\begin{gathered} \left\{I_i\right\}={\displaystyle {\int }_A\rho (z)\left(1, z, f, z^2, zf, f^2\right)}dA,(i=0,1,\dots 5), \\ {I}_6=I_2-I_1, I_7=I_5-I_4, I_8=2I_4-I_3-I_5. \end{gathered}$$

(13)

Equation (11) governs the forced vibration of a three-parameter viscoelastic foundation-FGM beam under a transverse dynamic load q(x,t). Specific cases include free damped vibration (q(x,t) = 0) and free undamped vibration (q(x,t) = 0 and C_d = 0). Notably, the elastic and inertia coefficients embed tension/compression-shear-bending coupling effects.

Based on Hamilton’s principle, the boundary conditions derived from Equation (10) are simplified and categorized into three beam configurations for investigation:

(i) Clamped-Clamped (C-C):

$$u=\phi =w={\displaystyle \frac{dw}{dx}}=0, x=0 \mathrm{and} x=L.$$

(14)

(ii) Clamped-Supported (C-S):

$$\begin{gathered} u=\phi =w={\displaystyle \frac{dw}{dx}}=0, x=0. \\ {\displaystyle \frac{du}{dx}}={\displaystyle \frac{d\phi }{dx}}=w={\displaystyle \frac{d^{2}w}{d{x}^2}}=0, x=L. \end{gathered}$$

(15)

(iii) Supported-Supported (S-S):

$${\displaystyle \frac{du}{dx}}={\displaystyle \frac{d\phi }{dx}}=w={\displaystyle \frac{d^{2}w}{d{x}^2}}=0, x=0\mathrm{and} x=L.$$

(16)

2.2. Governing Equations of Damped Free Vibration System

For a freely vibrating FGM beam, the displacement components can be assumed as:

$$\left\{\begin{array}{c}u(x,t)\\ \phi (x,t)\\ w(x,t)\end{array}\right\}=\left\{\begin{array}{c}U(x)\\ \Psi (x)\\ W(x)\end{array}\right\}{\exp }^{\mathrm{i}\omega t},$$

(17)

where ω is the complex characteristic frequency of the damped free vibration of the FGM beam; U(x), Ψ(x), W(x) are the displacement mode shape functions; and $i=\sqrt{-1}$ is the imaginary unit.

By setting q(x,t) = 0 and substituting Equation (17) into Equation (11), the governing differential equation for the free vibration of the FGM beam on a three-parameter viscoelastic foundation is derived as shown below:

$$\begin{gathered} S_1{\displaystyle \frac{{\mathrm{d}}^{2}U}{\mathrm{d}{x}^2}}+S_3{\displaystyle \frac{{\mathrm{d}}^2\mathsf{\Psi }}{\mathrm{d}{x}^2}}-S_7{\displaystyle \frac{{\mathrm{d}}^{3}W}{\mathrm{d}{x}^3}}+{\omega }^2(I_{0}U+I_2\mathsf{\Psi }+I_6{\displaystyle \frac{\mathrm{d}W}{\mathrm{d}x}})=0, \\ \begin{array}{l}{S}_3{\displaystyle \frac{{\mathrm{d}}^{2}U}{\mathrm{d}{x}^2}}+S_6{\displaystyle \frac{{\mathrm{d}}^2\mathsf{\Psi }}{\mathrm{d}{x}^2}}-S_0(\mathsf{\Psi }+{\displaystyle \frac{\mathrm{d}W}{\mathrm{d}x}})+S_8{\displaystyle \frac{{\mathrm{d}}^{3}W}{\mathrm{d}{x}^3}}+\\ {\omega }^2(I_{2}U+I_5\mathsf{\Psi }+I_7{\displaystyle \frac{\mathrm{d}W}{\mathrm{d}x}})=0\end{array}, \\ \begin{array}{l}{S}_7{\displaystyle \frac{{\mathrm{d}}^{3}U}{\mathrm{d}{x}^3}}-S_8{\displaystyle \frac{{\mathrm{d}}^3\mathsf{\Psi }}{\mathrm{d}{x}^3}}+S_9{\displaystyle \frac{{\mathrm{d}}^{4}W}{\mathrm{d}{x}^4}}+S_0({\displaystyle \frac{\mathrm{d}\mathsf{\Psi }}{\mathrm{d}x}}+{\displaystyle \frac{{\mathrm{d}}^{2}W}{\mathrm{d}{x}^2}})-\\ k_{\mathrm{w}}W+k_{\mathrm{p}}{\displaystyle \frac{{\mathrm{d}}^{2}W}{\mathrm{d}{x}^2}}-\mathrm{i}{c}_{\mathrm{d}}\omega W+\\ {\omega }^2(I_{0}W-I_6{\displaystyle \frac{\mathrm{d}U}{\mathrm{d}x}}-I_7{\displaystyle \frac{\mathrm{d}\mathsf{\Psi }}{\mathrm{d}x}}+I_8{\displaystyle \frac{{\mathrm{d}}^{2}W}{\mathrm{d}{x}^2}})=0\end{array}. \end{gathered}$$

(18)

Clearly, the governing differential equation (Equation (18)), combined with the boundary equations (Equations (14)–(16)), forms a boundary value problem for the damped free vibration of the FGM beam on a three-parameter viscoelastic foundation under three typical boundary conditions.

3. MGDQ Method for Solving for Complex Characteristic Frequencies

3.1. Introduction of Beam Boundary Condition Control Parameters

First, based on the generalized differential quadrature method [29], the k-th order derivatives of the displacement mode shape functions at discrete nodes x_i can be expressed as:

$${\displaystyle \frac{{\mathrm{d}}^k}{\mathrm{d}{x}^k}}{\left\{\begin{array}{c}U(x)\\ \Psi (x)\\ W(x)\end{array}\right\}}_{x=x_i}={\displaystyle \sum _{j=1}^N{C}_{ij}^{(k)}}\left\{\begin{array}{c}{U}_j(x_j)\\ {\Psi }_j(x_j)\\ W_j(x_j)\end{array}\right\},$$

(19)

where $C_{ij}^{\left(k\right)}$ is the weighting coefficient for the k-th order derivative of the displacement mode shape function, and N is the total number of discrete nodes.

Second, the weighting coefficients for the 0-th order derivative of any unknown function at x = 0 and x = L are defined as:

$$C_{1j}^{(0)}=\left\{\begin{array}{l}1\hspace{1em}(j=1)\hfill \\ 0\hspace{1em}(\mathrm{e}lse)\hfill \end{array}\right.; C_{Nj}^{(0)}=\left\{\begin{array}{l}1\hspace{1em}(j=N)\hfill \\ 0\hspace{1em}(else)\hfill \end{array}\right..$$

(20)

Using these definitions, the boundary equations (Equations (14)–(16)) for C-C, C-S, and S-S beams, respectively, can be uniformly discretized via the GDQ method as:

$$\left\{\begin{array}{c}\begin{array}{l}{\displaystyle \sum _{j=1}^N{C}_{1j}^{(n_0)}{U}_j=0}\\ {\displaystyle \sum _{j=1}^N{C}_{1j}^{(n_0)}{\mathsf{\Psi }}_j=0}\\ W_1=0\end{array}\\ {\displaystyle \sum _{j=1}^N{C}_{1j}^{(n_{0+1})}{W}_j=0}\end{array}\right., \left\{\begin{array}{c}\begin{array}{l}{\displaystyle \sum _{j=1}^N{C}_{Nj}^{(n_1)}{U}_j=0}\\ {\displaystyle \sum _{j=1}^N{C}_{Nj}^{(n_1)}{\mathsf{\Psi }}_j=0}\\ W_N=0\end{array}\\ {\displaystyle \sum _{j=1}^N{C}_{Nj}^{(n_{1+1})}{W}_j=0}\end{array}\right.,$$

(21)

where n₀ and n₁ are the boundary condition control parameters. By assigning n₀ = 0 or 1, and n₁ = 0 or 1, the boundary conditions are defined as follows: n₀ = 0 and n₁ = 0 for the C-C beam; n₀ = 0 and n₁ = 1 for the C-S beam; and n₀ = 1 and n₁ = 0 for the S-S beam.

3.2. Weighting Coefficients in the MGDQ Method

To address the case wherein the total number of discretized equations exceeds the number of unknown displacements (thereby preventing a direct conversion to an eigenvalue problem), this study introduces improved weighting coefficients for the displacement mode shape functions under C-C, C-S, and S-S boundaries. These coefficients are derived [29] as:

$$\left\{\begin{array}{l}{P}_{ij}^{(k)}=C_{ij}^{(k)}+{\displaystyle \frac{C_{i1}^{(k)}\cdot CUK 1+C_{iN}^{(k)}\cdot CUKN}{CUN}}\hfill \\ Q_{ij}^{(k)}=P_{ij}^{(k)}\hfill \\ R_{ij}^{(k)}=C_{ij}^{(k)}+{\displaystyle \frac{C_{i2}^{(k)}\cdot CWK 1+C_{i(N-1)}^{(k)}\cdot CWKN}{CWN}}\hfill \end{array}\right.,$$

(22)

$$\left\{\begin{array}{c}CUK1=C_{1N}^{(n_0)}{C}_{Nj}^{(n_1)}-C_{NN}^{(n_1)}{C}_{1j}^{(n_0)}\\ CUKN=C_{N1}^{(n_1)}{C}_{1j}^{(n_0)}-C_{11}^{(n_0)}{C}_{Nj}^{(n_1)}\\ CUN=C_{11}^{(n_0)}{C}_{NN}^{(n_1)}-C_{1N}^{(n_0)}{C}_{N1}^{(n_1)}\end{array}\right.,$$

(23)

$$\left\{\begin{array}{c}CWK1=C_{1(N-1)}^{(n_{0+1})}{C}_{Nj}^{(n_{1+1})}-C_{N(N-1)}^{(n_{1+1})}{C}_{1j}^{(n_{0+1})}\\ CWKN=C_{N2}^{(n_{1+1})}{C}_{1j}^{(n_{0+1})}-C_{12}^{(n_{0+1})}{C}_{Nj}^{(n_{1+1})}\\ CWN=C_{12}^{(n_{0+1})}{C}_{N(N-1)}^{(n_{1+1})}-C_{1(N-1)}^{(n_{0+1})}{C}_{N2}^{(n_{1+1})}\end{array}\right.,$$

(24)

where $P_{ij}^{\left(k\right)}$, $Q_{ij}^{\left(k\right)}$, and $R_{ij}^{\left(k\right)}$ are the improved weighting coefficients for the m-th order derivatives of the displacement mode shape functions U(x), Ψ(x), W(x), respectively, at internal nodes, x = 0, and x = L, respectively.

3.3. Unified Discretized Equations via the MGDQ Method

Applying the MGDQ method to discretize Equation (18), the unified discretized equations for C-C, S-S, and C-S boundary beams are obtained as follows:

$$\begin{array}{l}{S}_1{\displaystyle \sum _{j=2}^{N-1}{P}_{ij}^{(2)}}{U}_j+S_3{\displaystyle \sum _{j=2}^{N-1}{Q}_{ij}^{(2)}}{\mathsf{\Psi }}_j-S_7{\displaystyle \sum _{j=3}^{N-2}{R}_{ij}^{(3)}}{W}_j\\ +{\omega }^2\left(I_0{U}_i+I_2{\mathsf{\Psi }}_i-I_6{\displaystyle \sum _{j=3}^{N-2}{R}_{ij}^{(1)}}{W}_j\right)=0\end{array}, (i=2,3,\dots ,N-1),$$

(25)

$$\begin{array}{l}{S}_3{\displaystyle \sum _{j=2}^{N-1}{P}_{ij}^{(2)}}{U}_j+S_6{\displaystyle \sum _{j=2}^{N-1}{Q}_{ij}^{(2)}}{\Psi }_j+S_8{\displaystyle \sum _{j=3}^{N-2}{R}_{ij}^{(3)}}{W}_j-S_0{\Psi }_i-\\ S_0{\displaystyle \sum _{j=3}^{N-2}{R}_{ij}^{(1)}}{W}_j+{\omega }^2\left(I_2{U}_i+I_5{\Psi }_i+I_7{\displaystyle \sum _{j=3}^{N-2}{R}_{ij}^{(1)}}{W}_j\right)=0\end{array}, (i=2,3,\dots ,N-1),$$

(26)

$$\begin{array}{l}{S}_7{\displaystyle \sum _{j=2}^{N-1}{P}_{ij}^{(3)}}{U}_j-S_8{\displaystyle \sum _{j=2}^{N-1}{Q}_{ij}^{(3)}}{\Psi }_j+S_9{\displaystyle \sum _{j=3}^{N-2}{R}_{ij}^{(4)}}{W}_j+\\ S_0\left({\displaystyle \sum _{j=2}^{N-1}{Q}_{ij}^{(1)}}{\Psi }_j+{\displaystyle \sum _{j=3}^{N-2}{R}_{ij}^{(2)}}{W}_j\right)-k_{\mathrm{w}}{W}_i-\mathrm{i}{c}_{\mathrm{d}}\omega W_i+\\ k_{\mathrm{p}}{\displaystyle \sum _{j=3}^{N-2}{R}_{ij}^{(2)}}{W}_j+{\omega }^2\left(I_0{W}_i-I_6{\displaystyle \sum _{j=2}^{N-1}{P}_{ij}^{(1)}}{U}_j-\right.\\ I_7{\displaystyle \sum _{j=2}^{N-1}{Q}_{ij}^{(1)}}{\Psi }_j+\left.I_8{\displaystyle \sum _{j=3}^{N-2}{R}_{ij}^{(2)}}{W}_j\right)=0\end{array}, (i=3,4,\dots ,N-2).$$

(27)

3.4. Complex Characteristic Frequency Equation

After discretization, the total number of algebraic equations in the system equals the number of unknown displacement mode shape quantities (3N − 8). This system can be expressed as a matrix equation:

$$\begin{gathered} \left\{[K]+\mathrm{i}\omega [C]+{\omega }^2[M]\right\}X=\mathbf{0}, \\ X={\{U_2,U_3,\cdots ,U_{N-1},{\mathsf{\Psi }}_2,{\mathsf{\Psi }}_3,\cdots ,{\mathsf{\Psi }}_{N-1},W_3,W_4,\cdots ,W_{N-2}\}}^T, \end{gathered}$$

(28)

where K is the sub-stiffness matrix, C is the sub-damping matrix, M is the sub-mass matrix, and X is the nodal displacement vector.

The non-trivial solution condition for Equation (28) yields the complex characteristic frequency equation:

$$\det \left(\left[\mathit{K}\right]+i\omega \left[\mathit{C}\right]+{\omega }^2\left[\mathit{M}\right]\right)=0.$$

(29)

Solving Equation (29) provides the frequency response of the damped free vibration of the FGM beam.

For generality, the following non-dimensional parameters are adopted:

$$\begin{gathered} \lambda ={\displaystyle \frac{L}{h}}, K_{\mathrm{w}}={\displaystyle \frac{k_{\mathrm{w}}{L}^4}{E_{\mathrm{c}}I}}, K_{\mathrm{p}}={\displaystyle \frac{k_{\mathrm{p}}{L}^2}{E_{\mathrm{c}}I}}, C_{\mathrm{d}}={\displaystyle \frac{c_{\mathrm{d}}{L}^2}{\sqrt{E_{\mathrm{c}}I{\rho }_{\mathrm{c}}A}}}, \Omega =\omega L^2\sqrt{{\displaystyle \frac{{\rho }_{c}A}{E_{c}I}}}, \\ \overline{\omega }=-\Omega (\zeta \pm \mathrm{i}\sqrt{1-{\zeta }^2}), {\Omega }_{\mathrm{R}}=-\Omega \zeta , {\Omega }_{\mathrm{I}}=\Omega \sqrt{1-{\zeta }^2}, \delta =-{\Omega }_R, \end{gathered}$$

(30)

where λ is the span-to-thickness ratio, K_w and K_p are non-dimensional foundation stiffness coefficients, C_d is the non-dimensional viscous external damping coefficient, I is the moment of inertia, A is the cross-sectional area, Ω is the undamped natural frequency, Ω_R is the real part of and reflects the damping-induced decay, Ω_I is the imaginary part of $\overline{\omega }$ and represents the underdamped natural frequency, ζ is the damping ratio, and δ is the decay coefficient.

From the non-dimensional frequency definition in Equation (30), the real part of A is evidently negative, reflecting the influence of the external damping on the decay characteristics of the displacement response. When 0 < ζ < 1, the imaginary part of $\overline{\omega }$ corresponds to the underdamped natural frequency, when ζ = 1, the system reaches the critical damping state, and when ζ > 0, the system is in an overdamped state.

4. Analysis and Discussion

In the examples considered in this study, N was taken as 17. An FGM beam composed of metal and ceramic was considered, with the following physical coefficients: E_m = 70 GPa; ρ_m = 2702 kg/m³; E_c = 380 GPa; ρ_c = 3960 kg/m³; and μ_m = μ_c = 0.3 [20].

4.1. Validation of Numerical Results

To address the lack of data on the natural frequencies of FGM beams under viscoelastic damping effects, this study established a multi-dimensional validation framework. First, theoretical self-consistency verification was performed by degenerating the three-parameter foundation model into the classical Euler–Bernoulli beam (K_w = K_p = C_d = 0) under a short and thick beam condition with a λ of 5 to establish a baseline model. Subsequently, the numerical convergence was verified. As shown in

Table 1. Comparison of dimensionless fundamental frequencies for undamped free vibration in FGM beams (λ = 5).

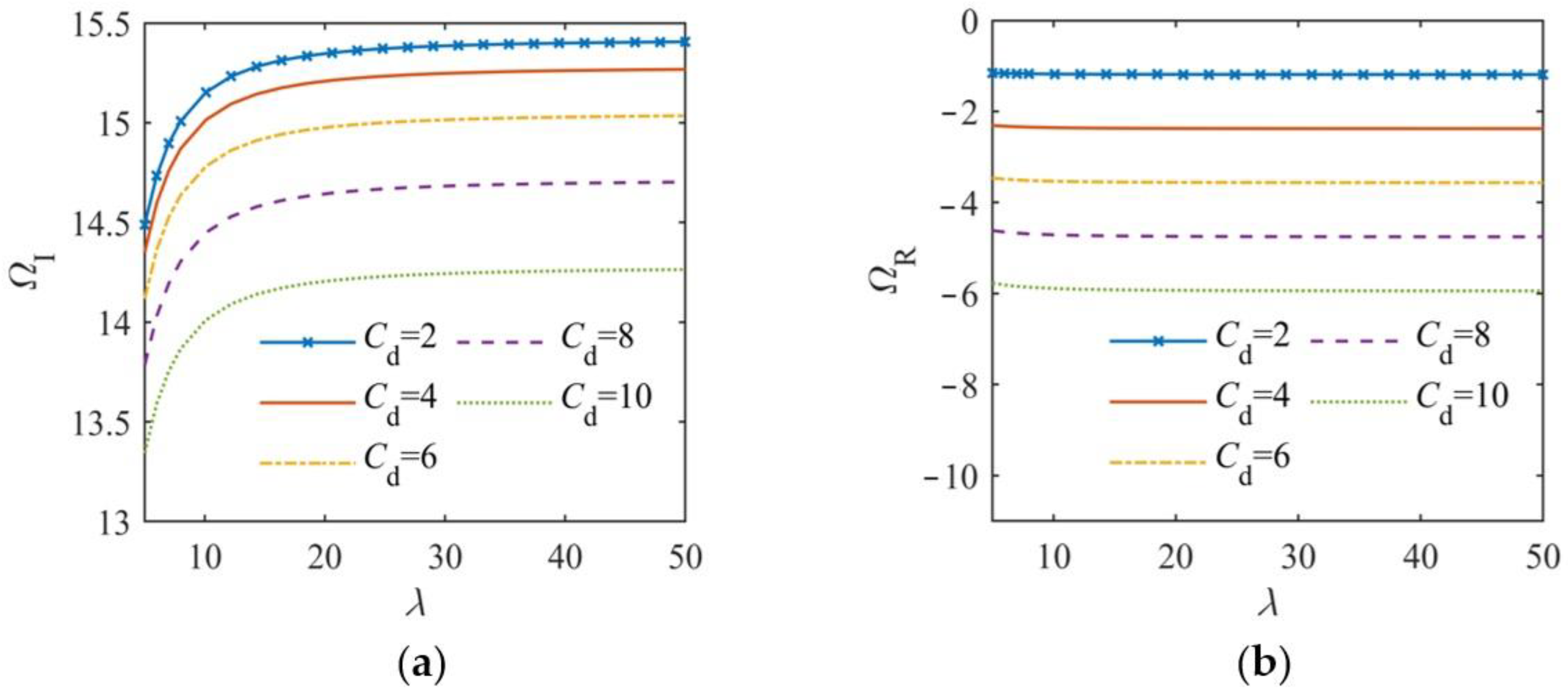
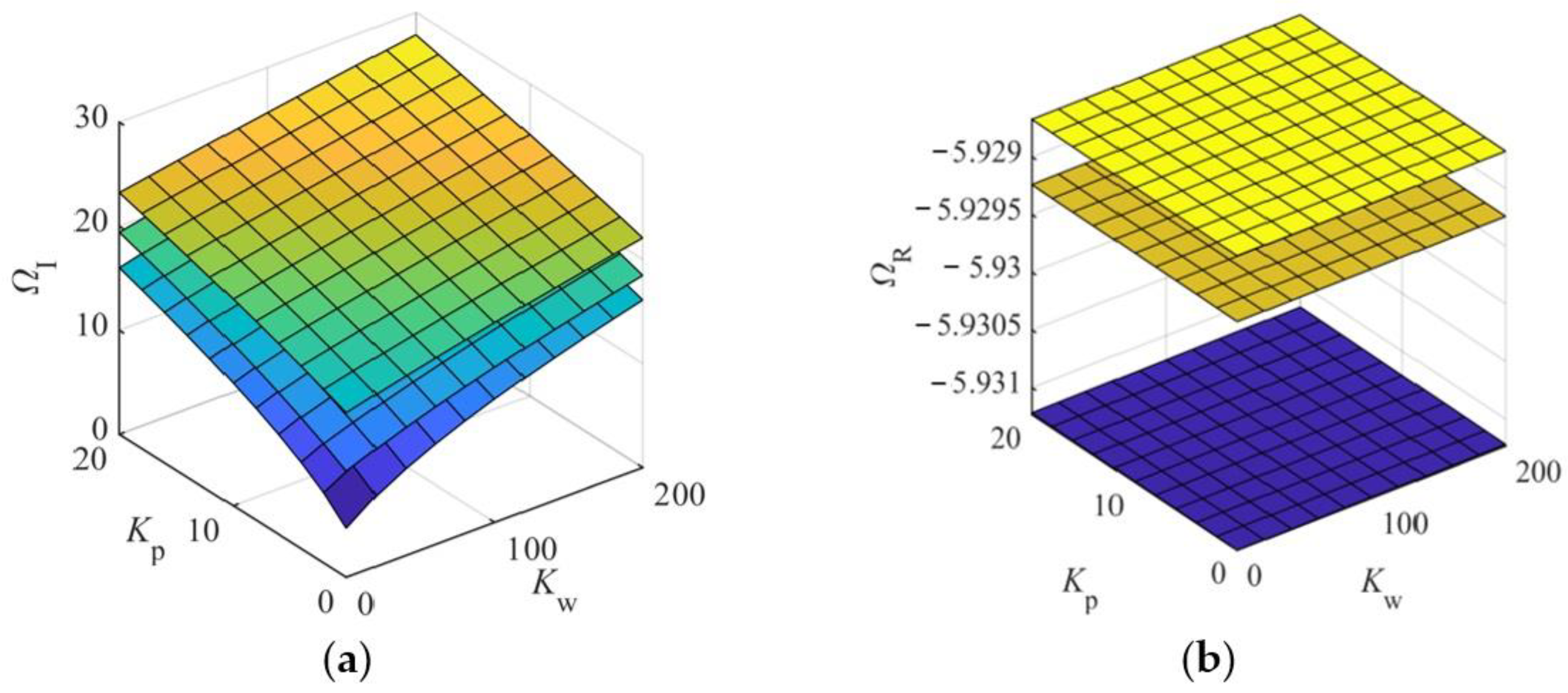
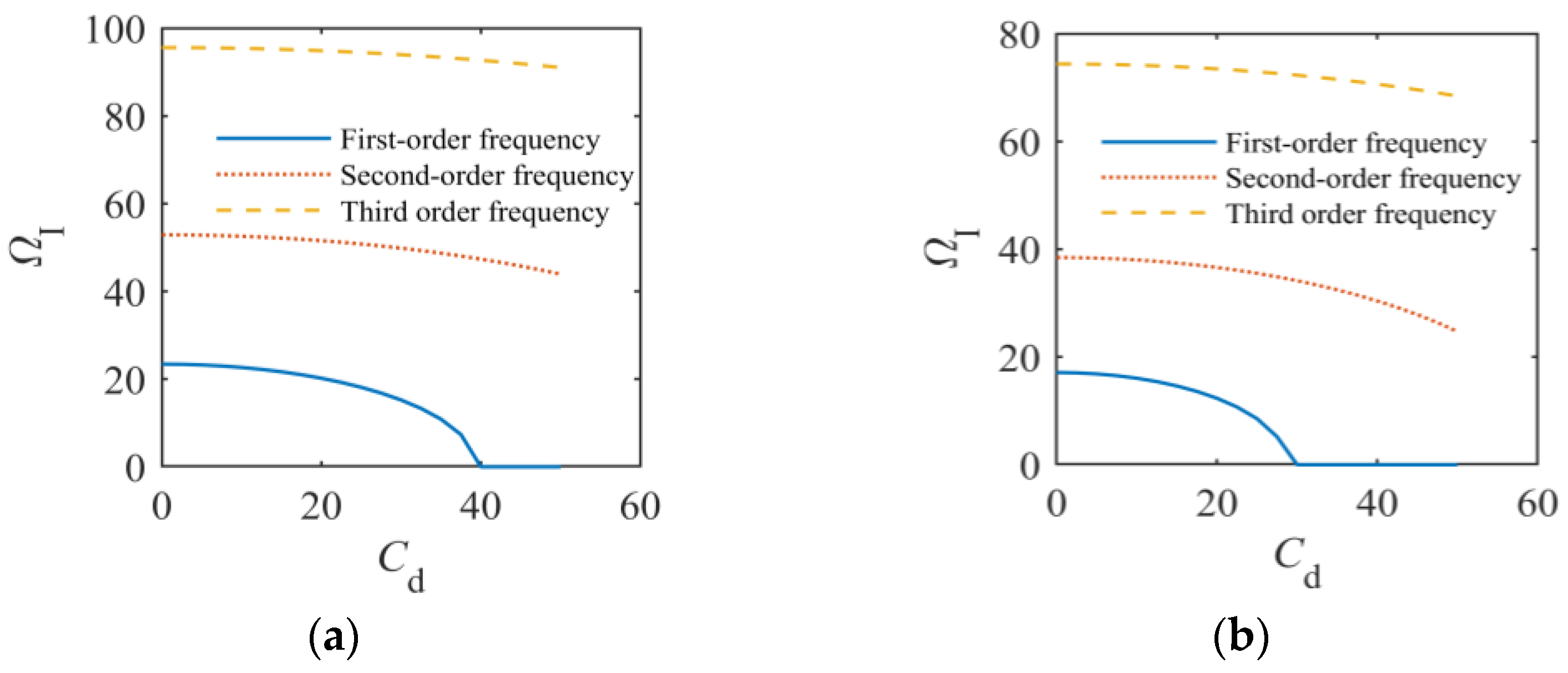
Boundary	Method	p = 0	p = 0.2	p = 1	p = 10
C-C	Ref. [14] *	10.0705	9.46641	7.95034	6.16515
	This study	10.0756	9.46932	7.95395	6.16797
S-S	Ref. [14] *	5.15274	4.80924	3.99042	3.28160
	This study	5.15274	4.80807	3.99041	3.28160

* The data source is open access and comes from Ref. [14].

, when Reddy’s third-order shear deformation theory (TBT, n = 3) was adopted and the dimensionless frequency definition was consistent with [14], and the relative errors of the dimensionless fundamental frequencies under C-C and S-S boundary conditions were both less than 0.5%, thereby significantly outperforming the 1.2% error level of the traditional differential quadrature method. This dual verification mechanism not only confirmed the computational stability of the MGDQ algorithm under geometrically constrained conditions but also revealed the physical consistency of the viscoelastic parameter modeling in this study, thus providing a reliable numerical benchmark for subsequent research on complex damping scenarios.

4.2. Influence of GBT Order n on Output Response Parameters

Next, the influence of the GBT order n on the free vibration response parameters of the three-parameter viscoelastic foundation FGM beam was explored, considering p = 1, λ = 5, K_w = 25, K_p = 5, and C_d = 10. Figure 2 illustrates the relationship curves of the dimensionless characteristic fundamental frequency (imaginary part Ω_I, real part Ω_R, and damping ratio ζ versus the GBT order n) for a clamped FGM short beam in an underdamped state.

From Figure 2a, it can be observed that Ω_I exhibited minor fluctuations and gradually stabilized as the positive integer n increased, eventually approaching the prediction of the FSBT. The maximum Ω_I value occurred at n = 2, and the minimum at n = 3, with an extreme difference of 0.43006. Additionally, for λ = 5 (a short and thick beam), the CBT neglected the effects of shear deformation, thereby overestimating the overall beam stiffness. Compared to the FSBT and TBT, the CBT overestimated Ω_I (underestimating the period of underdamped decay vibration in the FGM beam). From Figure 2b, unlike the undamped free vibration, the real part Ω_R of the fundamental frequency in the underdamped state was negative, indicating amplitude decay due to damping. Figure 2b,c show that, except for n = 1, the variations in the predicted values of Ω_R and ζ were negligible with changes in n. However, the CBT significantly overestimated Ω_R (underestimating the decay coefficient δ), thereby overestimating the amplitude; furthermore, it underestimated ζ, leading to an overestimation of the fundamental frequency Ω_I. Compared with the TBT, the relative errors in Ω_R and ζ predicted by the CBT reached 13.96% and 13.81%, respectively, which are not negligible.

4.3. Influence of Other Factors on Output Response Parameters

All examples considered herein adopted TBT to explore the influence of parameters, such as beam boundary conditions C_d, p, λ and foundation elastic stiffness on the free vibration response parameters of the FGM beam. Figure 3 describes the relationships between Ω_I, Ω_R, and ζ, and C_d for FGM beams under the three boundary conditions. With given parameters p = 1, λ = 20, K_w = 25, K_p = 5, the following observations were made.

From Figure 3a: Ω_I monotonically decreased as C_d increased. When C_d was equal to critical damping C_cr, Ω_I reached its minimum value. Increasing C_d further led to an overdamped state, where Ω_I approached zero, thereby indicating purely decaying motion of the beam. Under different boundary conditions, Ω_I was largest for C-C beams, intermediate for C-S beams, and smallest for S-S beams. The values of C_cr also varied across the boundary conditions, with C-C beams having the highest C_cr, S-S beams the lowest, and C-S beams falling in between.

From Figure 3b: In the underdamped stage, Ω_R was negative for all three boundary conditions and decreased linearly with increasing C_d. This implies that the decay coefficient increased with C_d, reflecting a gradual reduction in the vibrational energy. In other words, the underdamped free vibration of the FGM beam manifested as an amplitude-decaying motion, with densely packed linear segments in the Ω_R–C_d plot.

From Figure 3c: ζ of the three boundary-conditioned FGM beams increased linearly with C_d. For small damping (e.g., C_d < 5), boundary conditions had limited influence on ζ. For larger damping (e.g., C_d > 10), at the same C_d value, the S-S beams exhibited the highest ζ, C-C beams the lowest, and C-S beams falling in between.

Figure 4 shows the relationship curves of Ω_I, Ω_R, and ζ versus C_d for clamped FGM beams with different gradient index values. The parameters were set as λ = 20, K_w = 25, and K_p = 5.

From Figure 4a: For p = 0, 0.2, 1, and 10, Ω_I decreased monotonically with increasing C_d in the underdamped stage until Ω_I = 0 at their respective critical damping states. At the same C_d, a larger p resulted in a smaller Ω_I and lower C_cr. When the FGM beam entered the overdamped state, Ω_I = 0.

From Figure 4b: At C_d = 0, Ω_R = 0. In the underdamped stage (0 < C_d < C_cr), Ω_R was negative and decreased linearly with C_d. For the same C_d, a larger p led to a smaller Ω_R. In the overdamped stage (C_d > C_cr), Ω_R assumed two negative real numbers, and the Ω_R–C_d curves opened to the right with significant separation, indicating a pronounced influence of p on displacement decay. Notably, larger p-values resulted in wider curve openings. Furthermore, C_cr corresponded to the abscissa of the intersection point between the underdamped linear segment and overdamped curve segment of Ω_R–C_d.

From Figure 4c: ζ increased linearly with C_d. For small damping (e.g., 0 < C_d < 2), the ζ–C_d linear segments were densely packed, suggesting a limited influence of p on ζ. However, for large damping (e.g., C_d > 20), the linear segments diverged significantly, highlighting a strong dependence of ζ on p.

In general, small damping (e.g., C_d < 5) had a negligible effect on the natural frequencies and could be ignored in routine calculations. However, the influence of small damping on amplitude decay could not be neglected, as the amplitudes diminished exponentially.

Figure 5 illustrates the relationship curves of Ω_I and Ω_R versus λ for a clamped-simply supported FGM beam in an underdamped state, with parameters p = 1, K_w = 25, and K_p = 5.

From Figure 5a: As λ increased, the Ω_I curves initially rose significantly. When λ > 20, the increase in Ω_I slowed down, indicating that the period of underdamped decay vibration decreased with λ, but became less sensitive for λ > 20. Additionally, for the same λ, a larger C_d resulted in a smaller Ω_I.

From Figure 5b: The Ω_R–λ curves are nearly flat, suggesting that λ had minimal influence on the amplitude decay. For the same λ, a larger C_d led to a smaller Ω_R (i.e., a larger decay coefficient δ), thereby accelerating the amplitude attenuation.

Figure 6 shows the relationship curves of Ω_I and Ω_R versus p for a clamped FGM beam in an underdamped state, with parameters λ = 20, K_w = 25, and K_p = 5.

From Figure 6a: The Ω_I curves monotonically decreased as p increased (i.e., the ceramic content decreased). The most significant reduction occurred in the range 0 ≤ p ≤ 2, which could be attributed to the rapid weakening of the FGM beam’s overall stiffness due to the sharp decline in its alumina (Al₂O₃) content.

From Figure 6b: When C_d = 0, Ω_R = 0 (a horizontal line), indicating undamped free vibration with a constant amplitude. With damping, Ω_R decreased with p, most notably in the range 0 ≤ p ≤ 2. For p > 2, the decrease in Ω_R slowed down, reflecting that amplitude attenuation diminished gradually as the ceramic content reduced further.

Figure 7 illustrates the relationship surfaces of Ω_I and Ω_R versus the Winkler–Pasternak foundation elastic stiffness coefficients K_w and K_p for the three typical boundary conditions (C-C, C-S, S-S) of the FGM beams in an underdamped state, with parameters p = 1, λ = 20, and C_d =10.

From Figure 7a: As K_w and K_p increased, Ω_I rose, leading to a gradual reduction in the period of underdamped decay vibration. For a given foundation elastic stiffness, the Ω_I surface values were significantly higher for the C-C boundaries, intermediate for C-S boundaries, and lowest for S-S boundaries. This was attributed to the enhanced equivalent stiffness of the beam structure under strongly constrained boundaries, which would increase the vibrational frequency.

From Figure 7b: The Ω_R surfaces for all three boundary conditions are flat planes, indicating that increasing the foundation elastic stiffness had no effect on amplitude attenuation. Additionally, the Ω_R values were slightly lower for the C-C boundaries, slightly higher for the S-S boundaries, and intermediate for the C-S boundaries. However, the differences in Ω_R across the boundaries were minimal, reaffirming that the boundary conditions had a negligible influence on the amplitude attenuation in the underdamped states.

Figure 8 shows the relationship curves of Ω_I versus C_d for the clamped (C-C) and simply-supported (S-S) FGM beams, with parameters p = 1, λ = 20, K_w = 100, and K_p = 10.

The first three frequencies of the FGM beams under both these boundary conditions decreased gradually with increasing C_d. The first-order frequency decreased most rapidly, reaching zero at C_cr, after which it remained at zero in the overdamped state. However, the second- and third-order frequencies did not reach their respective critical damping states. This indicates that external damping effects more readily attenuated low-order vibrational mode frequencies, while higher-order frequencies were less significantly affected.

5. Conclusions

Based on the n-th order GBT, a systematic dynamic model was established under the Hamiltonian framework. The free vibration characteristics of a three-parameter viscoelastic foundation FGM beam were numerically analyzed using the MGDQ method. The key results are as follows:

(1) Compared to the FSBT and TBT, the CBT overestimated the frequency of underdamped free vibration in short FGM beams, thereby underestimating the period. It significantly underestimated the decay coefficient, leading to an overestimation of the vibration amplitude; moreover, it notably underestimated the damping ratio.

(2) As the external damping coefficient increased, the FGM beam transitioned through underdamped, critical damping, and overdamped states. The characteristic frequency equation exhibited complex roots of different forms. Vibrational behavior was observed only in the underdamped state.

(3) The C-C beams exhibited lower damping ratios and higher critical damping values, while the S-S beams showed higher damping ratios and lower critical damping values; the C-S beams lay between these extremes, thus demonstrating intermediate damping characteristics.

(4) The natural frequencies decreased with increasing external damping coefficient (C_d) and gradient index (p), but increased with span-to-thickness ratio (λ) and foundation elastic stiffness (K_w, K_p). Amplitude decay is significantly influenced by C_d and p, while boundary conditions, λ, and foundation stiffness have minimal impact on attenuation.

(5) Small damping (C_d < 5) negligibly affected the natural frequencies and can be ignored in routine calculations. However, its effect on the amplitude decay remained significant due to exponential attenuation.