Performance Evaluation of Current Design Models in Predicting Shear Resistance of UHPC Girders
Abstract
1. Introduction
2. Shear Resistance According to Various Prediction Models
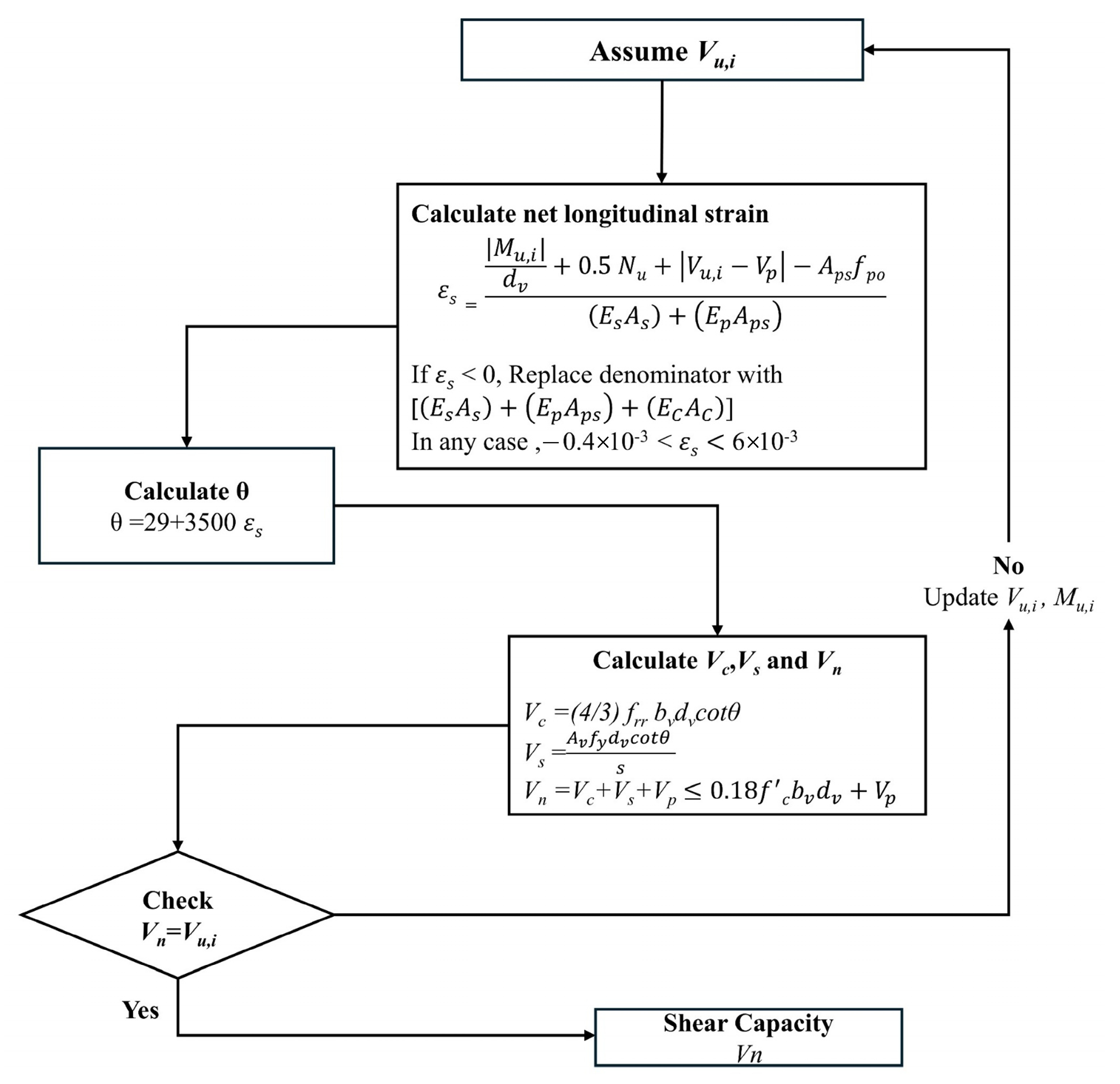
2.1. FHWA-HRT-23-077, 2023
2.2. ePCI-UHPC, 2021
2.3. French Standard, NF P 18-710, 2016
2.4. Canadian Standard CSA A23.3-04
2.5. Eurocode 2 and German DAfStb Guideline, 2022
2.6. Summary of the Prediction Models
- -
- Generally, the mentioned models agree that the shear resistance is composed of contributions from the tensile resistance of concrete, fibers, shear reinforcement, and prestressing. However, the FHWA, ePCI, and CSA methods have one term for the tensile resistance of concrete and steel fibers combined, while the AFGC and the DAfStb methods have separate terms for each.
- -
- It is worth mentioning that the FHWA method adopts the concept of localization tensile stress for the tensile resistance of concrete, which should be evaluated experimentally. The ePCI method adopts a fixed minimum residual tensile stress for UHPC (frr = 5.2 MPa). In the other codes, the tensile stress is related to the compressive strength of UHPC.
- -
- Regarding the inclination angle of the compression strut θ, the FHWA method adopts an iterative solution to determine it, while the ePCI and the CSA method adopt a similar equation form. In the three methods, the angle depends on the strain of the longitudinal reinforcement, εs. The AFGC method adopts a fixed minimum angle of 30 degrees. However, the DAfStb method includes the effect of the shear span-to-depth ratio on the inclination angle θ.
- -
- In the shear reinforcement contributions, all methods assume yielding stress in the shear reinforcement except for the FHWA method, where an iterative solution is required to determine the stress in the shear reinforcement.
- -
- In the FHWA, ePCI, and CSA methods, the effect of the prestressing level on the shear capacity of UHPC is included in determining the strain of the longitudinal reinforcement, εs, and as a vertical component resisting vertical shear, Vp. In the AFGC and the DAfStb methods, the prestressing level is included in the concrete contribution equation ().
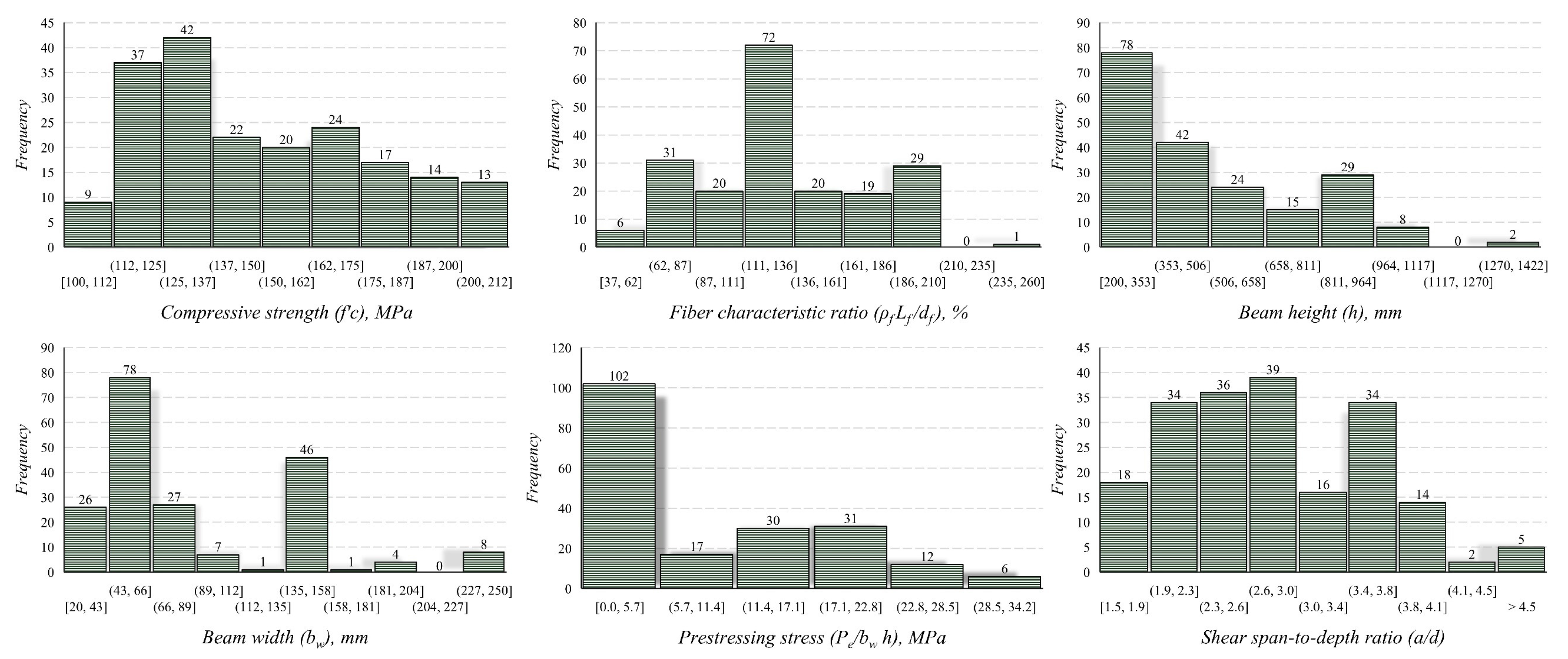
3. Database Analysis
4. Localization Tensile Stress and Strain for the FHWA Method
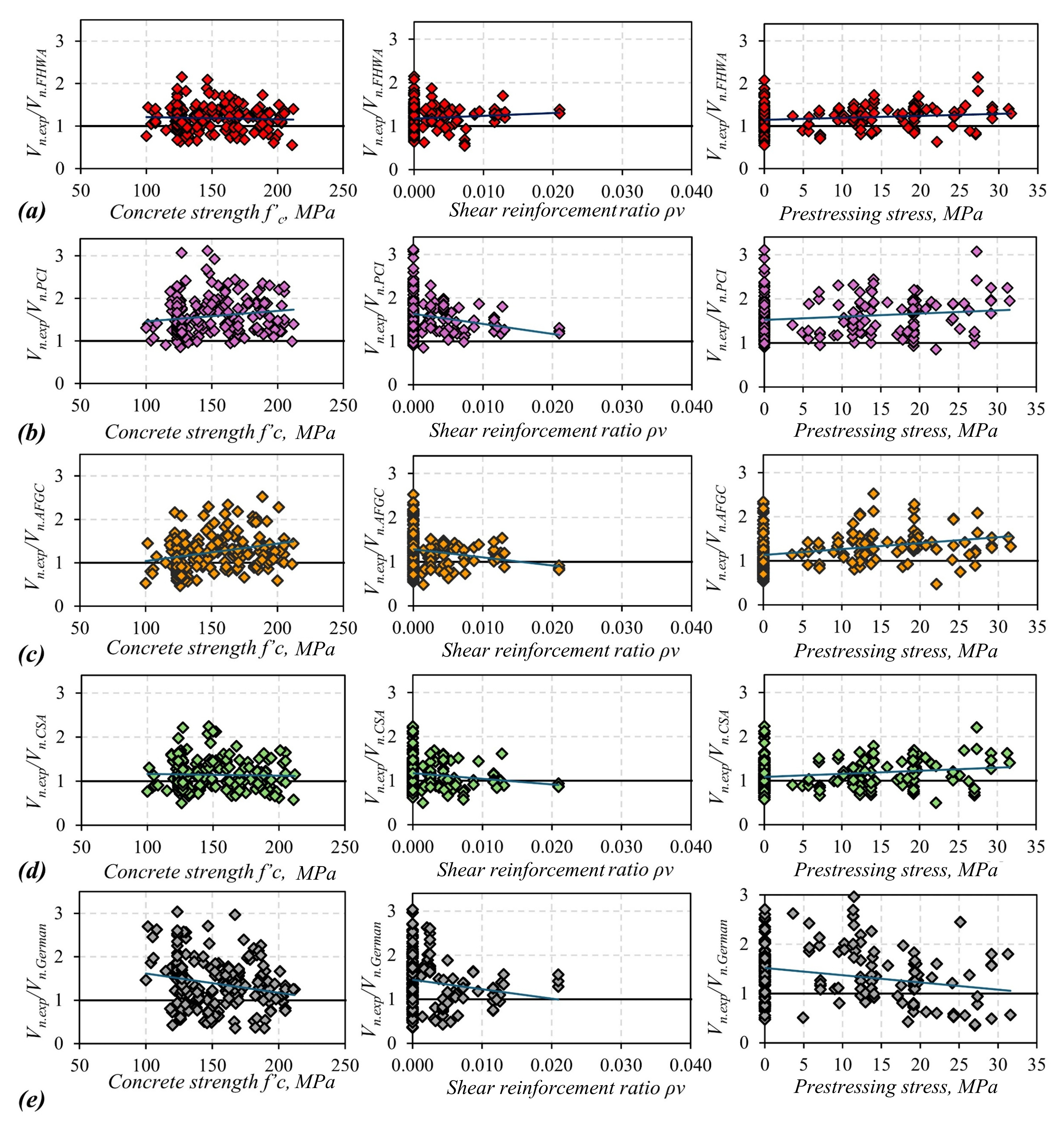
5. Performance and Comparison of Prediction Models
6. Conclusions
- The FHWA, ePCI, and CSA methods are based on modifications of the MCFT. On the other hand, the AFGC and DAfStb methods were shown to have separate terms for the concrete and steel fiber tensile contributions. The angle of inclination of the diagonal compressive strut (θ) in the FHWA, ePCI, and CSA methods is determined using the strain of the longitudinal reinforcement (εs), while the AFGC method uses a fixed minimum angle of 30°, and the DAfStb method incorporates the span-to-depth ratio (a/d). In addition, it was shown that all methods assume yielding stress in shear reinforcement except for the FHWA method, which determines the strain of the shear reinforcement.
- To determine the shear capacity according to the FHWA procedure, the localization strength and strain (ftloc, εt,loc) must be determined experimentally. Conversely, ftloc and εt,loc had not been determined for most of the specimens in the database. For that reason, a prediction model ft,loc was developed based on the concrete and steel fiber properties. Regarding the localization strain, a fixed value was assumed (εt,loc =0.004) for all specimens.
- The FHWA method showed a superior performance compared to other models in terms of statistical measures and consistent prediction conservatism across variables ranges. The FHWA method resulted in an Avg. of 1.19, SD of 0.29, and RRMSE of 35.7% for the strength ratios (Vexp/Vpred.). Out of 198 specimens, only 14 had a shear strength ratio of either more than 2.0 or below 0.75.
- The ePCI method showed the highest conservatism with an Avg. of 1.58 due to limiting the UHPC residual tensile strength to 5.2 MPa. The ePCI method resulted in 28 specimens with a shear strength ratio > 2.0 and no specimens falling below 0.75.
- The DAfStb method showed the highest SD and CoV, indicating the lowest prediction accuracy and the greatest scatter of the data. This method also accounted for the largest number of specimens having shear strength ratios above 2.0 and below 0.75. In addition, this method showed a reduction in conservatism at high geometrical or material properties values, including the shear span-to-depth ratio.
- It can be said that the ePCI, AFGC, and CSA methods showed similar behavior with different degrees of conservatism; they resulted in Avg. shear strength ratios of 1.58, 1.24, and 1.14, respectively.
- Except for the FHWA method, all methods showed a reduction in conservatism at a high transverse reinforcement ratio. This can be attributed to adopting yield stress for transverse reinforcement compared to the FHWA method which checks its strain.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A

References
- Resplendino, J. Introduction: What is a UHPFRC? In Designing and Building with UHPFRC; Wiley-ISTE: London, UK, 2011; pp. 3–14. [Google Scholar]
- Graybeal, B.; Brühwiler, E.; Kim, B.S.; Toutlemonde, F.; Voo, Y.L.; Zaghi, A. International perspective on UHPC in bridge engineering. J. Bridge Eng. 2020, 25, 04020094. [Google Scholar] [CrossRef]
- Buttignol, T.E.T.; Sousa, J.L.A.O.; Bittencourt, T.N. Ultra High-Performance Fiber-Reinforced Concrete (UHPFRC): A review of material properties and design procedures. Rev. IBRACON Estrut. Mater. 2017, 10, 957–971. [Google Scholar] [CrossRef]
- ACI Committee 239. Ultra-High-Performance Concrete: An Emerging Technology Report; (ACI 239R-18); American Concrete Institute: Farmington Hills, MI, USA, 2018. [Google Scholar]
- El-Helou, R.G.; Graybeal, B.A. Shear behavior of ultrahigh-performance concrete pretensioned bridge girders. J. Struct. Eng. 2022, 148, 04022017. [Google Scholar] [CrossRef]
- Wille, K.; Tue, N.V.; Parra-Montesinos, G.J. Fiber distribution and orientation in UHP-FRC beams and their effect on backward analysis. Mater. Struct. 2014, 47, 1825–1838. [Google Scholar] [CrossRef]
- Barros, J.A.O.; Antunes, J.A.B. Experimental characterization of the flexural behaviour of steel fibre reinforced concrete according to RILEM TC 162-TDF recommendations. RILEM TC 2003, 162, 77–89. [Google Scholar]
- Morcous, G.; Maguire, M.; Tadros, M.K. Shear capacity of ultra-high-performance concrete I-girders with orthogonal welded wire reinforcement. Spec. Publ. 2009, 265, 511–532. [Google Scholar]
- Baby, F.; Billo, J.; Renaud, J.C.; Massotte, C.; Marchand, P.; Toutlemonde, F.; Lussou, P. Shear resistance of ultra high performance fibre-reinforced concrete I-beams. In Fracture Mechanics of Concrete and Concrete Structures-High Performance, Fiber Reinforced Concrete, Special Loadings and Structural Applications; Korea Concrete Institute: Seoul, Republic of Korea, 2010; pp. 1411–1417. [Google Scholar]
- Yang, I.H.; Joh, C.; Kim, B.S. Shear behaviour of ultra-high-performance fibre-reinforced concrete beams without stirrups. Mag. Concr. Res. 2012, 64, 979–993. [Google Scholar] [CrossRef]
- Baby, F.; Marchand, P.; Toutlemonde, F. Shear behavior of ultrahigh performance fiber-reinforced concrete beams. I: Experimental investigation. J. Struct. Eng. 2014, 140, 04013111. [Google Scholar] [CrossRef]
- Zheng, H.; Fang, Z. Experimental study on shear behavior of prestressed ultra-high performance concrete I-girders. IABSE Symp. Rep. 2016, 106, 775–785. [Google Scholar]
- Jin, L.Z.; Chen, X.; Fu, F.; Deng, X.F.; Qian, K. Shear strength of fibre-reinforced reactive powder concrete I-shaped beam without stirrups. Mag. Concr. Res. 2020, 72, 1112–1124. [Google Scholar] [CrossRef]
- Pourbaba, M.; Sadaghian, H.; Mirmiran, A. A comparative study of flexural and shear behavior of ultra-high-performance fiber-reinforced concrete beams. Adv. Struct. Eng. 2019, 22, 1727–1738. [Google Scholar] [CrossRef]
- Ţibea, C.; Bompa, D.V. Ultimate shear response of ultra-high-performance steel fibre-reinforced concrete elements. Arch. Civ. Mech. Eng. 2020, 20, 49. [Google Scholar] [CrossRef]
- Metje, K.; Leutbecher, T. Experimental investigations on the shear bearing behavior of prestressed ultra—High performance fiber—Reinforced concrete beams with compact cross—Section. Struct. Concr. 2021, 22, 3746–3762. [Google Scholar] [CrossRef]
- Feng, J.; Li, P.; Wu, J.; Jiang, H.; Tian, Y.; Sun, X. Shear behavior of externally prestressed UHPC beams without stirrups. Case Stud. Constr. Mater. 2023, 18, e01766. [Google Scholar] [CrossRef]
- Li, P.; Cheng, Q.; Chen, N.; Tian, Y.; Fang, J.; Jiang, H. Experimental Study on Shear Behavior of Non-Stirrup Ultra-High Performance Concrete Beams. Materials 2023, 16, 4177. [Google Scholar] [CrossRef]
- Ahmed, F.B.; Biswas, R.K.; Sen, D.; Tasnim, S. Flexural and Shear Strengthening of Reinforced-Concrete Beams with Ultra-High-Performance Concrete (UHPC). Constr. Mater. 2024, 4, 468–492. [Google Scholar] [CrossRef]
- Binard, J.P. UHPC: A game-changing material for PCI bridge producers. PCI J. 2017, 62, 34–46. [Google Scholar] [CrossRef]
- Kodsy, A.; Morcous, G. Shear strength of ultra-high-performance concrete (UHPC) beams without transverse reinforcement: Prediction models and test data. Materials 2022, 15, 4794. [Google Scholar] [CrossRef]
- Hematibahar, M.; Kharun, M.; Beskopylny, A.N.; Stel’makh, S.A.; Shcherban’, E.M.; Razveeva, I. Analysis of models to predict mechanical properties of high-performance and ultra-high-performance concrete using machine learning. J. Compos. Sci. 2024, 8, 287. [Google Scholar] [CrossRef]
- Haruna, S.I.; Ibrahim, Y.E.; Umar, I.K. Machine Learning Approach for Prediction and Reliability Analysis of Failure Strength of U-Shaped Concrete Samples Joined with UHPC and PUC Composites. J. Compos. Sci. 2025, 9, 23. [Google Scholar] [CrossRef]
- Graybeal, B.A.; El-Helou, R. Structural Design with Ultra-High Performance Concrete; FHWA-HRT-23-077; U.S. Department of Transportation, Federal Highway Administration, Office of Infrastructure Research and Development: Washington, DC, USA, 2023.
- Tadros, M.; Lawler, J.; Abo El-Khier, M.; Gee, D.; Kurt, A.; Lucier, G.; Wagner, E. Implementation of ultra-high-performance concrete in long-span precast pretensioned elements for concrete buildings and bridges. PCI J. 2021. [Google Scholar]
- Toutlemonde, F.; Kretz, T.; Généreux, G.; Resplendino, J.; Pillard, W.; Guérinet, M.; Rougeau, P. French Standards for Ultra-High Performance Fiber-Reinforced Concrete (UHPFRC). In High Tech Concrete: Where Technology and Engineering Meet; Springer: Cham, Switzerland, 2018; pp. 1601–1609. [Google Scholar]
- CSA. Design of Concrete Structures; A23.3-04; Canadian Standards Association: Rexdale, ON, Canada, 2004; p. 232. [Google Scholar]
- Metje, K.; Leutbecher, T. Verification of the shear resistance of UHPFRC beams–Design method for the German DAfStb Guideline and database evaluation. Eng. Struct. 2023, 277, 115439. [Google Scholar] [CrossRef]
- Guide Specifications for Structural Design with Ultra-High Performance Concrete, 1st ed.; American Association of State Highway and Transportation Officials (AASHTO): Washington, DC, USA, 2024.
- AASHTO. Standard Method of Test for Uniaxial Tensile Response of Ultra-High-Performance Concrete; T 397; American Association of State Highway and Transportation Officials: Washington, DC, USA, 2022. [Google Scholar]
- Voo, Y.L.; Poon, W.K.; Foster, S.J. Shear strength of steel fiber-reinforced ultrahigh-performance concrete beams without stirrups. J. Struct. Eng. 2010, 136, 1393–1400. [Google Scholar] [CrossRef]
- Voo, Y.L.; Foster, S.J.; Gilbert, R.I. Shear strength of fiber reinforced reactive powder concrete prestressed girders without stirrups. J. Adv. Concr. Technol. 2006, 4, 123–132. [Google Scholar] [CrossRef]
- Graybeal, B.A. Material Property Characterization of Ultra-High Performance Concrete; FHWA-HRT-06-103; U.S. Federal Highway Administration, Office of Infrastructure Research and Development: Washington, DC, USA, 2006.
- Yao, D.; Jia, J.; Wu, F.; Yu, F. Shear performance of prestressed ultra-high strength concrete encased steel beams. Constr. Build. Mater. 2014, 52, 194–201. [Google Scholar] [CrossRef]
- Kurt, A. Implementation of Ultra-High Performance Concrete in Long-Span Precast Pretensioned Structural Elements for Buildings. Ph.D. Thesis, North Carolina State University, Raleigh, NC, USA, 2021. [Google Scholar]
- Lakavath, C.; Prakash, S.S. Influence of fiber dosage, fiber type, and level of prestressing on the shear behavior of UHPFRC I-girders. Eng. Struct. 2024, 300, 117146. [Google Scholar] [CrossRef]
- Feng, W.; Feng, H.; Zhou, Z.; Shi, X. Analysis of the shear capacity of ultrahigh performance concrete beams based on the modified compression field theory. Adv. Mater. Sci. Eng. 2021, 2021, 5569733. [Google Scholar] [CrossRef]
- Schramm, N.; Fischer, O. Querkraftversuche an profilierten Spannbetonträgern aus UHPFRC. Beton Stahlbetonbau 2019, 114, 641–652. [Google Scholar] [CrossRef]
- Wipf, T.J.; Phares, B.M.; Sritharan, S.; Degen, B.E.; Giesmann, M.T. Design and Evaluation of a Single-Span Bridge Using Ultra-High Performance Concrete; IHRB Project TR-529; Iowa State University, Institute for Transportation: Ames, IA, USA, 2009. [Google Scholar]
- Lim, W.Y.; Hong, S.G. Shear tests for ultra-high performance fiber reinforced concrete (UHPFRC) beams with shear reinforcement. Int. J. Concr. Struct. Mater. 2016, 10, 177–188. [Google Scholar] [CrossRef]
- Mészöly, T.; Randl, N. Shear behavior of fiber-reinforced ultra-high performance concrete beams. Eng. Struct. 2018, 168, 119–127. [Google Scholar] [CrossRef]
- Son, J.; Beak, B.; Choi, C. Experimental study on shear strength for ultra-high performance concrete beam. In Proceedings of the 18th International Conference on Composites Materials (ICCM-18), Jeju, Republic of Korea, 21–26 August 2011. [Google Scholar]
- Cao, X.; Deng, X.; Jin, L.Z.; Fu, F.; Qian, K. Experimental study on shear capacity of cementitious composite RPC beams using high strength steel. Proc. Inst. Civil Eng. Struct. Build. 2019, 174, 276–291. [Google Scholar] [CrossRef]
- Wu, X.; Han, S.M. First diagonal cracking and ultimate shear of I-shaped reinforced girders of ultra-high performance fiber reinforced concrete without stirrup. Int. J. Concr. Struct. Mater. 2009, 3, 47–56. [Google Scholar] [CrossRef]
- Pansuk, W.; Nguyen, T.N.; Sato, Y.; Den Uijl, J.A.; Walraven, J.C. Shear capacity of high performance fiber reinforced concrete I-beams. Constr. Build. Mater. 2017, 157, 182–193. [Google Scholar] [CrossRef]
- Yang, J.; Doh, J.H.; Yan, K.; Zhang, X. Experimental investigation and prediction of shear capacity for UHPC beams. Case Stud. Constr. Mater. 2022, 16, e01097. [Google Scholar] [CrossRef]
- Wang, Q.; Song, H.L.; Lu, C.L.; Jin, L.Z. Shear performance of reinforced ultra-high performance concrete rectangular section beams. Structures 2020, 27, 1184–1194. [Google Scholar] [CrossRef]
- Lee, J.H.; Hong, S.G. Shear strength of ultra-high performance fiber-reinforced concrete (UHPFRC) I-shaped beams without stirrup. J. Korea Concr. Inst. 2017, 29, 53–64. [Google Scholar] [CrossRef]
- Telleen, K.; Noshiravani, T.; Galrito, R.; Brühwiler, E. Experimental investigation into the shear resistance of a reinforced UHPFRC web element. In Proceedings of the 8th fib PhD Symposium in Kgs, Lyngby, Denmark, 20–23 June 2010. [Google Scholar]
- Thiemicke, J. Zum Querkrafttragverhalten von UHPC-Balken Mit Kombinierter Bewehrung aus Stahlfasern und Stabstahl; Kassel University Press GmbH: Kassel, Germany, 2015; Volume 25. [Google Scholar]
- El-Helou, R.G.; Haber, Z.B.; Graybeal, B.A. Mechanical behavior and design properties of ultra-high-performance concrete. ACI Mater. J. 2022, 119, 1. [Google Scholar]
- Yavaş, A.; Hasgul, U.; Turker, K.; Birol, T. Effective fiber type investigation on the shear behavior of ultra-high-performance fiber-reinforced concrete beams. Adv. Struct. Eng. 2019, 22, 1591–1605. [Google Scholar] [CrossRef]
- Valentim, D.B.; Aaleti, S.; Kreger, M. Evaluation of test methods to characterize tensile strength of ultra-high-performance concrete. ACI Mater. J. 2022, 119, 4. [Google Scholar]
- Haber, Z.B.; De la Varga, I.; Graybeal, B.A.; Nakashoji, B.; El-Helou, R. Properties and Behavior of UHPC-Class Materials; FHWA-HRT-18-036; United States Federal Highway Administration. Office of Infrastructure Research and Development: McLean, VA, USA, 2018.
- Chen, S.; Zhang, R.; Jia, L.J.; Wang, J.Y. Flexural behaviour of rebar-reinforced ultra-high-performance concrete beams. Mag. Concr. Res. 2018, 70, 997–1015. [Google Scholar] [CrossRef]
- Yoo, D.Y.; Sohn, H.K.; Borges, P.H.; Fediuk, R.; Kim, S. Enhancing the tensile performance of ultra-high-performance concrete through strategic use of novel half-hooked steel fibers. J. Mater. Res. Technol. 2020, 9, 2914–2925. [Google Scholar] [CrossRef]
- Yoo, D.Y.; Banthia, N.; Yoon, Y.S. Experimental and numerical study on flexural behavior of UHPFRC beams with low reinforcement ratios. Can. J. Civ. Eng. 2017, 44, 18–28. [Google Scholar] [CrossRef]




| Shear Span | a/d ≥ 2 | 0.5 ≤ a/d < 2 |
|---|---|---|
| Fiber contribution | ||
| Stirrup contribution | = 1.2 + 2.4 |
| Reference | # of Tests | Section | f’c (MPa) | Vf lf/df | a/d | h (mm) | bw (mm) | Aps (mm2) |
|---|---|---|---|---|---|---|---|---|
| [11] | 9 | I-shaped | 203–205 | 130–167 | 2.5 | 380 | 65 | 900 |
| [31] | 8 | I-shaped | 125–140 | 75–150 | 3.2 | 650 | 50 | 840 |
| [32] | 6 | I-shaped | 150–170 | 82–163 | 3.3 | 650 | 50 | 1680 |
| [13] | 3 | I-shaped | 103–105 | 48.8 | 2 | 400 | 70 | 280 |
| [10] | 8 | I-shaped | 168–189 | 65–130 | 2.5–3.4 | 700 | 40, 50 | 1680 |
| [33] | 3 | I-shaped | 193.0 | 130.0 | 2.5, 2.8 | 910 | 152 | 2230 |
| [12] | 6 | I-shaped | 125–127 | 120.00 | 1.1, 2, 3 | 300 | 50 | 420 |
| [5] | 6 | I-shaped | 137–160 | 130.0 | 3.1–3.9 | 889, 1092 | 76.2, 101.6 | 4560 |
| [25] | 13 | I-shaped | 126–155 | 125, 159, 190 | 1.2–3.4 | 863.6 | 50–101 | 3640 |
| [9] | 5 | I-shaped | 195–212 | 97–100 | 2.5–8.5 | 380 | 65 | 840 |
| [34] | 3 | Rectangle | 108.2 | 75 | 1.5–2.5 | 340 | 160 | 140 |
| [35] | 15 | I, box-shaped | 118–155 | 130, 160, 190 | 2.5–4 | 800–1500 | 50–152 | 1680 |
| [44] | 7 | I-shaped | 128–145 | 40, 75, 65, 100 | 1.60 | 500 | 65 | 372 |
| [37] | 2 | I-shaped | 126.0 | 130 | 1.1–3.3 | 600 | 70 | 280 |
| [38] | 10 | I-shaped | 152–189 | 77, 147 | 3.0–3.5 | 600–1000 | 40 | 1115 |
| [39] | 1 | I-shaped | 173.2 | 163 | 2.50 | 1067 | 114 | 6580 |
| [40] | 1 | Rectangle | 166.9 | 131.25 | 3 | 290 | 150 | 0 |
| [41] | 13 | I-shaped | 160–188 | 75, 150 | 3.25 | 350 | 60 | 0 |
| [42] | 2 | Rectangle | 100, 200 | 80 | 2 | 350 | 200 | 0 |
| [43] | 31 | T-shaped, Rectangle | 115–125 | 65, 130, 195 | 1.5–3.0 | 250 | 150 | 0 |
| [44] | 8 | I-shaped | 144–152 | 65.0, 130.0, 195 | 4–8.0 | 380, 460 | 50 | 0 |
| [27] | 2 | I-shaped | 147.69 | 52, 130 | 1.6–3 | 220, 400 | 50 | 0 |
| [18] | 3 | Rectangle | 140 | 200 | 1.23 | 300 | 200 | 0 |
| [46] | 8 | Rectangle | 130–152 | 172.25 | 1.5–3.0 | 350 | 250 | 0 |
| [47] | 11 | Rectangle | 127, 131 | 65, 130, 195, 260 | 1.5–2.3 | 250 | 150 | 0 |
| [48] | 9 | I-shaped | 160–170 | 97.5 | 2.5, 3.5 | 520, 700 | 40 | 0 |
| [49] | 2 | I-shaped | 150 | 195 | 2.8 | 200 | 20 | 0 |
| [50] | 3 | I-shaped | 198–211 | 37.1, 74.2 | 4 | 330 | 30 | 0 |
| Average | - | - | 149.58 | 130.2 | 2.8 | 517.1 | 91.1 | 1709.6 |
| Max. | - | - | 212 | 260 | 8 | 1422.4 | 250 | 6580 |
| Min. | - | - | 100 | 37.1 | 1.5 | 200 | 20 | 140 |
| Reference | No. of Specimens | Vf lf/df (%) | f’c (MPa) | ftloc (MPa) |
|---|---|---|---|---|
| [51] | 31 | 86.70–260.0 | 84.8–170.3 | 6.4–12.5 |
| [5] | 6 | 130.0 | 137.0–160.0 | 8.6–11.5 |
| [52] | 8 | 54.5–121.9 | 129.0–140.0 | 5.0–9.4 |
| [53] | 12 | 62.5 | 171.0–195.0 | 6.9–11.9 |
| [54] | 5 | 86.7–130.0 | 93.8–148.0 | 6.4–12.5 |
| [55] | 4 | 130.0 | 126.0 | 11.9 |
| [56] | 6 | 130.0–290.0 | 150.0 | 15.1–15.7 |
| [57] | 4 | 130.0 | 196.5 | 12.2 |
| Prediction Model | Vexp/Vpred. Avg. | Vexp/Vpred. SD. | Vexp/Vpred. CoV (%) | Vexp/Vpred. RRMSE (%) | Vexp/Vpred. >2 | Vexp/Vpred. <0.75 |
|---|---|---|---|---|---|---|
| FHWA method [24] | 1.19 | 0.29 | 24.6 | 35.7 | 2 | 11 |
| ePCI method [25] | 1.58 | 0.43 | 27.4 | 55.1 | 28 | 0 |
| AFGC method [26] | 1.24 | 0.44 | 35.5 | 37.9 | 13 | 20 |
| CSA method [27] | 1.14 | 0.34 | 29.5 | 38.6 | 5 | 15 |
| DAfStb method [28] | 1.40 | 0.55 | 39.6 | 59.7 | 28 | 23 |
| Non-Prestressed (n = 97) | Prestressed (n = 101) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| No Av (n = 60) | With Av (n = 37) | No Av (n = 72) | With Av (n = 29) | |||||||||
| Method | Avg. | CoV % | <0.75 | Avg. | CoV % | <0.75 | Avg. | CoV % | <0.75 | Avg. | CoV. % | <0.75 |
| FHWA [24] | 1.17 | 29.4 | 5 | 1.18 | 22.3 | 3 | 1.19 | 23.9 | 2 | 1.27 | 15.8 | 1 |
| ePCI [25] | 1.64 | 30.3 | 0 | 1.40 | 19.1 | 0 | 1.65 | 28.4 | 0 | 1.54 | 18.9 | 0 |
| AFGC [26] | 1.19 | 48.6 | 14 | 0.97 | 21.6 | 5 | 1.42 | 26.8 | 0 | 1.25 | 16.1 | 1 |
| CSA [27] | 1.20 | 31.7 | 4 | 0.94 | 22.2 | 3 | 1.15 | 28.9 | 7 | 1.25 | 22.1 | 1 |
| DAfStb [28] | 1.40 | 38.3 | 6 | 1.54 | 28.0 | 1 | 1.48 | 39.2 | 7 | 1.01 | 48.5 | 9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tarawneh, A.; Amirah, H.; Alghossoon, A.; Almasabha, G.; Al-Mazaidh, R.; Saleh, E. Performance Evaluation of Current Design Models in Predicting Shear Resistance of UHPC Girders. J. Compos. Sci. 2025, 9, 211. https://doi.org/10.3390/jcs9050211
Tarawneh A, Amirah H, Alghossoon A, Almasabha G, Al-Mazaidh R, Saleh E. Performance Evaluation of Current Design Models in Predicting Shear Resistance of UHPC Girders. Journal of Composites Science. 2025; 9(5):211. https://doi.org/10.3390/jcs9050211
Chicago/Turabian StyleTarawneh, Ahmad, Hadeel Amirah, Abdullah Alghossoon, Ghassan Almasabha, Ra’ed Al-Mazaidh, and Eman Saleh. 2025. "Performance Evaluation of Current Design Models in Predicting Shear Resistance of UHPC Girders" Journal of Composites Science 9, no. 5: 211. https://doi.org/10.3390/jcs9050211
APA StyleTarawneh, A., Amirah, H., Alghossoon, A., Almasabha, G., Al-Mazaidh, R., & Saleh, E. (2025). Performance Evaluation of Current Design Models in Predicting Shear Resistance of UHPC Girders. Journal of Composites Science, 9(5), 211. https://doi.org/10.3390/jcs9050211









