Adiabatic Blanking: Influence of Clearance, Impact Energy, and Velocity on the Blanked Surface
Abstract
1. Introduction
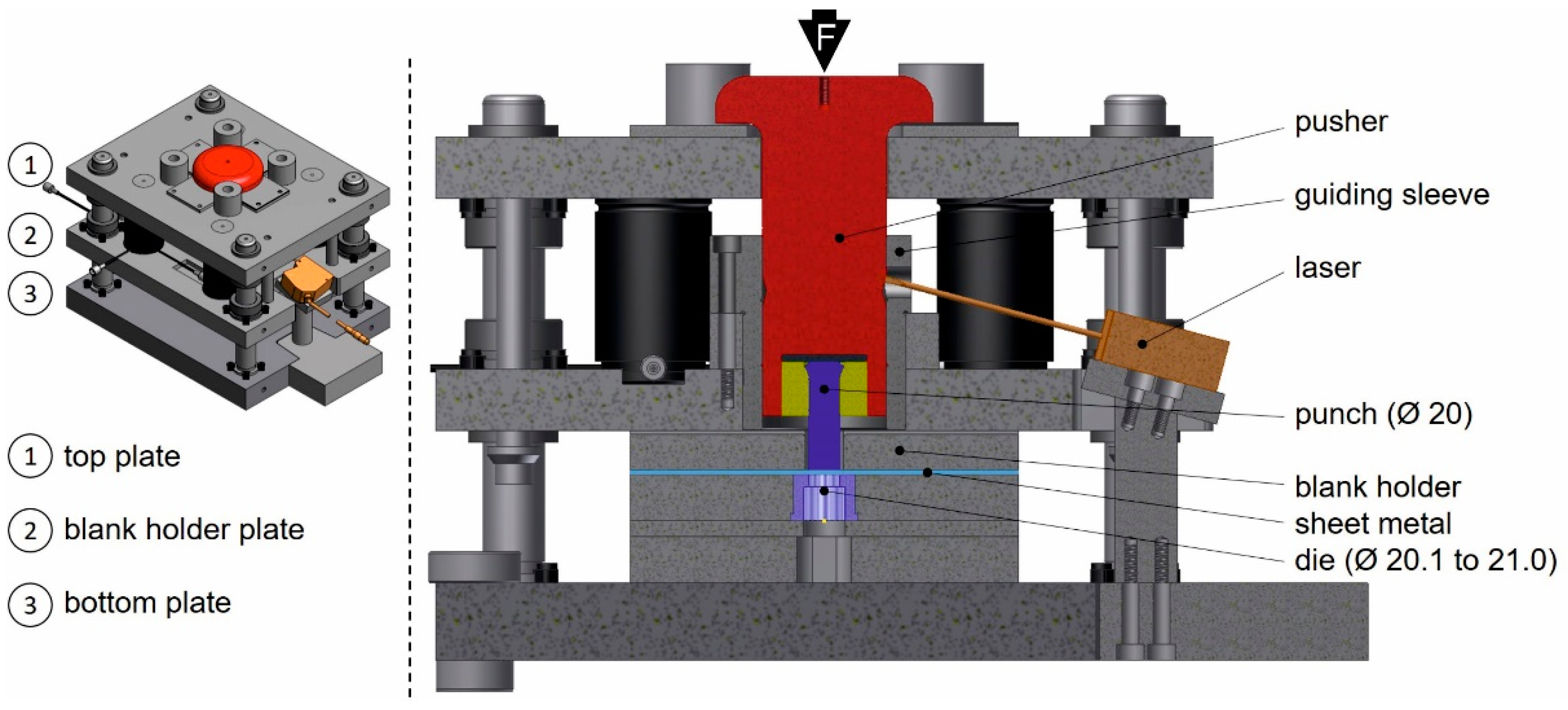
2. Materials and Methods
3. Results and Discussions
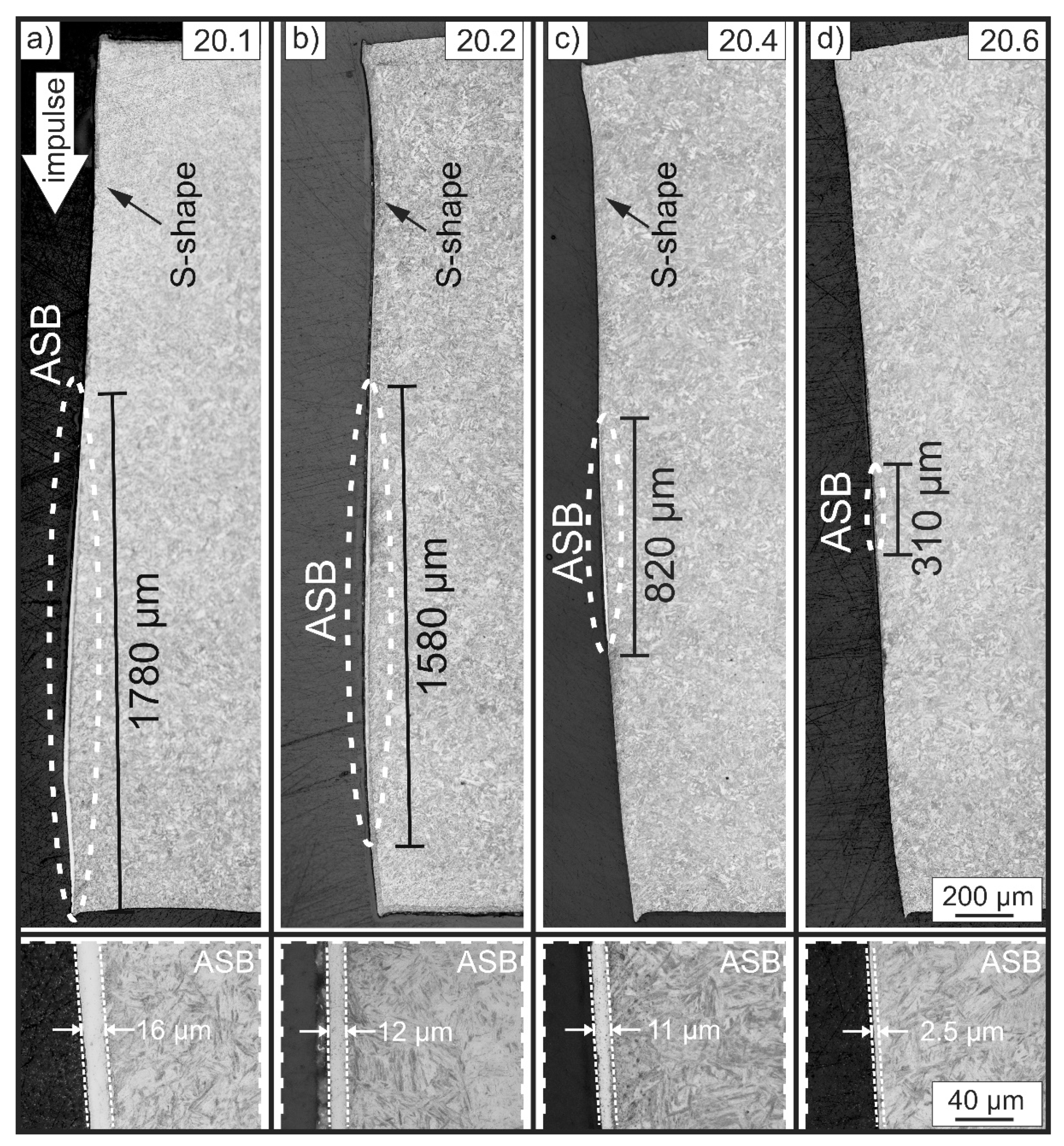
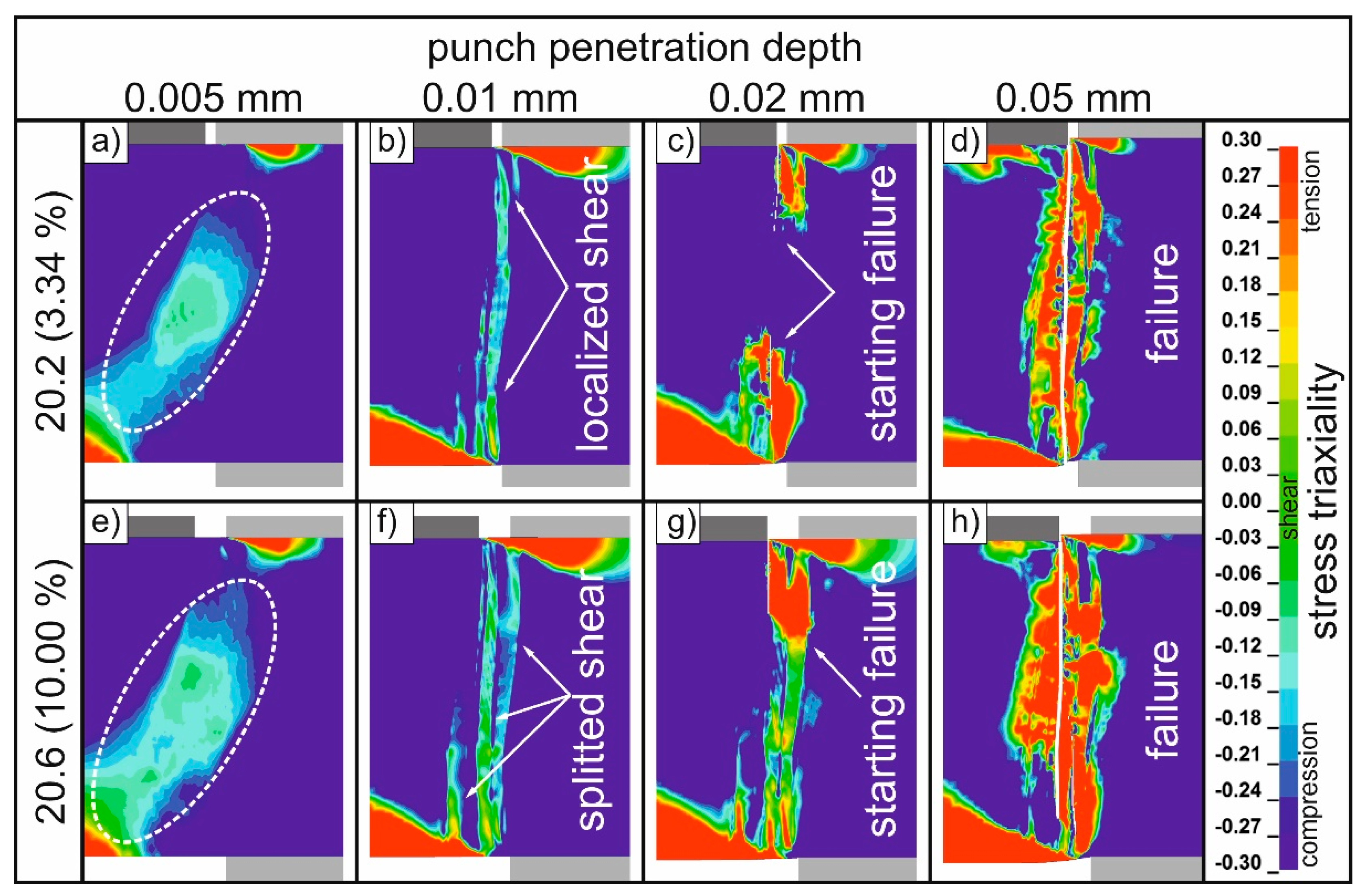
3.1. Influence of the Clearance on the Adiabatic Shear Band Initiation and the Blanked Surface Geometry
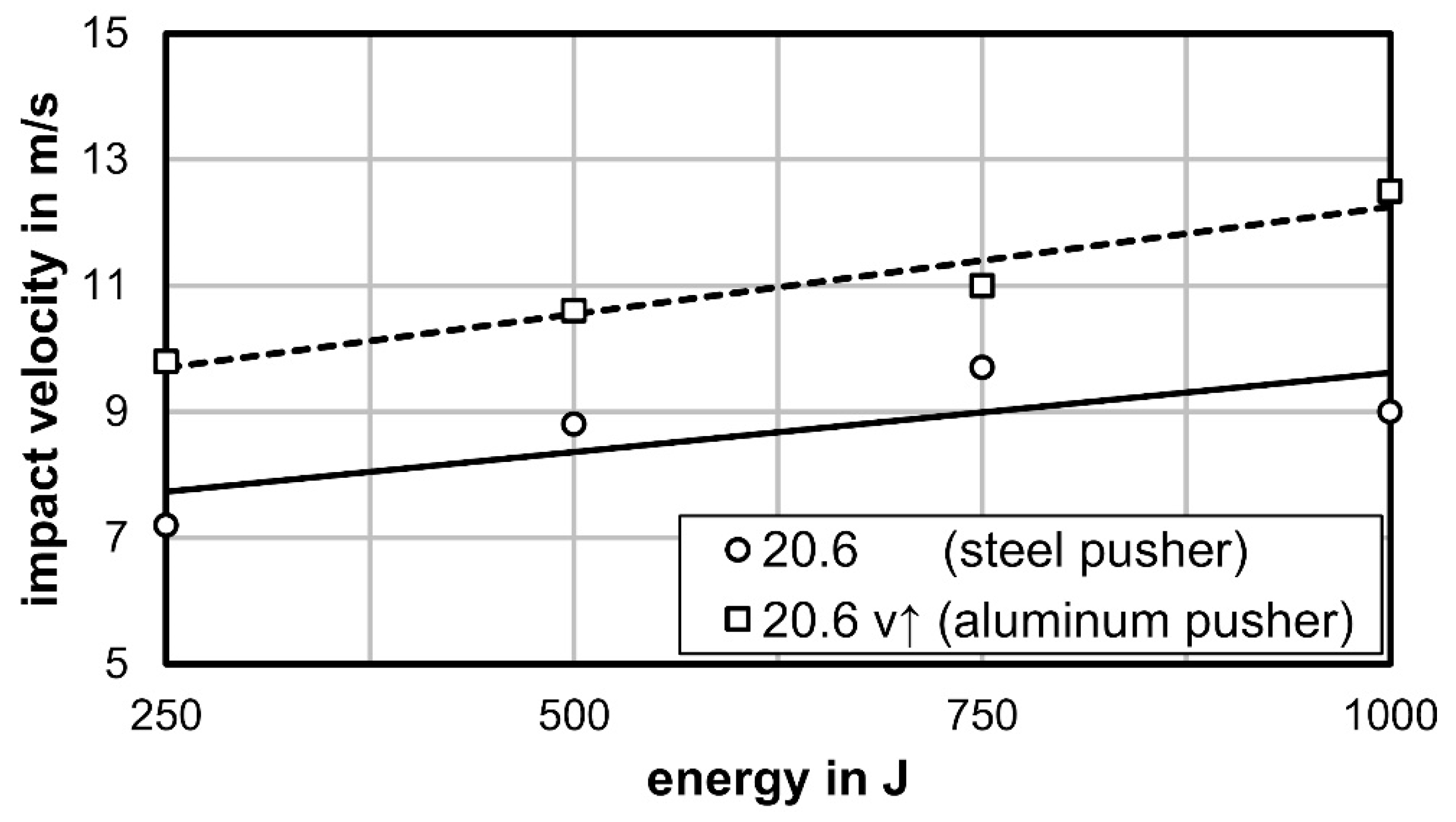
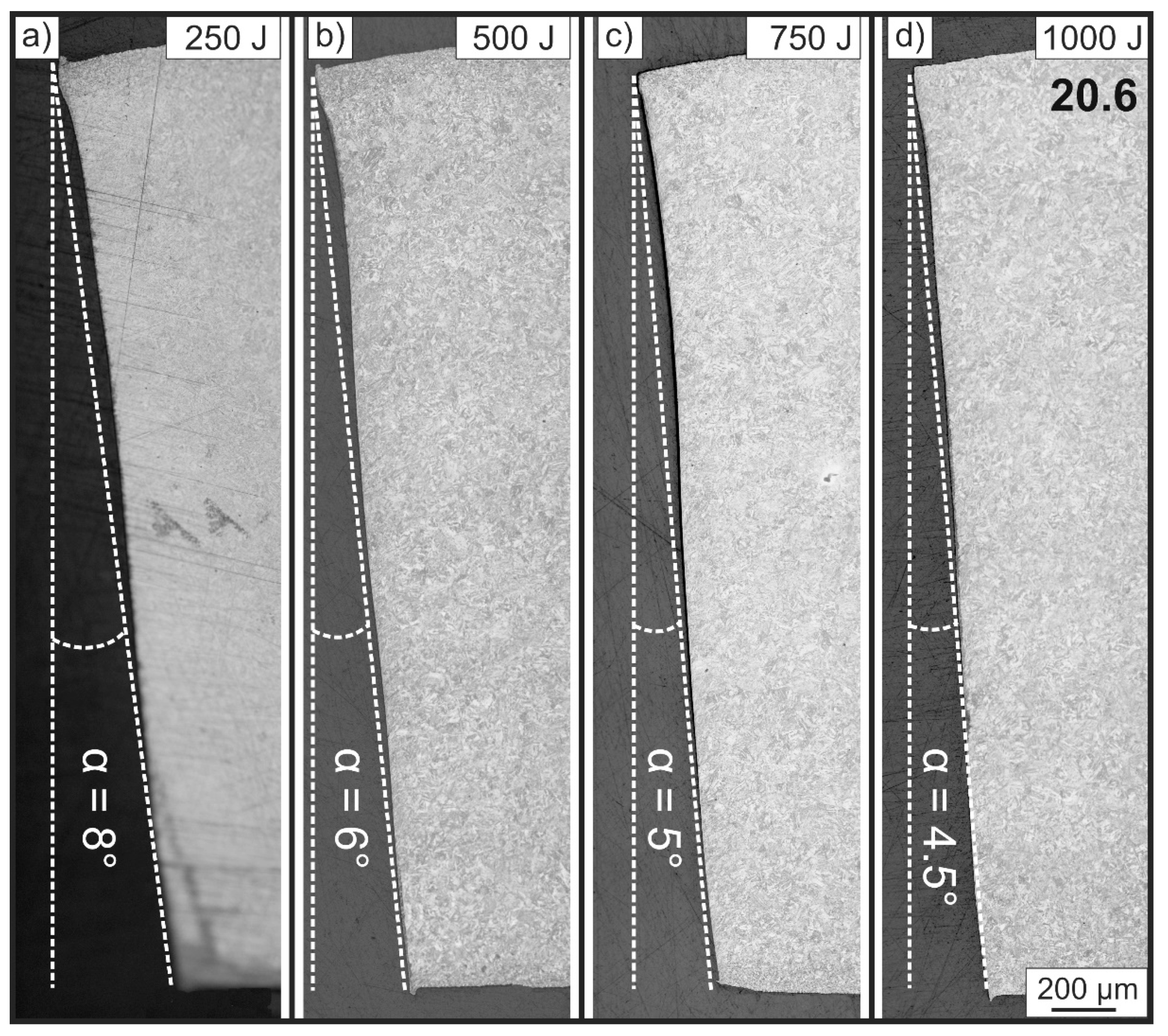
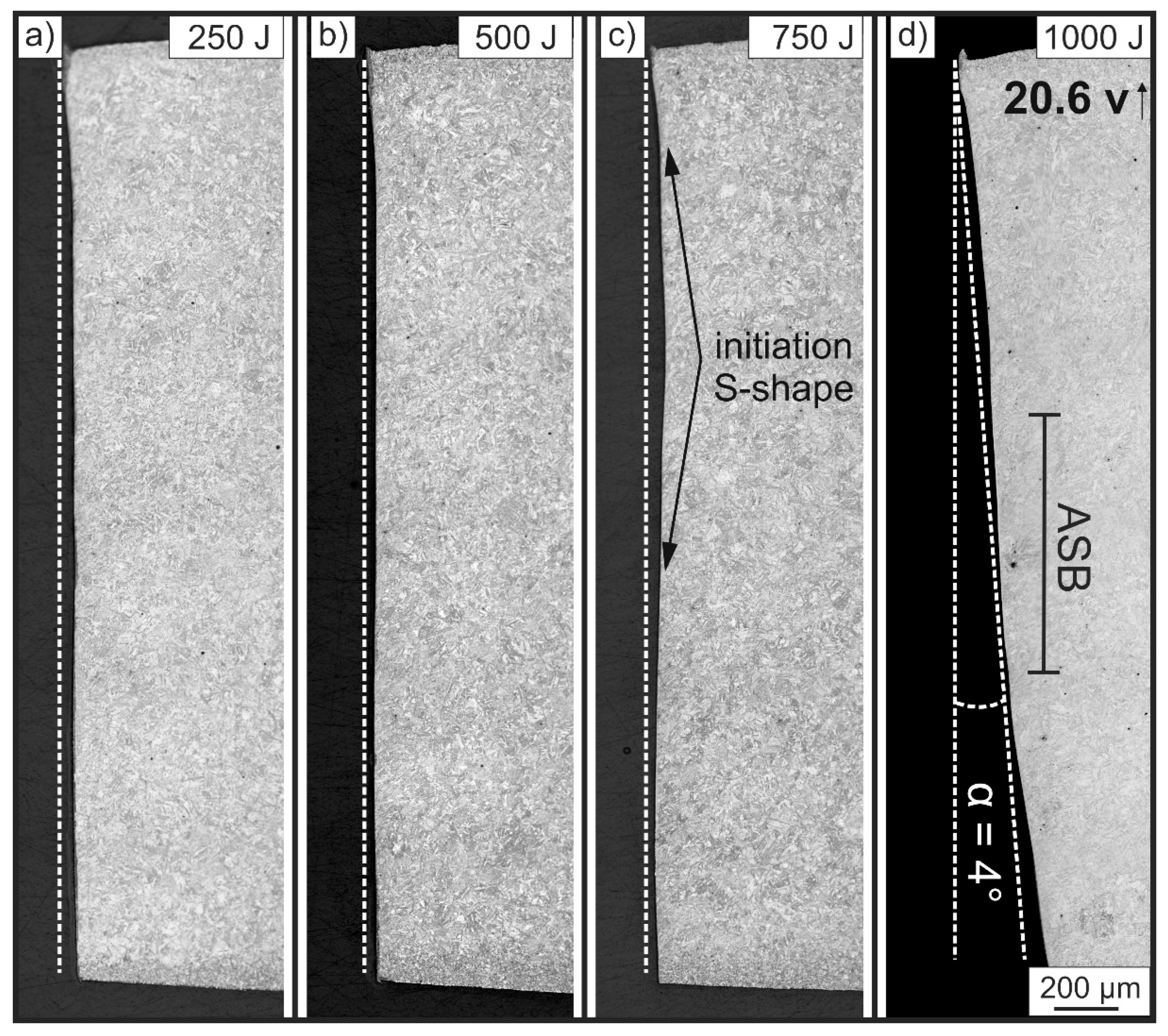
3.2. Influence of Impact Energy and Velocity on the Blanked Surface
4. Summary and Conclusions
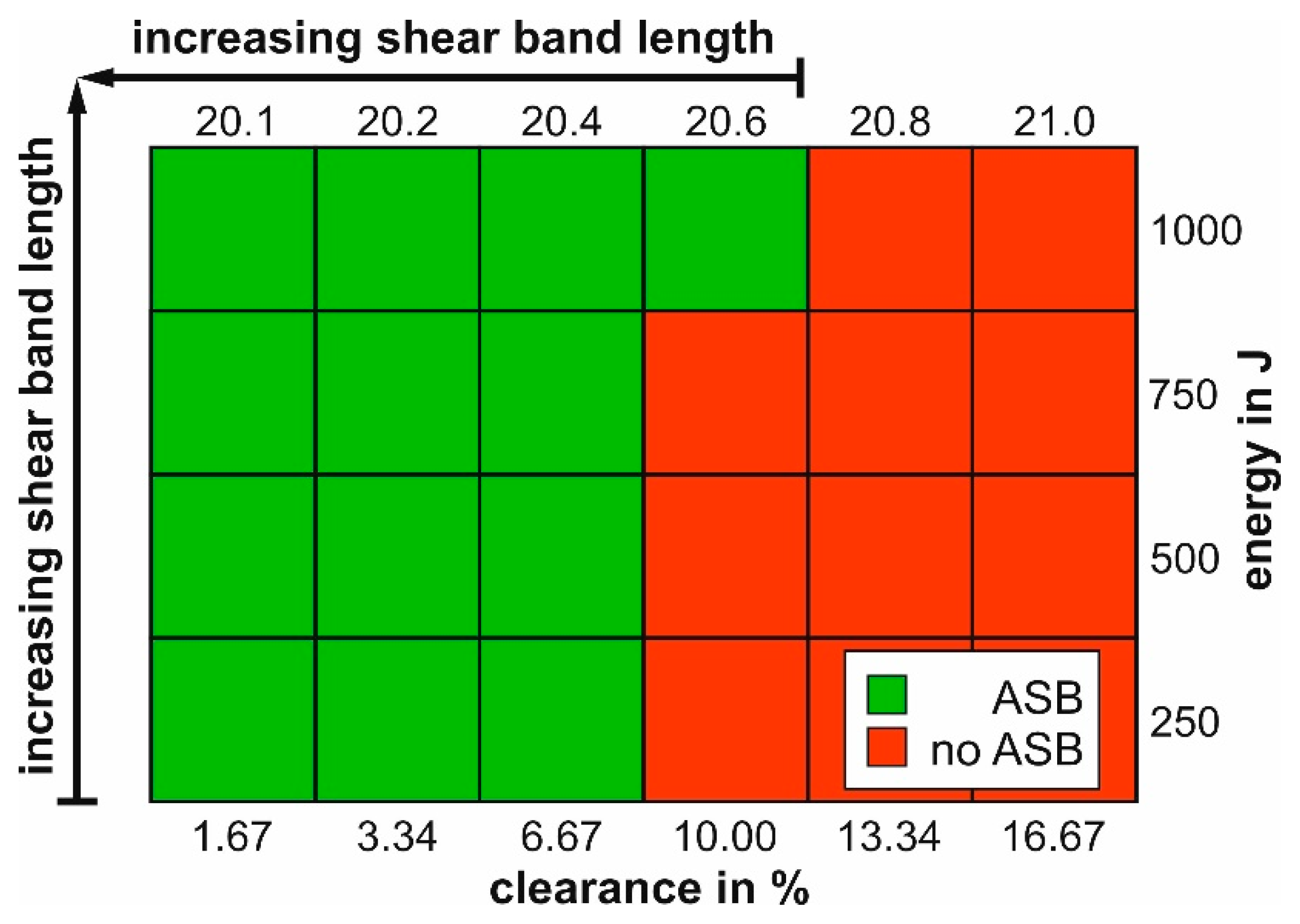
- If the relative clearance is ≤6.67% and the impact velocity is ≥7 m/s, an ASB is always formed regardless of the investigated impact energies (250 J to 1000 J). A smaller blanking clearance increases the amount of compressive stress in the shear zone, which is necessary for dynamic recrystallization and for the formation of an ASB. For larger clearances, the energy and velocity must be increased to form an ASB. Thereby, the impact velocity cannot be considered separately from the impact energy.
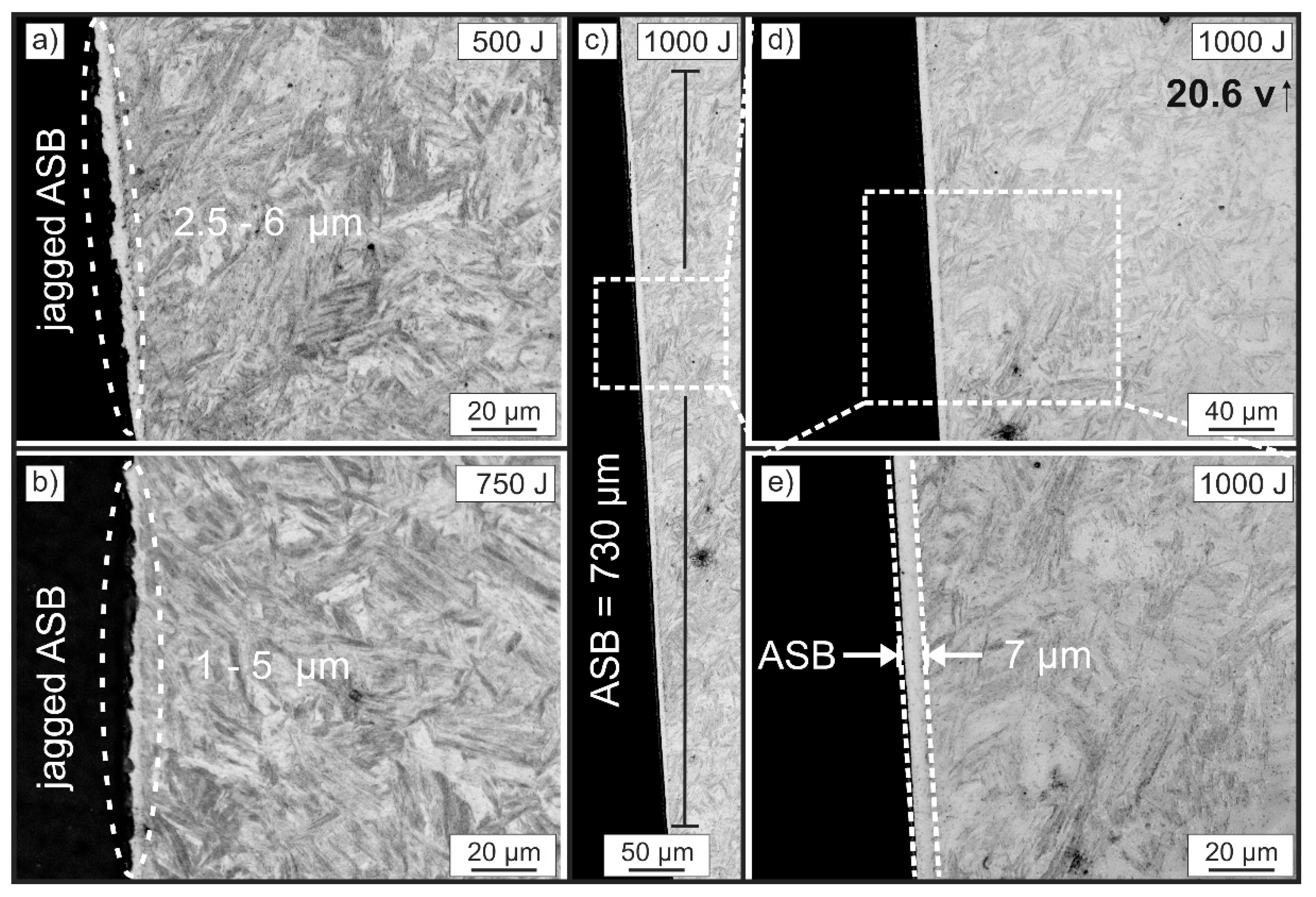
- The initiation of the ASB is centered in the shear zone in the area of the highest compressive stresses. A smaller blanking clearance, a higher impact energy, and a higher speed result in longer and wider ASB.
- Three different blanking surface types were identified depending on the process parameters. The initiation of an ASB promotes the formation of an S-shaped blanking surface. If the relative clearance is ≥10% and the velocity is less than 10 m/s, an angled fracture surface occurs, whereby an increase in velocity results in smaller angles. High velocity (10–12.5 m/s) combined with a blanking clearance of 10% and a maximum impact energy of 500 J results in a very straight blanking surface with high quality. This surface exhibits selectively jagged and very small ASB. This demonstrates that lower impact energies combined with increased velocity can lead to blanked surfaces of very high quality.
Author Contributions
Funding

Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sachnik, P.; Hoque, S.E.; Volk, W. Burr-free cutting edges by notch-shear cutting. J. Mater. Process. Technol. 2017, 249, 229–245. [Google Scholar] [CrossRef]
- Sahli, M.; Roizard, X.; Assoul, M.; Colas, G.; Giampiccolo, S.; Barbe, J.P. Finite element simulation and experimental investigation of the effect of clearance on the forming quality in the fine blanking process. Microsyst. Technol. 2020, 7. [Google Scholar] [CrossRef]
- Neugebauer, R.; Weigel, P.; Westkämpfer, E.; Verl, A.; Eicher, F. Investigation of Application Potentials and Limits of Adiabatic Cutting and Punching Operations; Germany Report P774; Verlag und Vertriebsgesellschaft mbH: Düsseldorf, Germany, 2010. [Google Scholar]
- Schmitz, F.; Winter, S.; Clausmeyer, T.; Wagner, M.F.X.; Tekkaya, A.E. Adiabatic blanking of advanced high-strength steels. CIRP Ann. 2020, 69, 269–272. [Google Scholar] [CrossRef]
- Neugebauer, R.; Kräusel, V.; Weigel, P. Hochgeschwindigkeitsscherschneiden hält Einzug in die Blechbearbeitung. Wt. Werkstattstech. Online 2008, 98, 813–814. [Google Scholar]
- Rosenthal, S.; Maaß, F.; Kamaliev, M.; Hahn, M.; Gies, S.; Tekkaya, A.E. Lightweight in Automotive Components by Forming Technology. Automot. Innov. 2020. [Google Scholar] [CrossRef]
- Zener, C.; Hollomon, J.H. Effect of Strain Rate Upon Plastic Flow of Steel. J. Appl. Phys. 1944, 15, 22–32. [Google Scholar] [CrossRef]
- Meyers, M.A.; Xu, Y.B.; Xue, Q.; Pérez-Prado, M.T.; McNelley, T.R. Microstructural evolution in adiabatic shear localization in stainless steel. Acta Mater. 2003, 51, 1307–1325. [Google Scholar] [CrossRef]
- Bai, Y.; Dodd, B. Adiabatic Shear Localization; Pergamon Press: Oxford, UK, 2012; ISBN 978-0-08-097781-2. [Google Scholar]
- Rogers, H. Adiabatic plastic deformation. Annu. Rev. Mater. Sci. 1979, 9, 283–311. [Google Scholar] [CrossRef]
- Wang, B.; Sun, J.; Wang, X.; Fu, A. Adiabatic shear localization in a near beta Ti-5Al-5Mo-5 V-1Cr-1Fe alloy. Mater. Sci. Eng. A 2015. [Google Scholar] [CrossRef]
- Xu, Y.; Bai, Y.; Meyers, M.A. Deformation, phase transformation and recrystallization in the shear bands induced by high-strain rate loading in titanium and its alloys. J. Mater. Sci. Technol. 2006, 22, 737–746. [Google Scholar]
- Meyers, M.A.; Wittman, C.L. Effect of metallurgical parameters on shear band formation in low-carbon (approx. 0.20 wt pct) steels. Metall. Trans. A, Phys. Metall. Mater. Sci. 1990, 21A, 3153–3164. [Google Scholar] [CrossRef]
- Nesterenko, V.F.; Meyers, M.A.; LaSalvia, J.C.; Bondar, M.P.; Chen, Y.J.; Lukyanov, Y.L. Shear localization and recrystallization in high-strain, high-strain-rate deformation of tantalum. Mater. Sci. Eng. A 1997, 229, 23–41. [Google Scholar] [CrossRef]
- Osovski, S.; Rittel, D.; Landau, P.; Venkert, A. Microstructural effects on adiabatic shear band formation. Scr. Mater. 2012, 66, 9–12. [Google Scholar] [CrossRef]
- Rittel, D.; Wang, Z.G.; Merzer, M. Adiabatic shear failure and dynamic stored energy of cold work. Phys. Rev. Lett. 2006, 96, 1–4. [Google Scholar] [CrossRef] [PubMed]
- Mendoza, I.; Villalobos, D.; Alexandrov, B.T. Crack propagation of Ti alloy via adiabatic shear bands. Mater. Sci. Eng. A 2015, 645, 306–310. [Google Scholar] [CrossRef]
- Gaudillière, C.; Ranc, N.; Larue, A.; Lorong, P. Investigations in high speed blanking: Cutting forces and microscopic observations. EPJ Web Conf. 2010, 6, 19003. [Google Scholar] [CrossRef]
- Winter, S.; Pfeiffer, S.; Bergelt, T.; Wagner, M.F.-X. Finite element simulations on the relation of microstructural characteristics and the formation of different types of adiabatic shear bands in a β-titanium alloy. IOP Conf. Ser. Mater. Sci. Eng. 2019, 480, 012022. [Google Scholar] [CrossRef]
- Winter, S.; Schmitz, F.; Clausmeyer, T.; Tekkaya, A.E.; Wagner, M.F.-X. High temperature and dynamic testing of AHSS for an analytical description of the adiabatic cutting process. IOP Conf. Ser. Mater. Sci. Eng. 2017, 181, 012026. [Google Scholar] [CrossRef]
- Pouya, M.; Winter, S.; Fritsch, S.; Wagner, M.F.-X. A numerical and experimental study of temperature effects on deformation behavior of carbon steels at high strain rates. IOP Conf. Ser. Mater. Sci. Eng. 2017, 181, 012022. [Google Scholar] [CrossRef]
- Psyk, V.; Scheffler, C.; Tulke, M.; Winter, S.; Guilleaume, C.; Brosius, A. Determination of Material and Failure Characteristics for High-Speed Forming via High-Speed Testing and Inverse Numerical Simulation. J. Manuf. Mater. Process. 2020, 4, 31. [Google Scholar] [CrossRef]
- Dodd, B.; Bai, Y. Width of adiabatic shear bands formed under combined stresses. Mater. Sci. Technol. 1989, 5, 557–559. [Google Scholar] [CrossRef]
- Xue, Q.; Meyers, M.A.; Nesterenko, V.F. Self-organization of shear bands in titanium and Ti-6Al-4V alloy. Acta Mater. 2002, 50, 575–596. [Google Scholar] [CrossRef]
- Nesterenko, V.F.; Meyers, M.A.; Wright, T.W. Self-organization in the initiation of adiabatic shear bands. Acta Mater. 1998, 46, 327–340. [Google Scholar] [CrossRef]
- Landgrebe, D.; Müller, R.; Sterzing, A.; Mauermann, R.; Rennau, A. Hochfeste Stähle—Chance für Leichtbau und für Effizienzsteigerung in der Produktion. In Proceedings of the 18 Werkstofftechnischen Kolloquium in Chemnitz 2016; Lampke, T., Ed.; Schriftenreihe Werkstoffe und Werkstofftechnische Anwendungen 59; Technische Universität Chemnitz: Chemnitz, Germany, 2016; pp. 34–44. [Google Scholar]
- Rodríguez-Martínez, J.A.; Vadillo, G.; Rittel, D.; Zaera, R.; Fernández-Sáez, J. Dynamic recrystallization and adiabatic shear localization. Mech. Mater. 2015, 81, 41–55. [Google Scholar] [CrossRef]

| in wt.-% | C | Mn | Si | Cr | B (ppm) | Fe |
|---|---|---|---|---|---|---|
| 22MnB5 | 0.23 | 1.21 | 0.15 | 0.18 | 21.00 | balance |
| Ø Die in mm (Sample Designation) | 20.1 | 20.2 | 20.4 | 20.6 | 20.8 | 21.0 |
|---|---|---|---|---|---|---|
| blanking clearance in mm | 0.05 | 0.10 | 0.20 | 0.30 | 0.40 | 0.50 |
| relative clearance in% | 1.67 | 3.34 | 6.67 | 10.00 | 13.34 | 16.67 |
| A in MPa | B in MPa | C | n | k | m | λ in W/(mK) |
|---|---|---|---|---|---|---|
| 1380 | 502 | 0.0011 | 0.15 | 0.41 | 0.0040 | 48.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Winter, S.; Nestler, M.; Galiev, E.; Hartmann, F.; Psyk, V.; Kräusel, V.; Dix, M. Adiabatic Blanking: Influence of Clearance, Impact Energy, and Velocity on the Blanked Surface. J. Manuf. Mater. Process. 2021, 5, 35. https://doi.org/10.3390/jmmp5020035
Winter S, Nestler M, Galiev E, Hartmann F, Psyk V, Kräusel V, Dix M. Adiabatic Blanking: Influence of Clearance, Impact Energy, and Velocity on the Blanked Surface. Journal of Manufacturing and Materials Processing. 2021; 5(2):35. https://doi.org/10.3390/jmmp5020035
Chicago/Turabian StyleWinter, Sven, Matthias Nestler, Elmar Galiev, Felix Hartmann, Verena Psyk, Verena Kräusel, and Martin Dix. 2021. "Adiabatic Blanking: Influence of Clearance, Impact Energy, and Velocity on the Blanked Surface" Journal of Manufacturing and Materials Processing 5, no. 2: 35. https://doi.org/10.3390/jmmp5020035
APA StyleWinter, S., Nestler, M., Galiev, E., Hartmann, F., Psyk, V., Kräusel, V., & Dix, M. (2021). Adiabatic Blanking: Influence of Clearance, Impact Energy, and Velocity on the Blanked Surface. Journal of Manufacturing and Materials Processing, 5(2), 35. https://doi.org/10.3390/jmmp5020035







