Further Development of Wear Calculation and Wear Reduction in Cold Forging Processes
Abstract
1. Introduction and Motivation
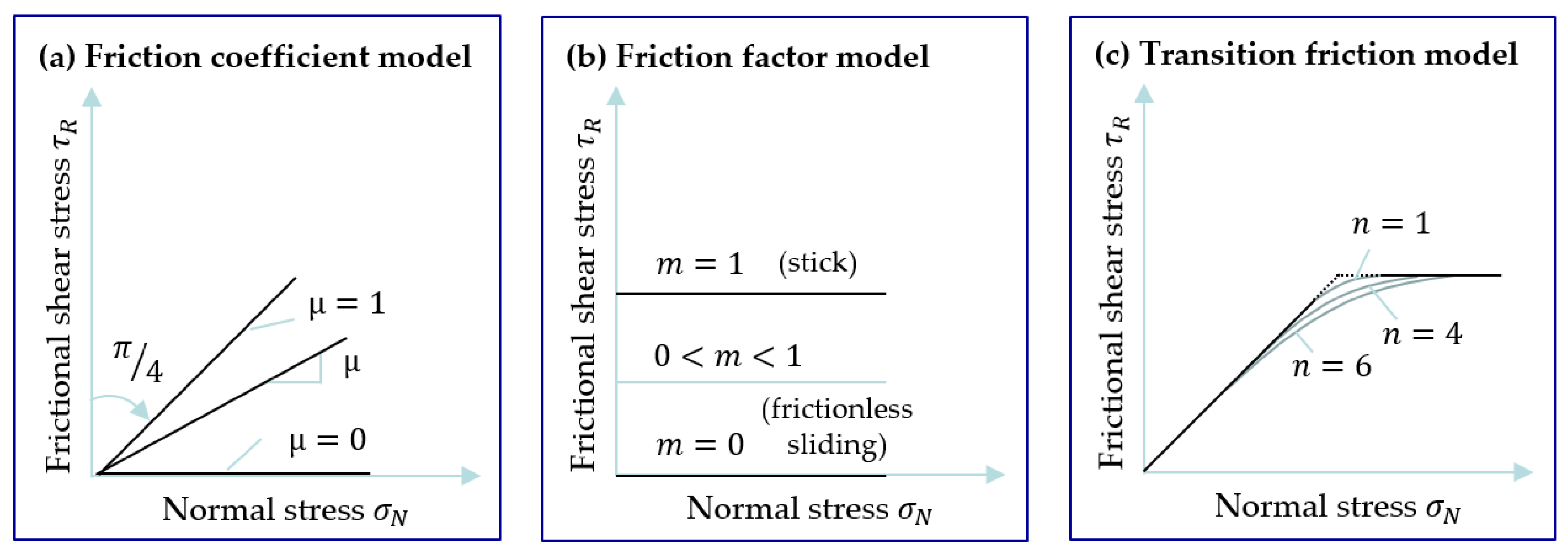
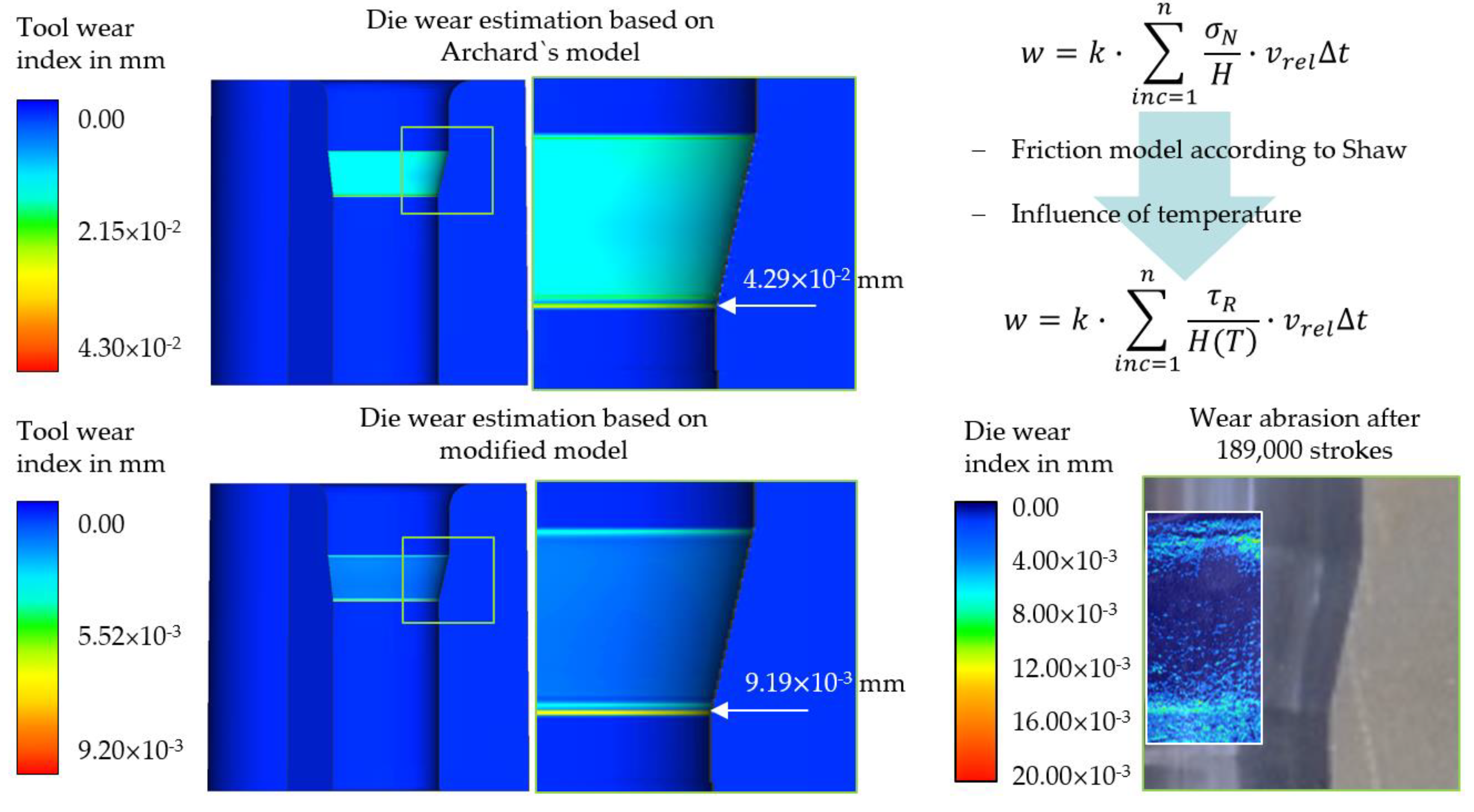
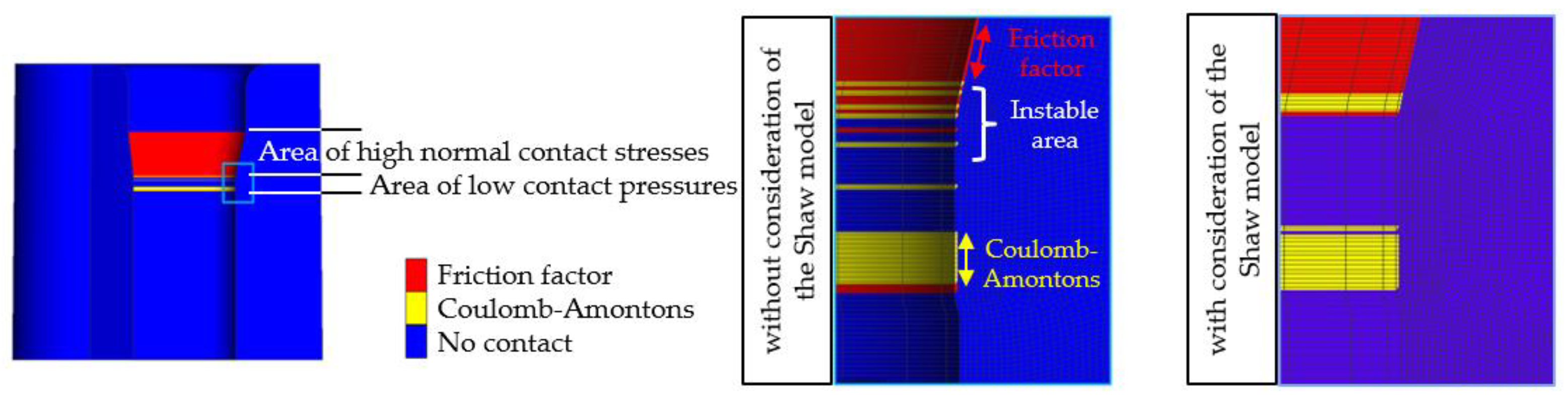
2. Development of the Modified Wear Model According to Archard
3. Material Characterisation and Experimental Wear Analysis
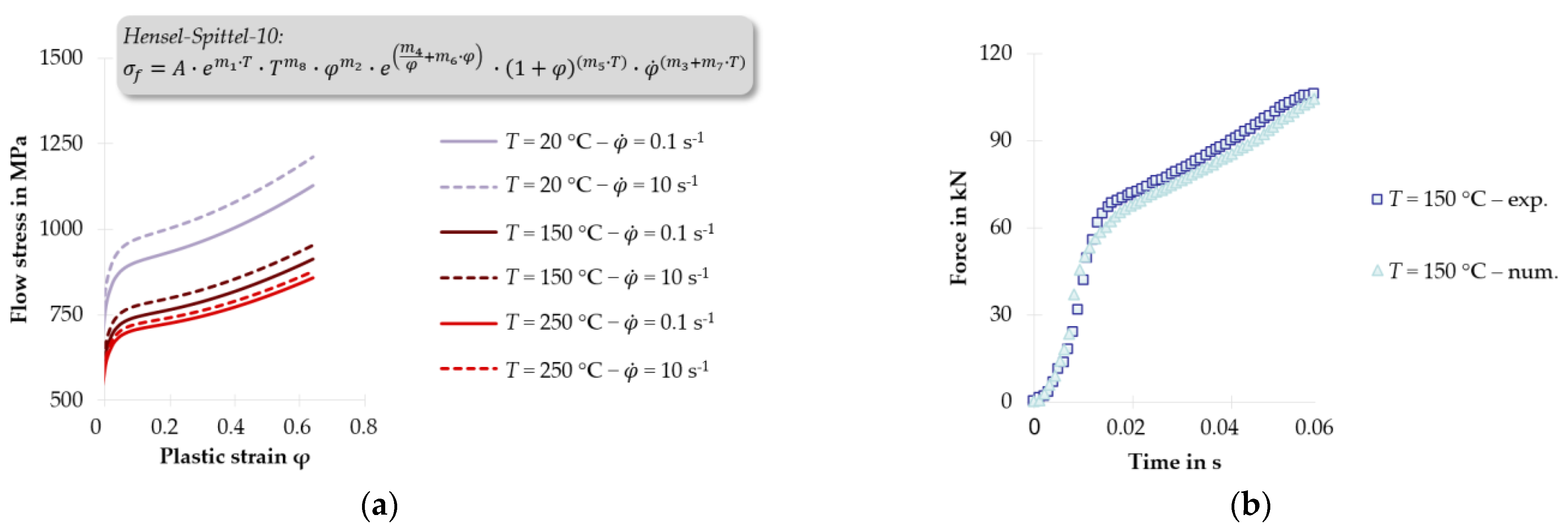
3.1. Characterisiation of Deformation Behaviour
3.2. Characterisiation of the CVD-TiN Coating
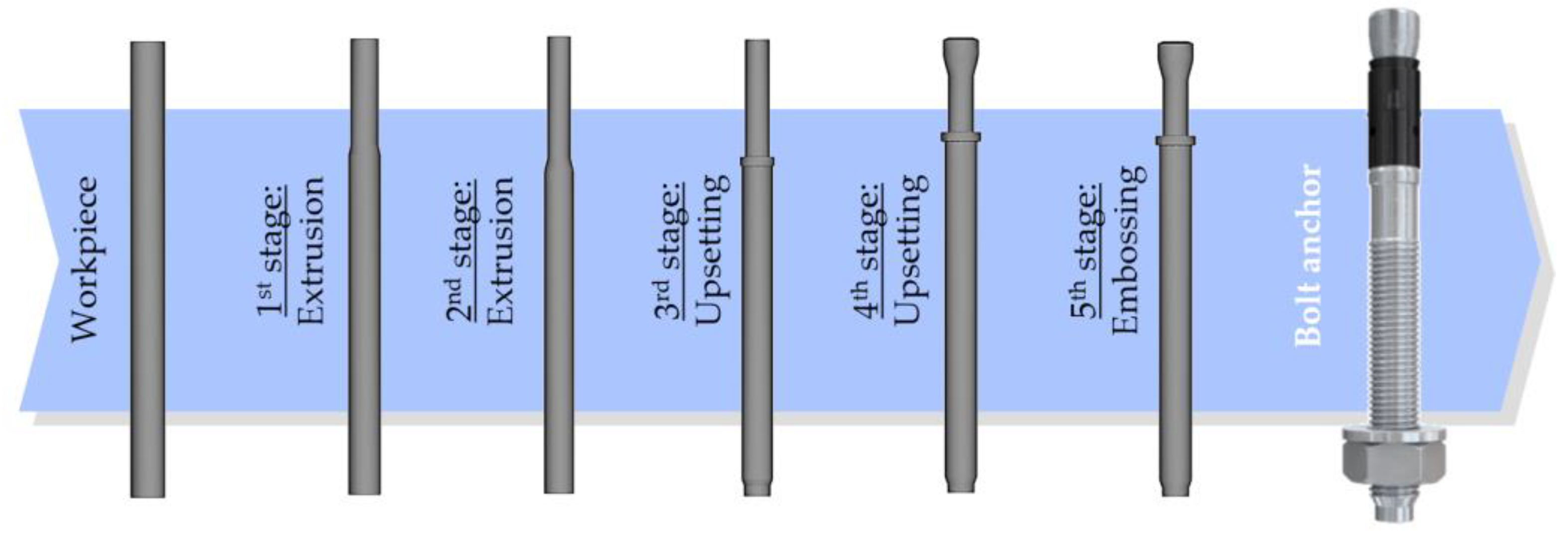
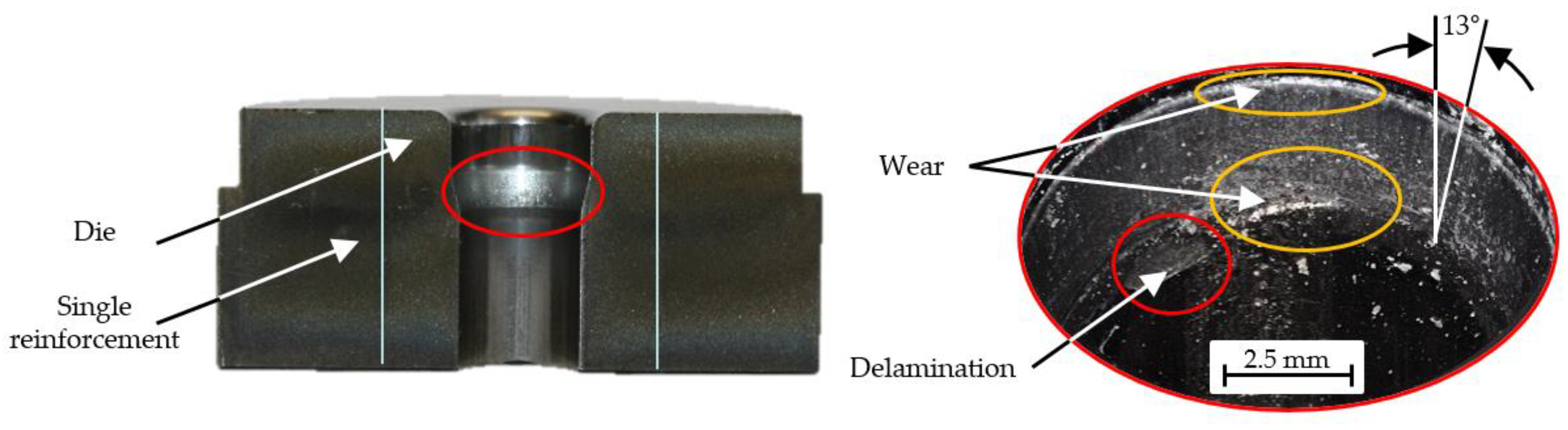
3.3. Experimental Wear Analysis of Industrial Dies
4. Numerical Wear Analysis and Design Improvements for Wear Reduction
4.1. Numerical Wear Analysis of Industrial Dies
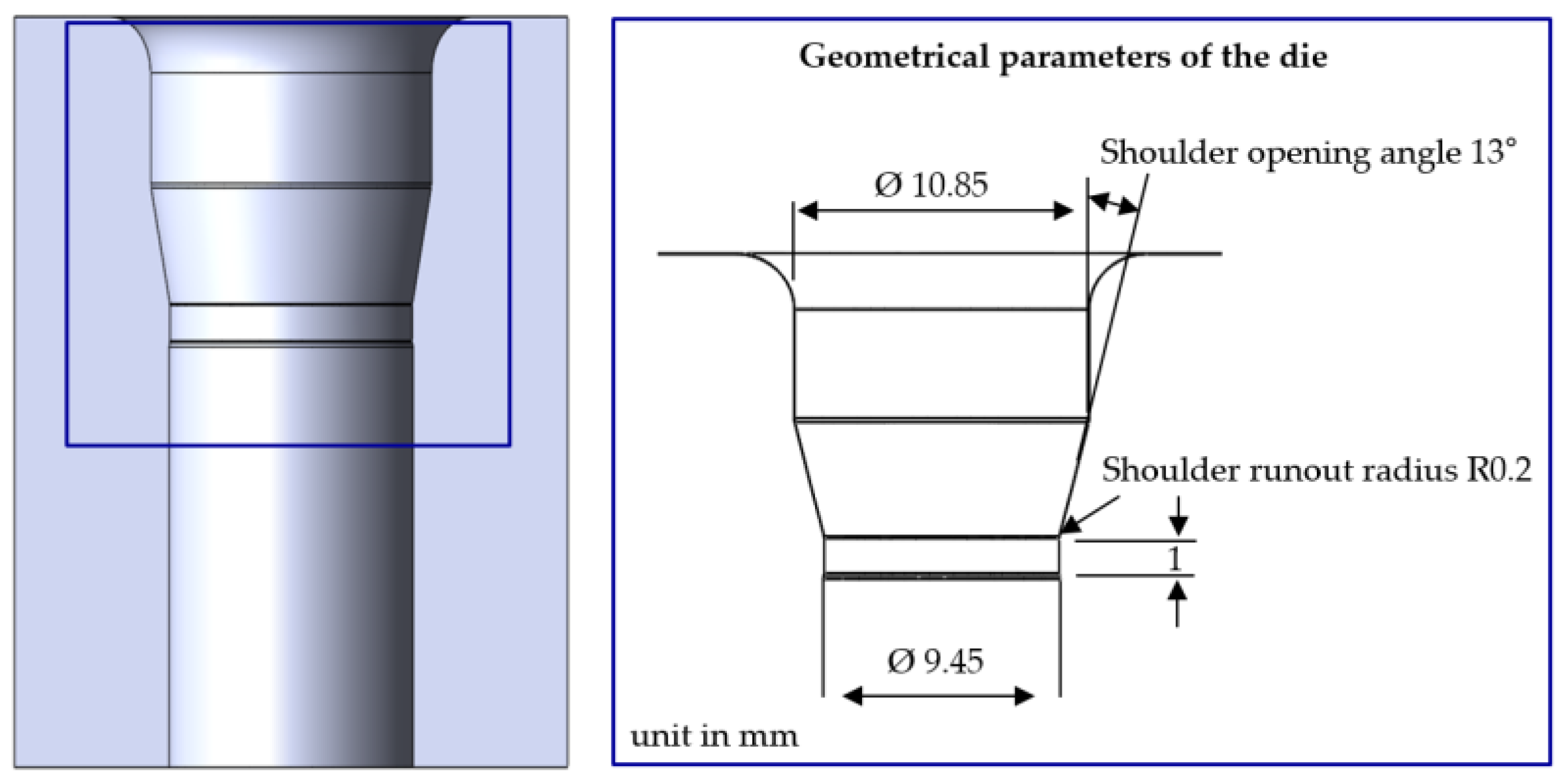
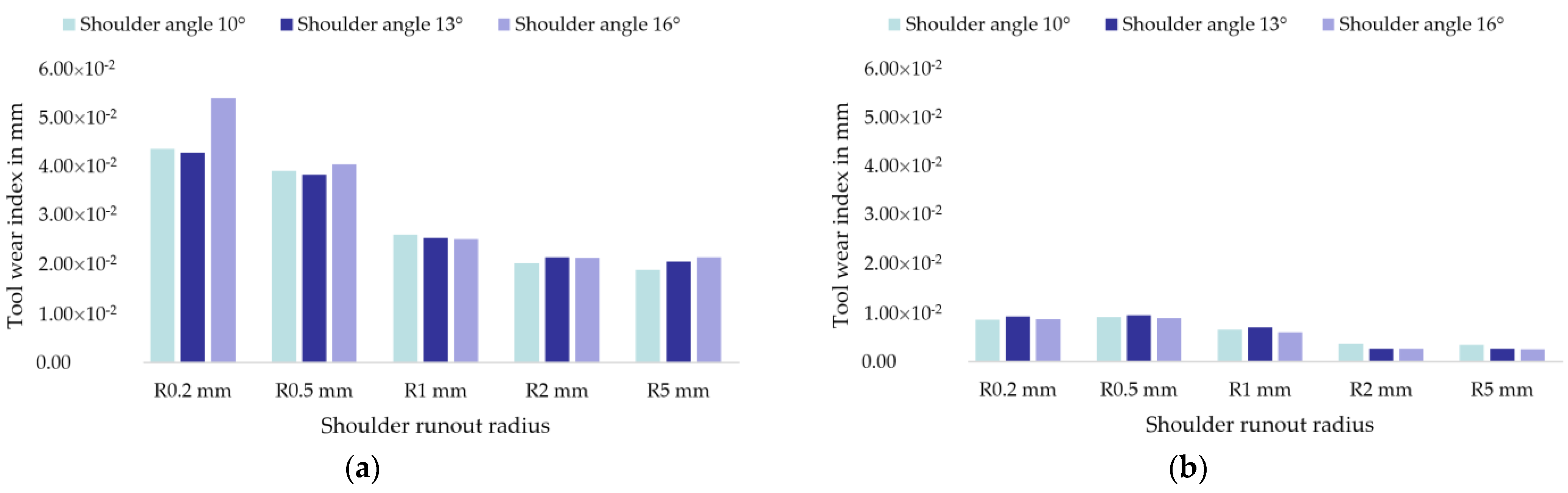
4.2. Design Improvements in the Die Geometry for Wear Reduction
5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hancock, G.J.; Murray, T.M.; Ellifritt, D.S. Cold-Formed Steel Structures to the AISI Specification; M. Dekker: New York, NY, USA, 2001. [Google Scholar]
- DeGarmo, E.P.; Black, J.T.; Kohser, R.A. Materials and Processes in Manufacturing, 9th ed.; John Wiley & Sons Ltd.: Chichester, UK, 2003. [Google Scholar]
- Kuzman, K. Problems of accuracy control in cold forming. J. Mater. Process. Technol. 2001, 113, 10–15. [Google Scholar] [CrossRef]
- Sieber, K. Erfahrungen beim Fließpressen von Hohlkörpern, Kalt, halbwarm und warm. Draht 1978, 29, 4. [Google Scholar]
- Dieter, G.E. Mechanical Metallurgy; Mc Graw-Hili Book Company: London, UK, 1988; p. 4. [Google Scholar]
- Long, H. Quantitative evaluation of dimensional errors of formed components in cold backward cup extrusion. J. Mater. Proces. Technol. 2006, 177, 591–595. [Google Scholar] [CrossRef]
- Lange, K.; Cser, L.M.; Geiger, M.; Kals, J.A.G. Tool Life and Tool Quality in Bulk Metal Forming. Ann. CIRP 1992, 41, 667–675. [Google Scholar] [CrossRef]
- Groche, P.; Moeller, N.; Hoffmann, H.; Suh, J. Influence of Gliding Speed and Contact Pressure on the Wear of Forming Tools. Wear 2011, 271, 2570–2578. [Google Scholar] [CrossRef]
- Pilz, F.; Merklein, M. Comparison of extrusion processes in sheet-bulk metal forming for production of filigree functional elements. CIRP J. Manuf. Sci. Technol. 2019, 26, 41–49. [Google Scholar] [CrossRef]
- Blau, P. Fifty Years of Research on Wear of Metals. Tribol. Int. 1997, 30, 321–331. [Google Scholar] [CrossRef]
- Kapoor, A. Wear by plastic ratchetting. Wear 1997, 212, 119–130. [Google Scholar] [CrossRef]
- Abachi, S.; Akkök, M.; Gökler, M.I. Wear Analysis of Hot Forging Dies. Tribol. Int. 2010, 43, 467–473. [Google Scholar] [CrossRef]
- Holm, R. Electrical Contacts; Hugo Gebers Förlag: Stockholm, Sweden, 1946. [Google Scholar]
- Archard, J.F. Contact and Rubbing of Flat Surfaces. J. Appl. Phys. 1953, 24, 981–988. [Google Scholar] [CrossRef]
- Andersson, S. Wear Simulation. Adv. Knowl. Appl. Pract. 2010, 15–36. [Google Scholar] [CrossRef]
- Cull, S.J.; Tucker, R.W. On the modelling of Coulomb friction. J. Phys. A Math. Gen. 1999, 32, 11. [Google Scholar] [CrossRef]
- Behrens, B.-A. New Developments in the FE Simulation of Closed Die Forging Processes. In Proceedings of the 40th North American Manufacturing Research Institution of the Society of Manufacturing Engineers, Notre Dame, IN, USA, 4–8 June 2012; pp. 1–10. [Google Scholar]
- Behrens, B.-A.; Bouguecha, A.; Vucetic, M.; Chugreev, A.; Rosenbusch, D. Advanced Finite Element Analysis of Die Wear in Sheet-Bulk Metal Forming Processes. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2016; Volume 1769, p. 130010. [Google Scholar]
- Behrens, B.-A.; Wester, H.; Matthias, T.; Hübner, S.; Wälder, J.; Müller, P. Investigation of the Influence of an Oscillation Superposition on the Wear Behaviour in an Industrial-like Process. In Proceedings of the 23rd International Conference on Material Forming (ESAFORM), Cottbus, Germany, 4–6 May 2020. [Google Scholar]
- Schey, J. Metal Deformation Processes Friction and Lubrication; Dekker: New York, NY, USA, 1970. [Google Scholar]
- Bouguecha, A.; Hadifi, T.; Mielke, J.; Behrens, B.-A. Increase of the computational accuracy of hot bulk forming process simulations with improved friction modeling. Materialwissenschaft und Werkstofftechnik 2012, 43, 839–850. [Google Scholar] [CrossRef]
- Shaw, M.C. The Role of Friction in Deformation Processing. Wear 1963, 6, 140–158. [Google Scholar] [CrossRef]
- Henke, T.; Bambach, M.; Hirt, G. Experimental Uncertainties affecting the Accuracy of Stress-Strain Equations by the Example of a Hensel-Spittel Approach. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2011; Volume 1353, pp. 71–76. [Google Scholar]
- Behrens, B.-A.; Wester, H.; Matthias, T.; Hübner, S.; Müller, P.; Wälder, J. Numerical Calculation of Tool Wear in Industrial Cold Forming Processes Using the Further Development of Wear Modelling. In Sheet Bulk Metal Forming. TCRC73 2020. Lecture Notes in Production Engineering; Merklein, M., Tekkaya, A.E., Behrens, B.A., Eds.; Springer: Berlin/Heidelberg, Germany, 2020. [Google Scholar]
- Simufact Engineering GmbH. Datenbank simufact.forming 16.0. Available online: https://www.simufact.de (accessed on 12 March 2021).
- Altan, T.; Vasquez, V. Status of process simulation using 2D and 3D finite element method. What is practical today? What can we expect in the future? J. Mater. Process. Technol. 1997, 71, 49–63. [Google Scholar] [CrossRef]
- Childs, T.H. Material Property Needs in Modelling Metal Machining. Mach. Sci. Technol. 1998, 2, 303–316. [Google Scholar] [CrossRef]
- Pape, F. Mikrotribologische Untersuchungen an Wälzlagern mit Polymeraddivierter Fettschmierung. Ph.D. Thesis, Leibniz University Hannover, Hannover, Germany, 2011. [Google Scholar]
- Buschhausen, A.; Weinmann, K.; Lee, J.Y.; Altan, T. Evaluation of lubrication and friction in cold forging using a double backward-extrusion process. J. Mater. Process. Technol. 1992, 33, 95–108. [Google Scholar] [CrossRef]
- Wißmeier, H.-J. Beitrag zur Beurteilung des Bruchverhaltens von Hartmetall-Fließpreßmatrizen. Ph.D. Thesis, University of Erlangen-Nuremberg, Erlangen, Germany, 1989. [Google Scholar]
- Basquin, O.H. The Exponential Law of Endurance Tests. Am. Soc. Test. Mater. Proc. 1910, 10, 625–630. [Google Scholar]
- Goodman, J. Mechanics Applied to Engineering; Longmans, Green, and Co.: London, UK, 1899. [Google Scholar]



| Coefficients | A | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| Value | 921.908 | −0.000516 | −0.081156 | 0.0182971 | −0.008566 | −0.001384 | 0.722791 | −8.29318 × 10−5 | 0.0580008 |
| T in °C | E in MPa | in 1/K | in W/(mK) | in J/(gK) | |
|---|---|---|---|---|---|
| 20 | 196,000 | 0.276 | - | 14.5 | 0.472 |
| 100 | 190,000 | 0.276 | 1.67 10−5 | 16 | 0.501 |
| 150 | 186,000 | 0.276 | 1.70 10−5 | 17 | 0.515 |
| 200 | 182,000 | 0.276 | 1.72 10−5 | 17.6 | 0.525 |
| 250 | 178,000 | 0.276 | 1.75 10−5 | 18.4 | 0.530 |
| Shoulder-Opening Angle in ° | Shoulder-Runout Radius in mm |
|---|---|
| 10, 13, 16 | 0.2, 0.5, 1, 2, 5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Behrens, B.-A.; Brunotte, K.; Hübner, S.; Wester, H.; Müller, F.; Müller, P.; Wälder, J.; Matthias, T. Further Development of Wear Calculation and Wear Reduction in Cold Forging Processes. J. Manuf. Mater. Process. 2021, 5, 36. https://doi.org/10.3390/jmmp5020036
Behrens B-A, Brunotte K, Hübner S, Wester H, Müller F, Müller P, Wälder J, Matthias T. Further Development of Wear Calculation and Wear Reduction in Cold Forging Processes. Journal of Manufacturing and Materials Processing. 2021; 5(2):36. https://doi.org/10.3390/jmmp5020036
Chicago/Turabian StyleBehrens, Bernd-Arno, Kai Brunotte, Sven Hübner, Hendrik Wester, Felix Müller, Philipp Müller, Jonas Wälder, and Tim Matthias. 2021. "Further Development of Wear Calculation and Wear Reduction in Cold Forging Processes" Journal of Manufacturing and Materials Processing 5, no. 2: 36. https://doi.org/10.3390/jmmp5020036
APA StyleBehrens, B.-A., Brunotte, K., Hübner, S., Wester, H., Müller, F., Müller, P., Wälder, J., & Matthias, T. (2021). Further Development of Wear Calculation and Wear Reduction in Cold Forging Processes. Journal of Manufacturing and Materials Processing, 5(2), 36. https://doi.org/10.3390/jmmp5020036







