Stability and Bifurcation Analysis of Fifth-Order Nonlinear Fractional Difference Equation
Abstract
1. Introduction
1.1. Motivation and Literature Review
1.2. Structure of the Paper
2. Main Results
Dynamics of
- (1)
- necessary condition.
- (2)
- sufficient condition.
- (i)
- (ii)
- (i)
- (ii)
- (iii)
3. Existence of Neimark-Sacker Bifurcation of
Direction of Neimark–Sacker Bifurcation
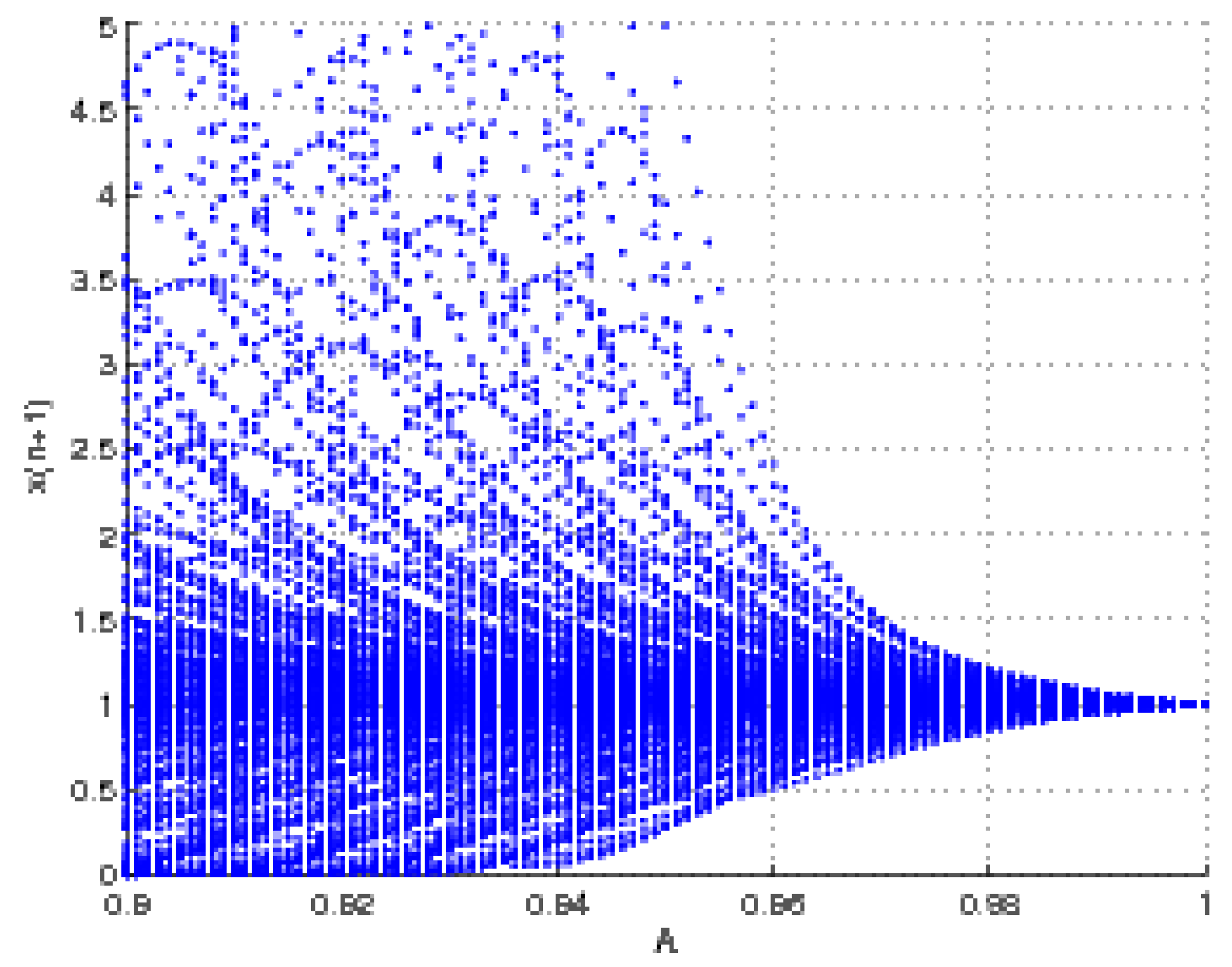
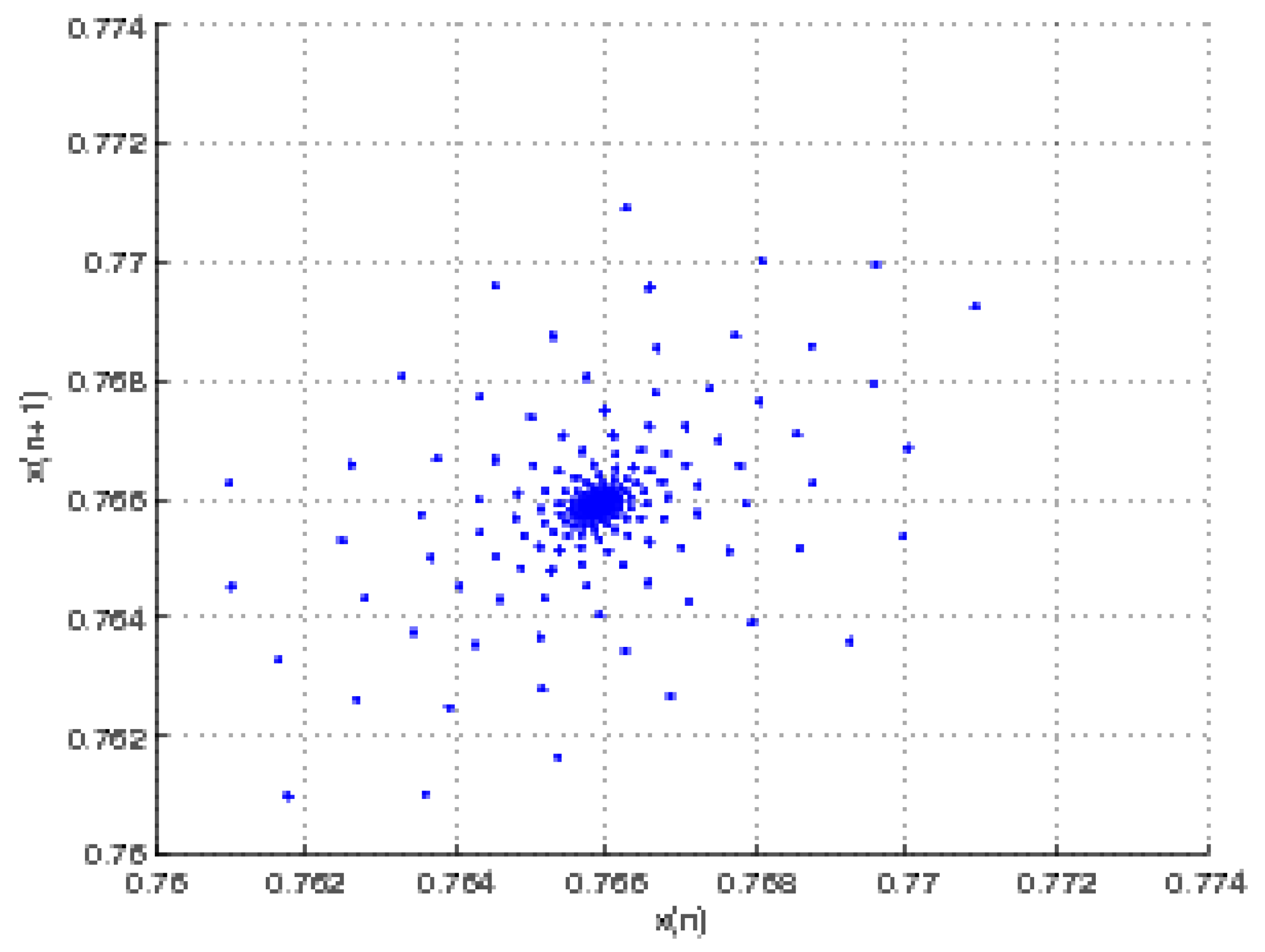
4. Numerical Simulation
5. Conclusions and Findings
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Camouzis, E.; Chatterjee, E.; Ladas, G. On the dynamics of xn+1 = ∂xn−2 + xn−3/A + xn−3. J. Math. Anal. Appl. 2007, 331, 230–239. [Google Scholar] [CrossRef][Green Version]
- Zhang, R.; Ding, X. The Neimark-Sacker bifurcation of xn+1 = ∂xn−2 + xn−3/A + xn−3. J. Differ. Equ. Appl. 2009, 15, 775–784. [Google Scholar]
- Camouzis, E. Global analysis of solutions of xn+1 = ∂xn + xn−2/A + Bxn + Cxn−1. J. Math. Anal. Appl. 2005, 316, 616–627. [Google Scholar]
- He, Z.; Qiu, J. Neimark-Sacker bifurcation of a third order rational difference equation. J. Differ. Equ. Appl. 2013, 19, 1513–1522. [Google Scholar] [CrossRef]
- Camouzis, E.; Ladas, G. Dynamics of Third-order Rational Difference Equations with Open Problems and Conjectures. J. Differ. Equ. Appl. 2013, 19, 1513–1522. [Google Scholar]
- Shareef, A.; Aloqeili, M. Neimark-Sacker bifurcation of a fourth order difference equation. Math. Meth. Appl. Sci. 2018, 19, 1–13. [Google Scholar] [CrossRef]
- Kalabušic, S.; Kulenovic, M.R.S.; Mehuljic, M. Global dynamics and bifurcations of two quadratic fractional second-order difference equations. Comput. Anal. Appl. 2016, 21, 132–143. [Google Scholar]
- Hrustic, S.J.; Kulenovic, M.R.S.; Nurkanovic, M. Global dynamics and bifurcations of certain second order rational difference equation with quadratic terms. Qual. Theory Dyn. 2016, 15, 283–307. [Google Scholar]
- Kulenovic, M.R.S.; Moranjkic, S.; Nurkanovic, Z. Naimark-Sacker bifurcation of second order rational difference equation with quadratic terms. J. Nonlinear Sci. Appl. 2017, 10, 3377–3489. [Google Scholar] [CrossRef]
- Valderde, J.C. Simplest normal forms of Hopf Neimark-Sacker Bifurcations. Int. J. Bifurc. Chaos 2003, 13, 1831–1839. [Google Scholar] [CrossRef]
- Cushing, J.M. Difference equations as models of evolutionary population dynamics. J. Bio Dyn. 2019, 13, 103–127. [Google Scholar] [CrossRef]
- Connor, M.K.; Pilav, E. The Neimark-Sacker bifurcation and global stability of perturbation of sigmoid beverton-holt difference equation. Dis. Dyn. Nat. Soc. 2021, 14, 2092709. [Google Scholar]
- Kalabuši, S.; Kulenovi, M.P.S.; Mehulji, M. Global Period-Doubling Bifurcation of Quadratic Fractional Second Order Difference Equation. Dis. Dyn. Nat. Soc. 2014, 13, 10–53. [Google Scholar] [CrossRef]
- Khaliq, A.; Alayachi, H.S.; Zubair, M.; Rohail, M.; Khan, A.Q. On stability analysis of a class of three-dimensional system of exponential difference equations. AIMS Math. 2023, 8, 5016–5035. [Google Scholar] [CrossRef]
- Kuznetsov, Y.A.; Kuznetsov, I.A.; Kuznetsov, Y. Elements of Applied Bifurcation Theory, 3rd ed.; Springer Applied Mathematical Science: New York, NY, USA, 2013; p. 632. [Google Scholar]
- Kocic, V.L.; Ladas, G. Global behaviour on non-linear difference equations of higher order with applications. Klu. Aca. Pub. 1993, 13, 10–53. [Google Scholar]
- Khyat, T.; Kulenovic, M.; Pilav, E. The Naimark-Sacker bifurcation and asymptotic approximation of the invariant curve of a certain difference equation. J. Comp. Anal. Appl. 2017, 19, 15–22. [Google Scholar]
- Hou, C.; Han, L.; Cheng, S. Complete Asymptotic and Bifurcation Analysis for a Difference Equation with Piece-wise Constant Control. Adv. Differ. Equ. 2010, 13, 542073. [Google Scholar]
- Gari-Demirovi, M.; Moranjki, S. Neimark–Sacker Bifurcation and Approximation of the Invariant Curve of Certain Homogeneous Second-Order Fractional Difference Equation. Dis. Dyn. Nat. Soc. 2020, 12, 625–4013. [Google Scholar] [CrossRef]
- Ibrahim, T.F. Bifurcation and periodically semi-cycles for fractional difference equation of fifth order. J. Nonlinear Sci. Appl. 2018, 11, 375–382. [Google Scholar] [CrossRef]
- Stubna, M.D.; Gilmour, R.F. Analysis of a Non-linear Partial Difference Equation and Its Application to Cardiac Dynamics. J. Differ. Equ. Appl. 2010, 12, 1147–1169. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khaliq, A.; Mustafa, I.; Ibrahim, T.F.; Osman, W.M.; Al-Sinan, B.R.; Dawood, A.A.; Juma, M.Y. Stability and Bifurcation Analysis of Fifth-Order Nonlinear Fractional Difference Equation. Fractal Fract. 2023, 7, 113. https://doi.org/10.3390/fractalfract7020113
Khaliq A, Mustafa I, Ibrahim TF, Osman WM, Al-Sinan BR, Dawood AA, Juma MY. Stability and Bifurcation Analysis of Fifth-Order Nonlinear Fractional Difference Equation. Fractal and Fractional. 2023; 7(2):113. https://doi.org/10.3390/fractalfract7020113
Chicago/Turabian StyleKhaliq, Abdul, Irfan Mustafa, Tarek F. Ibrahim, Waleed M. Osman, Bushra R. Al-Sinan, Arafa Abdalrhim Dawood, and Manal Yagoub Juma. 2023. "Stability and Bifurcation Analysis of Fifth-Order Nonlinear Fractional Difference Equation" Fractal and Fractional 7, no. 2: 113. https://doi.org/10.3390/fractalfract7020113
APA StyleKhaliq, A., Mustafa, I., Ibrahim, T. F., Osman, W. M., Al-Sinan, B. R., Dawood, A. A., & Juma, M. Y. (2023). Stability and Bifurcation Analysis of Fifth-Order Nonlinear Fractional Difference Equation. Fractal and Fractional, 7(2), 113. https://doi.org/10.3390/fractalfract7020113







